Quantitative Analysis of the Observed Environmental Changes of Geomagnetic Stations in Mainland China from 1997 to 2016
-
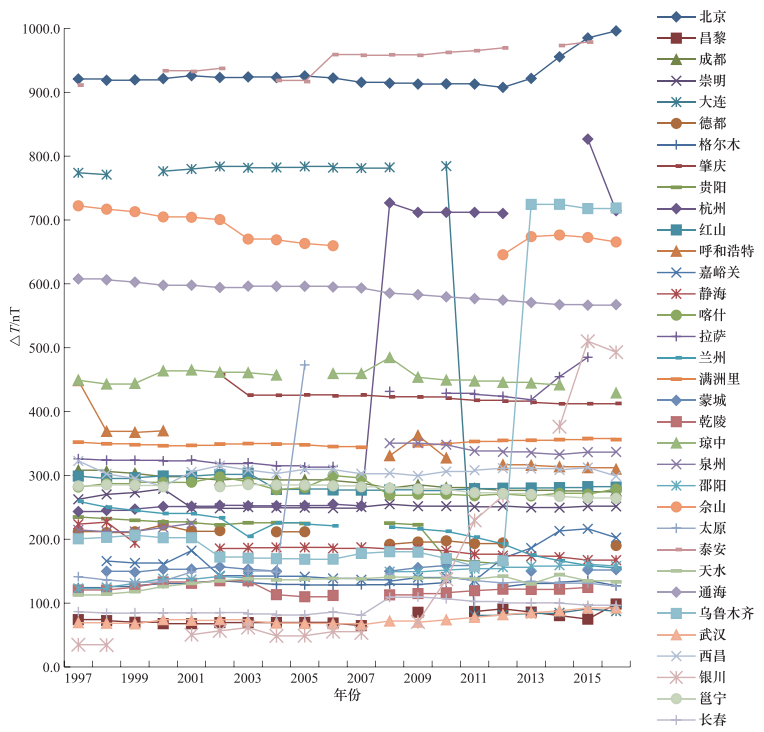
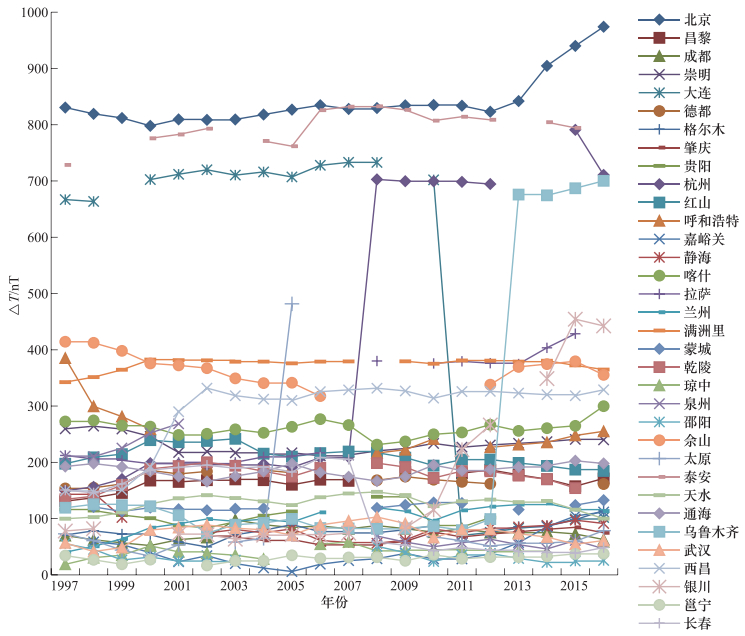
摘要: 本文利用CHAOS-6地磁场模型计算1997—2016年中国大陆地区34个地磁台站模型值，分析比较模型计算值与台站实测值之间的差异，利用国际参考场模型IGRF12计算结果进行佐证。结果表明：地磁异常场模量△T作为地磁台站观测环境的一种标志，年变化显示：①中国大陆地区34个地磁基准台绝大部分位于地磁正常区或弱磁异常区，只有少数几个地磁台位于强磁异常区；②判断1个地磁台的地磁场环境，只测量总强度（F）是不够的，必须进行三分量绝对观测，利用地磁异常场模量△T评价地磁台的地磁场环境更全面、更科学合理。Abstract: Using CHAOS-6 geomagnetic field model, we calculated the model values of 34 geomagnetic stations between 1997-2016 in mainland China. By analyzing and comparing the model calculation value and the observation value, and the difference between using IGRF12 calculation results, we found that the geomagnetic anomalous field modulus(△T) is a good indicator of the geomagnetic station observation environment. Our results show that:①for the most part the geomagnetic normal area or weak magnetic anomaly area of 34 geomagnetic stations in mainland China, only a handful of geomagnetic station located in the strong magnetic anomaly zone, ②to judge the geomagnetic field environment of a geomagnetic platform, it is not enough to make the measurement of total strength (F) only, but to make the absolute observation of three components. Instead, it will be more comprehensive, more scientific and reasonable by using the modulus of geomagnetic anomalies (△T) in evaluating the geomagnetic field environment of a geomagnetic platform in future.
-
Key words:
- Geomagnetic field /
- CHAOS-6 model /
- Geomagnetic station /
- Modulus of geomagnetic anomaly /
- IGRF12 model
-
图 1 中国大陆地区34个地磁台站分布
Figure 1. Location map of 34 geomagnetic observatory stations in mainland china
图 3 1997—2016年中国大陆地区地磁台站地磁环境变化空间分布（IGRF12模型）
Figure 3. Spatial geomagnetic environment changes of the geomagnetic stations in mainland China between 1997 and 2016
表 1 地理坐标与地心坐标计算结果比较
Table 1. Comparison of calculation results between geographic coordinates and geocentric coordinates
测项 地理坐标下的模型值/nT 地心坐标下的模型值/nT 台站观测值/nT dif地理/nT dif地心/nT X红山 29305.2 29503.5 29547.2 -242.0 -43.7 Y红山 -2993.2 -2946.6 -3072.9 79.7 126.3 Z红山 44739.8 44523.6 44321.7 418.1 201.9 X昌黎 27768.7 28041.0 28045.4 -276.7 -4.4 Y昌黎 -3681.9 -3670.7 -3725.0 43.1 54.3 Z昌黎 46217.1 45889.4 45808.7 408.4 80.7 
下载: 导出CSV
表 2 地磁台的地磁异常场（CHAOS-6模型）
Table 2. The geomagnetic anomaly field of the observatories
台站名称 △D/′ △I/° △H/nT △X/nT △Y/nT △Z/nT △F/nT △T/nT 北京 -18.6 -0.3 689.1 667.8 -231.5 592.0 865.4 928.0 昌黎 -6.6 0.0 -21.4 -27.9 -52.0 -45.3 -49.8 76.0 成都 3.9 0.3 -50.5 -49.4 40.0 280.80 172.10 288.6 崇明 14.8 0.1 72.0 84.7 136.7 197.3 193.3 254.7 大连 8.0 -0.5 323.6 329.4 25.8 -418.20 -177.10 548.4 德都 -13.1 -0.2 132.6 112.9 -112.3 -129.5 -64.0 204.9 格尔木 -7.6 -0.1 98.6 98.7 -66.9 50.1 97.4 129.7 肇庆 1.6 0.5 -50.4 -49.7 19.5 419.1 189.1 422.6 贵阳 -8.4 0.2 22.0 19.3 -90.0 184.8 135.3 213.8 杭州 -31.3 -0.3 96.3 66.3 -315.6 -233.4 -94.2 437.3 红山 -16.9 -0.2 51.4 37.7 -151.5 -239.2 -168.7 286.0 呼和浩特 -0.9 0.2 20.3 19.7 -9.2 343.8 308.1 345.2 嘉峪关 1.2 0.1 27.5 27.6 9.4 155.4 148.1 160.8 静海 -4.2 0.2 -76.2 -79.5 -27.4 165.8 98.3 186.0 喀什 7.1 0.1 30.9 27.2 58.1 272.8 249.7 280.8 台站名称 △D/′ △I/° △H/nT △X/nT △Y/nT △Z/nT △F/nT △T/nT 拉萨 -13.3 0.0 202.5 201.9 -134.3 235.8 309.7 368.8 兰州 -6.7 0.2 -62.6 -64.6 -57.9 193.8 121.4 212.8 满洲里 0.1 -0.1 -64.0 -63.1 10.7 -344.4 -343.7 350.4 蒙城 13.7 -0.1 65.7 54.4 -134.2 -49.1 4.3 153.1 乾陵 2.2 -0.1 51.5 52.6 17.6 -105.2 -51.9 121.6 琼中 -6.8 0.6 -65.0 -66.7 -76.5 441.6 129.6 453.3 泉州 -3.0 0.3 -29.5 -31.5 -29.5 271.2 138.8 296.2 邵阳 -1.4 -0.2 81.3 80.6 -18.6 -119.5 -17.6 145.7 佘山 -0.2 0.8 -323.1 -322.1 26.5 602.3 212.1 683.9 太原 -3.1 0.0 82.9 80.2 -33.2 93.1 123.4 158.9 泰安 23.9 -1.0 724.2 741.4 145.7 -573.6 -32.7 948.9 天水 1.8 -0.1 80.4 81.1 13.2 -103.1 -32.8 133.3 通海 -2.2 0.7 -99.1 -99.5 -22.0 578.8 254.6 587.8 乌鲁木齐 1.1 0.2 -124.5 -124.7 0.7 251.4 172.4 289.1 武汉 -0.1 -0.1 57.8 57.6 -5.2 -42.3 9.5 75.0 西昌 -19.9 0.0 146.0 140.9 -212.2 142.2 203.2 305.7 银川 -2.9 -0.1 79.2 77.8 -28.7 -127.1 -65.6 158.1 邕宁 -3.2 0.3 -31.0 -32.0 -34.3 274.1 123.7 278.2 长春 -7.7 0.0 6.2 -3.5 -58.4 3.5 6.2 92.2
下载: 导出CSV
表 3 地磁台的地磁异常场（IGRF12模型）
Table 3. The geomagnetic anomaly field of the observatories
台站名称 △D/′ △I/° △H/nT △X/nT △Y/nT △Z/nT △F/nT △T/nT 北京 -16.2 -0.4 724.2 704.8 -215.1 393.5 714.4 840.5 昌黎 -7.3 0.0 -65.7 -72.7 -52.1 -137.8 -151.7 165.3 成都 6.6 0.0 6.4 8.1 65.4 -30.0 -17.7 75.0 崇明 17.6 0.1 43.5 58.8 165.7 148.9 138.6 231.3 大连 10.5 -0.4 236.5 245.8 57.9 -373.3 -186.2 500.6 德都 -17.7 -0.1 17.6 -6.0 -120.9 -119.2 -101.5 170.5 格尔木 -4.3 0.1 -29.1 -29.1 -38.3 61.2 33.8 78.8 肇庆 4.8 0.0 -2.5 -0.6 53.1 38.2 19.2 67.5 贵阳 -6.2 0.0 10.5 8.5 -66.0 54.4 42.7 105.4 杭州 -32.1 -0.2 -2.9 -33.2 -315.4 -179.1 -125.3 392.5 红山 -13.9 0.0 -69.4 -80.2 -114.8 -157.1 -168.7 211.6 呼和浩特 4.2 0.1 -3.2 -0.3 34.1 255.3 219.6 258.5 嘉峪关 1.0 0.0 19.7 19.7 8.1 15.8 24.1 42.8 静海 1.8 0.1 -66.9 -64.6 22.0 48.7 5.0 85.6 喀什 8.7 0.2 -72.6 -76.9 64.4 239.9 168.1 260.6 拉萨 -13.6 0.0 159.1 158.5 -137.3 133.9 206.9 280.8 兰州 -1.9 -0.1 4.6 3.9 -17.1 -95.6 -75.2 98.7 满洲里 0.2 0.0 -163.7 -161.5 26.5 -336.0 -372.7 374.2 蒙城 -12.2 0.0 -24.2 -34.0 -113.2 -1.3 -16.4 120.8 乾陵 2.9 -0.1 -5.1 -3.7 27.1 -173.9 -140.9 177.2 琼中 -2.9 0.0 -7.6 -8.4 -33.2 -9.8 -11.8 41.7 泉州 1.3 -0.1 25.8 26.5 12.5 -70.1 -20.6 119.5 邵阳 -0.9 0.0 -15.2 -15.7 -8.7 -5.1 -14.9 32.7 佘山 4.0 0.4 -257.3 -253.0 61.5 256.8 8.8 367.1 太原 0.3 0.0 43.4 43.1 -0.9 19.2 39.9 85.9 泰安 22.2 -0.8 589.2 605.7 144.0 -497.5 -50.2 797.3 天水 6.2 -0.1 -2.5 -0.3 57.2 -109.5 -88.2 127.2 台站名称 △D/′ △I/° △H/nT △X/nT △Y/nT △Z/nT △F/nT △T/nT 通海 -1.2 0.2 -31.4 -31.6 -12.2 182.0 80.5 185.8 乌鲁木齐 1.6 0.2 -146.2 -146.7 3.8 151.3 72.9 214.2 武汉 1.7 -0.1 4.6 5.7 16.6 -63.4 -42.7 74.8 西昌 -20.2 -0.2 161.6 156.5 -215.6 -88.1 60.1 288.6 银川 -1.7 -0.1 -17.3 -18.2 -13.7 -139.1 -127.3 161.8 邕宁 -1.5 0.0 -9.5 -9.7 -16.4 9.3 -2.6 29.5 长春 -2.8 -0.1 31.8 27.7 -25.6 -109.3 -81.0 121.2
下载: 导出CSV
表 4 部分地磁台CHAOS-6和IGRF12模型的精度统计
Table 4. Accuracy from statistics of partial observatories by CHAO-6 and IGRF12 models
台站名称 △D/′ △I/° △H/nT △X/nT △Y/nT △Z/nT △F/nT △T/nT CHAOS-6 IGRF12 CHAOS-6 IGRF12 CHAOS-6 IGRF12 CHAOS-6 IGRF12 CHAOS-6 IGRF12 HAOS-6 IGRF12 CHAOS-6 IGRF12 CHAOS-6 IGRF12 琼中 -6.8 -2.9 0.6 0.0 -65.0 -7.6 -66.7 -8.4 -76.5 -33.2 441.6 -9.8 129.6 -11.8 453.3 41.7 通海 -2.2 -1.2 0.7 0.2 -99.1 -31.4 -99.5 -31.6 -22.0 -12.2 578.8 182.0 254.6 80.5 587.8 185.8 肇庆 1.6 4.8 0.5 0.0 -50.4 -2.5 -49.7 -0.6 19.5 53.1 419.1 38.2 189.1 19.2 355.0 67.5 邕宁 -3.2 -1.5 0.3 0.0 -31.0 -9.5 -32.0 -9.7 -34.3 -16.4 274.1 9.3 123.7 -2.6 278.2 29.5 成都 3.9 6.6 0.3 0.0 -50.5 6.4 -49.4 8.1 40.0 65.4 280.8 -30.0 172.1 -17.7 288.6 75.0 邵阳 -1.4 -0.9 -0.2 -0.0 81.3 -15.2 80.6 -15.7 -18.6 -8.7 -119.5 -5.1 -17.6 -14.9 113.0 32.7
下载: 导出CSV
-
安柏林, 康国发. 2017.基于CHAOS-5模型研究中国大陆地区地磁场长期变化.云南大学学报(自然科学版), 39(5):789-797.陈斌. 2011.自然正交分量方法在地震地磁监测中的应用.地震研究, 34(4):466-469. http://d.old.wanfangdata.com.cn/Periodical/yndxxb201705010 陈斌, 顾左文, 高金田等. 2012.IGR-11描述的2005-2010年中国地区地磁长期变化及其误差分析.地球物理学进展, 27(2):512-521. doi: 10.6038/j.issn.1004-2903.2012.02.014 丁鉴海, 索玉成, 余素荣. 2005.地磁场与电离层异常现象及其与地震的关系.空间科学学报, 25(6):536-542. doi: 10.3969/j.issn.0254-6124.2005.06.007 傅承义, 陈运泰, 祁贵仲. 1985.地球物理学基础.北京:科学出版社, 108-112. 顾春雷, 张毅, 徐如刚等. 2010.地震前后岩石圈磁场变化特征分析.地球物理学进展, 25(2):472-477. doi: 10.3969/j.issn.1004-2903.2010.02.013 顾左文, 张毅, 姚同起等. 2006.九江-瑞昌Ms5.7地震地磁异常的观测与分析.地震学报, 28(6):611-621. http://d.old.wanfangdata.com.cn/Periodical/dqwlxb200801020 李细顺, 高登平, 李琪等. 2015.CM4模型数据与台站实测数据的对比研究.震灾防御技术, 10(2):418-425. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20150223&journal_id=zzfyjs 李细顺, 高登平, 刘立申等. 2018.基于CM4模型的中国大陆地区地磁场时空分布特征分析.震灾防御技术, 13(1):98-113. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20180109&journal_id=zzfyjs 李琪, 杨星, 蔡绍平. 2015.极化方法应用于地磁台阵的震例分析.震灾防御技术, 10(2):412-417. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20150222&journal_id=zzfyjs 王振东, 顾左文, 陈斌等. 2017.CHAOS-6模型描述的中国地区地磁长期变化及误差分析.地震研究, 40(3):404-410. doi: 10.3969/j.issn.1000-0666.2017.03.015 徐文耀. 2002.地球主磁场的NOC模型.中国科学(D辑), 32(7):576-587. http://d.old.wanfangdata.com.cn/Periodical/zgkx-cd200207006 徐文耀. 2009.地球电磁现象物理学.合肥:中国科学技术大学出版社, 18-29. 杨云存, 高国明. 2014.1900-2010年地磁场水平分量梯度的全球变化.震灾防御技术, 9(S1):557-571. doi: 10.11899/zzfy2014s101 袁洁浩, 顾左文, 陈斌等. 2013.地震与火山电磁研究的国际学术交流与合作.地震研究, 36(1):132-140. doi: 10.3969/j.issn.1000-0666.2013.01.020 袁洁浩, 顾左文, 王粲等. 2016.2015年IUGG大会与世界华人地质大会的震磁研究进展.地震研究, 39(4):703-708. doi: 10.3969/j.issn.1000-0666.2016.04.022 Alldredge L. R.. 1987. On regional geomagnetic charts. Journal of Geomagnetism and Geoelectricity, 39(12):723-738. doi: 10.5636/jgg.39.723 Campbell W. H.. 2003. Introduction to geomagnetic fields. 2nd ed. New York:Cambridge University Press, 1-34. Finlay C. C., Olsen N., Tøffner-Clausen L.. 2015. DTU candidate field models for IGRF-12 and the CHAOS-5 geomagnetic field model. Earth, Planets and Space, 67:114. doi: 10.1186/s40623-015-0274-3 Finlay C. C., Olsen N., Kotsiaros S., et al.. 2016. Recent geomagnetic secular variation from Swarm and ground observatories as estimated in the CHAOS-6 geomagnetic field model. Earth, Planets and Space, 68:112. doi: 10.1186/s40623-016-0486-1 Haines G. V.. 1985. Spherical cap harmonic analysis. Journal of Geophysical Research:Solid Earth, 90(B3):2583-2591. doi: 10.1029/JB090iB03p02583 Hemant K., Maus S.. 2005. Geological modeling of the new CHAMP magnetic anomaly maps using a geographical information system technique. Journal of Geophysical Research:Solid Earth, 110(B12):B12103. doi: 10.1029/2005JB003837 Hemant K., Thébault E., Mandea M., et al.. 2007. Magnetic anomaly map of the world:merging satellite, airborne, marine and ground-based magnetic data sets. Earth and Planetary Science Letters, 260(1-2):56-71. doi: 10.1016/j.epsl.2007.05.040 Lesur V., Wardinski I., Rother M., et al.. 2008. GRIMM:the GFZ reference internal magnetic model based on vector satellite and observatory data. Geophysical Journal International, 173(2):382-394. doi: 10.1111/j.1365-246X.2008.03724.x Olsen N., Mandea M.. 2008. Rapidly changing flows in the Earth's core. Nature Geoscience, 1(6):390-394. doi: 10.1038/ngeo203 Olsen N., Mandea M., Sabaka T. J., et al.. 2009. CHAOS-2-a geomagnetic field model derived from one decade of continuous satellite data. Geophysical Journal International, 179(3):1477-1487. doi: 10.1111/j.1365-246X.2009.04386.x Olsen N., Mandea M., Sabaka T. J., et al.. 2010. The CHAOS-3 geomagnetic field model and candidates for the 11th generation IGRF. Earth, Planets and Space, 62:719-727. doi: 10.5047/eps.2010.07.003 Olsen N., Lühr H., Sabaka T. J., et al.. 2006. CHAOS-a model of the Earth's magnetic field derived from CHAMP, Ørsted, and SAC-C magnetic satellite data. Geophysical Journal International, 166(1):67-75. doi: 10.1111/j.1365-246X.2006.02959.x Olsen N., Lühr H., Finlay C. C., et al.. 2014. The CHAOS-4 geomagnetic field model. Geophysical Journal International, 197(2):815-827. doi: 10.1093/gji/ggu033 Thébault E., Finlay C. C., Beggan C. D., et al.. 2015. International geomagnetic reference field:the 12th generation. Earth, Planets and Space, 67:79 doi: 10.1186/s40623-015-0228-9 Wardinski I., Holme R.. 2006. A time-dependent model of the earth's magnetic field and its secular variation for the period 1980-2000. Journal of Geophysical Research:Solid Earth, 111(B12):B12101. http://cn.bing.com/academic/profile?id=14c7fcfc2d6313d7a31e469cc837b8e9&encoded=0&v=paper_preview&mkt=zh-cn -
点击查看大图
图(3) / 表(4)
计量
- 文章访问数: 132
- HTML全文浏览量: 14
- PDF下载量: 1
- 被引次数: 0
