Seismic Fragility Analysis of Self-centering Precast Segmental Concrete-filled Double Skin Steel Tubular Piers
-
摘要: 为评估自复位预制节段拼装中空夹层钢管混凝土（Concrete-filled Double Skin Steel Tubular, CFDST）桥墩在地震动作用下的易损性，本研究基于现有的低周反复荷载试验数据，采用有限元分析方法，选用墩顶水平位移角和残余位移角2个指标作为评估标准进行定量分析。针对3种不同类型地震动（远场、近场无脉冲和近场有脉冲），分别建立了关于水平位移角和残余位移角2个指标的易损性曲线，并分析了不同损伤指标和地震动类型对其地震易损性的影响。研究结果表明，在自复位预制节段拼装CFDST桥墩地震易损性分析中，仅采用水平位移角作为损伤指标是安全可靠的；相比远场地震动和近场无脉冲型地震动而言，近场脉冲型地震动对自复位预制节段拼装CFDST桥墩的变形和自复位有显著影响。Abstract: This paper evaluates the seismic vulnerability of self-centering precast segmental concrete-filled double skin steel tubular (CFDST) piers by quantitatively assessing their seismic performance under three types of ground motions: far-field, near-field without pulse, and near-field pulse-like motions, based on existing quasi-static tests. Using finite element analysis, vulnerability curves for the self-centering precast segmental CFDST piers are established based on two damage indices: the horizontal displacement angle and the residual displacement angle. The study analyzes the effects of different damage indices and ground motion types on the vulnerability curves of the self-centering precast segmental CFDST piers. The results indicate that utilizing only the horizontal displacement angle as the damage index is both safe and reliable for the seismic vulnerability analysis of these piers. Additionally, it was found that near-field pulse-like ground motions significantly affect the deformation and self-centering behavior of the self-centering precast segmental CFDST piers compared to far-field and near-field non-pulse-like ground motions.
-
引言
据河北省地震台网测定，2018年2月12日18时31分在河北省廊坊市永清县(39.37°N，116.67°E)发生4.3级地震，震源深度20km。此次地震未造成人员伤亡，在震中区域造成个别房屋墙皮脱落，河北省廊坊市、固安市震感较强，北京市、天津市普遍有感。地震发生后，中国地震局工程力学研究所对永清4.3级地震强震动记录进行了远程和现场记录回收。强震动记录是地震能量在地表或建筑结构上的真实反映，是工程地震、地震学研究的基础数据资料(朱升初等，2018)，本文对此次地震构造背景及强震动记录进行了分析，对永清4.3级地震烈度采用建立虚拟台站方法进行了校正，为河北地区烈度调查、地震区划等提供基础资料。
1. 发震构造背景
永清4.3级地震区域构造主要以NE、NW向断裂为主，地震发生在廊固凹陷内(图 1)，廊固凹陷是冀中坳陷北部的一个古近系湖盆，整个凹陷大体呈NE走向，南北长约90km，东西宽20—40km，面积约2600km2。该凹陷是受区域伸展并伴有走滑作用形成的典型箕状凹陷，整个凹陷表现为北断南超、西断东超的构造格局。由于受NE向断裂构造控制，表现出整体不均匀下降的箕状断陷特征。该凹陷内曾于1621年发生廊坊永清$ 5\frac{1}{2} $级地震(国家地震局震害防御司，1995)。
图 1 永清4.3级地震构造背景Figure 1. The seismotectonic background map of the Yongqing MS4.3 earthquake(1)施庄断裂(2)南口山前断裂(3)南口-孙河断裂(4)小汤山-东北旺断裂(5)黄庄-高丽营断裂 (6)顺义-前门-良乡断裂(7)南苑-通县断裂(8)新夏垫断裂(9)夏垫断裂(10)大兴凸起东缘断裂 (11)蓟县山前断裂(12)宝坻断裂(13)桐柏断裂(14)河西务断裂(15)天津断裂(16)大城东断裂 (17)沧东断裂(18)海河断裂(19)牛驼镇凸起东缘断裂(20)徐水断裂(21)东垒子-涞水断裂 (22)永定河断裂(23)徐水南断裂本次地震发生在廊固凹陷东侧控制断裂河西务断裂带附近，该断裂北起河西务西北，向西南经大王务，延伸至永清东码头镇，全长近50km，走向NE，倾向SE，为上陡下缓的铲形正断层，断层产状与震源机制参数基本一致(王晓山等，2018)，所以永清4.3级地震发震构造可能为河西务断裂。周月玲等(2018)通过浅层地震勘探和钻孔联合剖面探测，结合年代样品测试，对河西务断裂活动性进行了综合研究，揭示断裂上断点埋深约150m或以浅，第四系底界面垂直断错为20—45m，断裂最新活动时代为晚更新世早期。
2. 观测台站及记录获取
2.1 强震动台站分布
本次地震强震动数据主要由中国地震局工程力学研究所提供，共获取3个分量自由场记录74组，常规处理后得到222条3分向加速度记录，获取记录的强震动台站均为土层台，记录台站分布如图 2所示，由图 2可知，触发台站主要分布在震中北部，南部无台站触发，这可能是因为首都圈台站较密集，而河北省境内强震动台站稀疏，或与仪器型号不同且很多台站老旧未改造有关。触发台站的震中距为29.4—185.5km，其中震中距＜50km的记录有5组，震中距为50—100km的有29组，震中距为100—150km的有37组，震中距为150—200km的有3组，距震中最近的台站为长子营台(11ZZY)，震中距为29.4km。虽然此次地震获取的强震动记录较全面，但由于震级较小，未造成大的灾害损失，且震中附近台站相对稀疏，能供工程使用的强震动记录较少。
图 2 永清4.3级地震触发台站分布图Figure 2. The distribution of seismic trigger station of Yongqing MS4.3 earthquake2.2 强震动记录获取
在强震动记录使用分析前，首先对波形数据进行消除基线漂移，即原始强震动记录通过减去事前记录平均值的方法进行零线调整(吕俊强等，2015)，通过分析计算得到震中距R＜50km的5个台站强震动记录基本信息及相关参数，见表 1。其中距震中最近的长子营台记录到的最大峰值加速度为7.9cm/s2，觅子店台记录到的东西向最大峰值加速度为36.1cm/s2、南北向最大峰值加速度为40.4cm/s2，红星中学台记录到的垂直方向最大峰值加速度为10.8cm/s2，5个台站加速度时程曲线如图 3。由表 1可知，离震中较近的长子营台(震中距29.4km)较离震中较远的觅子店台(震中距38.4km)和于家务台(震中距36.8km)各分量加速度小，影响地震动强度的因素除震中距外，还包括仪器型号、场地特征条件和地质构造、场地土层结构、覆盖层厚度、地形及断裂分布等(王海云等，2010；温瑞智等，2013；郭秋娜等，2018)。本文根据温瑞智等(2015)提出的适用于我国强震动台站场地划分经验曲线，采用H/V谱比法对本次地震触发台站进行分类，分类结果见图 4。由图 4可知，记录到的本次地震台站大部分为Ⅰ类场地(51.35%)，其次为Ⅱ类场地(35.14%)和Ⅲ类场地(13.51%)，其中，觅子店台和红星中学台为Ⅰ类场地，于家务台和张家湾中学台为Ⅱ类场地，长子营台为Ⅲ类场地。长子营台与觅子店台和于家务台记录差异较大，这可能与场地类型有关。
表 1 强震动记录(震中距＜50km)及相关参数Table 1. Strong motion records(epicenter distance < 50km) and related parameters台站名称 台站代码 仪器型号 震中距/km PGA/cm·s-2 EW SN UD 觅子店 11MZD REFTEK/SLJ-100 38.4 36.1 40.4 8.5 红星中学 11YHZ REFTEK/SLJ-100 43.4 -5.4 -4.6 10.8 于家务 11YJW ETNA/ES-T 36.8 -21.7 -18.5 -8.1 张家湾中学 11ZJW REFTEK/SLJ-100 47.9 -5.8 4.2 -4.4 长子营 11ZZY REFTEK/SLJ-100 29.4 5.0 -7.9 -3.5 3. 谱分析
加速度反应谱是目前国内外工程抗震设计的重要依据，反应谱形状、幅值及卓越周期的差异体现了场地条件、震级对地面运动反应谱的影响(温瑞智等，2013；徐钦等，2017)。为避免地震时建筑结构发生共振，确保结构安全，有必要对加速度反应谱成分进行分析。本文选取震中距＜50km的5个典型强震动台站进行频谱分析，阻尼比取0.05，得到本次地震EW、SN、UD向加速度反应谱(图 5)。
由图 5可知，觅子店台记录到了本次地震EW向和SN向加速度反应谱最大值，红星中学台记录到了本次地震SN向加速度反应谱最大值。觅子店台与长子营台在EW和SN向差别最大，觅子店台与张家湾中学台在SN向差别最大。5个典型台站记录到的加速度反应谱高频成分丰富，谱卓越周期主要为0.1—0.3s，0.3s后迅速下降。根据现场调查，震区主要房屋类型为土木结构、木房顶砌体结构、预制板砌体结构、钢结构简易房屋4种，本次地震除少数土木结构房屋原有裂缝扩大、墙皮开裂外，其他类型房屋未发现新开裂、屋顶掉瓦、墙皮掉灰现象，中小城市主要建筑物结构自振周期为0.3—1s(王文才等，2018)，因此本次地震对该震区自振周期范围内的建筑物破坏影响较小。通过对比不同震中距台站各方向反应谱特征周期发现，震中距对特征周期的影响不明显。
傅氏谱能反映地震波在频域上的幅值特性，地震动记录中包含多种振动频率，不同地区的主要振动频率不同，结构自身具有不同频率、幅频响应，因而在不同频率振动作用下，结构振动不同。为避免地震作用下结构发生共振现象，确保结构安全，有必要对地震频率成分进行分析(冉志杰等，2012)。
本文计算了永清4.3级地震震中距50km以内的5个典型台站强震动记录傅氏谱(图 6)，由图 6可知典型台站傅氏谱谱型主要为单峰值型，强震动记录EW分量傅氏谱最大峰值为3.5—8.5Hz，SN分量傅氏谱最大峰值为2.9—6.2Hz，UD分量傅氏谱最大峰值为2.1—9.8Hz。总体来看，UD分量频率范围分布较宽，EW分量傅氏谱最大峰值大于SN分量和UD分量傅氏谱峰值。一般而言，随着震中距的增大，地震动记录长周期(低频)越显著，但本次地震5个典型台站特征不明显，可能与仪器型号及场地特征条件有关。另外，EW分量傅氏谱最大峰值随着震中距的增大呈先减小后增大的趋势，而SN分量和UD分量无该特征。
本文以东西向为正方向，统计峰值加速度反应谱与永清4.3级地震触发台站同震中所处方位角和震中距的关系(图 7)。由图 7可知，峰值加速度反应谱在同一震中距下整体随着方位角的增大而增大，达70°左右后下降，这可能与附近河西务断裂构造走向有关；峰值加速度反应谱值随着震中距的增加而减小。
图 7 峰值加速度反应谱与震中距、方位角关系图Figure 7. The relation among the peak ground acceleration and epicentral distance、azimuth angles4. 地震烈度分析
地震烈度是衡量地震破坏和地震动强弱程度的重要指标。Wald等(1999)认为当烈度较小时，用峰值加速度计算烈度较合适，因为人对加速度较敏感。随着强震动观测技术的发展，由于以各观测点峰值加速度作为依据可真实反映地震发生时各点地面运动强度(田秀丰等，2013)，基于地震动记录快速确定地震仪器烈度，越来越受到研究者的重视(崔建文等，2016)。目前国内外基于强震动记录资料的地震烈度计算方法较多，不同方法利用不同的地震动参数，包括时间、频度和强度等，建立地震烈度与地震动参数之间的关系。本文采用美国ShakeMap烈度计算方法(李俊等，2010)，计算永清4.3级地震事件记录台站烈度，并采用克里格插值方法进行网格化插值，绘制等值线图(图 8)。Wald等(1999)的研究认为，当烈度低于Ⅴ度时峰值加速度PGA与烈度相关性明显高于峰值速度PGV，而当烈度为Ⅴ—Ⅶ度时，PGA和PGV与烈度相关程度相当，考虑本次地震烈度较低，故采用峰值加速度计算烈度。由图 9可知，本次地震仪器烈度等震线长轴沿NW走向，且极震区位于震中东北部，而不是震中附近，但由现场宏观烈度调查(图 8)与震源机制解(王晓山等，2018)可知该地震等震线长轴沿NE向展布，这种差异现象的出现可能与台站分布不均匀有关，震中南部无强震动记录，所以图 8中震中北部附近出现数据空白现象，不利于获取实际烈度长轴方向真实性。
图 8 永清4.3级地震校正前仪器烈度分布图Figure 8. The distribution of the un-corrected instrumental seismic intensity of Yongqing MS4.3 earthquake可靠快速生成地震烈度可为人员伤亡估计、应急救援决策和工程抢险修复决策提供重要依据，为解决由于缺少地震动观测值出现空白区域现象及烈度长轴方向和实际方向不一致的问题，本文首先利用永清4.3级地震中获取的所有台站加速度峰值，按地震动峰值衰减模型(王国新，2001)确定该地区地震动衰减关系，然后在整个地震区内建立空间随机假设台站进行补点插值，得到新的地震烈度分布图。地震动峰值衰减模型(王国新，2001)如下：
$$ \lg Y = {{\rm{C}}_1} + {{\rm{C}}_2}M + {{\rm{C}}_3}M\lg \left({R + {R_0}} \right) + {{\rm{C}}_4}\lg \left({R + {R_0}} \right) \pm \varepsilon $$ (1) 式中，Y为PGA(峰值加速度)，M为震级，R为震中距，C1、C2、C3、C4为待求常数，R0为近场饱和因子，ε为统计分析误差项。采用通用全局优化法拟合得到地震动峰值加速度随震级和震中距的变化规律，考虑本次地震震级较小，故选取震中距＜100km的Ⅰ类场地强震动数据进行拟合，其中水平向按EW、NS向加速度峰值矢量拟合回归分析，拟合曲线如图 9，计算得到的衰减关系式为：
$$ \lg Y = 1.6882 + 0.3926M - 0.1546M\lg \left({R + 5} \right) + 0.7000\lg \left({R + 5} \right) \pm 0.2287 $$ (2) 
图 9 永清4.3级地震现场调查烈度分布图Figure 9. The distribution of site investigation intensity of Yongqing MS4.3 earthquake峰值加速度随震中距的变化如图 10所示，由图 10可知峰值加速度随着震中距的增加而减小。同一震中距不同台站测得的峰值加速度离散性较大。通过拟合各台站实际PGA值，与俞言祥等(2013)地震动衰减关系进行对比，可知20km范围内2条曲线吻合较好，而20km外拟合曲线呈衰减相对缓慢状态，这可能与本次地震震级较小、记录台站均为土层台、远场有一定放大作用有关(王海云，2011)。由观测值得到的预测方程虽存在一定离散性，但整体趋势与实际结果保持一致。
图 10 峰值加速度随震中距的变化Figure 10. `The change of peak ground acceleration with epicentral distance在震区空白区域随机产生N个空间坐标的假设台站，按式(2)计算水平峰值加速度，按美国ShakeMap烈度计算方法转换成仪器烈度，绘制增加假设台站的烈度分布图(图 11)，由图 11可知，增加假设台站的仪器烈度分布图与现场烈度调查分布图极震区方向基本一致，进一步验证了河西务断裂为本次地震发震构造的可能，解决了台站分布不均匀导致地震烈度影响场计算出现缺值现象的问题，为以后缺少台站记录震区提供准确快速制作烈度分布图的思路，为震害调查和地震应急救援提供重要依据。
图 11 永清4.3级地震校正后烈度分布图Figure 11. The distribution of the corrected instrumental seismic intensity of Yongqing MS4.3 earthquake5. 结论
本文介绍了河北永清4.3级地震发震构造背景，并对国家强震动台网中心在本次地震中获取的加速度记录特征进行了分析，为河北省地震动特征和震害调查提供了一定参考，结论如下：
(1) 河北永清4.3级地震在廊固凹陷东侧控制断裂河西务断裂带附近，地震烈度极震区走向与该断裂展布方向一致，结合震源机制解初步判定本次地震发震构造为河西务断裂。
(2) 74个强震动台站获取到强震动加速度记录，震中距位于29.4—185.5km，其中觅子店台记录到的本次地震东西向最大峰值加速度为36.1cm/s2，垂直方向最大加速度为40.4cm/s2，于家务台记录到的南北向最大峰值加速度为18.5cm/s2。采用H/V谱比法对触发台站进行分类，可知Ⅰ类场地居多。
(3) 通过对5个典型台站反应谱分析发现永清4.3级地震加速度反应谱高频成分丰富，谱卓越周期主要为0.1—0.3s。傅氏谱谱型主要为单峰值型，UD分量频率范围分布较宽，EW分量傅氏谱最大峰值大于SN分量和UD分量。
(4) 通过分析峰值加速度反应谱与震中距、方位角的关系，发现峰值加速度反应谱整体随着方位角的增大而增大，达70°左右后下降，这可能与附近河西务断裂构造走向有关，有待进一步研究。其次，峰值加速度反应谱值整体随着震中距的增加而减小。
(5) 由本次地震仪器烈度分布图可知，台站分布不均匀性直接影响烈度分布的真实性，为避免此类问题，拟合出该地区地震动衰减关系，随机产生空间假设台站进行弥补，为以后缺少台站记录震区提供准确快速制作烈度分布图的思路，为震害调查和地震应急救援提供重要依据。由于收集到的强震动数据有限，文中仅采用本次地震获取的峰值加速度拟合当地地震动衰减关系，拟合结果可能存在误差，今后将收集更多的强震动数据进行拟合，以校正该地区地震动衰减关系。
-
图 1 自复位预制节段拼装CFDST桥墩构造示意图
Figure 1. Diagram of self-centering precast segmental assembled CFDST pier
图 3 自复位预制节段拼装CFDST桥墩数值模型
Figure 3. Numerical model of self-centering precast segmental assembled CFDST pier
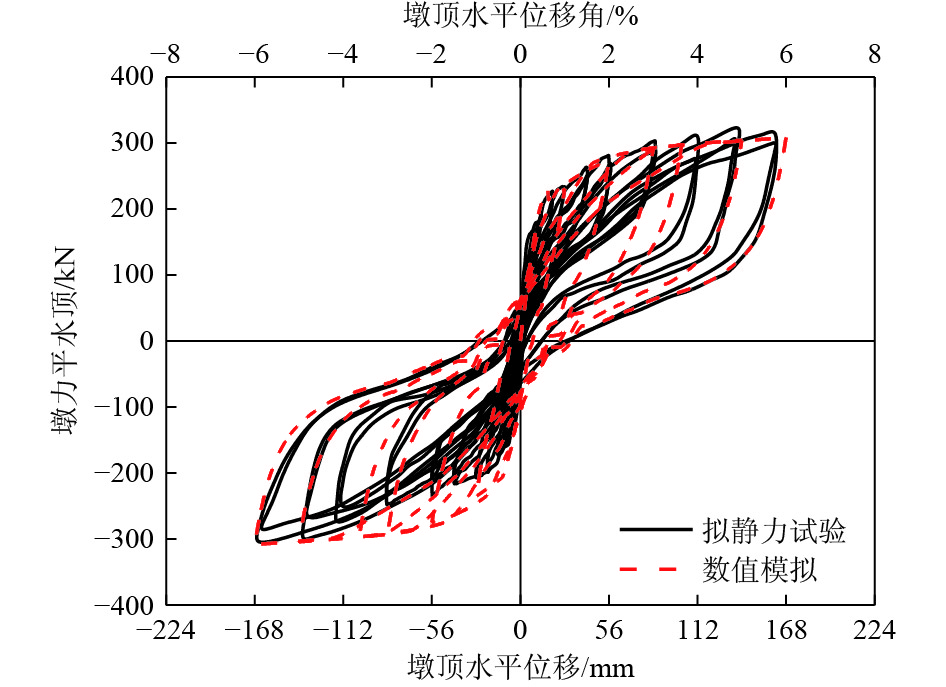
图 4 滞回曲线（Li等，2023b）
Figure 4. Hysteretic curve of self-centering precast segmental assembled CFDST piers （Li et al., 2023b）
图 5 基于最大水平位移角的地震概率需求模型
Figure 5. Earthquake probability demand model base on maximum horizontal displacement angle
图 6 基于残余位移角的地震概率需求模型
Figure 6. Earthquake probability demand model base on residual displacement angle
图 7 基于最大墩顶水平位移角指标的易损性曲线
Figure 7. Vulnerability curve based on the maximum horizontal displacement angle of pier top
图 8 基于墩顶残余位移角指标的易损性曲线
Figure 8. Vulnerability curve based on the residual displacement angle of pier top
图 9 近场无脉冲地震动作用下基于不同损伤指标的易损性曲线
Figure 9. Vulnerability curves based on different damage indexes under near-field non-pulse-like ground motion
图 10 近场脉冲地震动作用下基于不同损伤指标的易损性曲线
Figure 10. Vulnerability curves based on different damage indexes under near-field pulse-like ground motion
图 11 远场地震动作用下基于不同损伤指标的易损性曲线
Figure 11. Vulnerability curves based on different damage indexes under far-field ground motion
图 12 基于最大水平位移角的自复位预制节段拼装CFDST桥墩地震易损性曲线
Figure 12. Self-centering precast segmental CFDST pier vulnerability curves based on the maximum horizontal displacement angle under earthquake ground motions
图 13 基于残余位移角的自复位预制节段拼装CFDST桥墩地震易损性曲线
Figure 13. Self-centering precast segmental CFDST pier vulnerability curves based on the residual displacement angle under earthquake ground motions
表 1 关键性能指标
Table 1. Critical performance indexes
屈服位移/mm 屈服荷载/kN 峰值荷载/kN 弹性刚度/(kN·mm−1) 峰值残余位移/mm 试验 17.8 208 319 11.7 30.0 模拟 18.0 216 308 12.0 31.8 相对误差 1.1% 3.8% 3.4% 2.6% 6.0% 
下载: 导出CSV
表 2 远场地震动记录
Table 2. Far-field ground motion records
编号 地震名称 年份 站台名称 震级/级 Rrup/km T90%/s 1 "Northwest Calif-01" 1938 "Ferndale City Hall" 5.5 53.58 11.6 2 "Northwest Calif-02" 1941 "Ferndale City Hall" 6.6 91.22 22.2 3 "Northern Calif-01" 1941 "Ferndale City Hall" 6.4 44.68 15.5 4 "Borrego" 1942 "El Centro Array #9" 6.5 56.88 37.2 5 "Northwest Calif-03" 1951 "Ferndale City Hall" 5.8 53.77 15.4 6 "Kern County" 1952 "LA - Hollywood Stor FF" 7.36 117.75 33.5 7 "Kern County" 1952 "Pasadena - CIT Athenaeum" 7.36 125.59 29.5 8 "Kern County" 1952 "Santa Barbara Courthouse" 7.36 82.19 33.6 9 "Kern County" 1952 "Taft Lincoln School" 7.36 38.89 30.3 10 "Northern Calif-02" 1952 "Ferndale City Hall" 5.2 43.28 18.4 11 "Northern Calif-03" 1954 "Ferndale City Hall" 6.5 27.02 19.4 12 "El Alamo" 1956 "El Centro Array #9" 6.8 121.7 40.9 13 "Northern Calif-04" 1960 "Ferndale City Hall" 5.7 57.21 28.4 14 "Northern Calif-05" 1967 "Ferndale City Hall" 5.6 28.73 22.1 15 "Borrego Mtn" 1968 "El Centro Array #9" 6.63 45.66 49.3 16 "Borrego Mtn" 1968 "San Onofre - So Cal Edison" 6.63 129.11 28 17 "San Fernando" 1971 " 2516 Via Tejon PV"6.61 55.2 54.2 18 "San Fernando" 1971 "Carbon Canyon Dam" 6.61 61.79 18.9 19 "San Fernando" 1971 "Castaic-Old Ridge Route" 6.61 22.63 16.8 20 "San Fernando" 1971 "Fairmont Dam" 6.61 30.19 14.4
下载: 导出CSV
表 3 近场无脉冲型地震动
Table 3. Near-field non-pulse-like ground motion
编号 地震名称 年份 站台名称 震级/级 Rrup /km T90%/s 1 "Imperial Valley-02" 1935 "El Centro Array #9" 6.95 6.09 24.2 2 "Hollister-02" 1961 "Hollister City Hall" 5.5 18.08 16.5 3 "Parkfield" 1966 "Cholame - Shandon Array #12" 6.19 17.64 29 4 "Parkfield" 1966 "Cholame - Shandon Array #5" 6.19 9.58 7.5 5 "Parkfield" 1966 "Cholame - Shandon Array #8" 6.19 12.9 13.1 6 "Managua_Nicaragua-01" 1972 "Managua_ ESSO" 5.24 4.06 10.6 7 "Hollister-03" 1974 "Hollister City Hall" 5.17 9.39 10.9 8 "Coyote Lake" 1979 "Coyote Lake Dam - Southwest Abutment" 5.74 6.13 8.5 9 "Imperial Valley-06" 1979 "Calexico Fire Station" 6.53 10.45 14.8 10 "Imperial Valley-06" 1979 "Cerro Prieto" 6.53 15.19 36.4 11 "Imperial Valley-06" 1979 "Chihuahua" 6.53 7.29 24 12 "Imperial Valley-06" 1979 "Parachute Test Site" 6.53 12.69 18.6 13 "Imperial Valley-07" 1979 "El Centro Array #5" 5.01 11.23 7 14 "Imperial Valley-07" 1979 "El Centro Array #6" 5.01 10.37 6.5 15 "Mammoth Lakes-02" 1980 "Mammoth Lakes H. S." 5.69 9.12 3.9 16 "Mammoth Lakes-03" 1980 "Convict Creek" 5.91 12.43 6.3 17 "Mammoth Lakes-03" 1980 "Long Valley Dam （Downst）" 5.91 18.13 12.4 18 "Mammoth Lakes-03" 1980 "Long Valley Dam （Upr L Abut）" 5.91 18.13 8.4 19 "Mammoth Lakes-06 1980 "Fish & Game （FIS）" 5.94 12.93 5.1 20 "Westmorland" 1981 "Salton Sea Wildlife Refuge" 5.9 7.83 9.1
下载: 导出CSV
表 4 近场脉冲型地震动
Table 4. Near-field pulse-like ground motion records
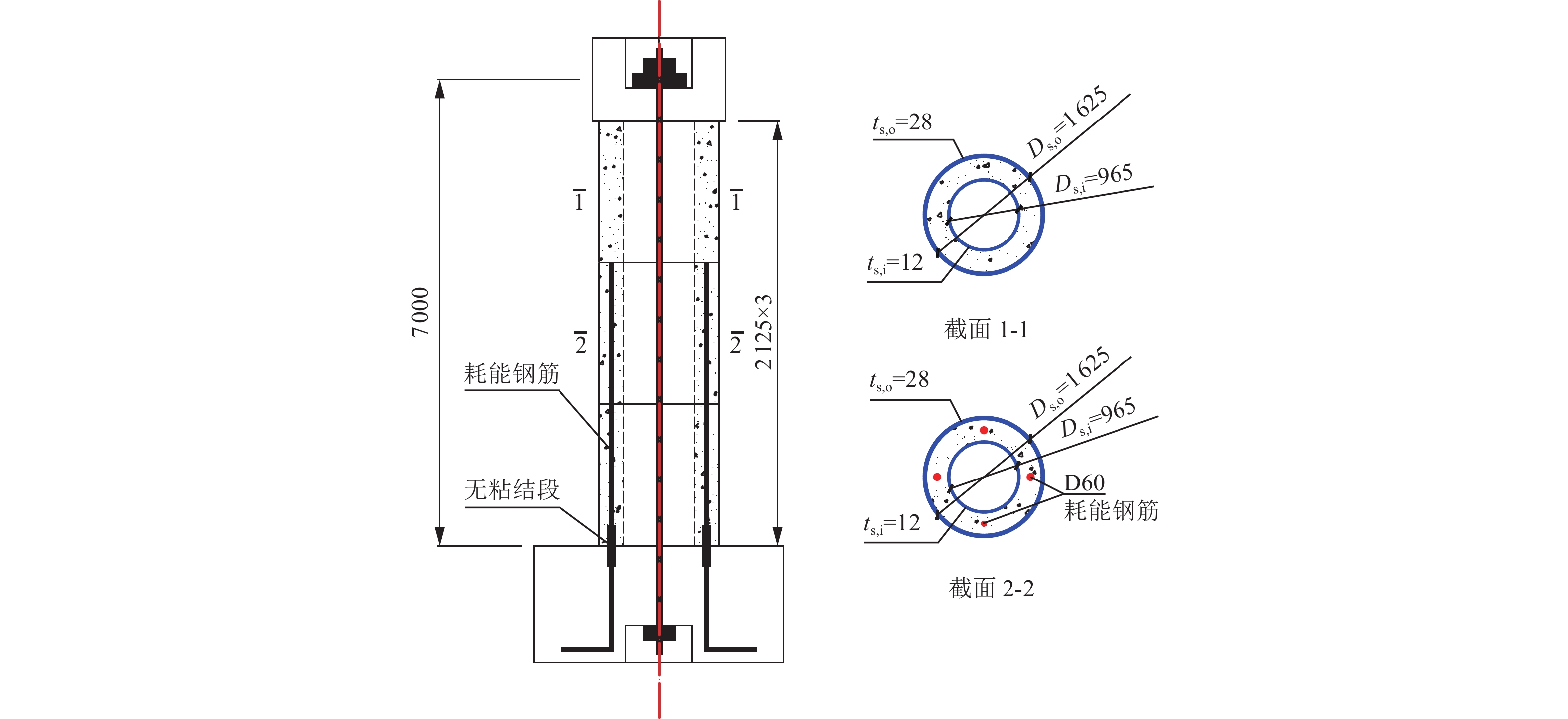
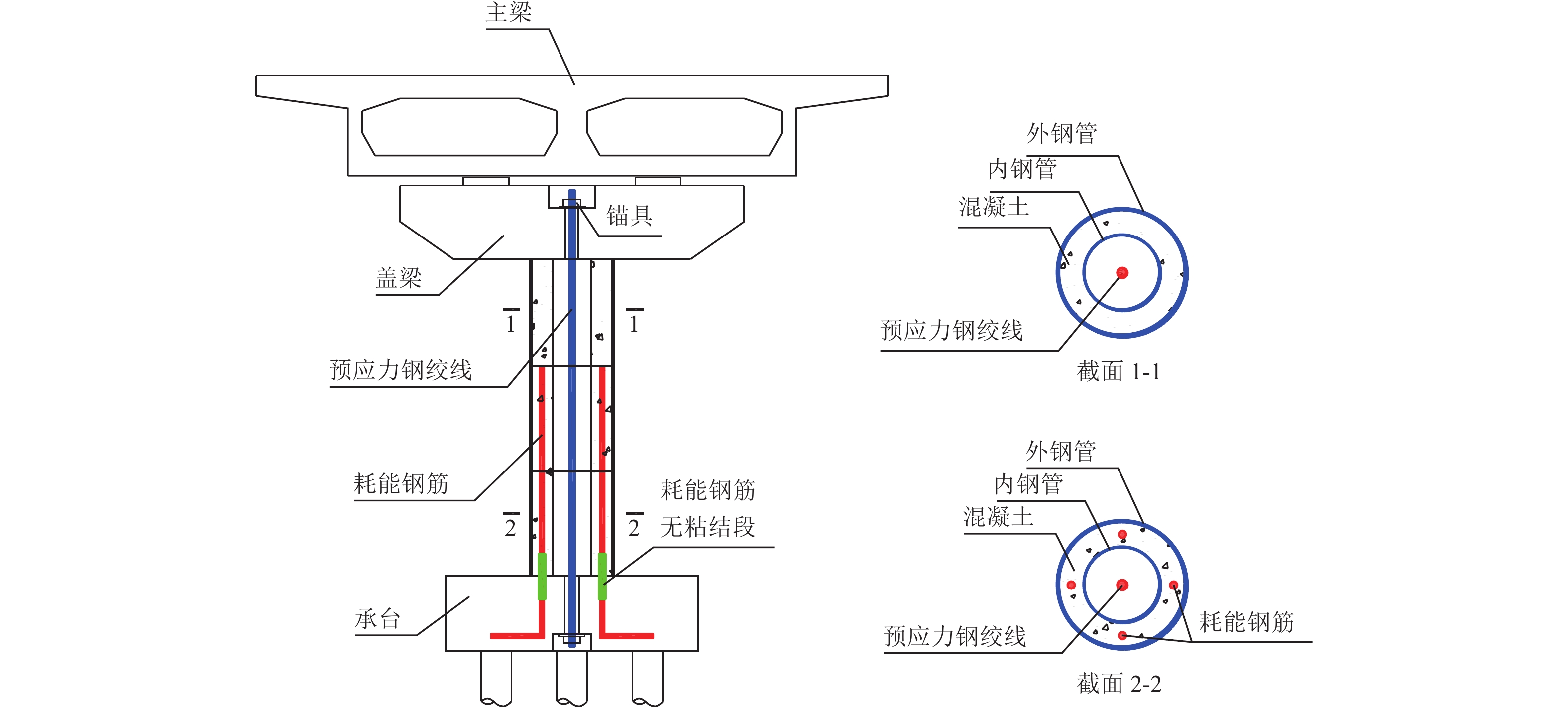
编号 地震名称 年份 站台名称 震级/级 Rrup/km T90%/s 1 "Coyote Lake" 1979 "Gilroy Array #2" 5.74 9.02 7.5 2 "Coyote Lake" 1979 "Gilroy Array #3" 5.74 7.42 8.7 3 "Coyote Lake" 1979 "Gilroy Array #4" 5.74 5.7 11 4 "Imperial Valley-06" 1979 "Agrarias" 6.53 0.65 13.3 5 "Imperial Valley-06" 1979 "Brawley Airport" 6.53 10.42 14.9 6 "Imperial Valley-06" 1979 "EC County Center FF" 6.53 7.31 13.2 7 "Imperial Valley-06" 1979 "El Centro Array #10" 6.53 8.6 12.8 8 "Imperial Valley-06" 1979 "El Centro Array #3" 6.53 12.85 14.1 9 "Imperial Valley-06" 1979 "Holtville Post Office" 6.53 7.5 12.8 10 "Irpinia_ Italy-01" 1980 "Bagnoli Irpinio" 6.9 8.18 19.6 11 "Irpinia_ Italy-01" 1980 "Sturno （STN）" 6.9 10.84 15.2 12 "Westmorland" 1981 "Parachute Test Site" 5.9 16.66 18.7 13 "Morgan Hill" 1984 "Gilroy Array #6" 6.19 9.87 7.3 14 "Kalamata_ Greece-02" 1986 "Kalamata （bsmt） （2 nd trigger）" 5.4 5.6 2.9 15 "Superstition Hills-02" 1987 "Kornbloom Road （temp）" 6.54 18.48 13.9 16 "Loma Prieta" 1989 "Gilroy - Historic Bldg." 6.93 10.97 13.1 17 "Loma Prieta" 1989 "Saratoga - W Valley Coll." 6.93 9.31 11.1 18 "Kocaeli_ Turkey" 1999 "Arcelik" 7.51 13.49 11.1 19 "Kocaeli_ Turkey" 1999 "Gebze" 7.51 10.92 8.2 20 "Coyote Lake" 1979 "Gilroy Array #2" 5.74 9.02 7.5
下载: 导出CSV
表 5 自复位预制节段拼装CFDST桥墩抗震性能水准指标取值范围
Table 5. Range of performance level indexes of self-centering precast segmental CFDST pier
性能等级 性能水准 耗能钢筋拉应变ε 钢绞线拉应变εp θdr θR Ⅰ 基本完好 $ < {\varepsilon _{\text{y}}}$ / <1.00 % / Ⅱ 轻微损伤 $ < {\varepsilon _{{\text{sh}}}} = 0.015$ / <2.25% <0.50% Ⅲ 可恢复损伤/生命安全 $ < 0.6{\varepsilon _{{\text{su}}}} = 0.06$ / <5.50% <1.00% Ⅳ 严重损伤/防止倒塌 $ < {\varepsilon _{{\text{su}}}} = 0.10$ $ < {\varepsilon _{{\text{py}}}} = 0.0086$ <8.50% <1.75% Ⅴ 局部失效/倒塌 $ > {\varepsilon _{{\text{su}}}} = 0.10$ $ > {\varepsilon _{{\text{py}}}} = 0.0086$ >8.50% >1.75%
下载: 导出CSV
表 6 桥墩概率地震需求模型
Table 6. Probabilistic earthquake demand model of pier
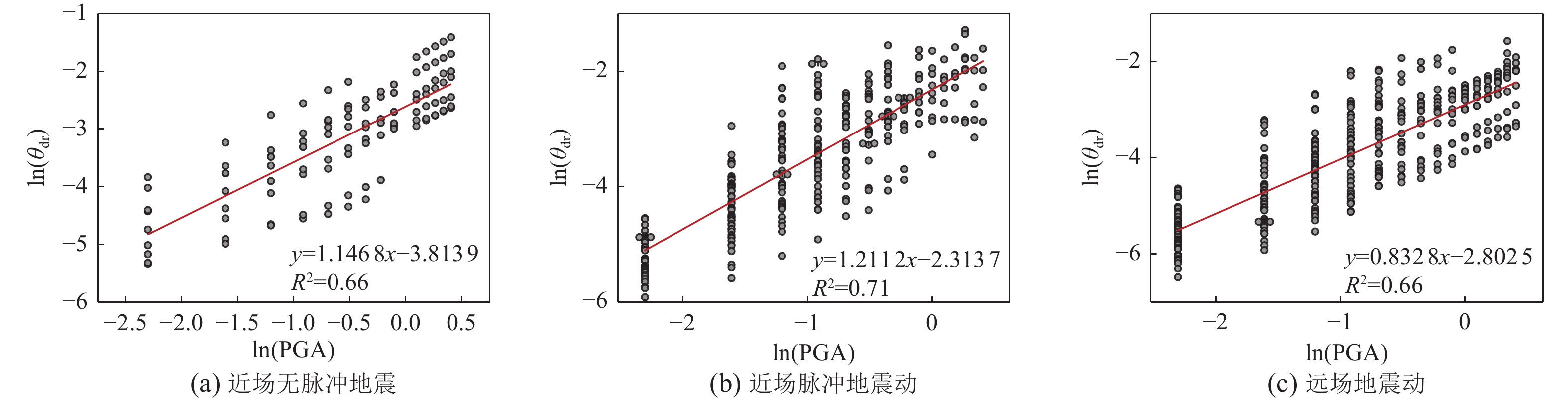
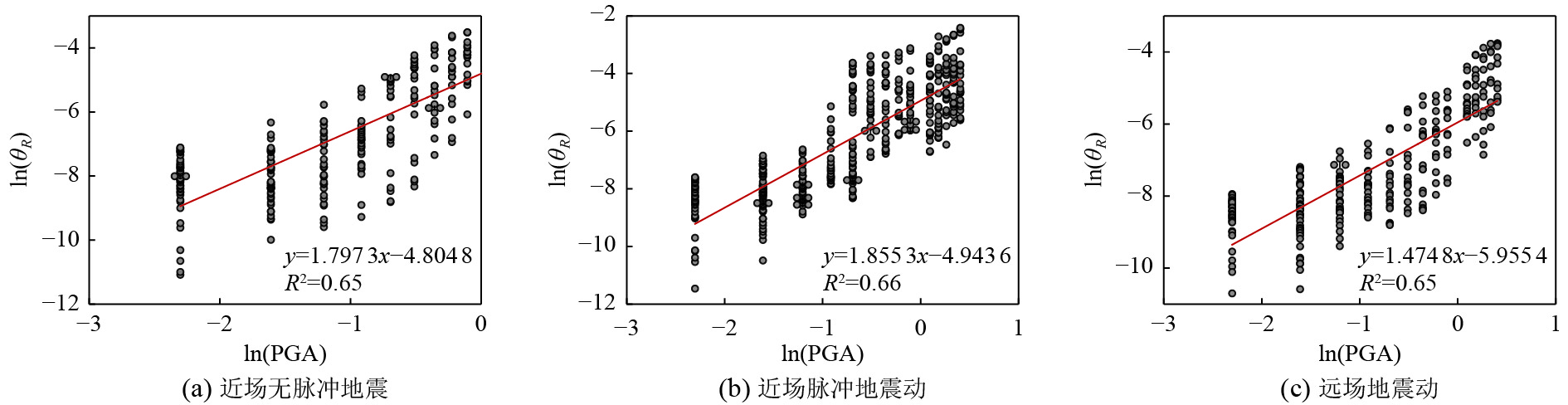
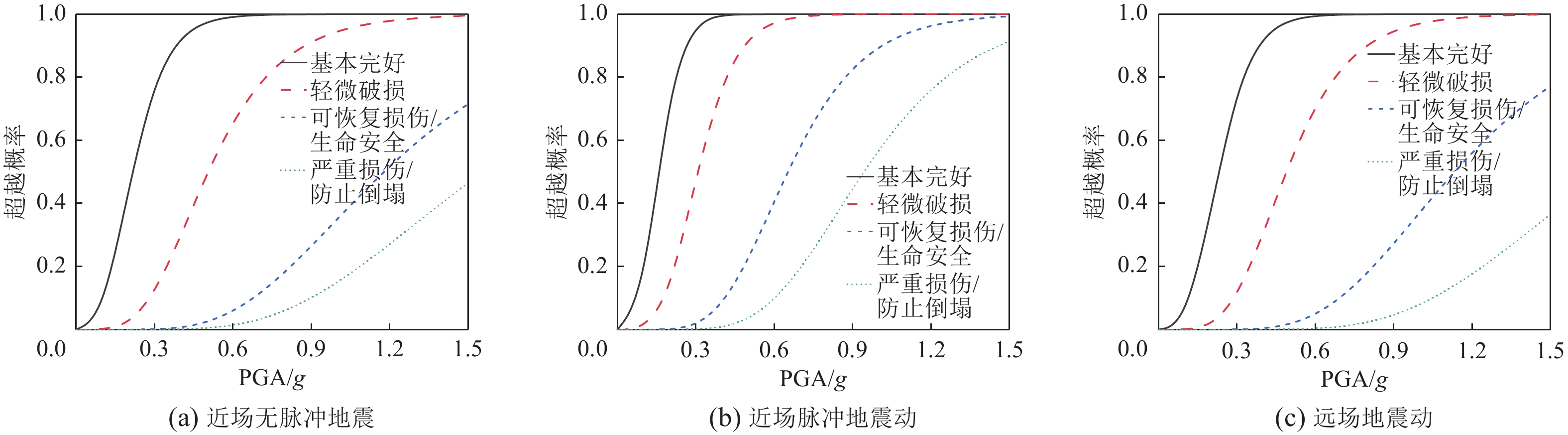
性能指标 近场无脉冲地震 近场脉冲地震动 远场地震动 墩顶最大水平位移角 ln(θdr) = 1.1468 ln(PGA)−3.8139 ln(θdr) = 1.21112 ln(PGA) −2.3137 ln(θdr) = 0.8328 ln(PGA) −2.8025 墩顶残余位移角 ln(θR) = 1.7973 ln(PGA) −4.8048 ln(θR) = 1.8553 ln(PGA) −4.9436 ln(θR) = 1.4748 ln(PGA) −5.9554
下载: 导出CSV
-
董慧慧,李辉,杜修力,2023. 近场脉冲型地震动横向激励下附加SCEB双柱式桥墩结构易损性分析. 北京工业大学学报,49(5):532−546. doi: 10.11936/bjutxb2021110002Dong H. H., Li H., Du X. L., 2023. Fragility analysis of the double-column bent with the SCEB under near-field pulse-like ground motions in transverse direction. Journal of Beijing University of Technology, 49(5): 532−546. (in Chinese) doi: 10.11936/bjutxb2021110002 葛继平,王志强,2011. 干接缝节段拼装桥墩振动台试验研究. 工程力学,28(9):122−128.Ge J. P., Wang Z. Q., 2011. Shake table tests of segmental bridge columns with match-cast dry joints. Engineering Mechanics, 28(9): 122−128. (in Chinese) 何益斌,李毅,郭健等,2012. 中空夹层钢管混凝土柱与钢-混凝土组合梁节点抗震性能试验研究. 建筑结构学报,33(7):106−115.He Y. B., Li Y., Guo J., et al., 2012. Experimental study on seismic behavior of concrete-filled double skin steel tubular column and steel-concrete beam composite joints. Journal of Building Structures, 33(7): 106−115. (in Chinese) 胡晓斌,贺慧高,2015. 强震下单自由度体系残余位移离散性研究. 地震工程与工程振动,35(6):213−218.Hu X. B., He H. G., 2015. Study on the dispersion of residual displacement of SDOF system under strong earthquake. Earthquake Engineering and Engineering Dynamics, 35(6): 213−218. (in Chinese) 胡志坚,闫明辉,周知等,2022. 预制拼装桥墩地震易损性分析. 土木工程学报,55(1):89−99,108.Hu Z. J., Yan M. H., Zhou Z., et al., 2022. Seismic vulnerability analysis of precast segmental bridge piers. China Civil Engineering Journal, 55(1): 89−99,108. (in Chinese) 贾晗曦,林均岐,刘金龙,2019. 建筑结构地震易损性分析研究综述. 震灾防御技术,14(1):42−51. doi: 10.11899/zzfy20190105Jia H. X., Lin J. Q., Liu J. L., 2019. Review of seismic fragility analysis of building structure. Technology for Earthquake Disaster Prevention, 14(1): 42−51. (in Chinese) doi: 10.11899/zzfy20190105 贾俊峰,赵建瑜,张强等,2017. 后张预应力节段拼装CFST桥墩抗侧力学行为试验. 中国公路学报,30(3):236−245. doi: 10.3969/j.issn.1001-7372.2017.03.026Jia J. F., Zhao J. Y., Zhang Q., et al., 2017. Experiment on lateral bearing behavior of post-tensioned segmental CFST bridge pier columns. China Journal of Highway and Transport, 30(3): 236−245. (in Chinese) doi: 10.3969/j.issn.1001-7372.2017.03.026 蒋欢军,王斌,吕西林,2010. 钢筋混凝土梁和柱性能界限状态及其变形限值. 建筑结构,40(1):10−14.Jiang H. J., Wang B., Lü X. L., 2010. Performance limit states and deformation limits of RC beams and columns. Building Structure, 40(1): 10−14. (in Chinese) 李宁,张双城,李忠献等,2020. 预制拼装钢管混凝土自复位桥墩变形分析模型及验证. 工程力学,37(4):135−143.Li N., Zhang S. C., Li Z. X., et al., 2020. Deformation analysis model and validation for precast segmental concrete filed steel tube self-centering bridge column. Engineering Mechanics, 37(4): 135−143. (in Chinese) 林上顺,厉良勇,叶世集等,2024. 采用混合连接装配式桥墩地震易损性分析. 地震工程学报,46(2):251−258,268.Lin S. S., Li L. Y., Ye S. J., et al., 2024. Seismic vulnerability analysis of assembled piers with a hybrid connection. China Earthquake Engineering Journal, 46(2): 251−258,268. (in Chinese) 刘黎明,徐超,卜春尧等,2021. 双向水平地震动作用对某钢筋混凝土连续梁桥易损性的影响. 震灾防御技术,16(4):671−679. doi: 10.11899/j.issn.1673-5722.2021.4.zzfyjs202104008Liu L. M., Xu C., Bu C. Y., et al., 2021. Influence of Bi-directional horizontal ground motion on the vulnerability of a reinforced concrete continuous beam bridge. Technology for Earthquake Disaster Prevention, 16(4): 671−679. (in Chinese) doi: 10.11899/j.issn.1673-5722.2021.4.zzfyjs202104008 刘艳辉,赵世春,强士中,2010. 城市高架桥抗震性能水准的量化. 西南交通大学学报,45(1):54−58,64. doi: 10.3969/j.issn.0258-2724.2010.01.009Liu Y. H., Zhao S. C., Qiang S. Z., 2010. Quantification of seismic performance levels for urban viaduct. Journal of Southwest Jiaotong University, 45(1): 54−58,64. (in Chinese) doi: 10.3969/j.issn.0258-2724.2010.01.009 罗征,李建中,2013. 低周往复荷载下空心矩形墩抗震性能试验研究. 振动与冲击,32(8):183−188.Luo Z., Li J. Z., 2013. Tests for a seismic performance of rectangular hollow thin-walled bridge columns under low-cycle reversed loading. Journal of Vibration and Shock, 32(8): 183−188. (in Chinese) 漆启明,邵长江,胡晨旭等,2020. 空心墩地震损伤评估及性能水准量化研究. 土木工程学报,53(11):116−128.Qi Q. M., Shao C. J., Hu C. X., et al., 2020. Study on seismic damage assessment and performance level quantification of hollow pier. China Civil Engineering Journal, 53(11): 116−128. (in Chinese) 任文静,邱大鹏,张智等,2024. 考虑构件地震相关性的近海桥梁二维地震易损性分析. 震灾防御技术,19(1):96−107. doi: 10.11899/zzfy20240110Ren W. J., Qiu D. P., Zhang Z., et al., 2024. The two-dimensional seismic fragility analysis of the offshore bridge in considering the seismic correlation between different components. Technology for Earthquake Disaster Prevention, 19(1): 96−107. (in Chinese) doi: 10.11899/zzfy20240110 石岩,张智超,李军等,2022. 考虑内力状态的大跨高墩连续刚构桥地震易损性分析. 地震研究,45(1):8−16.Shi Y., Zhang Z. C., Li J., et al., 2022. Seismic fragility of the long-span, continuous, rigid-frame bridge with high-rise pier involving the state of the internal force. Journal of Seismological Research, 45(1): 8−16. (in Chinese) 司炳君,谷明洋,孙治国等,2017. 近断层地震动下摇摆-自复位桥墩地震反应分析. 工程力学,34(10):87−97. doi: 10.6052/j.issn.1000-4750.2016.05.0386Si B. J., Gu M. Y., Sun Z. G., et al., 2017. Seismic response analysis of the rocking self-centering bridge piers under the near-fault ground motions. Engineering Mechanics, 34(10): 87−97. (in Chinese) doi: 10.6052/j.issn.1000-4750.2016.05.0386 孙治国,赵泰儀,石岩等. 2019. 摇摆-自复位桥墩抗震性能数值建模方法研究. 应用基础与工程科学学报, 27 (6):1357−1369.Sun Z. G., Zhao T. Y., Shi Y., et al., 2019. Research on numerical modeling method for rocking self-centering bridge piers. Journal of Basic Science and Engineering, 27 (6): 1357−1369. (in Chinese) 王军文,李海洋,闫聚考等,2018. 地震作用下钢筋混凝土桥墩残余位移研究. 振动与冲击,37(13):130−134.Wang J. W., Li H. Y., Yan J. K., et al., 2018. Residual displacements of RC piers under action of earthquake. Journal of Vibration and Shock, 37(13): 130−134. (in Chinese) 王震,2018. 自复位预制拼装UHPC空心墩抗震性能及设计方法研究. 南京:东南大学.Wang Z., 2018. Research on seismic performance and design method of self-centering precast segmental UHPC hollow bridge piers. Nanjing:Southeast University. (in Chinese) 魏标,2010. 典型非规则梁桥抗震设计理论. 上海:同济大学.Wei B., 2010. Seismic design theory of typical irregular continuous bridges. Shanghai:Tongji University. (in Chinese) 许成祥,罗恒,王粘锦,2022. 双层高架桥框架式桥墩地震易损性分析. 重庆交通大学学报(自然科学版),41(8):95−101. doi: 10.3969/j.issn.1674-0696.2022.08.14Xu C. X., Luo H., Wang Z. J., 2022. Seismic fragility analysis of double-deck Viaducts’ Frame piers. Journal of Chongqing Jiaotong University (Natural Science), 41(8): 95−101. (in Chinese) doi: 10.3969/j.issn.1674-0696.2022.08.14 曾武华,卓卫东,王东升,2021. RC桥墩残余位移指标影响因素分析及贝叶斯估计. 振动与冲击,40(19):145−150.Zeng W. H., Zhuo W. D., Wang D. S., 2021. Influence factors analysis and Bayesian estimation for residual displacement index of RC pier. Journal of Vibration and Shock, 40(19): 145−150. (in Chinese) 张勤,贡金鑫,周继凯,2017. 基于概率的单自由度体系震后残余变形计算. 建筑结构学报,38(8):74−82.Zhang Q., Gong J. X., Zhou J. K., 2017. Seismic residual deformation analysis of single degree of freedom system based on probability. Journal of Building Structures, 38(8): 74−82. (in Chinese) 张云,谭平,郑建勋等,2014. 基于性能的中小跨径装配式梁桥地震易损性分析. 振动工程学报,27(5):676−684. doi: 10.3969/j.issn.1004-4523.2014.05.005Zhang Y., Tan P., Zheng J. X., et al., 2014. Fragility analysis for performance-based seismic design of prefabricated bridge with middle-small span. Journal of Vibration Engineering, 27(5): 676−684. (in Chinese) doi: 10.3969/j.issn.1004-4523.2014.05.005 赵建锋,孙伟帅,李刚,2018. 不同轴压比钢筋混凝土圆柱桥墩地震易损性分析. 世界地震工程,34(4):31−37.Zhao J. F., Sun W. S., Li G., 2018. Seismic vulnerability analysis of cylindrical RC bridge piers with different axial compression ratios. World Earthquake Engineering, 34(4): 31−37. (in Chinese) 周雨龙,韩强,张劲泉等,2021. 消能自复位摇摆框架墩结构地震反应及易损性分析. 中国公路学报,34(11):153−164. doi: 10.3969/j.issn.1001-7372.2021.11.013Zhou Y. L., Han Q., Zhang J. Q., et al., 2021. Seismic response and fragility analysis of post-tensioned rocking bridge frames with dampers. China Journal of Highway and Transport, 34(11): 153−164. (in Chinese) doi: 10.3969/j.issn.1001-7372.2021.11.013 Ahmadi E., Kocakaplan S., Kashani M. M., 2022. Nonlinear seismic fragility analysis of a resilient precast post-tensioned segmental bridge pier. Sustainable and Resilient Infrastructure, 7(6): 823−841. doi: 10.1080/23789689.2022.2082644 Christopoulos C., Pampanin S., Priestley M. J. N., 2003. Performance-based seismic response of frame structures including residual deformations. Part I: single-degree of freedom systems. Journal of Earthquake Engineering, 7(1): 97−118. Dawood H., Elgawady M., Hewes J., 2014. Factors affecting the seismic behavior of segmental precast bridge columns. Frontiers of Structural and Civil Engineering, 8(4): 388−398. doi: 10.1007/s11709-014-0264-8 Filippou F. C., Popov E. P., Bertero V. V., 1983. Effects of bond deterioration on hysteretic behavior of reinforced concrete joints. Berkeley: Earthquake Engineering Research Center, University of California. Hewes J. T., Priestley M. J. N., 2002. Seismic design and performance of precast concrete segmental bridge columns. San Diego: University of California. Hose Y., Silva P., Seible F., 2000. Development of a performance evaluation database for concrete bridge components and systems under simulated seismic loads. Earthquake Spectra, 16(2): 413−442. doi: 10.1193/1.1586119 Japan Road Association, 2002. Design specifications for highway bridges: part v: seismic design. Japan: Maruzen Publishing Co, Ltd. Kawashima K., MacRae G. A., Hoshikuma J. I., et al., 1998. Residual displacement response spectrum. Journal of Structural Engineering, 124(5): 523−530. doi: 10.1061/(ASCE)0733-9445(1998)124:5(523) Kent D. C., Park R., 1971. Flexural members with confined concrete. Journal of the Structural Division, 97(7): 1969−1990. doi: 10.1061/JSDEAG.0002957 Kowalsky M. J., 2000. Deformation limit states for circular reinforced concrete bridge columns. Journal of Structural Engineering, 126(8): 869−878. doi: 10.1061/(ASCE)0733-9445(2000)126:8(869) Li Y. X., Li J. Z., Shen Y., 2021. Quasi-static and nonlinear time-history analyses of post-tensioned bridge rocking piers with internal ED bars. Structures, 32: 1455−1468. doi: 10.1016/j.istruc.2021.03.099 Li Z. X., Du C. Y., Liang X., et al., 2023a. Experimental and numerical investigation on hysteretic behavior of posttensioned precast segmental Concrete-filled double skin steel tubular piers. Structures, 54: 1772−1787. doi: 10.1016/j.istruc.2023.05.128 Li Z. X., Du C. Y., Liu D., et al., 2023b. Comparative study on seismic performance of concrete-filled double skin tubular piers and hollow concrete piers: experimental and analytical. Structures, 49: 1078−1092. doi: 10.1016/j.istruc.2022.11.116 Luco N., Cornell C. A., 2000. Effects of connection fractures on SMRF seismic drift demands. Journal of Structural Engineering, 126(1): 127−136. doi: 10.1061/(ASCE)0733-9445(2000)126:1(127) Mander J. B., Priestley M. J. N., Park R., 1988. Theoretical stress-strain model for confined concrete. Journal of Structural Engineering, 114(8): 1804−1826. doi: 10.1061/(ASCE)0733-9445(1988)114:8(1804) Mander J. B., Cheng C. T., 1997. Seismic resistance of bridge piers based on damage avoidance design. Buffalo: National Center for Earthquake Engineering Research, State University of New York. Mazzoni S., McKenna F., Scott M. H., et al., 2009. Open system for earthquake engineering simulation user command-language manual. version 2.0. Berkeley: Pacific Earthquake Engineering Research Center, University of California. Menegotto M., 1973. Method of analysis for cyclically loaded R. C. plane frames including changes in geometry and non-elastic behavior of elements under combined normal force and bending. In: Proceedings of the IABSE Symposium on Resistance and Ultimate Deformablility of Structures Acted on by Well-Difined Repeated. 15−22. Muntasir Billah A. H. M., Shahria Alam M., 2015a. Seismic fragility assessment of highway bridges: a state-of-the-art review. Structure and Infrastructure Engineering, 11(6): 804−832. doi: 10.1080/15732479.2014.912243 Muntasir Billah A. H. M., Shahria Alam M., 2015b. Seismic fragility assessment of concrete bridge pier reinforced with superelastic shape memory alloy. Earthquake Spectra, 31(3): 1515−1541. doi: 10.1193/112512EQS337M Ou Y. C., Wang P. H., Tsai M. S., et al., 2010. Large-scale experimental study of Precast segmental unbonded posttensioned concrete bridge columns for seismic regions. Journal of Structural Engineering, 136(3): 255−264. doi: 10.1061/(ASCE)ST.1943-541X.0000110 Zhang Y. Y., Li Y. H., Fan W., et al., 2022. Seismic damage and assessment model analysis of prestressed segmental bridge columns. Structures, 38: 797−807. doi: 10.1016/j.istruc.2022.02.018 -
下载:
点击查看大图
计量
- 文章访问数: 108
- HTML全文浏览量: 44
- PDF下载量: 24
- 被引次数: 0
