Seismic Vulnerability Analysis of Masonry Structures with Uncertain Parameters Based on DOE
-
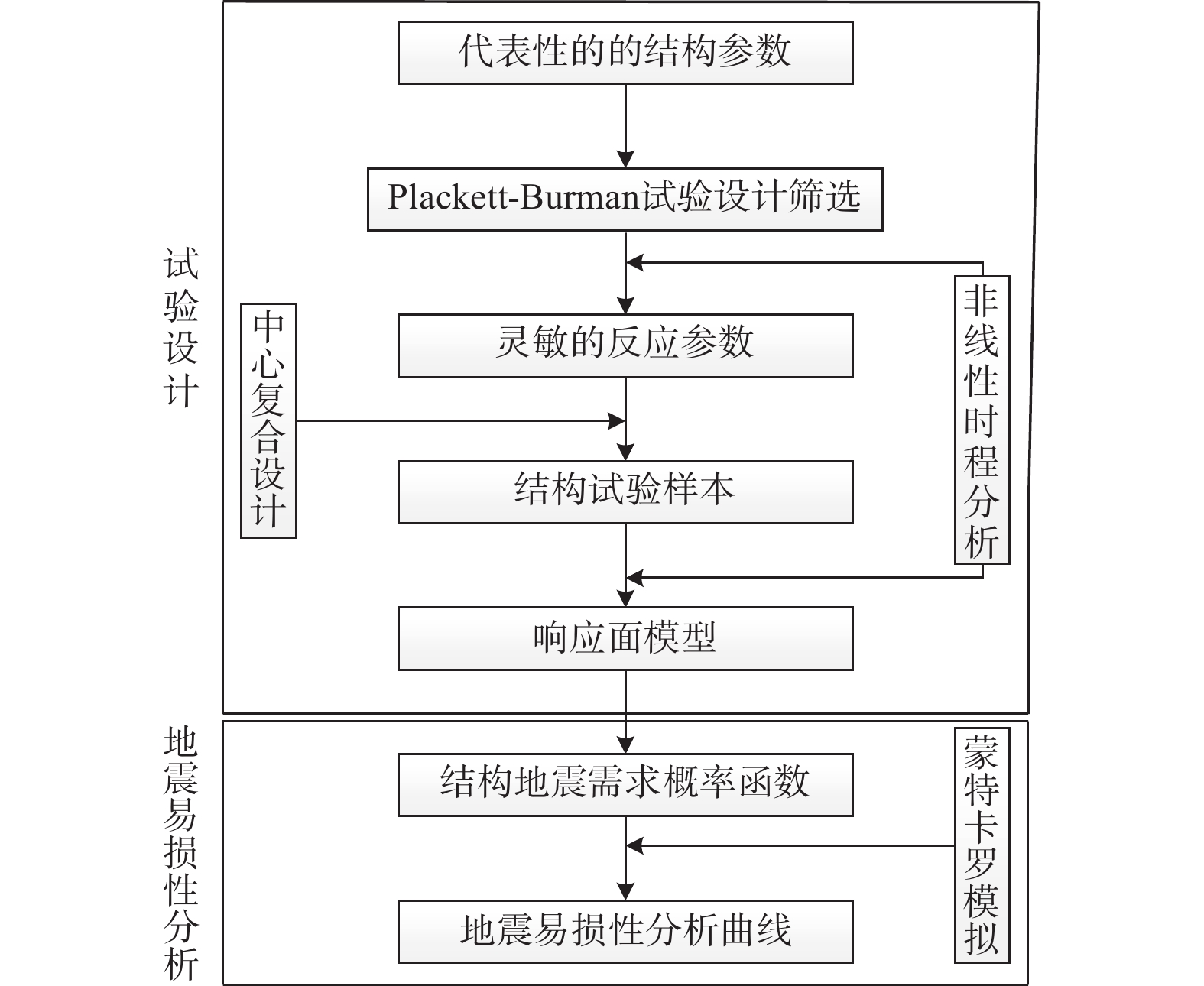
摘要: 砌体结构参数具有离散性高且其受到地震作用后响应结果非线性强等特点,所以在砌体地震易损性研究中结构参数不确定性对结果产生的影响不容忽视。针对汶川地震中具有代表性的砌体结构,提出基于DOE(试验设计)方法考虑在砌体结构地震易损性研究中结构参数不确定性的影响。首先,使用Plackett-Burman方法开展结构参数的灵敏度分析,筛选出对砌体结构地震响应影响较大的3个结构参数;然后,根据筛选结果及地震参数PGA进行试验设计,进而建立结构参数与最大层间位移角的响应面回归模型;最后,通过进行蒙特卡罗模拟获得地震易损性曲线,并进一步评估结构参数不确定性对砌体地震易损性分析的影响程度。研究结果表明,弹性模量、密度和抗拉强度是对砌体结构易损性影响较大的3个参数;当地震动PGA为0.1 g时,相较于其他结构参数,弹性模量对结构地震响应的影响最显著;当PGA为0.2 g时,3个结构参数的影响由大到小依次为弹性模量、密度和抗拉强度,当PGA为0.4 g时,3个结构参数的影响由大到小依次为弹性模量、抗拉强度与密度。本文所提出的方法计算量少、精度高,可为有效解决砌体结构参数种类众多和材料非线性强等难题提供新思路。Abstract: Given the high variability of masonry structural parameters and the strong nonlinearity of the seismic response after earthquake activity, the uncertainty of structural parameters plays a significant role in the seismic vulnerability assessment of masonry structures. This study proposes the use of a Design of Experiments (DOE) method to account for the influence of structural parameter uncertainty, with a focus on masonry structures affected by the Wenchuan earthquake. First, the Plackett-Burman method was employed to perform a sensitivity analysis of structural parameters, identifying the three parameters that most significantly affect the seismic response of masonry structures. Based on these results and the peak ground acceleration (PGA) as the seismic parameter, the DOE was conducted, leading to the development of a response surface regression model between the structural parameters and the maximum inter-story drift angle. Finally, a Monte Carlo simulation was performed to derive the seismic vulnerability curve, further assessing the impact of structural parameter uncertainty on the seismic vulnerability analysis of masonry structures. The results indicate that: (1) the elastic modulus, masonry density, and tensile strength are the three parameters with the greatest impact on the seismic vulnerability of masonry structures; (2) when the local PGA is 0.1g, the elastic modulus has the most significant influence on the seismic response compared to other parameters. At a PGA of 0.2 g, the influence order is elastic modulus, masonry density, and tensile strength, while at a PGA of 0.4 g, the order changes to elastic modulus, masonry tensile strength, and masonry density. The proposed method in this study has low computational complexity and high accuracy, offering an effective approach for addressing the numerous structural parameters and strong material nonlinearity in masonry structures. This approach provides new ideas in improving seismic vulnerability assessments for such structures.
-
引言
近年来,我国经济高速发展的同时能源需求持续增长,发展核能是确保我国能源供应安全的有力保障,同时全球自然灾害频发也对核能应用安全提出了更高要求,由于我国位于环太平洋地震带与欧亚地震带的交汇地带,核电结构地震安全性评价十分必要,除要求考虑土-结构相互作用外,还需考虑周围邻近结构的影响(Lou等,2011;王国波等,2018),即结构-土-结构相互作用。
相邻结构之间的相互作用是基于土-结构相互作用研究成果的拓展,当介质中存在多个结构时,由于结构响应引起土体扰动,并通过土体传播,土-结构问题演变为多个结构之间的交叉相互作用问题,Whitman(1969)首次提出基础通过土体相互耦合的问题,前期研究土-结构系统模型多采用弹性地基上单质量系统模型。Warburton等(1971)将地基考虑为弹性半空间,相邻上部结构简化为圆柱质量块,基础假设为刚性圆形基础,研究结果表明当1个质量块被外部谐波激发时,第2个质量块通过相对较小的扰动改变受激质量的位移分量。支承在柔性基础上的结构动力响应与支承在刚性基础上的结构动力响应有很大不同(Lehmann等,2001;李培振等,2014),刚性基础的假设与震时系统实际运动状态存在一定差距,产生差异的重要原因之一是结构振动产生的能量部分通过柔性基础耗散。田彼得等(1987)基于频域采用子结构法对相邻结构动力相互作用体系动力特性进行了分析,研究结果表明高大结构的存在使低矮结构顶层相对位移频响曲线的第1共振频率显著降低。目前基于频域仅可采用线性方法或等效线性化近似考虑非线性问题,而地震波激励下结构一般会进入非线性状态。柳玉印等(2018)对相邻新、旧重力坝的彼此相互作用进行模拟,结果表明相邻结构的存在对结构峰值响应有一定影响。Luco等(1973)、Murakami等(1977)及Wong等(1975)用简化的二维平面应变模型模拟无限长三维结构,研究结果表明在低频情况下,对于邻近较大结构物的较小结构物而言,结构-土-结构相互作用(Structure-Soil-Structure Interaction,SSSI)的影响较大,而高频情况下可忽略邻近结构,仅考虑单体结构;地震波能量主要集中在低频区域,可见在地震波激励下相邻高层建筑对底层建筑相邻结构的相互作用影响较大。但以上研究简化了上部结构模型,忽略了上部结构尺寸和土体之间相互作用的影响。Ghandil等(2016)利用等效线性模型近似考虑了土体中的大剪切应变,通过研究3个不同高度相邻建筑的相互作用,发现当建筑物间距为建筑基底宽度的一半时,相互作用不可忽视。Aldaikh等(2015,2016)探讨了地震波激励下SSSI对3个相邻建筑的影响,提出了3个相邻建筑通过土壤耦合的理论公式,并通过振动台试验证明该简单理论模型在描述线弹性半空间上相邻建筑群的动力相互作用是有效的。何涛等(2020)依托振动台试验并结合子结构试验技术,以2个4层钢框架为例,模拟了震时相邻结构的加速度放大系数,变化规律,研究结果表明考虑相邻结构影响时的震时地震响应峰值较单体结构小。Wang等(2022)针对不同尺度的土-结构相互作用(Soil-Structure Interaction,SSI)系统和SSSI系统进行了振动台试验,结果表明SSSI效应对结构反应的影响随着结构间距的增大而减小,随着结构尺寸的增大和地震波激励方向的改变而增大或减小。韩冰等(2019)采用间接边界元法研究了SSSI效应对系统频率的影响,并得出随着结构间距的增大相邻结构相互作用对结构系统频率的影响呈下降趋势的结论。以上研究充分说明了震时相邻结构的存在在一定程度上改变了原有结构的动力特性,且入射波角度的改变可能导致相邻结构系统处于最不利工况状态。
反应堆厂房通常不是独立于附属结构而存在,受限于核能发电的工艺要求,反应堆厂房周围需建造一系列辅助厂房,以维持整个核电站的正常运作,如果SSSI效应影响较大,应将其作为核电站抗震设计和安全评估的重要特性之一。近年来,关于核电站SSSI的研究发展迅速,Lee等(1973a,1973b)采用三维结构模型研究了SSSI效应对相邻反应堆厂房地震响应的影响,并提出了近似解析-数值方法,以求解弹性半空间表面上3个刚性圆形基础的相互作用。Kitada等(1999)对邻近反应堆厂房进行了一系列现场原位试验,研究发现SSSI的影响与外部激励荷载方向和相邻结构空间位置密切相关。Matthees等(1982)对核电站相邻结构在水平地震作用下相互作用的灵敏度进行了研究,结果表明相互作用现象对核电结构响应的影响是不可忽视的。上述研究中相邻结构的基础是分离的,考虑核电结构特殊性及其区别于一般民用建筑的空间布置,核电结构为增强整体性,减少地震作用下邻近基础的相互影响,设计时将核电附属厂房与反应堆厂房共用1个基础,应稼年等(1995)在同一基础上考虑了2个辅助厂房与安全壳在地震波激励下的平-扭耦联动力响应,并指出邻近厂房的存在会增加反应堆厂房震时响应幅值。
考虑相邻厂房对反应堆厂房的影响,即考虑SSSI效应,计算量较大,因此已有研究多将结构模型简化,若对结构进行较详细地建模分析,需采用高效的计算方法。本文在核电结构中土-结构相互作用分区分析方法(PASSI)的基础上,提出显-隐式单元层计算方法,可在满足高效性的同时,提高稳定性。以我国某核电站为研究对象,其4个辅助厂房同反应堆厂房建造在同个基础上,设计了4个工况模型,分析了周围辅助厂房对反应堆厂房自振频率及地震响应的影响。
1. 基本理论
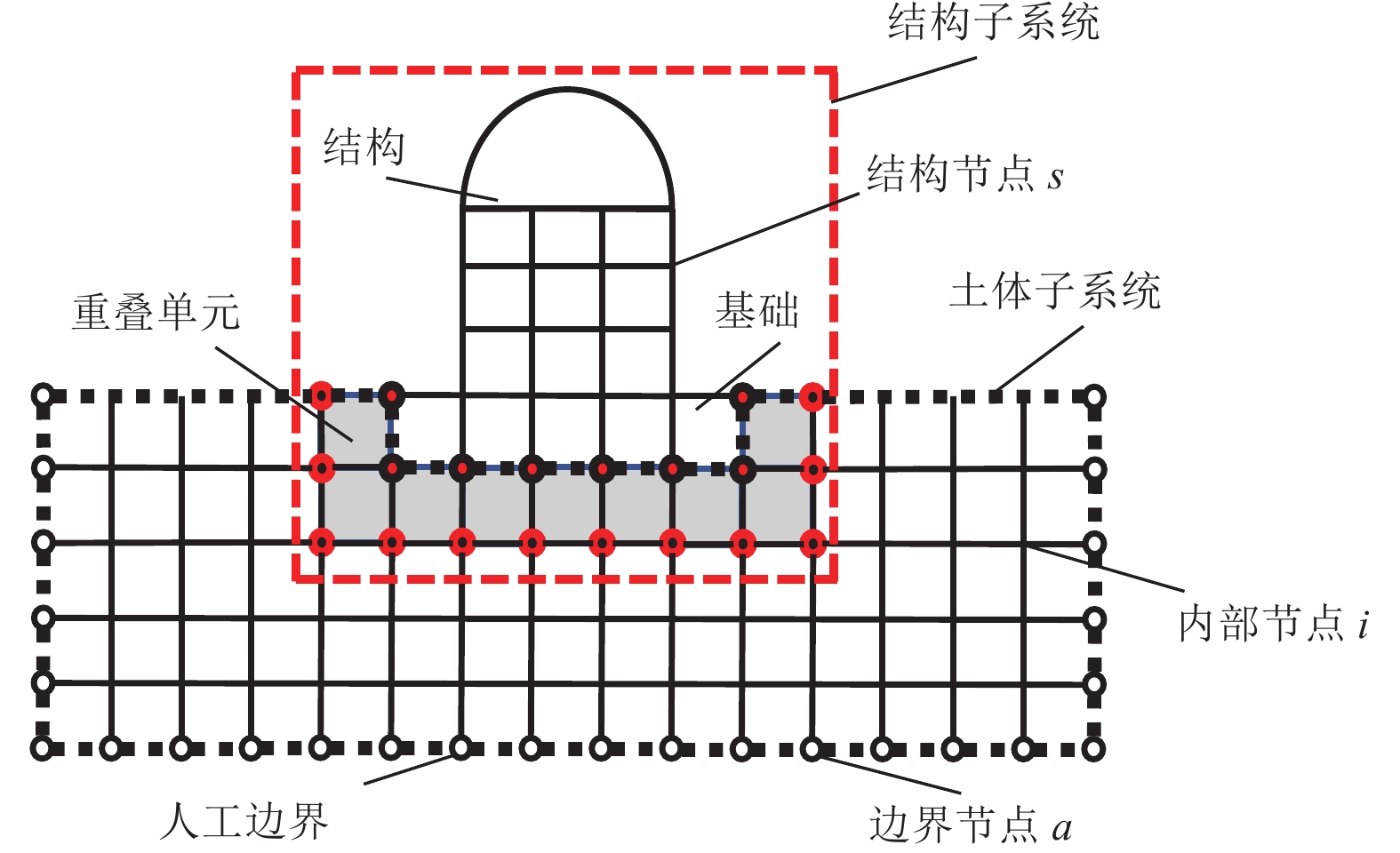
SSI系统分析模型如图1所示。该系统由两部分组成,分别为结构子系统和土体子系统,结构子系统包含上部结构、基础和部分土体,土体子系统为全部土体区域,其与结构子系统存在重叠区域。
1.1 土体子系统
将土体离散成八节点六面体单元,并将节点划分为内部点和人工边界点,如图1所示。内部点采用集中质量有限元法计算(廖振鹏,2002),动力方程的一般形式可写为:
$$ {{\boldsymbol{M}}_i}{\ddot {\boldsymbol{u}}_i} + \sum\limits_j^N {{{\boldsymbol{C}}_{ij}}{{\dot {\boldsymbol{u}}}_i}} + \sum\limits_j^N {{{\boldsymbol{K}}_{ij}}{{\boldsymbol{u}}_i}} = {{\boldsymbol{F}}_i} $$ (1) 式中,
$ {{\boldsymbol{M}}_i} $ 为集中在内部点i上的质量阵,具体形式如式(2)所示;$ {{\boldsymbol{C}}_{ij}} $ 和$ {{\boldsymbol{K}}_{ij}} $ 分别为内部点i与相邻节点j之间的阻尼阵和刚度阵;$ {\ddot {\boldsymbol{u}}_i} $ 、$ {\dot {\boldsymbol{u}}_i} $ 和$ {{\boldsymbol{u}}_i} $ 分别为内部点i的加速度向量、速度向量、位移向量;N为与节点i相邻节点总数;$ {F_{{i}}} $ 为内部点i的外部荷载向量。$$ {{\boldsymbol{M}}_i}{\text{ = }}\left[ {\begin{array}{*{20}{c}} {{m_i}}&0&0 \\ 0&{{m_i}}&0 \\ 0&0&{{m_i}} \end{array}} \right] $$ (2) 对式(1)采用以下时步积分格式离散:
$$ \dot {\boldsymbol{u}}_i^p = \frac{{{\boldsymbol{u}}_i^p - {\boldsymbol{u}}_i^{p - 1}}}{{\Delta t}} $$ (3) $$ \ddot {\boldsymbol{u}}_i^p = \frac{{{\boldsymbol{u}}_i^{p + 1} - 2{\boldsymbol{u}}_i^p + {\boldsymbol{u}}_i^{p - 1}}}{{\Delta {t^2}}} $$ (4) 式中,
$ {\boldsymbol{u}}_i^{p + 1}、{\boldsymbol{u}}_i^p、{\boldsymbol{u}}_i^{p - 1} $ 分别为i节点$ p + 1、p、p - 1 $ 时刻的位移;$ \Delta t $ 为时间步长;$ \dot {\boldsymbol{u}}_i^p、\ddot {\boldsymbol{u}}_i^p $ 分别为i节点$ p $ 时刻速度和加速度。进而得到:
$$ {\boldsymbol{u}}_i^{p + 1} = 2{\boldsymbol{u}}_i^p - {\boldsymbol{u}}_i^{p - 1} - \frac{{\Delta t}}{{{{{m}}_i}}}\sum\limits_e {{\boldsymbol{c}}_i^e} ({\boldsymbol{u}}_i^p - {\boldsymbol{u}}_i^{p - 1}) - \frac{{\Delta {t^2}}}{{{m_i}}}\sum\limits_e {{\boldsymbol{k}}_i^e} {\boldsymbol{u}}_i^p + \frac{{\Delta {t^2}}}{{{m_i}}}{{\boldsymbol{F}}_i} $$ (5) 采用刘晶波等(2005,2022)提出的黏弹性人工边界,在边界上设置切向、法向弹簧和阻尼,按式(6)、式(7)计算:
$$ {K_{{\rm{BT}}}} = {\alpha _{\rm{T}}}\frac{G}{R} ,\qquad {C_{{\rm{BT}}}} = \rho {C_{\text{S}}} $$ (6) $$ {K_{{\rm{NT}}}} = {\alpha _{\rm{N}}}\frac{G}{R} ,\qquad {C_{{\rm{NT}}}} = \rho {C_{\rm{P}}} $$ (7) 式中,G为场地的剪切模量;
$ {K_{{\rm{BT}}}}、{K_{{\rm{NT}}}} $ 为别切向和法向弹簧刚度;$ {\alpha _{\rm{T}}}、{\alpha _{\rm{N}}} $ 分别为粘弹性人工边界的切向和法向修正系数;R为波源点至人工边界的距离;$ {C_{{\rm{BT}}}}、{C_{{\rm{NT}}}} $ 分别为切向阻尼和法向系数;ρ为介质密度;$ {C_{\rm{S}}}、{C_{\rm{P}}} $ 分别为介质的剪切波和压缩波波速。在人工边界上施加地震动输入的等效节点力为:
$$ {{\boldsymbol{F}}_b} = ({{\boldsymbol{\sigma}} _b} + c{\dot {\boldsymbol{u}}_b} + k{{\boldsymbol{u}}_b})A $$ (8) 式中,
$ {{\boldsymbol{\sigma}} _b} $ 为边界点上b点在t时刻的自由场应力;c和k分别为式(6)、式(7)中的弹簧和阻尼系数;$ {\dot {\boldsymbol{u}}_b} $ 、$ {{\boldsymbol{u}}_b} $ 分别为人工边界处自由场速度向量和位移向量;A为边界点影响面积。1.2 结构子系统
结构子系统运动方程为:
$$ {\boldsymbol{M}}\ddot u + {\boldsymbol{C}}\dot u + {\boldsymbol{K}}u = {\boldsymbol{F}} $$ (9) 采用Newmark隐式算法进行瞬态动力直接积分得:
$$ ({b_1}{\boldsymbol{M}} + {b_4}{\boldsymbol{C}} + {\boldsymbol{K}}){{\boldsymbol{u}}^{p + 1}} = {{\boldsymbol{F}}^{p + 1}} + {\boldsymbol{M}}({b_1}{{\boldsymbol{u}}^p} - {b_2}{\dot {\boldsymbol{u}}^p} - {b_3}{\ddot {\boldsymbol{u}}^p}) + {\boldsymbol{C}}({b_4}{{\boldsymbol{u}}^p} - {b_5}{\dot {\boldsymbol{u}}^p} - {b_6}{\ddot {\boldsymbol{u}}^p}) $$ (10) $$ {b_1} = 1/(\beta \Delta {t^2}),{b_2} = 1/(\beta \Delta t),{b_3} = 1 - 1/(2\beta ),{b_4} = \gamma \Delta t{b_1},{b_5} = 1 + \gamma \Delta t{b_2},{b_6} = \Delta t(1 + \gamma {b_3} - \gamma ) $$ (11) 求解式(10)可得结构上各节点反应。
1.3 显-隐式单元层计算方法
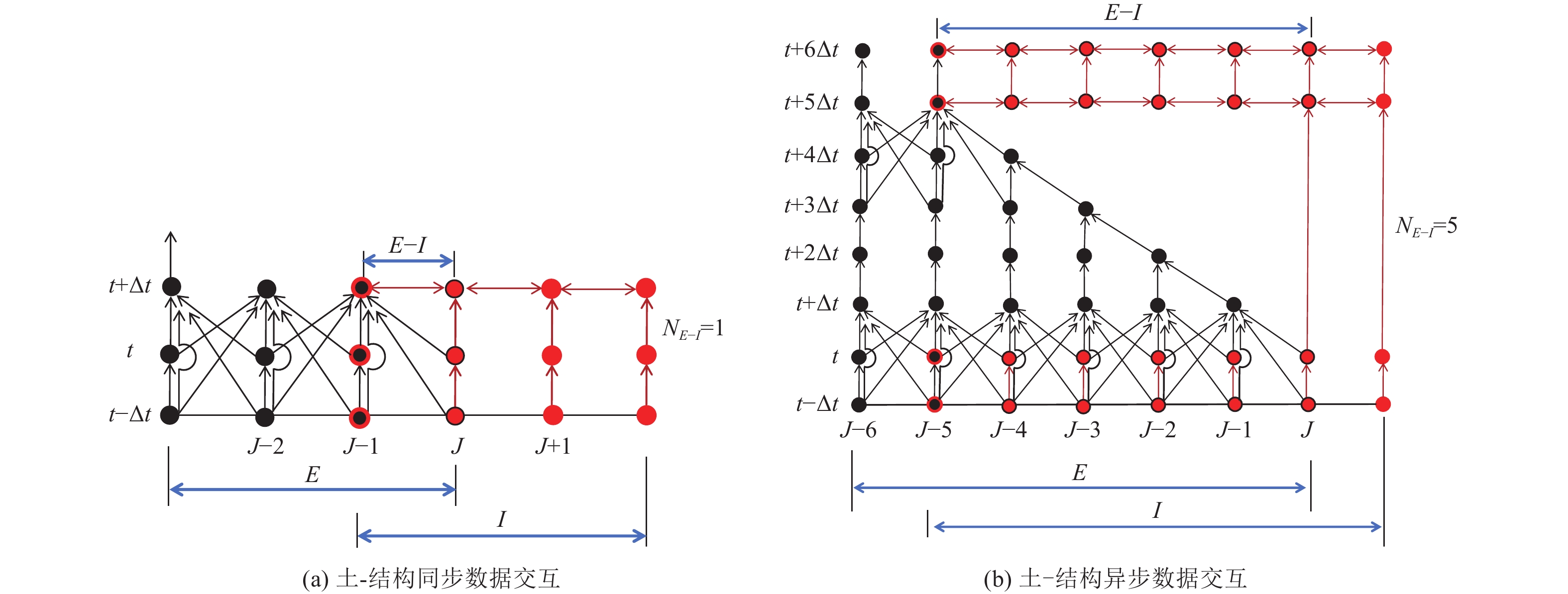
在PASSI中,显、隐式域不重叠,采用交互力和位移的方式进行耦合,当应用于柔性基础时,稳定性欠佳。设置显-隐式单元层,该单元层既属于显式单元,又属于隐式单元,为显、隐式单元的重叠区,通过交互位移的方式实现耦合分析(Belytschko等,1978)。结构子系统采用隐式分析,为隐式单元;土体子系统采用显式分析,为显式单元;结构子系统与土体子系统的重叠区域为显-隐式单元层。结构子系统边界点(图1中黑色实心、红色外圆点)同时也是土体子系统的内部节点,同样,土体子系统边界点(图1中红色实心、黑色外圆点)也是结构子系统的内部节点。以一维网格为例,说明显-隐式交替计算的实现,如图2(a)所示,将节点分为2个集合,即显式计算节点和隐式计算节点,分别用黑色和红色实心圆点表示;将单元分为3个集合,即显式单元(E)、隐式单元(I)和显-隐式单元(EI),显-隐式单元为显式单元和隐式单元重叠区,图中红色实心、黑色外圆点既是显式单元区的边界点,又是隐式单元区的内部点,计算中按隐式求解;黑色实心、红色外圆点既是隐式单元区的边界点,又是显式单元区的内部点,计算中按显式求解。显式计算点的网格信息流用黑色实线表示,隐式计算点的信息流用红色实线表示。假设
$ t $ 和($ t - \Delta t $ )时刻系统反应已知,可由式(5)将显式计算点(含隐式单元区的边界点,图2(a)中的J−1点)更新到($ t + \Delta t $ )时刻,显式内部点J−1的位移传递至隐式边界点J−1,可由式(10)计算得到隐式节点($ t + \Delta t $ )时刻的响应,再将隐式单元内部点(图2(a)中的J节点)的反应传递至显式单元区,从而将整个系统$ t $ 时刻响应更新到($ t + \Delta t $ )时刻,重复该过程,即可实现系统的时程分析。当隐式部分时间步长为显式部分时间步长的NE−I倍时,在隐式时间步长内,信息可在显式网格中跨越NE−I个节点,那么E−I单元层须包含NE−I层单元。以图2(b)所示N=5为例(Belytschko等,1979,1985),需在时间步(
$ t + 5\Delta t $ )内更新J−5之后的所有显式节点,求显式域$ u_{J - 5}^{t + 5\Delta t} $ 时,$ u_{J - 6}^{t + 4\Delta t} $ 、$ u_{J - 5}^{t + 4\Delta t} $ 、$ u_{J - 4}^{t + 4\Delta t} $ 、$ u_{J - 6}^{t + 3\Delta t} $ 、$ u_{J - 5}^{t + 3\Delta t} $ 、$ u_{J - 4}^{t + 3\Delta t} $ 需已知,根据图2(b),假设t为0时刻,系统0时刻及前一时刻响应已知,通过式(5)将显式域$ t $ 时刻响应更新到($ t + \Delta t $ )时刻,那么显式域($ t + 2\Delta t $ )时刻响应可由($ t + \Delta t $ )、$ t $ 时刻求得,按此递推方法,($ t + 3\Delta t $ )和($ t + 4\Delta t $ )时刻系统响应可分别由各自时刻的前2个时刻推得,最终可求得显式域($ t + 5\Delta t $ )时刻系统响应。首先将显-隐式单元层分配给显式,显式先集成分区,通过J−5更新隐式边界点;然后将隐式单元层利用显式的输入作为边界条件,通过式(10)求解,并将隐式域节点J−4到J节点的信息传输至显式域,因显式域是根据系统2个时刻响应得到下一时刻响应,所以隐式域需连续计算2个时刻,同时隐式域计算每个时刻节点J−4到J节点的信息均需传输至显式域,以保证显式域节点J−4到J节点的2个时刻系统响应已知,作为显式域的初始条件计算显式域的系统响应。重复以上步骤可获得整个系统的响应。基于PASSI的显-隐式单元层计算方法计算流程如下:
(1)设置人工边界,采用上文介绍的黏弹性边界;
(2)输入脉冲及地震波,采用式(8)等效节点力的方式输入;
(3)采用集中质量有限元法,由式(5)得到显式域土体节点位移;
(4)求得显-隐式交界点位移,由式(5)得到交界点(p+1)时刻位移
$ \left\{ {U_{Jp + 1}^E} \right\} $ ,并作为隐式结构子系统的边界位移输入;(5)获得结构子系统的边界条件,通过Fortran程序调用ANSYS软件进行动力时程分析,采用Newmark隐式计算方法,由式(10)得到隐式节点(p+1)时刻的位移,并将其更新到显式域节点上;
(6)重复执行步骤(1)~(5),得到系统任意时刻的响应。
2. 对比验证
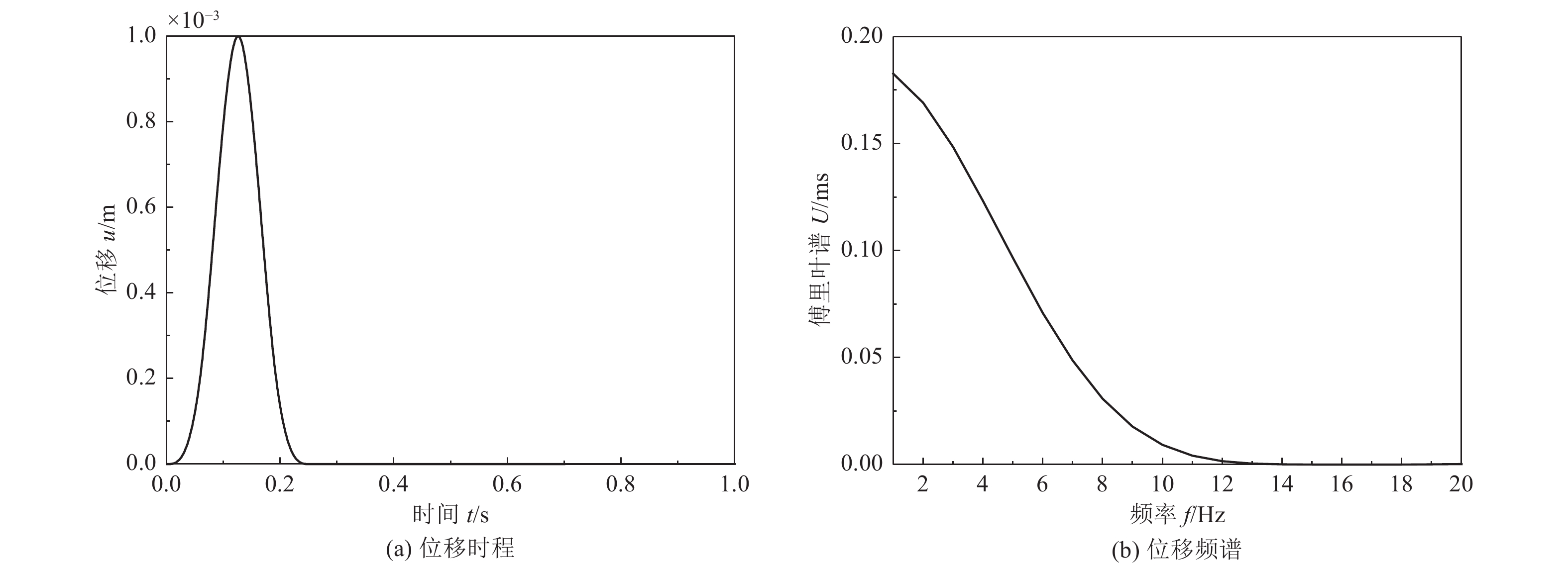
为验证上述方法的有效性,设计了场地分析和土-结构相互作用分析算例,分别采用本文显-隐式交互算法和ABAQUS软件进行分析。在土体模型底边界垂直入射单位脉冲位移波,其中脉冲宽度为0.25 s,时间步距为0.001 s,计算步数为1 000,计算时长为1.0 s,脉冲波位移时程及频谱如图3所示。
2.1 场地分析算例
首先从三维半无限空间中截取
$40 \; {\rm{m}}\; { \times }\; 40\; {\rm{m}}\; { \times }\; 50 \;{\rm{m}}$ 的有限土体,顶部自由,采用$1\; {\rm{m}}\; { \times }\; 1\; {\rm{m}}\; {\times }\; 1 \;{\rm{m}}$ 的单元离散,在其底部及4个侧面施加黏弹性人工边界,设置1层土,土体材料参数如表1所示,场地监测点如图4(b)所示分别取场地中心的顶部点a、中部点b和底部点c。表 1 土体参数Table 1. Soil parameters材料 厚度/m 弹性模量/GPa 泊松比 密度/kg·m−3 剪切波速/m·s−1 压缩波速/m·s−1 软土 50 0.108 0.35 1 000 200 416 结合ABAQUS软件二次开发功能(曹金凤等,2011;苏景鹤等,2016),采用前文阐述的黏弹性人工边界理论,在ABAQUS软件中实现黏弹性人工边界(刘晶波等,2007;王飞等,2018),ABAQUS软件中施加等效弹簧阻尼系统如图4(a)所示,场地监测点如图4(b)所示。
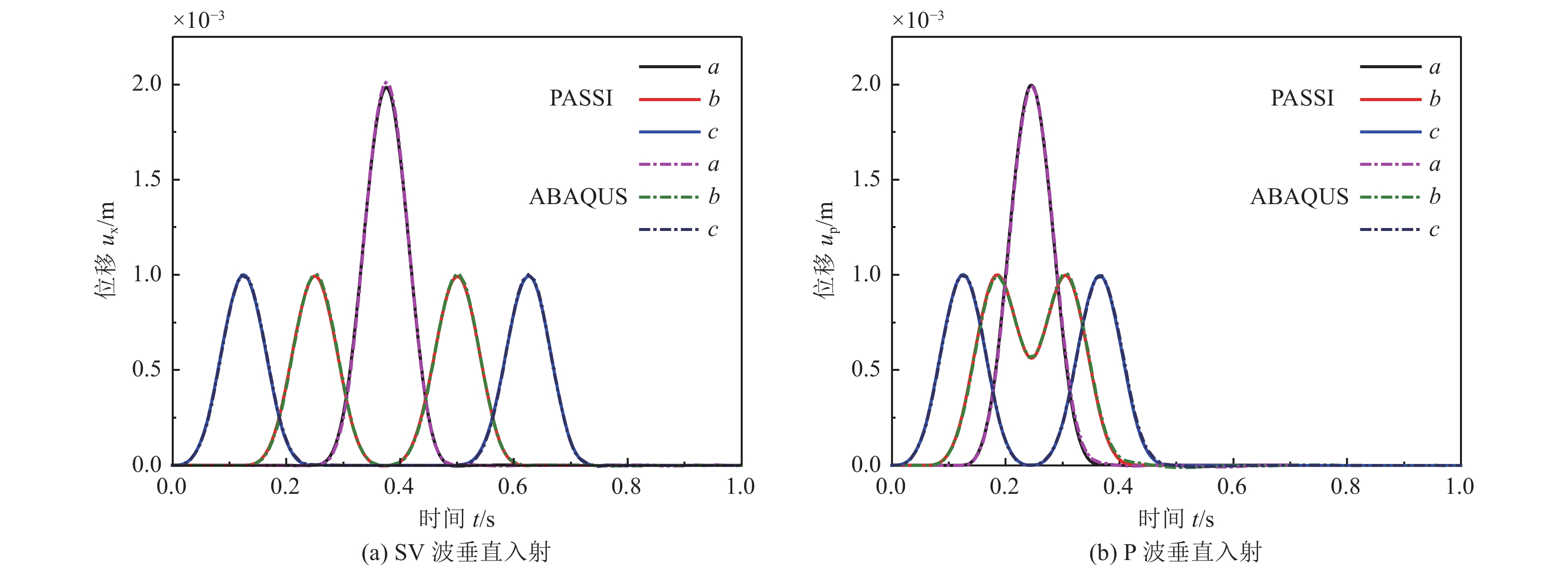
SV波和P波垂直入射场地位移时程响应如图5所示,实线为显-隐式单元层计算方法计算结果(Chen等,2022),虚线为ABAQUS软件计算结果。由图5可知,2种方法计算结果基本重合,在一定程度上检验了显-隐式单元层计算方法的准确性。
2.2 土-结构相互作用分析算例
结构模型采用
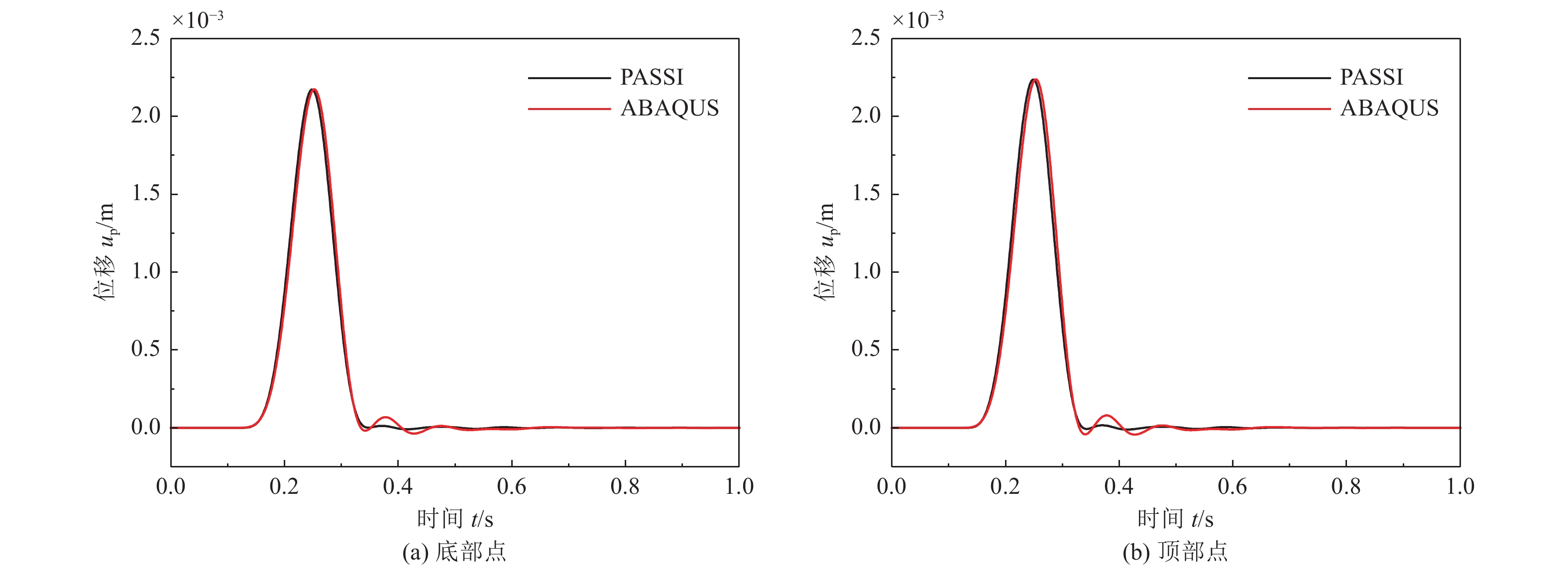
$1 \;{\rm{m}}\;{ \times }\; 1 \;{\rm{m}}\;{ \times }\; 10 \;{\rm{m}}$ 的结构柱,用$ 1\; {\rm{m}}\;{ \times }\; 1 \;{\rm{m}}\;{ \times }\; 1 \;{\rm{m}} $ 的实体单元进行离散,显-隐式单元层计算方法分为两部分,一部分为土体计算,另一部分为结构计算,考虑到核电结构体量大、单元种类多等特点,采用Fortran程序调用ANSYS软件计算上部结构相应。土体模型采用前文所述材料参数和单元尺寸,输入同样的激励,土-结构相互作用模型如图6所示。结构底部和顶部点位移响应如图7所示,由图7可知,显-隐式单元层计算方法与ABAQUS软件计算得到的结构受迫振动阶段结果基本相符,但结构自由振动阶段的计算结果有所差异,主要因为2种方法在阻尼设置上不同。
2.3 计算效率分析
本文算例分析在台式机上完成,采用Windows 10专业版操作系统,计算机处理器为Intel(R) Core(TM)i7-9700 CPU @3.00 Hz 3.00 GHz,内存16.0 GB,硬盘500 GB,计算效率如表2所示。
表 2 显-隐式单元层计算方法和ABAQUS软件计算效率Table 2. PASSI and ABAQUS calculation efficiency算例 单元数/个 节点数/个 自由度数/个 显-隐式单元层计算方法
计算时间/minABAQUS软件
计算时间/min场地 80 000 85 731 257 193 4 106 土-结构相互作用 80 010 85 771 257 313 33 106 注:自由度数=节点数*3(每个节点自由度个数) 由表2可知,在仅计算场地模型时,ABAQSU软件计算用时为显-隐式单元层计算方法的25倍左右。当考虑土-结构相互作用时,ABAQUS软件计算用时为显-隐式单元层计算方法的3倍左右。对于本文简单的上部结构模型,其计算量可忽略不计,相比于场地模型,ABAQSU软件计算时间基本不变,而显-隐式单元层计算方法每时步需调用ANSYS软件,需重启动,导致计算效率降低。对于简单的上部结构,由于显-隐式单元层计算方法重启动时间大于结构分析时间,因此显-隐式单元层计算方法计算效率相对于ABAQUS软件计算效率提高不明显,但对于大型复杂的核电结构,显-隐式单元层计算方法重启动时间远小于结构分析时间,效率提高更明显。
综上所述,基于PASSI的显-隐式单元层计算方法与ABAQUS软件计算精度相当,但计算效率较ABAQUS软件高。因此,采用显-隐式单元层计算方法进行辅助厂房对反应堆厂房的地震响应影响分析。
3. 地震波作用下相邻厂房对反应堆厂房的影响
3.1 模型建立
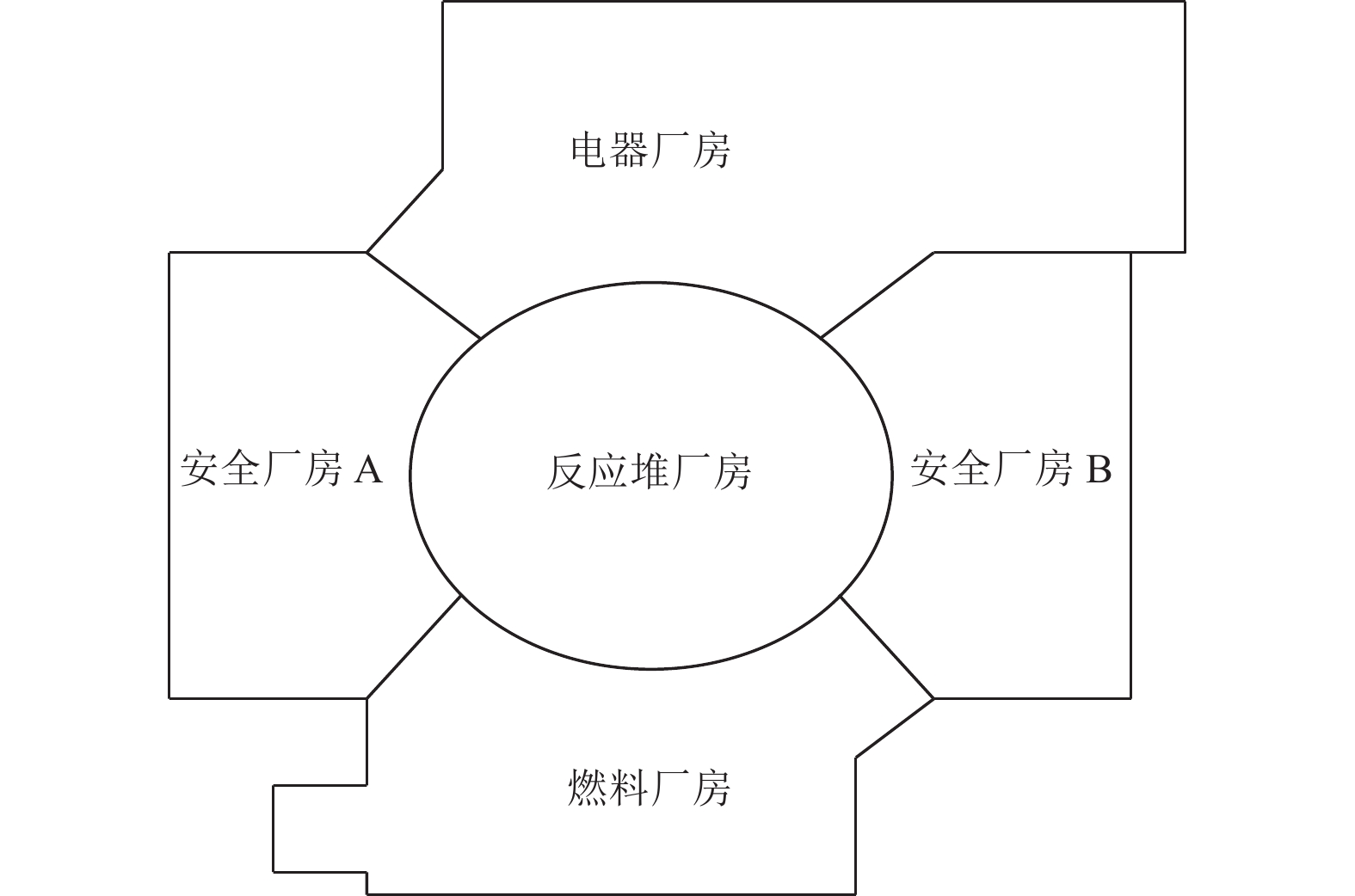
华龙一号核电站模型由反应堆厂房、燃料厂房、电器厂房和安全厂房组成(图8),4个辅助厂房呈四面环绕反应堆厂房对称布置,其中反应堆厂房设置了双层安全壳,外层安全壳、电器厂房、燃料厂房连成一体,中间不设缝。安全厂房与外层安全壳不连接,辅助厂房各自独立,所有厂房共用同块混凝土基础底板。
反应堆厂房模型如图9所示,反应堆厂房监测点分别取外壳顶部点a、内壳顶部点b、外壳中部点c、内壳中部点d、反应堆底板点f、反应堆上部点e。
核电站抗震计算模型采用三维有限元模型,需根据结构形式和受力特点,选择适当的单元进行模拟。墙体和楼板采用板壳单元模拟,板壳单元节点位于墙体和楼板厚度中心处;底板由于厚度较大,采用实体单元模拟,材料参数如表3所示。
表 3 核电站结构材料参数Table 3. Material parameters of nuclear power plant编号 材料 结构 弹性模量/GPa 泊松比 密度/kg·m−3 1 C30 厂房/基础 32.5 0.2 2 400 2 C40 内/外壳 36.0 0.2 2 450 x轴正向为反应堆厂房指向安全厂房方向,y轴正向为反应堆厂房指向电器厂房方向,z轴正向为竖直向上方向,坐标轴原点为反应堆厂房中心。
地基为弹性半空间,材料参数按表4所示的地质勘察资料取值,场地范围按规范要求(American Society of Civil Engineers(ASCE),2016;《核电厂抗震设计标准》(GB 50267—2019)(中华人民共和国住房和城乡建设部等,2019))选择
$340 \;{\rm{m}}\;{ \times }\; 400 \;{\rm{m}}\;{ \times }\;60 \;{\rm{m}}$ ,将计算区域离散为六面体八节点实体单元,单元尺寸为$2 \;{\rm{m}}\;{ \times } \;2 \; {\rm{m}}\;{ \times }\;2 \;{\rm{m}}$ ,在其底部及4个侧面边界施加黏弹性人工边界。表 4 核电站厂址参数Table 4. Soil parameters of nuclear power plant site材料 厚度/m 弹性模量/GPa 泊松比 密度/kg·m−3 剪切波速/m·s−1 压缩波速/m·s−1 岩石 60 46.9 0.26 2 650 2 673 4 639 将控制点处(地基表面)地震动幅值调为0.2 g,根据RG160规范谱人工合成的加速度时程如图10(a)所示,持续时间20.48 s,其反应谱如图10(b)所示。从PEER强震数据库中选出RSN5806_IWATE_55461NS(简称RSN5806)、RSN4872_CHUETSU_65053NS(简称RSN4872)地震波,用于RG160地震动输入结构响应规律验证,RSN5806加速度时程如图10(c)所示,持续时间60.00 s,其反应谱如图10(d)所示。RSN4872加速度时程如图10(e)所示,持续时间60.00 s,其反应谱如图10(f)所示。假定地震波垂直入射,由控制点处的地震动,按传递矩阵方法反演得到基岩半空间的入射波,并得到边界处的自由场,按式(8)得到人工边界点等效地震作用。
3.2 考虑相邻结构的工况模型
为考察相邻附属厂房对反应堆厂房地震响应的影响,考虑以下4种核电站结构模型:不考虑相邻厂房的反应堆厂房有限元模型,为Case1,如图11(a)所示;安全厂房A-反应堆厂房-安全厂房B有限元模型,为Case2,如图11(b)所示;燃料厂房-反应堆厂房-电器厂房有限元模型,为Case3,如图11(c)所示;核电站整体有限元模型,为Case4,如图11(d)所示。
对各工况进行模态分析,结果如表5所示。由表5可知,Case1和Case2模型前2阶模态频率相同,由于Case2模型中安全厂房与反应堆厂房分离,说明Case2模型前2阶模态是反应堆厂房的模态,整体而言,反应堆厂房的局部模态频率小于安全厂房。由于燃料厂房、电器厂房与反应堆厂房互相连为整体,增强了结构整体刚度,使Case3模型前35阶自振频率整体上大于Case1模型,且Case3模型第1阶自振频率最大。Case2模型第3~5阶频率基本与Case4模型第1~3阶频率相同,这3阶频率是安全厂房的自振频率。Case3模型第1~3阶频率基本与Case4模型第4~6阶频率相同,这是反应堆厂房、燃料厂房和电器厂房作为整体结构的前3阶频率,Case4模型前3阶频率基本与Case2模型第3~5阶频率相同,这3阶频率是安全厂房的自振频率,经燃料厂房和电器厂房加强后,反应堆厂房局部模态频率大于安全厂房频率。Case1和Case2模型第2阶振型参与系数最大,对应的频率均为3.007 Hz左右;Case3模型第1阶振型参与系数最大,Case4模型第4阶振型参与系数最大,对应的频率均为4.017 Hz左右。4种工况中参与系数最大的振型均与反应堆厂房局部振型相关,主要反映反应堆厂房的自振特性。
表 5 模态分析Table 5. Modality analysis振型 Case1 Case2 Case3 Case4 频率/Hz 振型参与系数 频率/Hz 振型参与系数 频率/Hz 振型参与系数 频率/Hz 振型参与系数 1 3.006 69 0.186 26 3.006 69 0.186 19 4.016 96 1.000 00 3.726 48 0.426 64 2 3.007 49 1.000 00 3.007 49 1.000 00 4.068 74 0.277 89 3.861 54 0.413 38 3 4.078 57 0.540 83 3.725 20 0.677 04 4.114 50 0.094 62 3.885 43 0.002 48 4 4.096 08 0.381 57 3.858 00 0.683 88 4.210 24 0.482 59 4.017 18 1.000 00 5 5.488 44 0.002 04 3.885 28 0.009 75 4.739 49 0.029 30 4.068 75 0.275 37 6 5.513 01 0.001 43 4.078 57 0.540 69 5.472 46 0.169 67 4.114 53 0.097 12 7 5.540 46 0.003 31 4.096 08 0.381 50 5.566 56 0.002 17 4.210 38 0.480 55 8 5.572 03 0.004 84 4.559 21 0.068 57 5.610 47 0.012 11 4.560 35 0.045 69 9 5.629 19 0.003 16 5.439 43 0.064 35 5.762 07 0.080 10 4.739 62 0.029 71 10 5.658 22 0.010 71 5.488 44 0.002 05 6.163 02 0.037 35 5.441 09 0.042 47 11 6.426 34 0.004 54 5.513 01 0.001 43 6.470 85 0.006 88 5.472 66 0.167 66 12 6.471 34 0.015 75 5.540 46 0.003 31 6.496 26 0.389 72 5.566 56 0.002 12 13 6.496 20 0.598 36 5.572 03 0.004 82 6.615 10 0.003 04 5.610 47 0.011 96 14 6.614 21 0.001 58 5.629 19 0.003 17 6.875 02 0.134 96 5.702 48 0.044 00 15 6.875 13 0.207 35 5.658 22 0.010 72 7.121 85 0.000 71 5.762 18 0.079 50 16 7.117 11 0.000 87 5.698 29 0.070 07 7.183 56 0.005 66 6.163 16 0.037 35 17 7.171 63 0.005 92 6.426 34 0.004 55 7.186 37 0.071 54 6.470 85 0.006 79 18 7.186 59 0.070 52 6.471 34 0.015 75 7.228 46 0.359 01 6.496 26 0.386 15 19 7.218 46 0.014 67 6.496 20 0.598 31 7.349 15 0.546 56 6.615 10 0.003 03 20 7.235 54 0.000 05 6.614 21 0.001 59 7.778 06 0.042 94 6.875 02 0.133 73 21 7.778 05 0.071 15 6.875 13 0.207 34 7.984 62 0.026 00 7.121 85 0.000 71 22 7.984 60 0.042 40 7.117 11 0.000 87 8.112 55 0.020 30 7.183 56 0.005 58 23 8.112 54 0.033 21 7.171 63 0.005 93 8.241 69 0.004 93 7.186 37 0.070 85 24 8.241 67 0.008 19 7.186 59 0.070 51 8.355 48 0.012 78 7.228 55 0.356 06 25 8.355 47 0.020 32 7.218 46 0.014 67 8.603 98 0.003 84 7.349 13 0.541 36 26 8.457 00 0.005 00 7.235 54 0.000 04 8.879 18 0.000 60 7.778 06 0.042 54 27 8.603 97 0.006 27 7.778 05 0.071 15 8.980 48 0.011 40 7.984 62 0.025 76 28 8.977 70 0.014 41 7.984 60 0.042 39 9.092 81 0.031 82 8.112 55 0.020 11 29 9.092 80 0.051 76 8.112 54 0.033 21 9.161 85 0.066 65 8.241 69 0.004 88 30 9.229 79 0.118 33 8.241 67 0.008 19 9.229 87 0.071 67 8.355 48 0.012 67 31 9.414 48 0.015 02 8.355 47 0.020 32 9.414 48 0.009 21 8.603 98 0.003 80 32 9.518 82 0.077 79 8.457 00 0.005 04 9.450 98 0.026 21 8.879 18 0.000 58 33 9.698 92 0.086 25 8.603 97 0.006 27 9.518 87 0.045 52 8.980 48 0.011 29 34 9.776 22 0.456 36 8.977 70 0.014 42 9.787 15 0.014 03 9.092 81 0.031 53 35 9.841 21 0.024 00 9.092 80 0.051 76 9.841 17 0.020 96 9.161 81 0.065 98 3.3 结果分析
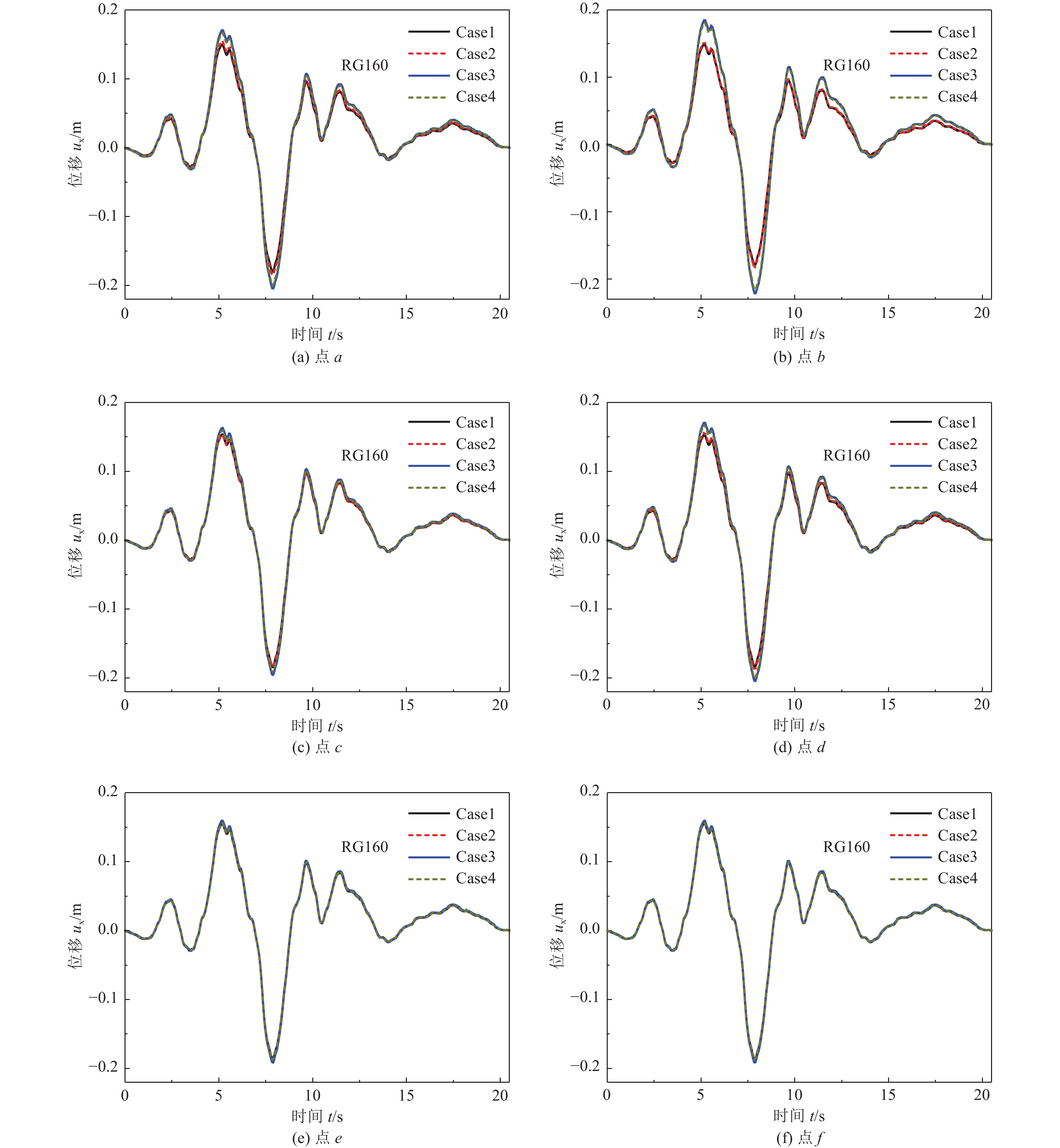
3条地震动输入下反应堆厂房位移响应规律基本一致,因此仅给出RG160地震动输下的反应堆厂房监测点位移响应,如图12所示。由图12可知,Case1和Case2模型对应的反应堆厂房位移响应较接近,Case3和Case4模型对应的反应堆厂房位移响应较接近,且响应较大。对于反应堆底板点f和上部点e,4种工况下的位移基本一致;对于外壳中部点c、内壳中部点d及外壳顶部点a、内壳顶部点b,Case3和Case4模型对应的反应堆厂房位移响应较Case1和Case2模型大,且顶部点位移差异较中部点更明显。综上所述,燃料厂房和电器厂房与外壳连接时,对反应堆厂房位移响应有影响,对反应堆底部和中部位移的影响较小,但对壳顶部位移的影响较大;安全厂房与反应堆厂房分离时,对反应堆厂房位移响应的影响较小。
RG160地震动输入下4种工况反应堆厂房监测点加速度反应谱如图13所示。由图13可知,Case1和Case2模型对应的反应堆厂房加速度反应谱较接近,说明安全厂房对反应堆厂房加速度反应谱的影响较小;Case3和Case4模型对应的反应堆厂房加速度反应谱基本一致,且与Case1有差异,说明燃料厂房和电器厂房对反应堆厂房加速度反应谱有影响。对于反应堆底板点f和上部点e,4种工况下的反应堆厂房加速度反应谱基本一致,但安全壳(测点a~d)加速度反应谱有所差异,即燃料厂房和电器厂房对反应堆底部和中部加速度反应谱的影响较小,对反应堆厂房安全壳加速度反应谱有明显影响。当频率>10 Hz时,4种工况下测点a~d加速度反应谱基本一致;当频率为6~10 Hz时,Case3和Case4模型对应的测点a~d加速度响应大于Case1和Case2模型;当频率为2~6 Hz时,Case3和Case4模型对应的加速度响应小于Case1和Case2模型;当频率<2 Hz时,Case3和Case4模型对应的加速度响应大于Case1和Case2模型,即燃料厂房和电器厂房的存在增大了反应堆厂房2 Hz以下的低频响应,因此增大了位移响应,如图13所示。
RSN5806和RSN4872地震动输入下4种工况反应堆厂房监测点加速度反应谱如图14所示,图中实线为RSN5806地震动输入下反应堆厂房加速度反应谱,虚线为RSN4872地震动输入下反应堆厂房加速度反应谱。由图14可知,在RSN5806和RSN4872地震动输入下,各工况对应的反应堆厂房加速度响应规律与RG160地震动输入时基本一致,即Case1和Case2模型对应的反应堆厂房加速度反应谱较接近,Case3和Case4模型对应的加速度反应谱基本一致,4中工况下应堆底板点f和上部点e加速度反应谱基本一致。
4. 结语
本文基于PASSI算法,提出显-隐式单元层计算方法,实现显、隐式交替计算。通过场地分析算例和土-结构相互作用分析算例,与ABAQUS软件计算结果进行对比,验证计算方法的可行性。以某核电站为研究对象,分析了相邻厂房对反应堆厂房地震响应的影响,得到以下主要结论:
(1)显-隐式单元层计算方法较PASSI算法稳定性好,但增加了重叠区计算量。
(2)安全厂房与反应堆厂房共用基础,但不连接,结构整体模态和自振频率为独立厂房模态和自振频率的集合。在基岩场地上,安全厂房通过基础和场地对反应堆厂房的作用较小,对反应堆厂房地震响应的影响较小。
(3)燃料厂房、电器厂房与反应堆厂房共用基础,并与反应堆厂房相连,增加了反应堆厂房刚度,作为整体结构时,与反应堆厂房相关的自振频率大于单独的反应堆厂房。在本文地震动输入下,燃料厂房和电器厂房加大了反应堆厂房位移峰值,减小了反应堆厂房加速度反应谱峰值,并使反应堆厂房顶部点加速度反应谱峰值向高频移动。
(4)对于与反应堆厂房共用同一基础的辅助厂房,应与反应堆厂房作为整体进行地震响应分析,至少应将与反应堆厂房相连的辅助厂房作为整体进行分析。
对于非基岩场地,结构-土-结构相互作用可能更明显,其对反应堆厂房地震响应的影响与输入地震动特性等有关,需另外讨论。
-
表 1 数值模型信息
Table 1. Numerical model information
建筑构件 单元类型 构件之间的相互作用 材料属性 悬挑梁 C3D8R Tie 设计规范混凝土本构模型 现浇楼板 C3D8R Tie 设计规范混凝土本构模型 钢筋 T3D2 Embedded region 双直线理想弹塑性模型 墙体 C3D8R Tie 整体式砌体结构本构模型 表 2 模型前2阶自振周期
Table 2. The first 2 vibration cycles of the model
振型 自振周期/s 振型特征 一阶 0.141 横向平动 二阶 0.115 纵向平动 表 3 结构参数及其分布
Table 3. Structural model parameters and their distribution
参数 平均值 变异系数 分布类型 砌体材料参数 阻尼比 0.05 0.3 正态 弹性模量/MPa 1 350 0.15 正态 抗压强度/kPa 2 448.29 0.17 对数正态 抗拉强度/kPa 235.938 0.2 对数正态 密度/(kg·m−3) 1 600 0.1 正态 混凝土材料参数 密度/(kg·m−3) 2 400 0.07 正态 结构几何参数 层高/m 3.3 0.05 — 墙厚/mm 240 0.05 — 表 4 输入参数及标准化
Table 4. Input parameters and standardization
输入参数 下限值 平均值 上限值 砌体阻尼比 输入值 0.035 0.05 0.065 标准化 −1 0 1 砌体弹性模量/MPa 输入值 1 147.5 1 350.0 1 552.5 标准化 −1 0 1 砌体抗压强度/kPa 输入值 2 032.08 2 448.29 2 864.50 标准化 −1 0 1 砌体抗拉强度/kPa 输入值 188.750 235.938 283.126 标准化 −1 0 1 砌体材料密度/(kg·m−3) 输入值 1 440 1 600 1 760 标准化 −1 0 1 混凝土密度/(kg·m−3) 输入值 2 328 2 400 2 568 标准化 −1 0 1 层高/m 输入值 3.135 3.300 3.465 标准化 −1 0 1 墙厚/mm 输入值 228 240 252 标准化 −1 0 1 表 5 Plackett-Burman试验设计
Table 5. A case of Plackett-Burman test design
项目 序号 1 2 3 4 5 6 7 8 9 10 11 12 砌体阻尼比 −1 1 −1 −1 1 1 −1 1 −1 −1 1 1 砌体密度 −1 −1 1 −1 −1 1 −1 1 1 1 1 −1 混凝土密度 −1 −1 1 1 1 1 −1 −1 1 −1 −1 1 砌体抗拉强度 −1 1 1 1 −1 1 1 −1 −1 −1 1 −1 砌体弹性模量 −1 1 −1 −1 1 1 1 −1 1 1 −1 −1 墙厚 −1 1 1 −1 −1 −1 1 1 1 −1 −1 1 层高 −1 −1 1 −1 1 −1 1 −1 −1 1 1 1 砌体抗压强度 −1 −1 −1 1 −1 1 1 1 −1 1 −1 1 表 6 中心复合试验设计
Table 6. A case of central composite test design
运行序 砌体弹性模量 砌体密度 砌体抗拉强度 1 1 1 −1 2 −1 −1 −1 3 1 0 0 4 0 1 0 5 1 −1 −1 6 −1 1 −1 7 0 0 0 8 1 1 1 9 −1 −1 1 10 −1 1 1 11 −1 0 0 12 1 −1 1 13 0 −1 0 14 0 0 1 15 0 0 −1 表 7 中心复合设计输出结果
Table 7. Output results from the central composite design
运行序 最大层间位移角(×10−7) 0.05 g 0.1 g 0.15 g ... 0.35 g 0.4 g $ {\hat y_{u1}} $ $ {\hat y_{\sigma 1}} $ $ {\hat y_{u2}} $ $ {\hat y_{u2}} $ $ {\hat y_{u3}} $ $ {\hat y_{\sigma 3}} $ ... ... $ {\hat y_{u7}} $ $ {\hat y_{\sigma 7}} $ $ {\hat y_{u8}} $ $ {\hat y_{\sigma 8}} $ 1 3 949.9 2 209.4 8 740.6 3 417.6 35 780 10 027 ... ... 29 832 7 952.2 35 779 1 002 2 2 998.8 1 969.57 7 020.4 2 665.2 28 183 6 577 ... ... 24 316 6 219.3 28 182 6 577.2 3 4 499.3 1 736.1 9 200.1 3733.5 39 739 12 563 ... ... 33 441 9 797.4 39 739 12 563 ... ... ... ... ... ... ... ... ... ... ... ... ... 15 4 235.6 1 558.1 8 759.3 2 412.7 39 003 12 186 ... ... 32 472 9 523.8 39 003 12 186 表 8 响应面模型检验结果
Table 8. Verification of response surface model
响应面标号 $ {R^2} $/% $ R_{\mathrm{A}}^2 $/% 1 99.59 98.31 2 98.61 97.52 3 98.36 97.62 4 98.76 97.32 5 97.79 96.80 6 98.26 97.82 7 97.12 96.32 8 95.30 93.25 表 9 极限状态限值
Table 9. Limitation value of limited state
极限状态 轻微破坏(LS1) 中等破坏(LS2) 严重破坏(LS3) 毁坏(LS4) $ {\theta _{\max }} $ 1/2 000 1/1 600 1/700 1/350 -
陈力波,黄才贵,谷音,2018. 基于改进响应面法的公路简支梁桥地震易损性分析. 工程力学,35(4):208−218. doi: 10.6052/j.issn.1000-4750.2017.06.0512Chen L. B., Huang C. G., Gu Y., 2018. Seismic vulnerability analysis of simply supported highway bridges based on an improved response surface method. Engineering Mechanics, 35(4): 208−218. (in Chinese) doi: 10.6052/j.issn.1000-4750.2017.06.0512 黄琼,2014. 基于响应面法的钢筋混凝土框架结构有限元模型修正研究. 兰州:兰州理工大学.Huang Q., 2014. Finite element model updating of reinforced concrete frame structure based on response surface method. Lanzhou:Lanzhou University of Technology. (in Chinese) 李龙师,2020. 松原查干花震区农村房屋抗震性能研究及震害预测. 哈尔滨:中国地震局工程力学研究所.Li L. S., 2020. Seismic Capacity Analysis and Earthquake damage prediction of rural houses in Chaganhua earthquake area of Songyuan City. Harbin:Institute of Engineering Mechanics,China Earthquake Administration. (in Chinese) 吕大刚,刘洋,于晓辉,2019. 第二代基于性能地震工程中的地震易损性模型及正逆概率风险分析. 工程力学,36(9):1−11,24.Lü D. G., Liu Y., Yu X. H., 2019. Seismic fragility models and forward-backward probabilistic risk analysis in second-generation performance-based earthquake engineering. Engineering Mechanics, 36(9): 1−11,24. (in Chinese) 宋帅,钱永久,钱聪,2018. 桥梁地震需求中随机参数的重要性分析方法研究. 工程力学,35(3):106−114. doi: 10.6052/j.issn.1000-4750.2016.11.0856Song S., Qian Y. J., Qian C., 2018. Research on methods for importance analysis of random parameter in bridge seismic demand. Engineering Mechanics, 35(3): 106−114. (in Chinese) doi: 10.6052/j.issn.1000-4750.2016.11.0856 孙柏涛,张桂欣,2012. 汶川8.0级地震中各类建筑结构地震易损性统计分析. 土木工程学报,45(5):26−30.Sun B. T., Zhang G. X., 2012. Statistical analysis of the seismic vulnerability of various types of building structures in Wenchuan M 8.0 earthquake. China Civil Engineering Journal, 45(5): 26−30. (in Chinese) 熊立红,吴文博,孙悦,2012. 汶川地震作用下约束砌体房屋的抗震能力分析. 土木工程学报,45(S2):103−108.Xiong L. H., Wu W. B., Sun Y., 2012. Seismic performance of confined masonry buildings during the Wenchuan earthquake. China Civil Engineering Journal, 45(S2): 103−108. (in Chinese) 杨卫忠,2008. 砌体受压本构关系模型. 建筑结构,38(10):80−82.Yang W. Z., 2008. Constitutive relationship model for masonry materials in compression. Building Structure, 38(10): 80−82. (in Chinese) 杨玉成,杨柳,高云学等,1982. 现有多层砖房震害预测的方法及其可靠度. 地震工程与工程振动,2(3):75−86.Yang Y. C., Yang L., Gao Y. X., et al., 1982. Method of damage prediction for existing multi story brick buildings and its reliability. Earthquake Engineering and Engineering Vibration, 2(3): 75−86. (in Chinese) 叶继红,江力强,2021. 考虑多重不确定性的我国多层冷成型钢结构地震风险评估. 土木工程学报,54(2):74−83,126.Ye J. H., Jiang L. Q., 2021. Seismic risk assessment of mid-rise cold-formed steel structures in China considering various uncertainties. China Civil Engineering Journal, 54(2): 74−83,126. (in Chinese) 于晓辉,2012. 钢筋混凝土框架结构的概率地震易损性与风险分析. 哈尔滨:哈尔滨工业大学.Yu X. H., 2012. Probabilistic seismic fragility and risk analysis of reinforced concrete frame structures. Harbin:Harbin Institute of Technology. (in Chinese) 于晓辉,吕大刚,2012. 考虑结构不确定性的地震倒塌易损性分析. 建筑结构学报,33(10):8−14.Yu X. H., Lü D. G., 2012. Seismic collapse fragility analysis considering structural uncertainties. Journal of Building Structures, 33(10): 8−14. (in Chinese) 张令心,李孟达,刘洁平等,2018. 考虑结构不确定性的框剪结构地震易损性分析. 自然灾害学报,27(4):112−118.Zhang L. X., Li M. D., Liu J. P., et al., 2018. Seismic fragility analysis of frame-shear wall structures considering structural parameter uncertainty. Journal of Natural Disasters, 27(4): 112−118. (in Chinese) 张尚荣,谭平,杜永峰等,2014. 基于响应面法的层间隔震结构地震易损性分析. 振动与冲击,33(15):42−48.Zhang S. R., Tan P., Du Y. F., et al., 2014. Seismic fragility analysis of inter-story isolation structures based on response surface method. Journal of Vibration and Shock, 33(15): 42−48. (in Chinese) 周强,2012. 砌体结构抗震试验及弹塑性地震反应分析. 哈尔滨:哈尔滨工程大学.Zhou Q., 2012. Seismic test and elastic-plastic seismic response analysis of masonry structure. Harbin:Harbin Engineering University. (in Chinese) 周强,闵全环,熊拥军等,2018. 江西典型村镇既有房屋抗震性能分析. 自然灾害学报,27(1):96−105.Zhou Q., Min Q. H., Xiong Y. J., et al., 2018. Seismic performance analysis of existing typical rural buildings in Jiangxi. Journal of Natural Disasters, 27(1): 96−105. (in Chinese) 朱震宇,2015. 砌体墙抗震性能分析模型及其工程应用. 上海:上海交通大学.Zhu Z. Y., 2015. Analysis model of seismic behavior of masonry walls and its engineering application. Shanghai:Shanghai Jiao Tong University. (in Chinese) Choudhury T., Kaushik H. B., 2018. Seismic response sensitivity to uncertain variables in RC frames with infill walls. Journal of Structural Engineering, 144(10): 04018184. doi: 10.1061/(ASCE)ST.1943-541X.0002190 Demirel İ. O., 2010. A nonlinear equivalent frame model for displacement based analysis of unreinforced brick masonry buildings. Ankara: Middle East Technical University. FEMA, 2009. Quantification of building seismic performance factors. Washington: Federal Emergency Management Agency. Freeny A., 1988. Empirical model building and response surfaces. Technometrics, 30(2): 229−231. doi: 10.1080/00401706.1988.10488371 Gokkaya B. U., Baker J. W., Deierlein G. G., 2016. Quantifying the impacts of modeling uncertainties on the seismic drift demands and collapse risk of buildings with implications on seismic design checks. Earthquake Engineering & Structural Dynamics, 45(10): 1661−1683. Loukas Y. L., 2001. A Plackett-Burman screening design directs the efficient formulation of multicomponent DRV liposomes. Journal of Pharmaceutical and Biomedical Analysis, 26(2): 255−263. doi: 10.1016/S0731-7085(01)00419-8 Montgomery D. C., 2001. Design and analysis of experiments. 5th ed. New York: John Wiley & Sons, Inc. Parisi F., Augenti N., 2012. Uncertainty in seismic capacity of masonry buildings. Buildings, 2(3): 218−230. doi: 10.3390/buildings2030218 Towashiraporn P., 2004. Building seismic fragilities using response surface metamodels. Atlanta: Georgia Institute of Technology. 期刊类型引用(0)
其他类型引用(1)
-





 下载:
下载:
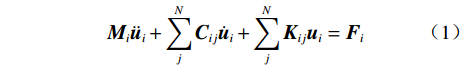
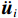
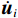
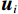
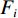
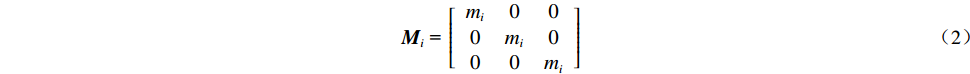
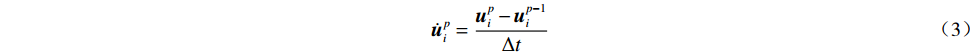
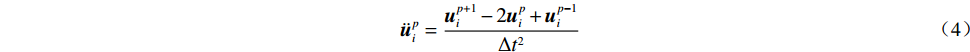
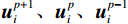
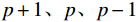
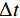
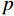
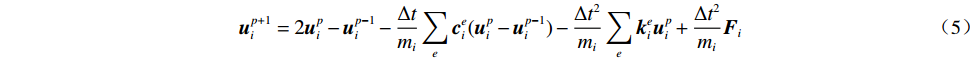
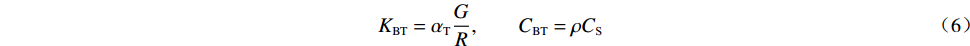
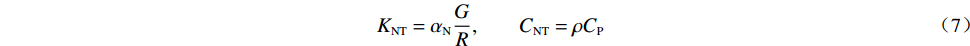
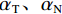
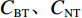
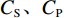
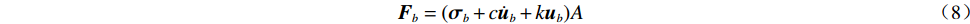

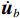
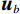
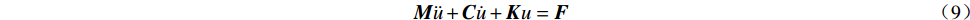
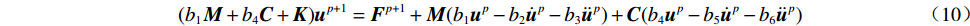
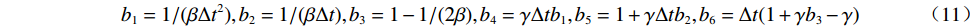


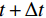

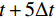
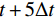
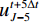
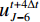
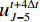
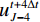
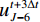
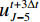
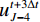


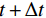
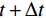
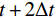
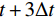
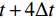
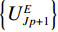

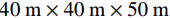
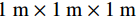



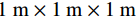




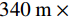







 下载:
下载: