Study on Seismic Response of Underground Structure in Saturated Soil Deposit Based on OpenSees
-
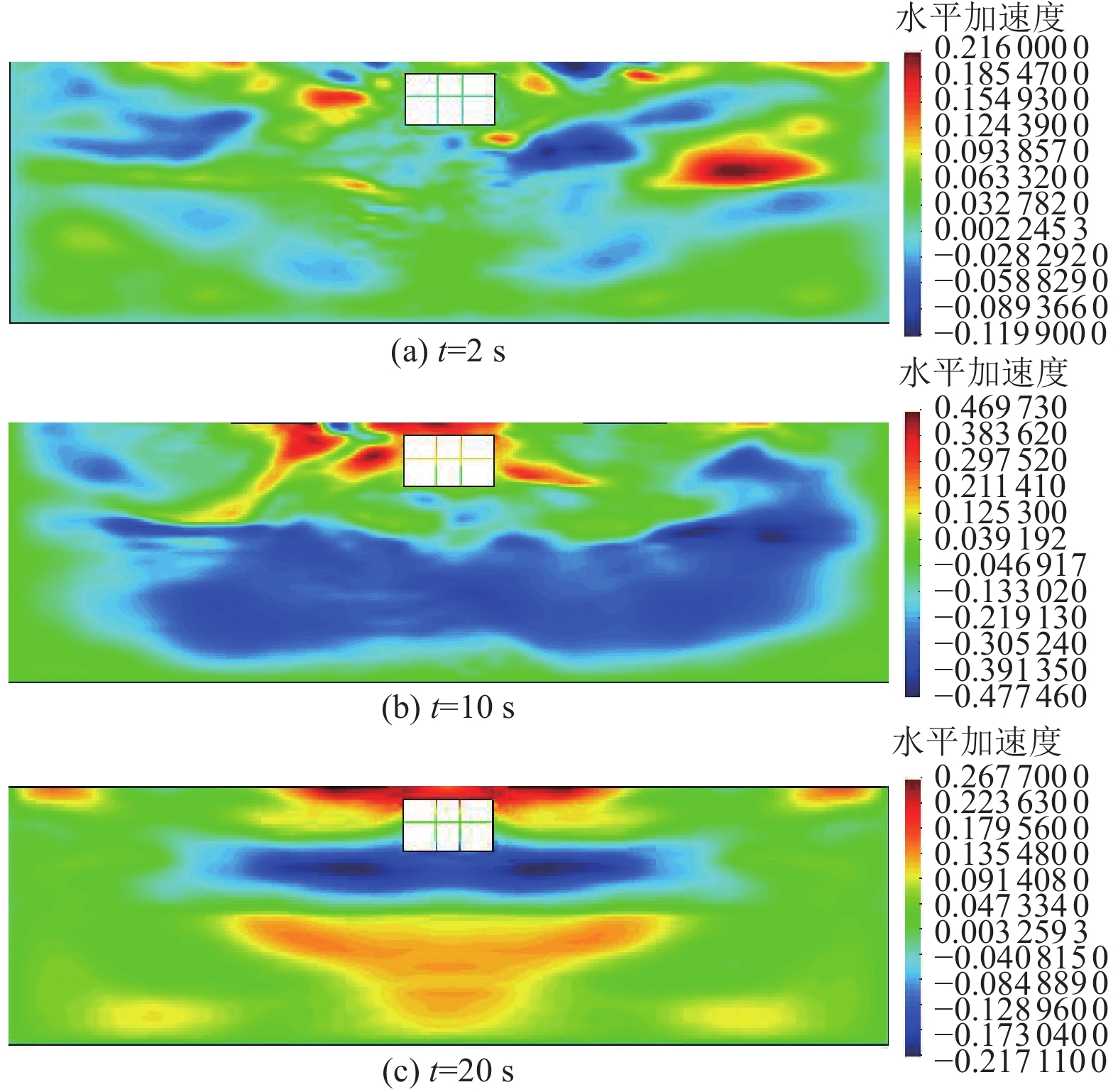
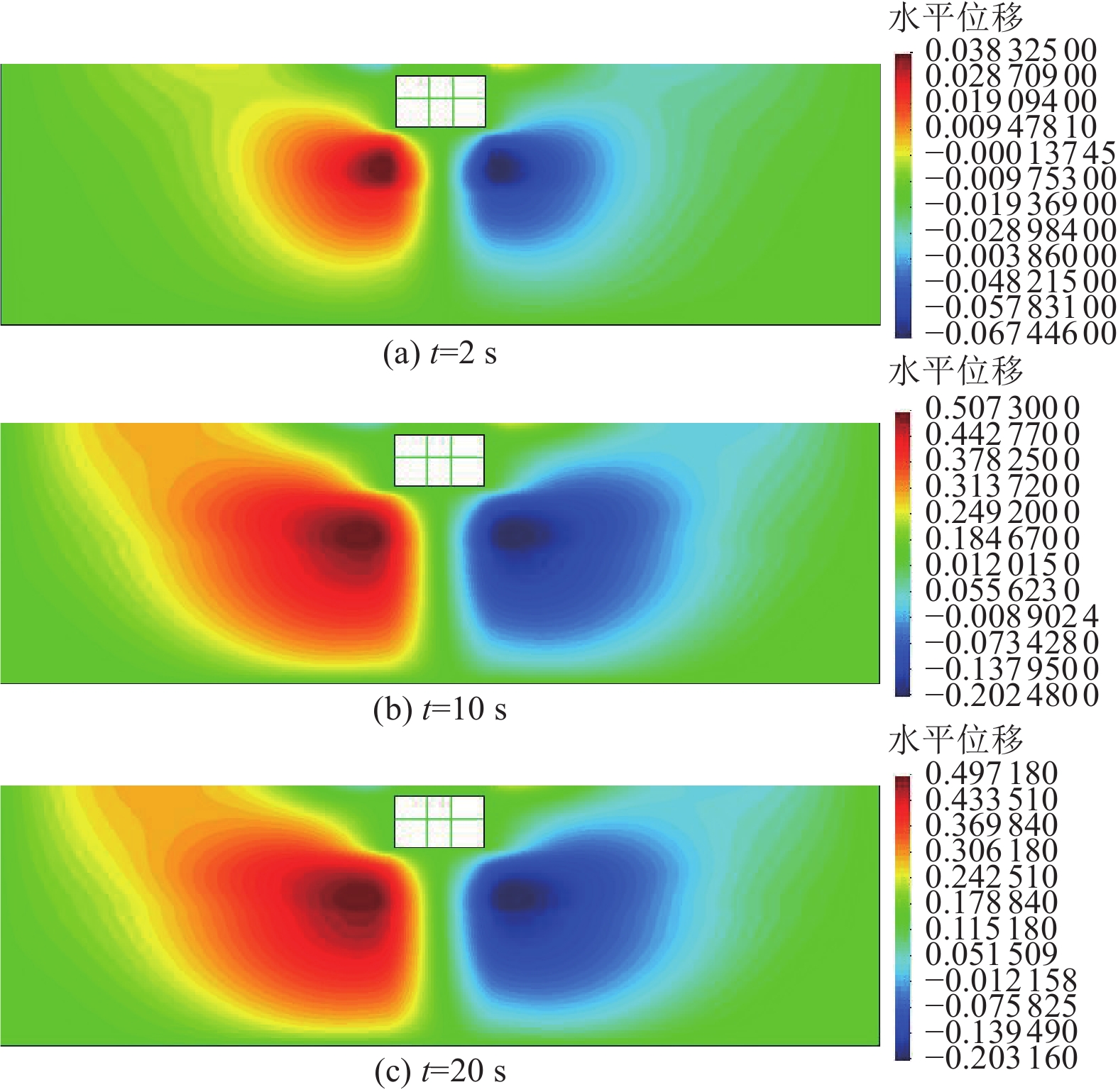
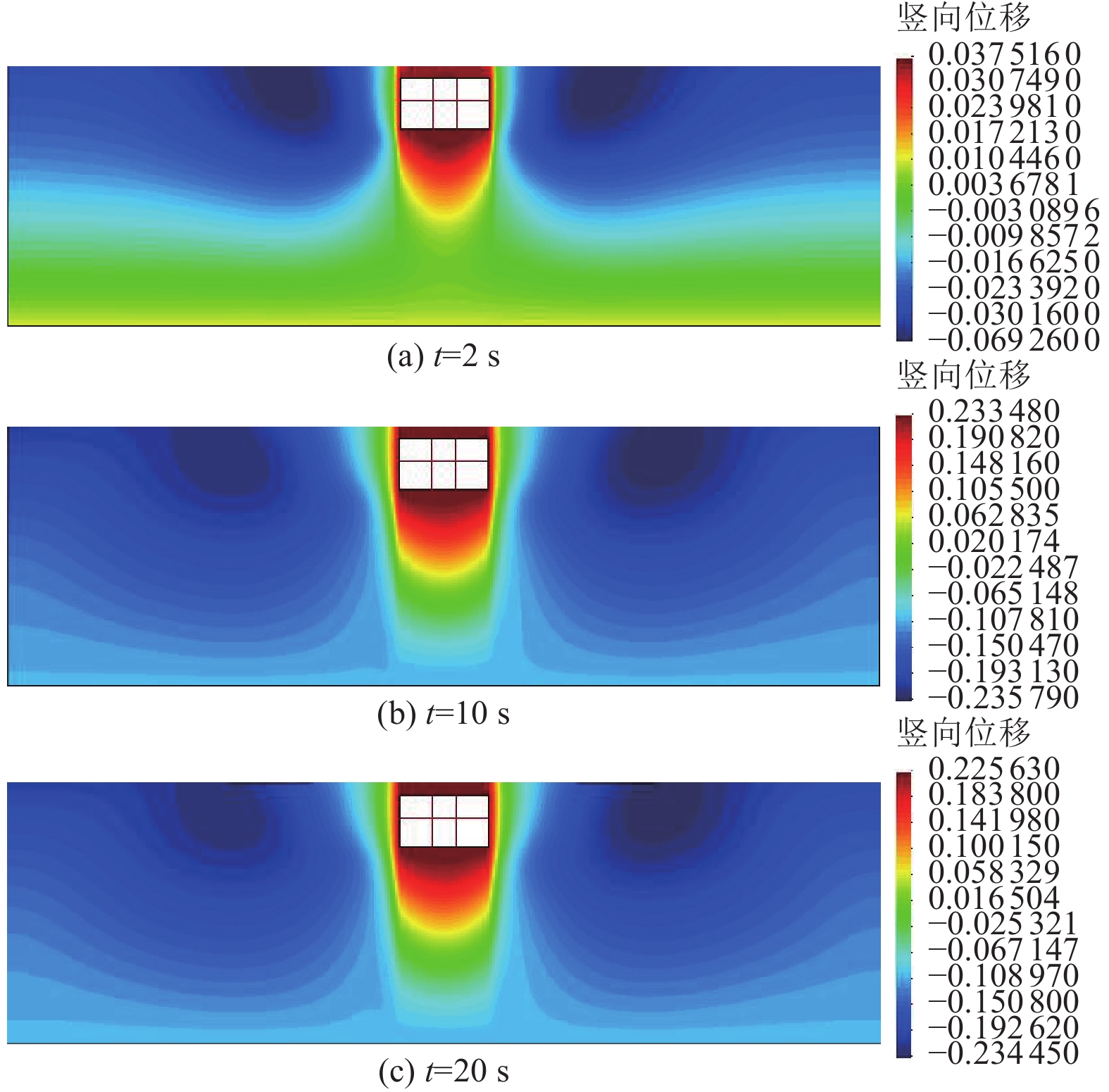
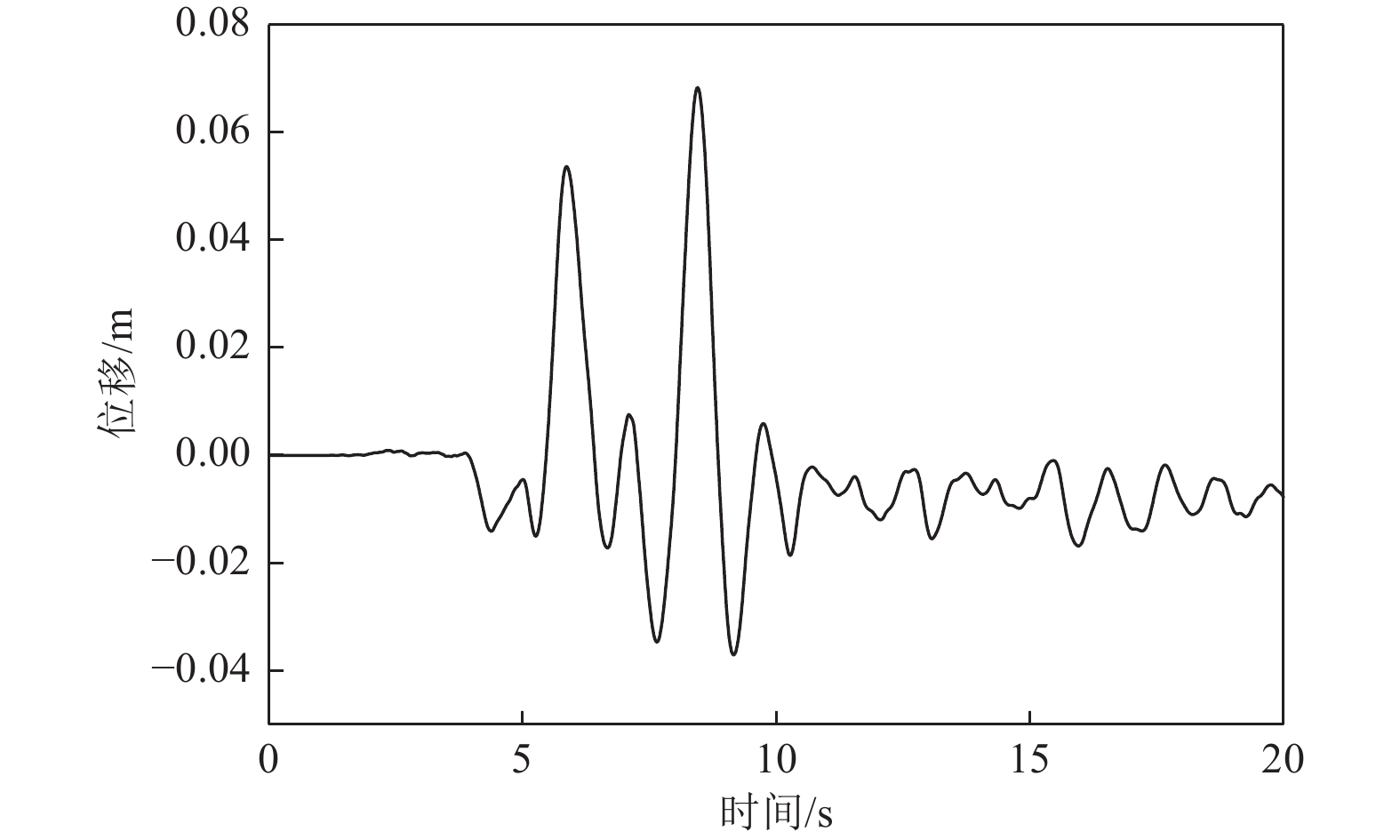
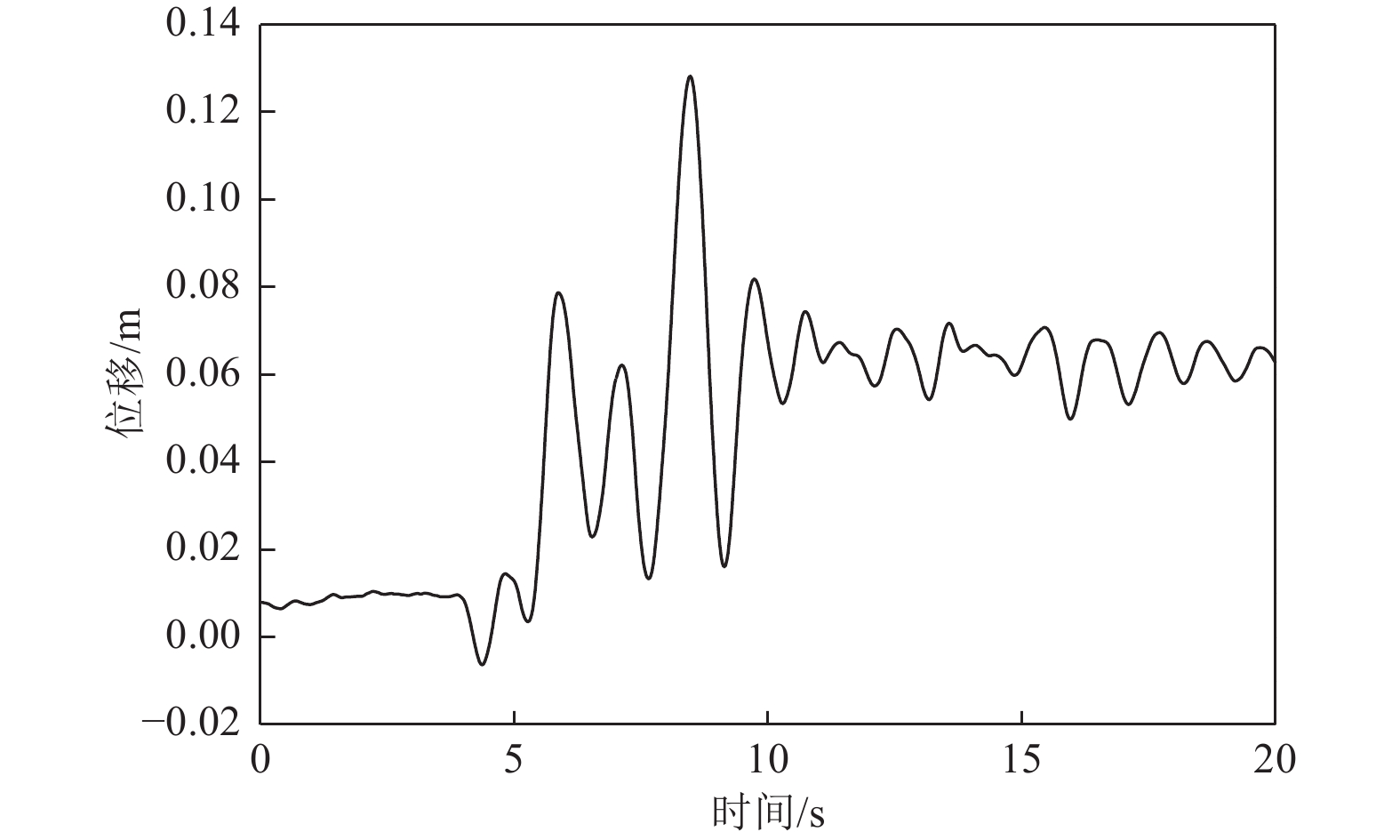
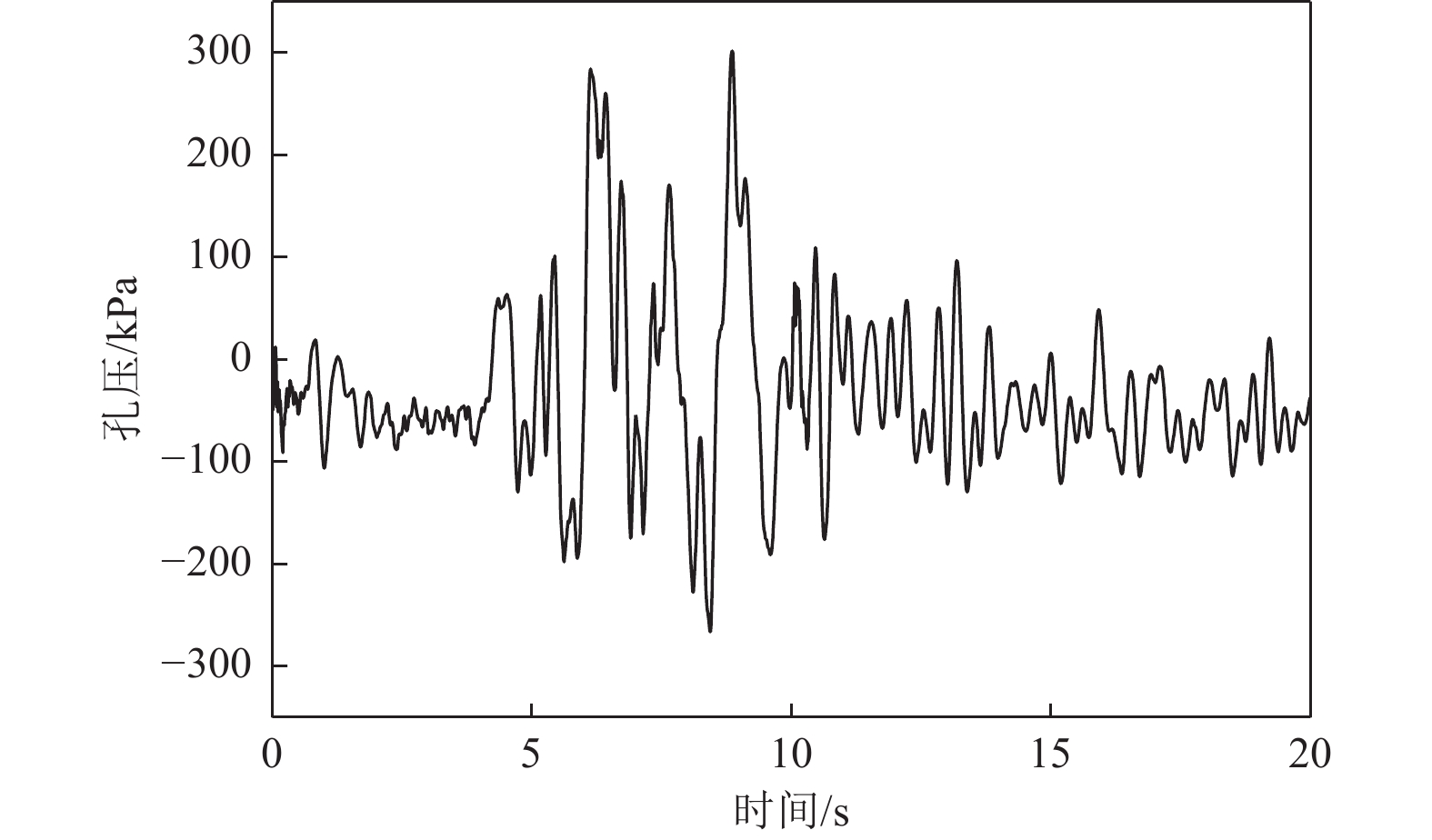
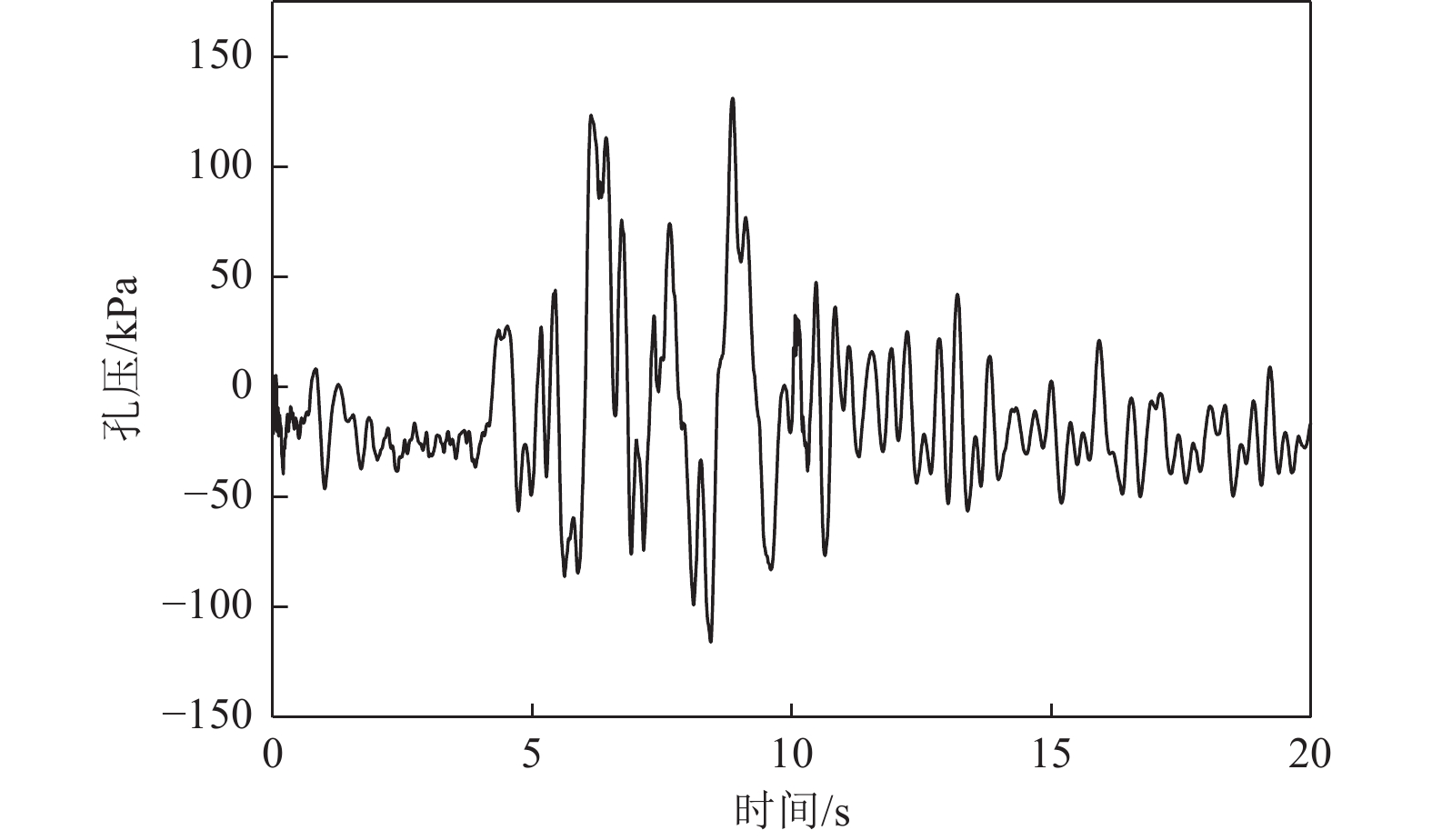
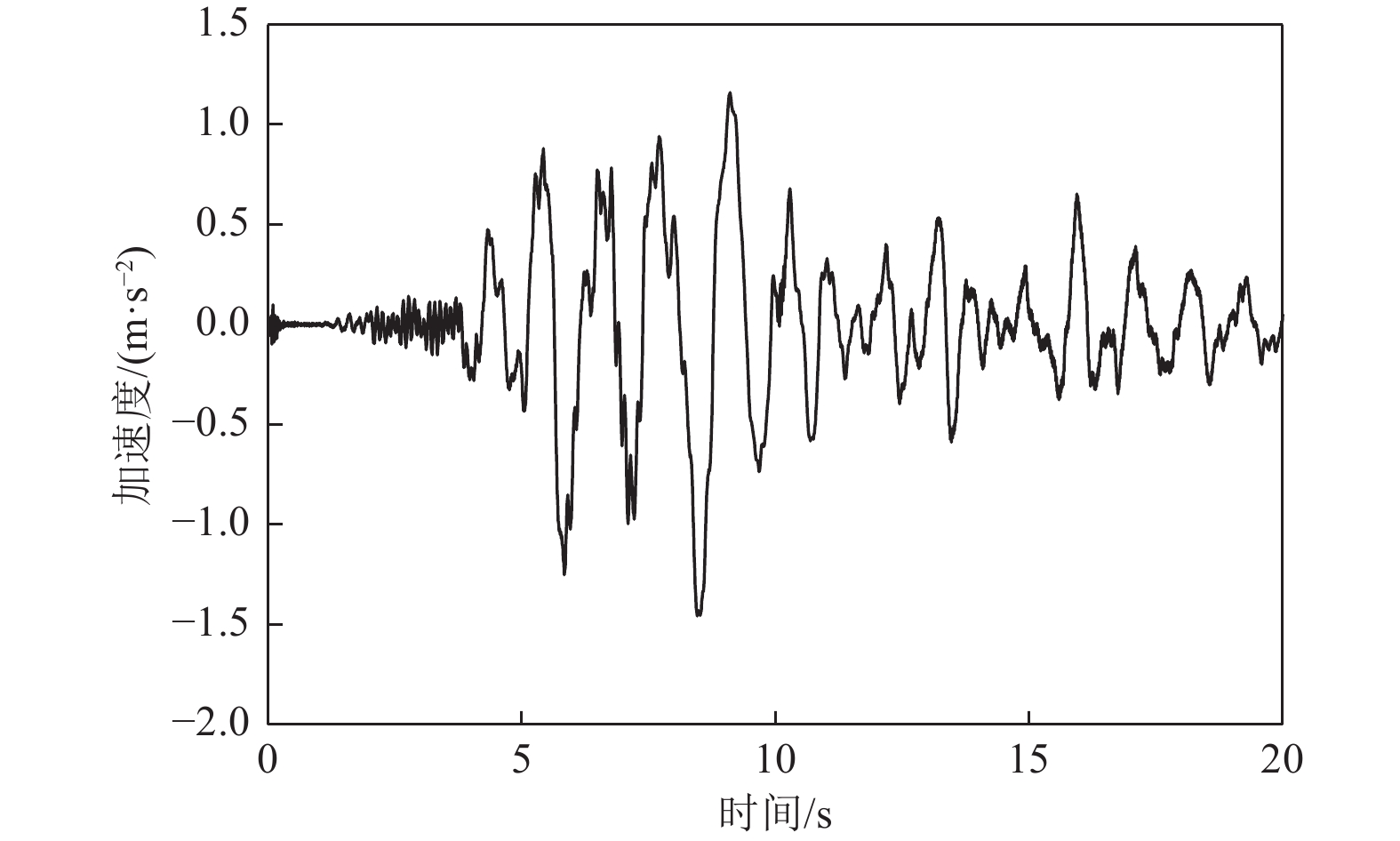
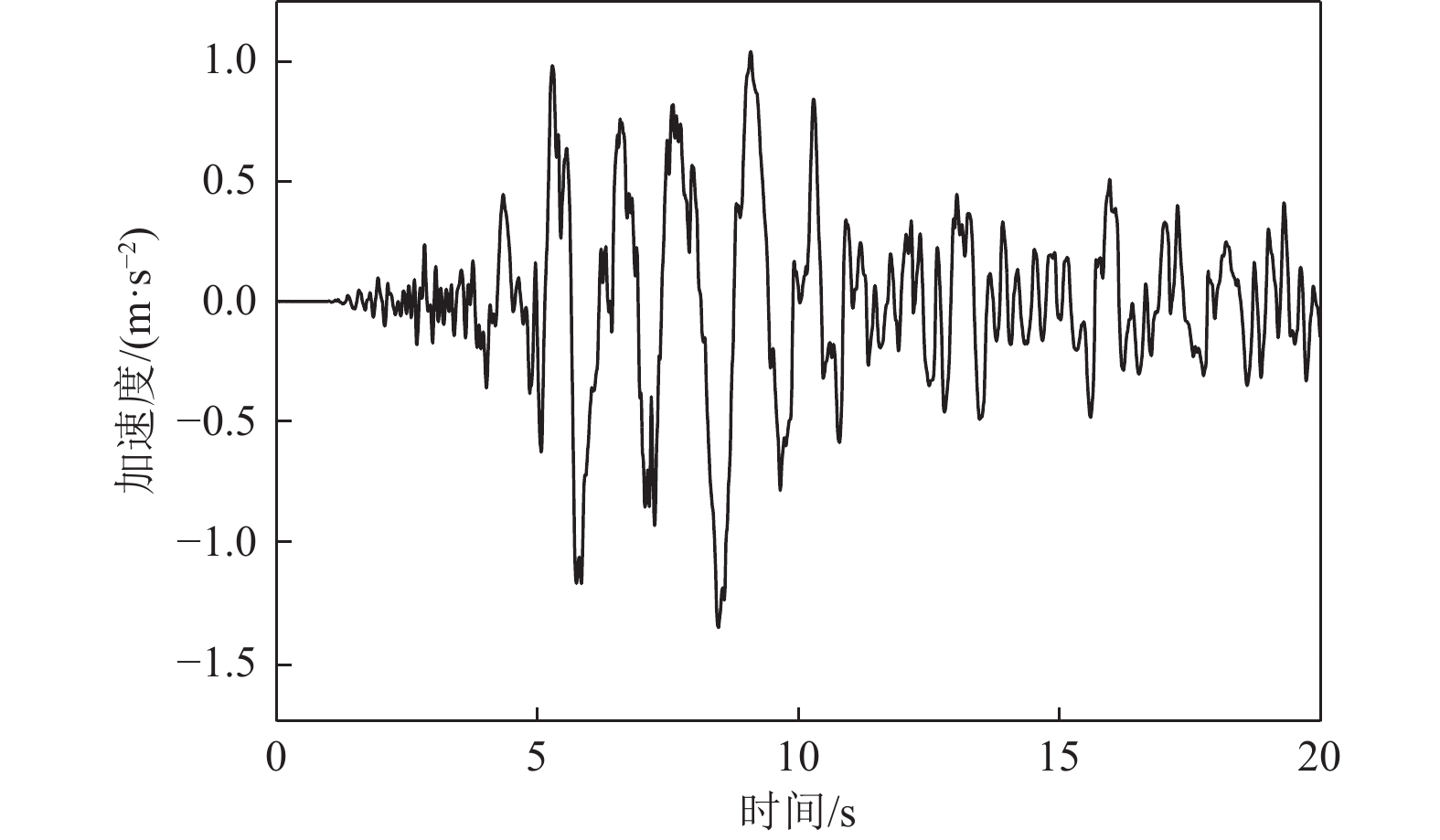
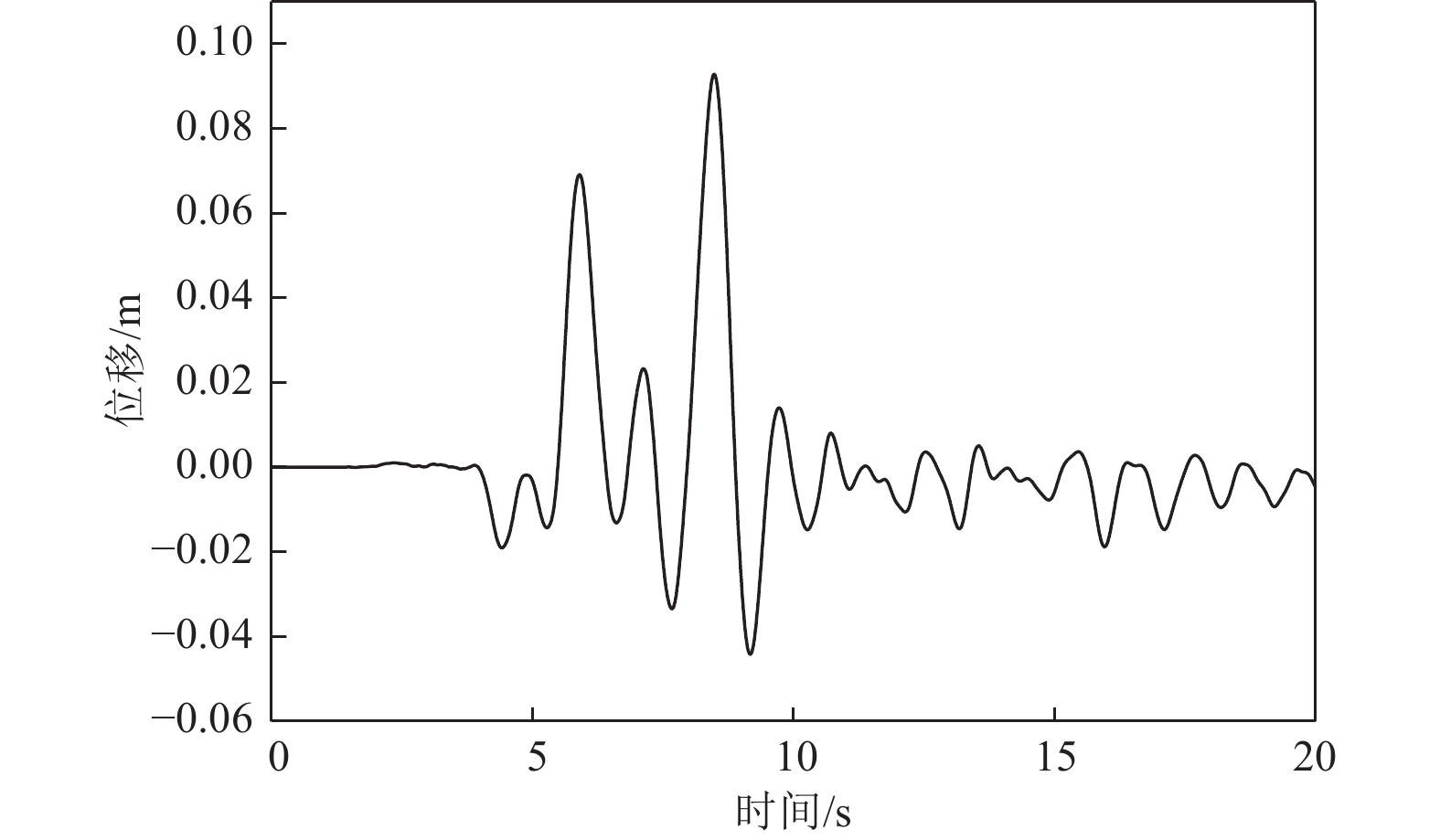
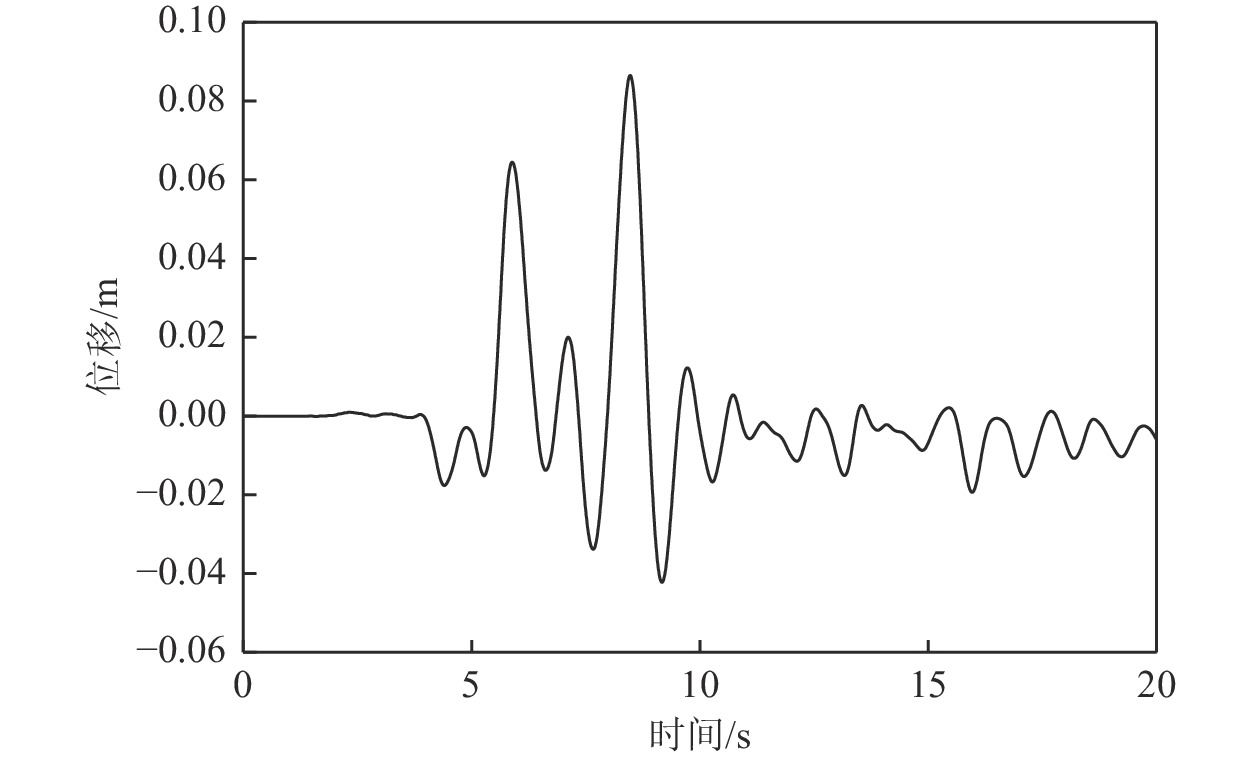
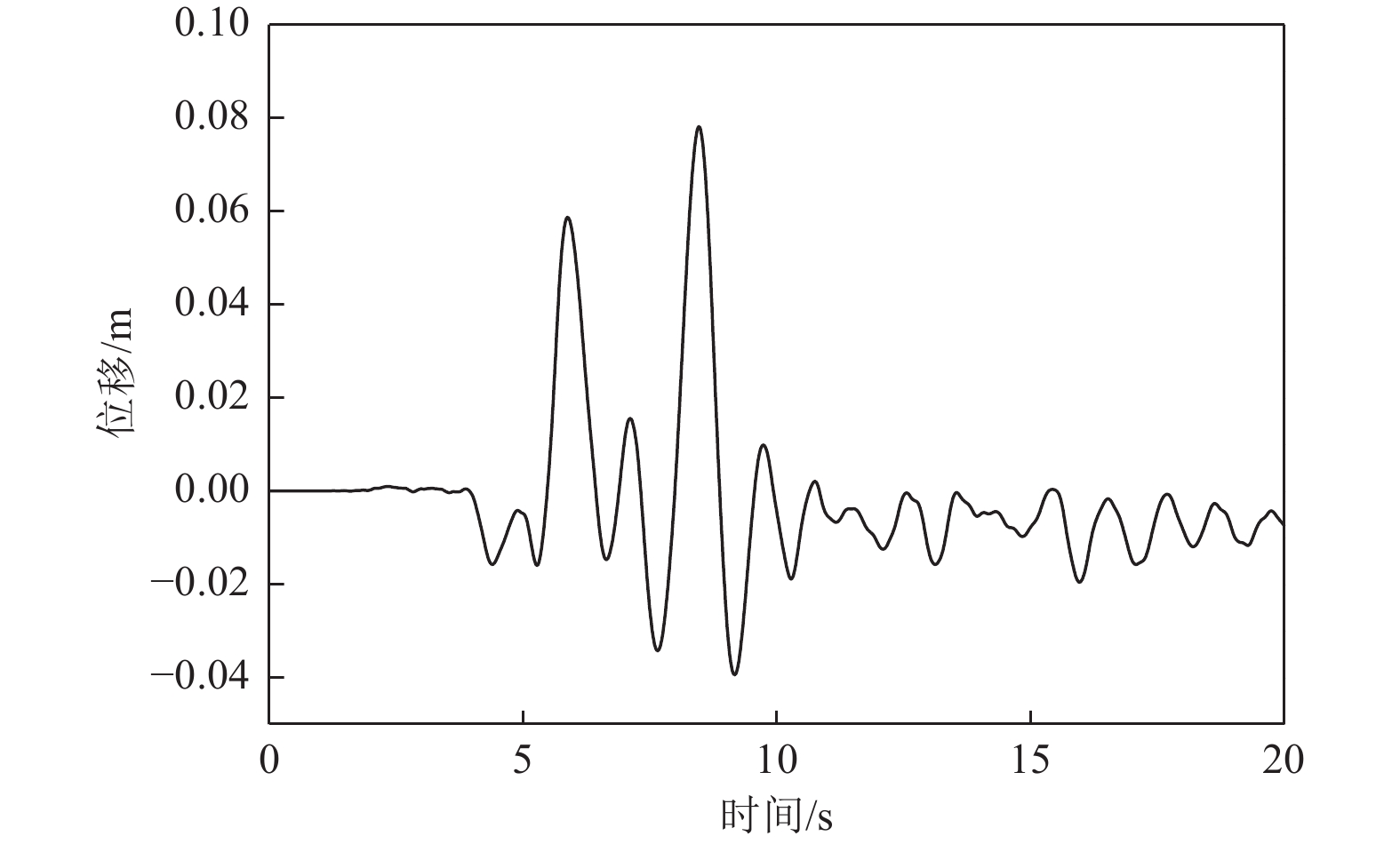
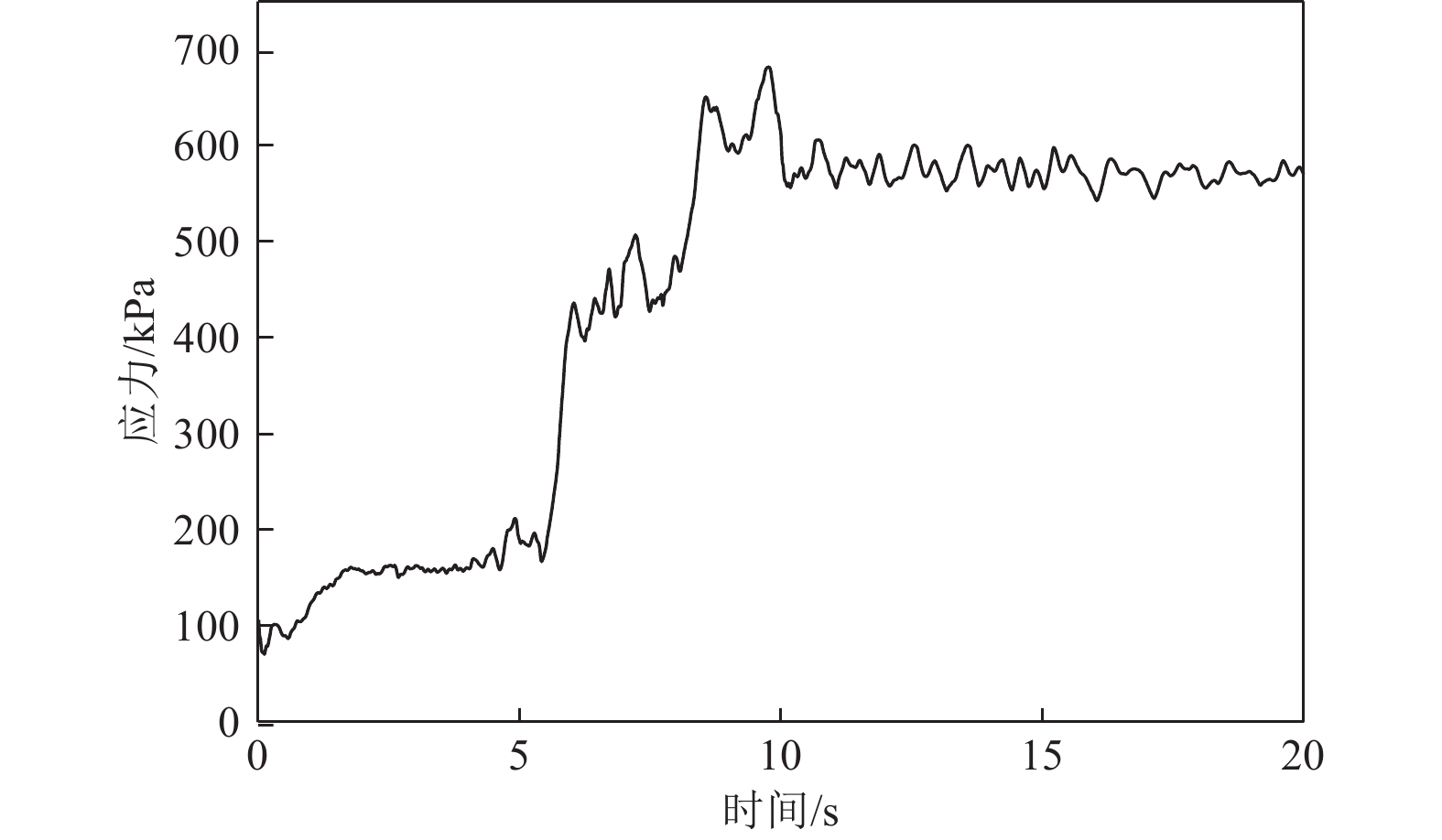
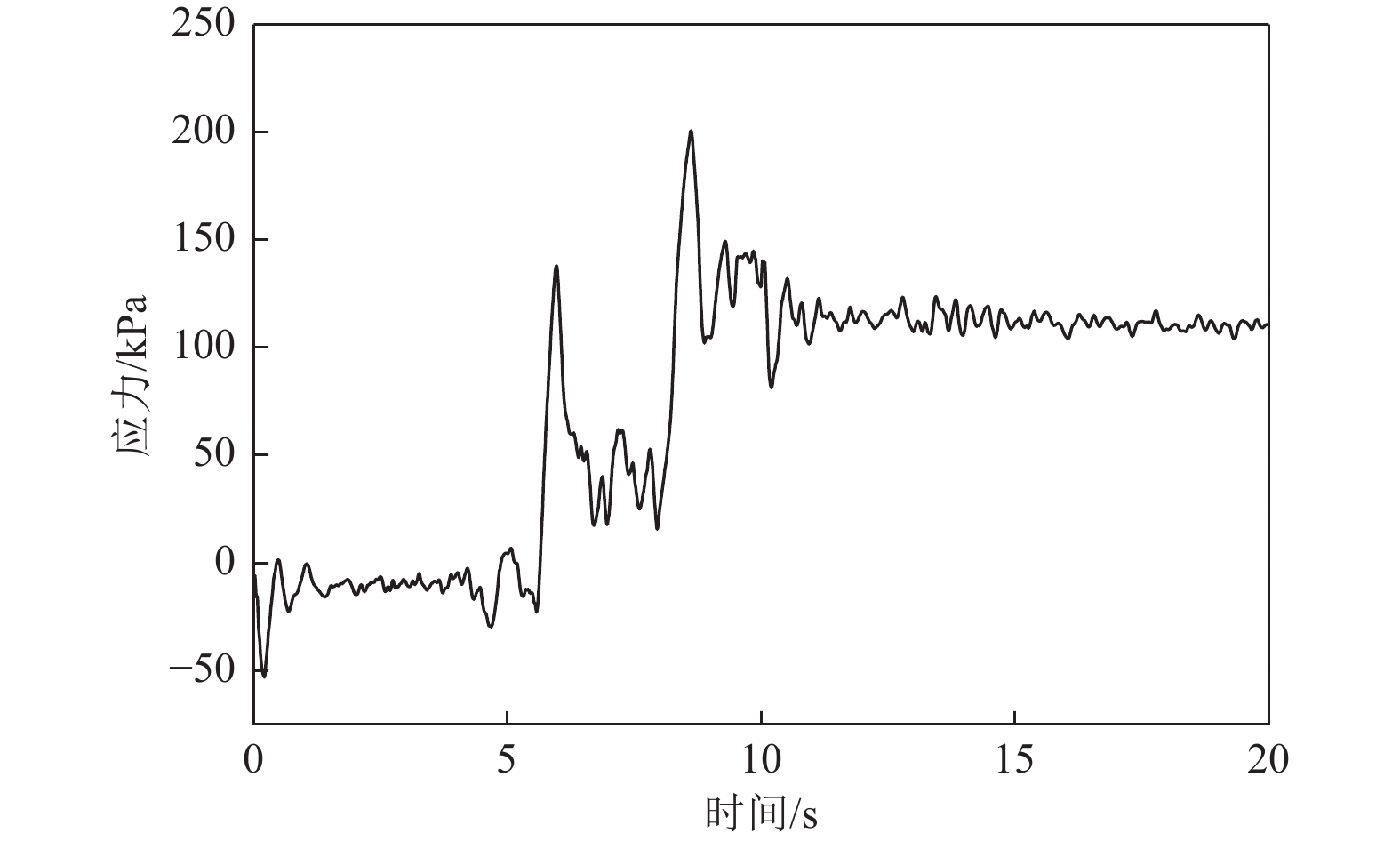
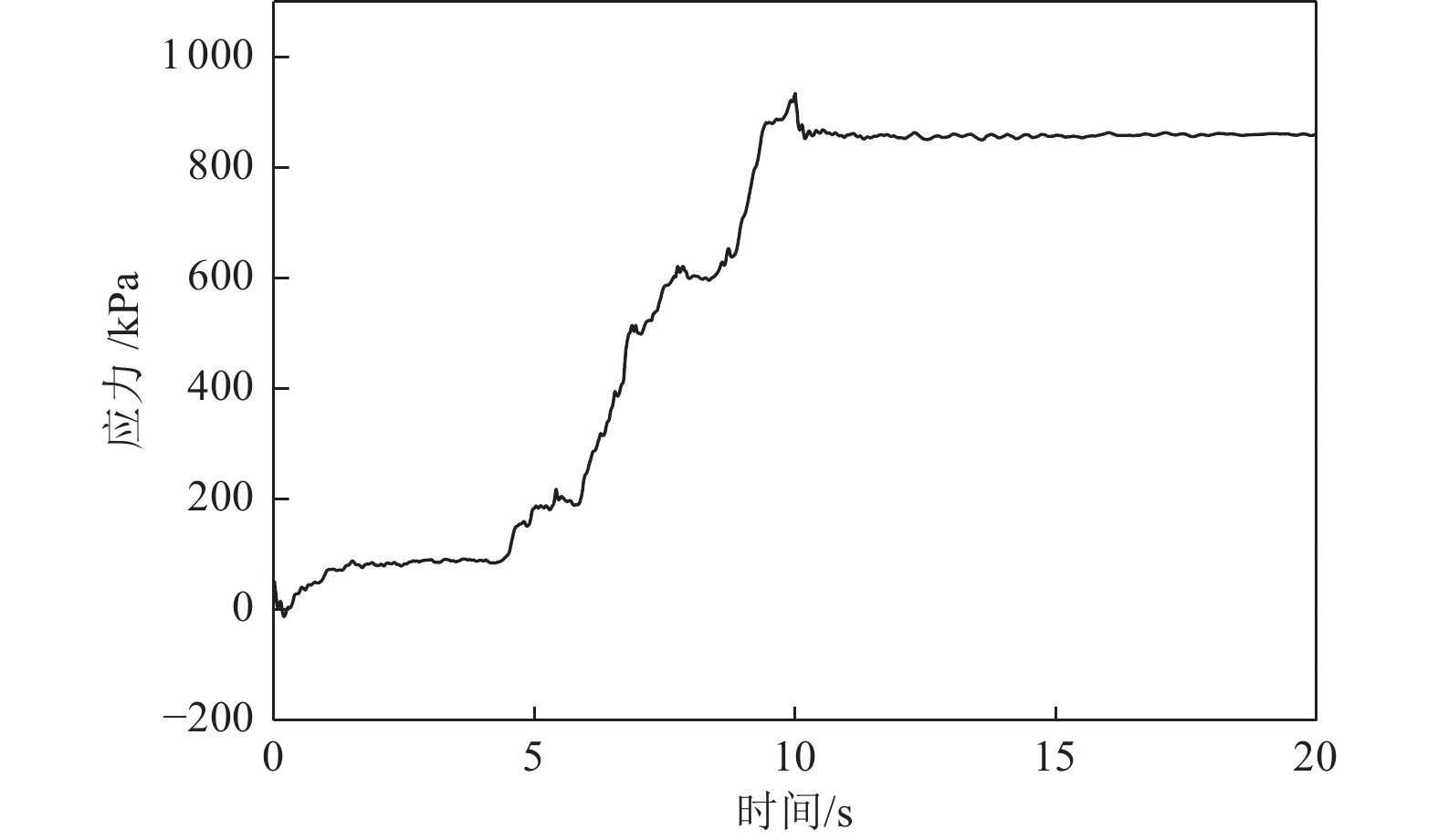
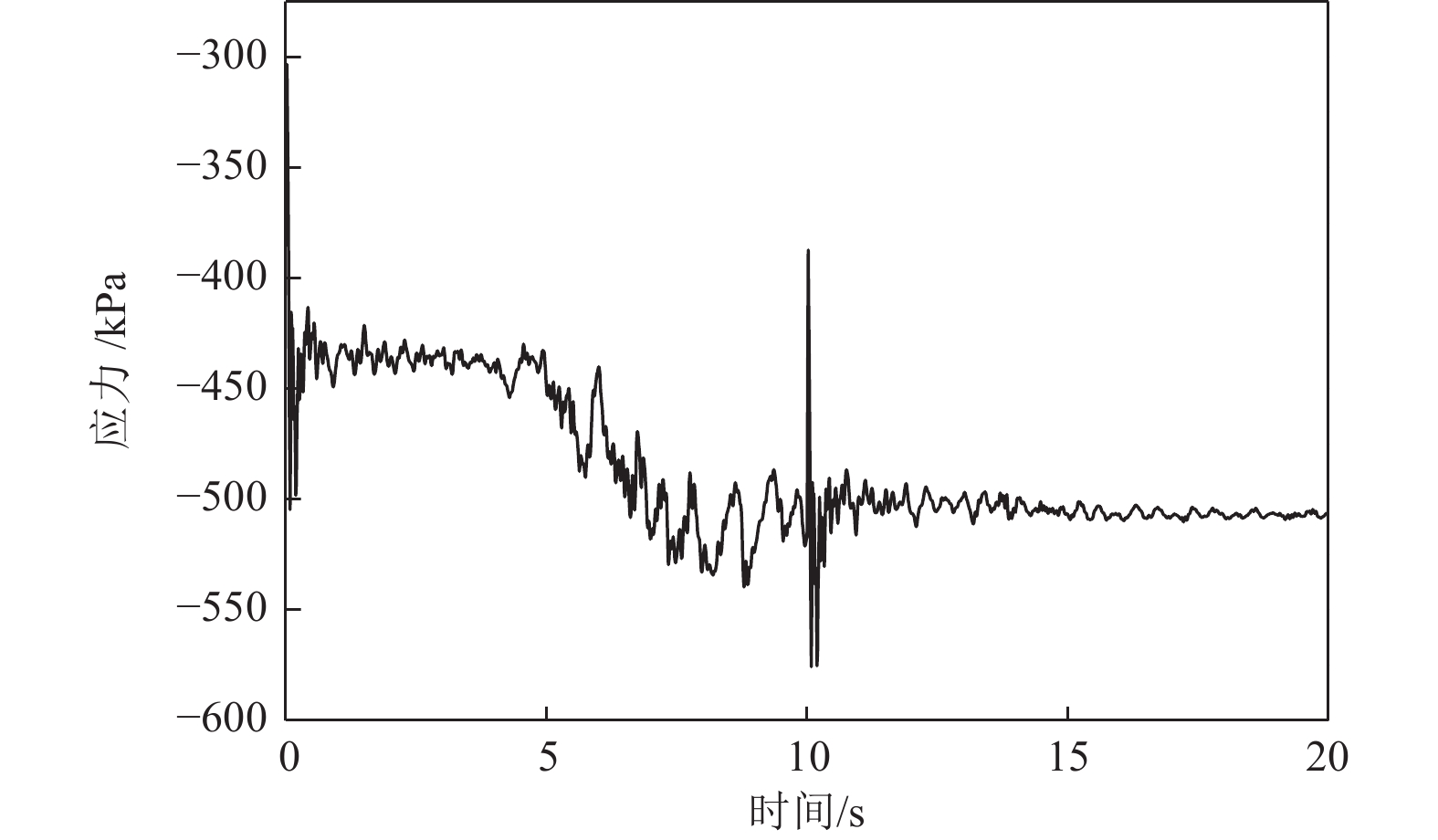
摘要: 应用OpenSees有限元计算程序,选取某地铁车站结构为主要分析对象,构建了饱和土-地下结构体系地震反应运算模型,并利用该模型进行系统的地震反应计算。将现场饱和土动力反应视为饱和两相介质近场波动问题,选取时域显式数值算法进行计算,同时考虑了土体的弹塑性。研究结果显示:(1)因选取弹塑性土体本构,土体-地下结构的位移反应时程和输入地震动的位移时程体现出了显著性差异。(2)对于两层三跨地下结构,在以剪切波形式输入的地震动作用下,顶板的峰值加速度和侧向位移最大,中板次之,底板最小,场地土体对地震波具有放大效应,顶层的层间位移大于底层。(3)在地震动作用下,地下结构不同区域应力时程的改变规律存在非常显著的差异。中柱底部与底板节点处的应力峰值最大。地震动输入结束时,结构存在残余应力。Abstract: Using the finite element calculation software OpenSees, this study focuses on a subway station structure as the primary analysis object while establishing an operational seismic response model for a saturated soil-underground structural system. This model enables a systematic calculation of the seismic response. The study considers the dynamic response of the saturated soil on site as a near-field fluctuation problem of a saturated two-phase medium. A time-domain explicit numerical algorithm is employed for calculations, incorporating the elastic-plastic dynamic response characteristics of the soil. The results of this study indicate several key findings: (1) The elastic-plastic dynamic response characteristics of the site soil are accounted for during the simulation analysis. This consideration reveals a significant difference between the displacement response time histories of the soil and underground structures compared to the displacement time histories of the input ground motion. (2) In the case of a two-story, three-span underground structure subjected to ground motion in the form of shear waves, the roof exhibits the highest peak acceleration and lateral displacement, followed by the middle plate, while the bottom plate shows the smallest values. Additionally, the inter-story displacement of the top layer is greater than that of the bottom layer. (3) Under earthquake loading, there are pronounced differences in the stress time history patterns across various regions of the underground structure. The stress peak at the joint between the bottom of the center column and the bottom plate is the highest observed. Furthermore, when ground motion input ceases, residual stresses remain in the structure.
-
引言
中缅油气管道工程是我国重大能源战略项目,全长1 726.8 km,其包括原油管道与天然气管道。两管道起自缅甸西海岸皎漂,经云南省瑞丽市58号界碑入境,在贵州安顺市分离,天然气管道向南经贵阳市到达贵港市。管道沿线地质背景复杂,地震活动强烈,穿越喜马拉雅地震带、滇西南地震带、鲜水河滇东地震带、右江地震带和长江中游地震带,历史上曾发生过8级地震1次、7~7.9级地震16次、6~6.9级地震65次,对管道站址影响烈度最大达8度以上。在云南境内,有3条曾发生过7级和7级以上地震的断裂与管道相交,分别为龙陵-瑞丽断裂、凤仪-定西岭断裂、小江断裂,在管线近场有18条活动断裂通过,这些断裂具备发生强烈地表错位的地震构造条件(高涵等,2020),存在未来发生强震的可能,对管道安全营运造成潜在威胁。
强烈地震对地下管道有破坏作用(Wang等,1991),这种作用主要有2种表现形式,即强烈振动引起的管道连接脱落和地面变形引起的管体变形破坏。1976年河北唐山大地震的地震动和地表破裂对秦皇岛至北京的输油管道造成了多处变形、管体破损(王亮亮等,2003);1985年墨西哥地震的地震动造成了管线管体破碎与接头脱落;2002年美国阿拉斯加发生7.9级地震,管道穿越的断层破裂长达1 m以上,但由于采取了特殊的抗震措施,避免了管道破裂(Hall等,2003);2016年日本福冈7.3级地震中,地下水管由于地震破裂而变形损坏,泄露的水损毁了地面道路(孟晨,2018)。为减轻地震对管道破坏造成的影响,多破坏性地震地区油气管道工程重视管道的抗震措施,如在阿拉斯加油气管道穿越断层时,在管道下方采用了滑轮设计,为管道横向和纵向移动保留了空间,避免了在2002年7.9级地震中受到损坏(郭守德等,2019)。此外,布设管道地震观测系统,在破坏性地震发生后能够快速判断管道状况,及时采取措施,这也是有效减轻油气管道地震灾害的方法。在中缅油气管道沿线建立地震观测系统,当管道沿线一定范围内发生破坏性地震时,对于观测管道遭受的地震动强度及管道穿越的断裂变形情况具有重要意义。
中缅油气管道地震观测系统由3套子系统构成,即观测管道地震动强度的强震动观测子系统、在管道与3条断层相交处观测断裂变形的GNSS(Global Navigation Satellite System)断裂变形观测子系统和观测管道变形的光纤管道变形观测子系统。本文主要介绍GNSS断裂变形观测子系统。
GNSS是高精度全球卫星定位导航系统,已大量应用于建筑物沉降、区域性地貌变化、地壳变形观测中(帅向华等,2011;李希亮等,2014;刘昌伟等,2019;李兆隆等,2019)。采用相对定位技术,GNSS可获得毫米级的定位精度,但需在观测点一定距离外布设参考站,而精密单点定位(Precise Point Positioning,PPP)无须布设参考站,数据处理更方便(张小红等,2015;邹冠华,2018)。中缅油气管道断裂变形观测子系统在管道穿越的断裂两侧各布设1台GNSS接收机,采用PPP技术精确定位同一时刻断裂两侧观测点的位置,即可确定断层两侧的相对位错,再结合油气管道容许变形,可估计穿越断层管道可能的变形情况。利用已建成的2条断层GNSS断裂变形观测子系统获取的观测数据,本文研究了跨断层GNSS观测的数据质量及观测误差,结果表明,本项目建立的GNSS断裂变形观测子系统能够有效观测破坏性地震引起的断层变形量。
1. GNSS断裂变形观测方法及观测系统设计
1.1 精密单点定位原理
精密单点定位算法采用双频相位和伪距观测数据,利用国际GPS服务组织(IGS)或其他分析中心发布的精密星历和钟差同时解算观测站坐标、钟差和对流层延迟及模糊度参数,PPP可利用单台双频GPS接收机的伪距和载波相位观测值进行精密绝对定位。
对于双频GNSS接收机,PPP的伪距和载波相位消电离层组合观测方程可表示为:
$$ P=\rho +c\left({\delta t}_{{\rm{r}}}-{\delta t}^{{\rm{s}}}\right)+{M}_{{\rm{W}}}{\xi }_{{\rm{T}}}+{\varepsilon }_{{\rm{P}}} $$ (1) $$ \varphi =\rho +c\left({\delta t}_{{\rm{r}}}-{\delta t}^{{\rm{s}}}\right)+\lambda N+{M}_{{\rm{W}}}{\xi }_{{\rm{T}}}+{\varepsilon }_{{\text{φ}} } $$ (2) 式中,
$ P、\varphi $ 分别为消电离层的伪距和载波相位;$ \rho $ 为卫星至接收机的几何距离;$ {\delta t}_{{\rm{r}}}\mathrm{和}{\delta t}^{{\rm{s}}} $ 分别为接收机和卫星的钟误差,后者可利用精密卫星钟差产品消除;$ {M}_{{\rm{W}}} $ 为对流层湿分量投影函数;$ {\xi }_{{\rm{T}}} $ 为信号传播方向的天顶对流层延迟;$ {\varepsilon }_{{\rm{P}}} $ 和$ {\varepsilon }_{\text{φ}} $ 为测量噪声;λ为消电离层组合观测值的波长;N为消电离层组合观测值的浮点模糊度。基于上述2个观测方程,综合考虑卫星端、接收机端及信号传输过程中有关误差的影响,使用各种改正模型对定位过程改正发展的数据解算方法,可在4颗以上卫星接收数据的情况下,获取观测的位置信息,其静态定位精度可达厘米级,可满足工程观测的需要。
1.2 基于PPP技术的断裂位错观测方法
在断裂两盘各布设1个GNSS观测点,利用PPP技术确定同一时刻2个观测点的相对位置,在断裂发生位错的情况下,由2个观测点相对位置变化可确定断裂的位错量:
$$ D=L-{L}_{0} $$ (3) $$ {L}_{0}=\sqrt{{{(x}_{0}^{A}-{x}_{0}^{B})}^{2}+{{(y}_{0}^{A}-{y}_{0}^{B})}^{2}+{{(z}_{0}^{A}-{z}_{0}^{B})}^{2}} $$ $$ L=\sqrt{{{(x}_{}^{A}-{x}_{}^{B})}^{2}+{{(y}_{}^{A}-{y}_{}^{B})}^{2}+{{(z}_{}^{A}-{z}_{}^{B})}^{2}} $$ 式中,D为断裂发生的位错量,(
$ {x}_{0}^{A},{y}_{0}^{A},{z}_{0}^{A} $ )、($ {x}_{0}^{B},{y}_{0}^{B},{z}_{0}^{B} $ )分别为观测点A、B的初始空间坐标,($ {x}_{}^{A},{y}_{}^{A},{z}_{}^{A} $ )、($ {x}_{}^{B},{y}_{}^{B},{z}_{}^{B} $ )分别为观测点A、B同一时刻GNSS的定位坐标,$ {L}_{0}、L $ 分别为断裂发生位错前、后2个观测点间的基线长度。当不考虑断裂的竖向位错时,有:
$$ {L}_{0}=\sqrt{{{(x}_{0}^{A}-{x}_{0}^{B})}^{2}+{{(y}_{0}^{A}-{y}_{0}^{B})}^{2}} $$ (4) $$ L=\sqrt{{{(x}_{}^{A}-{x}_{}^{B})}^{2}+{{(y}_{}^{A}-{y}_{}^{B})}^{2}} $$ (5) 将位错量分解到3个空间坐标方向上,可确定3个方向的位错量,有:
$$ \left\{\begin{aligned}&X={(x}^{A}-{x}_{0}^{A})-{(x}^{B}-{x}_{0}^{B})\\ &Y={(y}^{A}-{y}_{0}^{A})-{(y}^{B}-{y}_{0}^{B})\\ &Z={(z}^{A}-{z}_{0}^{A})-{(z}^{B}-{z}_{0}^{B})\end{aligned}\right. $$ (6) 1.3 GNSS断裂位错观测系统设计及建设
1.3.1 观测点选址
在云南境内管道将穿越多条断裂,根据地震地质资料,选择龙陵-瑞丽断裂、凤仪-定西岭断裂及小江断裂西支作为断裂位错观测对象。历史上这3条断裂均发生过7级以上的强震,仍存在再次发生7级以上破坏性地震的可能。
为保证观测结果能够真实反映断裂两盘的位错情况,观测站址应选择在断层两盘坚固完整的基岩上,不受地表水土流失、滑坡等因素影响。站址场地应开阔、无明显遮挡物。经实地考察,根据管道穿越断裂处地形地貌、断裂两盘情况,3个断裂位错GNSS观测子系统观测点布设如图1~图3所示。
(1)镇安观测站
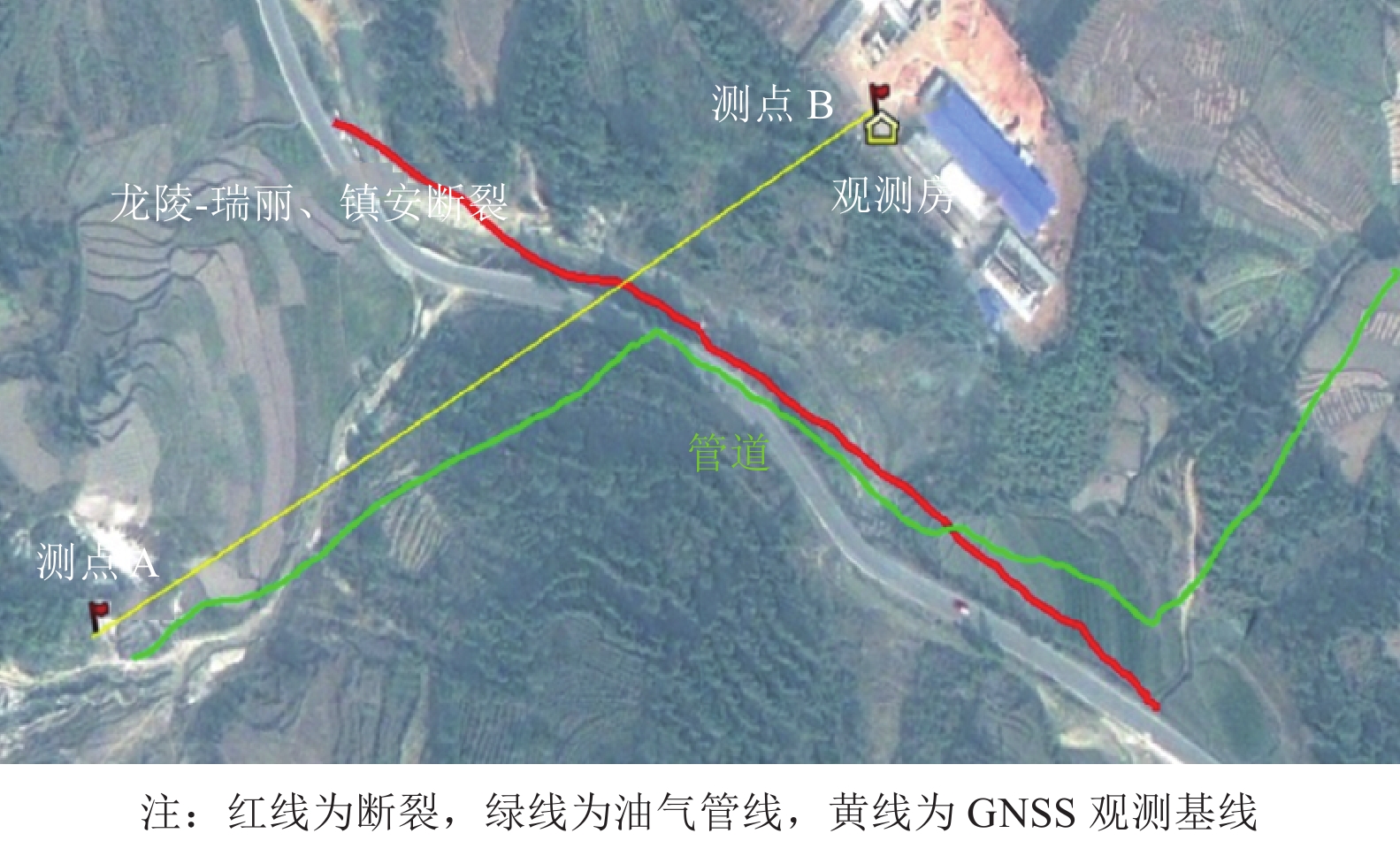
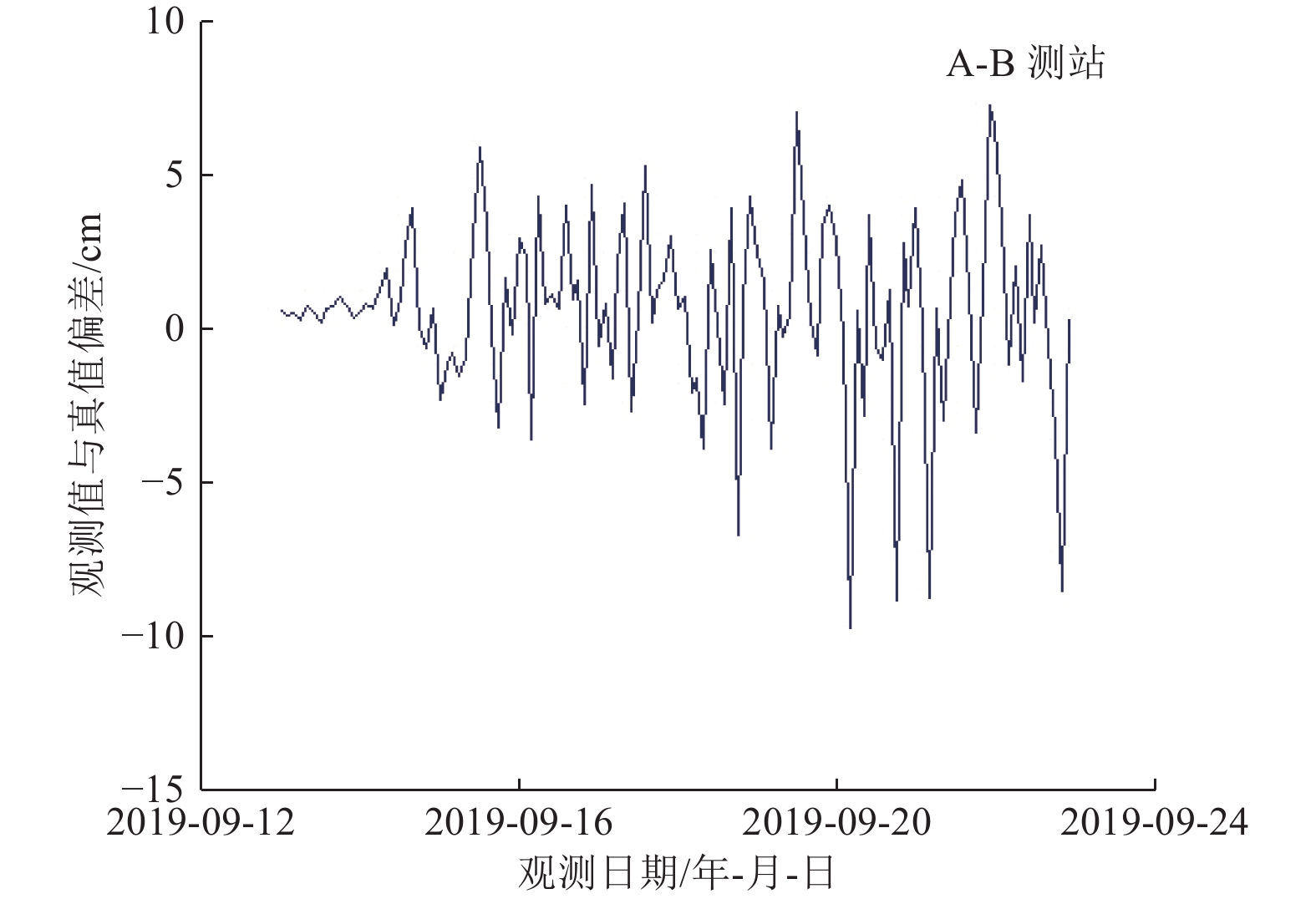
镇安GNSS观测站距龙陵县城17 km,距龙陵县镇安镇5 km,位于龙陵-瑞丽断裂上。龙陵-瑞丽断裂经龙陵、芒市、遮放、瑞丽盆地,延入缅甸中央盆地,全长约135 km,其北端与高黎贡断裂呈弧形相接。1976年5月29日,该断裂曾发生7.3级和7.4级大地震。经实地考察,选择A、B点作为断裂两盘GNSS观测点场址,两点间距159 m,如图1所示。
(2)定西岭观测站
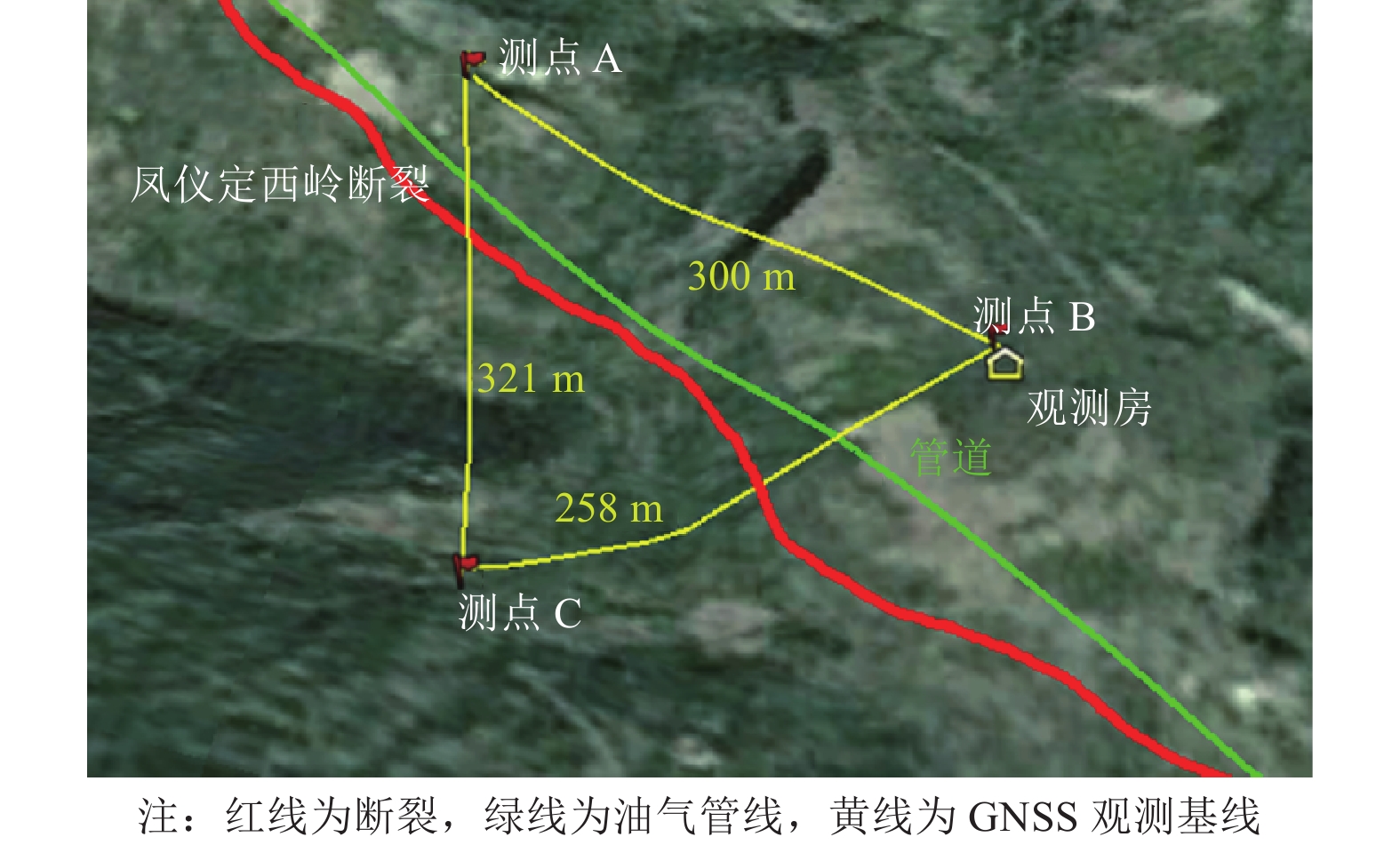
弥渡定西岭GNSS观测站位于弥渡县红岩镇定西岭,距弥渡红岩镇14 km,处在凤仪-定西岭断裂上。凤仪-定西岭断裂属红河大断裂带,红河断裂带是青藏高原东缘1条重要的大地构造和活动块体边界,该断裂及其周边地区地震活动较强烈,1925年曾发生凤仪7级地震。实地考察显示,在管道与断裂交汇处,断裂分为2支(图2),为观测交汇处断裂的位错,在被断裂分割的3个地块上各选择1个GNSS观测点,构成如图2所示的三角形测量体系,其两两间距分别为300、321、258 m。
(3)小碑当观测站
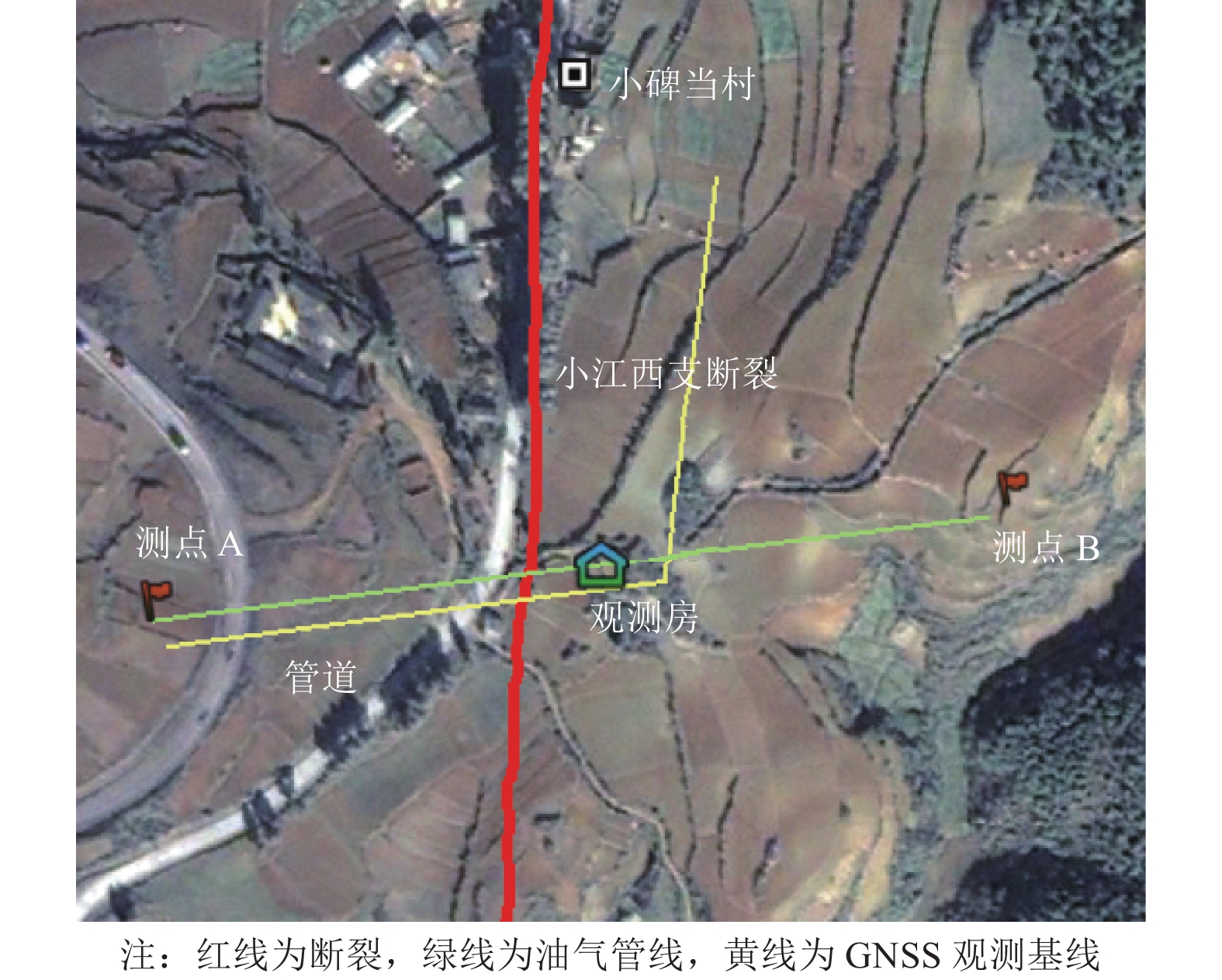
小碑当GNSS观测站位于寻甸县羊街镇小碑当村,距寻甸县城17 km,距羊街镇6 km,处在小江断裂带西支上。小江断裂带地处青藏高原东南侧,近SN走向,全长约400 km,系川滇菱形地块与华南地块的边界,其北起云南巧家,向南经东川分为东、西支,近平行延伸至抚仙湖,然后呈扫帚状向南撇开为多条断层。其构造运动十分活跃,地震活动频繁并诱发多种类型的地质灾害,1833年小江断裂带西支曾发生嵩明8级大地震。小江断裂与前述2条断裂相比,规模更大,在管道与断裂交汇处,断裂破碎带宽近300 m,两盘稳定地块间距应≥300 m,按相关规范的规定,选择的观测点如图3所示,观测点间距400 m。
1.3.2 GNSS断裂位错观测子系统建设
GNSS断裂位错观测子系统由2~3个跨断裂GNSS固定观测点观测墩、GNSS测量系统、供电系统、通信系统组成。
(1)观测墩
按照《全球导航卫星系统连续运行基准站网技术规范》(GB/T 28588—2012)(中华人民共和国国家质量监督检验检疫总局等,2012),GNSS混凝土观测墩应建在基岩上,如场地基岩埋深>20 m,则混凝土观测墩建在地下20 m深度处。观测墩高出地面3~5 m。建成的观测墩和观测房如图4、图5所示,观测房内包含1个观测墩。
(2)供电和通信
供电系统采用太阳能直流供电,太阳能供电系统以最大连续阴雨10 d计算,可在连续10 d无日照天气时为设备供电。
获取的观测数据通过4G移动网络远程传输到昆明数据中心,并可实现对设备的远程操控。
(3)GNSS测量系统
GNSS测量系统由GNSS天线和信号接收机组成,其技术性能指标如表1所示,采用S10接收机。
表 1 GNSS测量系统技术性能指标Table 1. Technical performance indexes of GNSS measurement system指标 基本性能 通道 ≥24(并行) 测量方式 独立完整的码与载波相位测量、高精度的多重相关 L1/L2 伪距测量 采样 最大20 Hz RTK网络 VRS、FKP、MAC 数据存储 ≥32 MB 通信 RJ45、RS232,无线802.11 b 功耗 2 W 电源 10.5~28 V 直流,带压电保护功能。 工作温度 −30 ℃~70 ℃ 静态精度(MonNET 后处理) 水平:3 mm+0.5 ppm RMS
垂直:5 mm+1 ppm RMS快速静态基线精度(后处理)
水平:5 mm+0.5 ppm
垂直:10 mm+1 ppm动态定位:RTK
水平:10 mm+1 ppm
垂直:20 mm+1 ppm天线类型 L1/L2 零相位微对中天线,抑径板可以减少多路径干扰 天线增益 50 dB,内置低噪音放大器 使用温度 −40 ℃~70 ℃ 天线包装 防水、密封,高技术材料外壳机械强度高 2. 观测数据及其处理
2.1 观测数据
率先建成的镇安、定西岭观测站于2019年9月5日投入运行。数据连续采集,采样间隔为15 s,数据每2 h生成1个观测文件,至2019年10月7日,共获取5 400条观测数据,期间由于故障等原因,2个观测站数据均存在部分缺失。数据文件格式为RINEX2.11,系统常驻可观测GPS系统卫星可达8颗,BDS卫星可观测到15颗,受数据处理方法的限制,本文处理的数据均源自GPS系统。
以仪器安装完成后测定的观测点初始位置(
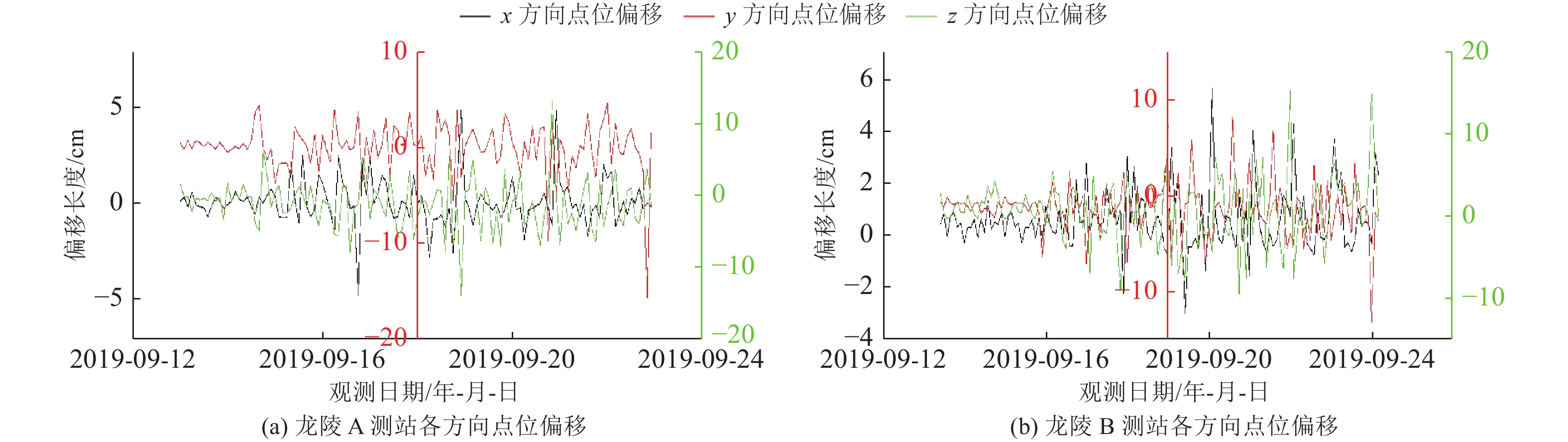
$ {X}_{0} $ 、$ {Y}_{0} $ 、$ {Z}_{0} $ )为参考点,龙陵观测站和定西岭观测站观测点PPP定位结果相对于测点初始位置偏移随时间的变化分别如图6、图7所示。由图6、图7可知,在3个方向上,各时刻的观测值围绕着初始位置波动,反映出各时刻确定的坐标位置有差异,在基础未发生位移的情况下,可将其视为GNSS观测的干扰背景,这种干扰包括观测数据解算算法本身包含的误差、大气环境等产生的影响。2.2 观测站测点间基线长度
确定GNSS观测站2个观测点同一时刻空间坐标后,即可按式(3)确定两测点间基线长度相对于初始长度的变化,当变化量超过测量误差时,则可认为两测点间的断裂两盘发生了错位。
不考虑竖向的变化,水平面内两观测点间基线长度变化可表示为:
$$ D=L-{L}_{0}=\sqrt{{({x}^{A}-{x}^{B})}^{2}+{({y}^{A}-{y}^{B})}^{2}}-\sqrt{{{(x}_{0}^{A}-{x}_{0}^{B})}^{2}+{{(y}_{0}^{A}-{y}_{0}^{B})}^{2}} $$ (7) 对于竖向点间距的变化,有:
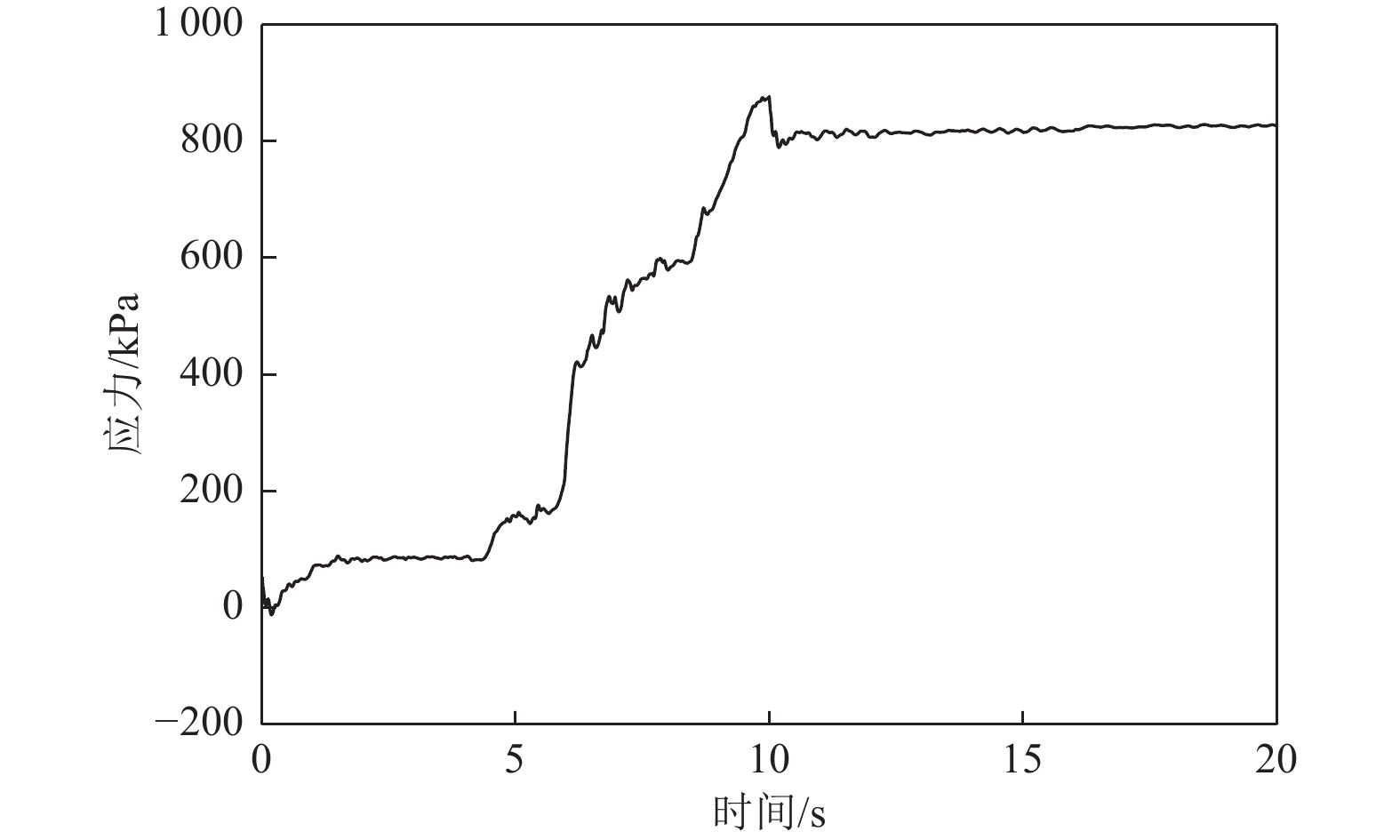
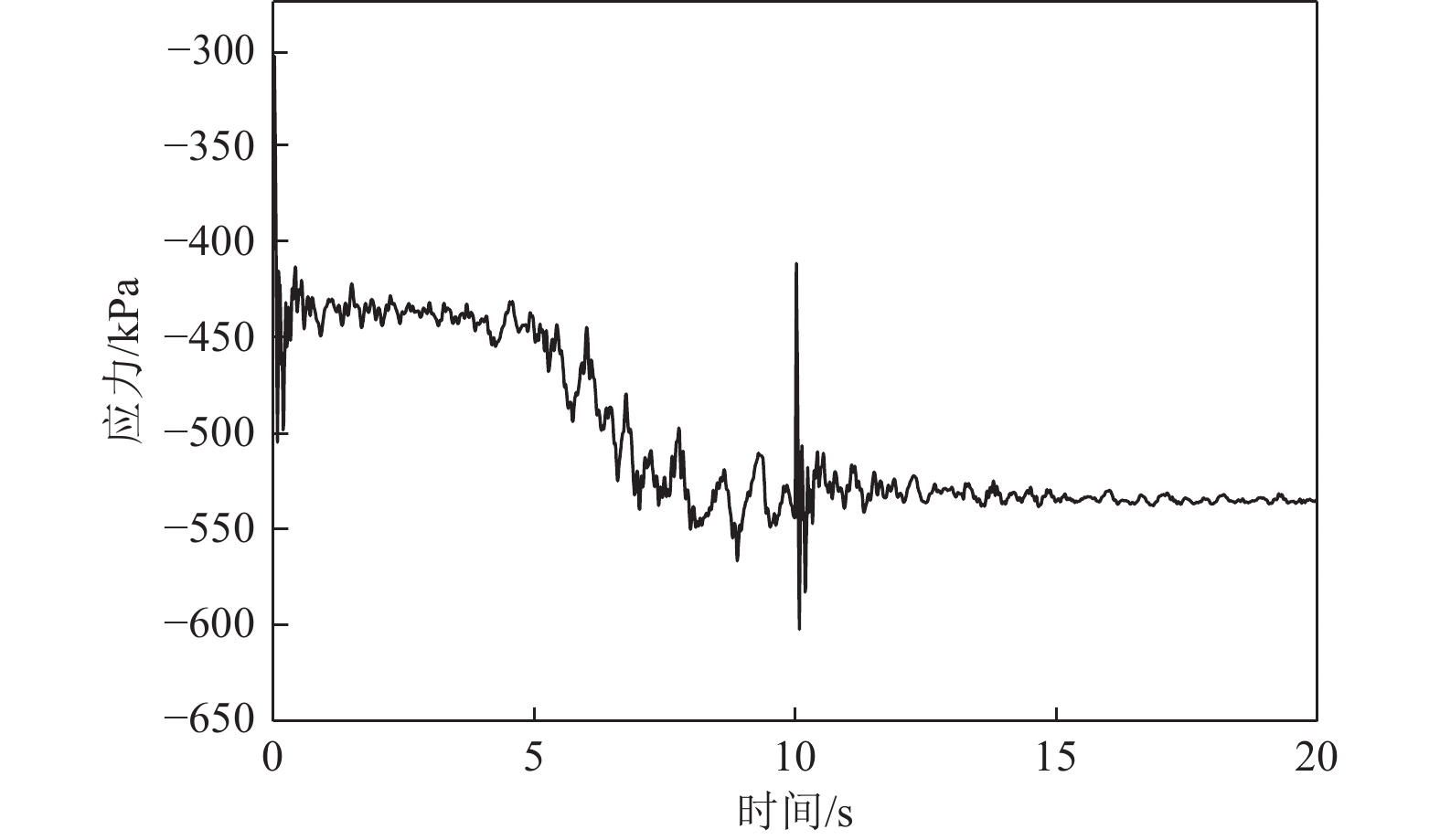
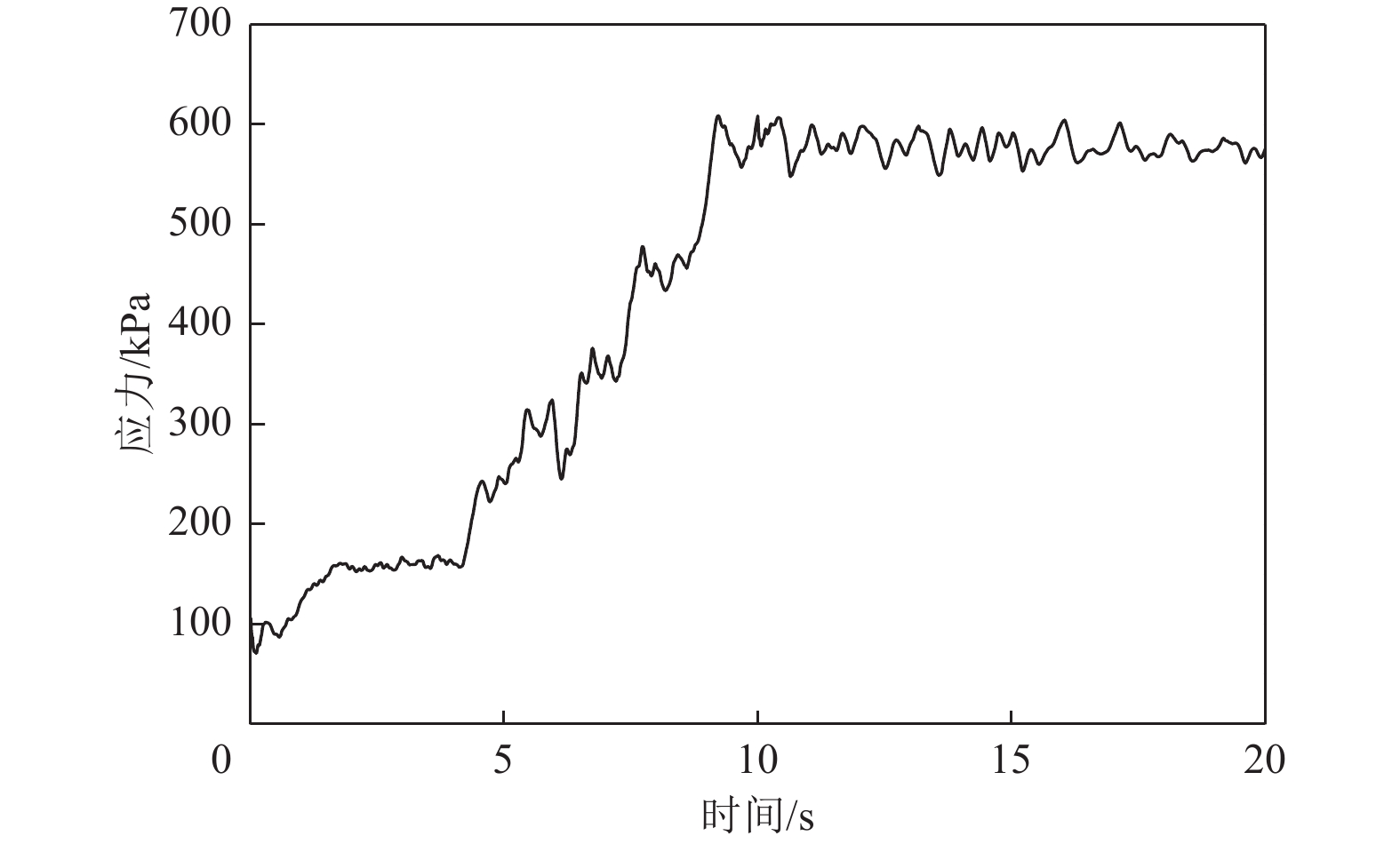
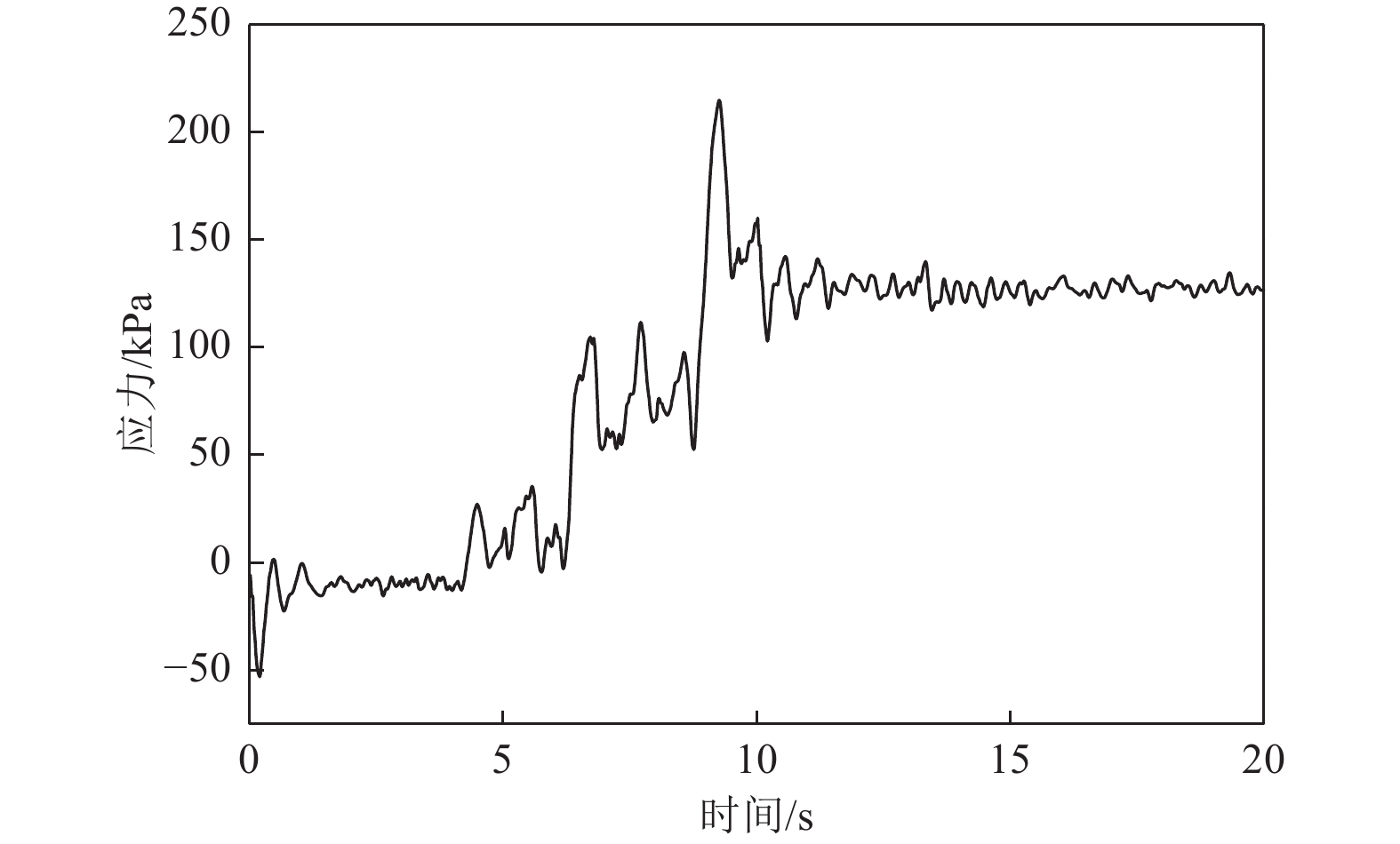
$$ Z={(z}^{A}-{z}_{0}^{A})-{(z}^{B}-{z}_{0}^{B}) $$ (8) 2个观测站观测点间水平面内基线长度在不同时刻的变化如图8、图9所示,2个观测站观测点间竖向间距在不同时刻的变化如图10、图11所示。
3. 结果分析
由图8~图11可知,2个观测站观测点间基线长度随时间变化,在所处理的时间段内,这种变化的最大值在水平向与竖向均≤10 cm,且无突出干扰值。由于在该时段内,未发生大的地震事件,可认为2个观测站间距在该波动范围内为正常状态。假定不发生地震时,2个观测站基线长度变化结果稳定在该数值范围内,则需解决的问题是观测时产生的测量误差满足管道变形安全要求。
GNSS观测数据解算误差有多种来源,如卫星轨道摄动、电离层、对流层、大气含水量、多路径效应影响等(李征航等,2010),目前虽发展了消除这些影响的方法,但在观测条件不利的情况下,如降雨、降水等,误差会相应增大。
3.1 误差分析
本文利用G-Nut/Anubis(以下简称Anubis)对布设的5个观测站数据从多路径效应、单点定位精度、信噪比、数据利用率及周跳方面进行数据质量分析(张涛等,2017;陈秀德等,2018;康朝虎等,2018)。
(1)多路径效应
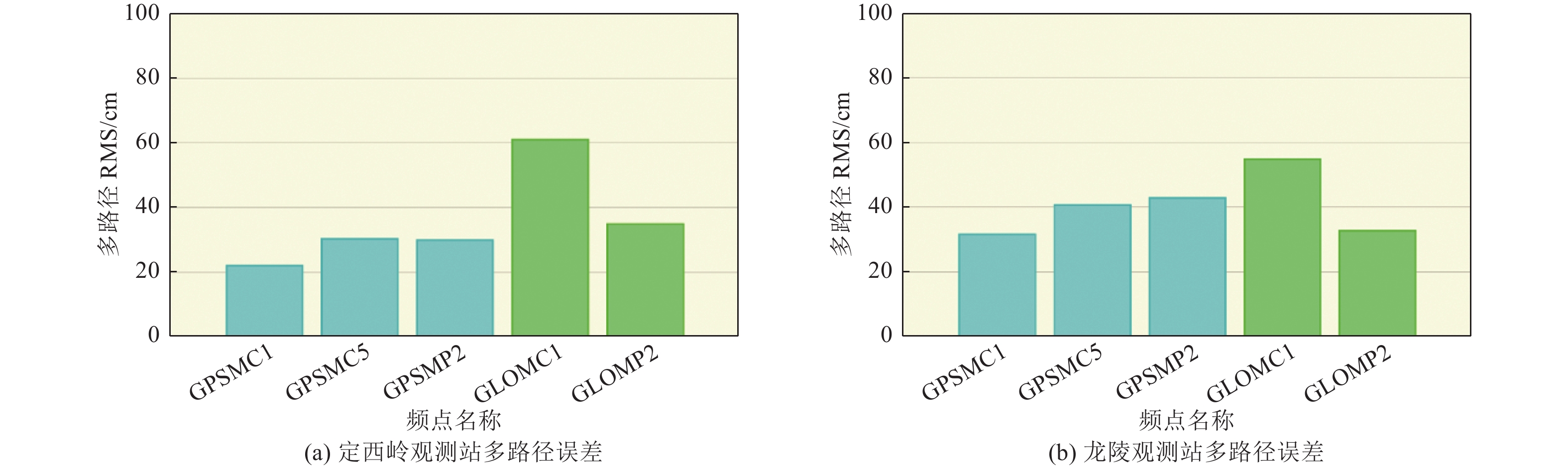
多路径效应是指接收机收到卫星发射的信号以外,同时接收到观测站附近物体反射的信号现象,接收到的不同路径信号会与原本接收到的卫星信号叠加,从而产生时延效应,带来误差,且该效应受环境的影响较大。不同观测站观测数据的多路径误差如图12所示。多路径均方根值受观测站周围环境影响,值越小表示数据质量越高。GPS系统观测的多路径误差多在40 cm上下浮动,GLONASS系统多路径误差较大。
(2)单点定位精度
不同观测站观测数据的单点定位误差如图13所示。单点定位误差值越小,精度越高。系统观测值N、E向的误差均<1 m,U向误差集中于2 m左右。
(3)信噪比
信噪比为载波信号与噪声的比值,影响信噪比的因素较多,接收机本身、观测环境及多路径效应均会对其造成一定影响,该值不仅可用于评估观测数据质量,同时也可作为GNSS接收机性能优劣的参考(布金伟等,2017;姚文敏等,2019)。不同观测站观测数据的信噪比如图14所示。由图14可知,观测数据信噪比基本在40 dB左右,各信道信噪比相差较小,较稳定。
(4)数据完整性
观测数据完整性是观测质量的直接决定因素,由于各种偶然因素及传输过程中的损耗,导致接收机实际接收的观测数据少于理论数据量。周跳也称为整周跳变,发生的次数越多,表示丢失的观测数据越多,可反映卫星信号失锁导致的整周计数偏差情况。对图14观测数据进行统计,可知GPS系统的数据可利用率远高于GLONASS系统,而其周跳次数经长时统计,所有台站周跳频次维持在较低水平,能够保证观测精度及数据可靠性(表2)。
表 2 数据利用率及周跳频次统计Table 2. Statistics of data utilization and weekly hop frequency测站 数据利用率/% 周跳频次 MDGCDS 100 0.006 2 MDGCF 100 0.012 0 MDGCDX 83 0.002 8 LLGCD 100 0.004 4 LLGCF 100 0.005 6 3.2 观测结果的有效性
观测的最终目的是对天然气管道进行保护,观测结果是站点的坐标变动,如果观测精度低于管道容许压缩应变范围,观测失去意义(张慧敏,2009;孟立朋等,2016)。
中缅油气管道跨越断层时一般在管沟中平直铺设,荷载垂直于管道轴向且沿轴向均匀分布,管道尺寸参数如表3所示。
表 3 管道尺寸参数Table 3. Design parameters of different pipeline projects管道项目 外径/m 壁厚/m 设计压力/MPa 天然气管道 0.010 6 0.022 9 15 原油管道 0.813 0 0.028 6 15 成品油管道 0.219 1 0.009 5 15 根据理论,管道受到地震影响时,由于管道自身的抗震设计,不会直接发生破裂,但如果管道受地震影响过大,其变形量超出容许范围时,会发生不可逆的损害,管道容许变形长度对于GNSS观测系统警报阈值计算至关重要。确定管道容许变形长度时分为2种情况,一种为管道受拉状态下产生的最大位移,另一种为管道受压状态下的最大位移。当管道受到拉伸、压缩时管道最大容许长度变化及土壤与管道外表面之间单位长度上的摩擦力根据《输油(气)钢质管道抗震设计规范》(SY/T 0450—2004)(国家发展和改革委员会,2004)中的相关规定计算。
考虑管道变形时,还需考虑弯曲变形对管道的危害,通过计算轴向运行弯曲变形能力,可得到管道能够承受的沉降偏差最大值,根据《输气管道工程设计规范》(GB 50251—2015)(中华人民共和国住房和城乡建设部,2015)中的相关规定计算。
不同管道项目容许拉伸量及容许压缩量如表4所示。
表 4 管道容许变形计算Table 4. Calculation of pipeline ultimate deformation管道项目 容许拉伸量/cm 容许压缩量/cm 容许弯曲量/cm 天然气管道 41.13 27.57 8.4 原油管道 132.59 70.56 152.9 成品油管道 66.08 20.04 63.77 由于PPP精度随观测时长而收敛,进行5、6 h以上时长收敛,可达厘米级精度(李浩军等,2010;赵爽,2018;淦邦,2020)。
具体定位精度的评价因素较多,主要包括平面位置精度因子(HDOP)、高程精度因子(VDOP)、空间位置精度因子(PDOP)、接收机钟差精度因子(TDOP)及几何精度因子(GDOP),以上因子带来的影响体现为观测值与真值的差值∆x、∆y、∆z进行坐标变换后得到的对应值,即站心坐标系下对应于观测站本身水平和高程方向的定位误差(代桃高,2017;王何鹏等,2019),转换公式为:
$$ \left[\begin{array}{c}E\\ N\\ U\end{array}\right]=\left[\begin{array}{*{20}{c}}-\sin\lambda & \cos\lambda &0\\ -\cos\lambda \sin\varphi & -\cos\lambda \sin\varphi & \cos\varphi \\ \cos\lambda \cos\varphi & \sin\lambda \cos\varphi & \sin\varphi \end{array}\right]\left[\begin{array}{c}\Delta_x\\ \Delta_ y\\ \Delta_z\end{array}\right] $$ (9) 式中,
$ \lambda $ 、$\varphi$ 分别为该点所在的经、纬度。大理弥渡和保山龙陵观测站GNSS观测精度如图15、图16所示。由图15可知,采样时间前段数据值波动较大,这是因为台站安置初期会出现基座沉降导致的安置点位变化及PPP初期带来的较大观测误差,PPP观测须经过长时间收敛才会趋于稳定值,如图15、图16所示,布设观测站观测结果的均方根误差基本在第400个历元后开始收敛并趋于稳定,取第400个历元后的误差均值为误差收敛值。
处理后PPP定位误差的收敛值如表5所示。由表5可知,N、E向误差收敛值均处于毫米级水平,U向误差收敛值由于GNSS本身的局限性而处于厘米级水平,将基线误差收敛值与管道容许拉伸量和容许压缩量进行对比,可知基线误差收敛值均小于3种管道容许拉伸量和容许压缩量,说明GNSS观测精度能够满足油气管道检测需求。
表 5 PPP基线误差收敛值Table 5. Convergence value of PPP baseline error方向 定西岭观测站基线
误差收敛值/cm龙陵观测站基线
误差收敛值/cmN 0.514 0.412 E 0.381 0.379 U 3.341 4.314 3.3 GNSS管道观测阈值
在系统实际应用中,由于偶然因素多,加上管道自身存在微小变形,会导致起始坐标、起始间距发生变化,综合各种因素,对于GNSS管道观测的阈值,不能单纯用极限变形量与极限误差收敛值评估,应结合数据的摇摆及观测误差进行考虑,可按下式计算:
$$ {\sigma }_{{\rm{t}}}=\left[{\Delta L}_{{\rm{t}}}\right]+{\sigma }_{g}+{\sigma }_{i} $$ (10) $$ {\sigma }_{{\rm{c}}}=\left[{\Delta L}_{{\rm{c}}}\right]+{\sigma }_{g}+{\sigma }_{i} $$ (11) $$ {\sigma }_{{\rm{h}}}={h}_{\max}+{\sigma }_{g}+{\sigma }_{i} $$ (12) 式中,
$ {\sigma }_{{\rm{t}}} $ 为GNSS管道拉伸观测阈值,${\sigma }_{{\rm{c}}}$ 为GNSS管道压缩观测阈值,$ {{\sigma }}_{{\rm{h}}} $ 为GNSS管道偏差阙值,$ {\sigma }_{g} $ 为第g条基线的误差收敛值,$ {\sigma }_{i} $ 为第i个观测点一时间序列上实测水平距或高程差与真实水平距和高程差间差值的标准差。不同观测站观测值与真实值差值的标准差如表6所示。观测时长内每条基线间的警报阈值计算结果如表7~表9所示。
表 6 不同观测站观测值与真实值差值的标准差Table 6. Difference standard deviation between observed values and true values at different stations基线段 标准差/cm 定西岭观测站A-C 0.058 定西岭观测站B-C 0.060 定西岭观测站A-B 0.058 龙陵观测站A-B 0.029 表 7 N向GNSS管道观测阈值Table 7. Observation threshold of GNSS pipeline in horizontal N direction基线段 拉伸阈值/cm 压缩阈值/cm 弯曲阈值/cm 天然气管道 原油管道 成品油管道 天然气管道 原油管道 成品油管道 天然气管道 原油管道 成品油管道 定西岭观测站A-C 41.702 133.162 66.652 28.142 71.132 20.612 8.972 153.472 64.342 定西岭观测站B-C 41.704 133.164 66.654 28.144 71.134 20.614 8.974 153.474 64.344 定西岭观测站A-B 41.702 133.162 66.652 28.142 71.132 20.612 8.972 153.472 64.342 龙陵观测站A-B 41.571 133.031 66.521 28.011 71.001 20.481 8.943 153.443 64.313 表 8 E向GNSS管道观测阈值Table 8. Observation threshold of GNSS pipeline in horizontal Edirection基线段 拉伸阈值/cm 压缩阈值/cm 弯曲阈值/cm 天然气管道 原油管道 成品油管道 天然气管道 原油管道 成品油管道 天然气管道 原油管道 成品油管道 定西岭观测站A-C 41.569 133.029 66.519 28.007 70.999 20.479 8.839 153.339 64.209 定西岭观测站B-C 41.571 133.031 66.521 28.011 71.001 20.481 8.841 153.341 64.211 定西岭观测站A-B 41.569 133.029 66.519 28.009 70.999 20.479 8.839 153.339 64.209 龙陵观测站A-B 41.538 132.998 66.488 27.978 70.968 20.448 8.808 153.308 64.178 表 9 U向GNSS管道观测阈值Table 9. Observation threshold of GNSS pipeline in vertical U direction基线段 拉伸阈值/cm 压缩阈值/cm 弯曲阈值/cm 天然气管道 原油管道 成品油管道 天然气管道 原油管道 成品油管道 天然气管道 原油管道 成品油管道 定西岭观测站A-C 44.529 135.989 69.479 30.969 73.959 23.439 11.799 156.299 67.169 定西岭观测站B-C 44.531 135.991 69.481 30.971 73.961 23.441 11.801 156.301 67.171 定西岭观测站A-B 44.529 135.989 69.479 30.969 73.959 23.439 11.799 156.299 67.169 龙陵观测站A-B 45.473 136.933 70.423 31.913 73.93 24.383 12.743 157.243 68.113 4. 结语
本文针对中缅油气管道工程范围内的2条断裂带,利用2019年沿断裂布设的GNSS固定台站观测数据,确保观测数据在误差允许范围内对断裂带变形趋势进行长期观测与分析,以降低中缅油气管道受地震的影响。当精度达到容许拉伸应变误差允许范围内时,布设GNSS观测站对断裂带进行有针对性的观测切实可行,相较于从IGS特征站观测数据内插得到断裂带数据更直接快捷,能够直观反映断裂带变形趋势,且规避了参考站受地震波影响导致观测结果产生的误差,从而保证准确获得地震位移波形。
本研究还存在部分问题,PPP观测精度虽随观测时长而收敛,且观测时长满足其收敛要求,但由于观测精度与拉伸应变误差较接近,不能完全保证突发的偶然误差能够得到处理。有研究表明采用BDS与GPS双系统乃至引入3个以上系统能够显着提高PPP观测精度,而本项目使用了GPS单系统,仍有改进空间;进行精度论证时,仅评价了N、E、U向上的精度差,由于N、E、U向均为一维方向,并未进行三维精度论证,结果不够严谨,需改进。
-
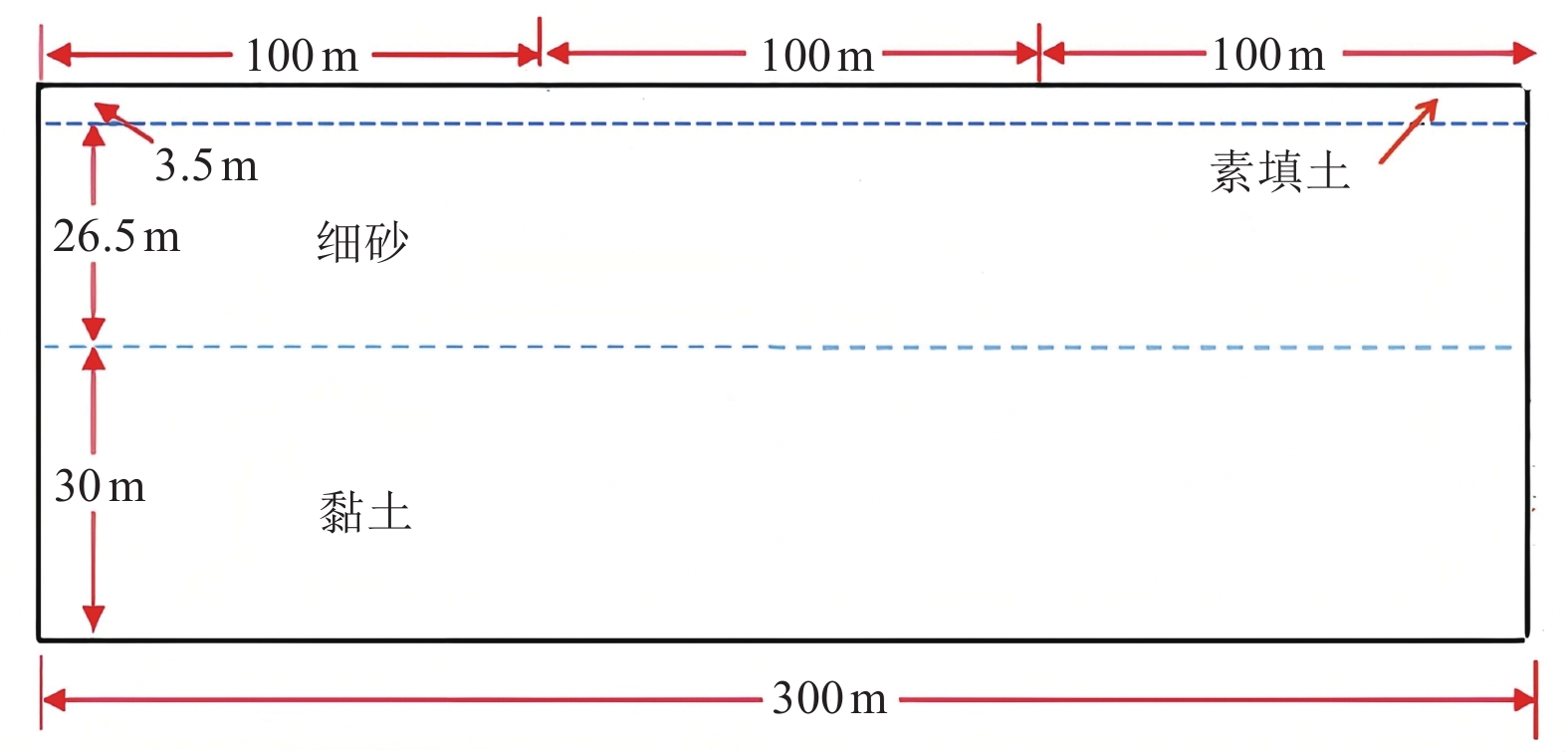
表 1 场地土体物理力学参数
Table 1. Site soil physical and mechanical parameters
土层 重度/(kN·m−3) 内摩擦角/(°) 弹性模量/MPa 泊松比 孔隙率 素填土 19.0 16 1.0 0.4 — 细砂 19.0 30 7.5 0.3 0.474 黏土 19.3 16 13.2 0.42 — -
陈少林,朱学江,赵宇昕等,2019. 考虑土骨架非线性的饱和土-结构相互作用分析. 地震工程与工程振动,39(1):114−127.Chen S. L., Zhu X. J., Zhao Y. X., et al., 2019. Analysis of saturated soil-structure interaction considering soil skeleton nonlinearity. Earthquake Engineering and Engineering Dynamics, 39(1): 114−127. (in Chinese) 程学磊,李文东,海然等,2022. 地震作用下饱和软土场地地下结构动力参数敏感性模拟分析. 广西大学学报(自然科学版),47(1):92−102.Cheng X. L., Li W. D., Hai R., et al., 2022. Parametric sensitivity simulation analyses of subway station structure surrounded by saturated soft soil foundation under earthquake. Journal of Guangxi University (Natural Science Edition), 47(1): 92−102. (in Chinese) 崔智谋,2012. 基于流固耦合动力模型的饱和两相介质-地下结构动力相互作用研究. 北京:北京工业大学.Cui Z. M., 2012. Study of dynamic interaction between fluid-saturated porous media of fluid-soild coupling model and underground structure. Beijing:Beijing University of Technology. (in Chinese) 丁伯阳,宋宥整,2019. 饱和土地下源u-P形式解答动力响应计算. 岩土力学,40(2):474−480.Ding B. Y., Song Y. Z., 2019. Dynamic response calculation for u-P solution in saturated soil subjected to an underground point source. Rock and Soil Mechanics, 40(2): 474−480. (in Chinese) 丁海滨,管凌霄,童立红等,2023. 基于非局部Biot理论的循环荷载下饱和土地基动力特性研究. 工程力学,40(3):141−152.Ding H. B., Guan L. X., Tong L. H., et al., 2023. On investigating the dynamic characteristics of saturated soil foundation subjected to cyclic load based on nonlocal Biot theory. Engineering Mechanics, 40(3): 141−152. (in Chinese) 谷音,庄舒曼,卓卫东等,2015. 考虑饱和土的地铁车站结构非线性地震反应研究. 岩土力学,36(11):3243−3251.Gu Y., Zhuang S. M., Zhuo W. D., et al., 2015. Analysis of nonlinear seismic response of subway station considering saturated soil. Rock and Soil Mechanics, 36(11): 3243−3251. (in Chinese) 李立云,2007. (准)饱和土与地下结构非线性动力相互作用问题研究. 北京:北京工业大学. 刘光磊,宋二祥,刘华北,2007. 可液化地层中地铁隧道地震响应数值模拟及其试验验证. 岩土工程学报,29(12):1815−1822. doi: 10.3321/j.issn:1000-4548.2007.12.012Liu G. L., Song E. X., Liu H. B., 2007. Numerical modeling of subway tunnels in liquefiable soil under earthquakes and verification by centrifuge tests. Chinese Journal of Geotechnical Engineering, 29(12): 1815−1822. (in Chinese) doi: 10.3321/j.issn:1000-4548.2007.12.012 刘华北,宋二祥,2005. 可液化土中地铁结构的地震响应. 岩土力学,26(3):381−386,391.Liu H. B., Song E. X., 2005. Earthquake induced liquefaction response of subway structure in liquefiable soil. Rock and Soil Mechanics, 26(3): 381−386,391. (in Chinese) 宋佳,2017. 饱和土场地波动数值模拟方法及其工程应用. 北京:北京工业大学.Song J., 2017. Wave numerical method of saturated site soil and its engineering application. Beijing:Beijing University of Technology. (in Chinese) 王相宝,2014. 基于流固耦合两相介质动力模型的饱和土体-地下结构体系近场波动问题研究. 北京:北京工业大学. 王子辉,2008. 饱和两相与单相土互层场地中地铁车站地震反应分析. 北京:北京交通大学.Wang Z. H., 2008. Seismic analysis of subway station in a site interbedded by saturated two-phase and single-phase soil. Beijing:Beijing Jiaotong University. (in Chinese) 许民泽,崔春义,李静波等,2021. 饱和砂土场地中地铁车站结构地震易损性分析. 工程力学,38(S1):251−258.Xu M. Z., Cui C. Y., Li J. B., et al., 2021. Seismic vulnerability analysis of subway station embedded in saturated sand layers. Engineering Mechanics, 38(S1): 251−258. (in Chinese) 杨军,宋二祥,陈肇元,2003. 饱和土一维简谐响应解析解的求解和应用:Ⅱ应用. 岩土力学,24(5):710−714. doi: 10.3969/j.issn.1000-7598.2003.05.008Yang J., Song E. X., Chen Z. Y., 2003. Application of analytical solution of 1-D harmonic response in saturated soil. Rock and Soil Mechanics, 24(5): 710−714. (in Chinese) doi: 10.3969/j.issn.1000-7598.2003.05.008 禹海涛,王治坤,刘中宪,2022. SV波入射下均匀饱和地层渗透系数对深埋隧道的影响机制. 岩土工程学报,44(2):201−211.Yu H. T., Wang Z. K., Liu Z. X., 2022. Influence mechanism of permeability coefficient in homogeneously saturated strata on responses of deep tunnels under incidence of SV waves. Chinese Journal of Geotechnical Engineering, 44(2): 201−211. (in Chinese) 袁宗浩,蔡袁强,曾晨,2015. 地铁列车荷载作用下轨道系统及饱和土体动力响应分析. 岩石力学与工程学报,34(7):1470−1479.Yuan Z. H., Cai Y. Q., Zeng C., 2015. Dynamic response of track system and underground railway tunnel in saturated soil subjected to moving train loads. Chinese Journal of Rock Mechanics and Engineering, 34(7): 1470−1479. (in Chinese) 周巧芳,2008. 饱和土中地铁车站的地震响应分析. 北京:北京交通大学. Biot M. A., 1956. Theory of propagation of elastic waves in a fluid-saturated porous solid. I. Low‐frequency range. The Journal of the Acoustical Society of America, 28(2): 168−178. doi: 10.1121/1.1908239 Li L., Jiao H. Y., Du X. L., et al., 2020. Fully fluid-solid coupling dynamic model for seismic response of underground structures in saturated soils. Earthquake Engineering and Engineering Vibration, 19(2): 257−268. doi: 10.1007/s11803-020-0560-3 Yang Z. H. , Lu J. C. , Elgamal A. , 2008. OpenSees soil models and solid-fluid fully coupled elements user’s manual. San Diego: University of California. Zhu J., Li X. J., Liang J. W., 2020. 3D seismic responses of a long lined tunnel in layered poro-viscoelastic half-space by a hybrid FE-BE method. Engineering Analysis with Boundary Elements, 114: 94−113. doi: 10.1016/j.enganabound.2020.02.007 Zienkiewicz O. C., 1982. Basic formulation of static and dynamic behaviours of soil and other porous media. Applied Mathematics and Mechanics, 3(4): 457−468. doi: 10.1007/BF01908222 期刊类型引用(3)
1. 熊政辉,曲哲,宁超列,卜春尧,尹建明,薄涛. 我国地震巨灾保险试点实践对比研究. 灾害学. 2025(01): 48-52+159 .  百度学术
百度学术2. 李云仙,子芝兰. 基于AHP-FCE的地震巨灾保险运行效果评价——以大理州政策性农房地震巨灾保险为例. 保险理论与实践. 2024(10): 33-55 .  百度学术
百度学术3. 景冰冰,施唯. 2023年土耳其M_S7.8地震灾区损失与保险研究. 震灾防御技术. 2023(03): 495-504 .  本站查看
本站查看其他类型引用(6)
-




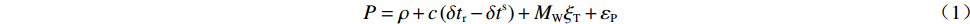
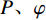
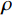
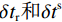
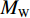
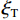
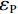
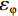
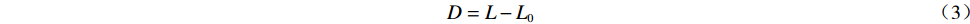
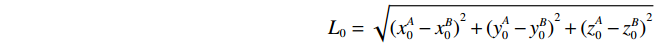
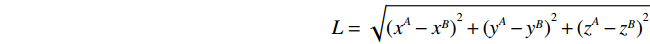

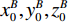
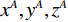
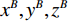
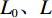
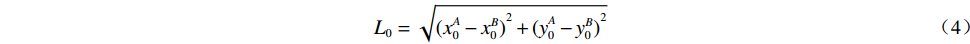
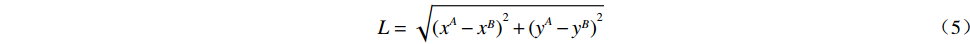
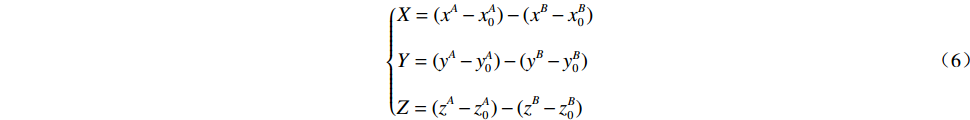

 下载:
下载:






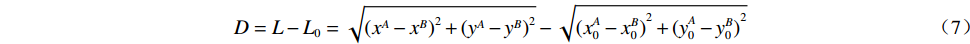
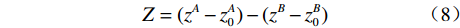







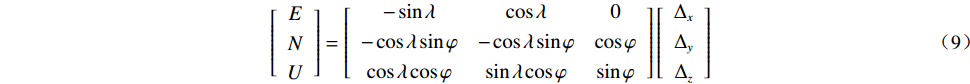
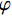





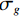

 下载:
下载: