Preliminary Study on Shallow Seismic Activity Model in Taiwan Region
-
摘要: 本文从中国台湾地区气象厅(CWB)网站收集到了1900—2022年ML2.0以上震源深度小于350 km的地震目录数据集,按照台湾地区更新完善后的地震目录(1900—2014)建议的方法,将该地震目录的地震震级统一为矩震级MW。以此地震目录数据集为基础分析台湾地区的地震活动特征,探讨台湾地区不同时期记录的完备性震级下限MC的历史沿革。本文采用目前国际比较流行的Gardner-Knopoff除丛方法删除了地震目录中的前震和余震,分别选用Gardner-Knopoff时空窗、Grünthal时空窗、Uhrhammer时空窗计算获得了相应的主震目录。结果表明,不同的除丛方法对地震目录影响显著。本文推荐使用Gardner-Knopoff除丛方法的Uhrhammer时空窗参数去除地震目录中的前震和余震事件,并建议在概率地震危险性分析中应该考虑由多种除丛方法引起的认知不确定性。文中采用Stepp(1971)方法对台湾地区的地震目录进行了完整性分析,根据各震级档地震平均发生率估计的方差偏离所绘制的预期回归线的时间,给出了各震级档的完整性时间表,避免了人为主观判断所引入的认知不确定性。最后,分别利用Weichert(1980)、Bender(1983)以及Kijko等(2012)的极大似然估计方法对台湾地区G-R关系中的活动性参数进行估算,获得了台湾地区的地震活动性参数值。本文的研究结果能够为后续台湾地区的概率地震危险性分析提供数据输入,为下一代区划图的编制提供地震活动参数计算方法和参考。Abstract: This paper compiles an earthquake catalogue dataset containing earthquakes with magnitudes above ML2.0 and focal depths less than 350 km, sourced from the Taiwan Meteorological Bureau (CWB) for the period from 1900 to 2022. All magnitudes in this catalogue are standardized to moment magnitudes (MW). Utilizing this dataset, we analyze the characteristics of seismic activity in Taiwan and discuss the historical evolution of the lower limit for completeness magnitude (MC) recorded during different periods. To refine the earthquake catalogue, we apply the widely used Gardner-Knopoff declustering method to remove foreshocks and aftershocks. Three time-distance window parameters—Gardner-Knopoff, Grünthal, and Uhrhammer—are employed to derive the corresponding mainshock catalogue through calculations. Our findings indicate that different declustering methods significantly impact the resulting earthquake catalogue. In this study, we recommend the Gardner-Knopoff declustering method combined with the Uhrhammer time-distance window parameters for effectively removing foreshock and aftershock events. We emphasize that epistemic uncertainty arising from various declustering approaches must be considered in probabilistic seismic hazard analyses. The completeness of the earthquake catalog in Taiwan is assessed using the Stepp (1971) method, which establishes completeness times for each magnitude bin based on the point at which the estimated variance of the average earthquake occurrence rate deviates from the expected regression line, thus minimizing subjective bias. Finally, we estimate the activity parameters of the Gutenberg-Richter (G-R) relationship in Taiwan using maximum likelihood estimation methods developed by Weichert (1980), Bender (1983), and Kijko et al., (2012). The results of this research provide valuable data inputs for future probabilistic seismic hazard analyses in Taiwan and serve as a reference for calculating seismic activity parameters for the upcoming seismic ground motion parameters zonation map of China.1)
1 2 中国地震动参数区划图编制组, 2011. 中国地震动参数区划图技术报告-地震活动性参数确定专题报告. -
引言
台湾位于环太平洋地震带西部,是世界上地震最活跃的地区之一。菲律宾板块沿着台湾岛以东的琉球海沟,向北俯冲至欧亚板块之下。中国南海板块位于台湾岛南端,是欧亚板块的一部分,向东俯冲到菲律宾海板块之下(Tsai等,1977)。俯冲过程始于晚中新世时期的弧-陆碰撞,造成了台湾地形高度变形。由此产生的应力在台湾岛上形成大量的褶皱和逆冲构造。逆冲和褶皱构造首先在台湾岛东北部形成,并逐渐延伸至中南部,表现为现今该地区地壳应力值高、构造变形速度快、地震活动频繁的特征。台湾岛北部的构造背景不同于中部、南部,直接与板块碰撞带相关,导致该地区地震活动相当频繁(Cheng等,2007)。因此,台湾岛经常发生灾难性地震。如1999年9月21日台湾省南投县集集镇发生了里氏7.6级地震,造成
2321 人死亡,39人失踪,8722 人受伤。2018年2月6日,台湾花莲近海发生里氏6.0级地震,造成4栋建筑物倒塌,4人死亡,225人受伤,173人失联。对台湾地震空间、时间和强度的地震活动性模型研究,可以为台湾地区的地震危险性和风险评估提供科学依据。早在1988年Chang等(1988)为研究台湾地区埋地管道的地震危险性和地震风险,使用1936—1985年的地震目录估算了各潜在震源区在此期间的最大震级和平均震源深度。由于没有低于4.3级的可靠历史地震记录,采用的地震震级下限为5.0级,运用最小二乘法方法估计了b值。Campbell等(2002)收集了1604—1999年之间发生的震级大于4级的1894次地震,利用推导出的ML和MW转换关系,将台湾地区的里氏震级ML转换为矩震级MW。而后基于除丛和完整性时间表修正的历史地震目录,利用最小二乘法确定了指数模型的震级-频率分布。台湾集集地震之后,Cheng等(2007)利用1900—1999年地震目录中的主震数据,评估了区域背景源和俯冲带板内源截断指数模型的地震复发率。Chen等(2013)利用1900—2010年震源深度小于40公里的地震事件,采用时空窗的方法删除了地震目录中的余震,并使用最大曲率方法评估了台湾气象厅(CWB)台网监测能力的最小完备震级MC。检验了地震事件时间分布模型,给出了台湾地区10年内发生7.0级以上地震的概率。李姜一等(2016)对1500年以来中国大陆东部102个中强潜在震源区内5~6级地震的复发间隔进行了统计分析,结果表明中强地震复发间隔不仅受潜源内断层活动性影响,还与发震构造是否交汇及邻区构造活动等因素有一定关系。
Wang等(2016)在研究台湾地震危险性模型(TEM PSHA2015)时,为避免地震活动性低的地区数据不足的问题,从全台湾MW>3.0的浅源背景地震中获得了描述大、小地震比例关系的b值,然后在28个潜源中统一使用估计的b值和各潜源对应的a值。Cheng等(2015)利用台湾气象厅1900—2010年台湾地区的地震目录,基于最小完备震级MC的时间分布,确定使用1900年以后M > 6.0和1973年后 M > 2.0地震目录用于后续分析。利用最大似然估计法,对除丛后的地震目录进行截断指数模型回归,得到了每个区域源的活动性参数。Chang等(2016)基于Chen等(2008)研究中1900—2006年MW震级的地震目录将台湾地区1900—2014年地震目录中的原始震级统一换算为与Harvard MW兼容的矩震级。Chan等(2020)在研究台湾地震危险性模型(TEM PSHA2020)时,以Wu等(2008)利用台湾气象厅地震台网(CWBSN)的记录重新定位的地震数据库为基础,将地震目录补充至1973—2016年M≥3.0,并用于后续的危险性分析。背景震源模型排除了震源深度大于30 km的地震,并根据除丛方法从目录中删除了前震和余震。但他们的研究没有考虑由各种除丛方法引起的认知不确定性。徐伟进等(2021)对中国海域及邻区地震时间分布特征的研究结果表明,地震活动存在长期记忆性。震级相对较小的地震(M<6)受更大地震的影响,从而在时间上表现出丛集特征。故需要把地震目录中与主震相关的前震、余震进行除丛。
本次研究从台湾气象厅网站收集到了1900—2022年ML>2.0、震源深度小于350 km的地震目录数据集,按照Chang等(2016)推荐的方法将该地震目录的地震震级统一为矩震级MW。进一步分析台湾地区地震活动的空间分布特征,探讨了台湾不同时期记录的地震震级下限。采用了目前国际上比较流行的Gardner-Knopoff除丛方法删除了地震目录中的前震和余震(Knopoff等,1972),然后利用Stepp(1971)方法对台湾地区的地震目录进行完整性分析,给出了3个不同时空窗的震级完整性时间表。最后,分别选用3种不同的极大似然估计方法对台湾地区G-R关系的活动性参数进行统计,给出了台湾地区震级-频度关系中的活动性参数值。本文的研究结果可为后续的概率地震危险性分析提供数据输入,为下一代区划图的编制中地震活动参数确定提供参考。
1. 地震目录数据
1.1 不同震级的转换
本次研究区域范围为21°00′N—26°00′N,119°00′E—123°00′E 。本研究使用的地震目录来源于中国台湾气象厅网站,地震目录的时间范围为1900年5月15日—2022年9月13日,震级范围为2~10级,震源深度范围为0~350 km。地震事件数目超过40万个,其中震源深度小于40 km的地震事件超过30万个。台湾地区的平均地壳厚度为40 km(Yeh等,1981;Lin,1996;Ustaszewski等,2012)。
台湾地区自1897年安装第一台地震仪以来,便开始了地震的定位工作。但由于地震特征、台网覆盖范围和观测方法的变化,震级的定义和计算方法也随之不断发生变化。本文采用了台湾地区更新完善后的地震目录(1900—2014),按照Chang等(2016)推荐的方法,对地震目录震级进行转换。对于1900—1972年的地震事件,台湾地震的震级MH是根据地震烈度数据确定的。利用台湾地区地震信息中心(NEIC)地震目录中常见的MH和面波震级MS之间的关系,结合MS和MW之间的关系,得到该时期各地震事件的矩震级MW。对于1973—1987年6月的地震事件,台湾地区地震的震级MD(A)是根据模拟地震记录测得的总信号持续时间确定的。在NEIC和台湾地震目录中列出的上述时间段内的地震中,我们找到了MD(A)和MW之间的转换关系。对于1987年6月—1991年2月的地震事件,震级 MD(D)是由数字地震记录上测量的总信号持续时间确定的。由于MD(D)和MW值的观测数据太少,故转换关系直接使用了与MD(A)和MW转换关系相同的斜率。对于1991年3月—2022年的地震事件,里氏震级ML是根据伍德安德森地震记录确定的。对于震级换算,本文使用了Chang等(2016)给出的ML与MW之间的转换关系。转换后的台湾地区MW>2且震源深度≤40 km的地震目录如图1所示。
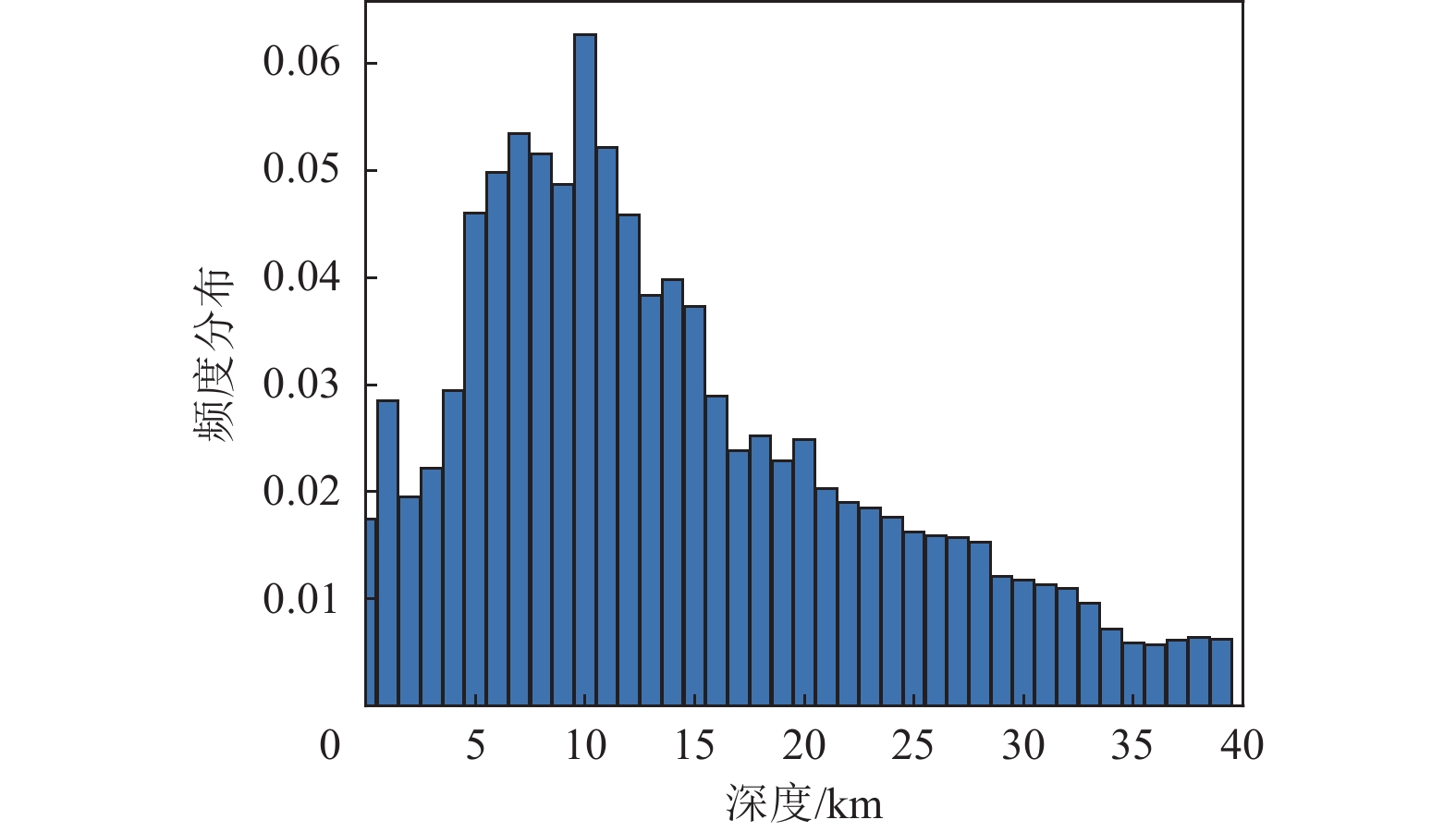
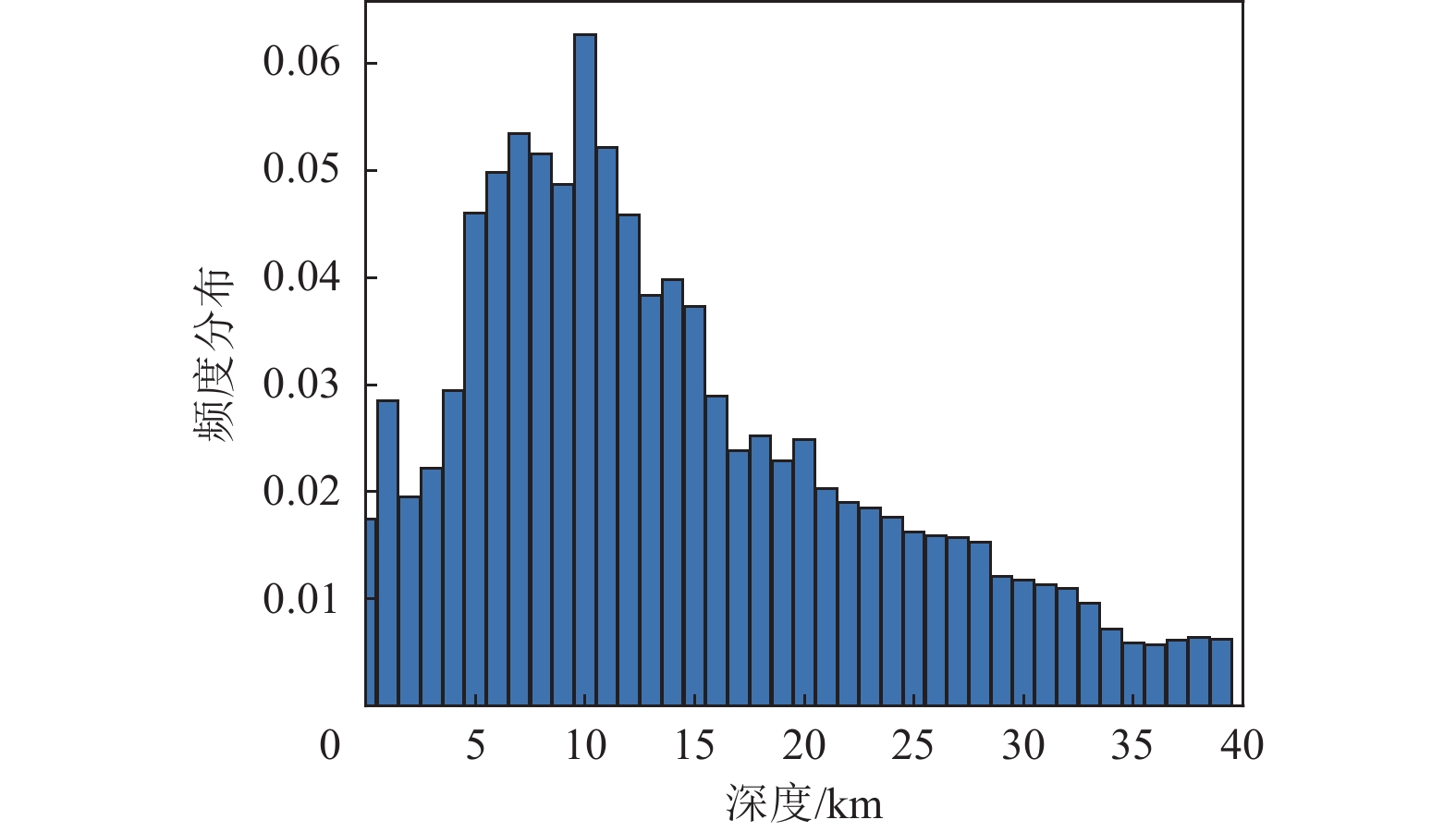
从图1可以看出,台湾地区的地震活动主要以台湾岛中央山脉为界,分布在山脉的东西两侧,地震活动有北强南弱、东强西弱的特点。台湾浅源地震的震源主要集中在5~20 km深度范围内(图2)。中央山脉以西的地震震中主要分布在台湾岛上,并以台中—南投—台南一带最为活跃。台湾主要以自东向西逆冲的北北东-近南北向推覆构造为主,强震多分布在山麓与平原交界的断裂附近,如集集地震发生在车笼埔断层上。中央山脉以东的地震则多发生于欧亚大陆与菲律宾海板块碰撞带上,且处于2个俯冲方向各自岛弧上不同的连结部位,因此中央山脉以东的地震活动非常频繁,并且该地区北部如宜兰—花莲及其近海的地震活动强度比南部的台东更为强烈。
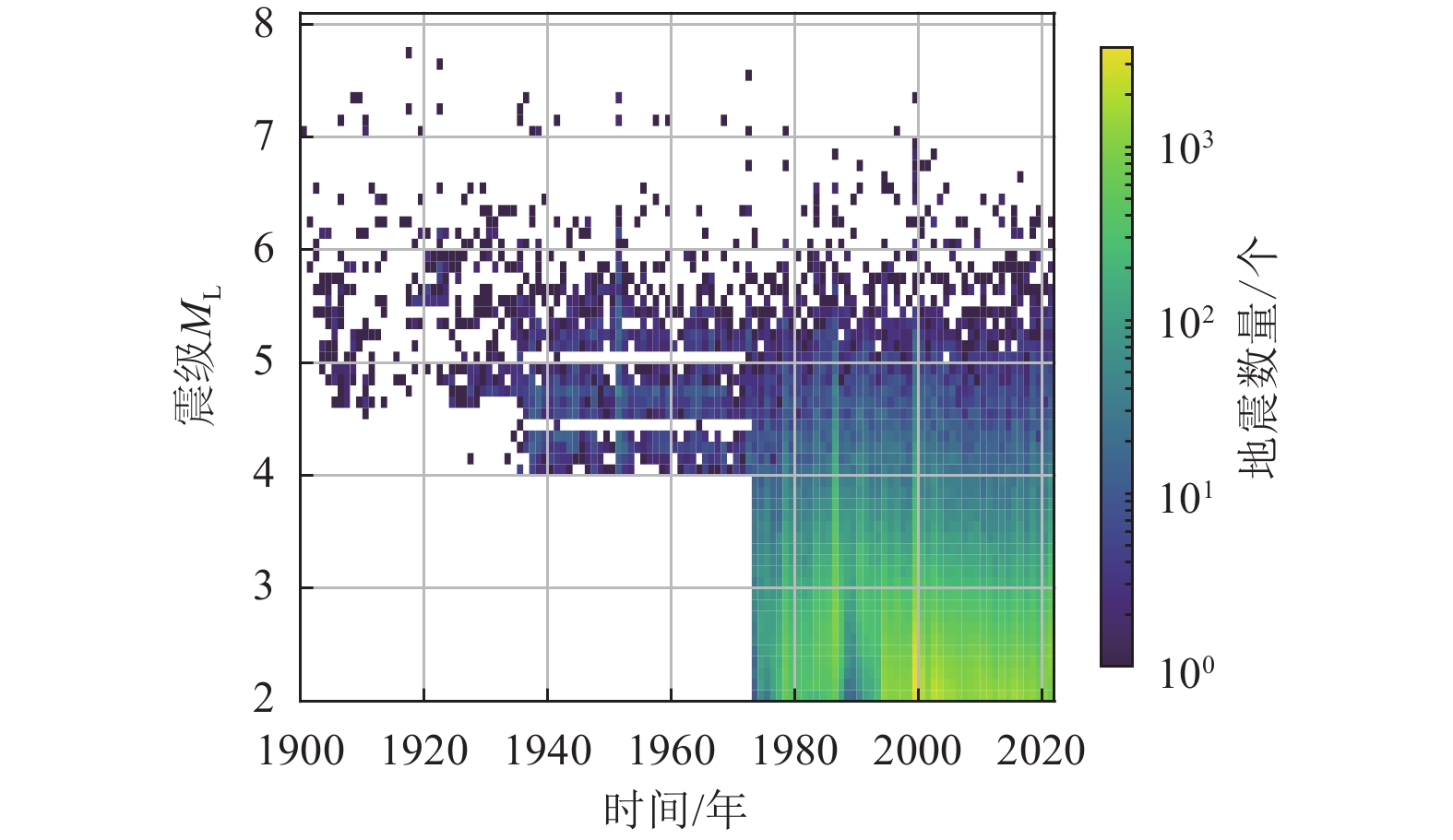
图3是台湾地区里氏震级ML≥2的浅源地震震级-发震时间密度分布图。1900—1940年左右,主要记录M >4.5的地震,1940—1973年左右收录的地震目录震级下限提升至M4.0。1973年台湾遥测地震台网(TTSN)建立以后,已经能够记录M >2的地震。不过直到1990年,对M≥2.5地震的记录才保持稳定(Chen等,2013)。这主要是因为1994年后,台站的记录方式由触发记录模式转变为连续记录模式(Wu等,2008)。
2. 地震除丛
地震危险性分析中的一个常用假设是地震发生的次数可以用泊松过程较好地描述 (Cornell,1968)。事件的发生率是恒定的,不依赖于空间或时间上邻近的其他事件的发生是泊松过程的基本性质,换句话说,地震事件是相互独立的。对地震目录进行除丛的目的是去除与主震相关地震事件,以便估算出一段时间内的地震发生率。
为使地震活动与泊松过程的假设相一致,通常会删除与主震相关的地震事件,即前震和余震。本文采用目前国际上比较流行的Gardner-Knopoff除丛方法,选用Gardner-Knopoff时空窗(Knopoff等,1972)、Grünthal时空窗(Van Stiphout等,2012)、Uhrhammer时空窗(Uhrhammer,1986)删除了地震目录中的前震和余震,为便于描述,3个时空窗分别简称为“Gk时空窗”、“Gr时空窗”和“Uh时空窗”。本次研究中搜索前震和余震的时空窗大小设置相同。台湾地区浅源地震目录除丛结果如图4所示。
Gk时空窗是应用最广泛的地震除丛算法,利用与主震震级成固定比例的时间-距离窗口来识别前震和余震,如式(1)、式(2)所示。
$$ d=10^{0.1238M+0.983} $$ (1) $$ t=\left\{\begin{aligned} & 10^{0.0320M+2.7389}\quad,M\geqslant6.5 \\ & 10^{0.5409M-0.5470}\quad,M < 6.5\end{aligned}\right.$$ (2) Gr 时空窗计算公式如式(3)、式(4)所示。
$$ d=\mathrm{e}^{1.77+\sqrt{0.037+1.02M}} $$ (3) $$ t=\left\{\begin{aligned} & 10^{0.024M+2.8}\quad,M\geqslant6.5 \\ & \mathrm{e}^{-3.95+\sqrt{0.62+17.32M}}\quad,M < 6.5\end{aligned}\right. $$ (4) Uh时空窗计算公式如式(5)、式(6)所示。
$$ {d}={\mathrm{e}}^{-1.024+0.804M} $$ (5) $$ {{t}=\mathrm{e}}^{-2.87+1.235M}$$ (6) 式中,d表示距离;t表示时间;M表示地震震级。
3. 地震目录的完整性
不完整的地震目录会导致确定的地震复发模型产生偏差,可能对场点的地震危险性估计产生严重影响。因此,识别地震目录震级的完整性是处理地震危险性分析输入数据的明确要求。地震样本完整性是根据地震目录的统计特性定义的完整性,而不是“基于概率的完整性”,后者主要是指基于特定地震台网的探测概率(Schorlemmer等,2008)。本文采用Stepp(1971)方法对台湾地区的地震目录进行完整性分析。
在Stepp(1971)方法中,地震按不同的震级区间分组,每个震级区间被建模为时间上的一个点过程。利用统计估计的基本原理,即统计样本均值的方差与样本中观测值的数量成反比。在数据报告时间完整且过程平稳(每个时刻观测值的均值、方差与其他时刻的相同)的情况下,如果观测数据足够多,方差就可以尽可能小。地震序列用泊松分布来模拟,假设k1, k2, ···, kn为各单位时间间隔内的地震次数,则该样本单位时间间隔内的平均发生率的无偏估计为:
$$ \lambda {\text{ = }}\frac{1}{n}\sum\limits_{i = 1}^n {{T_i}} $$ (7) 式中,λ为单位时间间隔内的地震平均发生率;Ti是观测的时间间隔。λ的方差
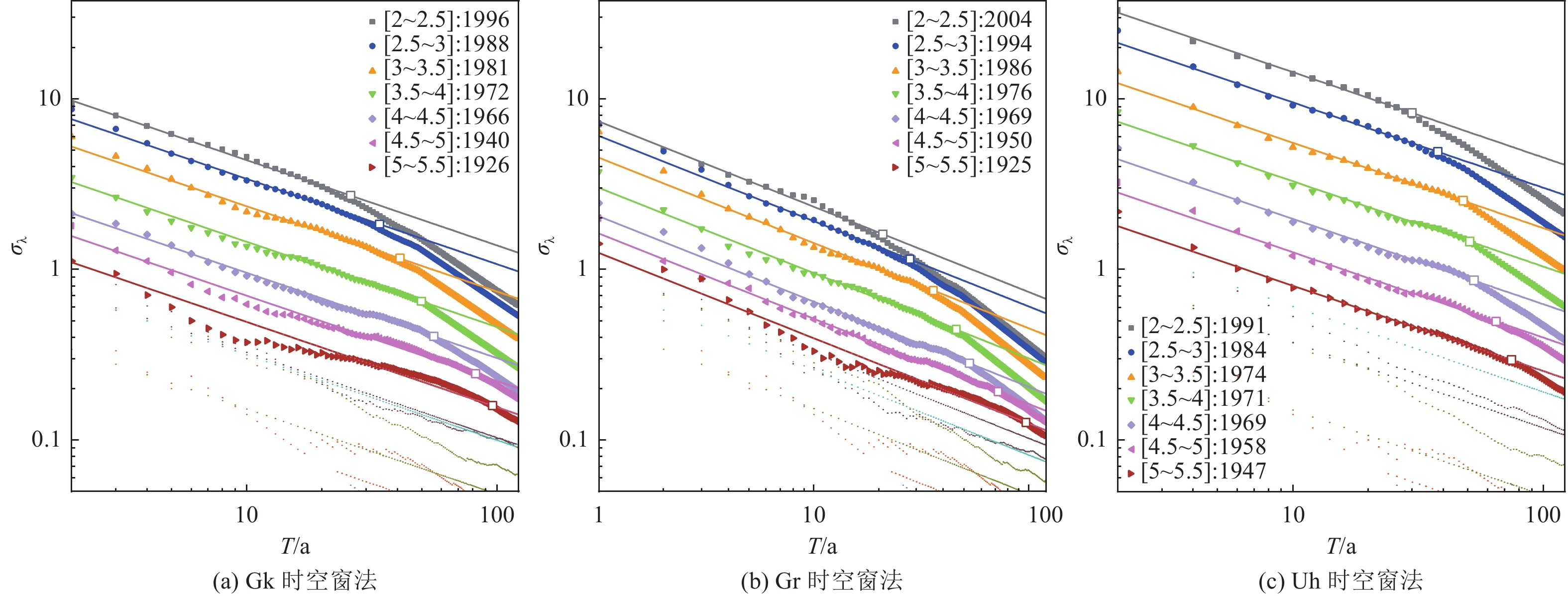
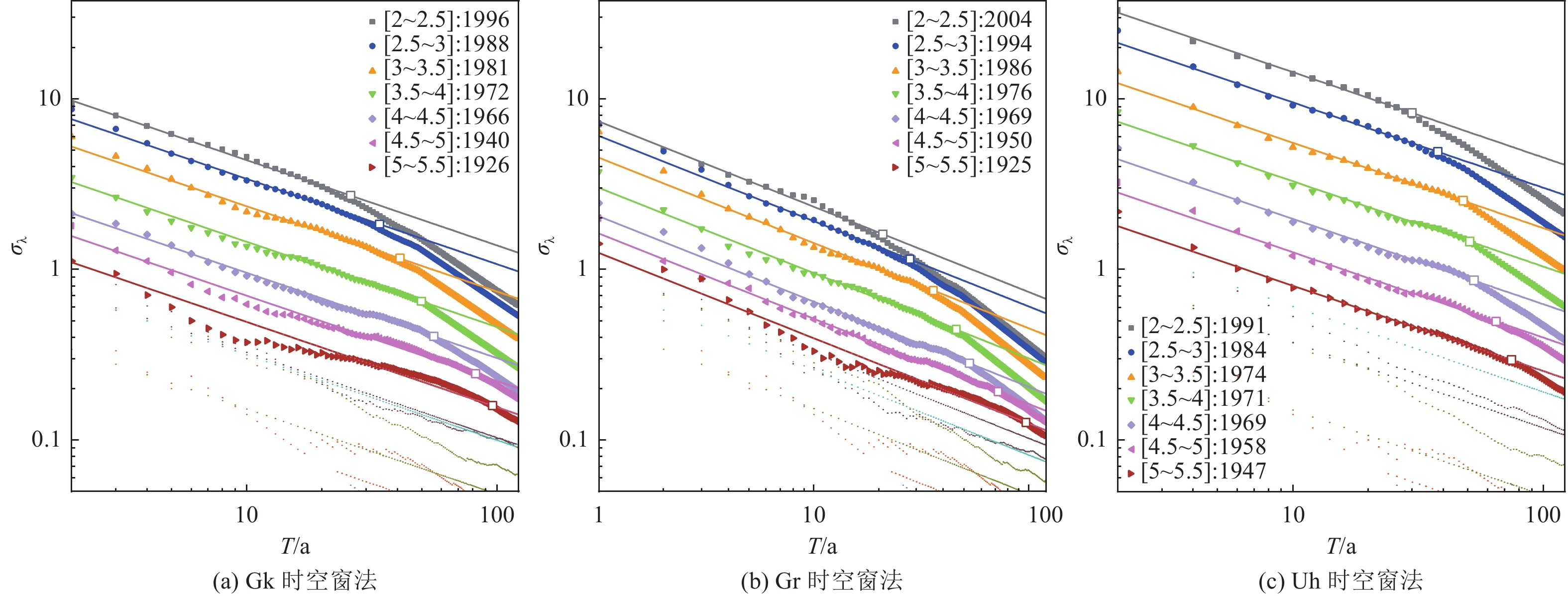
$ {\sigma }_{{ \lambda }}^{\text{2}} $ = λ/T (T为观测周期)。假设时间完整,在一个震级区间内的地震平均发生率就是恒定的,则${\sigma }_{{ \lambda }} $ 与统计时长在给定地震目录样本中表现为斜率1/$ \sqrt{{T}} $ 的线性关系。方差偏离所绘制的预期回归线时,完整性时间范围将被识别出来。基于台湾地区的地震目录,利用Stepp(1971)方法,分别对Gk、Gr、Uh时空窗除丛后的地震目录进行完整性分析,结果如表1和图5所示。由图5可以直观地识别出相应震级的完整性时间范围,以此来估计不同震级区间地震的完整性时间表。
表 1 不同除丛时空窗方法计算得到的台湾地区完整性时间表Table 1. Integrity schedule in Taiwan calculated by different declustering process震级档 完整性起始年份 Gk时空窗 Gr时空窗 Uh时空窗 2.0~2.5 1996 2001 1991 2.5~3.0 1988 1994 1984 3.0~3.5 1981 1986 1974 3.5~4.0 1972 1976 1971 4.0~4.5 1966 1969 1969 4.5~5.0 1940 1950 1958 5.0~5.5 1926 1925 1947 4. 地震活动性参数统计
Gutenberg等(1944) 对全球和区域的地震数据进行统计分析后,提出了地震震级与大于给定震级的地震次数存在对数线性关系(G-R关系)。G-R关系在实际工程应用中符合双截断的指数分布,其概率密度函数表示为:
$$ {f_{\mathrm{M}}}(m) = \frac{{\beta \exp \left[ { - \beta (m - {m_{\min }})} \right]}}{{1 - \exp \left[ { - \beta ({m_{\max }} - {m_{\min }}} \right]}} $$ (8) 式中,mmin是与特定工程应用相关的最小震级;mmax表示所考虑的震源或区域物理上可能出现的最大震级。
震级-频度分布通常是根据大于给定震级地震的发生率来定义的。由于给定震级的不同,计算大于该震级的地震发生率时通常会重复使用一些观测数据,因此这些震级的发生率并不是相互独立的。这种依赖关系意味着震级-频度分布的参数不能使用最小二乘法和L2范数优化方法进行估计。本文采用了3种极大似然估计方法对台湾地区的G-R关系活动性参数进行统计,具体如下:
(1) Bender(1983)极大似然估计法
该方法调整了Aki(1965)和Bender(1983)方法,在完整性上考虑了时间变化。将用于活动性分析的地震目录分为s个子目录,其中每个子目录对应一个相对完整的时间范围。对每个子目录的a、b值按子目录中的事件数加权平均,则可以得到平均的a和b值。
(2) Kijko等(2012)极大似然估计法
该方法是对Aki(1965)估计方法的改进,主要对包含不同完整性时间范围的地震目录 b 值进行估计。该方法将地震目录划分为s个完备性震级档的子目录,其中第i个完备性震级档由ni个地震事件组成,则β的似然函数如下:
$$ {\boldsymbol{L}} = \prod\limits_{i = 1}^s {\prod\limits_{j = 1}^{{n_i}} {\beta \exp \left(\left[ { - \beta (m_j^i - m_{\min }^i)} \right]\right)} } $$ (9) β的最大似然估计为:
$$ \beta = \left(\frac{{{r_1}}}{{{\beta _1}}} + \frac{{{r_1}}}{{{\beta _1}}} + \cdots + \frac{{{r_s}}}{{{\beta _s}}}\right)^{ - 1} $$ (10) 式中,ri =
$ \dfrac{{{n}}_{{i}}}{{n}} $ ,n =$ \displaystyle\sum\limits_{i = 1}^s {{n_i}} $ 。(3)Weichert(1980)极大似然估计法
该方法提出了针对地震目录不同震级档完整性时间表的最大似然估计方法。该方法的最大似然函数如下:
$$ {\boldsymbol{L}}(\beta |{n_i},{m_i},{t_i}) = \frac{{N!}}{{\displaystyle\prod\nolimits_i {{n_i}!} }}\prod\limits_i {p_i^{{n_i}}} $$ (11) 式中,L是β的似然估计值;ni是观测周期ti内震级mi的地震数量。参数p定义为:
$$ {p_i} = \frac{{{t_i}\exp ( - \beta {m_i})}}{{\displaystyle\sum\nolimits_j {{t_j}\exp ( - \beta {m_j})} }} $$ (12) ln(L)的极值由下式计算:
$$ \mathrm{ln}\left({{\boldsymbol{L}}}\right)=\frac{\displaystyle\sum {t}_{i}{m}_{i}\mathrm{e}\mathrm{x}\mathrm{p}\left(-\beta {m}_{i}\right)}{{\displaystyle\sum\nolimits _j{{t_j}\exp ( - \beta {m_j})} }} $$ (13) Weichert(1980)最大似然估计地震活动性参数的算法,在大多数应用中已被证明是有效的、无偏的,并且该算法在许多国家的地震危险性模型分析中得到了广泛应用(Petersen等,2008,2020)。
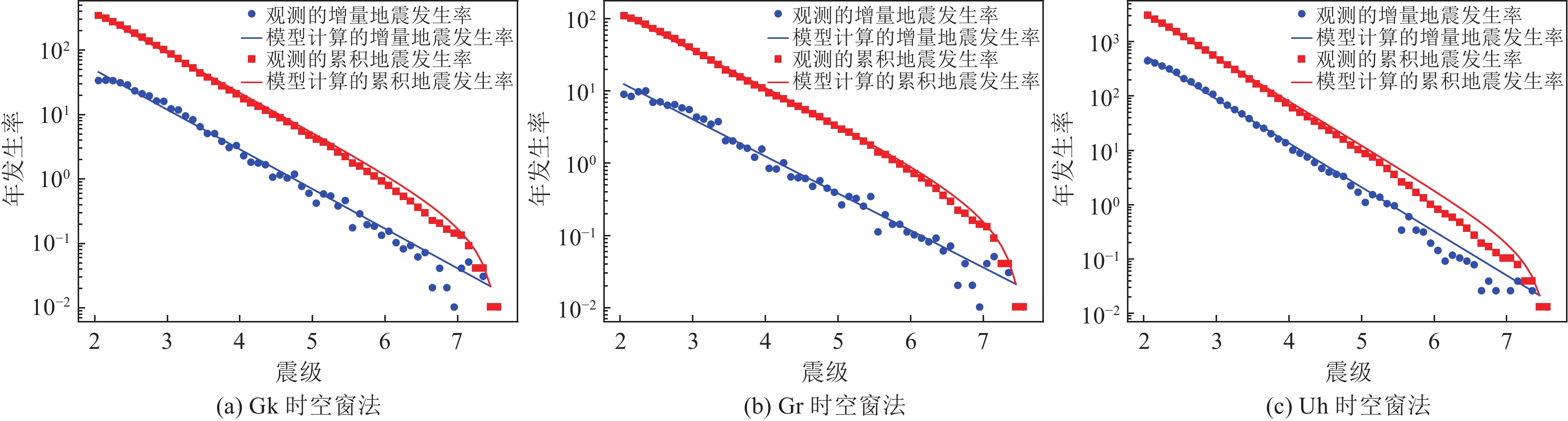
本文采用了3种极大似然估计方法,对3种除丛时空窗方法得到的台湾地区地震目录G-R关系活动性参数进行统计。此处仅给出Weichert(1980)极大似然估计方法对3种除丛时空窗方法得到的台湾地区地震目录的G-R关系活动性参数统计图(表2、图6)。
表 2 不同极大似然估计方法计算的台湾地区地震活动性参数Table 2. Seismic activity parameters calculated by different maximum likelihood estimation methods5. 比较与讨论
本文采用Gardner-Knopoff除丛方法从地震目录中删除了前震和余震,并选用3个时空窗进行地震目录的除丛,所获得的地震目录结果存在显著差别。针对本文的地震目录,原始地震目录中包含426 049个地震事件,利用Uh时空窗除丛后,剩下126 826个主震事件,其它删除前震、余震的方法具有较强的侵略性,中强地震删除的比例较大。Chen(2013)利用1900—2010年震源深度<40 km的地震目录,采用时空窗法删除目录中的余震,认为震级≥4.5的地震可以被认定为主震。将时空窗参数t和d分别固定为3 d和5 km,当其余地震事件的震中和发生时间在主震的时空窗口内时,震级≥4.5级的地震可以被认定为主震。删除由M≥4.5的主震产生的余震事件后剩余364 308个主震。Cheng等(2015) 利用Wyss(1979)、Arabasz等(1976)、Gardner-Knopoff(1972)和Uhrhammer(1986)开发的时空窗对地震目录进行除丛,结果表明如果地震事件满足4种除丛方法中的2种及以上,则可将其视为前震或余震。Chan等(2020)在研究台湾地震危险性模型(TEM PSHA2020)的背景地震时,没有考虑由各种除丛方法引起的认知不确定性,只根据Gardner-Knopoff除丛方法从地震目录中删除了前震和余震,所获得的地震目录直接用于后继的概率地震危险性分析。
在地震目录完整性分析中,我们使用Stepp(1971)方法估计其完整性性。Chen等(2013)使用最大曲率方法评估了台湾气象厅地震目录的完整性时间表,他们认为1973年之前完备震级下限MC值较高,在4.3~4.8级之间。直到台湾遥测地震台网(TTSN)建立后,MC下降到2.0~3.0级之间。本文基于3个不同的时空窗除丛后的地震目录样本,根据相应震级档地震平均发生率估计的方差偏离所绘制的预期回归线时间,给出了不同震级相应的完整性时间表,避免了人为主观判断引起认知不确定性。本文得到的4.0~4.5级地震完整性时间(特别是Gr和Uh时空窗)与Chen等(2013)评估结果比较一致。细微的差别主要归因于本文不但删除了余震,同时还删除了前震,同时使用了随震级变化的时空窗参数,大于Chen等(2013)使用的时空窗参数,因此本文除丛后的地震目录条目较少,完整性时间相对较早。
本文基于相应的完整性时间表,采用了3种极大似然估计方法对不同除丛方法得到的台湾地区地震目录进行活动性参数统计回归。基于Uh时空窗除丛法得到的地震目录活动性参数统计结果,在震级小于5.5级时较基于其它2种时空窗的统计结果相对更稳定。而Gr和Gk时空窗删除中强地震过多,进而表现为地震的年发生率下降(a值减小),大震的比重增加(b值减小)。将基于Uh时空窗除丛地震目录的3种最大似然法统计的地震活动性参数结果,与台湾地区不同时期浅层背景地震活动性参数结果进行比较。Chang等(1988)选用线性最小二乘法分3个区(台湾东北部、东部和西部地区)进行统计,b值分别为1.284、0.947和1.411,相较于本文研究的b值结果偏大,可能与其使用的地震目录时间较短、拟合的震级下限(5.0级)较高且拟合方法的差异有关。Chen(2013)利用1900—2010年浅源地震目录,统计给出的b值结果为0.908,稍大于本文b值结果。我国第五代地震动参数区划图中利用地震资料统计的台湾东、西部地震统计区多方案b值结果均值
1 分别为0.791和0.868,与本文结果比较接近。Cheng等(2015)利用最大似然估计对除丛地震目录进行回归分别获得21个背景源截断指数模型的b值范围为0.564~1.119,台湾地震危险性模型(TEM PSHA2020)的21个浅层背景源b值统一取1.10,接近Cheng等(2015)21个背景源b值的上限,而本文的结果相当于全台湾地区的平均水平。6. 结论
本文从台湾气象厅网站收集了1900—2022年ML2.0以上、震源深度小于350 km的地震目录数据,利用震级转换关系将台湾地区的地震目录震级统一为矩震级MW。采用目前国际比较流行的Gardner-Knopoff除丛方法的3种时空窗删除了地震目录中的前震和余震,并采用Stepp(1971)方法对台湾地区的地震目录进行完整性检验,给出了相应的震级完整性时间表。最后,利用3种极大似然估计方法对台湾地区G-R关系的活动性参数进行统计,得到以下结论:
(1)概率地震危险性模型假定地震的发生遵循泊松过程,地震之间是相互独立的。使用除丛后的地震目录进行活动性参数统计是至关重要的,但是不同的除丛方法对地震目录的影响显著。本文推荐使用Gardner-Knopoff除丛方法Uh时空窗删除地震丛聚,建议概率地震危险性模型应该考虑由各种除丛方法引起的认知不确定性。
(2)利用Stepp(1971)方法进行了各震级档的完整性分析,根据各震级档地震的平均发生率估计的方差偏离所绘制的预期回归线的时间,给出了不同震级档相应的完整性时间表,避免了人为主观判断造成的认知不确定性。
(3)震级-频率分布通常是根据累计频度定义的,多个震级值的累计频度将共享一些观测数据,因此不同的震级频度关系不是独立的。这种依赖关系意味着震级-频度分布参数不适合用最小二乘法进行估计,应该选用极大似然估计方法。
致谢 本研究使用的地震目录数据来源于台湾气象厅网站,部分地震活动性分析工具使用了GEM的HMKT软件包,在此表示衷心感谢!
-
表 1 不同除丛时空窗方法计算得到的台湾地区完整性时间表
Table 1. Integrity schedule in Taiwan calculated by different declustering process
震级档 完整性起始年份 Gk时空窗 Gr时空窗 Uh时空窗 2.0~2.5 1996 2001 1991 2.5~3.0 1988 1994 1984 3.0~3.5 1981 1986 1974 3.5~4.0 1972 1976 1971 4.0~4.5 1966 1969 1969 4.5~5.0 1940 1950 1958 5.0~5.5 1926 1925 1947 表 2 不同极大似然估计方法计算的台湾地区地震活动性参数
Table 2. Seismic activity parameters calculated by different maximum likelihood estimation methods
-
李姜一,周本刚,2016. 中国东部中强地震潜在震源区地震复发概率分布. 震灾防御技术,11(1):1−10. doi: 10.11899/zzfy20160101Li J. Y., Zhou B. G., 2016. Recurrence interval probability distribution of moderate-strong earthquakes in potential seismic source of east China. Technology for Earthquake Disaster Prevention, 11(1): 1−10. (in Chinese) doi: 10.11899/zzfy20160101 徐伟进,李雪婧,谢卓娟等,2021. 中国海域及邻区地震时间分布特征研究. 震灾防御技术,16(1):39−50.Xu W. J., Li X. J., Xie Z. J., et al., 2021. Temporal distribution characteristics of earthquakes in the China sea and adjacent areas. Technology for Earthquake Disaster Prevention, 16(1): 39−50. (in Chinese) Aki K., 1965. Maximum likelihood estimate of b in the formula logN=a-bM and its confidence limits. Bulletin of the Earthquake Research Institute, 43(2): 237−239. Arabasz W. J., Robinson R., 1976. Microseismicity and geologic structure in the northern South Island, New Zealand. New Zealand Journal of Geology and Geophysics, 19(5): 569−601. doi: 10.1080/00288306.1976.10426309 Bender B., 1983. Maximum likelihood estimation of b values for magnitude grouped data. Bulletin of the Seismological Society of America, 73(3): 831−851. doi: 10.1785/BSSA0730030831 Campbell K. W., Thenhaus P. C., Barnhard T. P., et al., 2002. Seismic hazard model for loss estimation and risk management in Taiwan. Soil Dynamics and Earthquake Engineering, 22(9-12): 743−754. doi: 10.1016/S0267-7261(02)00095-7 Chan C. H., Ma K. F., Shyu J. B. H., et al., 2020. Probabilistic seismic hazard assessment for Taiwan: TEM PSHA2020. Earthquake Spectra, 36(S1): 137−159. Chang S. Y., Loh C. H., Chen S. S., 1988. Distribution of seismic hazard and risk analysis of buried pipelines in Taiwan. Journal of the Chinese Institute of Engineers, 11(1): 11−23. doi: 10.1080/02533839.1988.9677037 Chang W. Y. , Chen K. P. , Tsai Y. B. , 2016. An updated and refined catalog of earthquakes in Taiwan (1900-2014) with homogenized M w magnitudes. Earth, Planets and Space, 68 (1): 45. Chen C. H., Wang J. P., Wu Y. M., et al., 2013. A study of earthquake inter-occurrence times distribution models in Taiwan. Natural Hazards, 69(3): 1335−1350. doi: 10.1007/s11069-012-0496-7 Chen K. P., Tsai Y. B., 2008. A catalog of Taiwan earthquakes (1900-2006) with homogenized Mw magnitudes. Bulletin of the Seismological Society of America, 98(1): 483−489. doi: 10.1785/0120070136 Cheng C. T., Chiou S. J., Lee C. T., et al., 2007. Study on probabilistic seismic hazard maps of Taiwan after Chi-Chi earthquake. Journal of Geoengineering, 2(1): 19−28. Cheng C. T. , Hsieh P. S. , Lin P. S. , et al. , 2015. Probability seismic hazard mapping of Taiwan. In: Beer M. , Kougioumtzoglou I. A. , Patelli E. , et al. , eds. , Encyclopedia of Earthquake Engineering. Berlin: Springer, 1−25. Cornell C. A., 1968. Engineering seismic risk analysis. Bulletin of the Seismological Society of America, 58(5): 1583−1606. doi: 10.1785/BSSA0580051583 Gutenberg B., Richter C. F., 1944. Frequency of earthquakes in California. Bulletin of the Seismological society of America, 34(4): 185−188. doi: 10.1785/BSSA0340040185 Kijko A., Smit A., 2012. Extension of the Aki‐Utsu b‐value estimator for incomplete catalogs. Bulletin of the Seismological Society of America, 102(3): 1283−1287. doi: 10.1785/0120110226 Knopoff L., Gardner J. K., 1972. Higher seismic activity during local night on the raw worldwide earthquake catalogue. Geophysical Journal International, 28(3): 311−313. doi: 10.1111/j.1365-246X.1972.tb06133.x Lin C. H., 1996. Crustal structures estimated from arrival differences of the first P-waves in Taiwan. Journal of the Geological Society of China, 39(1): 1−12. Petersen M. D. , Frankel A. D. , Harmsen S. C. , et al. , 2008. Documentation for the 2008 update of the United States National Seismic Hazard Maps. Reston: U. S. Geological Survey, 20. Petersen M. D., Shumway A. M., Powers P. M., et al., 2020. The 2018 update of the US National Seismic Hazard Model: Overview of model and implications. Earthquake Spectra, 36(1): 5−41. doi: 10.1177/8755293019878199 Schorlemmer D., Woessner J., 2008. Probability of detecting an earthquake. Bulletin of the Seismological Society of America, 98(5): 2103−2117. doi: 10.1785/0120070105 Stepp J. C. , 1971. An investigation of earthquake risk in the Puget Sound area by use of the type I distribution of largest extremes. Pennsylvania: The Pennsylvania State University. Tsai Y. B., Teng T. L., Chiu J. M., et al., 1977. Tectonic implications of the seismicity in the Taiwan region. Memoir of the Geological Society of China, (2): 13−41. Uhrhammer R. A., 1986. Characteristics of northern and central California seismicity. Earthquake Notes, 57(1): 21. Ustaszewski K., Wu Y. M., Suppe J., 2012. Crust–mantle boundaries in the Taiwan–Luzon arc-continent collision system determined from local earthquake tomography and 1D models: implications for the mode of subduction polarity reversal. Tectonophysics, 578: 31−49. doi: 10.1016/j.tecto.2011.12.029 Van Stiphout T. , Zhuang J. C. , Marsan D. , 2012. Seismicity declustering, community online resource for statistical seismicity analysis. 1-25. doi: 10.5078/corssa-52382934. http://www.corssa.org . Wang Y. J. , Chan C. H. , Lee Y. T. , et al. , 2016. Probabilistic seismic hazard assessment for Taiwan. Terrestrial, Atmospheric and Oceanic Sciences, 27 (3): 325−340. Weichert D. H., 1980. Estimation of the earthquake recurrence parameters for unequal observation periods for different magnitudes. Bulletin of the Seismological Society of America, 70(4): 1337−1346. doi: 10.1785/BSSA0700041337 Wu Y. M., Chen C. C., Zhao L., et al., 2008. Seismicity characteristics before the 2003 Chengkung, Taiwan, earthquake. Tectonophysics, 457(3-4): 177−182. doi: 10.1016/j.tecto.2008.06.007 Wyss M., 1979. Estimating maximum expectable magnitude of earthquakes from fault dimensions. Geology, 7(7): 336−340. doi: 10.1130/0091-7613(1979)7<336:EMEMOE>2.0.CO;2 Yeh Y. H. , Tsai Y. B. , 1981. Crustal structure of central Taiwan from inversion of P-wave arrival time. Bulletin of the Institute of Earth Sciences, Academia Sinica, 1 : 83−102. -





 下载:
下载:



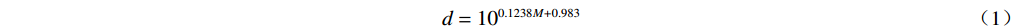
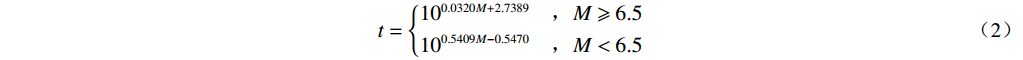
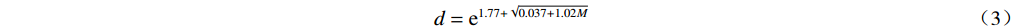
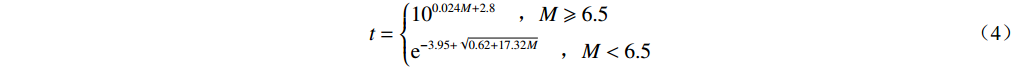
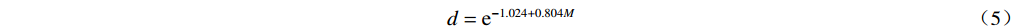
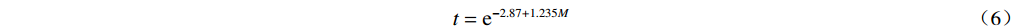
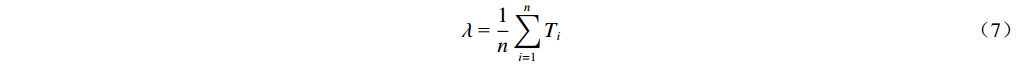
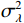
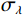
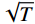

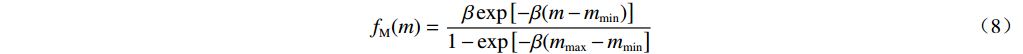
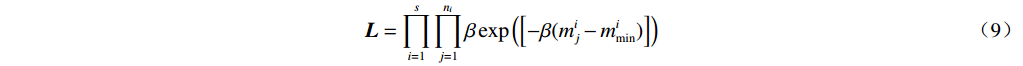
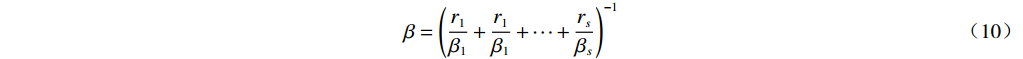
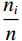
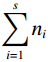
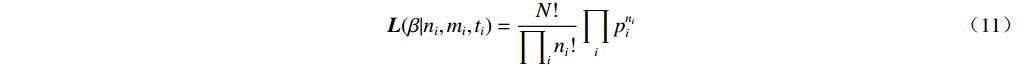
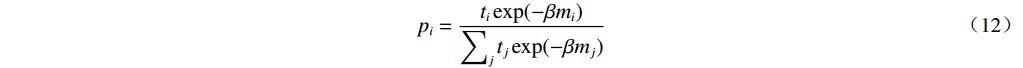
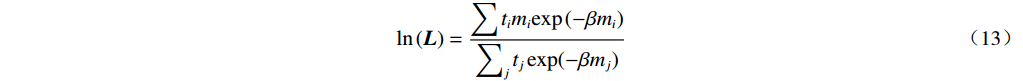


 下载:
下载: