Inversion of Site Velocity Structure Based on Near-field Seismic Records at Single Station
-
摘要: 基于近场加速度记录反演场地浅部速度结构，可为补充强震台站场地信息提供一种简便的途径，有利于记录的充分利用。本文详细推导了基于单一台站记录的P波地震图法，分析了其中的反演目标函数；通过数值模拟大量近场场地上的理论地震图，分析了场地覆盖层剪切波速、厚度等特征参数及震源持时对PS波与P波延时的影响，给出了地震记录的筛选原则；通过增加P波初到时刻径向分量与竖向分量幅值比函数作为反演目标函数，对单台地震图法进行改进；最后，利用汶川地震主震及余震记录，反演了武都强震台的场地波速结构，并与表面波法和钻孔法进行对比，结果表明，改进的单台地震图法搜索能力得到了增强，反演效率和精度得到显著提高。Abstract: The P-wave seismogram method offers a fast and convenient approach to investigating the velocity structure at seismic stations using near-field strong aftershock records, thus maximizing the utility of these data. This method was thoroughly derived, and its inversion objective function was analyzed. Through numerical simulations of theoretical seismograms from multiple near-field sites, the impact of site characteristic parameters and seismic source duration on the delay between the PS-wave and P-wave was examined. The applicability of the seismic records was also assessed. To improve the single-station seismogram method, the amplitude ratio between the initial vertical and radial components at the first arrival of the P-wave was incorporated as an additional inversion objective function. The improved method was then applied to invert the S-wave velocity structure at the Wudu station using mainshock and aftershock records from the Wenchuan earthquake. Comparisons with the surface wave method and the borehole method demonstrate that the enhanced single-station seismogram method improved search capability, as well as inversion efficiency and accuracy.
-
Key words:
- Shear-wave velocity /
- P-wave seismograms method /
- Seismic station /
- Inversion
-
引言
建筑结构震害预测是地震工程学科的重要内容之一（于天洋等，2018；贾晗曦等，2019），其研究方法主要分为专家经验法、模糊类比法和基于机器学习相关算法组成的方法。其中，多层砖房作为国内量大面广的结构形式之一，许多学者对其进行震害预测研究，如尹之潜等（1991）基于易损性概率分析方法得到震害等级与平均折算抗剪强度的关系；欧盛（2011）基于最小二乘法对尹之潜方法中的修正系数进行修正；刘章军等（2007）使用模糊数学理论对多层砖房进行震害预测；部分学者基于反向传播神经网络（刘本玉等，2002；姜伟等，2011）和蚁群聚类径向基网络模型（杨秀萍等，2013）对多层砖房进行震害评估。
通过不同方法均可进行震害预测，但机器学习算法模型无需进行任何假设，适合内部因素错综复杂的问题，因此机器学习算法更适用于震害预测。而机器学习模型中，最关键的问题是选择合适的输入参数，即多层砖房震害影响因素。汤皓等（2006）使用灰色关联分析法对不同因素进行分析，但仅对内部关系进行分析，并未得到因素的重要性排序。集成学习算法被证明在因素重要度评估中具有良好表现（Jia等，2019）。因此，本文使用集成学习中的AdaBoost算法（Asim等，2018）对多层砖房震害影响因素进行重要度分析，以期弥补灰色关联分析的不足。
1. 影响因素与数据选取
影响多层砖房地震破坏的因素较多，主要源于以下3个方面：地震、场地和结构本身。本文选取汤皓等（2006）与姜伟等（2011）研究中的影响因素作为模型输入参数，包括房屋高度、施工质量、砂浆等级、结构合理性、砖墙面积率、房屋整体性、场地条件和地震峰值加速度（PGA）。在地震方面未选择震级和烈度是因为其与PGA具有一定关系，三者仅选择其一避免重复，且震级和烈度变化小、分级少，1次地震只出现1个震级（余震不计），不同地区烈度基本从Ⅵ度到Ⅹ度变化，仅有5个分级。
震害对应的破坏状态分为以下5个等级：基本完好、轻微破坏、中等破坏、严重破坏和倒塌，各影响因素具体分类及特征和取值见表 1，多层砖房影响因素部分数据集见表 2。
表 1 多层砖房震害影响因素特征及取值Table 1. Characteristics and influencing factors of seismic damage of multi-storey brick buildings影响因素 特征 取值 房屋高度 — — 施工质量 其他条件相同时，施工质量越好，破坏程度越低 优 10 中 8 差 6 砂浆等级 — 按实际等级取值 结构合理性 结构合理度越大，破坏程度越低 取结构抗震性能良好隶属度的值（谭克艰等，1997） 砖墙面积率 $砖墙面积率= \frac{砖墙净面积}{建筑面积}$ 按实际计算取值 房屋整体性 整体性越好，破坏程度越低 楼盖 现浇 5 预制 4 木制 3 屋盖 现浇 5 预制 4 木制 3 圈梁 有 1 构造柱 有 1 是否有地下室和筏板基础 是 1 否 0 房屋是否开裂 是 -1 否 0 场地条件 场地条件越好，破坏程度越低 Ⅰ类 10 Ⅱ类 8 Ⅲ类 6 场地条件 场地条件越好，破坏程度越低 Ⅳ类 4 地形地貌是否不利 是 -1 否 0 地下水位是否较高 是 -1 否 0 PGA PGA越大，破坏程度越高 按实际值取值 表 2 数据集Table 2. Data set序号 房屋层数 施工质量 砂浆等级/MPa 结构合理性 砖墙面积率/% 房屋整体性 场地条件 PGA/g 破坏程度 1 2 10 25 0.50 8.20 8.00 6 0.20 中等破坏 2 2 10 10 0.40 10.00 8.00 7 0.25 严重破坏 3 3 10 10 0.30 11.50 9.00 8 0.25 中等破坏 4 2 10 35 0.45 9.70 9.50 7 0.15 轻微破坏 5 4 10 25 0.36 7.60 7.50 10 0.20 中等破坏 6 2 10 25 0.55 4.30 10.00 9 0.10 轻微破坏 7 3 10 25 0.43 6.87 8.00 3 0.25 严重破坏 8 3 10 10 0.40 7.52 9.50 7 0.30 中等破坏 9 2 10 35 0.38 8.32 8.50 6 0.20 轻微破坏 10 5 10 10 0.46 11.50 8.00 8 0.25 基本完好 11 2 10 25 0.41 4.65 7.50 9 0.05 轻微破坏 12 3 10 10 0.50 9.42 12.00 8 0.20 基本完好 13 3 10 10 0.47 13.60 9.00 5 0.25 轻微破坏 14 2 10 25 0.52 10.70 8.50 7 0.20 轻微破坏 15 4 10 25 0.48 3.60 7.50 4 0.15 倒塌 16 3 10 25 0.54 6.69 8.00 8 0.25 轻微破坏 17 3 10 10 0.40 8.40 9.50 8 0.20 轻微破坏 18 4 10 25 0.45 8.50 9.46 8 0.30 严重破坏 19 2 10 25 0.47 10.30 7.00 7 0.25 中等破坏 20 4 10 25 0.47 3.23 8.00 8 0.15 轻微破坏 21 2 10 10 0.48 3.50 9.00 8 0.10 轻微破坏 22 3 10 5 0.35 8.64 8.00 7 0.20 严重破坏 23 3 10 10 0.37 6.00 8.00 7 0.20 严重破坏 24 3 10 10 0.34 9.00 10.00 7 0.25 严重破坏 25 3 10 10 0.40 9.00 7.00 7 0.30 倒塌 26 3 10 10 0.44 6.00 9.00 7 0.15 中等破坏 27 3 10 25 0.40 10.00 10.00 8 0.30 倒塌 28 3 10 25 0.40 9.00 10.00 7 0.25 倒塌 29 2 10 10 0.50 9.00 12.00 10 0.20 基本完好 30 4 10 10 0.50 9.00 9.00 9 0.15 轻微破坏 2. AdaBoost算法
AdaBoost算法是集成学习中具有代表性的一种算法，其原理是将若干个弱分类器训练集合成一个强分类器（Shin等，2009）。该算法具有强自适应性，排在前位的弱分类器算错的数据被重新计算，用于训练下个弱分类器，在这个过程中加入新的弱分类器。整个AdaBoost算法结束的标志为达到期望的错误率或达到自设的最大迭代次数，达到二者其一，迭代终止。AdaBoost算法具体迭代步骤如下：
（1）对数据集中的每个数据权值进行初始化处理。样本总数为N，将1/N的权重赋予每个数据，这些权重组成向量S。
（2）训练基本分类器。在每轮训练中，数据分为以下2种情况：当被正确分类时，加入下个训练集中时权重被自动减小；未被正确分类时，权重增加。被更新过的训练集用于训练新增的基本分类器，不断重复这个过程。AdaBoost算法为每个分类器都分配1个权重$\partial $。
（3）把每次训练后得到的基本分类器集成为1个强分类器。每轮迭代结束后，误差率$\varepsilon $低的基本分类器权重被增加，在集成的强分类器中占比更大，重要度也更高；误差率$\varepsilon $高的基本分类器权重被减小，在集成的强分类器中占比更小，重要度也更低。
具体计算公式如下：
$$ \varepsilon \rm{=}\frac{未正确分类的样本数目}{N}$$ (1) $$\partial = \frac{1}{2}\left({\frac{{1 - \varepsilon }}{\varepsilon }} \right)$$ (2) 数据被正确分类后的权重向量值为：
$${\boldsymbol{S}}_i^{(t + 1)} = \frac{{{\boldsymbol{S}}_i^{(t)}{e^\partial }}}{{sum({\boldsymbol{S}})}}$$ (3) 数据未被正确分类后的权重向量值为：
$${\boldsymbol{S}}_i^{(t + 1)} = \frac{{{\boldsymbol{S}}_i^{(t)}{e^{(- \partial)}}}}{{sum({\boldsymbol{S}})}}$$ (4) 式中，i是弱分类器，t是迭代次数。
3. 评估模型训练及结果
3.1 AdaBoost算法训练过程
本模型直接调用sklearn库中的AdaBoostClassifier分类器，默认使用CART决策树。分类算法选择基于类别概率、效果更好的SAMME.R算法，具体参数选择为：max_depth=2，min_samples_split=20，min_samples_leaf=5，n_estimators=300，learning_rate=0.5。
n_estimators表示采用的最大弱分类器个数，分类器过多模型易出现过拟合情况，但太少会出现欠拟合情况。learning_rate表示学习率，即整个模型迭代速度，学习率越小，迭代次数越多，该值下降会影响模型精度。n_estimators和learning_rate对整个模型的影响最大，因此进行重点调整，其取值不同时的系统分类精度如图 1。由图 1可知，当n_estimators和learning_rate分别为300和0.5时，模型精度最高。同时也可看出AdaBoost算法精度较高。选用的模型算法除须保证精度外，还须确保模型的稳定性，每次计算结果不能相差太大。模型3次计算结果如图 2，由图 2可知，每次计算不同参数的重要度虽不相同，但排序基本一致。这证明AdaBoost算法精度和稳定性均较好，适用于多层砖房震害影响因素评估。
图 1 不同参数下的模型精度Figure 1. Model accuracy under different parameters importance of influencing factors of seismic fatality3.2 AdaBoost算法训练结果
模型计算结果即为每个因素的重要度，AdaBoost算法训练结果见表 3，并列出灰色关联分析法中的排序。由表 3可知，多层砖房结构合理性的重要度最高，约占1/4；PGA的重要度仅次于结构合理性，约占1/5；砖墙面积率与场地条件的重要度相近；结构合理性、PGA、砖墙面积率与场地条件的重要度占比超过80%，远超其他因素，可见房屋整体性、砂浆等级、房屋高度、施工质量的重要性较低。以上分析仅针对AdaBoost算法训练结果，并未分析数据本身。
表 3 影响因素重要度排序及与灰色关联分析法结果的对比Table 3. Ranking of factors of influence factors and comparison with results of grey correlation analysis method影响因素 重要度（AdaBoost结果） 灰色关联分析法中的排名 结构合理性 0.266878512 4 PGA 0.196731755 1 砖墙面积率 0.170314083 2 场地条件 0.168439716 5 房屋整体性 0.089834515 8 砂浆等级 0.067375887 6 房屋高度 0.040425532 7 施工质量 0.000000000 3 从算法原理和数据本身来看，分级越多的影响因素重要度越高。在本模型中，施工质量全部为优，因此默认分级只有一级，数据无变化，算法默认重要度为0。但在实际工程中，施工质量对震害程度的影响很大，因此灰色关联分析法中其排名为第3。随着数据量的增加，此类问题逐渐减少。
AdaBoost算法训练结果与灰色关联分析法的结果相差较小，只有结构合理性和房屋整体性的排名出入较大。结构合理性在本模型中至关重要，但在灰色关联分析法结果中仅排在中等位置。灰色关联分析法中，如果某影响因素和破坏程度呈正相关性或负相关性，则模型自动认为此因素较重要，模型结果反映各影响因素对震害相关性强弱的顺序。2种算法内部机理不同，灰色关联分析法更注重影响因素与破坏程度的趋势关系，而AdaBoost算法更注重影响因素本身对破坏程度的贡献度及整个模型的自适应性，通过不断迭代筛选最优解。因此，对于重要度排序而言，AdaBoost算法更有优势。综上所述，多层砖房结构合理性更应被重视，而房屋整体性可排在次要位置。
通过以上对比分析可知，AdaBoost算法在处理高度非线性问题上更有优势，训练结果更接近震害经验，调节合适的参数后，其精度高达96%，且稳定性较高。因此，AdaBoost算法在多层砖房震害评估中具有适用性。灰色关联分析法作为特征重要度评估的经典方法，有其可取之处，在特征之间的关系较明晰、数据量较少的情况下同样适用。
4. 结论
本文基于AdaBoost算法对多层砖房震害影响因素重要度进行评估，得到不同影响因素的重要度排序，并将结果与灰色关联分析法进行比较。其中，结构合理性、PGA、砖墙面积率和场地条件最重要，在震害预测影响因素选择中应着重考虑。具体结论如下：
（1）AdaBoost算法具有良好的稳定性和精度，适用于多层砖房震害影响因素重要度评估。
（2）房屋结构合理性在诸多影响因素中排名第1，重要度约占1/4，可见其重要性，设计建造时应着重考虑；地震峰值加速度排名第2，重要度约占1/5，设计阶段应予以高度重视。
（3）砖墙面积率与场地条件的重要度相对较高，而房屋整体性、砂浆等级、房屋高度与施工质量的重要度较低，其中施工质量重要度排序与灰色关联分析法差异最大，这是数据有限的原因。
此外，多层砖房震害影响因素远不止文中所列，由于时间和数据限制，本文仅提出探索性模型，仍需进一步拓展影响因素、补充数据，将数据质量较差的例子删除，以期提升模型准确度和适用性，以便推广使用。
-
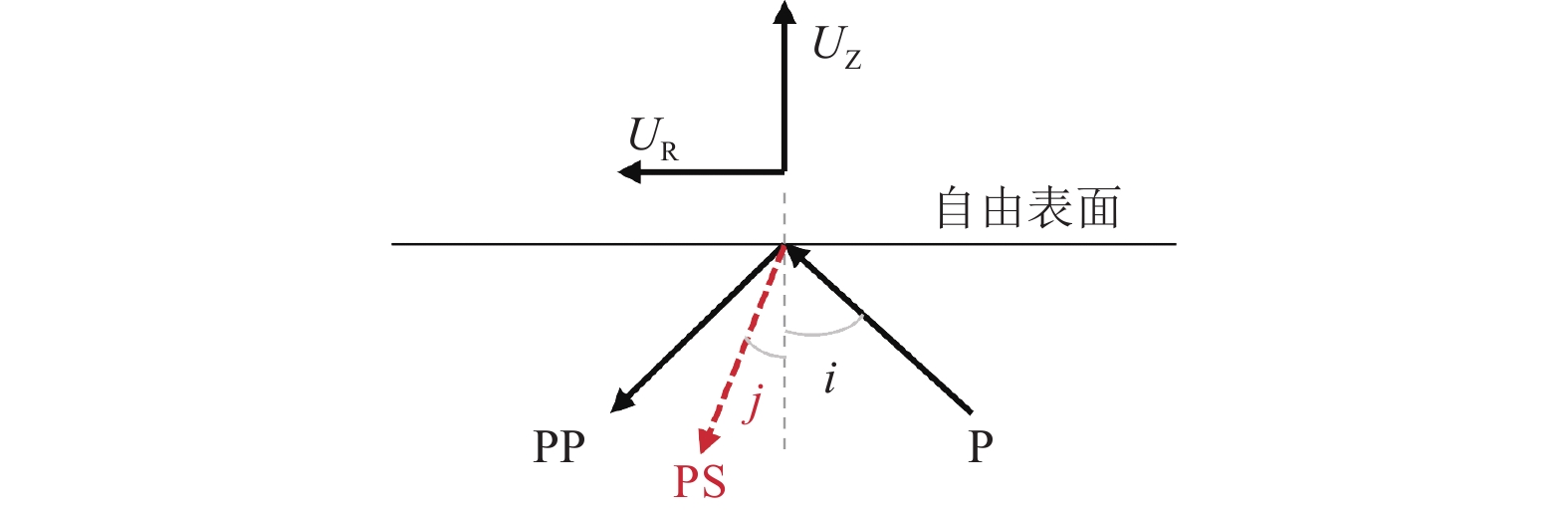
图 1 入射P波在自由表面处的转换（Li等，2014）
Figure 1. Interaction between incident P-wave and free surface (from Li et al., 2014)
图 4 不同波速结构模型对应的理论地震图和UZ/UR随剪切波速变化情况
Figure 4. Theoretical seismograms corresponding to different velocity structure models and UZ/UR
图 5 汶川地震主余震分布
Figure 5. Distribution of main shock and aftershocks of Wenchuan earthquake
图 8 不同波速基岩场地模型与PS/P幅值比
Figure 8. Site structures with different velocity VSbo and the radial PS/P amplitude of synthetic seismograms
图 9 满足反演目标函数的理论地震图
Figure 9. Synthetic seismograms matching the inversion objective functions
图 10 武都WUD台站剪切波速结构反演结果对比
Figure 10. Comparison of shear wave velocity structure inversion results at WuDu station
图 11 不同VS30对应的衰减关系及PGA标准差与断层距关系
Figure 11. Attenuation relationship corresponding to different VS30 and relationship between PGA standard deviation and rupture distance
表 1 武都台记录的地震信息
Table 1. Earthquakes information of recorded at Wudu station
发震时间 纬度/
(°N)经度/
(°E)震源
深度/km震级 震中距/
km2008-07-31 13:56:03 33.3 105.1 33 3.3 15.2 2008-08-02 21:45:03 32.7 105.7 10 4.0 97.8 2008-08-31 15:24:03 32.7 105.8 10 4.1 104.4 2008-05-15 21:04:03 32.7 105.6 33 4.3 89.8 2008-05-25 17:34:03 33.0 104.9 33 4.7 37.8 2008-05-25 16:21:03 32.5 105.4 33 6.4 96.4 2008-08-05 17:49:03 32.7 105.6 10 6.5 90.9 
下载: 导出CSV
-
陈成锋,张瑞青,强正阳等,2022. 川南泸州地区地震重定位与地壳三维速度结构研究. 地球物理学报,65(12):4672−4684. doi: 10.6038/cjg2022P0905Chen C. F., Zhang R. Q., Qiang Z. Y., et al., 2022. Seismicity and three-dimensional crustal structure of the Luzhou region in the southern Sichuan Basin, China. Chinese Journal of Geophysics, 65(12): 4672−4684. (in Chinese) doi: 10.6038/cjg2022P0905 方洪健,刘影,姚华建等,2023. 区域尺度地震体波和面波走时联合成像:进展与展望. 地球与行星物理论评(中英文),54(3):252−269.Fang H. J., Liu Y., Yao H. J., et al., 2023. Regional-scale joint seismic body- and surface-wave travel time tomography. Reviews of Geophysics and Planetary Physics, 54(3): 252−269. (in Chinese) 郝奥伟,张海江,韩守诚等,2023. 基于多台站的接收函数和重力联合反演确定莫霍面起伏和地壳平均波速比. 地震学报, 45 (1):1−16.Hao A. W. ,Zhang H. J. ,Han S. C. ,et al. ,2023. Joint inversion of multi-station receiver functions and gravity data for imaging Moho variations and average crustal v P/ v S ratios. Acta Seismologica Sinica, 45 (1):1−16. (in Chinese) 黄海波,丘学林,胥颐等,2011. 利用远震接收函数方法研究南海西沙群岛下方地壳结构. 地球物理学报,54(11):2788−2798. doi: 10.3969/j.issn.0001-5733.2011.11.009Huang H. B., Qiu X. L., Xu Y., et al., 2011. Crustal structure beneath the Xisha Islands of the South China Sea simulated by the teleseismic receiver function method. Chinese Journal of Geophysics, 54(11): 2788−2798. (in Chinese) doi: 10.3969/j.issn.0001-5733.2011.11.009 贾俊峰,杜修力,韩强,2015. 近断层地震动特征及其对工程结构影响的研究进展. 建筑结构学报,36(1):1−12.Jia J. F., Du X. L., Han Q., 2015. A state-of-the-art review of near-fault earthquake ground motion characteristics and effects on engineering structures. Journal of Building Structures, 36(1): 1−12. (in Chinese) 刘平,罗奇峰,陈兴周,2020. 汶川地震竖向地震动衰减关系. 哈尔滨工业大学学报,52(2):119−128. doi: 10.11918/201809108Liu P., Luo Q. F., Chen X. Z., 2020. Vertical ground motion attenuation relationship of Wenchuan earthquake. Journal of Harbin Institute of Technology, 52(2): 119−128. (in Chinese) doi: 10.11918/201809108 刘启元,Kind R.,李顺成,1997. 中国数字地震台网的接收函数及其非线性反演. 地球物理学报,40(3):356−368. doi: 10.3321/j.issn:0001-5733.1997.03.008Liu Q. Y., Kind R., Li S. C., 1997. The receiver functions at the stations of the Chinese Digital Seismic Network (CDSN) and their nonlinear inversion. Acta Geophysica Sinica, 40(3): 356−368. (in Chinese) doi: 10.3321/j.issn:0001-5733.1997.03.008 刘振东,吕庆田,严加永等,2012. 庐枞盆地浅表地壳速度成像与隐伏矿靶区预测. 地球物理学报,55(12):3910−3922. doi: 10.6038/j.issn.0001-5733.2012.12.004Liu Z. D., Lü Q. T., Yan J. Y., et al., 2012. Tomographic velocity structure of shallow crust and target prediction for concealed ore deposits in the Luzong Basin. Chinese Journal of Geophysics, 55(12): 3910−3922. (in Chinese) doi: 10.6038/j.issn.0001-5733.2012.12.004 卢育霞,马林伟,卢芳琴等,2014. 武都台的地震记录特征及场地条件分析. 地震工程与工程振动,34(S1):118−125.Lu Y. X., Ma L. W., Lu F. Q., 2014. Characteristics of strong ground motion and site condition on 62WUD seismic station. Earthquake Engineering and Engineering Dynamics, 34(S1): 118−125. (in Chinese) 王海云,谢礼立,2008. 近断层地震动模拟现状. 地球科学进展,23(10):1043−1049. doi: 10.3321/j.issn:1001-8166.2008.10.006Wang H. Y., Xie L. L., 2008. A review on near-fault ground motion simulation. Advances in Earth Science, 23(10): 1043−1049. (in Chinese) doi: 10.3321/j.issn:1001-8166.2008.10.006 王霞,宋美琴,王亮等,2015. 口泉断裂及其邻近地区的地壳速度结构. 地震地质,37(4):939−952. doi: 10.3969/j.issn.0253-4967.2015.04.001Wang X., Song M. Q., Wang L., et al., 2015. Study on crustal velocity structure beneath Kouquan fault and adjacent area. Seismology and Geology, 37(4): 939−952. (in Chinese) doi: 10.3969/j.issn.0253-4967.2015.04.001 肖卓,高原,2017. 利用双差成像方法反演青藏高原东北缘及其邻区地壳速度结构. 地球物理学报,60(6):2213−2225. doi: 10.6038/cjg20170615Xiao Z., Gao Y., 2017. Crustal velocity structure beneath the northeastern Tibetan plateau and adjacent regions derived from double difference tomography. Chinese Journal of Geophysics, 60(6): 2213−2225. (in Chinese) doi: 10.6038/cjg20170615 周宝峰,宋廷苏,于海英等,2014a. 芦山强震记录中的奇异波形研究. 地震工程与工程振动,34(S1):93−99.Zhou B. F., Song T. S., Yu H. Y., et al., 2014a. The study on the wizard wave in the strong motion record. Earthquake Engineering and Engineering Dynamics, 34(S1): 93−99. (in Chinese) 周宝峰,温瑞智,谢礼立,2014b. 强震记录中的“尖刺”现象初步研究. 土木工程学报,47(S2):295−299.Zhou B. F., Wen R. Z., Xie L. L., 2014b. The preliminary study on the “spike” in strong motion records. China Civil Engineering Journal, 47(S2): 295−299. (in Chinese) 周龙泉,刘杰,张晓东,2007. 2003年大姚6.2和6.1级地震前三维波速结构的演化. 地震学报,29(1):20−30. doi: 10.3321/j.issn:0253-3782.2007.01.003Zhou L. Q., Liu J., Zhang X. D., 2007. Evolution of 3D velocity structure before Dayao M 6.2 and M 6.1 earthquakes in 2003. Acta Seismologica Sinica, 29(1): 20−30. (in Chinese) doi: 10.3321/j.issn:0253-3782.2007.01.003 Aki K. , Richards P. G. , 2002. Quantitative seismology. 2nd ed. Sausalito, California: University Science Books. Chen K. C., Chiu J. M., Yang Y. T., 1996. Shear-wave velocity of the sedimentary basin in the upper Mississippi embayment using S-to-P converted waves. Bulletin of the Seismological Society of America, 86(3): 848−856. doi: 10.1785/BSSA0860030848 Chiu S. C. C., Langston C. A., 2011. Waveform inversion for one-dimensional near-surface structure in the New Madrid seismic zone. Bulletin of the Seismological Society of America, 101(1): 93−108. doi: 10.1785/0120100025 Kim B., Hashash Y. M. A., Rathje E. M., et al., 2016. Subsurface shear wave velocity characterization using P -Wave seismograms in central and eastern North America. Earthquake Spectra, 32(1): 143−169. doi: 10.1193/123013EQS299M Langston C. A., 2003. Local earthquake wave propagation through Mississippi embayment sediments, part I: body-wave phases and local site responses. Bulletin of the Seismological Society of America, 93(6): 2664−2684. doi: 10.1785/0120030046 Li Z. W., Ni S. D., Somerville P., 2014. Resolving shallow shear-wave velocity structure beneath station CBN by waveform modeling of the Mw 5.8 mineral, Virginia, earthquake sequence. Bulletin of the Seismological Society of America, 104(2): 944−952. doi: 10.1785/0120130190 Ni S., Li Z., Somerville P., 2014. Estimating subsurface shear velocity with radial to vertical ratio of local P waves. Seismological Research Letters, 85(1): 82−90. doi: 10.1785/0220130128 Randall G. E., 1989. Efficient calculation of differential seismograms for lithospheric receiver functions. Geophysical Journal International, 99(3): 469−481. doi: 10.1111/j.1365-246X.1989.tb02033.x Zhu L. P., Rivera L. A., 2002. A note on the dynamic and static displacements from a point source in multilayered media. Geophysical Journal International, 148(3): 619−627. doi: 10.1046/j.1365-246X.2002.01610.x 期刊类型引用(0)
其他类型引用(1)
-
下载:
点击查看大图
计量
- 文章访问数: 113
- HTML全文浏览量: 19
- PDF下载量: 11
- 被引次数: 1
