Calibration of Seismic Input Parameter Based on Reliability and Fragility
-
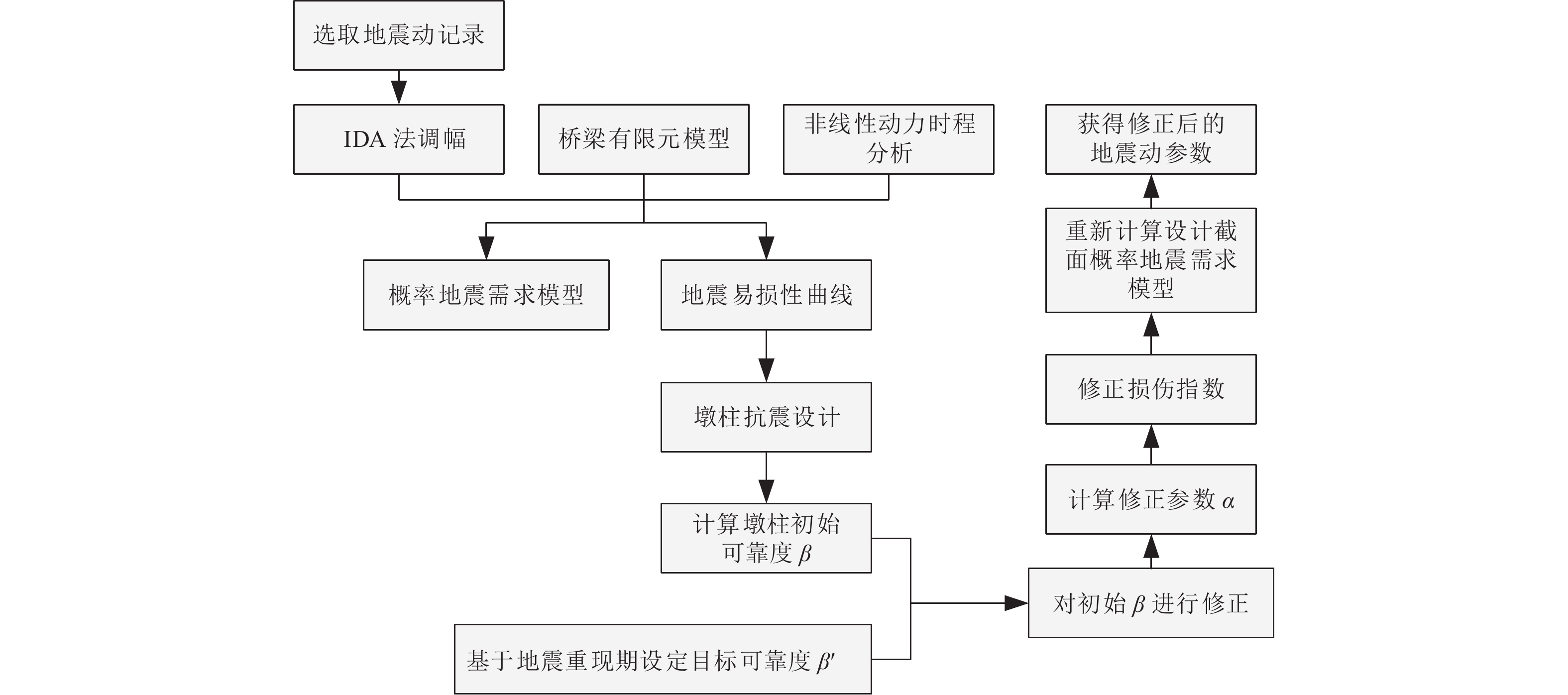
摘要: 在基于性能的地震工程理念与经典可靠度理论框架下,分析了地震易损性与结构可靠度的共同特性,阐释了将两者相结合的理论基础与概率方法;以一座三跨连续梁桥为例,基于OpenSees平台建立桥梁有限元模型,通过非线性动力时程分析对结构进行概率地震需求及易损性分析,基于易损性曲线考虑3种抗震目标对桥墩进行抗震设计,并对设计墩柱进行恒载与车辆活载作用下的可靠度分析;考虑不同地震重现期,根据泊松随机过程计算各类地震作用下的目标可靠度,基于概率方法对结构的初始可靠度进行修正,进一步结合地震易损性,确定不同抗震目标下的地震动水平,提出基于可靠度与易损性的地震动输入参数确定方法。结果表明,1000年、475年和50年地震重现期下,修正PGA均值分别为0.25 g、0.24 g和0.22 g,高于仅考虑易损性时的0.21 g。本方法将地震易损性与可靠度相结合,为结构不同层次的抗震设计提供了思路与方法。Abstract: Within the framework of Performance-Based Earthquake Engineering (PBEE) and classical reliability theory, this study reviews the common characteristics of seismic fragility and reliability, interpreting the theoretical foundation and probabilistic methods for their combination. In the case study, probabilistic engineering demand parameters and seismic fragility for a continuous girder bridge are first analyzed using non-linear dynamic time-history analysis. Then, three types of seismic performance objectives are considered for the seismic design of the pier. Additionally, reliability analysis is conducted considering dead load and vehicle live load. Various seismic return periods are set to calculate target reliability indexes according to the Poisson random process. This process calibrates the initial reliability index, which is then combined with the fragility curve using a probabilistic approach to determine seismic input parameters for different seismic performance objectives. The results show that the calibrated input parameters are 0.25 g, 0.24 g, and 0.22 g for return periods of
1000 , 475, and 50 years, respectively. These values are slightly larger than the 0.21 g determined by the fragility curve alone. The proposed method simultaneously considers seismic fragility and reliability, providing a new approach for the seismic design of structures with varying seismic requirements.-
Key words:
- PBEE /
- Seismic fragility /
- Reliability /
- Seismic parameter
-
引言
近年来,由于遥感技术的不断进步,遥感地质学在矿产普查、区域地质和水文地质等方面开始发挥日益重要的作用(赵鸿燕等,2010;齐信等,2012;魏永明等,2015;段瑞琪等,2017)。通过遥感影像识别断裂各类构造地貌信息,进而讨论断裂新构造运动特征的研究方法已颇为成熟(贾营营等,2010;郑颖平等,2017)。目前,随着对构造地貌理解的不断深入,众多学者已通过不同构造地貌类型的组合和发育现状反演构造活动历史,开展更全面的运动学研究(刘华国等,2011;文力等,2018)。
断裂构造地貌是由断裂活动在地表塑造的地貌,由于断裂活动方式多样,在地表塑造的地貌类型也不尽相同(杨景春等,2011)。在各类地貌中,线性特征是断裂最明显的构造标志,也是断裂遥感解译中最重要的地物之一。通常情况下,走滑断裂的线性特征较倾滑断裂更加典型。此外,断裂垂向错动还可形成断层陡坎(中屠炳明等,1991)、台地(师亚芹等,2009)及河流裂点(毕丽思等,2011)等地貌;断裂水平扭动错断山脊线和水系,可形成眉脊和断尾沟等地貌。这些地貌的形成与发育和断裂活动方式、强度、频度、岩性特征、地形、气候等因素密切相关。通过构造地貌的识别与分析,可开展断裂空间几何学、运动学等方面的研究。随着高分辨率遥感影像的逐步应用和GIS技术的不断提高,结合断裂构造地貌特征与现有地质资料,有助于提高断裂解译的精度和深度。
盘谷寺断裂为太行山南缘盘谷寺-新乡断裂的西段,是我国东部1条重要的岩石圈构造带(邓起东等,1980;马杏垣,1989;张培震,1999)、地壳厚度陡变带(江娃利等,1984;徐杰等,2000)和地震活动带(李清武,2015),同时也是太行山断块隆起和南华北坳陷的分界断裂。盘谷寺断裂活动始于燕山运动,于燕山运动晚期—喜马拉雅运动中期强烈活动,最终止于中更新世(中国地震局地球物理勘探中心,2015)。目前针对盘谷寺断裂新构造运动特征的深入分析仍较少,分析该断裂新构造运动特征对研究太行山东南缘构造地貌演化,乃至我国东部新构造活动格局具有重要意义。本文综合利用多波段与全色波段遥感数据,在ArcGIS平台上,采用陆地成像仪(Operational Land Imager,OLI)数据及先进星载热发射和反射辐射仪全球数字高程模型(Advanced Spaceborne Thermal Emission and Reflection Radiometer Global Digital Elevation Model,ASTER GDEM)数据,通过地物判读、提取坡向坡度及构建三维地貌模型等手段,对盘谷寺断裂进行遥感解译,确定该断裂空间展布形态,并利用地壳掀斜规律和剖面分析,初步总结断裂活动特征,取得良好效果。
1. 区域地震地质背景
研究区地处河南省西北部,与山西省相邻,构造上位于太行山断块隆起与南华北坳陷的交界部位(图 1)。南太行基底由太古界登封群和与之不整合接触的下元古界嵩山群组成,表现为紧闭线型复式褶皱;盖层为中元古界汝阳群和寒武系-中奥陶统,多呈单斜构造和开阔褶皱(河南省地质矿产局,1989)。
根据已有成果,在太行山南缘发育的1组近东西走向、陡倾的阶梯状正断层控制了本地区构造地貌的形成和发育(马寅生等,2007)。自新生代以来,本地区共经历3次快速的构造隆升运动,在间歇期于海拔1800m、1100—1300m和350—500m处分别形成了北台期、太行期和唐县期三级夷平面(程绍平等,1989;吴忱等,1999)。进入第四纪后,断裂活动性减弱,仅山前断裂——盘谷寺断裂错断了第四纪沉积物(马寅生等,2007)。
盘谷寺-新乡断裂为太行山隆起与济源-开封凹陷的分界断裂,该断裂大致以柏山、大高村为界,由西向东划分为3个次级段落。西段位于柏山以西,为盘谷寺断裂,中段和东段均为隐伏断裂。盘谷寺断裂南部济源凹陷的西界和东界分别为封门口断裂和武陟断裂(图 1),与凹陷内部发育的多条近东西向次级正断层共同控制了凹陷整体的形成和发展过程。该凹陷在古近纪时期强烈断陷下沉,南北向伸展率可达35%—60%(潘澄雨,2013),内部堆积了巨厚的古近系。进入新近纪和第四纪后,凹陷伸展断陷作用显著减弱(马寅生等,2007)。
盘谷寺-新乡断裂西段为中更新世活动段,中段和东段为晚更新世活动段(中国地震局地球物理勘探中心,2015)。断裂附近曾于1587年发生2次破坏性地震,震级分别为5.5级和6.0级。现今小震活动主要集中于南部的济源-开封凹陷内。
2. 数据选取与处理
2.1 数据选取
遥感影像数据选用Landsat 8 OLI陆地成像仪和ASTER GDEM数据。Landsat 8 OLI陆地成像仪发射于2013年,共9个波段,包括1个分辨率15m的全色波段,空间分辨率为30m,成像宽幅185km×185km,选取的影像云量均小于1%。
高程数据选用ASTER GDEM数据,该数据发布于2011年,其空间分辨率为30m,垂直精度约20m,每幅影像覆盖面积约60km×60km(ASTER GDEM Validation Team,2009)。
2.2 数据处理
本文所用遥感影像数据和高程数据均来源于地理空间数据云,根据该网站提供的产品信息,Landsat 8 OLI影像已经系统辐射校正和几何校正,故本次数据处理主要包括影像镶嵌、融合与裁剪等(图 2)。
遥感影像的处理在ENVI环境下进行,首先将选取的2幅遥感影像各波段数据进行拼接,然后将OLI数据多光谱波段和全色波段进行融合处理,通过重采样生成分辨率为15m的多光谱数据,采用6、5、4波段进行假彩色合成,通过线性拉伸、边缘增强等处理突出纹理特征,地质信息丰富,视觉效果得到明显提升。ASTER GDEM数据的镶嵌和裁剪处理均在ArcGIS下进行。最终将OLI遥感影像与DEM数据叠加,构建地区三维地貌模型。
3. 盘谷寺断裂信息提取
3.1 侵蚀冲沟发育特征
流水型构造地貌对构造活动的响应十分灵敏(史兴民等,2003),构造运动通过改变地表地形高差、地壳掀斜特征等影响冲沟和河谷的发育及形态。在气候、岩性相近的条件下,构造运动是影响冲沟形态最重要的因素。正断裂发生差异升降运动后,上盘相对下降,局部侵蚀基准面随之降低,由下盘而来的水流将在沟谷中产生强烈的溯源侵蚀作用,最终在下盘发育一系列与断裂近垂直的冲沟。断裂作为溯源侵蚀作用的起点,其差异升降运动的强弱往往决定着溯源侵蚀作用的强弱。反之,一系列近平行的冲沟可作为断裂解译的标志。
通过判读盘谷寺断裂的遥感影像,发现沿盘谷寺断裂走向,在断裂下盘发育的冲沟形态差别十分明显,大致以济源市河口村为界,在河口村以西,山前发育一系列密集、近平行、长约数百米的深切冲沟,冲沟沟尾线性相连,如图 3(a)所示,是判断断裂位置的良好标志;在河口村以东,断裂线性特征较清晰,影像中显示清晰的断层三角面及大型山间沟谷,如图 3(b)中红框所示;沿走向继续向东,断层三角面形态逐渐消失,断裂下盘表现为经大面积强烈侵蚀作用后的低矮残丘,如图 3(c)所示。残丘与断裂上盘仍存在一定高差,以致断裂在影像上虽依稀表现出线性特征,但较断裂西段已明显弱化。
基于以上解译认识,基本可确定断裂的空间展布方式。由西向东,在断裂下盘依次发育深切冲沟→断层三角面→侵蚀残丘,客观反映了溯源侵蚀作用由西向东逐渐减弱的规律,据此初步判断断裂差异升降运动由西向东逐渐减弱。
3.2 坡向和坡度分析
坡状地貌也是构造地貌学研究的重要内容之一,坡状地貌的形成演化是构造运动和侵蚀作用长期共同作用的结果(Davis,1899;杨景春等,2011)。构造运动是坡状地貌的诱发因素,具有脉冲式特点,而侵蚀作用则不断将坡状地貌夷平,具有长期性。通过评价坡状地貌形态可反演地区历经的构造运动强度和规模。坡向和坡度是定量描述坡状地貌的2个重要参数。在ArcGIS平台上,采用ASTER GDEM数据提取了盘谷寺断裂沿线坡向、坡度图,如图 4所示。由图 4可知,由于构造运动引起的地壳掀斜和后期侵蚀堆积作用,盘谷寺断裂上盘地表普遍向南倾斜,倾斜区域呈楔形分布,宽度由西向东逐渐变小,至博爱县北附近,地表倾斜现象已不明显,如图 4(a)所示。由坡度图可知,由于断裂的存在,太行山脉和济源凹陷间存在1条地形坡度分界线,该界线以北为太行山脉,地形坡度大,起伏多变;以南为济源凹陷,地形平缓。地形坡度的差异由断裂活动造成,该地形坡度分界线即为盘谷寺断裂的构造位置,断裂活动造成两侧地形坡度产生差异。
通过对比坡向图和坡度图可知,断裂西段地壳掀斜范围、地形倾角变化率均宽于和大于断裂东段,盘谷寺断裂西段对地表地形的影响强于东段,这是由于断裂西段的活动性较强。
3.3 剖面分析
分析地形剖面可获得断裂垂向断错特征,地形剖面形态主要与构造活动、侵蚀及堆积过程有关,通过识别地形剖面中的夷平面、断面及洪积扇堆积方式等要素,可研究断裂的活动期次与活动强度等特征(杨源源等,2012)。
笔者提取了4条近垂直穿过盘谷寺断裂的地形剖面(图 5),这4条剖面由西向东依次穿过了该断裂的西段和东段,充分反映了断裂整体的垂向断错特征。
A—A'剖面位于济源市以西,剖面显示,断裂下盘的太行山脉海拔普遍为700—1000m,沟壑纵横,地形起伏大;断裂活动在地表形成了数百米高且陡直的断层面;在断裂上盘,由于靠近断裂的位置存在洪积扇体堆积,造成局部地形坡度较大,向南地形平缓,海拔较低,普遍不超过200m。
B—B'剖面位于济源市以东,与A—A'剖面类似,断裂两侧地形迥异,下盘海拔普遍为700—1000m,上盘地形平坦且海拔不超过200m,断裂活动在地表形成了数百米高且陡直的断层面。
C—C'剖面位于沁阳市西侧,跨越盘谷寺断裂东段,剖面北部为太行山脉,海拔由900m向南逐渐降至300m,断裂活动未在地表形成高耸的断层面,而是表现为斜坡状地形,斜坡顶部与底部高差仅数十米,该剖面反映断裂在该处的活动性变弱,大面积侵蚀和堆积作用开始在塑造地形的过程中占据主导地位。
D—D'剖面位于盘谷寺断裂东端,剖面整体形态与C—C'剖面类似,由于断裂活动性弱,海拔高度由北部太行山向济源凹陷连续过渡,地形剖面几乎已无法表现出断面形态。
结合地形剖面与前文所述冲沟发育、坡向坡度特征,认为盘谷寺断裂差异升降运动由西向东逐渐减弱,因此济源凹陷西部的沉降幅度较东部大,西部新近系底界埋深也大于东部,如图 1所示(河南省地质矿产局,1989;王志铄,2017)。
3.4 基于OLI和DEM的三维地貌模型
将OLI数据和高程数据叠加,可获得盘谷寺断裂三维地貌模型。叠加后的三维地貌模型较原遥感影像更立体。通过增加阴影、扩大Z值因子等手段,对盘谷寺断裂地貌细节进行突出显示(图 6)。
由图 6可知,经过三维地貌模型突出显示后,断裂两盘地形地貌反差明显,通过突出细节,使地表迹线更清晰,便于研究断裂的空间展布方式和表现形式。该模型同时直观地展示出盘谷寺断裂下盘由西向东依次发育了不同的构造地貌:断裂西段下盘表现为深切侵蚀沟,断裂由沟尾附近穿过;向东发展,断裂下盘表现为较完整的断层三角面;至断裂东段,由于断裂活动性减弱,以大面积侵蚀作用为主,断裂下盘主要表现为低矮的侵蚀残丘。
4. 结论
本文采用Landsat 8 OLI数据和ASTER GDEM数据,以ArcGIS为平台,对太行山南缘盘谷寺断裂开展了构造地貌提取和分析,通过分析断裂下盘冲沟发育特征、断裂两盘坡向和坡度变化规律及跨断层地形剖面等手段,判定断裂空间展布形态,得到以下结论:
(1) 盘谷寺断裂下盘由西向东依次发育了深切侵蚀(冲)沟、断层三角面和低矮的侵蚀残丘3类构造地貌,这是伴随构造活动强度的减弱,侵蚀方式由强烈的溯源侵蚀逐渐向大面积侵蚀作用转变的结果。
(2) 不同类型的构造地貌表现与地形剖面图共同说明盘谷寺断裂为1条倾向南的正断层,断裂差异升降运动由西向东逐渐减弱,导致新近系底界在断裂西部的埋深大于东部,至中更新世时期断裂停止活动。
(3) 断裂西部的沉降幅度大于东部,说明该断裂新构造运动以来的差异升降运动不均匀,西部差异升降运动强于东部,因此推断太行山在隆升剥露的过程中并非同步抬升,值得进一步深入研究。
-
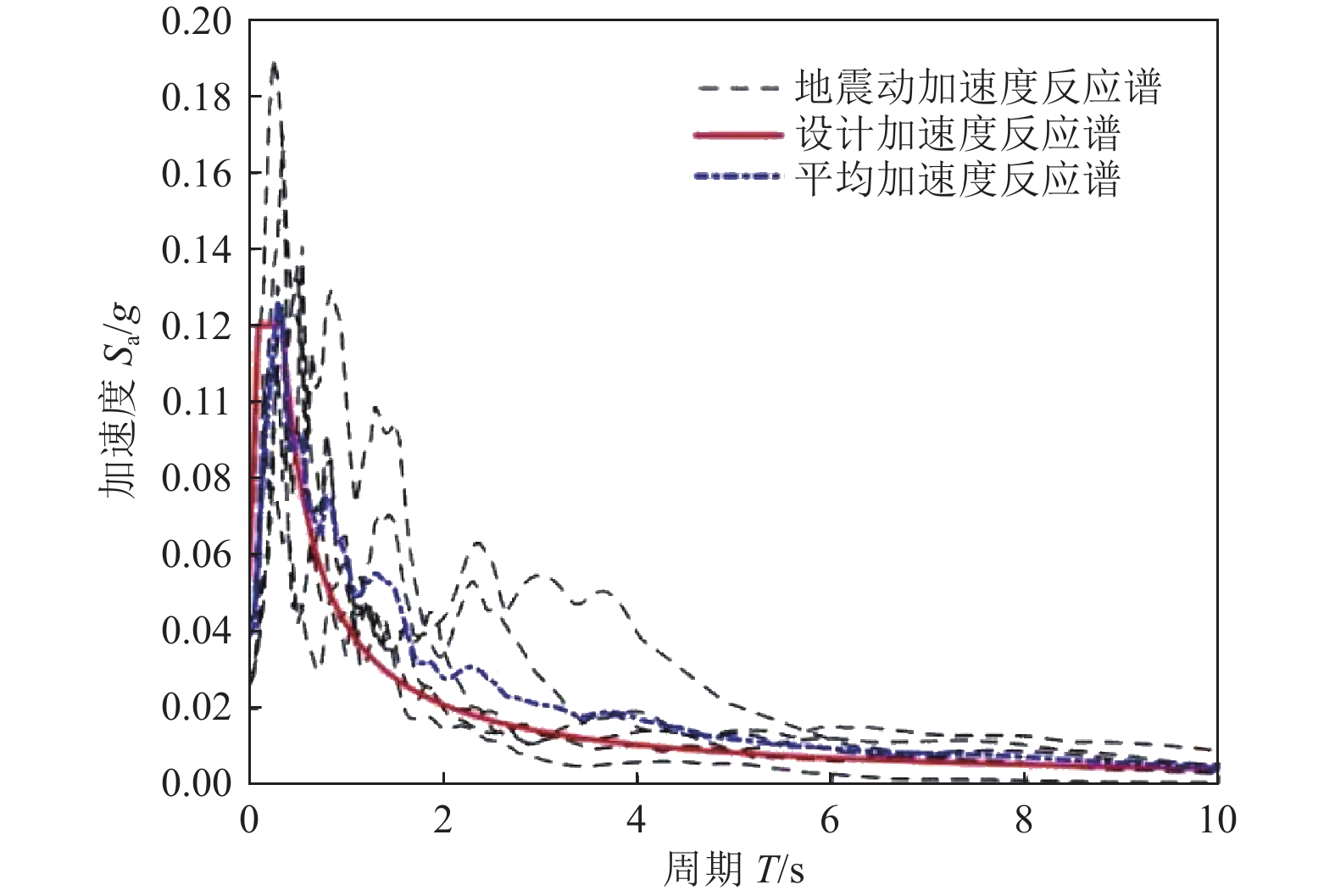
表 1 基础地震动记录
Table 1. Ground motions set as benchmark
序号 地震记录 记录地点 震中距/km PGA/g 1 Borrego El Centro Array #9 56.88 0.066 2 Kern County LA - Hollywood Stor FF 114.62 0.042 3 San Fernando 2516 Via Tejon PV55.2 0.026 4 San Fernando LB - Terminal Island 58.99 0.029 5 San Fernando Port Hueneme 68.84 0.027 6 San Fernando San Juan Capistrano 108.01 0.043 表 2 桥梁损伤状态描述及损伤指标
Table 2. Bridge damage states and their measurements by displacement ductility ratios
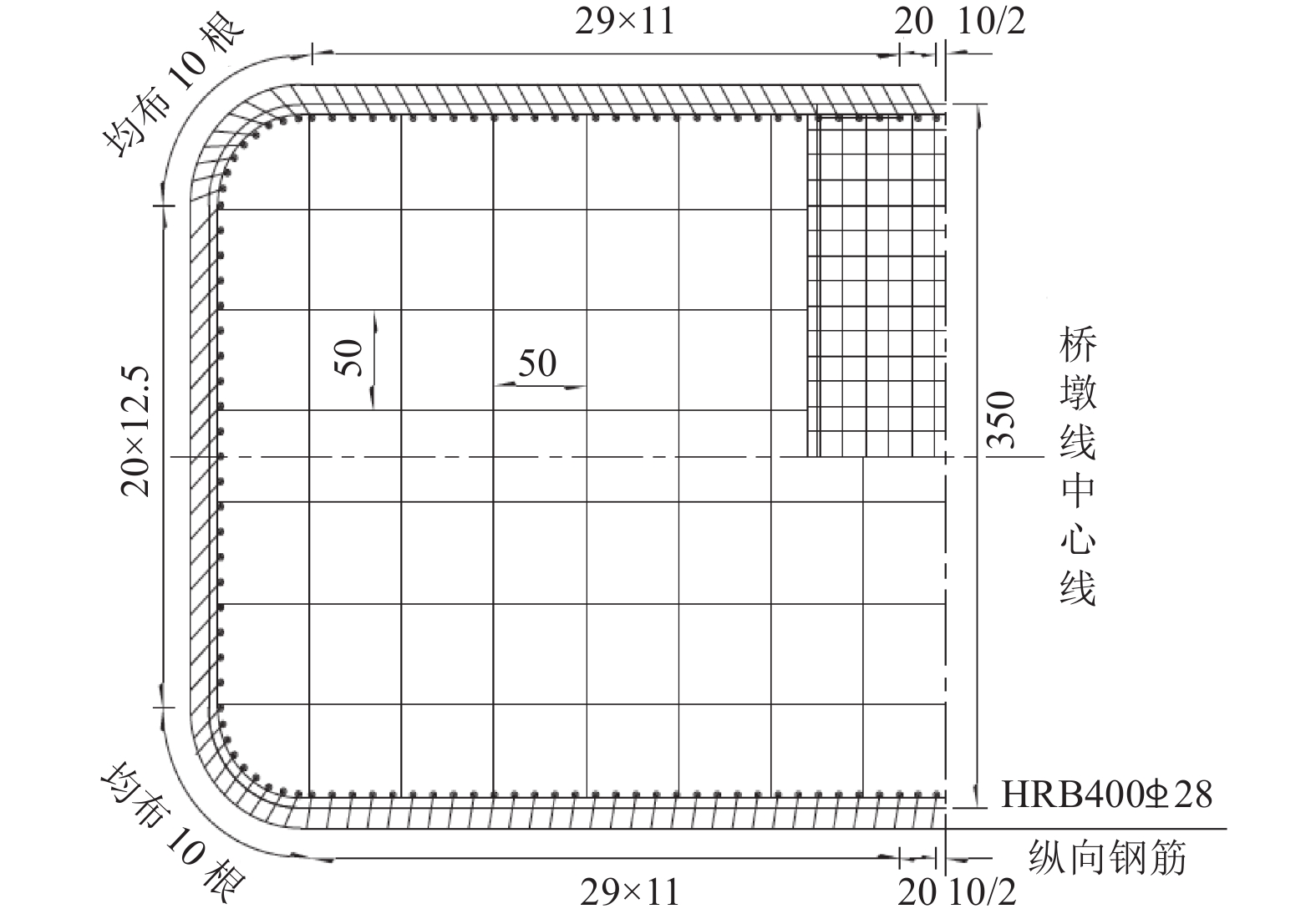
损伤状态 具体描述 准则 位移延性比 无破坏 墩柱无明显裂缝, 钢筋无屈服 $ 0 < \mu \leqslant {\mu }_{\mathrm{c}\mathrm{y}1} $ $ 0 < \mu \leqslant 1 $ 轻微破坏 墩柱表面出现明显裂缝,最外侧钢筋首次出现理论屈服 $ {\mu }_{\mathrm{c}\mathrm{y}1} < \mu \leqslant {\mu }_{\mathrm{c}\mathrm{y}} $ $ 1 < \mu \leqslant 1.12 $ 中等破坏 表层混凝土部分脱落,墩柱产生非线性变形,墩底塑性铰开始形成 $ {\mu }_{\mathrm{c}\mathrm{y}} < \mu \leqslant {\mu }_{\mathrm{c}4} $ $ 1.12 < \mu \leqslant 1.97 $ 严重破坏 塑性铰完全形成,保护层混凝土全部剥落,核心混凝土部分开裂,纵筋大量屈服 $ {\mu }_{\mathrm{c}4} < \mu \leqslant {\mu }_{\mathrm{c}\mathrm{m}\mathrm{a}\mathrm{x}} $ $ 1.97 < \mu \leqslant 4.97 $ 完全破坏 核心混凝土压碎,箍筋断裂 $ {\mu }_{\mathrm{c}\mathrm{m}\mathrm{a}\mathrm{x}}\leqslant \mu $ $ 4.97\leqslant \mu $ 表 3 抗力和荷载效应的统计特征参数
Table 3. Statistics parameters for resistance and load effect
随机变量类别 均值/(kN·m) 标准差 抗力 $ {\mu }_{{M}_{{\mathrm{R}}}}=48\;863.72 $ $ {\sigma }_{{M}_{{\mathrm{R}}}}=2\;855.02 $ 恒载 $ {\mu }_{{M}_{{\mathrm{G}}}}=19\;722.05 $ $ {\sigma }_{{M}_{{\mathrm{G}}}}=8\;492.84 $ 活载 $ {\mu }_{{M}_{{\mathrm{Q}}}}=1\;105.61 $ $ {\sigma }_{{M}_{{\mathrm{Q}}}}=173.55 $ 表 4 不同地震重现期下的修正可靠度与修正系数
Table 4. Calibrated reliability index and calibration coefficient for various return periods
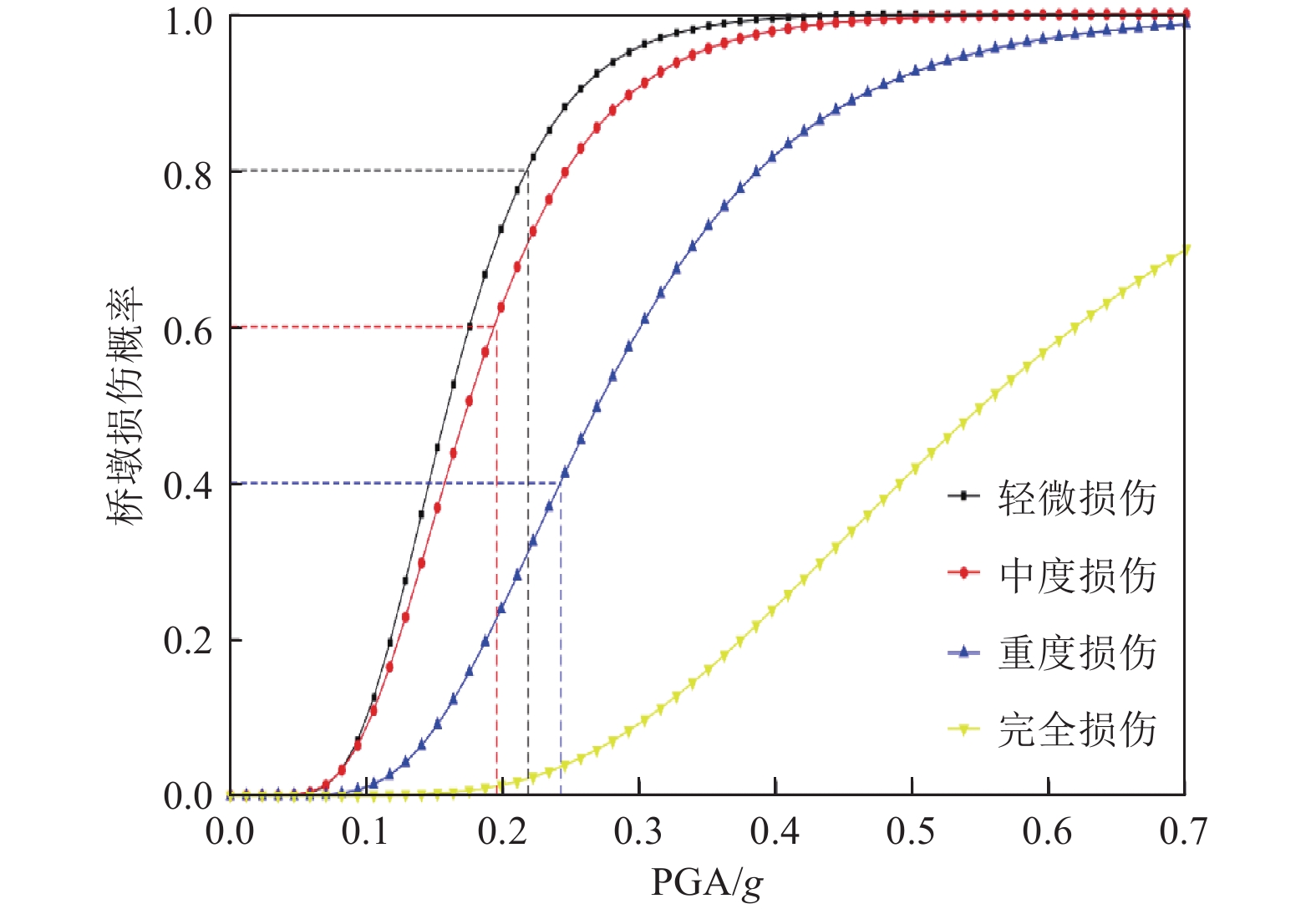
重现期$ {T}_{0} $ 设计基准期t 修正后$ {\beta }'' $ 初始$ \beta $ 修正系数$ \alpha $ 1000 100 3.9141 3.3039 1.1847 475 100 3.7441 3.3039 1.1332 100 100 3.4304 3.3039 1.0383 75 100 3.3888 3.3039 1.0257 50 100 3.3445 3.3039 1.0123 25 100 3.3091 3.3039 1.0016 10 100 3.3039 3.3039 1.0000 5 100 3.3039 3.3039 1.0000 表 5 重现期为1000年时的地震动参数修正值(
${\boldsymbol{ \alpha }} $ = 1.1847)Table 5. Calibrated peak ground accelerations for the return period of
1000 a(${\boldsymbol{ \alpha }} $ = 1.1847)损伤状态 修正前 修正后 PGA/g $ {u}_{\mathrm{D}\mathrm{I}} $ $ {u}'_{\mathrm{D}\mathrm{I}} $ PGA/g 80%轻微损伤 0.210 1.531 $ \alpha \cdot DI=1.814 $ 0.253 60%中度损伤 0.183 1.280 $ \alpha \cdot DI=1.516 $ 0.220 40%重度损伤 0.229 1.714 $ \alpha \cdot DI=2.030 $ 0.276 表 6 重现期为475年时的地震动参数修正值(
$ {\boldsymbol{\alpha}} $ = 1.1332)Table 6. Calibrated peak ground accelerations for the return period of 475 a(
${\boldsymbol{ \alpha}} $ = 1.1332)损伤状态 修正前 修正后 PGA/g $ {u}_{\mathrm{D}\mathrm{I}} $ $ {u}'_{\mathrm{D}\mathrm{I}} $ PGA/g 80%轻微损伤 0.210 1.531 $ \alpha \cdot DI=1.735 $ 0.244 60%中度损伤 0.183 1.280 $ \alpha \cdot DI=1.450 $ 0.213 40%重度损伤 0.229 1.714 $ \alpha \cdot DI=1.942 $ 0.266 表 7 重现期为50年时的地震动参数修正值(
${\boldsymbol{ \alpha}} $ =1.0123)Table 7. Calibrated peak ground accelerations for the return period of 50 a(
${\boldsymbol{ \alpha }} $ = 1.0123)损伤状态 修正前 修正后 PGA/g $ {u}_{\mathrm{D}\mathrm{I}} $ $ {u}'_{\mathrm{D}\mathrm{I}} $ PGA/g 80%轻微损伤 0.210 1.531 $ \alpha \cdot DI=1.550 $ 0.224 60%中度损伤 0.183 1.280 $ \alpha \cdot DI=1.296 $ 0.195 40%重度损伤 0.229 1.714 $ \alpha \cdot DI=1.735 $ 0.244 -
冯莉,樊燕燕,李子奇等,2020. 高速铁路连续梁桥全寿命周期概率地震损失分析. 铁道科学与工程学报,17(4):815−822. doi: 10.19713/j.cnki.43−1423/u.T20190690Feng L., Fan Y. Y., Li Z. Q., et al., 2020. Probabilistic whole life cycle seismic loss analysis of high-speed railway continuous girder bridge. Journal of Railway Science and Engineering, 17(4): 815−822. (in Chinese) doi: 10.19713/j.cnki.43−1423/u.T20190690 李宏男,成虎,王东升,2018. 桥梁结构地震易损性研究进展述评. 工程力学,35(9):1−16. doi: 10.6052/j.issn.1000-4750.2017.04.0280Li H. N., Cheng H., Wang D. S., 2018. A review of advances in seismic fragility research on bridge structures. Engineering Mechanics, 35(9): 1−16. (in Chinese) doi: 10.6052/j.issn.1000-4750.2017.04.0280 李杰,2018. 工程结构整体可靠性分析研究进展. 土木工程学报,51(8):1−10.Li J., 2018. Advances in global reliability analysis of engineering structures. China Civil Engineering Journal, 51(8): 1−10. (in Chinese) 陆新征,叶列平,2010. 基于IDA分析的结构抗地震倒塌能力研究. 工程抗震与加固改造,32(1):13−18. doi: 10.3969/j.issn.1002-8412.2010.01.003Lu X. Z., Ye L. P., 2010. Study on the seismic collapse resistance of structural system. Earthquake Resistant Engineering and Retrofitting, 32(1): 13−18. (in Chinese) doi: 10.3969/j.issn.1002-8412.2010.01.003 吕大刚,李晓鹏,王光远,2006. 基于可靠度和性能的结构整体地震易损性分析. 自然灾害学报,15(2):107−114. doi: 10.3969/j.issn.1004-4574.2006.02.018Lü D. G., Li X. P., Wang G. Y., 2006. Global seismic fragility analysis of structures based on reliability and performance. Journal of Natural Disasters, 15(2): 107−114. (in Chinese) doi: 10.3969/j.issn.1004-4574.2006.02.018 于晓辉,周洲,吕大刚,2022. 钢筋混凝土框架结构的损伤状态相关余震易损性分析. 建筑结构学报,43(4):8−16. doi: 10.14006/j.jzjgxb.2020.0323Yu X. H., Zhou Z., Lü D. G., 2022. Damage-state-dependent aftershock fragility analysis for reinforced concrete frame structures. Journal of Building Structures, 43(4): 8−16. (in Chinese) doi: 10.14006/j.jzjgxb.2020.0323 袁万城,王征南,庞于涛等,2016. 连续梁桥在主震-余震序列波下的地震易损性分析. 哈尔滨工程大学学报,37(12):1671−1676. doi: 10.11990/jheu.201509064Yuan W. C., Wang Z. N., Pang Y. T., et al., 2016. Seismic fragility analysis of a continuous girder bridge subject to an earthquake mainshock-aftershock sequence. Journal of Harbin Engineering University, 37(12): 1671−1676. (in Chinese) doi: 10.11990/jheu.201509064 张明,金峰,2015. 结构可靠度计算. 北京:科学出版社.Zhang M. , Jin F. , 2015. Structural reliability computations. Beijing: Science Press. (in Chinese) Deierlein G. G. , Krawinkler H. , Cornell C. A. , 2003. A framework for performance-based earthquake engineering. In: Proceedings of 2003 Pacific Conference on Earthquake Engineering. Vancouver. Dong Y., Frangopol D. M., Saydam D., 2014. Sustainability of highway bridge networks under seismic hazard. Journal of Earthquake Engineering, 18(1): 41−66. doi: 10.1080/13632469.2013.841600 Ghosh J., Padgett J. E., Sánchez-Silva M., 2015. Seismic damage accumulation in highway bridges in earthquake-prone regions. Earthquake Spectra, 31(1): 115−135. doi: 10.1193/120812EQS347M Hwang H. , Liu J. B. , Chiu Y. H. , 2001. Seismic fragility analysis of highway bridges. Memphis: Center for Earthquake Research and Information the University of Memphis. Karim K. R., Yamazaki F., 2001. Effect of earthquake ground motions on fragility curves of highway bridge piers based on numerical simulation. Earthquake Engineering & Structural Dynamics, 30(12): 1839−1856. Mackie K. , Stojadinović B. , 2003. Seismic demands for performance-based design of bridges. Berkeley: Pacific Earthquake Engineering Research Center, University of California, 1−113. Moehle J. , Deierlein G. G. , 2004. A framework methodology for performance-based earthquake engineering. In: Proceedings of the 13th World Conference on Earthquake Engineering. Vancouver: WCEE. Muntasir Billah A. H. M., Shahria Alam M., 2015. Seismic fragility assessment of highway bridges: a state-of-the-art review. Structure and Infrastructure Engineering, 11(6): 804−832. doi: 10.1080/15732479.2014.912243 Pathak S., Watt S., Khennane A., et al., 2020. Dynamical systems approach for the evaluation of seismic structural collapse and its integration into PBEE framework. Soil Dynamics and Earthquake Engineering, 135: 106184. doi: 10.1016/j.soildyn.2020.106184 Porter K. A. , 2003. An overview of PEER’s performance-based earthquake engineering methodology. In: Proceedings of the Ninth International Conference on Applications of Statistics and Probability in Civil Engineering (ICASP9). San Francisco. Shome N., Allin Cornell C., Bazzurro P., et al., 1998. Earthquakes, records, and nonlinear responses. Earthquake Spectra, 14(3): 469−500. doi: 10.1193/1.1586011 Yoon Y. H., Ataya S., Mahan M., et al, 2019. Probabilistic damage control application: implementation of performance-based earthquake engineering in seismic design of highway bridge columns. Journal of Bridge Engineering, 24(7): 04019068. doi: 10.1061/(ASCE)BE.1943-5592.0001443 -





 下载:
下载:





 下载:
下载: