Damage Analysis of Girder Bridge Under Near-fault Pulse-like Earthquake Motion Considering Wave Propagation Effect
-
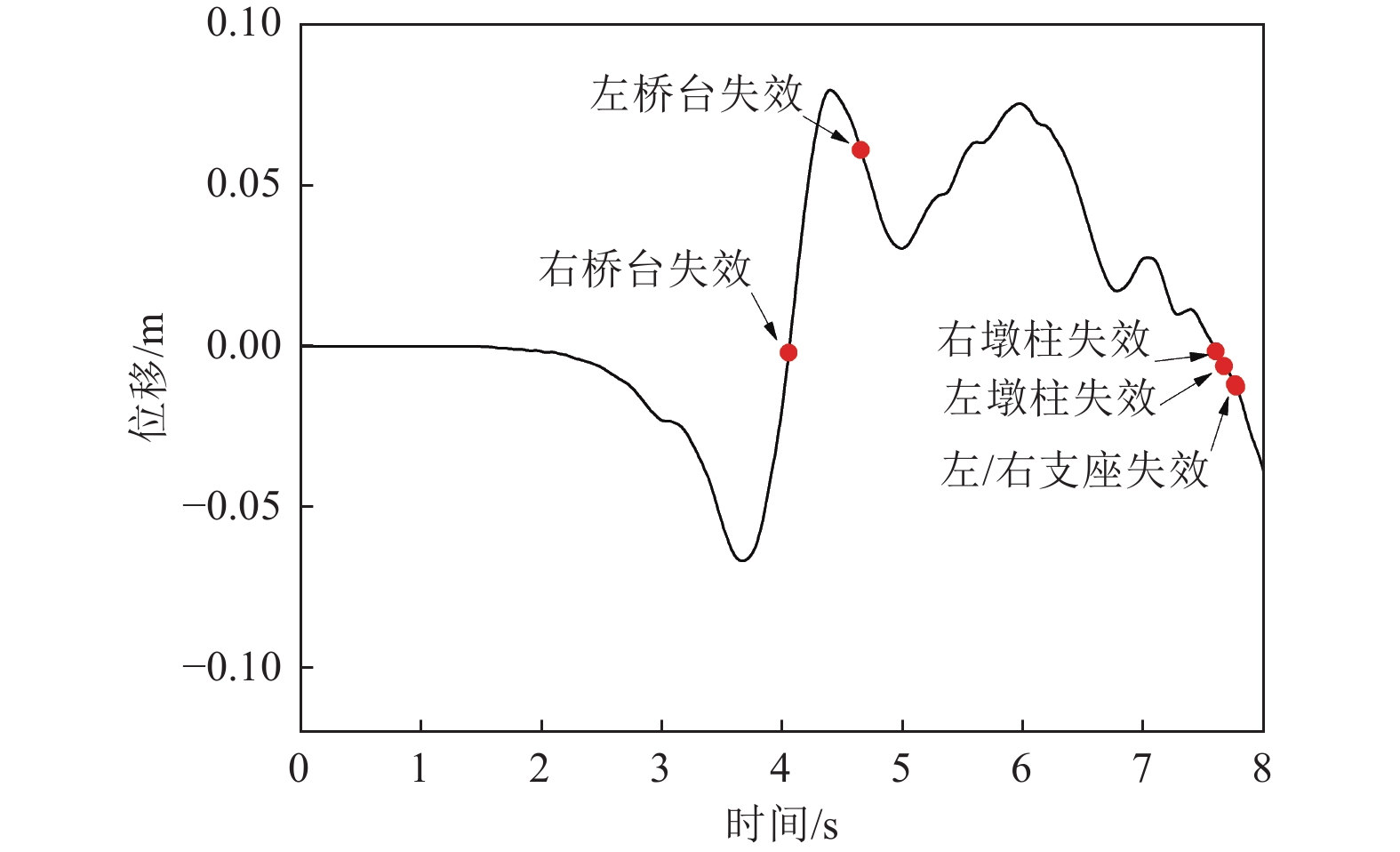
摘要: 在近断层地震动激励的初始阶段，输入地震动从结构底部以波动形式向上传播，然而波动效应对近断层脉冲型地震动作用下梁桥结构地震破坏过程的影响特征尚不明确。为此，利用数值分析方法对某三跨梁桥在近断层脉冲型地震动作用下考虑波动效应的破坏过程、破坏机理开展研究。研究结果表明，在近断层脉冲型地震动作用下，墩柱底部截面曲率发展较合理，梁桥墩顶支座抗震能力较强，梁桥桥台处容许剪切位移是桥梁结构的抗震弱点，因此，在近断层地区的梁桥桥台抗震措施设计中应保证具有充足的容许剪切位移。Abstract: During the initial phase of near-fault seismic excitation, the input ground motion propagates inside the structure from bottom to top in the form of waves, but the influence of the wave propagation effect on the seismic damage process of girder bridge structures under the action of near-fault pulse-type ground motion is not clear. In this paper, numerical analysis method is utilized to study the failure process and failure mechanism of a three-span girder bridge under the action of near-fault pulse-type earthquake considering the wave propagation effect. The results show that the development of the curvature of the bridge pier section is reasonable enough during the seismic excitation process. The bearing at the pier top shows great aseismic capability, the allowable shear displacement of the abutment is the weak point of the whole girder bridge system. Thus, it is important to guarantee a reasonable allowable shear displacement for the abutment of girder bridges located in the near-fault region. Our results provide a reference value for the seismic design of girder bridges in near-fault regions.
-
引言
悬索桥结构主要由锚碇、索塔、缆索系统、加劲梁和附属结构组成，具有结构简单、轻便、易于标准化、构件易运输、方便悬吊拼装、施工限制较少等特点（铁道部大桥工程局桥梁科学研究所，1996；钱炜，2007），是特大桥梁的主要类型（李光军，2012）。地震是造成桥梁结构破坏的主要原因之一，桥梁是联系外界与地震灾区的重要纽带，桥梁一旦破坏，将中断通往灾区的通道，影响救灾工作进展，造成较大损失（刘润舟，2008）。因此，对悬索桥结构进行地震响应分析具有重要意义。国内外学者已开展了大跨度桥梁多点激励地震响应研究，如许基厚等（2019）对非对称单侧混合梁斜拉桥多点激励地震响应进行研究，分析了行波效应对塔顶、主梁、塔底等关键位置动力响应的影响，并研究了不同入射角对桥梁动力响应的影响，认为多点激励下主塔内力计算结果偏小，而主梁内力计算结果偏大，地震激励对非对称大跨度斜拉桥内力产生较大影响，内力变化可达20%；张凡等（2016）对不同波速下斜拉桥地震响应进行了分析，认为波速的影响显著；王再荣等（2016）分析行波效应下斜拉桥地震响应，得出辅助墩耗能降低、桥塔损伤增大的结论；刘旭政等（2018）考虑2种地震波和3种视波速的不同组合，以一座实际工程高桩大跨度连续刚构桥为例，研究行波效应对桥梁地震响应的影响，认为随着视波速的增大，行波效应对桥梁内力的影响程度减小；梅泽洪等（2017）研究了考虑局部场地效应桥梁结构在非一致激励下地震响应的特点，认为下部结构响应所受影响较小，而上部结构所受影响明显，考虑行波效应的非一致激励对桥梁地震响应有减弱效果；黎璟等（2019）以铁路工程实际桥梁为例，研究了非一致激励下大跨度铁路斜拉桥地震响应规律，认为在非一致激励下塔顶位移响应峰值与墩底弯矩响应峰值均随着相位差呈周期性变化，且变化周期与结构一阶自振周期基本一致。
本文选取某三跨两铰连续体系悬索桥，已有研究结果表明线性分析方法和非线性分析方法得到的该桥地震响应结果相近（Fleming等，1980；李丽等，2018a）。因此利用大型通用有限元软件ANSYS建立三维弹性有限元模型，分析一致激励与多点激励下悬索桥地震响应，研究其抗震性能。
1. 桥梁结构动力响应分析方法
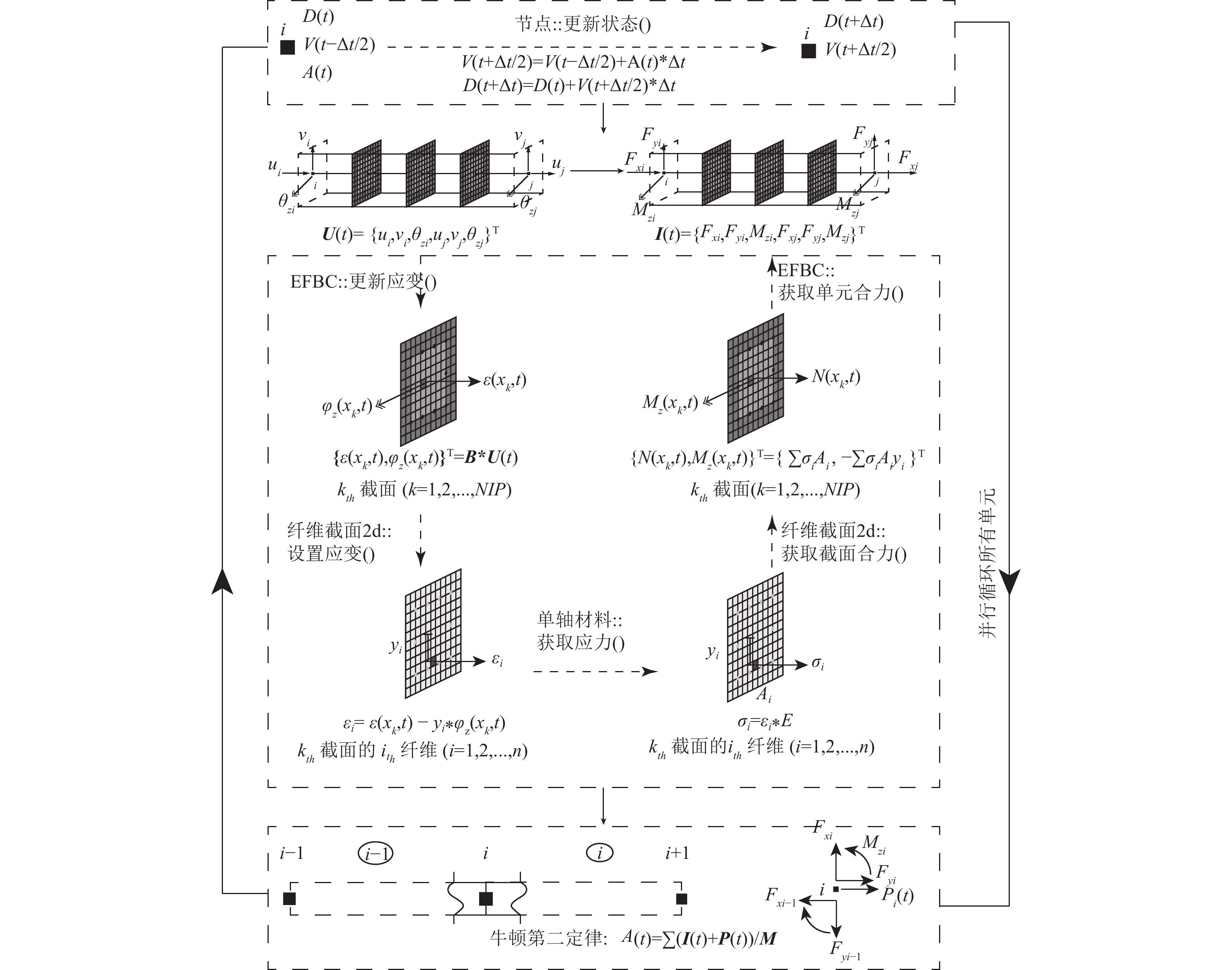
桥梁结构动力响应分析方法主要包括一致激励动态时程分析方法及多点激励动态时程分析方法，目前多点激励动态时程分析方法的应用较多，该方法考虑地震行波效应和局部场地效应，对各独立基础或支撑结构输入不同的设计反应谱或加速度时程进行计算（张沧海，2011），主要包括相对运动法和大质量法（范立础等，2001；Leger等，1990）。相对运动法通过支撑点的加速度时程计算非支撑点的动力响应。大质量法是对结构模型进行动力等效的分析方法，在处理多点激励问题时需解除支撑点沿地震作用方向的约束，并赋予节点大质量，其值通常远大于结构体系的总质量（苏成等，2008）。本文采用相对运动法计算悬索桥在多点激励下的响应。
2. 桥梁结构概况
三跨连续悬索桥主跨124m，主梁设计为连续加劲梁，加劲梁采用正交异性板流线形扁平钢箱梁，梁高1.88m，宽（含风嘴）10.8m；纵向分配梁宽0.16m，厚0.28m；纵向斜腹杆，横向内侧竖杆，横向内、外侧斜腹杆截面宽度均为0.16m，厚度均为0.2m；纵向上、下弦杆截面宽度均为0.3m，厚度均为0.2m；纵向竖杆截面宽0.2m，厚0.18m；横向外侧竖杆截面宽0.2m，厚0.24m；横向上弦杆截面宽0.18m，厚0.24m；横向下弦杆截面宽0.18m，厚0.2m；抗风桁架截面宽0.12m，厚0.12m。主塔为H形，塔高36m，南、北桥塔未设置上、下横梁。塔柱截面宽2m，厚4m；柱间连接件截面宽4m，厚2m。桥面以上有3根主缆，南、北边跨吊索各12根，主跨共30根，索间距4m。主缆截面直径为0.079m，吊索截面直径为0.039m。桥梁结构各构件材料物理参数见表 1。
表 1 桥梁结构材料物理参数Table 1. The material physical parameters of bridge structure构件 材料 弹性模量/Pa 泊松比 密度/kg·m-3 主缆 钢丝绳 21.0×1010 0.167 7850 吊索 钢丝绳 21.0×1010 0.167 7850 加劲桁架、纵梁 C30混凝土 3.0×1010 0.300 2500 桥塔、桥面板 C20混凝土 2.8×1010 0.300 2500 3. 输入地震动
选取常用的Kobe地震波对悬索桥进行地震响应分析，顺桥向输入的加速度时程曲线如图 1，加速度峰值为0.345g，持时为24.79s。
图 1 输入Kobe波加速度时程曲线（顺桥向）Figure 1. The input Kobe wave of acceleration time history curve (Along the bridge)对于大跨度悬索桥，输入地震动时常将水平地震系数的1/2或2/3作为竖向地震系数，组合方式通常为顺桥向与竖向组合、横桥向与竖向组合（李国豪，2003），因此，进行一致激励下的地震响应分析时，本文将竖向地震系数取为水平地震系数的1/2，并选取顺桥向与竖向组合；进行多点激励下的地震响应分析时，将竖向地震系数取为水平地震系数的1/2，先对加速度时程曲线进行2次积分，第1次积分得到速度时程曲线，并将其进行基线校正，将校正后的速度时程曲线再次积分并进行基线校正，得到位移时程曲线，如图 2。
图 2 Kobe波位移时程曲线（顺桥向）Figure 2. The input Kobe wave of displacement time history curve (Along the bridge)多点激励位移输入位置为图 3中①-④桥梁支座位置，计算时考虑不同支座输入波形相位滞后现象，采用对不同支座延迟输入位移时程的方法考虑行波效应（刘春城等，2004；王蕾等，2006；严琨等，2017），根据大跨建筑结构多点输入地震响应计算结果（李丽等，2018b；景鹏旭等，2017）与抗震设计方法研究结果（江洋，2010），取视波速为1000m/s，根据支座间距计算各支座位移输入延迟时间，见表 2。
图 3 桥梁结构立面图及荷载激励点布置Figure 3. The elevation and load excitation point layout of the bridge表 2 输入位移时程延迟时间（s）Table 2. The delay time of input displacement time history (s)支座编号 ① ② ③ ④ 时间间隔 0.000 0.050 0.174 0.224 4. 有限元模型的建立
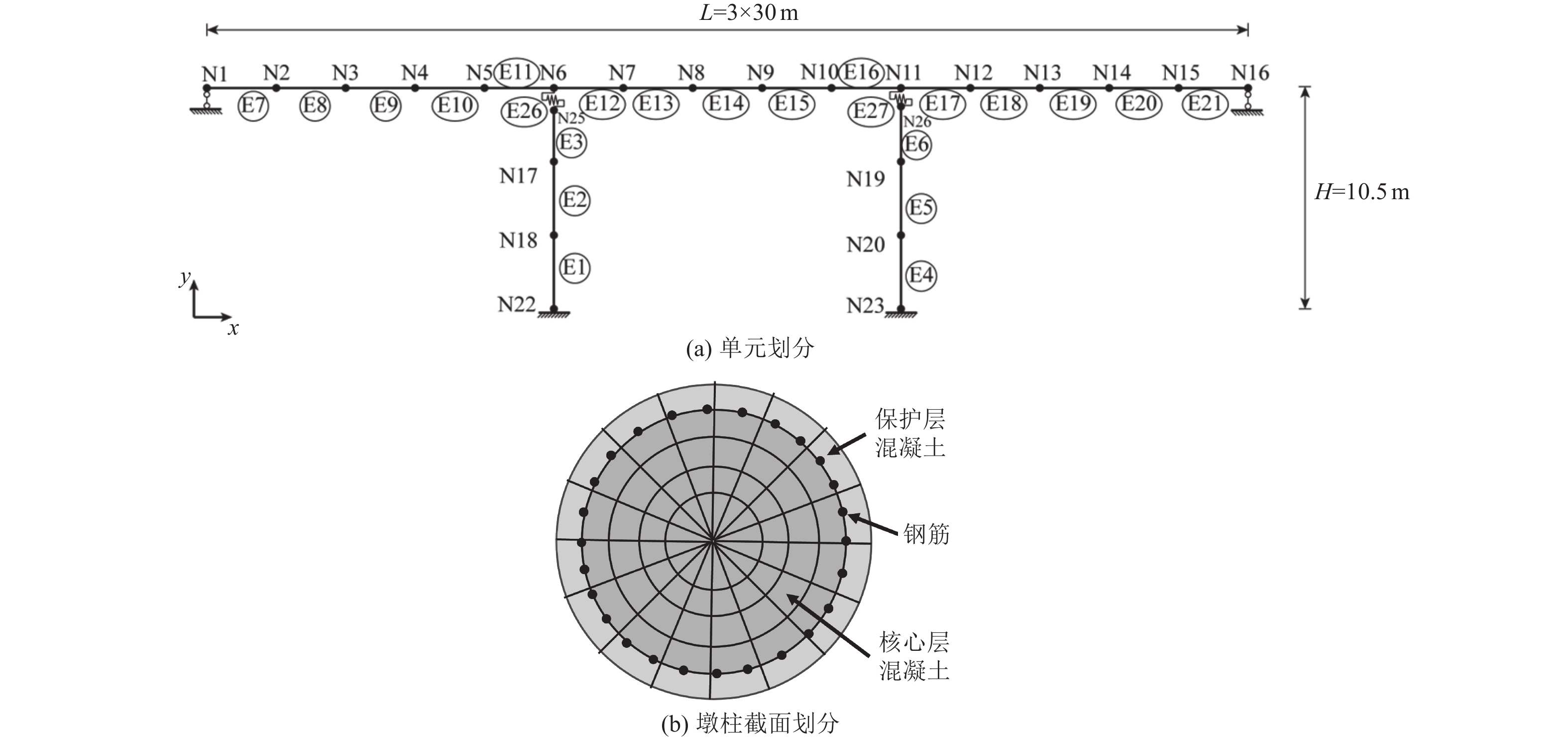
应用大型通用有限元软件ANSYS建立悬索桥三维有限元模型（图 4），应用link10单元模拟主缆及吊索，应用beam4单元模拟加劲桁架、纵向分配梁、抗风桁架、桥塔，应用shell63单元模拟桥面板。主缆及吊索初应变设为0.0043，先对结构进行重力分析，得到结构初始应力，然后对其进行模态分析，计算桥梁结构前2阶振型圆频率，应用APDL语言编制求解瑞利阻尼系数α和β：
ALPHAD, 2*DAMPRATIO*FREQ1*2*3.1415926
BETAD, 2*DAMPRATIO/(FREQ1*2*3.1415926)
5. 地震响应分析结果
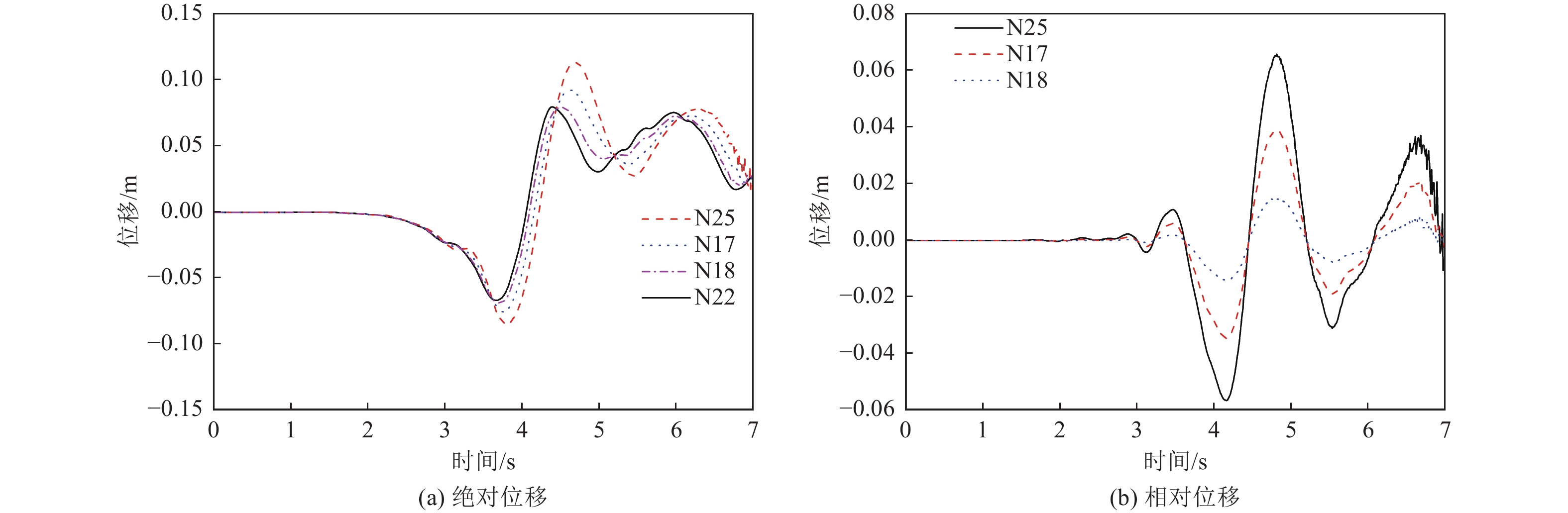
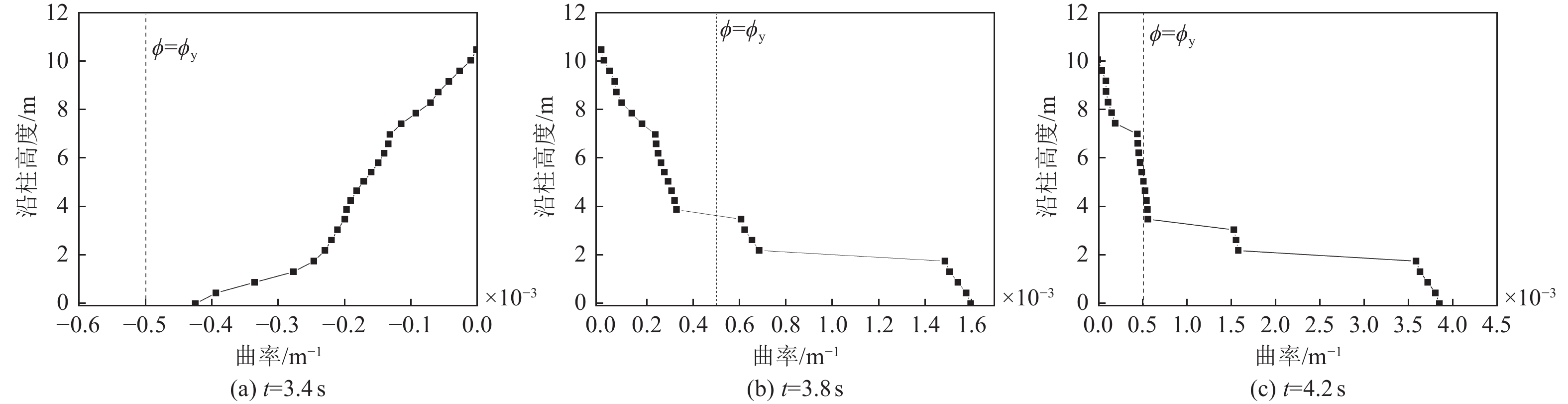
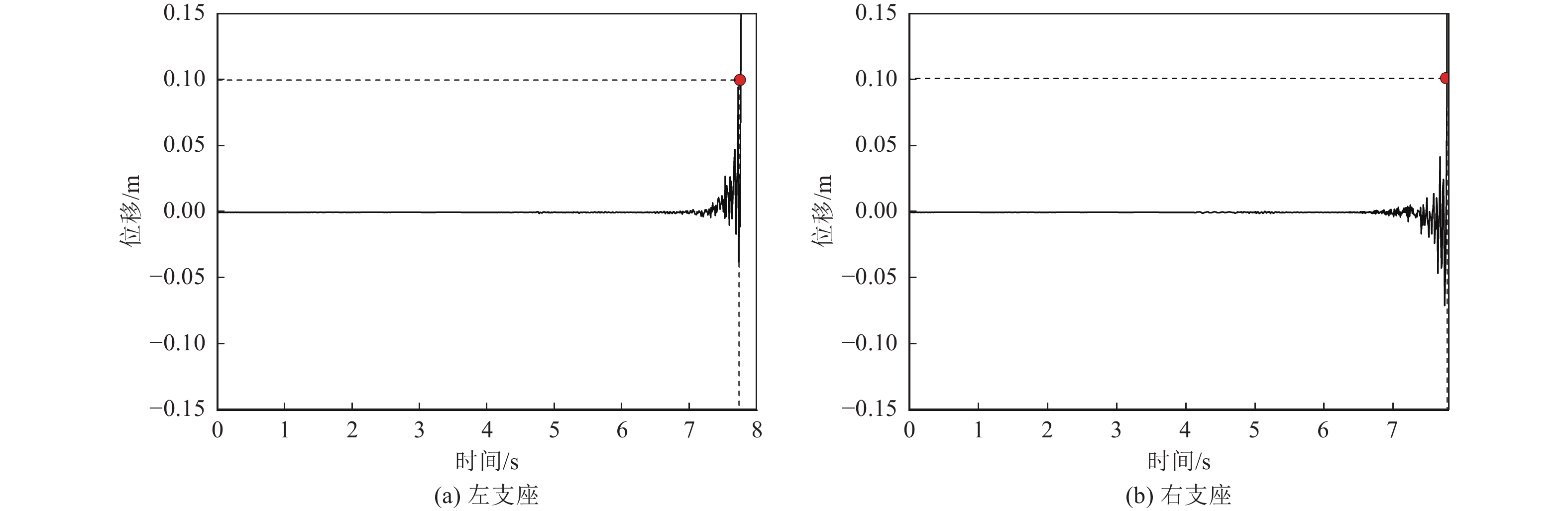
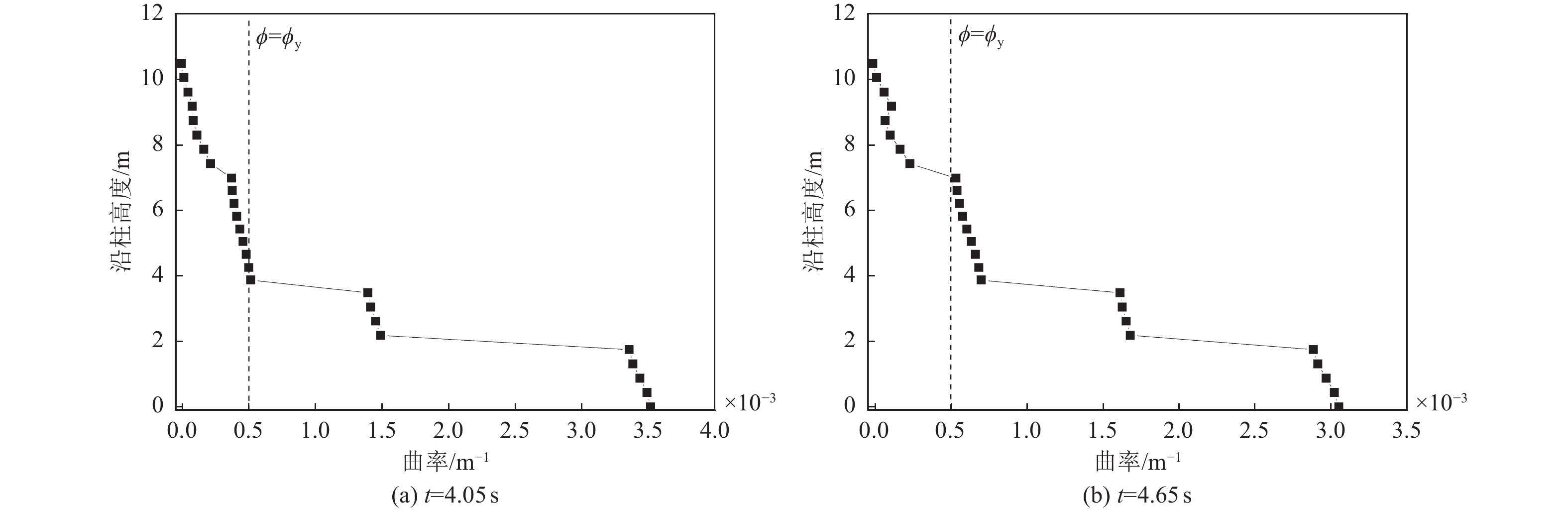
计算得到主梁位移及南、北桥塔塔柱沿柱高方向的位移、轴力、剪力及弯矩包络图，如图 5-7。
图 5 主梁顺桥向最大位移包络图Figure 5. Envelope diagram of maximum displacement of main beam along bridge direction
图 6 南桥塔纵向位移、轴力、剪力及弯矩包络图Figure 6. The envelope of displacement, axial force, shearing force and bending moment of the occurrence bridge tower longitudinal
图 7 北桥塔纵向位移、轴力、剪力及弯矩包络图Figure 7. The envelope of displacement, axial force, shearing force and bending moment of the north bridge tower longitudinal由图 5可知，多点激励下主梁最大位移略高于一致激励下主梁最大位移，总体变化不大。由图 6、图 7可知，一致激励下南、北桥塔沿塔高的位移、轴力、剪力及弯矩计算结果相同，这符合有限元计算基本原理。多点激励下南、北桥塔计算结果不对称，说明地震波输入方式对计算结果具有一定影响，由于本研究中悬索桥跨度不大，所以一致激励和多点激励下计算结果差别不大。
6. 结语
本文对某对称悬索桥在一致激励及视波速1000m/s的多点激励下的地震响应进行分析，并对计算结果进行分析。计算结果表明地震波输入方式对模拟结果具有一定影响，计算结果存在一定差异，主梁在多点激励下的位移计算结果略高于一致激励下的计算结果，桥塔最大位移发生在塔中与塔顶之间，最大弯矩、轴力、剪力均发生在塔根处。
-
图 1 三跨梁桥有限元模型示意
Figure 1. Schematic diagram for the finite element model of three-span girder bridge
图 6 RSN77作用下左墩不同高度结点纵桥向位移时程
Figure 6. Nodal displacement of variant height along the left pier under RSN77 seismic excitation
图 12 地震动RSN77作用下构件失效过程
Figure 12. Failure process of structural members under the seismic excitation of RSN77
图 13 不同时刻左墩柱截面曲率沿墩高分布情况
Figure 13. Section curvature distribution of height along the left pier at different time
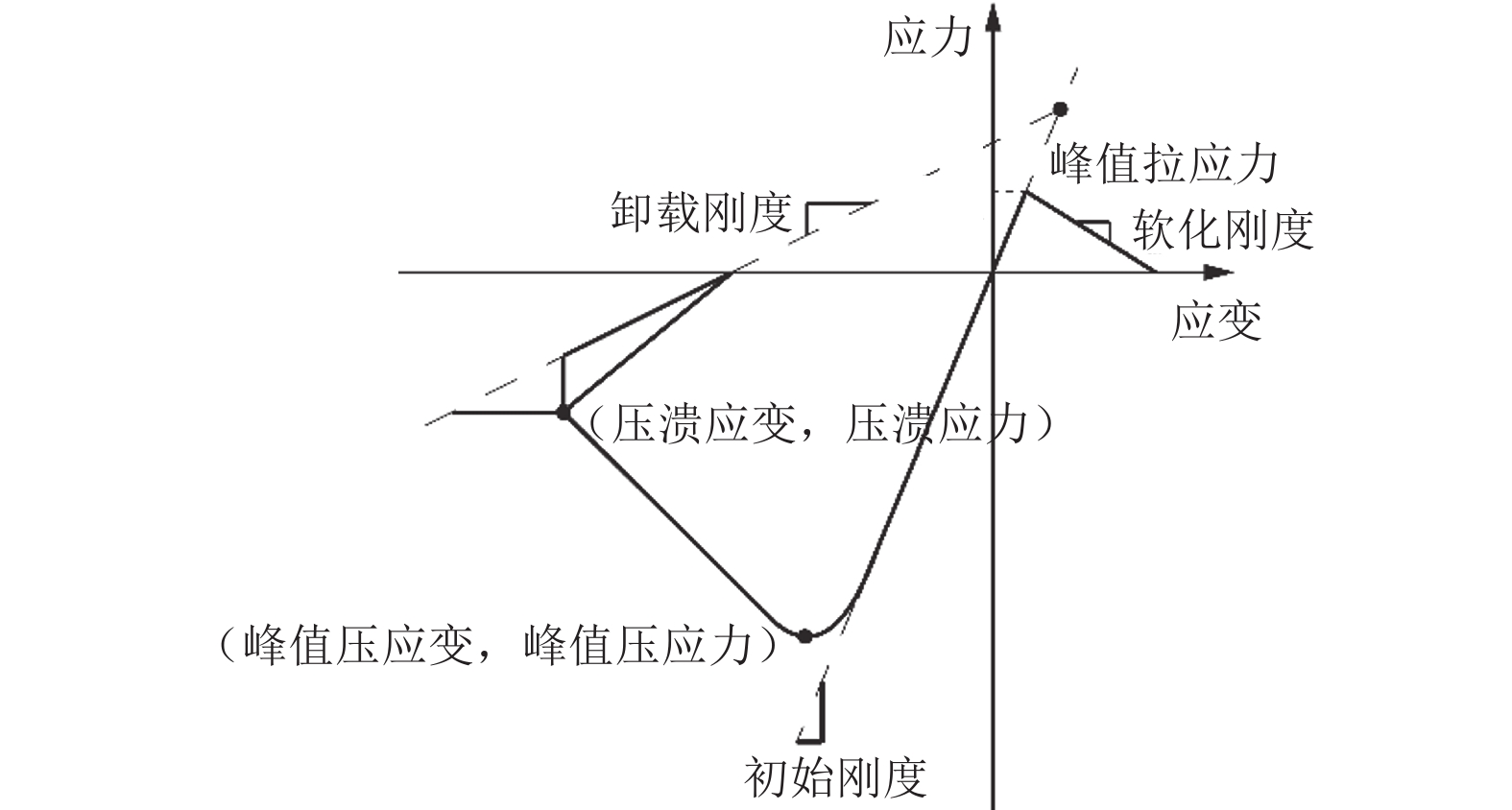
表 1 混凝土材料本构参数
Table 1. Parameters for the core and cover concrete material
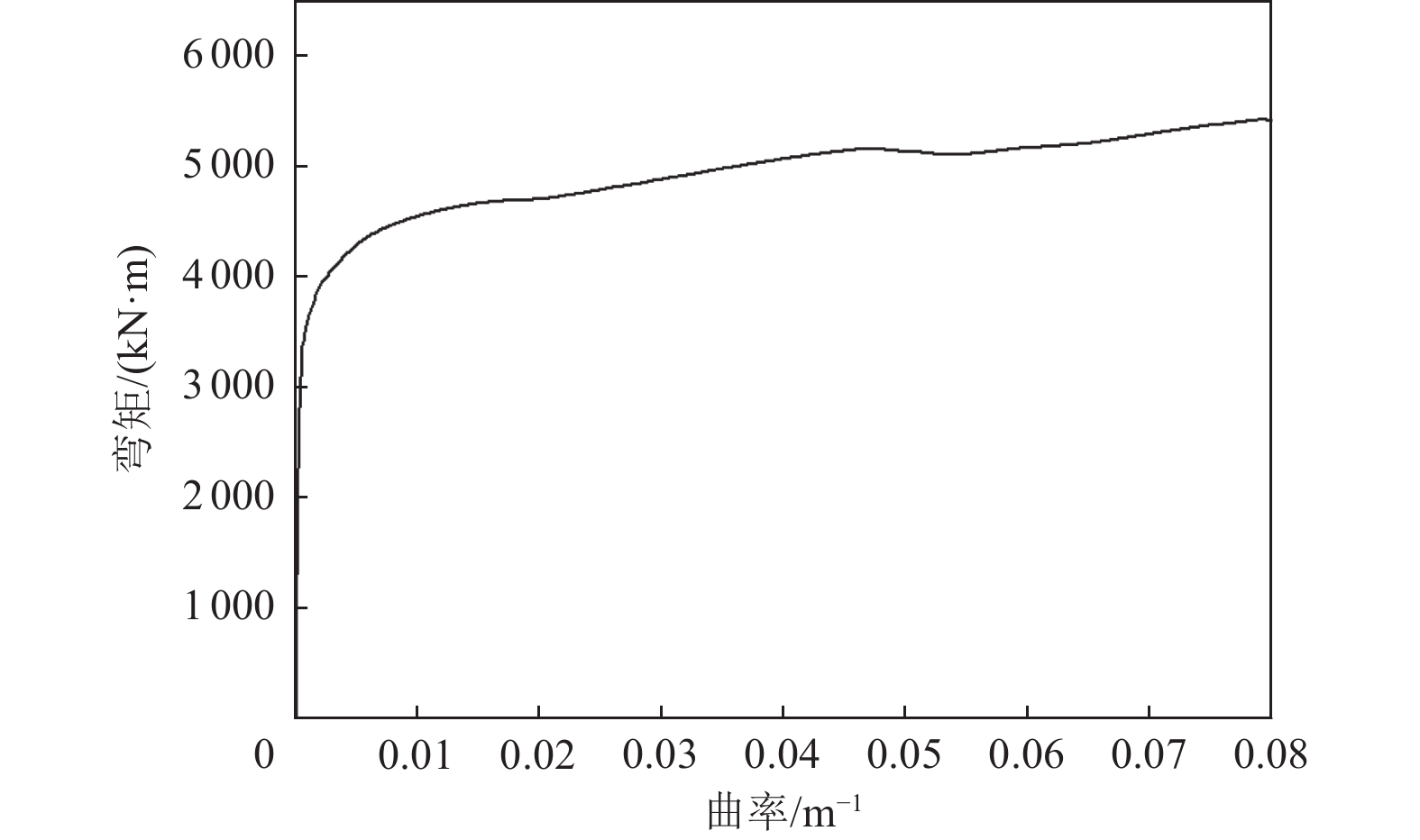
材料参数 峰值压应力$ /\mathrm{M}\mathrm{P}\mathrm{a} $ 峰值压应变$ {\varepsilon }_{0} $ $ \mathrm{压}\mathrm{溃}\mathrm{应}\mathrm{力}/\mathrm{M}\mathrm{P}\mathrm{a} $ 压溃应变${\varepsilon }_{{\rm{u}}}$ 峰值拉应力$ /\mathrm{M}\mathrm{P}\mathrm{a} $ 软化刚度$ /\mathrm{G}\mathrm{P}\mathrm{a} $ 核心层 −30.85 −0.002 1 −6.2 −0.012 2.16 300 保护层 −30.00 −0.002 0 −6.0 −0.005 2.10 300 
下载: 导出CSV
表 2 不同国家规范给出的等效塑性铰长度计算公式
Table 2. Computing formula for the equivalent length of pier plastic hinge in China, USA, Europe and Japan
规范名称 等效塑性铰长度$ {L}_{\mathrm{p}} $计算公式 按规范公式计算
得到的$ {L}_{\mathrm{p}} $/m美国Version 1.7《Seismic design criteria》 $0.08 L+0.022{f}_{{\rm{s}}}{d}_{{\rm{s}}}$ 0.86 中国JTG/T 2231-01—2020《公路桥梁抗震设计规范》 $0.08 L+0.022{f}_{{\rm{s}}}{d}_{{\rm{s}}}\geqslant \mathrm{m}\mathrm{i}\mathrm{n}\left(0.044{f}_{{\rm{s}}}{d}_{{\rm{s}}},2/3 h\right)$ 0.86 欧洲BS EN 1998-2: 2005+A2:2011《Eurocode 8-Design of structures for earthquake resistance-part 2: bridges》 $0.1 L+0.015{f}_{{\rm{s}}}{d}_{{\rm{s}}}$ 1.18 日本《Specifications for highway bridges-part V seismic design》 $0.2 L-0.1 h;0.1 h\leqslant{L}_{{\rm{p}}}\leqslant 0.5 h$ 0.75
下载: 导出CSV
-
姜迎春, 2013. 结构地震响应波动分析的被研究块体方法. 大连: 大连理工大学.Jiang Y. C. , 2013. Investigated lump method for wave propagation analysis of earthquake response of structure. Dalian: Dalian University of Technology. (in Chinese) 罗韧, 许慧荣, 李鸿晶等, 2010. 汶川地震中百花大桥倒塌模拟分析. 土木建筑与环境工程, 32（S2）: 49—51. 吕大刚, 王光远, 2001. 基于损伤性能的抗震结构最优设防水准的决策方法. 土木工程学报, 34（1）: 44—49Lü D. G. , Wang G. Y. , 2001. Decision-making method of optimal fortification level for aseismic structures based on damage performance. China Civil Engineering Journal, 34(1): 44—49. (in Chinese) 邵光强, 刘开, 蒋丽忠等, 2017. 高速铁路桥墩等效塑性铰长度研究. 铁道工程学报, 34（7）: 53—59Shao G. Q. , Liu K. , Jiang L. Z. , et al. , 2017. Study of plastic hinge length in high-speed railway bridge piers. Journal of Railway Engineering Society, 34(7): 53—59. (in Chinese) 王伟军, 虞庐松, 李子奇等, 2022. 近断层地震特性对隔震曲线连续梁桥地震响应的影响. 地震工程学报, 44（1）: 86—92, 127Wang W. J. , Yu L. S. , Li Z. Q. , et al. , 2022. Influence of near-fault ground motion characteristics on the seismic response of isolated continuous curved girder bridges. China Earthquake Engineering Journal, 44(1): 86—92, 127. (in Chinese) 夏春旭, 2020. 近断层地震动脉冲识别及考虑波动效应的桥梁地震破坏分析. 大连: 大连理工大学.Xia C. X. , 2020. Pulse identification of near-fault earthquake ground motion and analysis of the seismic damage of bridge considering wave-propagation effect. Dalian: Dalian University of Technology. (in Chinese) 杨伟, 欧进萍, 2009. 基于能量原理的Park & Ang损伤模型简化计算方法. 地震工程与工程振动, 29（2）: 159—165Yang W. , Ou J. P. , 2009. A simplified method for computing Park & Ang damage model based on energy concept. Journal of Earthquake Engineering and Engineering Vibration, 29(2): 159—165. (in Chinese) 曾永平, 董俊, 陈克坚等, 2020. 近断层高铁简支梁桥抗震性能分析研究. 铁道工程学报, 37（12）: 70—76Zeng Y. P. , Dong J. , Chen K. J. , et al. , 2020. Research on the seismic performance of simply supported beam bridge of near-fault high-speed railway. Journal of Railway Engineering Society, 37(12): 70—76. (in Chinese) 张海燕, 樊燕燕, 李江龙, 2022. 铁路梁式桥地震灾害损失评估. 世界地震工程, 38（1）: 139—147Zhang H. Y. , Fan Y. Y. , Li J. L. , 2022. Earthquake disaster loss assessment of railway beam bridge. World Earthquake Engineering, 38(1): 139—147. (in Chinese) 张鹏, 2018. 跨断层简支梁桥的概率性地震损伤特性研究. 北京: 北京交通大学.Zhang P. , 2018. Study on the probabilistic seismic damage character of simply supported girder bridge under fault-crossing earthquakes. Beijing: Beijing Jiaotong University. (in Chinese) Beolchini G. C., Galeota D., Giammatteo M. M., et al., 2000. Seismic behavior of high strength RC columns. In: Proceedings of the 12 th World Conference on Earthquake Engineering. Auckland: WCEE, 1—8. Bi K. M. , Ren W. X. , Cheng P. F. , et al. , 2015. Domino-type progressive collapse analysis of a multi-span simply-supported bridge: a case study. Engineering Structures, 90: 172—182. doi: 10.1016/j.engstruct.2015.02.023 Priestley M. J. N. , Park R. , 1987. Strength and ductility of concrete bridge columns under seismic loading. ACI Structural Journal, 84(1): 61—76. Salem H. M. , Helmy H. M. , 2014. Numerical investigation of collapse of the Minnesota I-35 W bridge. Engineering Structures, 59: 635—645. doi: 10.1016/j.engstruct.2013.11.022 Xia C. X. , Liu C. G. , 2018. Explicit fiber element and its application to piers under multipulse near-fault earthquake motion. The Structural Design of Tall and Special Buildings, 27(18): e1547. doi: 10.1002/tal.1547 Xia C. X. , Liu C. G. , 2019. Identification and representation of multi-pulse near-fault strong ground motion using adaptive wavelet transform. Applied Sciences, 9(2): 259. doi: 10.3390/app9020259 Xu Z. , Lu X. Z. , Guan H. , et al. , 2013. Progressive-collapse simulation and critical region identification of a stone arch bridge. Journal of Performance of Constructed Facilities, 27(1): 43—52. doi: 10.1061/(ASCE)CF.1943-5509.0000329 Ye L. P. , Otani S. , 1999. Maximum seismic displacement of inelastic systems based on energy concept. Earthquake Engineering & Structural Dynamics, 28(12): 1483—1499. Zhou R. , Zong Z. H. , Huang X. Y. , et al. , 2014. Seismic response study on a multi-span cable-stayed bridge scale model under multi-support excitations. Part II: numerical analysis. Journal of Zhejiang University-SCIENCE A, 15(6): 405—418. doi: 10.1631/jzus.A1300340 Zong Z. H. , Xia Z. H. , Liu H. H. , et al. , 2016. Collapse failure of prestressed concrete continuous rigid-frame bridge under strong earthquake excitation: testing and simulation. Journal of Bridge Engineering, 21(9): 04016047. doi: 10.1061/(ASCE)BE.1943-5592.0000912 -
下载:
点击查看大图
计量
- 文章访问数: 79
- HTML全文浏览量: 62
- PDF下载量: 18
- 被引次数: 0
