Effect of Saturated Complex Site on the Seismic Response of Continuous Long-span Beam Bridges
-
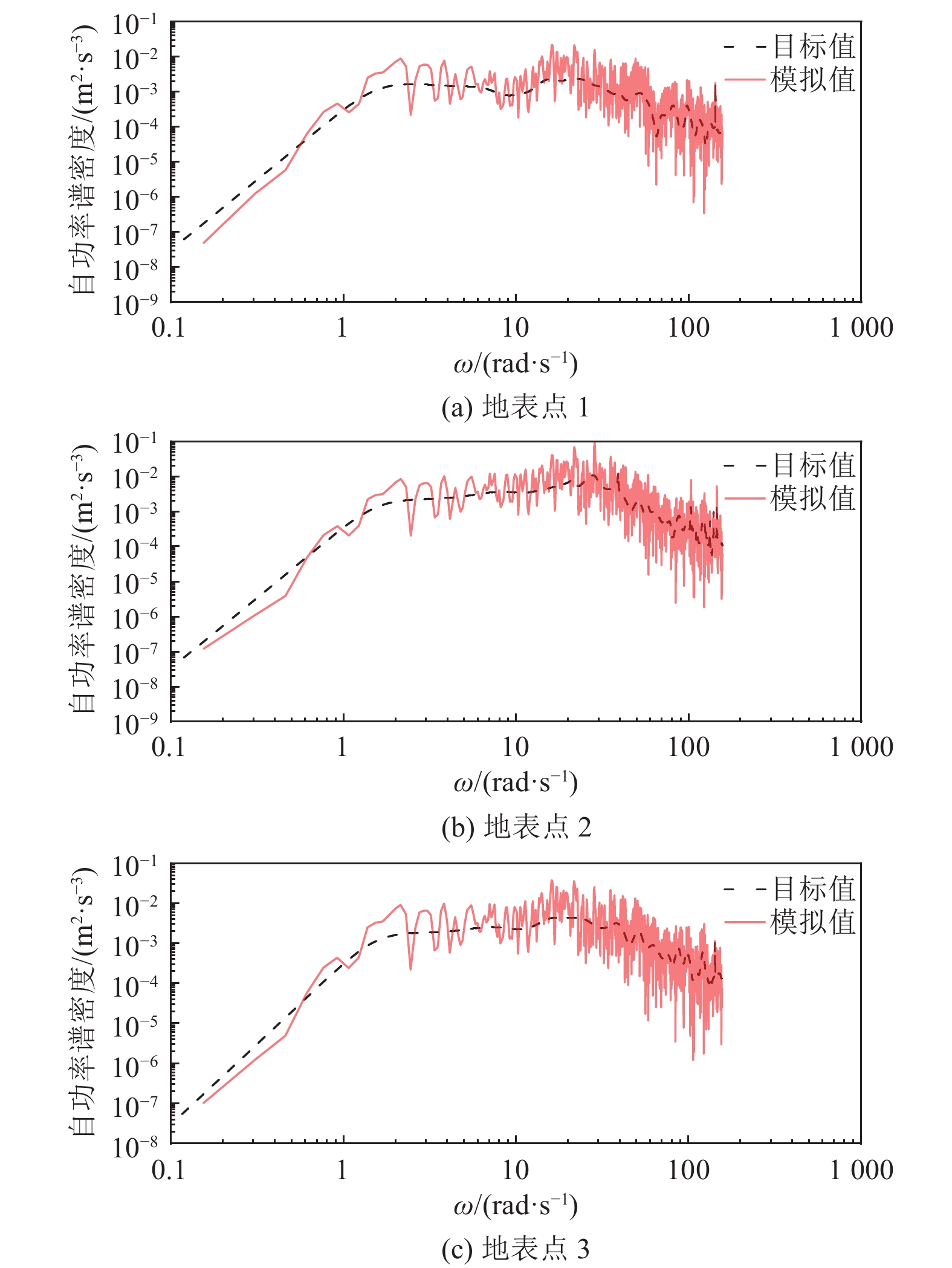
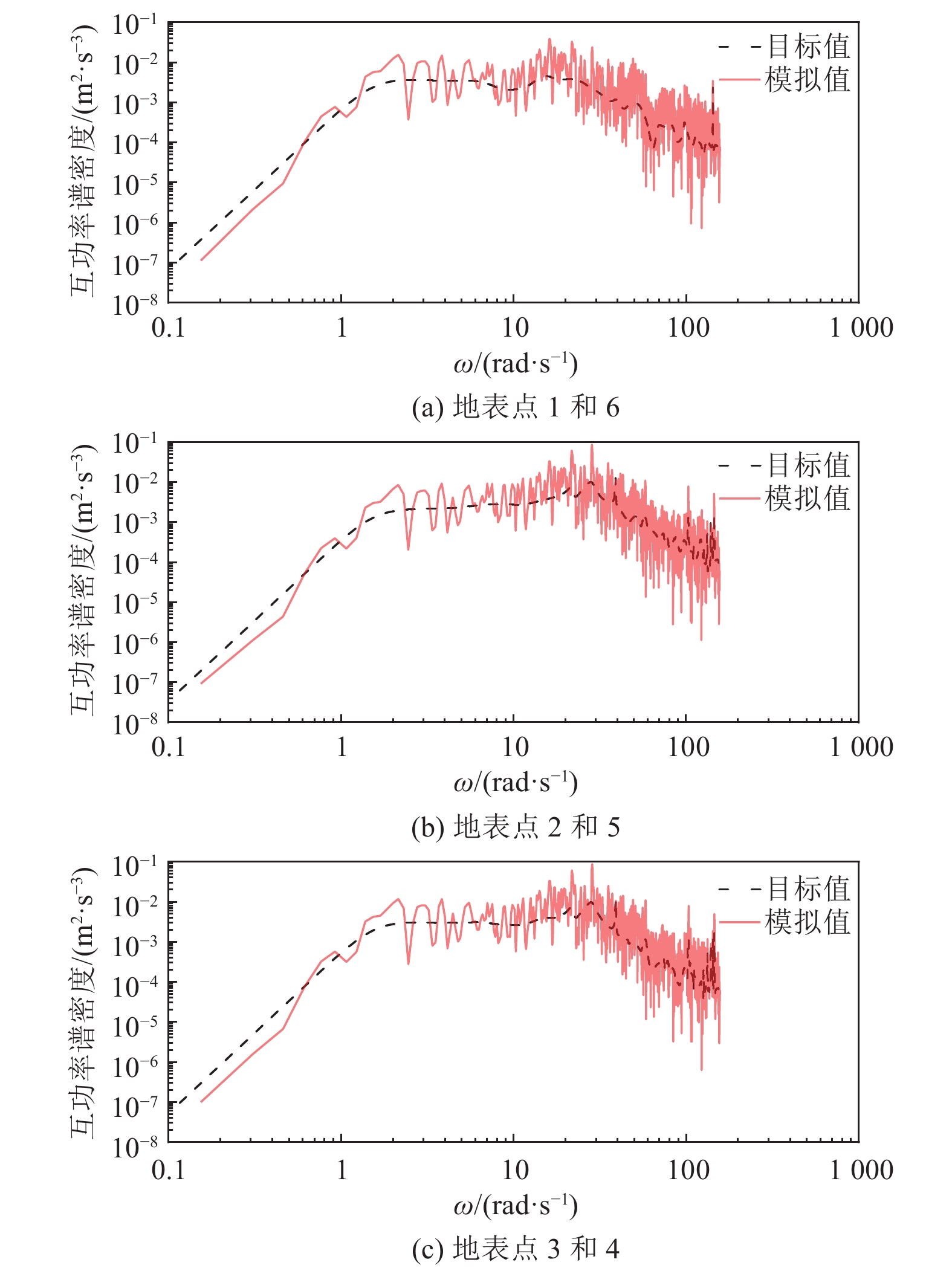
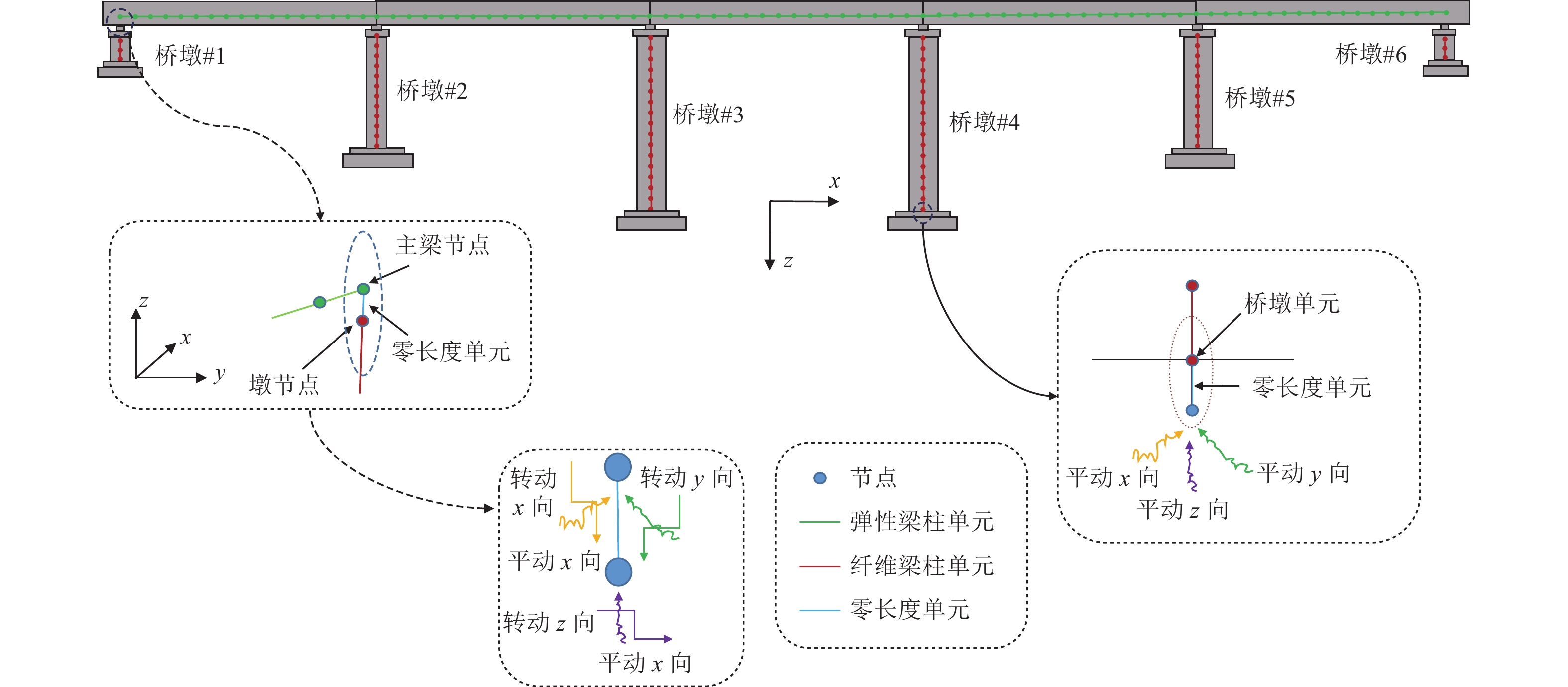
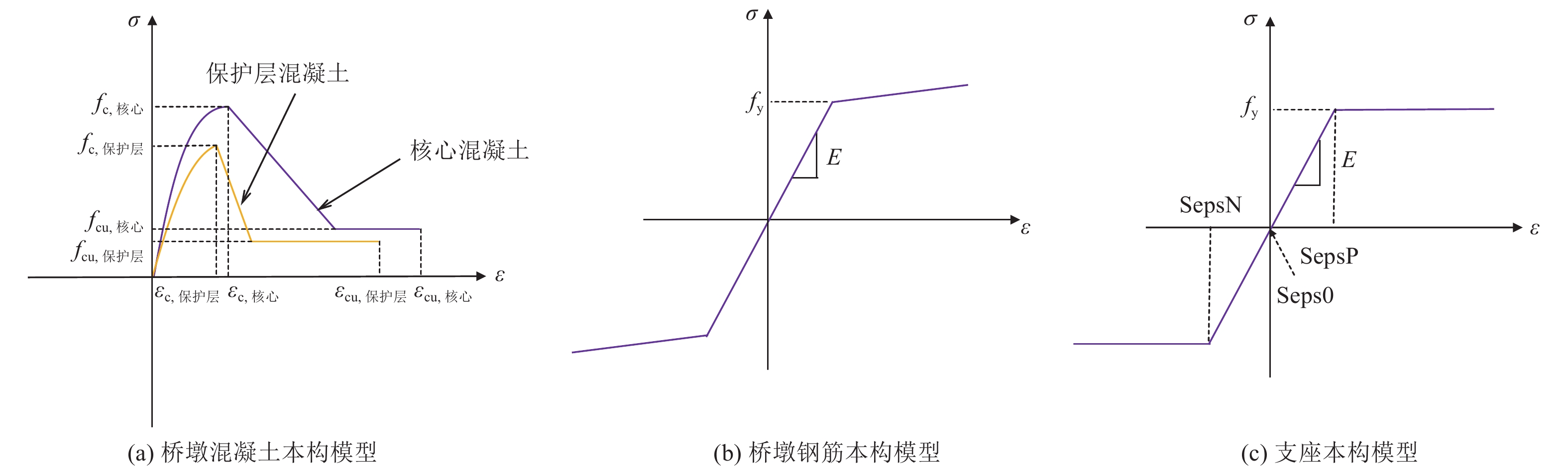
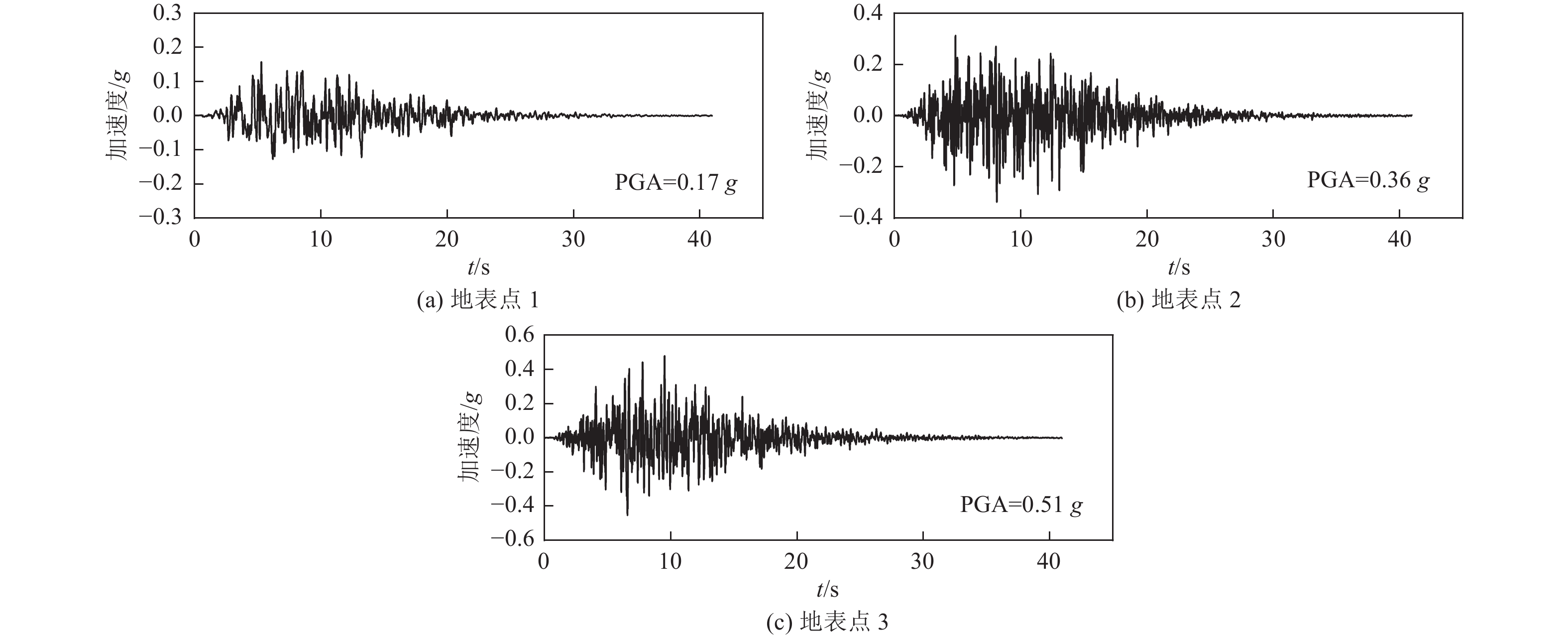
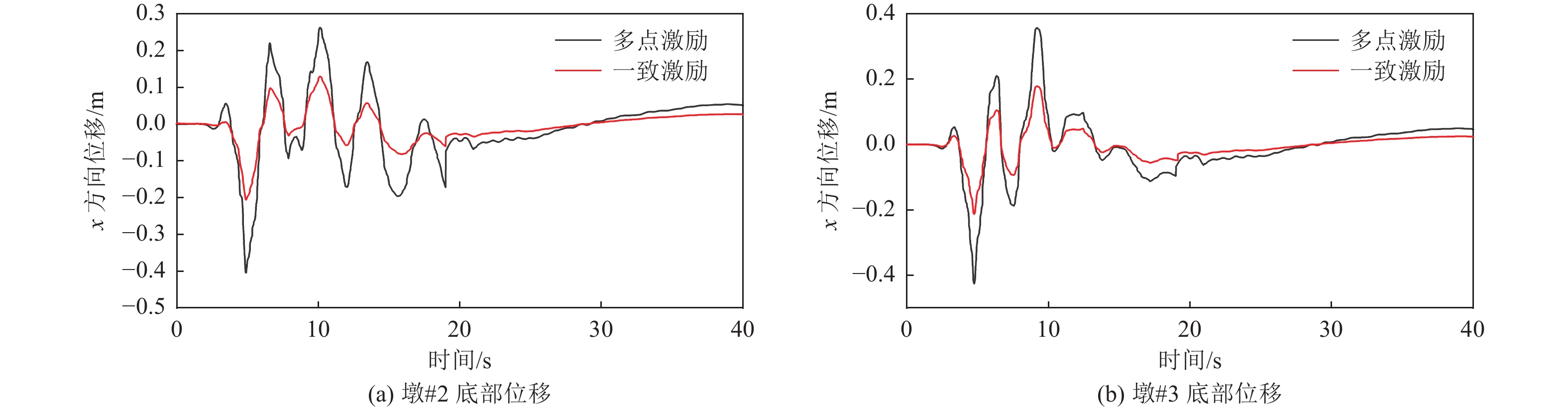
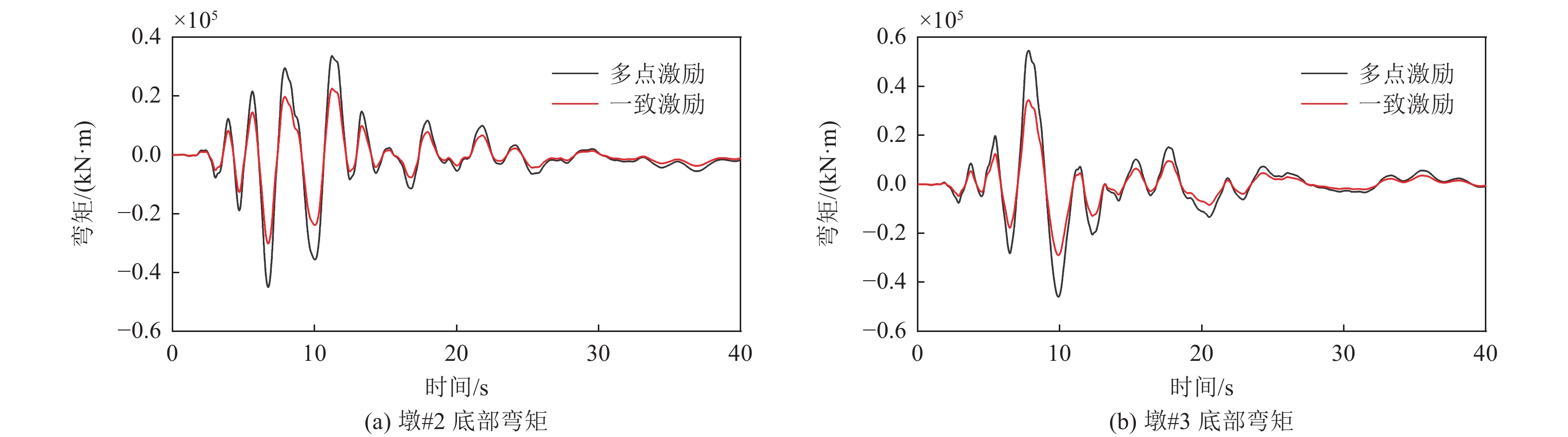
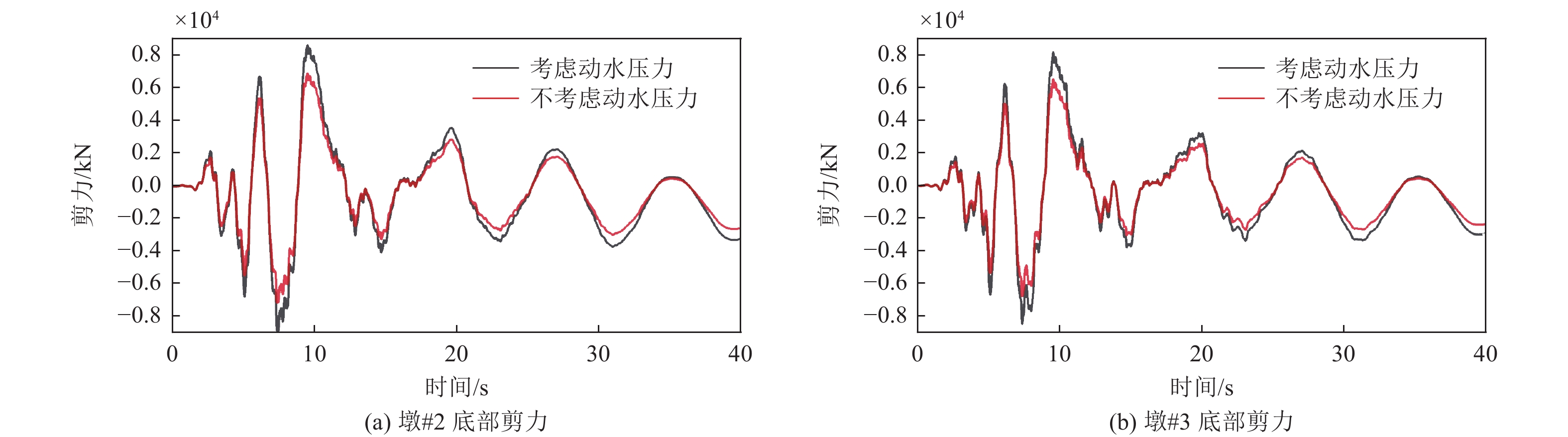
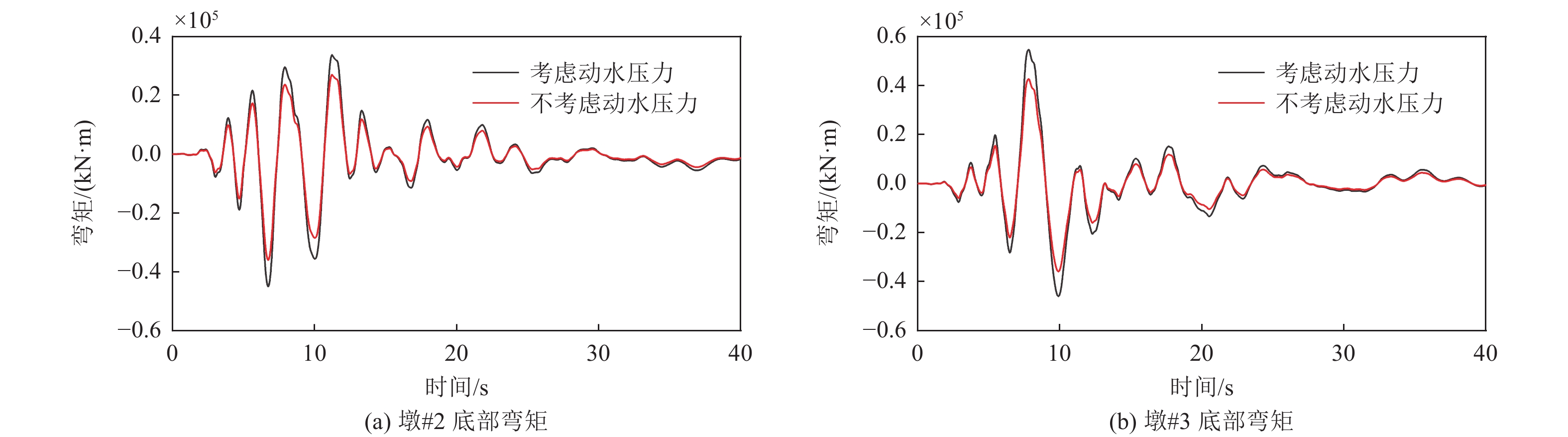
摘要: 我国沿海地区大量大跨桥梁位于饱和复杂场地,此类场地通常存在土饱和特性、起伏地形、上覆水层,其对地震波的散射效应将导致地震动空间变化,然而目前缺乏针对此类场地条件的实测地震记录。针对此问题,本文建立了适用于饱和复杂场地大跨桥梁抗震分析的多点地震动模拟方法。首先,基于边界元法求解饱和复杂场地地震动传递函数;然后采用谱表示法生成空间变化人工地震动加速度时程;最后,以五跨连续梁桥为例,开展大跨桥梁地震响应分析,研究局部场地效应、动水压力效应对大跨连续梁桥地震响应的影响规律。结果表明,饱和复杂场地地震动加速度峰值表现出明显的空间变化特性,多点输入可导致饱和场地中地震动峰值加速度放大2~3倍,进而引起墩顶位移较一致地震作用结果增大95%~110%;动水压力可引起墩顶位移、墩底内力增大34%、29%。饱和复杂场地大跨桥梁抗震分析时忽略场地条件对地震动、桥梁地震响应的影响偏于不安全。Abstract: In China, many long-span bridges are situated across saturated complex sites characterized by soil saturation, undulating topography and superimposed water, in which the propagation of seismic waves induces spatial variations in ground motions due to scattering effect. However, the seismic records under such specific site are lack. Addressing this concern, we establish a multi-point ground motion simulation approach to analyze the seismic response of long-span bridges in saturated sites. Initially, the boundary element method is employed to solve the ground motion transfer function of saturated sites. Subsequently, the spectral representation technique is used to synthesize spatially varying ground motion acceleration time-histories. Conclusively, a continuous five-span beam bridge is set as an example, and the seismic response is investigated to study the impact of local site effects and hydrodynamic pressures on the seismic behavior of the continuous beam bridge. The results indicate that the peak ground acceleration in the saturated regions has significantly spatial variances, and multipoint input can cause the peak acceleration of seismic motion in a saturated site to be amplified by 2~3 times. Consequently, situation leads to a 95%~110% increase in the displacement of the pier top compared to the results under uniform ground motions. Furthermore, dynamic hydrodynamic pressure is shown to precipitate pier displacement and internal forces by 34% and 29%, respectively. Disregarding the influence of saturated site conditions when evaluating ground motions and bridge responses for long-span bridges in saturated complex sites tends to engender hazardous outcomes.
-
引言
蔚广盆地南缘断裂带位于山西地堑系北端的京西北盆岭构造区内,是蔚县-广灵半地堑盆地(简称蔚广盆地)南边界断裂带,是一条由多条断层组成的具有正断性质的活动断裂带。前人对该断裂带的分段性(徐锡伟等,2002)、新构造运动特征(周廷儒等,1991;王乃樑等,1996;李树德,1997)、断层几何结构特征(王林等,2011;Wang等,2013)等都有过一定的研究。已有的研究大都集中于盆地的主边界断裂,对于盆内山前地区冲洪积扇体上发育的较新活动断层的研究相对有限,仅对断裂带东段局部有过一定研究(程绍平等,1998),然而这些较新的山前活动断层正是近期或将来孕育、发生地震可能性较高的断层,因此加强对这些山前断层的研究对于首都圈区域地震危险性的分析和判断具有一定的科学意义。
在“我国地震重点监视防御区活动断层地震危险性评价项目”之“首都圈地区蔚县-广灵断裂条带状地质填图”课题的支持下,本文通过高分辨率遥感影像解译、野外地质地貌调查以及开挖探槽等手段,对蔚广盆地南缘断裂带唐山口段山前断层的活动性进行了研究,得到了该段断层古地震期次、断层活动速率等方面的一些参数以及一些新的认识。
1. 地质构造概况
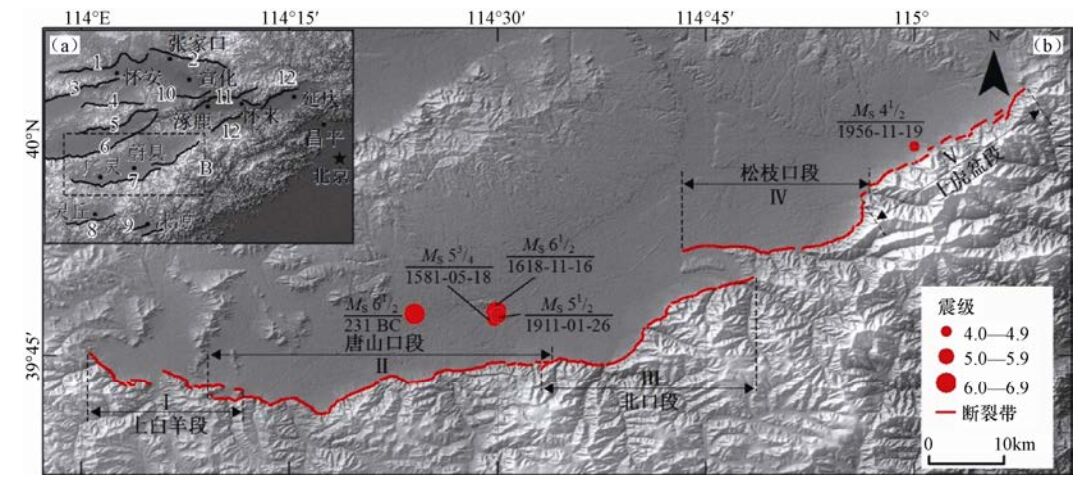
京西北盆岭构造区中发育有怀涿、蔚广、延矾、阳原、灵丘、怀安和涞源等一系列断陷盆地单元(图 1(a)),这些断陷盆地多为半地堑盆地,盆地的主控断裂一般总体走向为NEE,个别断裂走向近EW。该区内的蔚广盆地就是一个受南部边界正断层控制的、南深北浅的不对称半地堑盆地,蔚广盆地南缘断裂带即为控制该盆地南边界的正断层系(图 1(b))。蔚广盆地在新生代N2晚期至Q1早期之间开始裂陷并发育(周廷儒等,1991;徐锡伟等,2002),其南缘断裂带经历了长期的构造演化历程,上升盘不断地抬升形成山体区,下降盘不断地沉降和接受沉积而形成盆地区。自从有历史记载以来,盆地内已经发生过5次地震,主要包括公元前231年MS 6级地震,1581年MS 5级地震,1618年MS 6级地震,1911年MS 5级地震以及1956年MS 4级地震等。
 图 1 蔚广盆地的构造位置(a)及盆地内断裂的几何展布与分段特征(b)Figure 1. The tectonic location of the Yuguang basin (a) and the spatial distribution and segmentation of the main faults in the basin (b)图(a)中的断层名称:1怀安-万全盆地北缘断裂;2张家口断裂;3天镇-阳高盆地北缘断裂;4怀安镇盆地南断裂;5阳原盆地北缘断裂;6六棱山北麓断裂;7蔚广盆地南缘断裂;8太白维山北麓断裂;9涞源盆地南缘断裂;10宣化盆地南缘断裂;11怀涿盆地北缘断裂;12延矾盆地北缘断裂
图 1 蔚广盆地的构造位置(a)及盆地内断裂的几何展布与分段特征(b)Figure 1. The tectonic location of the Yuguang basin (a) and the spatial distribution and segmentation of the main faults in the basin (b)图(a)中的断层名称:1怀安-万全盆地北缘断裂;2张家口断裂;3天镇-阳高盆地北缘断裂;4怀安镇盆地南断裂;5阳原盆地北缘断裂;6六棱山北麓断裂;7蔚广盆地南缘断裂;8太白维山北麓断裂;9涞源盆地南缘断裂;10宣化盆地南缘断裂;11怀涿盆地北缘断裂;12延矾盆地北缘断裂该断裂带先是继承和利用了燕山运动中、晚期形成的北东向压型结构面,在此基础之上才进一步发展成为张性断裂体系,经历了一个构造反转的过程(周廷儒等,1991;王乃樑等,1996;李树德,1997)。根据次级断层空间展布的几何结构和长期断裂作用造成的断错地貌差异,蔚广盆地南缘断裂带可以划分为5个地震破裂段落,自西向东依次为上白羊段、唐山口段、北口段、松枝口段以及上虎盆段(徐锡伟等,2002),如图 1(b)所示。蔚广盆地南缘断裂晚更新世以来的活动部位主要集中在松枝口、北口、唐山口等3个段落上,它们是控制蔚广盆地第四纪持续活动的主边界断裂段,与盆地的沉降中心部位相对应。而东、西两端的上虎盆段、上白羊段晚更新世以来的活动性已经十分微弱。
2. 山前断层的地貌特征
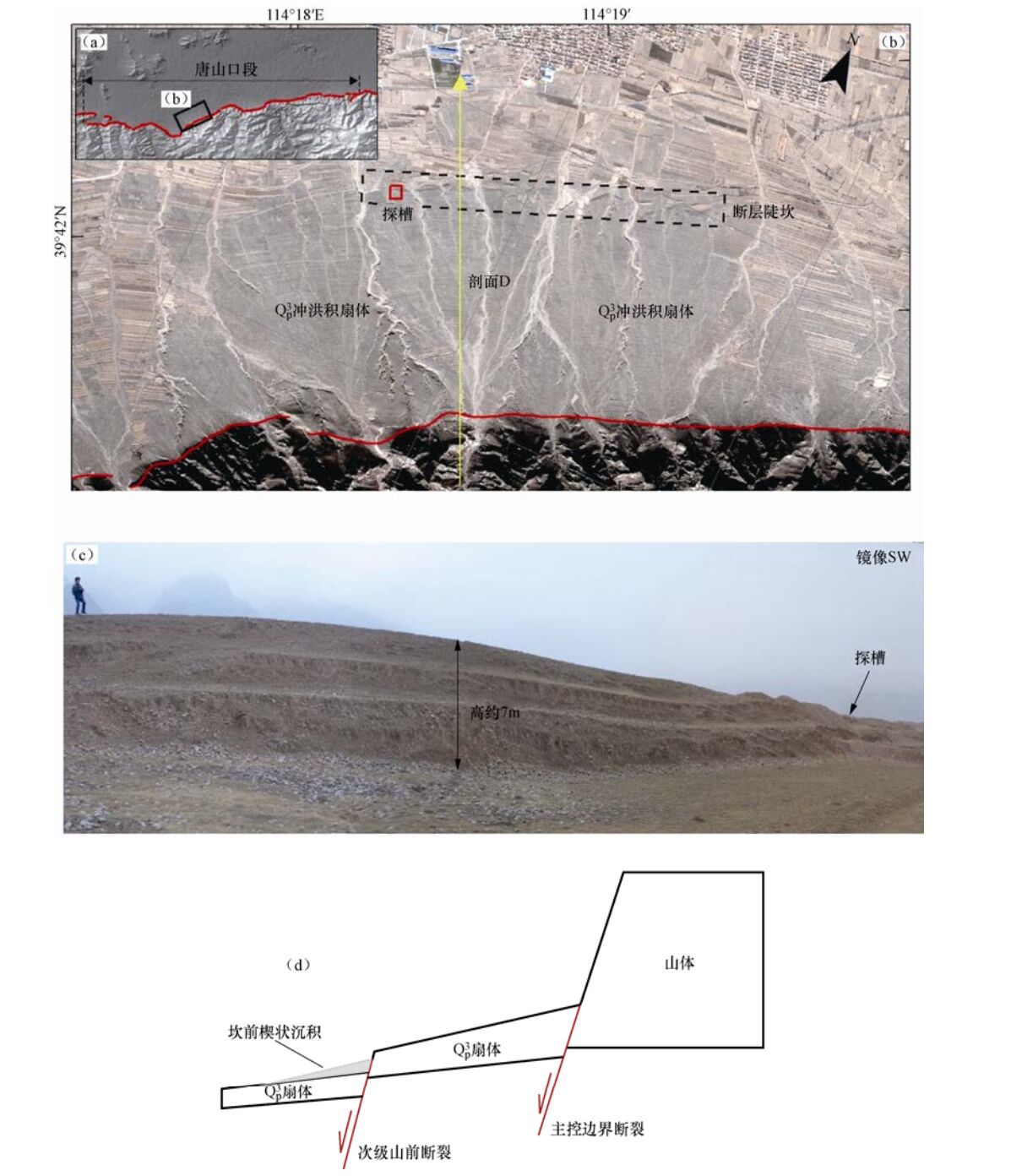
唐山口段的山前断裂在高分辨率遥感影像上呈明显的线性特征,在地表形成了断层陡坎。陡坎高约7m(图 2(c)),沿着陡坎的走向,由于沉积、侵蚀环境的差异造成了陡坎断续分布的形态(图 2(b))。有的地方存在河流冲沟,侵蚀性较强,陡坎形态侵蚀破坏较为严重,与此同时在陡坎前缘还形成了较新的小型扇体;有的地方发育干沟,侵蚀程度相对较弱,陡坎形态遭到部分破坏;有的地方则未发育任何冲沟,陡坎形态相对完整。
图 2(d)为横跨盆地边界断裂和扇体中山前断裂的剖面结构示意图,反映出了总体剖面结构。其中较新的次级山前断裂将Qp3扇体的扇面明显错断,在地表形成线性良好的断层陡坎,并在陡坎前缘形成了楔状沉积。
3. 探槽分析
在野外工作中,横跨断层陡坎开挖的探槽进一步揭示并确定了断层的存在,并在多套地层采集了OSL样品、14C样品。在室内工作中,首先对探槽壁的照片进行校正、拼接,制作了探槽照片拼接图,在此基础之上,对地层进行了精确的解译和矢量化,进一步得到了探槽地层剖面图,并以相应地层的颜色以及砾石砾径、分选性、磨圆性等特征制定了具体形象的填充符号,对不同的地层加以形象的显示。以探槽照片拼接图、探槽地层剖面图为基础,结合OSL样品、14C样品的年龄测试结果,对断层的古地震事件期次、断层活动速率等方面进行了详细的研究。
3.1 地层特征
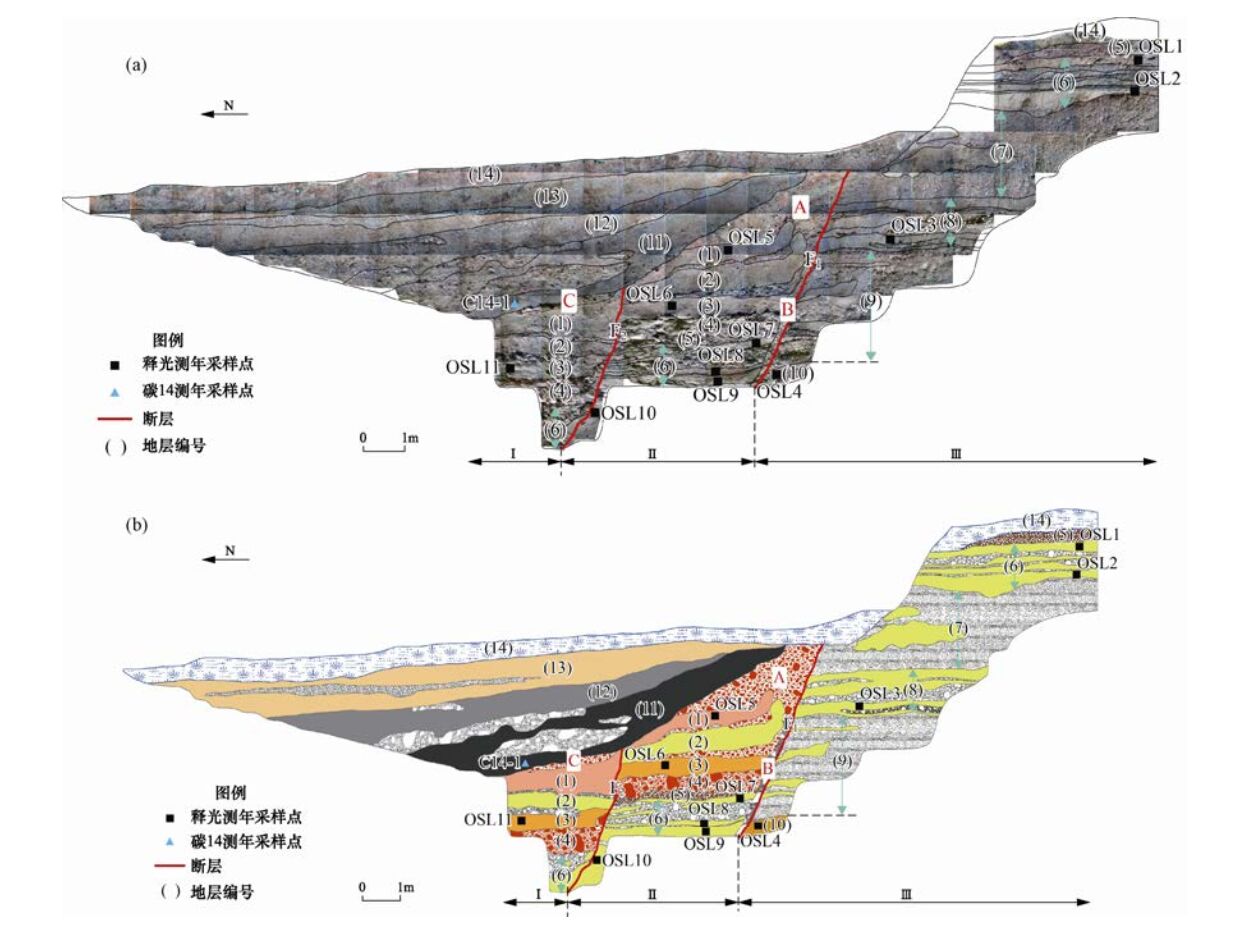
探槽照片拼接图、探槽地层剖面图如图 3(a)和3(b)所示,OSL样品、14C样品的测试结果分别见表 1、表 21。通过探槽照片拼接图以及探槽地层剖面图,初步判定存在2条断层F1、F2,将地层整体上切割成了3个断块Ⅰ、Ⅱ、Ⅲ。
表 1 探槽OSL样品测年结果表Table 1. Dating results of the OSL samples编号 α粒子计数率/ksec K2O/% 含水量/% 饱和含水系数 环境剂量率/Gy·ka-1 等效剂量/Gy 年龄/ka OSL1 10.71±0.22 2.12 5.00 0.06 4.40±0.34 78.52±2.63 17.89±1.55 OSL2 10.92±0.23 2.12 5.00 0.05 4.43±0.42 69.05±1.87 15.57±1.32 OSL3 12.17±0.24 2.28 15.02 0.64 4.36±0.13 97.95±4.76 22.45±2.10 OSL4 10.89±0.23 2.32 15.98 0.75 4.01±0.37 113.50±5.30 28.28±2.62 OSL5 9.67±0.22 2.10 15.85 0.52 3.79±0.35 25.38±1.12 6.69±0.63 OSL6 10.16±0.22 2.32 7.98 0.33 4.32±0.41 39.76±1.22 9.20±0.79 OSL7 10.24±0.22 2.28 8.82 0.38 4.27±0.37 75.91±2.00 17.78±1.50 OSL8 12.17±0.24 2.06 5.97 0.24 4.59±0.44 78.43±1.86 17.07±1.42 OSL9 10.63±0.22 2.00 5.96 0.27 4.20±0.40 71.91±1.98 17.12±1.45 OSL10 11.38±0.24 2.08 5.17 0.22 4.46±0.41 77.68±2.66 17.43±1.52 OSL11 11.17±0.21 2.24 8.47 0.36 4.45±0.40 38.94±1.05 8.75±0.74 表 2 探槽14C样品测年结果表Table 2. Dating results of the 14C sample编号 实验室编号 野外编号 距今年代/a BP C14-1 CG-7182 YX1011C14-1 7850±140 1 OSL样品由中国地震局地壳应力研究所光释光实验室测试完成;14C样品由中国地震局地质研究所地震动力学国家重点实验室测试完成。
探槽内各层的特征简述如下:
层(1):浅红色粉砂质粘土。该层OSL5样品年龄值为(6.69±0.63)ka。
层(2):黄色粉砂质粘土。
层(3):黄褐色粉砂质粘土。该层位于断块Ⅰ、Ⅱ中的样品OSL6、11的年龄值分别为(9.20±0.79)ka、(8.75±0.74)ka,可以看到两者相差不大,取两者的均值约9.0ka作为该层的年龄值。
层(4):红色粗砾砾石层,砾径5—20cm,分选度差,磨圆度差。
层(5):红色中砾砾石层,砾径1—5cm,分选度中等,磨圆度中等,基质填充,基质呈暗褐色。
层(6):黄色粉砂质粘土层与中砾砾石层互层。其中砾石层砾径1—5cm,分选度中等,磨圆度中等。该层位于断块Ⅱ中的样品OSL7、8、9、10的年龄值分别为(17.78±1.50)ka、(17.07±1.42)ka、(17.12±1.45)ka、(17.43±1.52)ka;位于断块Ⅲ中的样品OSL1、2的年龄值分别为(17.89±1.55)ka、(15.57±1.32)ka,可以看到大部分样品的年龄值都在17ka左右,取所有这些样品的平均值约17.1ka作为该层的年龄值。
层(7):中砾-粗砾砾石层,中砾、粗砾呈双向粒序,分选度好,磨圆度中等,局部夹杂黄色粉砂质粘土层透镜体。
层(8):黄色粉砂质粘土层夹中砾-粗砾砾石层。其中中砾砾径1—5cm,分选度中等,磨圆度中等;粗砾砾径10—15cm,分选度差,磨圆度差。该层位于断块Ⅲ中的样品OSL3的年龄值为(22.45±2.10)ka。
层(9):中砾-粗砾砾石层,中砾、粗砾呈双向粒序,分选度好,磨圆度中等,局部夹杂黄色粉砂质粘土薄层或透镜体。
层(10):黄褐色粉砂质粘土。该层位于断块Ⅲ中的样品OSL4的年龄值为(28.28±2.62)ka。
层(11):含14C的黑色腐殖质粘土层,局部夹砾石透镜体。其中砾石透镜体为中砾-粗砾混杂堆积,分选度差,磨圆度差。该层C14-1样品年龄值为(7.85±0.14)ka。
层(12):灰黑色粉砂质粘土,少量的细砾、中砾零星均匀地分布其中,局部夹砾石透镜体。其中砾石透镜体为中砾-粗砾混杂堆积,分选度差,磨圆度差。
层(13):淡黄色粉砂质粘土,少量的细砾、中砾零星均匀地分布其中,局部夹砾石层。其中砾石层为中砾,砾径1—5cm,分选度中等,磨圆度中等。
层(14):地表耕植土。
3.2 构造现象
在探槽中出露并发现了构造张裂楔、崩积楔、堆积楔等现象,以及地层断错、拖曳、韵律变化等现象(图 3(a)、(b)),下面对这些现象分别进行简要介绍:
① 剖面中的楔体A兼具构造楔和崩积楔的特征。一方面,它沿着F1的倾向向下方尖灭,表现出构造楔的特征,这应该是断层活动过程中断面张性开裂及后续的填充形成的;另一方面,它沿着水平方向在层(1)与层(11)之间尖灭,表现出崩积楔的特征,这应该是断层活动过程中以及后来一段时间内的快速崩积作用形成的。
楔体A由红色的中砾-粗砾混杂堆积而成,砾径1—15cm,分选度差,磨圆度差,局部夹黄色粉砂质粘土小块。楔体A的年龄介于下面的层(1)与上面的层(11)的年龄之间。
② 断层破碎带B的宽度可以达到0.3m左右,反映了F1的多次活动,破碎带内存在明显的砾石长轴定向排列的现象(图 4中黑色虚线段所示),两侧的各套近水平地层到破碎带之处都戛然而止。
③ 剖面中存在地层的倾斜、不整合现象。除了楔体A之外,断块Ⅰ、Ⅱ、Ⅲ中各套地层基本上都近于水平分布,而上覆于断块Ⅰ、Ⅱ的层(11)—(13)则均向南倾,其中层(11)南倾约20°并不整合于断块Ⅱ中层(1)的左半部层位之上,层(12)、(13)倾角依次减小,层(13)已近水平。
④ 剖面中位于F1的下降盘存在着楔状堆积体,由层(11)—(13)组成,分别由黑色、灰黑色、淡黄色的粉砂质粘土构成,它们覆于断块Ⅰ、Ⅱ之上,其中层(11)是富含14C的黑色腐殖质粘土层,这3套地层属于静水堆积,层位均向北尖灭,整体上呈现水平的楔状形态,属于堰塞塘堆积。
⑤ 剖面中显示了地层沉积韵律的变化。断块Ⅱ中层(4)以后(包括层(4))的堆积显示了由粗到细的两个沉积韵律的变化,分别对应着后面将要描述的2次最新的古地震事件。层(4)为粗砾,代表了一些近源物质较短时间内速度较快的混杂堆积,层(1)—(3)以粉砂质粘土为主,代表以较长时间内速度较慢的静水堆积,层(4)至层(1)—(3)完成了沉积由粗至细的韵律变化。类似地,楔体A为粗砾,代表了一些近源物质在较短时间内速度较快的混杂堆积,层(11)—(13)以粉砂质粘土为主,表示其以较长时间内速度较慢的静水堆积为主,层(4)至层(11)—(13)完成了沉积由粗至细的韵律变化。
此外,断块Ⅲ中层(10)以后(不包括层(10))的堆积也显示了2个沉积韵律的变化。层(9)与层(7)的组成与结构相近,均为呈双向粒序、分选性好、磨圆性中等的中砾-粗砾砾石层,而层(8)与层(6)的组成与结构相近,均为黄色粉砂质粘土层与砾石层互层,那么从层(9)到层(8),然后再从层(7)到层(6)则为2个相邻的相似的沉积韵律的变化,根据层(10)、层(8)与层(6)内的样品年龄值,可以估计出沉积韵律的变化周期约为6ka,这种沉积韵律的变化可能更多地代表一种气候的变化和旋回。
⑥ 剖面中断块Ⅰ中层(3)、层(4)与层(6)右端沿F2发生拖曳,断块Ⅱ中层(1)与层(2)右端沿F1发生拖曳。
3.3 古地震事件分析
确认探槽内存在的最早一次古地震事件E3的主要依据有2点:① 层(6)与层(3)之间较长时间的沉积间断;② 层(4)中分选性差、磨圆性差的红色粗砾砾石层。层(6)OSL样品均值约为17.1ka,层(3)OSL样品均值约为9.0ka,两者之间时间间隔约8.1ka,这么长的时间间隔却只存在快速堆积的一套厚约0.5m的层(4),而前述层(9)到层(8)、层(7)到层(6)的2个沉积韵律变化的周期均约为6ka,对应的沉积层厚度却分别达到了3.9m、3.3m左右,相比之下可以推断层(6)与层(3)之间存在较长时间的沉积间断。层(6)形成之后可能处于局部相对抬升的状态,沉积作用停止,在其表面逐渐形成了层(5)这一套类似风化层的物质,之后在某一时刻F1发生第一次活动,即E3发生,使得近源物质在较短时间内大量快速堆积形成了一套分选性、磨圆性均较差的层(4)。层(4)虽然在形态上不如楔体A那么显著和典型,但是从物质成分、结构、成因等方面来看却与楔体A有着同样的性质,即都是断层活动造成的近源快速堆积体。根据层(6)与层(3)的年龄,E3约发生在距今17.3—9ka,但应更接近于层(3)的年龄,即9ka左右。
探槽内的第二次古地震事件E2主要以楔体A以及次级断层F2的存在为代表,其中楔体A在E2之后形成,是F1活动的结果,而F2则可能是E2发生时与F1同时活动的结果。楔体A下面存在沉积层(1),上面覆盖有层(11),E2应该发生在层(1)的地层年代之后以及层(11)的地层年代之前,而层(1)中OSL5年龄值为(6.69±0.63)ka,层(11)中C14-1样品年龄值为(7.85±0.14)ka,两者虽然出现反序,但是差异不大,取两者平均值约7.3ka作为E2距今的大致年代。F2活动的最直接的体现就是楔体C的存在以及F2两侧被错断的一系列地层,即层(1)—(6),并使得断块Ⅰ中的层(3)、层(4)与层(6)右端沿F2发生拖曳现象,其中层(5)在断块Ⅱ中存在,而在断块Ⅰ中由于F2活动时的构造扰动而卷入层(6)上半部的粉砂质粘土薄层、砾石层之中,三者混杂在一起难以区分,因此未将层(5)表示出来,笼统地将这三者归入层(6)之中。F2发生活动之后其断块Ⅰ下降,间接造成了后来的层(11)形成过程中对陡坎之处出露的新鲜的层(1)的左上部分的冲刷、侵蚀,最终造成了层(1)左上角的缺失,使得层(11)与层(1)在此处不整合接触。F2断错了层(1)而被层(11)覆盖,因此F2应该发生在层(1)的地层年代之后以及层(11)的地层年代之前,这点与楔体A是相似的,即约为距今7.3ka左右。
从崩积楔A进一步被断错的形态来看,后来应该还存在最新的一次古地震事件E1,它仍是F1再次活动的结果,这次事件使得断块Ⅱ中之前形成的沉积层(1)、层(2)右端最终形成了较为严重的拖曳,并使得崩积楔A的物质进一步崩落并夹杂于层(1)与层(2)以及层(2)与层(3)之间。然而,由于该剖面上部可能有人为扰动,导致这次活动的坎前堆积被破坏,而无法识别出被断错的最新地层,难以对事件E1进行准确定年。
如果将E1、E2、E3近似地视为在同一断层重复发生的一组特征地震,那么它们的震级应该是相近的,同震位移量也是相近的。根据被断错的层(6)可以得到F1的累积垂直位移量约为6.9m,根据被断错的层(1)、层(2)、层(3)可以得到F2的累积垂直位移量约为1.2m,两者之和8.1m即为E1、E2、E3这3次事件形成的总的累积位移量,约为8.1m,由此可以估算出整条山前断裂各次事件的平均同震位移量约为2.7m,而根据E3、E2的发生时间可以估算出整条山前断裂的平均复发周期约为1.7ka,最后通过平均同震位移量与平均复发周期的比值可以估算出整条山前断裂的平均滑动速率约为1.6mm/a(表 3)。
表 3 蔚广盆地南缘断裂带唐山口段山前断层探槽全新世古地震事件Table 3. The Holocene paleoearthquake events of the piedmont fault along the Tangshankou fault segment古地震事件 发生时间 复发周期 累积位移量 平均同震位移量 平均滑动速率 E3 9ka 1.7ka 8.1m 2.7m 约1.6mm/a E2 7.3ka E1 - 邓起东等(1992)曾对华北地区正走滑断层进行统计,得到了震级(M)和单次同震位移量(D)的回归关系:
$$M = 7.56 + 0.69\lg D$$ (1) 将D=2.7m代入该上式估算出单次古地震震级约为7.9级。
董瑞树等(1993)通过综合回归得到了中国东部地区震级(M)和活动断层段长度(L)之间的关系:
$$M = 4.094 + 1.699\lg L$$ (2) 根据前述前人的分段结果,唐山口段的长度L约为34km,将其代入上式估算出该段断层的最大潜在地震震级约为6.7级。
综合上面2种地震震级的估算方法,取两者的平均水平7.3级作为古地震震级的估计值。
以上讨论的仅是同震(累积)垂直位移量。从前述探槽的地貌特征来看,横跨陡坎的各种冲沟、干沟并未表现出明显的左旋或右旋走滑特征,因此认为山前断层以垂直倾滑运动为主,并无走滑运动分量。
4. 结论与讨论
(1)蔚广盆地南缘断裂带唐山口段山前断裂属于全新世活动断裂。
(2)该断裂至少由2条断层——F1和F2组成。F1在全新世时期有过3次古地震事件,它在距今约9ka时首次活动(E3),之后在距今约7.3ka时再次活动并引发了F2的形成与同步活动(E2)。最后它们又发生了第三次活动(E1),然而由于人为破坏,导致这次活动相应的地表沉积缺失,无法识别出被断错的最新地层,难以确定E1发生的时间,因此最新一次古地震的离逝时间尚无法估计。
(3)3次古地震事件的同震累积垂直位移量约为8.1m,单次平均同震垂直位移量约为2.7m,整条山前断裂的平均复发周期约为1.7ka、平均滑动速率约为1.6mm/a,估算出古地震的参考震级约为7.3级。
-
-
何颖, 于琴, 刘中宪, 2019. 考虑散射效应沉积河谷空间相关多点地震动模拟. 岩土力学, 40(7): 2739—2747, 2788He Y. , Yu Q. , Liu Z. X. , 2019. Simulation of multi-point spatially correlated earthquake ground motions of sedimentary valleys considering scattering effect. Rock and Soil Mechanics, 40(7): 2739—2747, 2788. (in Chinese) 黄磊, 刘中宪, 张雪等, 2020. 含流体层的河谷场地对地震波散射的间接边界元法模拟. 地震学报, 42(6): 657—668Huang L. , Liu Z. X. , Zhang X. , et al. , 2020. IBEM simulation of seismic wave scattering by valley topography with fluid layer. Acta Seismologica Sinica, 42(6): 657—668. (in Chinese) 李丽, 赵国峰, 李吉等, 2020. 一致激励与多点激励对悬索桥地震响应影响分析. 震灾防御技术, 15(2): 252—259Li L. , Zhao G. F. , Li J. , et al. , 2020. Analysis of the influence of uniform excitation and multi-point excitation on the seismic response of suspension bridge. Technology for Earthquake Disaster Prevention, 15(2): 252—259. (in Chinese) 李平, 薄景山, 肖瑞杰等, 2018. 地震动河谷场地效应研究. 震灾防御技术, 13(2): 331—341Li P. , Bo J. S. , Xiao R. J. , et al. , 2018. The study of effect by the valley site on ground motion. Technology for Earthquake Disaster Prevention, 13(2): 331—341. (in Chinese) 李伟华, 赵成刚, 2008. 平面SV波在饱和土半空间中圆柱形孔洞周边的散射. 地震工程与工程振动, 28(6): 1—7Li W. H. , Zhao C. G. , 2008. An analytical solution for the scattering of plane SV-waves around cylindrical cavity in a fluid-saturated porous media half space. Journal of Earthquake Engineering and Engineering Vibration, 28(6): 1—7. (in Chinese) 李忠献, 李笑穹, 李宁, 2014. 空间相关多点多维地震动的模拟. 地震工程与工程振动, 34(4): 64—72Li Z. X. , Li X. Q. , Li N. , 2014. Simulation of multi-point and multi-dimension spatially correlated earthquake ground motions. Earthquake Engineering and Engineering Dynamics, 34(4): 64—72. (in Chinese) 梁建文, 吴孟桃, 巴振宁, 2021. 流体饱和半空间二维地形三分量弹性波散射间接边界元模拟. 地球物理学报, 64(8): 2766—2779Liang J. W. , Wu M. T. , Ba Z. N. , 2021. IBEM simulation of three-component scattering of elastic waves in a fluid-saturated half-space with 2 D topography. Chinese Journal of Geophysics, 64(8): 2766—2779. (in Chinese) 闫磊, 李青宁, 岳克锋等, 2019. 多维多点激励下考虑支座摩擦滑移及结构碰撞的非规则桥梁抗震性能研究. 世界地震工程, 35(2): 68—77Yan L. , Li Q. N. , Yue K. F. , et al. , 2019. Seismic behavior of irregular bridges under multi-dimensional and multi-point excitation considering friction slip of bearings and structural pounding. World Earthquake Engineering, 35(2): 68—77. (in Chinese) Bi K. M. , Hao H. , 2012. Modelling and simulation of spatially varying earthquake ground motions at sites with varying conditions. Probabilistic Engineering Mechanics, 29: 92—104. doi: 10.1016/j.probengmech.2011.09.002 Huang H. C. , Chiu H. C. , 1995. The effect of canyon topography on strong ground motion at Feitsui damsite: quantitative results. Earthquake Engineering & Structural Dynamics, 24(7): 977—990. Li X. Q. , Li Z. X. , Crewe A. J. , 2018. Nonlinear seismic analysis of a high-pier, long-span, continuous RC frame bridge under spatially variable ground motions. Soil Dynamics and Earthquake Engineering, 114: 298—312. doi: 10.1016/j.soildyn.2018.07.032 Liu Z. X. , Li W. X. , Jin L. G. , et al. , 2023 a. Efficient simulation of stochastic seismic response of long-span bridges in river valleys using hybrid BEM-FEM. Soil Dynamics and Earthquake Engineering, 165: 107690. doi: 10.1016/j.soildyn.2022.107690 Sánchez-Sesma F. J. , Campillo M. , 1991. Diffraction of P, SV, and Rayleigh waves by topographic features: a boundary integral formulation. Bulletin of the Seismological Society of America, 81(6): 2234—2253. Wang P. G. , Zhao M. , Du X. L. , 2019. Simplified formula for earthquake-induced hydrodynamic pressure on round-ended and rectangular cylinders surrounded by water. Journal of Engineering Mechanics, 145(2): 04018137. doi: 10.1061/(ASCE)EM.1943-7889.0001567 Wu Y. X. , Gao Y. F. , Zhang N. , et al. , 2016. Simulation of spatially varying ground motions in V-shaped symmetric canyons. Journal of Earthquake Engineering, 20(6): 992—1010. doi: 10.1080/13632469.2015.1010049 Zanardo G. , Hao H. , Modena C. , 2002. Seismic response of multi-span simply supported bridges to a spatially varying earthquake ground motion. Earthquake Engineering & Structural Dynamics, 31(6): 1325—1345. -




 下载:
下载:



 下载:
下载: