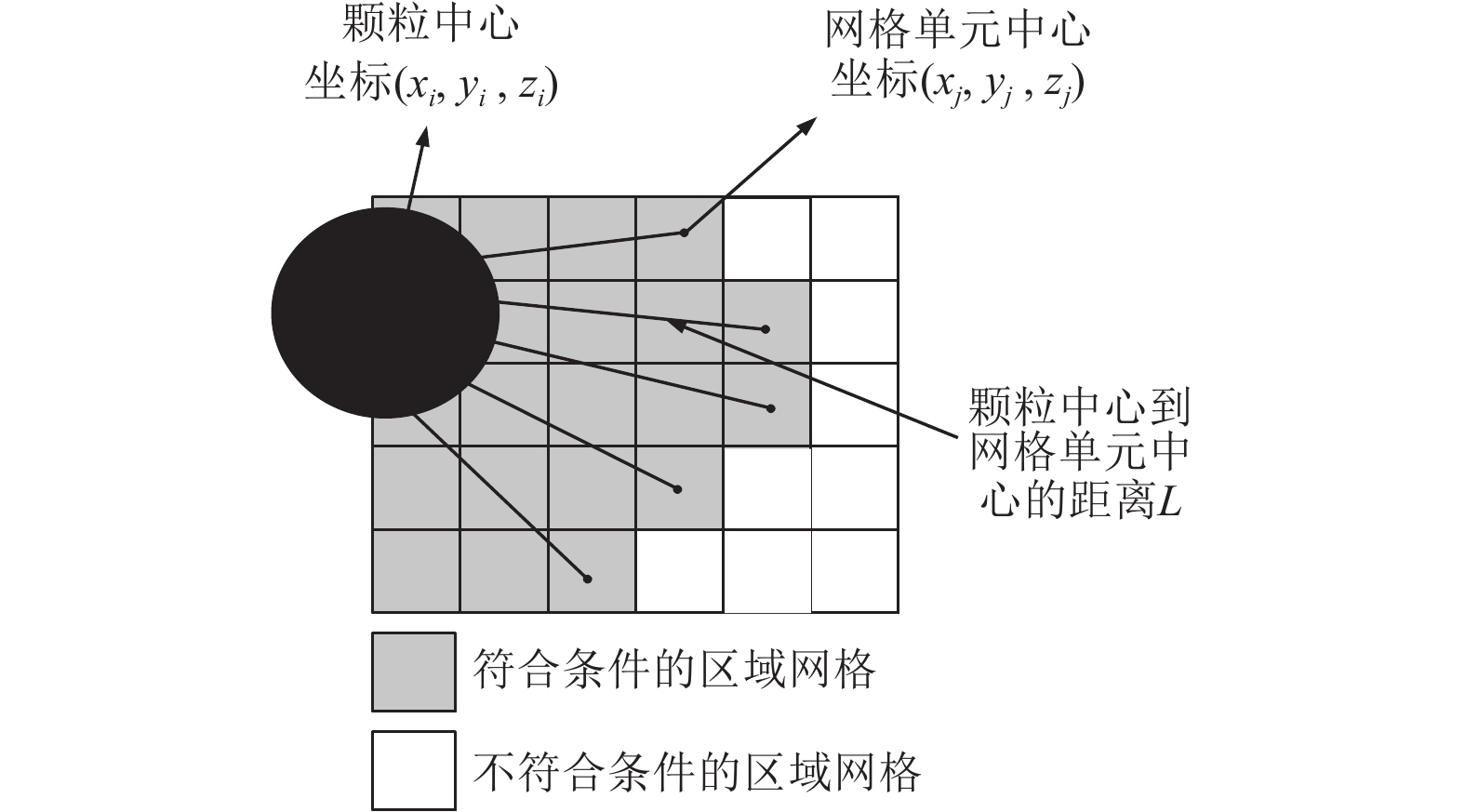
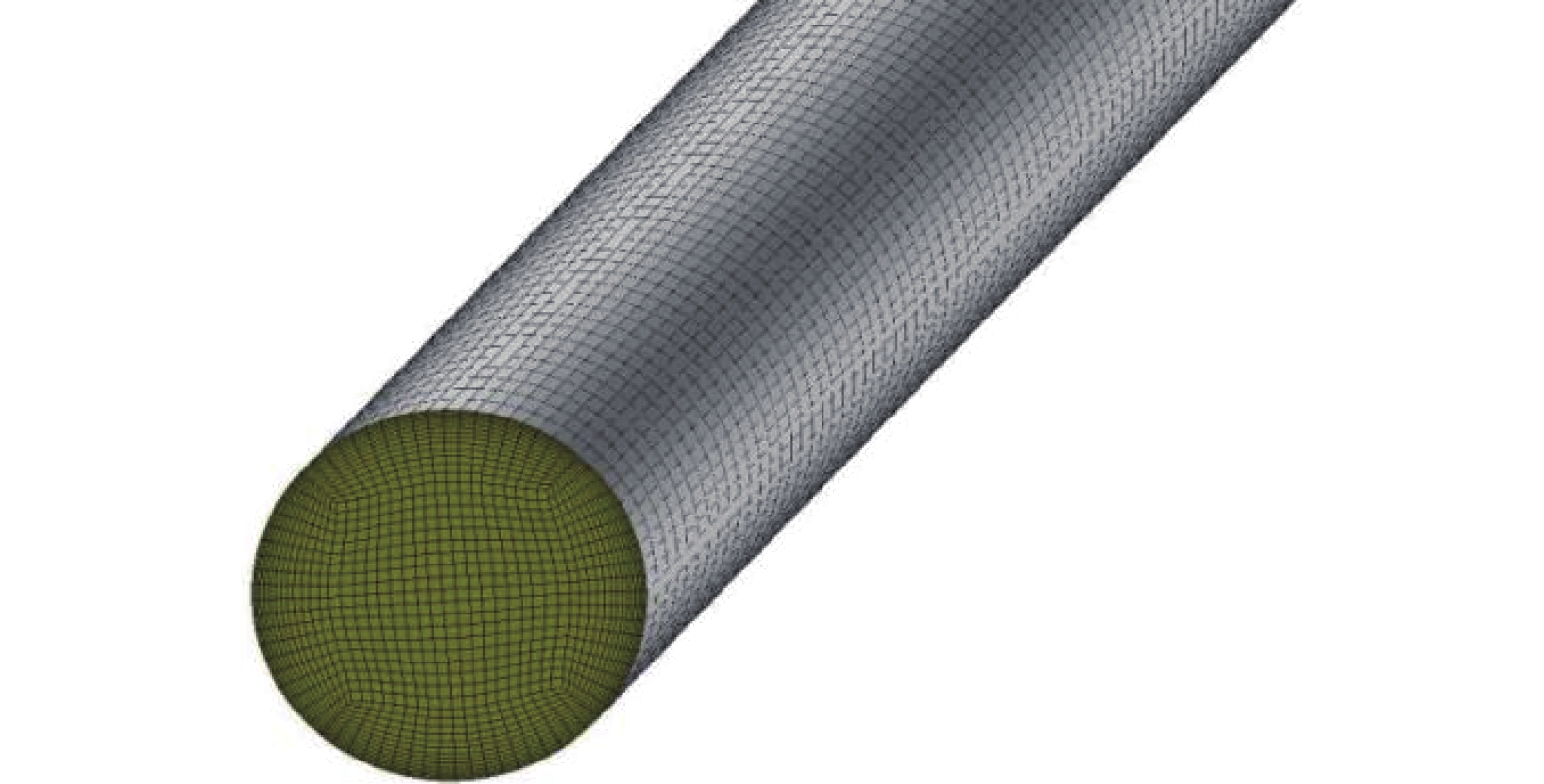
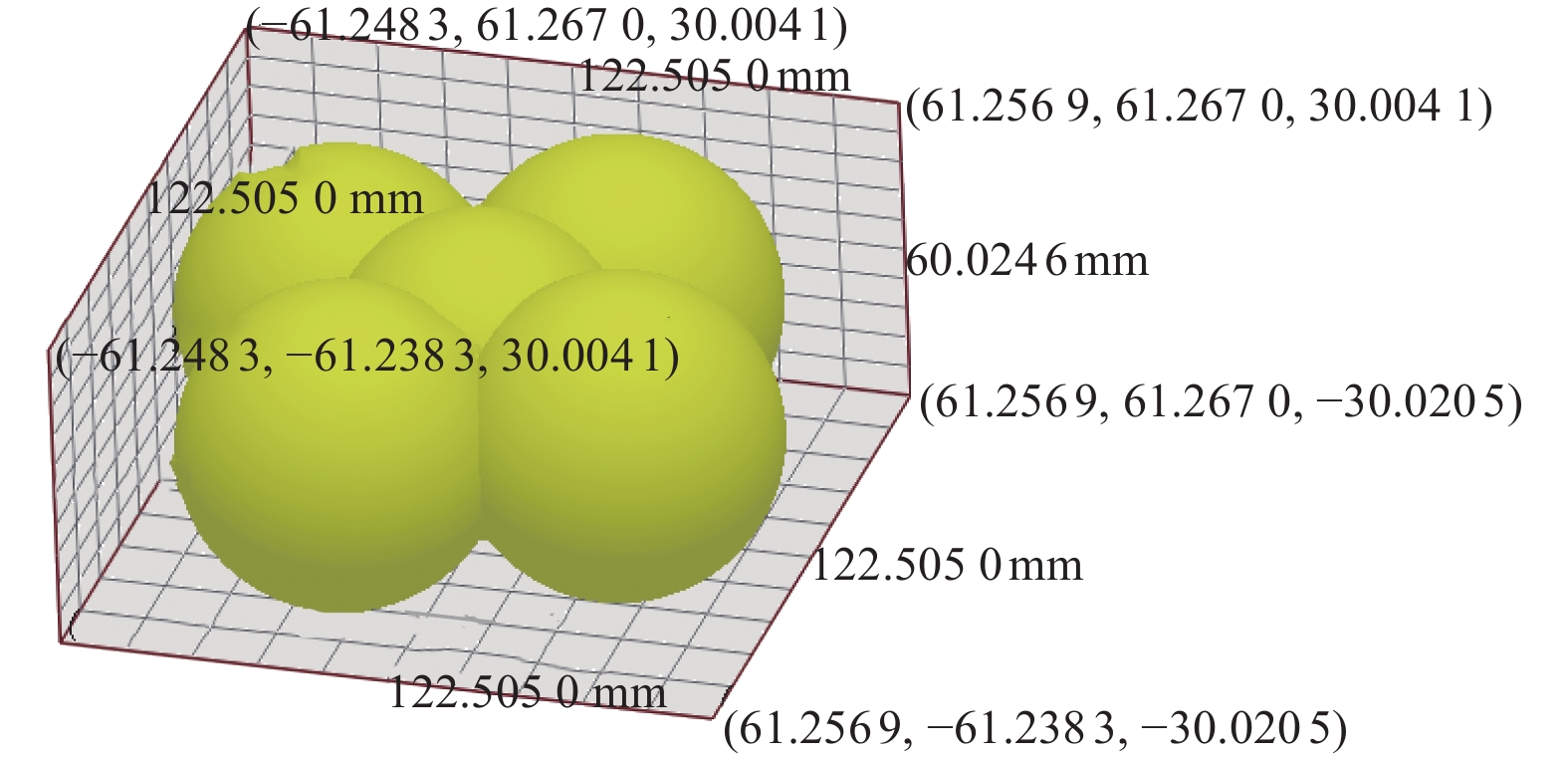
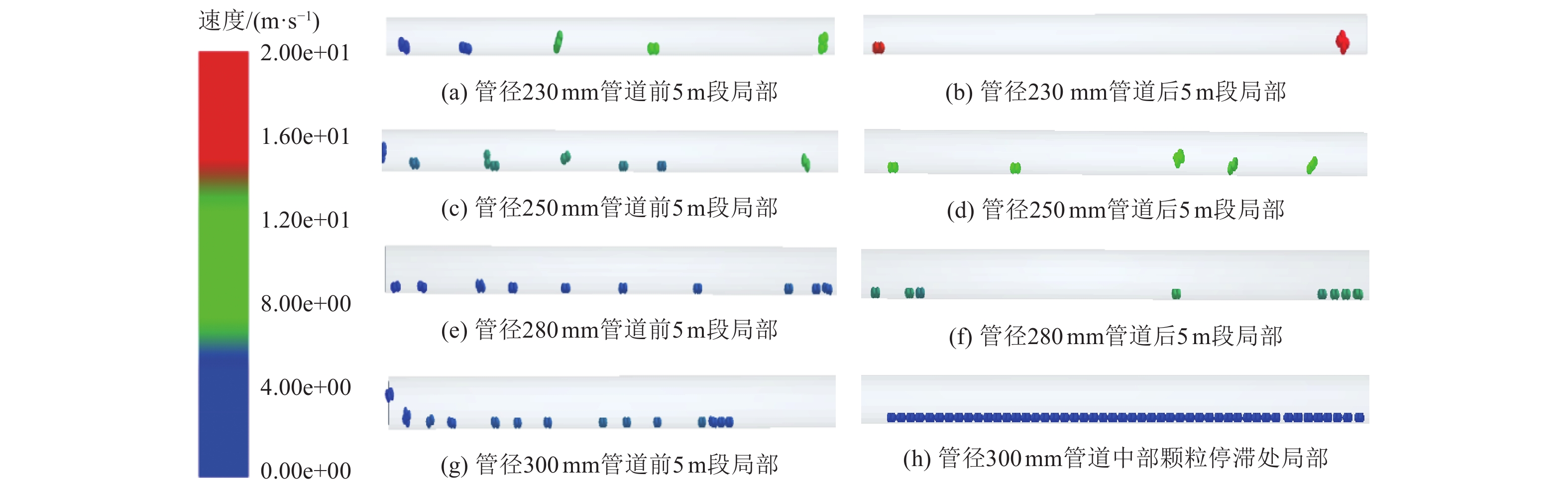
Numerical Simulation of Negative Pressure Transport of Extra Large Particles of Suction Earthquake Rescue Machine
-
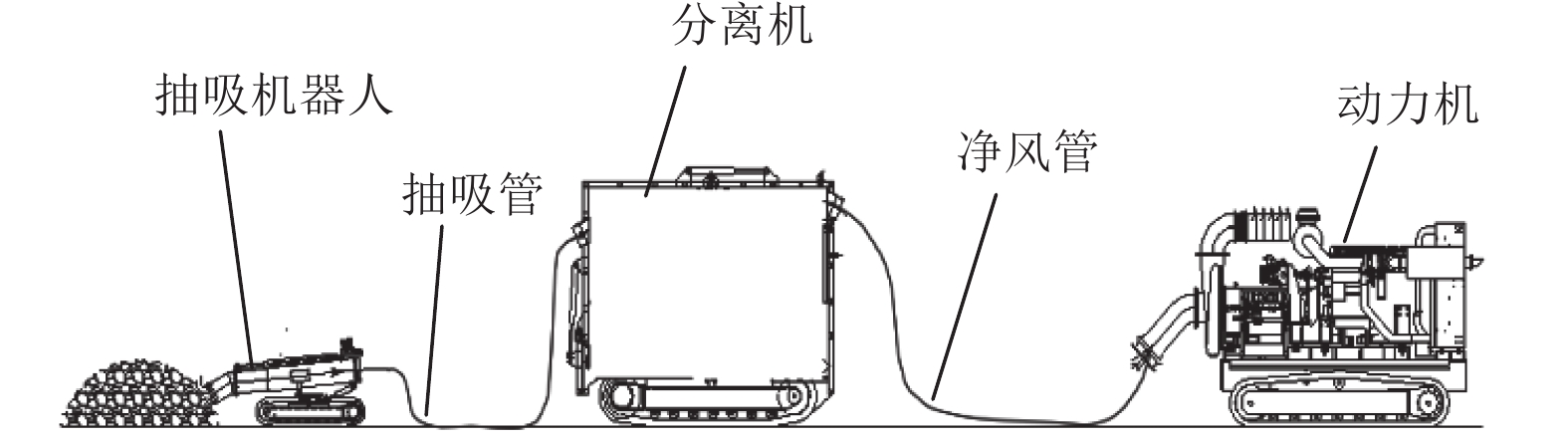
摘要: 针对某抽吸式地震救援机气力输送系统工作时存在的易堵塞和能耗高等问题,选用不同输送管径开展特大颗粒物料气固两相流数值模拟,研究风机以额定功率工作时特大颗粒在不同管道中的运动特性和管道内压损失。利用计算流体力学和离散单元法进行耦合求解,在传统耦合接口的基础上更改体积分数算法实现颗粒粒径大于流体域网格单元的气力输送数值仿真,利用软件Fluent进行连续相气体的流动分析和曳力计算,利用软件EDEM进行离散相颗粒的受力分析和运动轨迹计算。研究结果表明,管径为280 mm的管道输送性能最佳,管中颗粒运动速度最大为7.1 m/s,0~25 s内的运输量达66.4 kg,管道内压损失在5.5 s时达到稳定,为3.04 kPa,与试验结果基本吻合,可为抽吸式地震救援机气力输送系统设计和优化提供参考。Abstract: Aiming at the problems of easy blockage and high energy consumption in the pneumatic conveying system of a pneumatic suction seismic rescue machine, the gas-solid two-phase flow of large particles in different pipes with different diameters was numerically simulated to study the movement characteristics and pressure loss of large particles in different pipes when the fan works at rated power. Computational fluid mechanics and discrete element method were used to solve the coupling, and the volume fraction algorithm was changed on the basis of the traditional coupling interface to realize the numerical simulation of pneumatic conveying with the particle size larger than the fluid domain grid element. The flow analysis and drag calculation of continuous phase gas were carried out by using the software Fluent. The force analysis and motion trajectory calculation of discrete phase particles are carried out by using Edem software. The results show that the pipe with a diameter of 280 mm has the best transport performance, the particle movement velocity in the pipe is up to 7.1 m/s, the transport volume within 0~25 s is up to 66.4 kg, and the pressure loss in the pipe reaches a stable level of 3.04 kPa at 5.5 s, which is basically consistent with the test results. It can provide reference for the design and optimization of pneumatic conveying system of suction earthquake rescue aircraft.
-
引言
1976年唐山大地震在唐山与天津地区引发了范围广、灾害重的液化震害。地震发生后,铁道部科学研究院等单位于1977、1978年对液化场地进行了钻孔勘察及静力触探测试(CPT)(刘恢先,1985)。当时使用的是单桥静力触探(以下简称“单桥CPT”)测试,在数据指标方面存在缺陷,与国际标准不接轨。单桥静力触探仅能提供比贯入阻力ps,不同的土层可能有相同的ps值,土层划分分辨率极低(孟高头等,2000)。因此,中国地震局工程力学研究所联合California Polytechnic State University及东南大学于2007年对上述唐山地区部分测点进行了现代多功能孔压静力触探(CPTU)测试(邱毅,2008;Moss等,2011)。CPTU测试在数据指标方面进行了补充,可提供锥尖阻力qc、侧壁摩阻力fs、摩阻比Rf(侧壁摩阻力fs与锥尖阻力qc的比值)及孔压u。相比单桥CPT,多了3项指标,在土层划分时分辨率较高,且有较丰富的经验和成熟的方法(刘松玉等,2013;董林等,2017,2018)。
对于CPTU测试数据,Moss等(2011)和Boulanger等(2014)先后给出了唐山地震CPT液化数据库。但是,由于CPTU测试与单桥CPT相距30年,唐山市经历了恢复重建,且改革开放后城市建设日新月异,地下水位变化及测点重新定位误差等均会影响新CPTU数据的代表性。因此,需对2007年得到的CPTU测试数据能否代表地震时的液化点与非液化点进行判断。本文对唐山大地震液化数据库进行检验,通过对比单桥CPT与CPTU测试数据沿深度的变化趋势,结合标贯击数随深度的变化趋势,判明场地力学特性是否一致。利用Robertson土质分类图,进行新CPTU数据土类分层检验,对比单桥CPT测试时钻孔柱状图,检验土层土类是否一致。通过土类筛选,并结合单桥CPT测试时标贯击数与CPTU测试锥尖阻力,选定液化层。将经过检验的液化数据库带入我国《岩土工程勘察规范》(GB 50021—2001)(中华人民共和国建设部,2004)液化判别方法,检验新CPT数据库的可信度。
1. 方法介绍
1.1 Robertson土质分类图
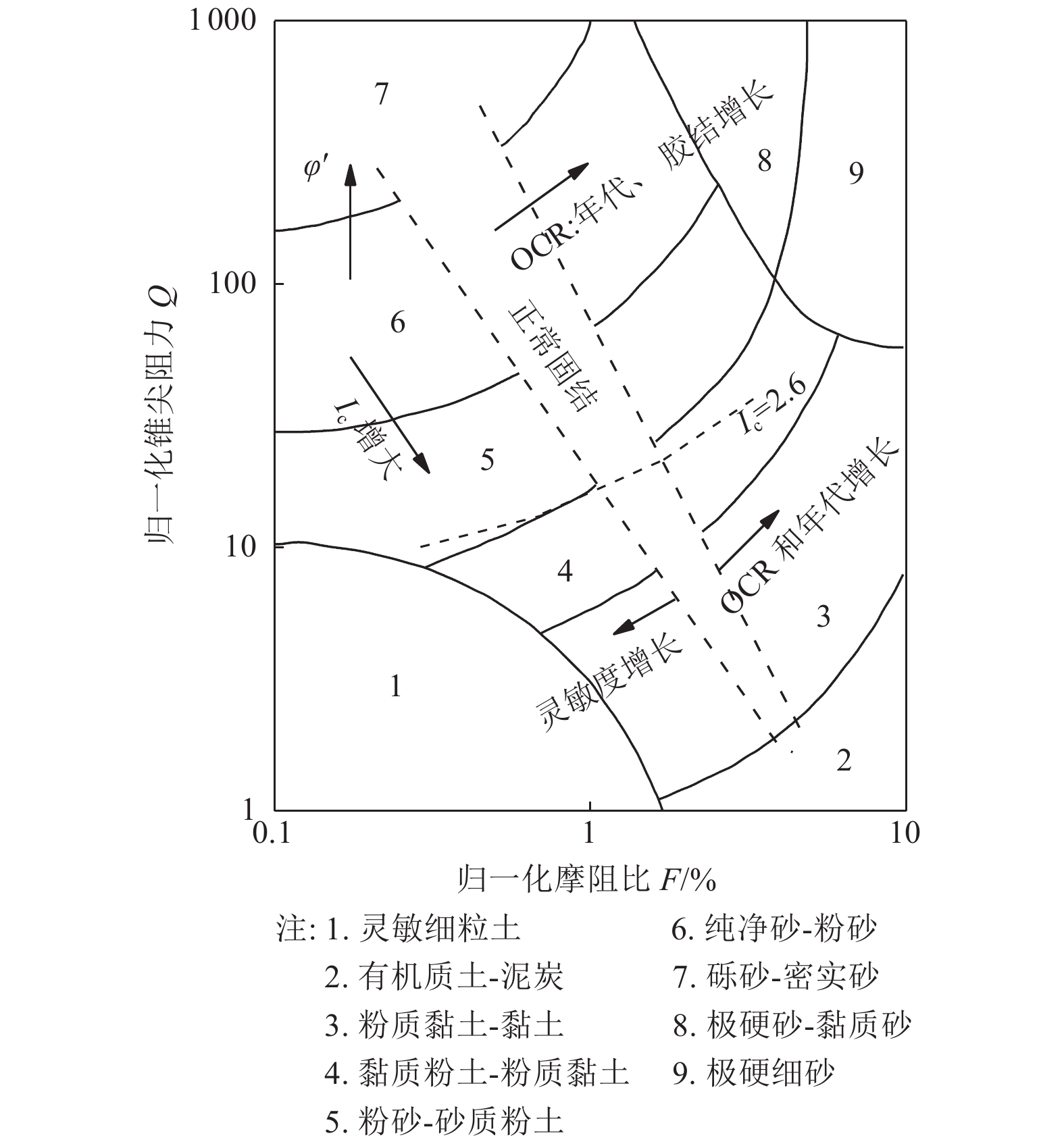
Robertson等(1998)基于大量现场实测数据与经验,建立了基于CPT指标的土质分类图(图1),根据摩阻比与土体细粒含量和塑性指数成正比的关系,构建了土类指数Ic,对于图1中土类2~7,Ic为5组同心圆的半径,按下式计算:
$$ {I_{\text{c}}} = {\left[ {{{\left( {3.47 - \log Q} \right)}^2} + {{\left( {1.22 + \log F} \right)}^2}} \right]^{0.5}} $$ (1) $$ Q = [\left( {{q_{\rm{c}}} - {\sigma _{{\rm{v}}}}} \right)/{P_{\rm{a}}}][{\left( {{P_{\rm{a}}}{\text{/}}\sigma _{{\rm{v}}}^{'}} \right)^n}] $$ (2) $$ F = \left[ {{f_{\rm{s}}}/\left( {{q_{\rm{c}}} - {\sigma _{{\rm{v}}}}} \right)} \right] \times 100\% $$ (3) 式中,Q为归一化锥尖阻力;F为归一化摩阻比;
$ {\sigma _{{\text{v}}}} $ 为总上覆压力;${\sigma '_{{\text{v}}}}$ 为有效上覆压力;Pa为1个标准大气压;n为应力指数。n值通过以下方法确定:首先假设n取为1.0,若计算得到Ic>2.6,则土为黏土,n取为1.0;若Ic<2.6,则n改取为0.5,重新计算Ic。若重新计算的Ic<2.6,则n为0.5;若Ic>2.6,则n取为0.7。
1.2 分层依据
由于CPTU测试与单桥CPT测试相距30年,考虑再次定位的误差、场地高程变化及地下各土层厚度沿水平方向变化的复杂性,本研究按照CPTU测试数据进行分层,将单桥CPT测试时钻孔柱状图与比贯入阻力沿深度的变化趋势作为参考。分层时首先利用锥尖阻力和比贯入阻力在砂层处突然变大、摩阻比变小(砂土摩阻比一般<2%,黏土摩阻比基本>3%)的特性确定砂层位置。表层填土由于土质不均、成分混杂,曲线振荡幅度较大、无明显规律,所以分层时不再考虑。同一土层中,锥尖阻力和摩阻比一般较均匀,所以本次主要根据摩阻比和锥尖阻力沿深度的变化趋势,结合单桥CPT测试比贯入阻力沿深度分层的趋势特征,对CPTU测试数据进行分层。对于分层时的超前滞后效应,均按照单桥CPT测试时的超前滞后效应确定。分层后求出各土层平均锥尖阻力、侧壁摩阻力及摩阻比,利用Robertson土质分类图进行各土层土类检验。
2. 液化数据库检验
CPTU测试在唐山地区共选取16个测点,其中液化点11个,非液化点5个。限于篇幅,本文仅介绍6个测点,主要体现检验CPTU数据的过程及与Moss等(2011)数据库、Boulanger等(2014)数据库的主要区别。 (1)T1液化点(唐山陡河桥,10度区,地下水位3.700 m)
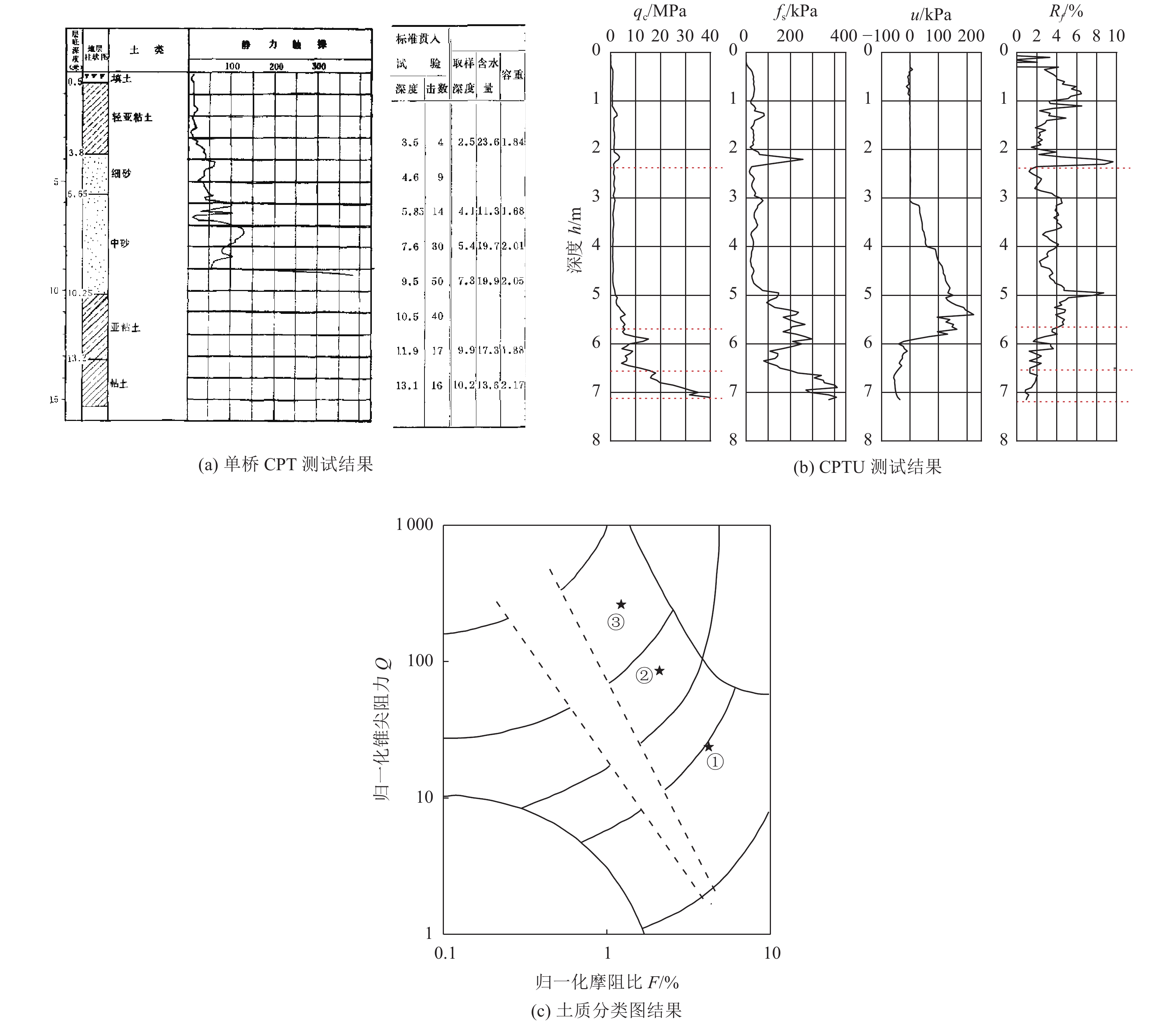
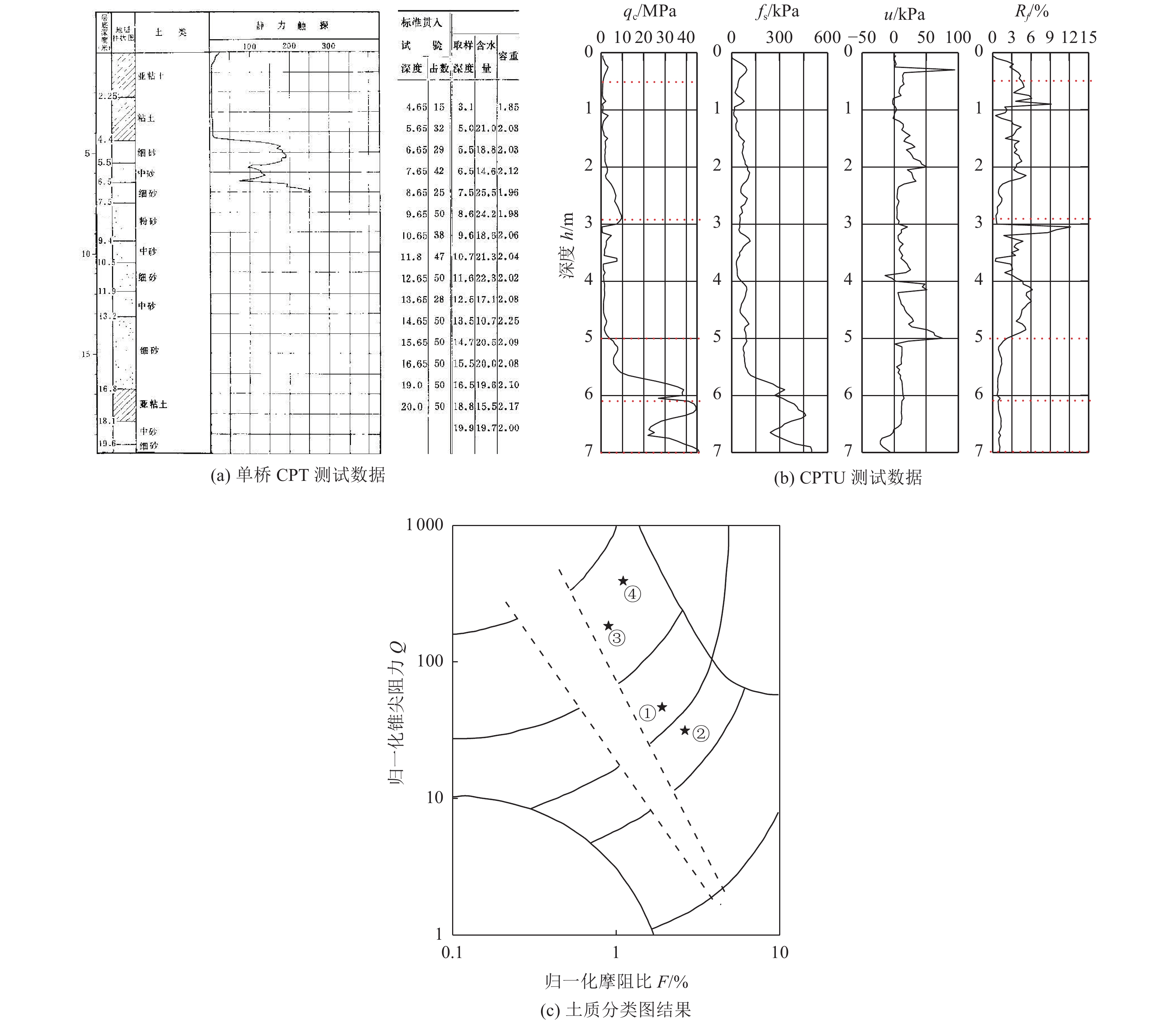
T1测点数据检验结果如图2所示。单桥CPT测试比贯入阻力-深度关系曲线(ps-h曲线)和CPTU测试锥尖阻力-深度关系曲线(qc-h曲线)趋势基本一致(qc-h曲线对应ps-h曲线0~6 m段)。根据CPTU测试摩阻比-深度关系曲线(Rf-h曲线)可知,T1测点土层自上而下可分为2类,分别为细粒土和砂土土层,与单桥CPT测试时钻孔柱状图土层土类对应程度较好。根据单桥CPT测试ps-h曲线可知,深度达3 m后,ps开始增大,但深度达3.8 m后才为砂层。根据CPTU测试qc-h曲线可知,深度达4.9 m后,锥尖阻力明显增大,所以按相同的超前滞后效应确定砂层位置从深度5.7 m处开始。确定砂层后,根据锥尖阻力和摩阻比进行分层,分层结果如表1所示。分层后将各土层数据代入Robertson土质分类图进行检验,检验结果如图2(c)所示。将图2(c)检验结果与单桥CPT测试时钻孔柱状图中的土类进行对比,结果如表2所示。由表2可知,2次测试的土层土类契合度较高,说明2次测试土层条件基本一致。深度存在的差别可能是场地填高或场地范围内土层水平向厚度不同导致的。对单桥CPT测试时的标贯击数与CPTU测试的平均锥尖阻力进行综合分析,细砂层标贯击数为9,平均锥尖阻力为8.23 MPa,中砂层标贯击数为22,平均锥尖阻力为26.67 MPa,标贯击数与平均锥尖阻力随深度变化趋势基本相符,综合判断T1测点为正确点。T1测点地下水位为3.700 m,根据2次测试得到的力学指标确定液化层为埋深5.7~6.55 m的细砂层。本研究对T1测点液化层的选取与Moss等(2011)数据库、Boulanger等(2014)数据库均一致。
表 1 T1测点CPTU分层结果Table 1. CPTU soil layer classification result of site T1分层深度/m qc/MPa fs/kPa Rf /% σv /kPa σ'v/kPa n Q F/% Ic 2.40~5.70 1.72 69.16 3.73 73.03 69.60 1.0 23.71 4.19 2.79 5.70~6.55 8.23 175.64 2.36 109.78 86.01 0.5 87.56 2.16 2.18 6.55~7.15 26.67 341.91 1.40 122.69 91.82 0.5 277.04 1.29 1.68 表 2 T1测点土层土类检验结果Table 2. Soil layer inspection results of site T1序号 单桥CPT测试钻孔土类 Robertson土质分类图土类 ① 轻亚黏土(深度0.50~3.80 m) 粉质黏土-黏土(深度2.40~5.70 m) ② 细砂(深度3.80~5.65 m) 粉砂-砂质粉土(深度5.70~6.55 m) ③ 中砂(深度5.65~10.20 m) 纯净砂-粉砂(深度6.55~7.15 m) (2)T2液化点(唐山洼里,10度区,地下水位1.250 m)
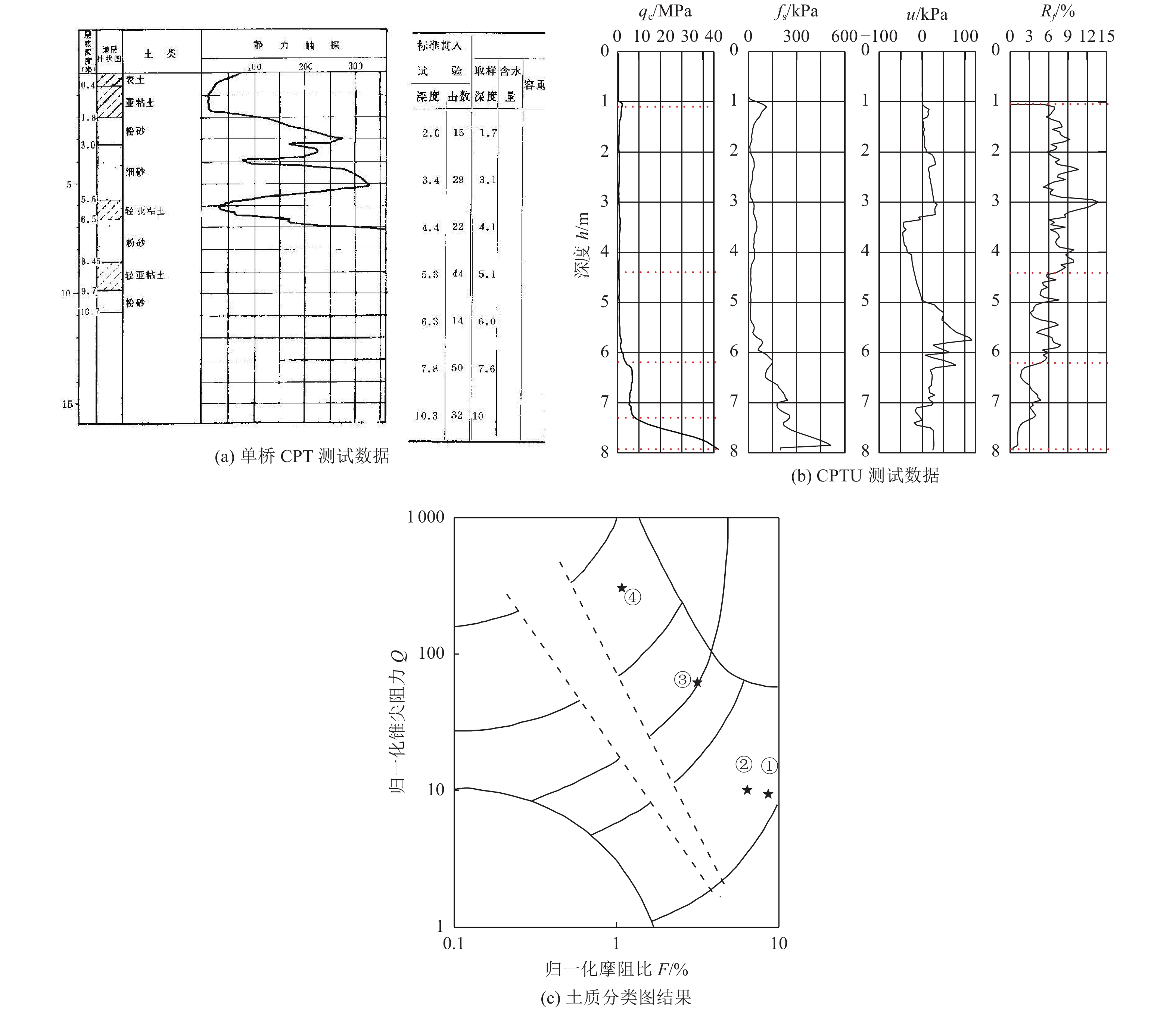
T2测点数据检验结果如图3所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势不一致。由CPTU测试Rf-h曲线可知,T2测点多为细粒土土层,仅在末端存在层厚较小的砂层,与单桥CPT测试时钻孔柱状图土层土类不符。按照CPTU测试数据重新分层,结果如表3所示,将各土层数据代入Robertson土质分类图,结果如图3(c)所示。将图3(c)土类结果与单桥CPT测试时钻孔柱状图中的土类进行对比,结果如表4所示。由表4可知,2次测试各土层土类条件不一致,推测2次测试不在同一测点。综合判断T2测点为错误点,应剔除。Moss等(2011)数据库认为T2测点为正确点,本研究与Boulanger等(2014)数据库均认定T2测点为错误点。
表 3 T2测点CPTU分层结果Table 3. CPTU soil layer classification result of site T2分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 0.35~2.20 1.09 72.25 6.57 24.62 24.37 1.0 43.65 6.79 2.75 2.20~4.20 2.97 245.18 8.36 61.78 42.67 1.0 68.10 8.44 2.70 4.20~6.90 3.99 232.30 5.86 107.15 65.01 1.0 59.73 5.98 2.62 6.90~8.30 6.52 257.71 3.75 146.73 84.50 0.5 69.30 4.05 2.45 8.30~8.45 18.63 302.42 1.79 161.75 91.93 0.5 192.58 1.64 1.86 表 4 T2测点土层土类检验结果Table 4. Soil layer inspection results of site T2序号 单桥CPT测试钻孔土类 Robertson土质分类图土类 ① 亚黏土(深度0~2.3 m) 粉质黏土-黏土(深度0.35~2.20 m) ② 细砂(深度2.3~3.7 m) 极硬细砂(深度2.20~4.20 m) ③ 中砂(深度3.7~4.4 m) 粉质黏土-黏土(深度4.20~6.90 m) ④ 淤泥质亚黏土(深度4.4~5.0 m) 黏质粉土-粉质黏土(深度6.90~8.30 m) ⑤ 粉砂(深度5.0~6.8 m) 纯净砂-粉砂(深度8.30~8.45 m) (3)T3非液化点(丰南县胥各庄,10度区,地下水位1.500 m)
T3测点数据检验结果如图4所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势不一致。由CPTU测试Rf-h曲线可知,深度达6.2 m后出现砂土类土层,与单桥CPT测试时钻孔柱状图土层土类不相符。按照CPTU测试数据重新分层,结果如表5所示。分层后将各土层数据代入Robertson土质分类图,结果如图4(c)所示。将图4(c)土层土类结果与单桥CPT测试时钻孔柱状图中的土类进行对比,结果如表6所示。由表6可知,2次测试的土层土类不契合。单桥CPT测试时标贯击数与比贯入阻力值均较大,而CPTU测试的锥尖阻力均较小,标贯击数与平均锥尖阻力随深度的变化趋势不相符。综合判断,2次测试不在同一测点,T3测点为错误点,应剔除。本研究与Moss等(2011)数据库、Boulanger等(2014)数据库均认定T3测点为错误点。
表 5 T3测点CPTU分层结果Table 5. CPTU soil layer classification result of site T3分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 1.05~4.40 0.44 33.49 7.94 52.61 40.60 1.0 9.64 8.56 3.29 4.40~6.20 0.77 42.84 5.42 102.32 65.08 1.0 10.32 6.38 3.18 6.20~7.30 5.87 179.92 3.11 130.48 79.03 0.5 64.56 3.13 2.39 7.30~7.95 29.33 316.81 1.28 148.01 87.98 0.5 311.11 1.09 1.59 表 6 T3测点土层土类检验结果Table 6. Soil layer inspection results of site T3序号 单桥CPT测试钻孔土类 Robertson土质分类图
土类① 无 粉质黏土-黏土
(深度1.05~4.40 m)② 无 粉质黏土-黏土
(深度4.40~6.20 m)③ 亚黏土
(深度0.4~1.8 m)黏质粉土-粉质黏土
(深度6.20~7.30 m)④ 粉砂
(深度1.8~3.0 m)纯净砂-粉砂
(深度7.30~7.95 m)(4)T6液化点(唐山西大夫坨,10度区,地下水位1.500 m)
T6测点数据检验结果如图5所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势一致。由CPTU测试Rf-h曲线可知,T6测点土层自上而下可分为2类,分别为细粒土和砂土土层,与单桥CPT测试时钻孔柱状图土层土类对应程度好。按照CPTU测试数据重新分层,由单桥CPT测试ps-h曲线可知,深度达4.2 m后ps开始增大,但深度达4.4 m后才为砂层。由CPTU测试qc-h曲线可知,深度达4.8 m后锥尖阻力明显增大,所以按照相同的超前滞后效应确定砂层位置从深度5 m处开始。确定砂层深度后,根据锥尖阻力和摩阻比进行分层,分层结果如表7所示。分层后将各土层数据代入Robertson土质分类图,结果如图5(c)所示。将图5(c)土类结果与单桥CPT测试钻孔柱状图中的土类进行对比,结果如表8所示。由表8可知,2次测试的土层土类契合度较高,深度存在的差别可能是场地填高或场地范围内土层水平向厚度不同导致的。对比单桥CPT测试时的标贯击数与CPTU测试的平均锥尖阻力,细砂层标贯击数为15,平均锥尖阻力为17.71 MPa,中砂层标贯击数为32,平均锥尖阻力为35.30 MPa,标贯击数与平均锥尖阻力随深度的变化趋势基本相符,综合判断T6测点为正确点,液化层选为深度为5.0~6.1 m的细砂层。Moss等(2011)数据库对该测点液化层的选取与本研究一致。Boulanger等(2014)数据库将该测点液化层选为深度2.4~3.0 m,虽然该层摩阻比较小,属于砂土类,锥尖阻力较小,易液化,但与单桥CPT测试时钻孔柱状图中的土层土类无法对应,这样选取显然是错误的。
表 7 T6测点CPTU分层结果Table 7. CPTU soil layer classification result of site T6分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 0.50~2.85 3.04 60.75 3.04 32.33 30.62 0.5 54.36 2.02 2.31 2.85~5.00 2.48 67.54 4.16 75.77 52.01 0.5 33.36 2.81 2.56 5.00~6.10 17.71 164.39 1.07 106.50 66.81 0.5 215.47 0.93 1.65 6.10~7.00 35.30 384.7 1.11 125.42 75.94 0.5 403.68 1.09 1.53 表 8 T6测点土层土类检验结果Table 8. Soil layer inspection results of site T6序号 单桥CPT测试钻孔土类 Robertson土质分类图土类 ① 亚黏土(深度0~2.25m) 粉砂-砂质粉土(深度0.50~2.85m) ② 黏土(深度2.25~4.40m) 黏质粉土-粉质黏土(深度2.85~5.00m) ③ 细砂(深度4.40~5.50m) 纯净砂-粉砂(深度5.00~6.10m) ④ 中砂(深度5.50~6.50m) 纯净砂-粉砂(深度6.10~7.00m) (5)T15液化点(滦县佘庄,9度区,地下水位1.000 m)
T15测点数据检验结果如图6所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势基本一致。由CPTU测试qc-h曲线和Rf-h曲线可知,所测土层均为砂层,由于单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势基本一致,所以按照单桥CPT测试ps-h曲线拐点进行分层,结果如表9所示。分层后将各土层数据代入Robertson土质分类图,结果如图6(c)所示,将图6(c)检验结果与单桥CPT测试时钻孔柱状图中的土层土类进行对比,结果如表10所示。由表10可知,2次测试的土层土类契合度较高,说明2次测试的土层条件基本一致。中砂层标贯击数为11,平均锥尖阻力为17.23 MPa,粉砂层标贯击数为21,平均锥尖阻力为12.66 MPa,更深的砂层标贯击数与锥尖阻力均变的较大,不易液化,标贯击数与平均锥尖阻力变化趋势基本一致。综合判断T15测点为正确点,液化层选为深度为1.4~4.8 m的粉砂层。Moss等(2011)数据库、Boulanger等(2014)数据库均将T15测点作为错误点剔除是不对的。
表 9 T15测点CPTU分层结果Table 9. CPTU soil layer classification result of site T15分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 0~0.3 5.35 36.79 0.80 2.98 2.98 0.5 309.53 0.69 1.44 0.3~1.4 17.23 126.53 0.80 17.02 17.02 0.5 417.42 0.73 1.38 1.4~4.8 12.66 97.74 0.81 60.39 39.81 0.5 199.69 0.78 1.61 4.8~6.5 23.57 212.09 0.89 109.784 64.21 0.5 292.79 0.90 1.55 6.5~6.9 34.00 319.35 0.93 130.90 75.04 0.5 390.93 0.94 1.48 表 10 T15测点土层土类检验结果Table 10. Soil layer inspection results of site T15序号 单桥CPT测试钻孔土类 Robertson土质分类图土类 ① 细砂(深度0~1.1 m) 纯净砂-粉砂(深度0~0.3 m) ② 中砂(深度1.1~2.6 m) 纯净砂-粉砂(深度0.3~1.4 m) ③ 粉砂(深度2.6~6.2 m) 纯净砂-粉砂(深度1.4~4.8 m) ④ 细砂(深度6.2~9.3 m) 纯净砂-粉砂(深度4.8~6.5 m) ⑤ 中砂(深度9.3~10.5 m) 纯净砂-粉砂(深度6.5~6.9 m) (6)T16非液化点(滦县东坨子头,9度区,地下水位3.500 m)
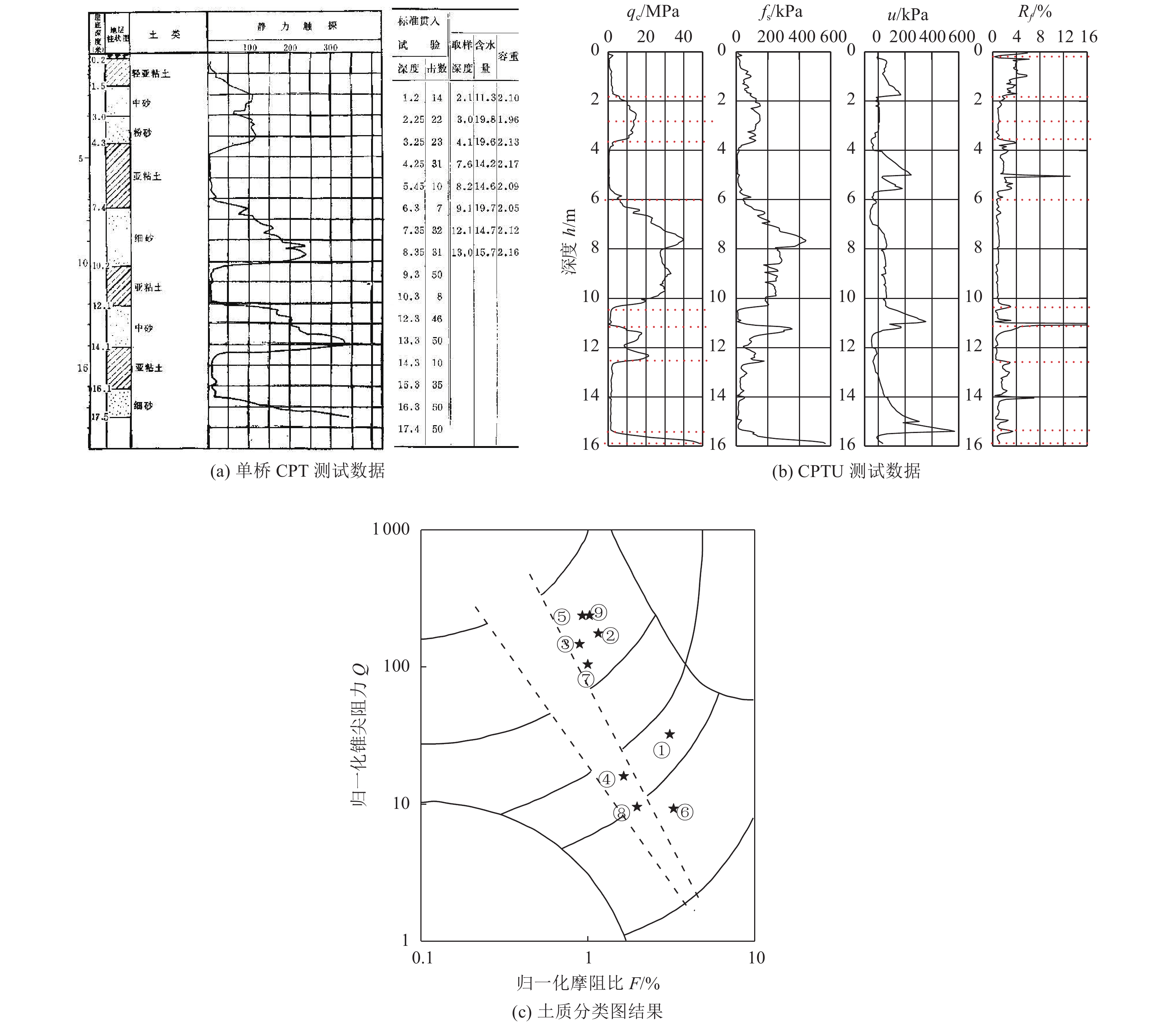
T16测点数据检验结果如图7所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势高度一致,所以按照单桥CPT测试土层曲线拐点对CPTU测试数据进行分层,结果如表11所示。分层后将各层数据代入Robertson土质分类图,结果如图7(c)所示。将图7(c)检验结果与单桥CPT测试时钻孔柱状图中的土层土类进行对比,结果如表12所示。由表12可知,2次测试的土层土类对应程度较高,说明2次测试的土层条件基本一致。深度存在的差别可能是场地范围内土层水平向厚度不同导致的。综合判断T16测点为正确点,临界液化层选为深度6.0~10.4 m的细砂层。Boulanger等(2014)数据库将液化层选为深度6.0~7.2 m的土层,本研究选为深度6.0~10.4 m的土层,该层细砂实际锥尖阻力较大,两端增大和减小段是出亚黏土层和进入亚黏土层的超前滞后效应造成的。
表 11 T16测点CPTU分层结果Table 11. CPTU soil layer classification result of site T16分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 0.25~1.80 1.65 55.03 3.67 18.98 18.98 0.5 37.62 3.36 2.58 1.80~2.80 11.57 132.98 1.21 42.94 42.94 0.5 175.99 1.15 1.77 2.80~3.60 11.85 113.82 1.00 60.89 60.89 0.5 151.18 0.96 1.77 3.60~6.00 1.37 22.76 2.23 91.47 78.73 1.0 16.35 1.77 2.69 6.00~10.40 25.55 234.77 0.97 160.48 114.42 0.5 237.38 0.92 1.61 10.40~11.10 1.53 43.13 4.26 213.75 142.70 1.0 9.28 3.26 3.04 11.10~12.50 14.29 138.92 1.09 235.05 153.71 0.5 113.38 0.99 1.87 12.50~15.50 1.91 35.20 1.74 277.65 174.75 1.0 9.37 2.15 2.94 15.50~15.90 33.09 334.69 0.97 309.59 190.03 0.5 237.85 1.02 1.65 表 12 T16测点土层土类检验结果Table 12. Soil layer inspection results of site T16序号 单桥CPT测试钻孔土类 Robertson土质分类图
土类① 轻亚黏土(0.2~1.5 m) 黏质粉土-粉质黏土(深度0.25~1.8 m) ② 中砂(1.5~3.0 m) 纯净砂-粉砂(深度1.8~2.8 m) ③ 粉砂(3.0~4.3 m) 纯净砂-粉砂(深度2.8~3.6 m) ④ 亚黏土(4.3~7.4 m) 黏质粉土-粉质黏土(深度3.6~6 m) ⑤ 细砂(7.4~10.2 m) 纯净砂-粉砂(深度6~10.4 m) ⑥ 亚黏土(10.2~12.1 m) 粉质黏土-黏土(深度10.4~11.1 m) ⑦ 中砂(12.1~14.1 m) 纯净砂-粉砂(深度11.1~12.5 m) ⑧ 亚黏土(14.1~16.1 m) 粉质黏土-黏土(深度12.5~15.5 m) ⑨ 细砂(12.1~14.1 m) 纯净砂-粉砂(深度15.5~15.9 m) 通过上述检验过程,本文剔除2个错误点T2、T3,剩下14个测点。其中,液化点10个,非液化点4个。
3. 液化数据库分析
根据《岩土工程勘察规范》(GB 50021—2001)(中华人民共和国建设部,2004)规定:当实测计算比贯入阻力或锥尖阻力小于液化比贯入阻力临界值pscr或液化锥尖阻力临界值qccr时,应判别为液化土,并按下列公式计算:
$$ p_{{\rm{s c r}}}=p_{{\rm{s}} 0} \alpha_{w} \alpha_{u} \alpha_{p}$$ (4) $$ q_{{\rm{c c r}}}=q_{{\rm{c}} 0} \alpha_{w} \alpha_{u} \alpha_{p}$$ (5) $$ \alpha_{w}=1-0.065\left(d_{w}-2\right) $$ (6) $$ \alpha_{\mathrm{u}}=1-0.05\left(d_{\mathrm{u}}-2\right) $$ (7) 式中,pscr、qccr分别为饱和土静力触探液化比贯入阻力临界值及锥尖阻力临界值;ps0、qc0分别为地下水深度dw=2 m、上覆非液化土层厚度(计算时应将淤泥和淤泥质土层厚度扣除)du=2 m时,饱和土液化判别比贯入阻力基准值和液化判别锥尖阻力基准值(MPa),可按表13取值,10度区基准值按规范原始文献取值(周神根,1980);αw为地下水位埋深修正系数,地面常年有水且与地下水有水力联系时取1.13;αu为上覆非液化土层厚度修正系数,对于深基础取1.0;αp为与静力触探摩阻比有关的土性修正系数,可按表14取值。
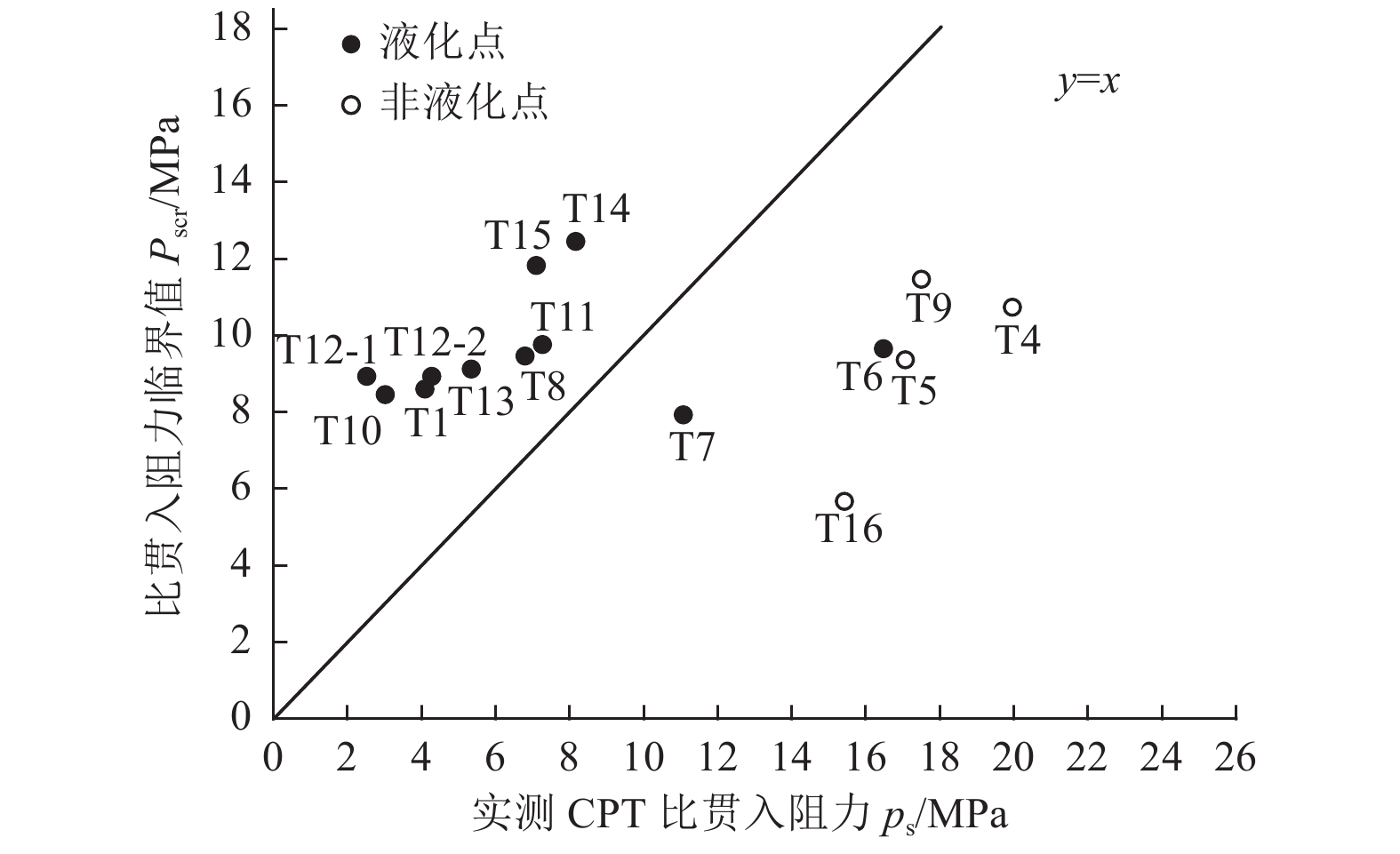
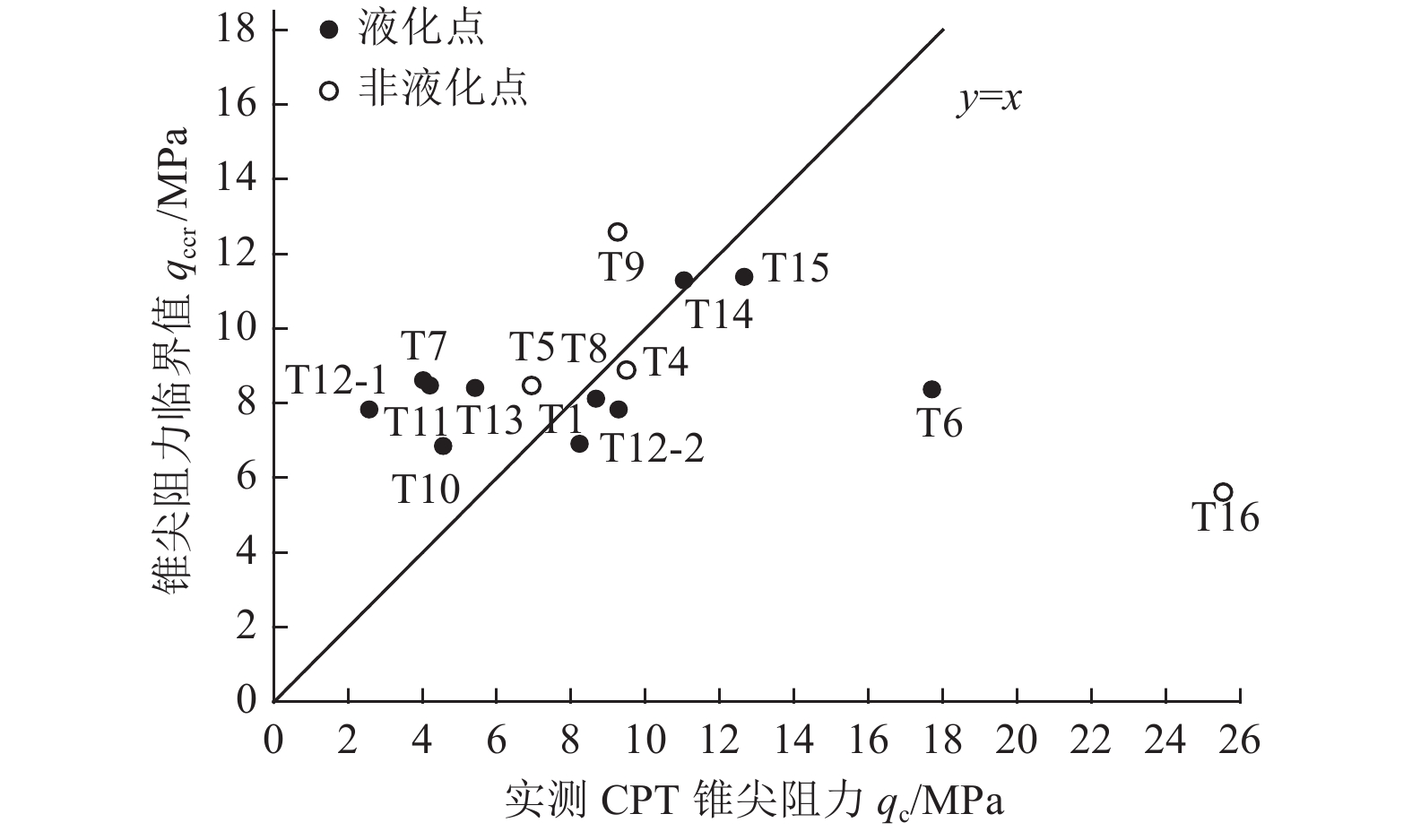
表 13 比贯入阻力和锥尖阻力基准值ps0、qc0Table 13. Liquefied reference value of specific penetration resistance and cone tip resistance参数 抗震设防烈度 7度 8度 9度 ps0/MPa 5.0~6.0 11.5~13.0 18.0~20.0 qc0/MPa 4.6~5.5 10.5~11.8 16.4~18.2 表 14 土性修正系数αp值Table 14. Values of soil property correction factor αp参数 土类 砂土 粉土 摩阻比Rf Rf≤0.4 0.4<Rf≤0.9 Rf>0.9 αp 1. 00 0.60 0.45 对于本研究通过检验的14个测点,分别针对单桥CPT测试比贯入阻力与CPTU测试锥尖阻力,分析液化可能性,建立液化数据库,如表15、16所示,液化判别结果如图8、9所示。
表 15 基于单桥CPT测试的唐山地震液化数据库Table 15. Database of ps-based liquefaction case histories in Tangshan earthquake测点 液化
情况地震
烈度/度土层
深度/mPs0/MPa dw/m du/m αw αu αp Pscr/MPa 实测Ps/MPa 液化判别 T1 液化 10 3.80~5.65 23.5 3.70 3.80 0.89 0.91 0.45 8.56 4.05 是 T4 非液化 10 2.90~3.50 23.5 1.10 2.90 1.06 0.96 0.45 10.69 19.92 否 T5 非液化 10 3.15~5.20 23.5 3.00 3.15 0.94 0.94 0.45 9.32 17.02 否 T6 液化 10 4.40~5.50 23.5 1.50 4.40 1.03 0.88 0.45 9.61 16.44 否 T7 液化 10 6.05~7.05 23.5 3.00 6.05 0.94 0.80 0.45 7.89 11.03 否 T8 液化 10 3.95~7.00 23.5 2.20 3.95 0.99 0.90 0.45 9.42 6.75 是 T9 非液化 10 6.70~8.20 23.5 1.10 6.70 1.06 0.77 0.60 11.42 17.46 否 T10 液化 9 3.00~5.50 19.0 1.45 3.00 1.04 0.95 0.45 8.41 2.98 是 T11 液化 9 0.85~3.40 19.0 0.85 0.85 1.07 1.06 0.45 9.71 7.22 是 T12-1 液化 9 1.80~3.20 19.0 1.55 1.80 1.03 1.01 0.45 8.89 2.48 是 T12-2 液化 9 3.20~10.20 19.0 1.55 1.80 1.03 1.01 0.45 8.89 4.24 是 T13 液化 9 2.00~3.80 19.0 1.05 2.00 1.06 1.00 0.45 9.08 5.30 是 T14 液化 9 1.25~2.10 19.0 1.25 1.25 1.05 1.04 0.60 12.40 8.13 是 T15 液化 9 2.60~6.20 19.0 1.00 2.60 1.07 0.97 0.60 11.78 7.06 是 T16 非液化 9 7.40~10.20 19.0 3.50 7.40 0.90 0.73 0.45 5.63 15.38 否 表 16 基于CPTU测试的唐山地震液化数据库Table 16. Database of qc-based liquefaction case histories in Tangshan earthquake测点 液化
情况地震
烈度/度土层
深度/mqc0/MPa dw/m du/m αw αu αp qccr/MPa 实测qc/MPa 液化判别 T1 液化 10 5.70~6.55 21.2 3.70 5.70 0.89 0.8150 0.45 6.92 8.23 否 T4 非液化 10 4.40~5.00 21.2 1.10 4.40 1.06 0.8800 0.45 8.89 9.49 否 T5 非液化 10 3.00~4.20 21.2 3.00 3.00 0.94 0.9500 0.45 8.47 6.94 是 T6 液化 10 5.00~6.10 21.2 1.50 5.00 1.03 0.8500 0.45 8.37 17.71 否 T7 液化 10 3.00~4.00 21.2 3.00 3.00 0.94 0.9500 0.45 8.47 4.20 是 T8 液化 10 4.75~7.40 21.2 2.20 4.75 0.99 0.8630 0.45 8.12 8.67 否 T9 非液化 10 3.30~4.80 21.2 1.10 3.30 1.06 0.9350 0.60 12.59 9.25 是 T10 液化 9 5.00~6.70 17.3 1.45 5.00 1.04 0.8500 0.45 6.85 4.93 是 T11 液化 9 1.40~2.60 17.3 0.85 1.40 1.07 1.0300 0.45 8.62 4.02 是 T12-1 液化 9 2.45~4.80 17.3 1.55 2.45 1.03 0.9775 0.45 7.83 2.57 是 T12-2 液化 9 4.80~9.40 17.3 1.55 2.45 1.03 0.9775 0.45 7.83 9.28 否 T13 液化 9 1.65~3.00 17.3 1.05 1.65 1.06 1.0175 0.45 8.41 5.42 是 T14 液化 9 1.25~2.10 17.3 1.25 1.25 1.05 1.0375 0.60 11.29 11.04 是 T15 液化 9 1.40~4.80 17.3 1.00 1.40 1.07 1.0300 0.60 11.39 12.66 否 T16 非液化 9 6.00~10.40 17.3 3.50 6.00 0.90 0.8000 0.45 5.62 25.55 否 对比表15、16可知,CPTU测试qc大于单桥CPT测试ps的测点有T1、T6、T8、T10、T12-1、T12-2、T14、T15、T16,CPTU测试测点液化层深度大于单桥CPT测试的测点有T1、T4、T6、T8、T10、T11、T12-1、T12-2。从液化判别结果来看,基于ps指标的液化判别方法判别成功率较高,因为我国规范CPT液化判别方法是利用这些数据构建的。而基于qc指标的液化判别方法判别成功率较低,将液化判别为不液化的有测点T1、T6、T8、T12-2、T15,将不液化判别为液化的测点有T5、T9,液化点整体有向右移动的趋势。
综合来看,经过30年的时间,土层液化可能性已发生较大改变,利用CPTU测试数据建立的液化数据库可靠性较低,并不能代表1976年唐山地震时的液化情况。
4. 结论
本文通过对比2次静力触探数据,利用Robertson土质分类图,进行新CPTU数据土类分层检验,将检验结果与单桥CPT测试时钻孔柱状图进行对比,发现大部分测点土层土类均能较好对应,现场测试力学指标沿深度的变化趋势较相符,仅剔除了错误点T2、T3。
对所有测点选定液化层,分别建立了基于单桥CPT测试ps指标和基于CPTU测试qc指标的液化数据库。利用我国规范CPT液化判别方法,检验了2个数据库的数据,发现基于单桥CPT测试ps指标的数据库液化点和非液化点得到了很好的区分,而基于CPTU测试qc指标的数据库判别效果较差,说明经过30年的时间,土层液化可能性已发生较大改变。因此,基于CPTU测试建立的液化数据库可靠性较低,基于该数据库对液化判别方法进行改进意义较小。
-
表 1 数值仿真过程参数
Table 1. Corresponding parameters of soil layers
种类 参数 数值 气相性质 密度/(kg·m−3)
粘度/(kg·m−1·s−1)
质量入口/(kg·s−1)
压力出口/Pa1.169
1.55×10−5
4.6
0颗粒性质 密度/(kg·m−3)
泊松比
剪切模量/Pa
粒径/mm
生成速率/(kg·s−1)1 800
0.3
1.0×107
101.6
3.3壁面性质 密度/(kg·m−3)
泊松比
剪切模量/Pa1 210
0.4
7.0×107颗粒-颗粒 碰撞恢复系数
静摩擦系数
滚动摩擦系数0.4
0.5
0.04颗粒-管壁 碰撞恢复系数
静摩擦系数
滚动摩擦系数0.5
0.2
0.03表 2 不同直径管道内气体流速与运输量
Table 2. Gas flow velocity and transport volume in different diameters
管径/mm 气体质量流量/(kg·s−1) 空管时管内气体平均流速/(m·s−1) 0~25 s内运输颗粒总质量/kg 230 4.6 94.6 73.9 250 4.6 80.1 72.2 280 4.6 63.9 66.4 300 4.6 55.7 0 表 3 不同颗粒生成速率下的运输量
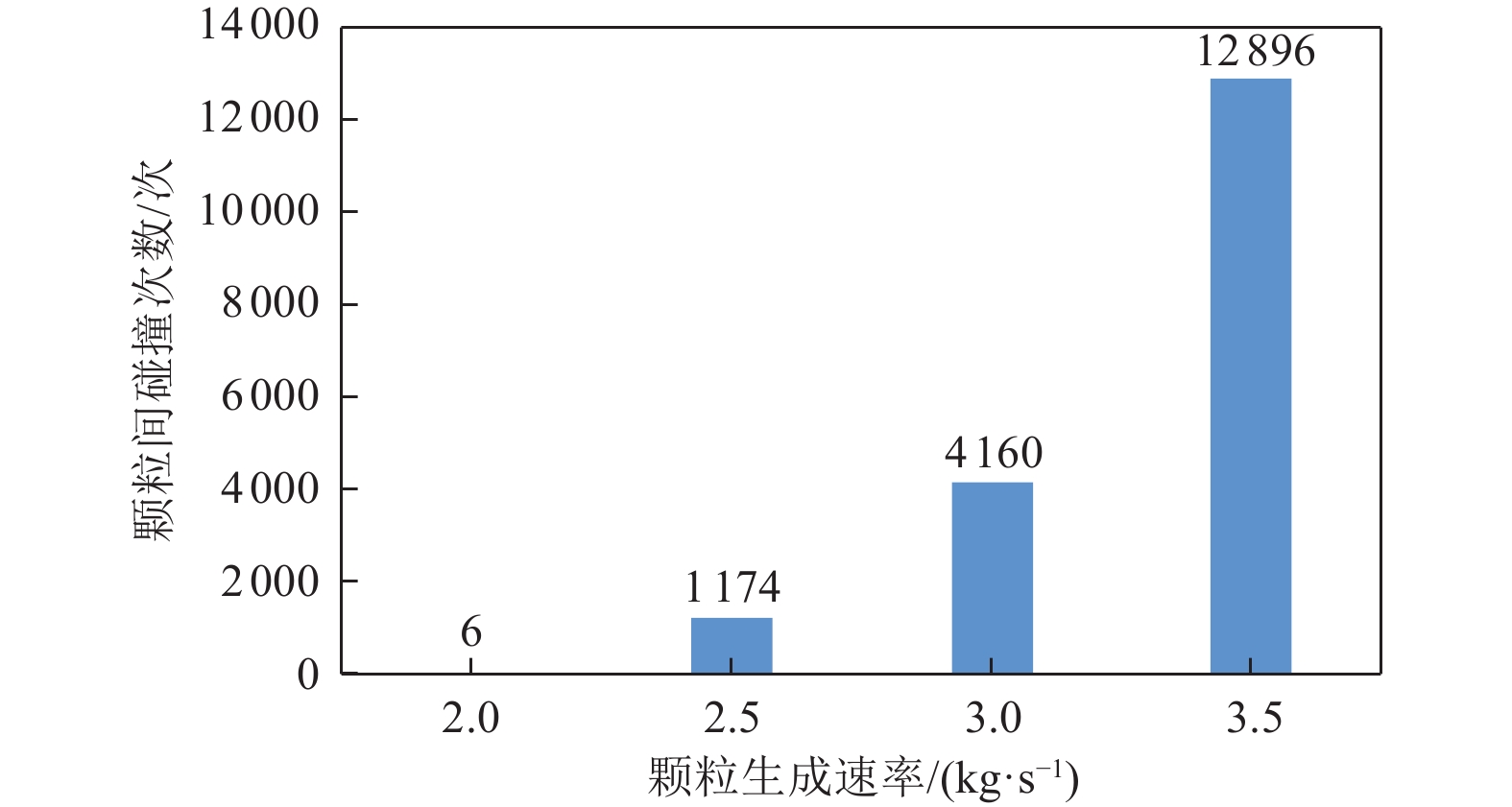
Table 3. The amount of transport at different generation rates
颗粒生成速率/(kg·s−1) 0~25 s内运输颗粒总质量/kg 3.3 0 3.0 41.5 2.5 34.0 2.0 18.3 表 4 试验测量与仿真结果对比
Table 4. Experimental results and simulation results
管径/mm 试验测量管道
内压损失/Pa仿真得到管道
内压损失/Pa仿真结果
相对误差/%试验测量运输
速率/(t·h−1)试验测量0~25 s内
运输量/kg仿真得到0~25 s内
运输量/kg仿真结果
相对误差/%230 8253 7543 −8.6 9.7 67.6 73.9 9.3 250 6028 5558 −7.8 9.3 64.2 72.2 12.5 280 3352 3004 −10.4 8.3 57.3 66.4 13.7 -
崔益华, 2021. 水平管道负压气力输送CFD-DEM数值模拟. 港口装卸, (1): 63—67Cui Y. H. , 2021. CFD-DEM numerical simulation of horizontal pipeline negative pressure pneumatic conveying. Port Operation, (1): 63—67. (in Chinese) 杜俊, 2015. 基于CFD-DEM方法的稀相气力输送数值模拟研究. 武汉: 武汉大学.Du J., 2015. Simulation of dilute pneumatic conveying by CFD-DEM. Wuhan: Wuhan University. (in Chinese) 封凯, 聂伟, 陈凤官等, 2021. 气力输送系统中弯管的易磨损位置及其机理分析. 化学反应工程与工艺, 37(2): 106—112Feng K. , Nie W. , Chen F. G. , et al. , 2021. Positioning the erosion wear of bend pipes in the pneumatic conveying system. Chemical Reaction Engineering and Technology, 37(2): 106—112. (in Chinese) 胡国明, 2010. 颗粒系统的离散元素法分析仿真. 武汉: 武汉理工大学出版社.Hu G. M., 2010. Analysis and simulation of granular system by Discrate element method using EDEM. Wuhan: Wuhan University of Technology Press. (in Chinese) 胡昱, 黄小华, 陶启友等, 2019. 基于CFD-EDM的自动投饵饲料颗粒气力输送数值模拟. 南方水产科学, 15(3): 113—119Hu Y. , Huang X. H. , Tao Q. Y. , et al. , 2019. Simulation of automatic pneumatic conveying process of automatic feeding system based on computational fluid dynamics-discrete element method. South China Fisheries Science, 15(3): 113—119. (in Chinese) 柳波, 吴中鼎, 尹高冲等, 2018. 供料器内颗粒碰撞条件下的两相流压力特性仿真分析. 现代制造工程, (3): 93—98Liu B. , Wu Z. D. , Yin G. C. , 2018. Simulation analysis of the pressure characteristics of two-phase flow in feeding device based on particle collision. Modern Manufacturing Engineering, (3): 93—98. (in Chinese) 刘德天, 傅旭东, 王光谦, 2017. CFD-DEM耦合计算中的体积分数算法. 清华大学学报(自然科学版), 57(7): 720—727Liu D. T. , Fu X. D. , Wang G. Q. , 2017. Volume fraction allocation using characteristic points for coupled CFD-DEM calculations. Journal of Tsinghua University (Science and Technology), 57(7): 720—727. (in Chinese) 庞林祥, 贾志勇, 2018. 我国地震救援装备现状与发展趋势. 水利水电快报, 39(6): 27—30. 王国强, 郝万军, 王继新, 2010. 离散单元法及其在EDEM上的实践. 西安: 西北工业大学出版社.Wang G. Q. , Hao W. J. , Wang J. X. , 2010. Discrete element method and its practice on EDEM. Xi’an: Northwestern Polytechnical University Press. (in Chinese) 周甲伟, 上官林建, 许兰贵等, 2022. 粗重颗粒轻介共流气力输送机理及特性. 机械工程学报, 58(14): 308—319Zhou J. W. , Shangguan L. J. , Xu. L. G. , 2022. Mechanism and characteristics of light medium mixed flow pneumatic conveying for coarse particle. Journal of Mechanical Engineering, 58(14): 308—319. (in Chinese) Bharadwaj R. , Ketterhagen W. R. , Hancock B. C. , 2010. Discrete element simulation study of a Freeman powder rheometer. Chemical Engineering Science, 65(21): 5747—5756. doi: 10.1016/j.ces.2010.04.002 Laín S. , Sommerfeld M. , 2019. Numerical prediction of particle erosion of pipe bends. Advanced Powder Technology, 30(2): 366—383. doi: 10.1016/j.apt.2018.11.014 Yang D. L. , Wang Y. X. , Li J. P. , et al. , 2020. Simulation study on interaction coefficient of DEM in non–spherical large size (5–30 mm) coal particles. Particuology, 53: 142—153. doi: 10.1016/j.partic.2020.03.001 期刊类型引用(5)
1. 张婷. 基于微信小程序的江苏地震应急联络通讯录设计与实现. 地震科学进展. 2024(02): 159-163 .  百度学术
百度学术2. 符捷,陈梅,刘霞. 基于微信小程序的医院设施设备报修系统设计. 电子设计工程. 2024(19): 58-61+66 .  百度学术
百度学术3. 程树岐,王西宝,刘海刚,王帅合,赵小贺. 地震中心站观测设备实时跟踪管理系统设计与开发. 防灾减灾学报. 2023(03): 52-57 .  百度学术
百度学术4. 刘彦会. 基于自适应遗传算法的微信小程序界面信息布局设计方法. 湖北科技学院学报. 2023(06): 135-140 .  百度学术
百度学术5. 王渊博,李晶,马世鹏. 基于微信小程序的评价工具设计. 湘潭大学学报(自然科学版). 2022(04): 110-117 .  百度学术
百度学术其他类型引用(2)
-





 下载:
下载:

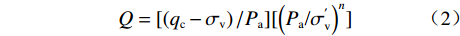

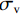
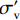






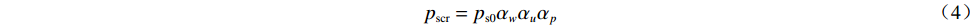
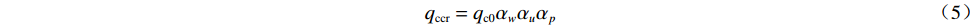
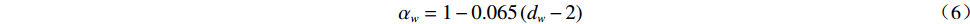
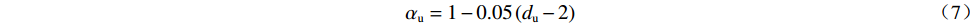


 下载:
下载: