内置草绳增强承重夯土墙体抗震性能试验研究

虞庐松; 宋书豪; 李子奇; 王力; 李健宁

doi:10.11899/zzfy20230418

内置草绳增强承重夯土墙体抗震性能试验研究

doi: 10.11899/zzfy20230418

虞庐松^1,,
宋书豪¹,
李子奇^{1, 2, ,},
王力^{1, 2},
李健宁^{1, 2}

1.
兰州交通大学, 土木工程学院, 兰州 730070
2.
兰州交通大学, 甘肃省道路桥梁与地下工程重点实验室, 兰州 730070

基金项目: 甘肃省高等学校青年博士基金项目（2023QB-045）；中央引导地方科技发展资金项目（ 22ZY1QA005）；陇原青年创新创业人才（团队）项目；兰州交通大学甘肃省重点实验室开放课题（2022055）

详细信息

作者简介:
虞庐松，男，生于1968年。博士，教授，硕士生导师。主要从事桥梁抗震工作。E-mail：yulusong@mail.lzjtu.cn

通讯作者:
李子奇，男，生于1974年。博士，副教授，硕士生导师。主要从事结构抗震工作。E-mail：46939099@qq.com

计量
- 文章访问数: 70
- HTML全文浏览量: 19
- PDF下载量: 6
- 被引次数: 0
出版历程
- 收稿日期: 2022-09-07
- 刊出日期: 2023-12-01

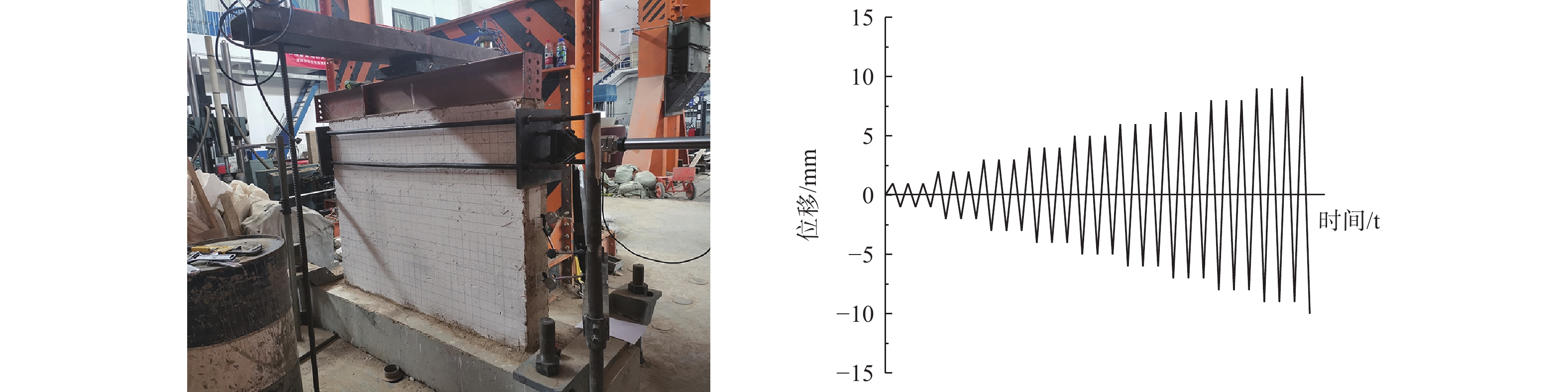
Experimental Study on Seismic Performance of Built-in Straw Rope Reinforced Load-bearing Rammed Earth Wall

Yu Lusong^1
,,
Song Shuhao¹,
Li Ziqi^{1, 2
, ,},
Wang Li^{1, 2},
Li Jianning^{1, 2}

1.
School of Civil Engineering, Lanzhou Jiaotong University, Lanzhou 730070, China
2.
Key Laboratory of Road and Bridge and Underground Engineering of Gansu Province, Lanzhou Jiaotong University, Lanzhou 730070, China

摘要

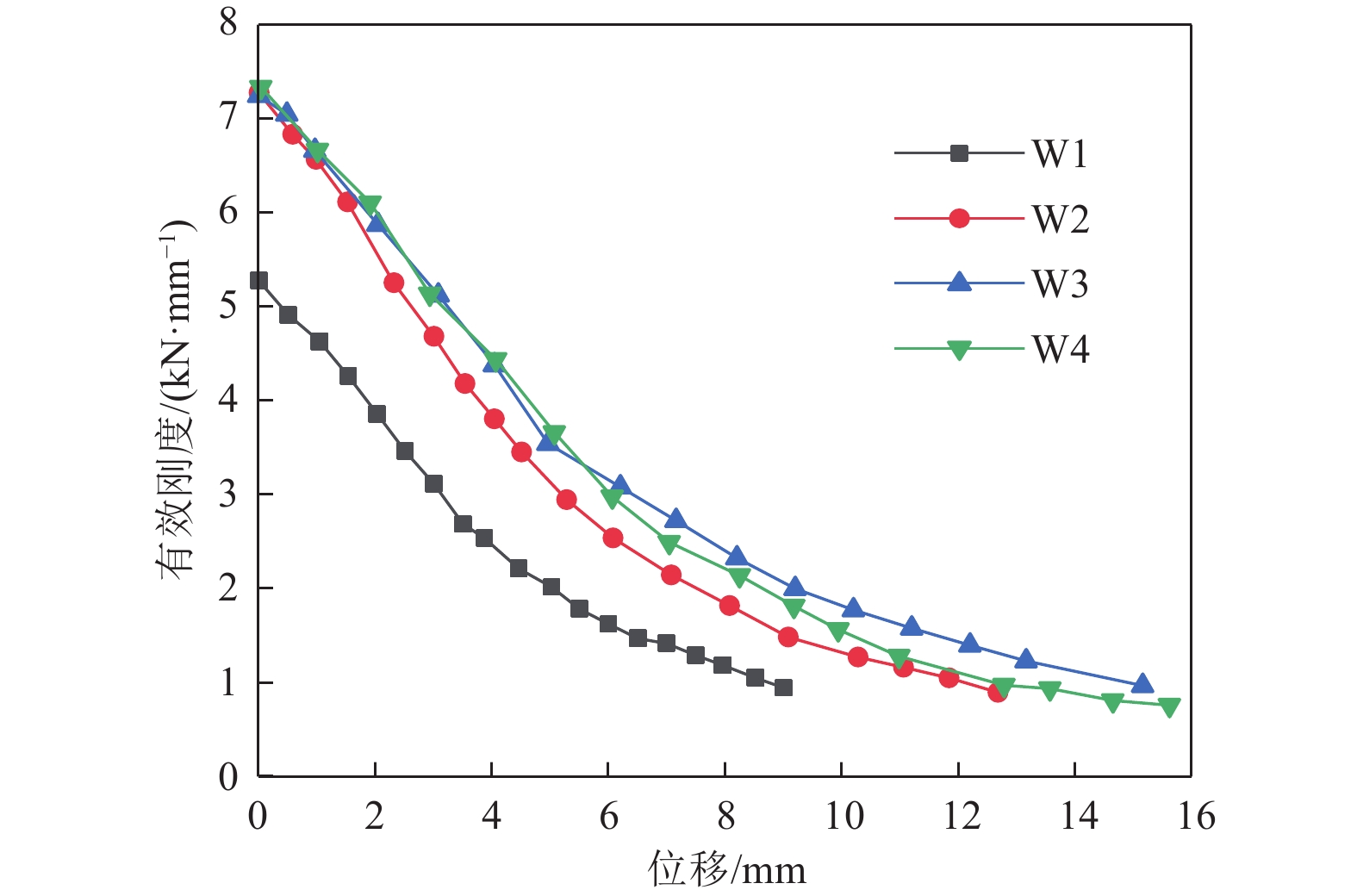
摘要: 为了解决夯土墙体夯筑分层处受力薄弱的问题，本文提出一种内置草绳增强承重夯土墙体性能的方法，以草绳含量及埋置高度为变量，对4片夯土墙体1/3缩尺模型进行拟静力试验，探究其破坏形态、承载及变形能力、耗能能力、刚度退化等抗震性能。试验结果表明：内置草绳可提高墙体各夯筑层间粘结性，延缓夯筑分层处水平裂缝的开展及贯通，从而提高墙体整体性，此外其对墙体承载能力、变形能力均有提升，且提升率与草绳高度呈正相关，二者分别提升108.3%和35.6%；内置草绳的墙体试件滞回曲线更为饱满，达到破坏荷载时，其等效黏滞阻尼系数在0.16～0.23之间，同时初始刚度较无草绳墙体提升40%，且加载过程中刚度始终高于无草绳墙体。实际应用中，建议内置草绳高度应超过1/2墙高。研究成果可对夯土建筑的抗震设计及应用推广提供参考依据。
- 生土结构 /
- 夯土墙体 /
- 拟静力试验 /
- 抗震性能
Abstract: In order to solve the problem of weak stress at the rammed layer of rammed earth wall, a load-bearing rammed earth wall reinforced by built-in grass rope is proposed. Taking the grass rope content and embedded height as variables, in order to explore the seismic performance of the wall, such as failure mode, bearing capacity, deformation capacity, energy dissipation capacity and stiffness degradation, a quasi-static test was carried out on four rammed earth walls with scale ratio being 1/3. The test results show that the built-in grass rope can improve the adhesion between the rammed layers of the wall, delay the development and penetration of the horizontal cracks at the rammed layers, and thus improve the integrity of the wall; In addition, the bearing capacity and deformation capacity of the wall are improved, and the lifting rate is positively correlated with the height of the grass rope, which is increased by 108.3% and 35.6% respectively; The hysteresis curve of the wall specimen with built-in grass rope is fuller than that of the wall specimen without grass rope. When the failure load is reached, the equivalent viscous damping coefficient is between 0.16 and 0.23, and the initial stiffness is 40% higher than that of the wall without grass rope. In practical applications, it is recommended that the height of the built-in grass rope should exceed 1/2 wall height. The research results can provide reference for seismic design and application of rammed earth buildings.
- Raw soil structure /
- Rammed earth wall /
- Quasi-static test /
- Seismic performance

HTML全文

引言

地震前兆观测主要关注观测数据随时间的相对变化，装置系数误差不影响观测数据的相对变化，但不正确的装置系数可能导致地电阻率观测结果出现系统误差（王兰炜等，2014），因此，正确的装置系数有利于不同区域观测数据的对比和地震前兆数据的研究。自2009年起，河北大柏舍台，甘肃天水台、武都台、平凉台，陕西合阳台实施了井下地电阻率垂直观测试验，井孔深100—225m，供电极距60—120m，测量极距20—60m（刘君等，2015；王兰炜等，2015）。上述台站地电阻率垂向观测通常为1个钻孔，供电电极和测量电极均布设于1个钻孔中，部分垂向观测的供电电极A接近地表，如天水台、武都台、合阳台的供电电极A埋深仅4—5m；部分垂向观测的供电电极A埋深为40m左右，如大柏舍台。垂向地电阻率观测中的装置系数与电流的空间分布及电极位置有关，现有垂向观测装置系数计算方法依据地下点、地表点电流源产生的电场计算得出，忽略了供电电极A的埋深。江宁台深井垂向地电阻率观测装置为在2口深井进行垂向观测的试验装置，与传统垂向地电阻率观测装置不同。本文根据地下点电流源产生的电场讨论装置系数计算方法，并比较计算方法对江宁台垂向地电阻率观测的影响。

1. 台址概况

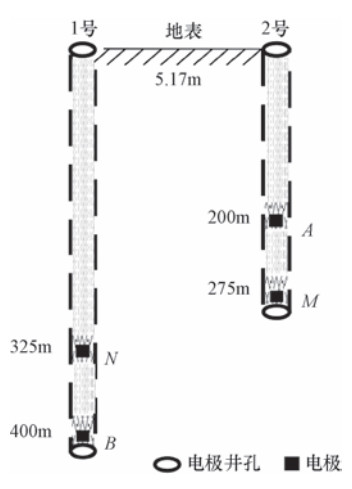
江宁台地处南京市江宁区禄口街道水荆墅村，地形开阔平坦，周围无大中型工矿企业，测区位于南京-湖熟断裂南西盘和方山-小丹阳断裂西盘的楔形地块上，东距茅山断裂带30km，西北距长江36km。测区内现有垂向地电阻率观测装置为在2口深井进行垂向观测的试验装置，井距5.17m，供电电极A、测量电极M分别布设在深275m的2号井内200m和275m处，供电电极B、测量电极N分别布设在深400m的1号井内400m和325m处（图 1）。该垂向观测系统采用ZD8BI型地电仪，根据《地震台站建设规范（地电台站第1部分）》（DB/T.18.1—2006）中关于地电阻率台站的技术要求，对新建垂向观测装置的场地进行高密度电法、电测深等测试。高密度电法探测和电测深报告中NW—SE和NS测线结果表明，观测区域电测深曲线具有K形特征，电性结构等效为3层（樊晓春等，2018），场地电性层参数见表 1。

图 1 江宁台垂向观测电极布极图

Figure 1. The diagram of electrodes deployment

下载: 全尺寸图片幻灯片

表 1 江宁台场地电性层参数

Table 1. The underground electrical structure of Jiangning geoelectric station

NW—SE测线		NS测线
层厚/m	电阻率/Ω·m	层厚/m	电阻率/Ω·m
29.40	39.29	24.56	18.71
220.94	143.06	203.42	274.52
∞	60.39	∞	54.79

下载: 导出CSV

| 显示表格

2. 点电流源在非全空间产生的电场

点电流处于不完全全空间时，对点电流源位于地下和地表 2种情况进行讨论。地下点电流源产生的电场指点电流源的电流I在地下一定深度时流入地下介质中产生的电场，为不完全全空间。假设地下介质电性均匀，介质电阻率为ρ，电流I从地下A点流入（图 2），采用镜像法计算（刘昌谋等，1994；刘国兴，2005），见式（1）。地表点电流源产生的电场指地表点电流源电流I流入地下介质，从无限远处流出时在介质中产生的电场，为半无限空间。假设地下介质电性均匀，介质电阻率为ρ，电流I从地表A点流入地下，电流线的分布以A为中心向周围呈辐射状，该情况为图 2的特例（王兰炜等，2014），见式（2）。

图 2 地下点电源产生的电场示意图

Figure 2. The schematic diagram of the electric field generated by underground point power supply

下载: 全尺寸图片幻灯片

（1）地下点电流源产生的电场（不完全全空间）

$$ {V_{{\rm{MN}}}} = \frac{{\rho I}}{{4{\rm{ \mathsf{ π} }}}}\left({\frac{1}{{\overline {AM} }} + \frac{1}{{\overline {{A_1}M} }} - \frac{1}{{\overline {{A_1}N} }} - \frac{1}{{\overline {AN} }}} \right) $$

(1)

（2）地表点电流源产生的电场（半无限空间）

$$ {V_{{\rm{MN}}}} = \frac{{\rho I}}{{2{\rm{ \mathsf{ π} }}}}\left({\frac{1}{{\overline {AM} }} - \frac{1}{{\overline {AN} }}} \right) $$

(2)

3. 装置系数计算方法

装置系数是地电阻率观测中特有的参数，通常用K表示，与观测装置中电极分布情况有关，表征地电阻率是视电阻率（王兰炜等，2014）。当测区区域介质电阻率均匀分布时，地电阻率ρ_s与介质真实的电阻率ρ数值相同。

根据奥斯定理和镜像原理（钱家栋等，1985），假设测区为均匀介质，垂向观测中的装置系数计算方法如下：

（1）方法Ⅰ：传统垂向观测装置系数计算方法（王兰炜等，2014）

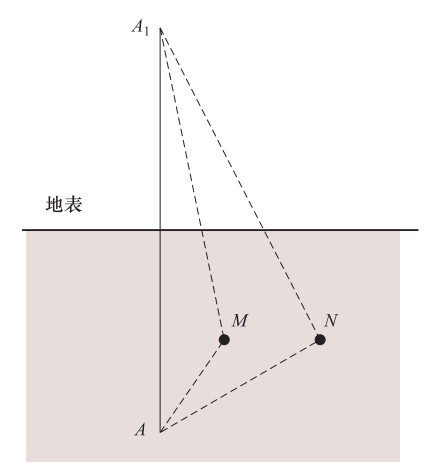
传统方法井下垂向观测装置忽略电极A的埋深，在点电源B与地面对称的位置设镜像点B₁，见图 3（a）。根据式（1）和式（2），则K为：

图 3 江宁台垂向观测示意

Figure 3. The schematic diagram of vertical geo-resistivity observation

下载: 全尺寸图片幻灯片

$$ K = \frac{{4{\rm{ \mathsf{ π} }}}}{{\left({\frac{2}{{\overline {AM} }} - \frac{2}{{\overline {AN} }}} \right) - \left({\frac{1}{{\overline {BM} }} + \frac{1}{{\overline {{B_1}M} }} - \frac{1}{{\overline {{B_1}N} }} - \frac{1}{{\overline {BN} }}} \right)}} $$

(3)

不考虑江宁台垂向观测电极A埋深时，因江宁台垂向观测AM=BN，AN=BM，则：

$$ K = \frac{{4{\rm{ \mathsf{ π} }}}}{{\frac{3}{{\overline {AM} }} - \frac{3}{{\overline {AN} }} - \frac{1}{{\overline {{B_1}M} }} + \frac{1}{{\overline {{B_1}N} }}}} $$

(4)

（2）方法Ⅱ：采用全空间方式的装置系数计算方法（钱家栋等，1985；王兰炜等，2014）

当电极埋深h远大于供电极长度AB时，为全空间，则K为地表观测装置系数的2倍，即：

$$ K = \frac{{4{\rm{ \mathsf{ π} }}}}{{\frac{1}{{\overline {AM} }} - \frac{1}{{\overline {AN} }} - \frac{1}{{\overline {BM} }} + \frac{1}{{\overline {BN} }}}} $$

(5)

（3）方法Ⅲ：采用不完全全空间方式的装置系数计算方法

江宁台垂向观测的电极A、M、N、B分别位于埋深200m、275m、325m、400m处，应按地下点电源产生的电场模型计算（不完全全空间），如图 3（b）所示。在点电源A、B与地面对称的位置设镜像点A₁、B₁，忽略1号井和2号井的水平距离l，根据式（1），则供电电流I（+I和-I）在M、N间产生的电位差为：

$$ {V_{MN}} = \frac{{\rho I}}{{4{\rm{ \mathsf{ π} }}}}\left[ {\left({\frac{1}{{\overline {AM} }} + \frac{1}{{\overline {{A_1}M} }} - \frac{1}{{\overline {{A_1}N} }} - \frac{1}{{\overline {AN} }}} \right) - \left({\frac{1}{{\overline {BM} }} + \frac{1}{{\overline {{B_1}M} }} - \frac{1}{{\overline {{B_1}N} }} - \frac{1}{{\overline {BN} }}} \right)} \right] $$

(6)

则K为：

$$ K = \frac{{4{\rm{ \mathsf{ π} }}}}{{\left({\frac{1}{{\overline {AM} }} + \frac{1}{{\overline {{A_1}M} }} - \frac{1}{{\overline {AN} }} - \frac{1}{{\overline {{A_1}N} }}} \right) - \left({\frac{1}{{\overline {BM} }} + \frac{1}{{\overline {{B_1}M} }} - \frac{1}{{\overline {BN} }} - \frac{1}{{\overline {{B_1}N} }}} \right)}} $$

(7)

因江宁台垂向观测AM=BN，AN=BM，则：

$$ K = \frac{{4{\rm{ \mathsf{ π} }}}}{{\frac{2}{{\overline {AM} }} - \frac{2}{{\overline {AN} }} + \frac{1}{{\overline {{A_1}M} }} - \frac{1}{{\overline {{A_1}N} }} - \frac{1}{{\overline {{B_1}M} }} + \frac{1}{{\overline {{B_1}N} }}}} $$

(8)

（4）方法Ⅳ：采用不完全全空间方式（考虑井距l）的装置系数计算方法

按照地下点电源产生电场的模型计算（不完全全空间），在点电源A、B与地面对称的位置设镜像点A₁、B₁，h₁、h₂、h₃表示供电电极A、B、M的电极埋深，井距l表示2口井孔水平距离（图 3（c）），则：

$$ \overline {AM} = {h_3} - {h_1} $$

(9)

$$ \overline {AN} = \sqrt {{l^2} + {{({h_2} - {h_3})}^2}} $$

(10)

$$ \overline {{A_1}M} = {h_1} + {h_3} $$

(11)

$$ \overline {{A_1}N} = \sqrt {{l^2} + {{({h_2} + 2{h_1} - {h_3})}^2}} $$

(12)

$$ \overline {{B_1}M} = \sqrt {{l^2} + {{({h_2} + {h_3})}^2}} $$

(13)

$$ \overline {{B_1}N} = 2{h_2} - {h_3} + {h_1} $$

(14)

将式（9）至式（14）代入式（8），则K变为：

$$ K = \frac{{4\pi }}{{\frac{2}{{{h_3} - {h_1}}} - \frac{2}{{\sqrt {{l^2} + {{({h_2} - {h_3})}^2}} }} + \frac{1}{{{h_1} + {h_3}}} - \frac{1}{{\sqrt {{l^2} + {{({h_2} + 2{h_1} - {h_3})}^2}} }} - \frac{1}{{\sqrt {{l^2} + {{({h_2} + {h_3})}^2}} }} + \frac{1}{{2{h_2} - {h_3} + {h_1}}}}} $$

(15)

4. 江宁台垂向地电阻率观测装置系数计算

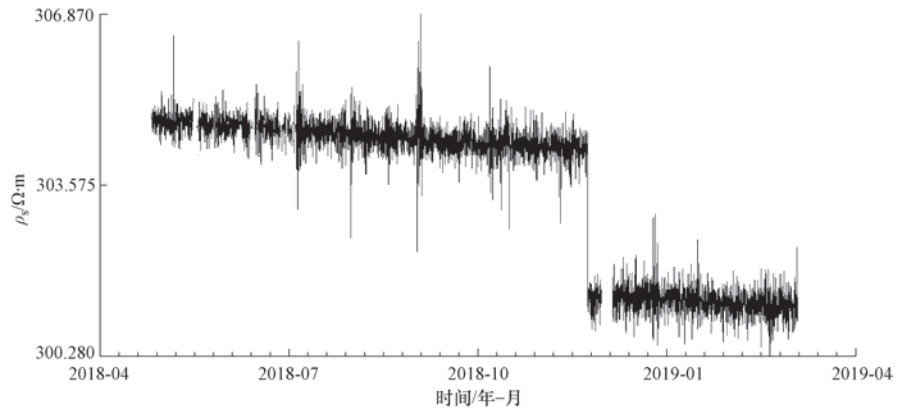
采用方法Ⅰ—Ⅳ分别计算江宁台垂向地电阻率观测的装置系数K，结果见表 2。考虑江宁台垂向地电阻率观测的电极布设不同于传统垂向观测装置，所有电极埋深均在200m以上，本文认为应以方法Ⅳ为参考值，采用式（16）计算不同装置系数计算方法的相对误差。方法Ⅰ、Ⅱ、Ⅲ相对误差分别为-32.01%、1.37%、0.43%，可知方法Ⅰ不适用于江宁台垂向地电阻率观测，该计算方法通常仅适用于供电电极A埋深小于5m的垂向观测，如天水台、合阳台。方法Ⅱ相对误差较小，江宁台垂向观测于2018年4月25日至2018年11月22日曾采用该方法。考虑仅当电极埋深远大于供电极距时称为全空间，而江宁台垂向装置最小电极埋深仅与供电极距相当，因此，方法Ⅱ同样不适用于江宁台垂向观测。除江宁台外，大部分台站观测装置电极埋深明显小于供电极距，均不宜采用方法Ⅱ。方法Ⅲ相对误差最小，江宁台垂向地电阻率观测于2018年11月23日至2019年10月30日曾采用该方法，2018年11月出现的台阶是由调整装置系数导致的（图 4）。由于江宁台垂向地电阻率观测为多孔观测，须考虑井距才能准确计算其装置系数，因而最终采用方法Ⅳ进行计算。

$$ \sigma = \frac{{k_{方法}} - {k_{方法Ⅳ}}}{{{k_{方法Ⅳ}}}} $$

(16)

表 2 江宁台垂向观测的装置系数

Table 2. The configuration coefficient of vertical geo-resistivity observation in Jiangning earthquake station

计算方法	AM/m	AN/m	B₁M/m	B₁N/m	A₁M/m	A₁N/m	装置系数K
Ⅰ	75.000	125.000	675.000	725.000	—	—	790.000
Ⅱ	75.000	125.000	—	—	—	—	1178.000
Ⅲ	75.000	125.000	675.000	725.000	475.000	525.000	1167.000
Ⅳ	75.000	125.374	675.020	725.000	475.000	525.292	1162.000

下载: 导出CSV

| 显示表格

图 4 江宁台垂向观测整点值曲线

Figure 4. The hourly observational value curves of vertical geo-resistivity observation at Jiangning Seismic Station

下载: 全尺寸图片幻灯片

5. 结论

本文以江宁台垂向地电阻率观测为例，提出2种以不完全全空间方式计算的新方法，并与现有垂向观测装置计算方法进行比较。研究结果表明，方法Ⅳ最符合江宁台垂向地电阻率观测装置。考虑方法Ⅳ中部分参数存在小数部分，认为保留小数点后三位能满足装置系数精度要求。方法Ⅳ除适用于2口井垂向观测装置外，同样适用于忽略井距时供电电极A埋深超过5m的单口井垂向观测装置。

致谢: 衷心感谢中国地震局地壳应力研究所王兰炜研究员对本文提出的建议和意见。

图 1 W4试件尺寸（单位：毫米）

Figure 1. W4 specimen size（Unit：mm）

下载: 全尺寸图片幻灯片

图 2 夯土墙试件

Figure 2. Rammed soil wall specimens

下载: 全尺寸图片幻灯片

图 3 试验装置及加载制度

Figure 3. Test device and loading diagram

下载: 全尺寸图片幻灯片

图 4 各试件破坏图

Figure 4. Failure diagram of each specimen

下载: 全尺寸图片幻灯片

图 5 各试件滞回曲线

Figure 5. Hysteresis curves of each specimen

下载: 全尺寸图片幻灯片

图 6 各试件骨架曲线图

Figure 6. Skeleton curves of specimens

下载: 全尺寸图片幻灯片

图 7 各试件刚度退化曲线

Figure 7. Stiffness degradation curves of specimens

下载: 全尺寸图片幻灯片

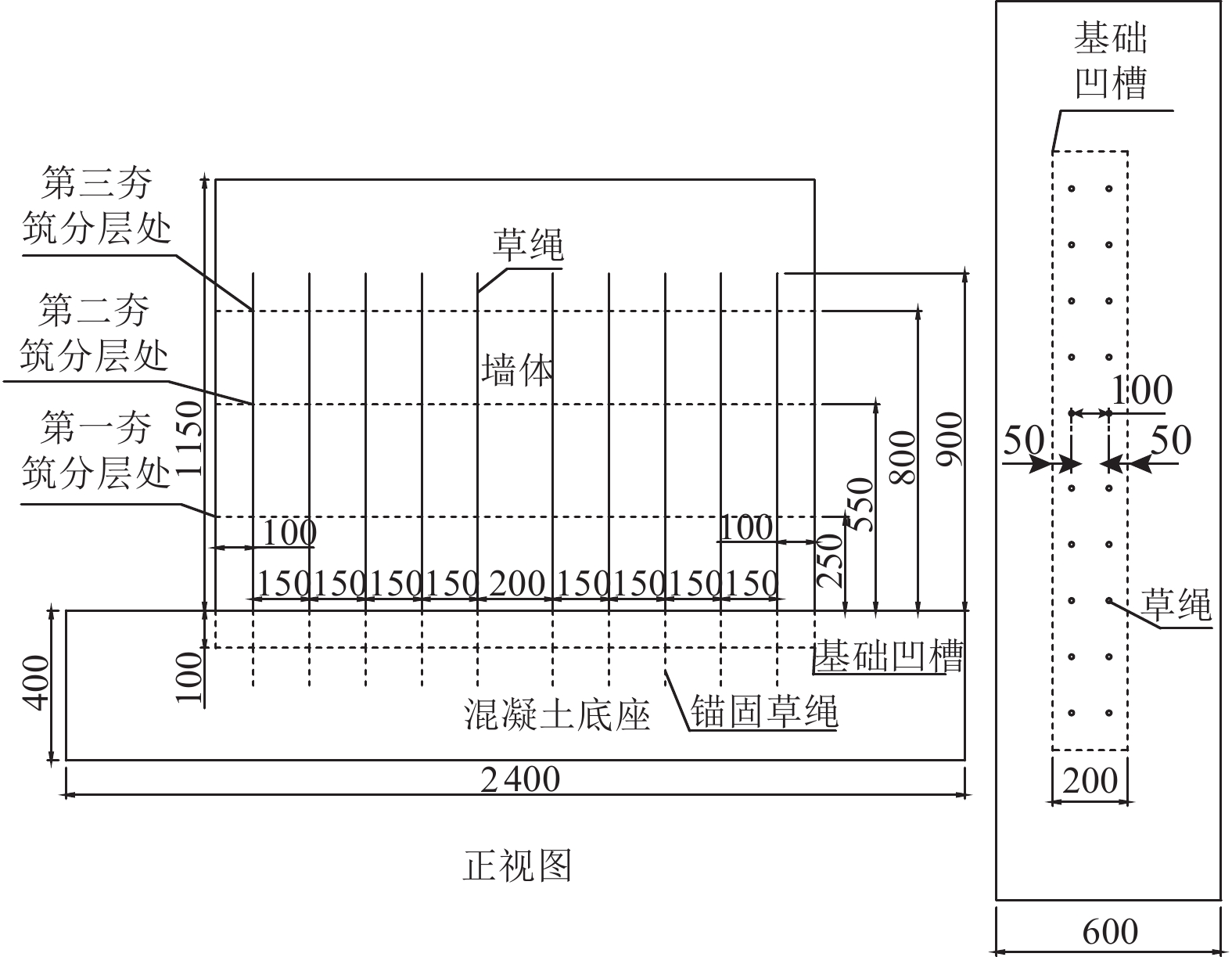
表 1 试件方案设计表

Table 1. Sample scheme design table

试件编号	生土掺料	草绳高度/mm	草绳/ 墙高
W1	0.8%麦秸秆	无	无
W2	0.8%麦秸秆	300	1/4
W3	0.8%麦秸秆	600	1/2
W4	0.8%麦秸秆	900	3/4

下载: 导出CSV

表 2 各试件特征点处力学性能参数

Table 2. Mechanical property parameters at characteristic points of each specimen

试件编号	屈服位移 /mm	屈服荷载 /kN	峰值位移 /mm	峰值荷载 /kN	极限位移 /mm	极限荷载 /kN	延性系数
W1	3.73	9.20	5.02	10.10	9.50	8.40	2.27
W2	3.90	13.80	7.90	17.70	11.10	11.20	2.80
W3	3.88	17.37	7.04	19.51	12.76	16.42	3.28
W4	3.92	17.40	6.12	21.04	12.89	17.58	3.28

下载: 导出CSV

表 3 特征点处等效黏滞阻尼系数

Table 3. Equivalent viscous damping coefficient at characteristic points

试件编号	开裂荷载阻尼系数	屈服荷载阻尼系数	极限荷载阻尼系数	破坏荷载阻尼系数
W1	0.0744	0.0865	0.0982	0.1145
W2	0.0811	0.1125	0.1410	0.1687
W3	0.0902	0.1236	0.1665	0.2058
W4	0.0948	0.1358	0.1796	0.2243

下载: 导出CSV

参考文献(22)

陈忠范, 郑怡, 沈小俊等, 2012. 村镇生土结构建筑抗震技术手册. 南京: 东南大学出版社.

和法国, 吕燃, 粟华忠等, 2019. SH材料加固夯筑遗址土耐久性试验及机理研究. 岩土力学, 40（S1）: 297—307

He F. G. , Lü R. , Su H. Z. , et al. , 2019. Durability test and reinforced mechanism on adding SH materials into soil of archaeological sites. Rock and Soil Mechanics, 40(S1): 297—307. (in Chinese)

李志华, 周明卿, 陈伟康等, 2016. 加筋夯土墙片的平面内抗剪性能试验. 中南大学学报（自然科学版）, 47（8）: 2835—2841

Li Z. H. , Zhou M. Q. , Chen W. K. , et al. , 2016. Experimental investigation on in-plane shear behavior of reinforced rammed earth panels. Journal of Central South University (Science and Technology), 47(8): 2835—2841. (in Chinese)

蔺广涵, 叶洪东, 2018. 棉花秸秆改性生土材料试验研究. 天津城建大学学报, 24（3）: 196—199 doi: 10.19479/j.2095-719x.1803196

Lin G. H. , Ye H. D. , 2018. Experimental research on raw soil materials modified by cotton straw. Journal of Tianjin Chengjian University, 24(3): 196—199. (in Chinese) doi: 10.19479/j.2095-719x.1803196

刘蕾, 姚勇, 张玲玲, 2021. 改性夯土墙材的强度影响因素相关性研究. 硅酸盐通报, 40（4）: 1286—1295 doi: 10.16552/j.cnki.issn1001-1625.2021.04.027

Liu L. , Yao Y. , Zhang L. L. , 2021. Correlation of strength influencing factors of modified rammed earth wall materials. Bulletin of the Chinese Ceramic Society, 40(4): 1286—1295. (in Chinese) doi: 10.16552/j.cnki.issn1001-1625.2021.04.027

刘强, 童丽萍, 许启铿, 2018. 带竖向销键夯土墙抗震性能试验. 建筑科学与工程学报, 35（3）: 79—86

Liu Q. , Tong L. P. , Xu Q. K. , 2018. Experiment on seismic performance of rammed earth wall with vertical pin. Journal of Architecture and Civil Engineering, 35(3): 79—86. (in Chinese)

卢家成, 刘嘉良, 李威翰等, 2020. 生土建筑与材料研究现状. 材料导报, 34（S2）: 269—272

Lu J. C. , Liu J. L. , Li W. H. , et al. , 2020. Research status of raw soil building and materials. Materials Reports, 34(S2): 269—272. (in Chinese)

王兰民, 王强, 2011. 西北地区农村民房现状及抗震技术研究. 华南地震, 31（4）: 14—22 doi: 10.13512/j.hndz.2011.04.001

Wang L. M. , Wang Q. , 2011. Study on status and anti-seismic technology of rural houses in Northwest China. South China Journal of Seismology, 31(4): 14—22. (in Chinese) doi: 10.13512/j.hndz.2011.04.001

王毅红, 梁楗, 张项英等, 2015. 我国生土结构研究综述. 土木工程学报, 48（5）: 98—107

Wang Y. H. , Liang J. , Zhang X. Y. , et al. , 2015. Review of raw-soil structure in China. China Civil Engineering Journal, 48(5): 98—107. (in Chinese)

王赟, 冯映雪, 张波等, 2021. 竹片网水泥砂浆加固承重夯土墙体抗震试验研究. 世界地震工程, 37（3）: 104—110

Wang Y. , Feng Y. X. , Zhang B. , et al. , 2021. Experimental study on seismic performance of bamboo mesh and cement mortar reinforced rammed earth wall. World Earthquake Engineering, 37(3): 104—110. (in Chinese)

徐舜华, 王兰民, 王强等, 2011. 甘肃武都典型夯土民房承重墙体抗剪强度试验研究. 西北地震学报, 33（4）: 354—358, 385

Xu S. H. , Wang L. M. , Wang Q. , et al. , 2011. Experimental study on shearing strength of typical rammed earth wall in Wudu area, Gansu Province. Northwestern Seismological Journal, 33(4): 354—358, 385. (in Chinese)

张琰鑫, 童丽萍, 2012. 夯土住宅结构性能分析及加固方法. 世界地震工程, 28（2）: 72—78

Zhang Y. X. , Tong L. P. , 2012. Performance analysis and strengthening method of rammed earth buildings. World Earthquake Engineering, 28(2): 72—78. (in Chinese)

张又超, 王毅红, 张项英等, 2015. 单面钢丝网水泥砂浆加固承重夯土墙体抗震试验研究. 西安建筑科技大学学报（自然科学版）, 47（2）: 255—259

Zhang Y. C. , Wang Y. H. , Zhang X. Y. , et al. , 2015. Experimental study on strengthening rammed-earth wall with single side ferro-cement surface layer. Journal of Xi'an University of Architecture & Technology (Natural Science Edition), 47(2): 255—259. (in Chinese)

中华人民共和国住房和城乡建设部, 2008. JGJ 161—2008 镇（乡）村建筑抗震技术规程. 北京: 中国建筑工业出版社.

Ministry of Housing and Urban-Rural Development of the People's Republic of China, 2008. JGJ 161—2008 Seismic technical specification for building construction in town and village. Beijing: China Architecture & Building Press. (in Chinese)

中华人民共和国住房和城乡建设部, 2015. JGJ/T 101—2015 建筑抗震试验规程. 北京: 中国建筑工业出版社.

Ministry of Housing and Urban-Rural Development of the People's Republic of China, 2015. JGJ/T 101—2015 Specification for seismic test of buildings. Beijing: China Architecture & Building Press. (in Chinese)

周铁钢, 段文强, 穆钧等, 2013. 全国生土农房现状调查与抗震性能统计分析. 西安建筑科技大学学报（自然科学版）, 45（4）: 487—492

Zhou T. G. , Duan W. Q. , Mu J. , et al. , 2013 a. Statistical analysis and survey on the aseismatic performance of theraw-soil building status in China's rural areas. Journal of Xi'an University of Architecture & Technology (Natural Science Edition), 45(4): 487—492. (in Chinese)

Arslan M. E. , Emiroğlu M. , Yalama A. , 2017. Structural behavior of rammed earth walls under lateral cyclic loading: a comparative experimental study. Construction and Building Materials, 133: 433—442. doi: 10.1016/j.conbuildmat.2016.12.093

ASTM, 2007. ASTM E2126—07 a Standard test methods for cyclic (reversed) load test for shear resistance of vertical elements of the lateral force resisting systems for buildings. West Conshohocken: ASTM International.

Jové-Sandoval F. , Barbero-Barrera M. M. , Flores Medina N. , 2018. Assessment of the mechanical performance of three varieties of pine needles as natural reinforcement of adobe. Construction and Building Materials, 187: 205—213. doi: 10.1016/j.conbuildmat.2018.07.187

Karanikoloudis G. , Lourenço P. B. , 2018. Structural assessment and seismic vulnerability of earthen historic structures. Application of sophisticated numerical and simple analytical models. Engineering Structures, 160: 488—509.

Miccoli L. , Müller U. , Pospíšil S. , 2017. Rammed earth walls strengthened with polyester fabric strips: experimental analysis under in-plane cyclic loading. Construction and Building Materials, 149: 29—36. doi: 10.1016/j.conbuildmat.2017.05.115

Prabakar J. , Sridhar R. S. , 2002. Effect of random inclusion of sisal fibre on strength behaviour of soil. Construction and Building Materials, 16(2): 123—131. doi: 10.1016/S0950-0618(02)00008-9

施引文献

资源附件(0)

访问统计