Influence of PGV and PGD on Structural Nonlinear Seismic Response of A 6-story Steel Building
-
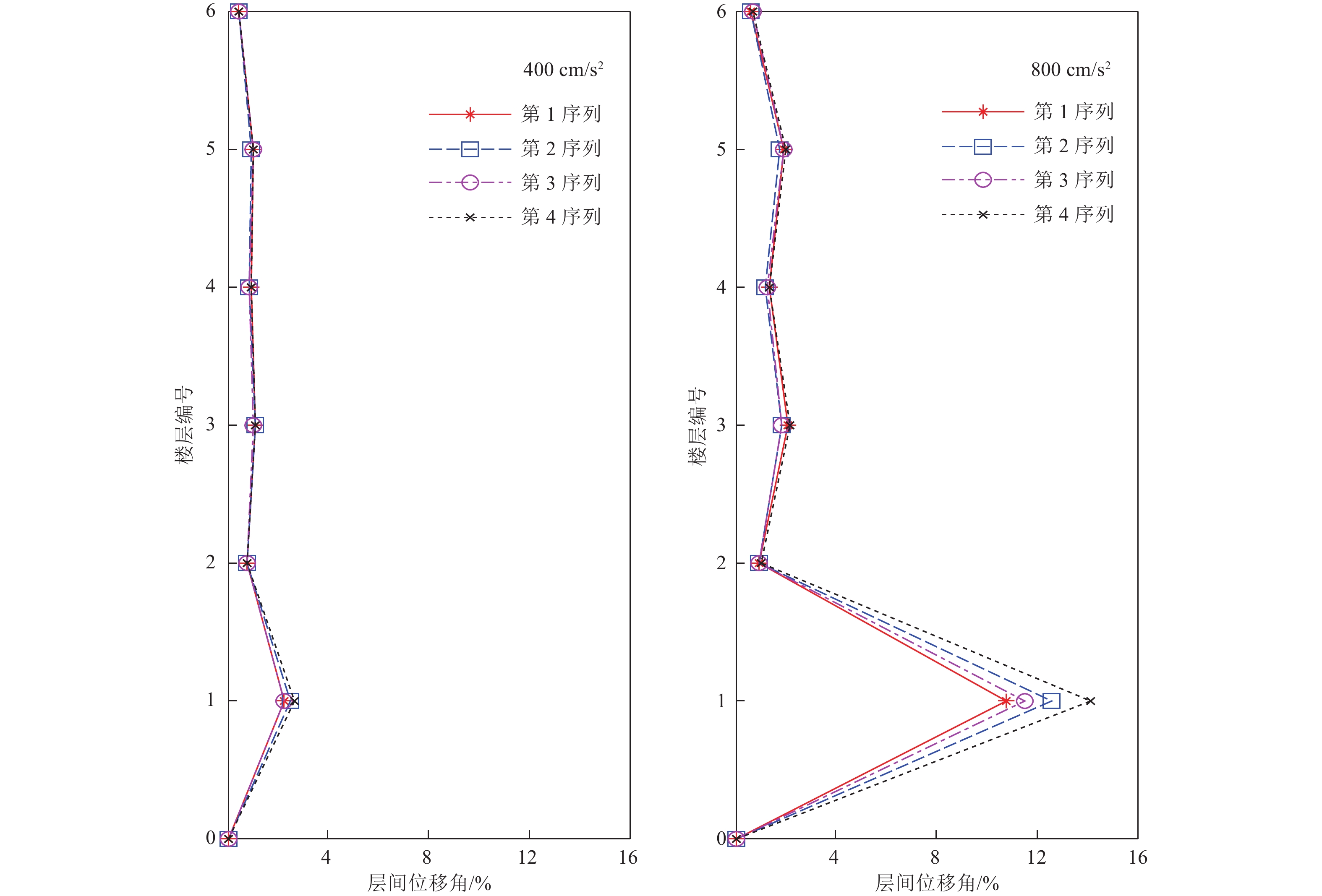
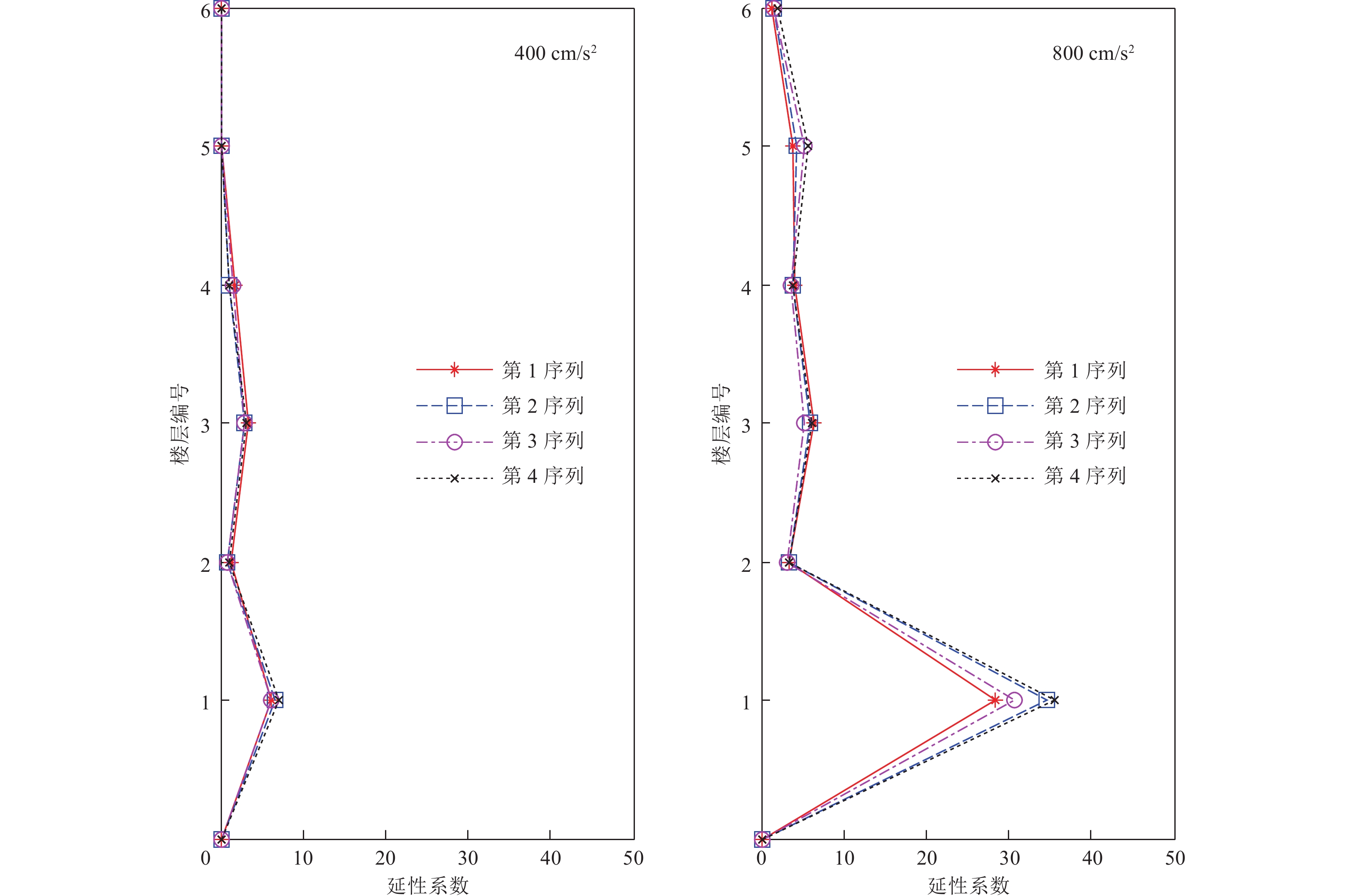
摘要: 深入揭示地震动峰值特性影响是推进地震动工程特性研究的有效手段。地震动峰值速度和峰值位移特性对结构弹塑性地震反应的影响规律尚需要探索。本文基于窄带时程叠加方法，人工合成具有相同加速度反应谱但峰值速度和峰值位移不同的4个序列地震动时程。其中第1、2序列地震动峰值速度为0.20 m/s，峰值位移分别为0.20 dm和0.40 dm，而第3、4序列地震动峰值位移为0.30 dm，峰值速度分别为0.15 m/s和0.30 m/s。将地震动峰值加速度分别标定至400 cm/s2和800 cm/s2，并以此作为输入开展建设地震观测系统的6层钢结构弹塑性地震反应分析，使得结构发生不同弹塑性地震反应，对比分析在不同序列地震动作用下层间位移角和延性系数等结构工程需求参数差别，探索峰值位移和峰值速度对结构弹塑性地震反应的影响规律。分析表明，在非线性反应阶段后，结构层间位移角和延性系数的变异系数随着输入地震动峰值的增加而增大，地震动峰值特性对结构层间位移角和延性系数等参数有一定影响，影响幅度随输入地震动增加而增大，且峰值速度较峰值位移的影响更为显著。在进行结构设计地震动参数选取时，应重视地震动速度和位移峰值特性的影响。Abstract: Revealing the influences of amplitude of strong motions is an effective method to push forward the earthquake engineering research. Influence pattern to structural inelastic response of the peak velocity and peak displacement is required to carry out. Based on a specific acceleration response spectrum, 4 sets of artificial ground motions are synthesized with 30 samples. The peak accelerations of the 1st and 2nd sets are identical with value of 1 m/s2 and their peak velocities are similarly the same with the value of 0.20 m/s. Their peak displacements differ from each other, with the values at 0.2 dm for the 1st set and 0.4 dm for the 2nd set. The peak accelerations and peak displacements are consistent with the peak velocity of 0.15 m/s for the 3rd set and 0.30 m/s for the 4th set. The ground motions are separately scaled to 400cm/s2 and 800cm/s2 and they are introduced as the input motions to a 6-story steel building to render certain different degree of non-linear seismic response. Engineering demand parameters including inter-story drift ratio and ductility coefficient are compared for the response under different sets of ground motions. It is inferred from the comparsions that the influence appears a tendency to increase with the amplitude of input ground motions. Analyses also indicate peak velocities have large influences on nonlinear responses and the influence of peak displacement is relatively weaker. Therefore, when ground motions are selected for structural seismic design, it is not appropriate to merely consider spectral characteristics, ignoring the influence of amplitude characteristics.
-
引言
镇江地区历史上发生过多次中强震，地震预警系统的建设有一定意义，2015年12月镇江市地震预警系统建成并投入使用，该系统分为2个主要部分，即地震波接收台点（下文称烈度台点）、预警信号发出台点（下文称预警终端）。但该系统建成后，镇江地区未发生过中强震，有感地震震级也较小，仅在很小的范围产生影响，预警系统并没有产生一定作用，因此该系统使用方（目前主要是学校师生）对预警系统的实际应用效果并不了解，地震部门对预警终端的增补也缺少相应的依据。为了解决这些问题，本文根据镇江地区预警台点分布情况及地震动峰值加速度和地震烈度衰减关系，从理论上对该系统的预警效能进行分析，确定目前本市地震预警系统的布点合理性及不同地点布设的预警终端对本市地震的理论预警时间。
1. 地震预警系统
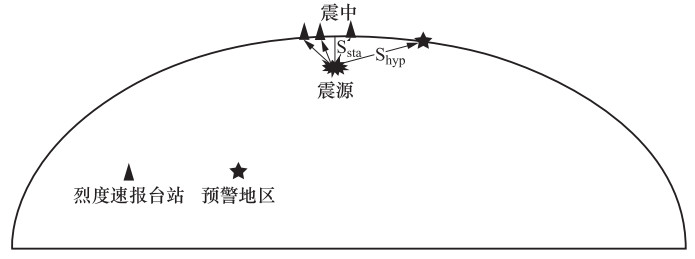
地震预警指地震发生后，对震中附近一定距离区域尚未感知到但即将有可能感知到地震的民众发布的警报。地震波从地震发生地（震源）传播到周边一定范围，根据距离远近需要几秒到几十秒，如果在地震发生地布设地震探测仪器，地震发生时仪器最先接收纵波，经过短暂时间，经计算机自动处理，估算出地震三要素，通过电磁波信号以比横波更快的速度（3×105km/s）发送给离震中一定范围外的民众，达到地震预警目的。
地震预警系统虽能发挥一些社会效用，但仍存在一些亟待解决的问题，主要包含技术层面与非技术层面的问题。技术层面主要为预警信号发出区离震中太近存在盲区，无法预警，而离太远发出的预警信号无实际意义。本文主要分析技术层面的问题，同时结合镇江地区地震背景和各台点建设情况，分析各预警终端有效预警时间。
地震预警时间计算方法可用下式表示（郭凯等，2016）：
$$T_{\mathrm{war}}=\frac{S_{\mathrm{hyp}}}{v_{\mathrm{s}}}-\frac{S_{\mathrm{sta}}}{v_{\mathrm{p}}}-T_{\mathrm{dat}}-T_{\mathrm{cen}}-T_{\mathrm{iss}} $$ (1) 式中，Twar为地震预警时间，Shyp为震源与目标区域的距离，Ssta为震源到台站的距离（假设3个台触发阈值才发出预警信息），vs为S波波速，vP为P波波速，Tdat为预警系统计算所需P波数据时长，Tcen为数据传输到数据处理中心的时延和系统计算时间之和；Tiss为预警信息发布时间，震源视为点源。
纵波速度快，离台站最近，最先到达烈度台点，而破坏性较大的横波速度慢，离预警地区较远，较迟到达预警地区（图 1）。
当地震预警时间Twar＞0时，预警有效；当Twar≤0时，预警无效，根据式（1）可以计算出预警盲区，在震中附近一定区域无法预警。
但如果距震中过远，地震波到达时造成的地震烈度等级较低，有的甚至无法为人所感知，这种预警无实际意义。
2. 镇江市及周边地区地震预警系统建设情况
目前，镇江地区共有烈度台点17个，预警终端8个。烈度台点装有烈度仪，仪器感知地震动时向数据处理中心发出地震动信号，但该台站并不对外发出预警信号，预警信号主要由预警终端发出，该终端配备2-3个60w的喇叭，当接收到预警信号时，通过喇叭向使用者发出报警。为便于维护和管理，烈度仪安装在各辖市区原有地震台、强震台、宏观观测点及各乡镇镇政府内。预警终端在各辖市区选择1-2所学校布设，测试使用。
3. 预警盲区与台点间距
假设需3个台点触发阈值，计算机自动处理并开始计算地震三要素，最好的情况是地震发生地点在3个台构成的三角形中心下方。因研究区域较小，将地表近似视为平面，假设台点间距相同，3个台点构成等边三角形，则：
$$S_{\mathrm{sta}}^{2}=h^{2}+\frac{1}{3} a_{0}^{2} $$ (2) 式中，a0为台点间距，h为震源深度。
根据式（1），当Twar≤0时无法预警，设Twar=0，则式（1）变为：
$$S_{\mathrm{hyp}}=v_{\mathrm{s}}\left(\frac{S_{\mathrm{sta}}}{v_{\mathrm{p}}}+T_{\mathrm{dat}}+T_{\mathrm{cen}}+T_{\mathrm{iss}}\right) $$ (3) 各参数可赋值为：Tdat=3s，Tcen=2s，Tiss=1s，vP=5.8km/s，vs=3.6km/s。
设震中距为Δepi，则：
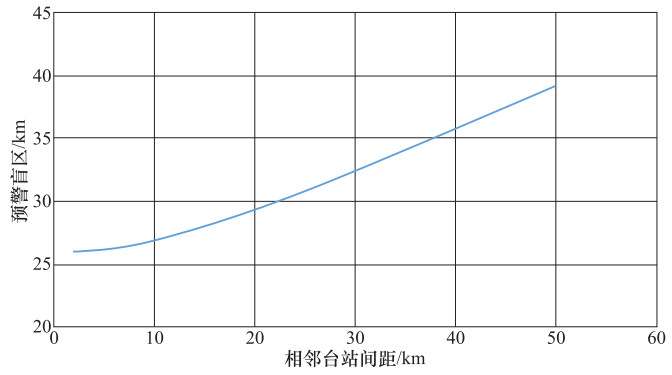
$$\mathit{\Delta }_{\text {epi }}=\sqrt{S_{\text {hyp }}^{2}-h^{2}} $$ (4) 可计算出不同台网密度（台点间距）对应的预警盲区（震中距），计算结果如图 2所示。
图 2 台点间距与预警盲区关系曲线Figure 2. Relation curves between station density and warning blind area计算得出台点间距达1km时，预警盲区为25.96km，随台点间距增大，盲区扩大。
根据镇江市烈度台点分布，台点间距近似用下式计算：
$$a_{0}=\frac{a_{12}+a_{22}+, \ldots, +a_{172}}{34} $$ (5) 其中，a12+a+2+, …, +a172为各台到最近2个台点的距离和。
台点间距离计算方法如下：
设两点经纬度分别为A（WA，JA）、B（WB，JB），根据大地坐标系与直角坐标系换算关系（孔祥元等，2001），A点直角坐标为：
$$\left\{\begin{array}{l} X_{\mathrm{A}}=\frac{a \cos W_{\mathrm{A}}}{W} \cos J_{\mathrm{A}} \\ Y_{\mathrm{A}}=\frac{a \cos W_{\mathrm{A}}}{W} \sin J_{\mathrm{A}} \\ Z_{\mathrm{A}}=\frac{b \sin W_{\mathrm{A}}}{V} \end{array}\right. $$ (6) B点直角坐标为：
$$\left\{\begin{array}{l} X_{\mathrm{B}}=\frac{a \cos W_{\mathrm{B}}}{W} \cos J_{\mathrm{B}} \\ Y_{\mathrm{B}}=\frac{a \cos W_{\mathrm{B}}}{W} \sin J_{\mathrm{B}} \\ Z_{\mathrm{B}}=\frac{b \sin W_{\mathrm{B}}}{V} \end{array}\right. $$ (7) 式中，a为椭圆长半轴，b为椭圆短半轴，W、V为含椭圆偏心率的辅助函数。
为简化计算，将地球考虑为正球体而非椭球体，W、V取值为1，式（1）、式（2）中a、b则为地球半径，因a、b仅参与弧度计算，可将a、b假设为1。
AB间弧长即地面AB两点间的球面距离△AB：
$${\mathit{\Delta }_{{\rm{AB}}}} = {\rm{R}}C = {\rm{R}} \times {\mathop{\rm acos}\nolimits} \left({\frac{{a_1^2 + b_1^2 - c_1^2}}{{2{a_1}{b_1}}}} \right) $$ (8) 式中，C为OA与OB直线间夹角（弧度），R为地球半径，a1为A点到球心的距离，b1为B点到球心的距离，c1为A、B点直线距离。
$$c_{1}^{2}=\left(X_{\mathrm{A}}-X_{\mathrm{B}}\right)^{2}+\left(Y_{\mathrm{A}}-Y_{\mathrm{B}}\right)^{2}+\left(Z_{\mathrm{A}}-Z_{\mathrm{B}}\right)^{2} $$ (9) 地球半径R取6371.004km。计算得出镇江市烈度台点平均台点间距a0为16.3km，预警盲区为28.4km，即预警系统对震中28.4km范围内的地区无法预警。如果台点间距缩至10km，盲区范围为震中27km范围内，盲区面积缩小10%，缩小范围较小。总体说来，镇江市烈度速报台站台点间距较合理。
4. 预警有效范围
当预警地区地震动峰值加速度或地震烈度达到一定程度时，认为地震预警有效果。根据《中国地震烈度表》（GB/T 17742-2008）（中华人民共和国国家质量监督检验检疫总局等，2009），地震动峰值加速度达22cm/s2或地震烈度达Ⅴ度时，室内绝大多数人有感，房屋颤动，不稳定器物摇动或翻倒，因东部沿海地区民众对地震关注度不高，容忍程度较低，地震烈度为Ⅴ度时预警已有一定意义。根据东部地区地震动峰值加速度（PGA）衰减关系（王国新等，2014）和中强地震活动区地震烈度衰减关系（卢建旗等，2009），可计算不同震级时地震动峰值加速度和地震烈度与震中距的关系如下：
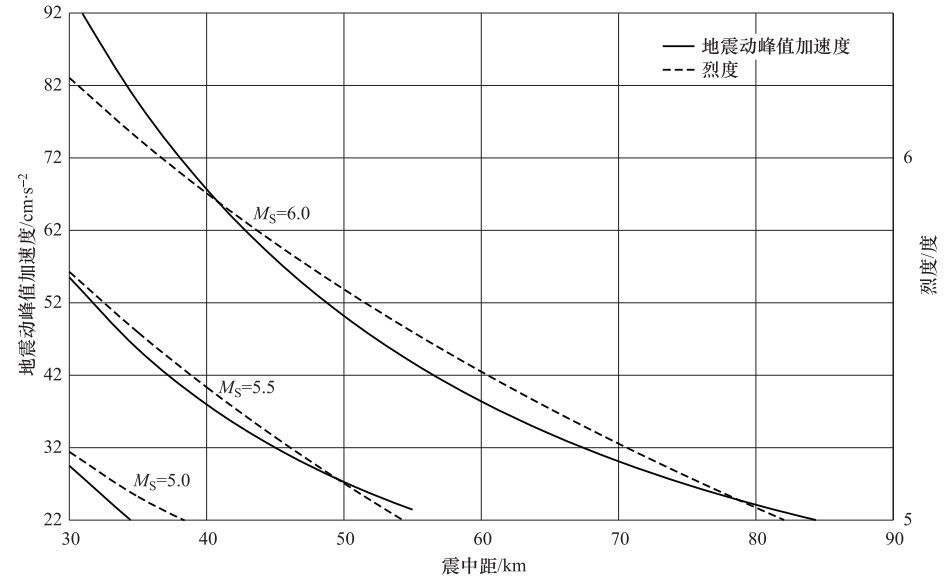
$$\ln \left(Y_{1}\right)=5.304+1.7196 M_{S}-2.5903 \ln \left(L+2.789 \mathrm{e}^{0.451 M_{\mathrm{S}}}\right) $$ (10) $$\ln \left(Y_{2}\right)=2.726+1.3467 M_{S}-1.7636 \ln \left(L+1.046 \mathrm{e}^{0.451 M_{\mathrm{S}}}\right) $$ (11) $$I_{\mathrm{a}}=5.841+1.071 M_{S}-3.657 \log (L+15) $$ (12) $$I_{\mathrm{b}}=3.944+1.071 M_{S}-2.845 \log (L+7) $$ (13) 其中，Y1、Y2分别为长、短轴地震动峰值加速度，Ia、Ib分别为长、短轴地震烈度，MS为震级，L为震中距（km）。
发生MS5.0以下地震时，在前文所述的28.4km盲区外，地震烈度达不到Ⅴ度，地震动峰值加速度达不到22cm/s2，对预警来说意义不大。因此根据长轴公式（10）、（12）绘制MS5.0、MS5.5、MS6.0地震时地震动峰值加速度、地震烈度和震中距关系曲线图（图 3）。由图 3可知，震中距越大，地震动峰值加速度越小，地震烈度越小；当发生MS5.0地震时，震中距38km内地震动峰值加速度可达22cm/s2，震中距34km内地震烈度达Ⅴ度；当发生MS5.5地震时，震中距57km内地震动峰值加速度可达22cm/s2，震中距54km内地震烈度达Ⅴ度；当发生MS6.0地震时，震中距84km内地震动峰值加速度可达22cm/s2，震中距82km内地震烈度达Ⅴ度。因此，本预警系统对于MS5.0地震预警有效范围为34-38km，对于MS5.5地震预警有效范围为54-57km，对于MS6.0地震预警有效范围为82-84km。
图 3 地震动峰值加速度和地震烈度衰减关系曲线Figure 3. Curves of seismic peak acceleration and seismic intensity attenuation laws with various magnitudes5. 镇江市地震预警系统预警有效性分析
镇江市处于华北地震区长江下游-黄海地震带，属中强地震活动带。根据《中国历史强震目录（公元前23世纪-公元1911年）》（国家地震局震害防御司，1995）、《中国近代地震目录（公元1912年-1990年，MS≥4.7）》（中国地震局震害防御司，1999），镇江行政区域内共发生4次破坏性地震（MS≥4.7)，分别为1630年2月4日江苏镇江句容$4 \frac{3}{4} $级地震、1872年7月24日江苏镇江西$4 \frac{3}{4}$级地震、1913年4月3日江苏镇江MS5.5地震、1930年1月3日江苏镇江MS5.5地震。因此，镇江地区存在发生MS5.0以上地震的背景。
根据1970年以来的中国台网地震目录，1970年至今，镇江市虽未发生过MS5.0以上地震，但在距镇江行政边界90km范围内，发生过2次MS5.0以上地震（1974年4月22日溧阳MS5.5地震、1979年7月9日溧阳MS6.0地震）、2次接近MS5.0地震（1979年7月11日溧阳MS4.7地震、2012年7月20日高邮MS4.9地震）。因此，在镇江及周边地区建设地震预警系统具有一定意义。
计算有意义的最大预警时间时，假设地震发生在距各预警终端最远的3个烈度台点中心点，计算步骤如下：
（1）根据各烈度台点经纬度得出每3个烈度台点中心点经纬度，再根据各预警终端经纬度求出各预警终端与各中心点的距离，可假设离各预警终端最远的中心点为震中，计算出震中与预警终端的震中距Δepi，根据震源深度h（本文取10km）计算出震源距Shyp；
（2）假设地震P波到达离其最近的第3个台站时开始进行数据处理，则根据震中经纬度（3个烈度台点中心点经纬度）和3个烈度台点经纬度，求得震中与烈度台点的距离，取其最大值，即为震中距Δsta，再根据震源深度h（本文取10km）计算出震源距Ssta；
（3）根据公式（1）计算出最大预警时间Tmax。
（4）引入前述预警有效范围，当地震发生时，如果Δepi超过该震级对应的最大预警有效范围ΔMS，则Δepi取值为该震级对应的最大预警有效范围ΔMS，Ssta可根据公式（2）取本市平均台点间距近似计算，再根据公式（1）计算出有意义的最大预警时间Tmea-MS。如果Δepi＞ΔMS，则有意义的最大预警时间Tmea-MS=Tmax。
计算结果见表 1。
表 1 各地震预警终端有效预警时间Table 1. Effective earthquake early warning time of the earthquake early warning terminals预警终端 Δsta3/km Δepi/km Tmea-Ms5.0/s Tmea-Ms5.5/s Tmea-Ms6.0/s 镇江市地震台 17.3 49.2 2.5 4.5 4.5 镇江实验学校万科魅力之城分校 17.3 53.5 2.5 5.7 5.7 江滨实验小学 17.3 57.8 2.5 7.7 6.9 辛丰小学 17.3 55.8 2.5 6.3 6.3 丹阳华南实验学校 17.3 46.8 2.5 3.9 3.9 扬中市外国语小学 17.3 80.8 2.5 7.7 13.1 句容实验小学 16.0 61.8 2.5 7.7 8.1 镇江新区实验小学 17.3 68.7 2.5 7.7 9.8 根据计算结果，当镇江市发生MS5.0地震时，因各地震预警终端最远震中距在该震级预警有效范围外，因此计算得出的有效预警时间相同，即可提前0-2.5s预警本地地震烈度在Ⅴ度或地震动峰值加速度达22cm/s2的地震；当镇江市发生MS5.5地震时，4个预警终端最远震中距大于震级预警有效范围，可提前0-7.7s预警本地地震烈度在Ⅴ度或地震动峰值加速度达22cm/s2的地震，另外4个预警终端最多可提前3.9-6.3s预警本地地震烈度在Ⅴ度或地震动峰值加速度达22cm/s2的地震；当镇江市发生MS6.0地震时，各预警终端最远震中距均在该震级预警有效范围内，最多可提前3.9-13.1s预警本地烈度在Ⅴ度或地震动峰值加速度达22cm/s2的地震。
6. 结论
（1）根据三台触发最优结果为地震发生在三台中心点的原则，台点间距为1km时，预警盲区为25.96km，且预警盲区随台点间距的增大而增大。根据镇江市各烈度台点至最近的2个烈度台点的距离，计算得出镇江市地震烈度台点平均台点间距为16.3km，预警盲区为28.4km。
（2）根据地震动峰值加速度和地震烈度随震中距的衰减关系，计算得出发生不同震级地震时地震动峰值加速度和地震烈度与震中距的关系。为使当地地震预警具有一定意义，本文假设当地地震动峰值加速度达22cm/s2或地震烈度达Ⅴ度时预警有效，计算知：发生MS5.0以下地震时，在28.4km盲区外，地震烈度达不到Ⅴ度，地震动峰值加速度达不到22cm/s2，对预警来说意义不大；当发生MS5.0地震时，震中距38km内地震动峰值加速度能达22cm/s2，震中距34km地震内烈度达Ⅴ度；当发生MS5.5地震时，震中距57km内地震动峰值加速度能达22cm/s2，震中距54km内地震烈度达Ⅴ度；当发生MS6.0地震时，震中距84km内地震动峰值加速度能达22cm/s2，震中距82km内地震烈度达Ⅴ度。
（3）根据各烈度台点和预警终端经纬度，再引入预警有效范围，可计算得到发生不同震级地震时，不同地点预警终端最多可提前2.5-13.1s预警本地地震烈度为Ⅴ度或地震动峰值加速度达22cm/s2的地震。
-
图 3 6层钢结构地震反应观测系统传感器布设位置
Figure 3. The sensor location of the structural seismic response observation system in the 6-story steel building
图 4 模态分析计算出的结构前9阶自振振型
Figure 4. The first 9 vibration modes of the building identified from modal analysis
图 5 基于自振频率的瑞雷阻尼比计算
Figure 5. Rayleigh damping ratio based on the first 9 natural frequencies
图 6 结构数值模拟结果与观测结果的加速度时程对比
Figure 6. Comparison of acceleration time history between simulated results and observation results
图 7 结构数值模拟结果与观测结果的位移反应对比
Figure 7. Comparison of displacement time history between simulated results and observation results
图 8 地震动输入为400 cm/s2时4个序列地震动作用下的结构层间位移角分布
Figure 8. Inter-story drift distributions for four sets of ground motions when the input acceleration amplitude is 400 cm/s2
图 9 不同地震动输入下的结构层间位移角平均值
Figure 9. Average inter-story drift ratio for four sets of ground motions with different input acceleration amplitudes
图 10 不同地震动输入下的结构延性系数平均值
Figure 10. Average ductility coefficient for four sets of ground motions with different input acceleration amplitudes
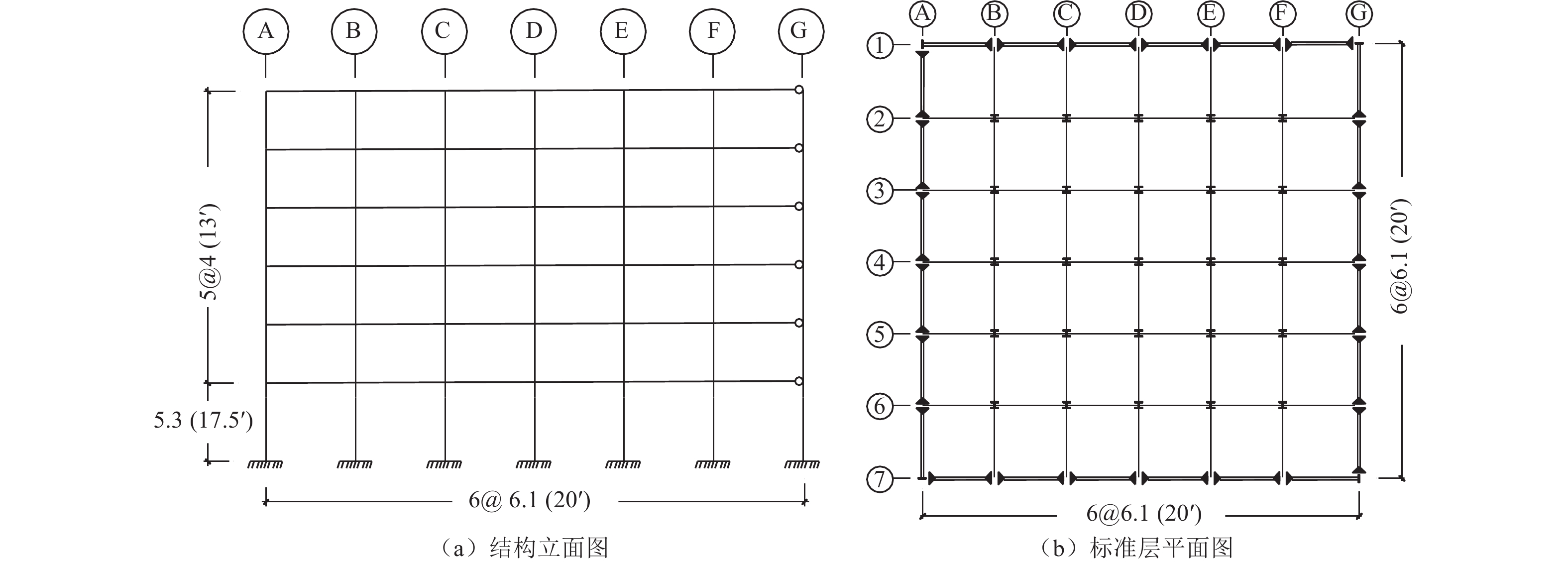
表 1 人造地震动特征
Table 1. The characteristics of peak values of artificial ground motion
合成地震动
序列峰值加速度
PGA/ （m·s−2）峰值速度
PGV/（m·s−1）峰值位移
PGD/dm第1序列 1.0 0.20 0.20 第2序列 1.0 0.20 0.40 第3序列 1.0 0.15 0.30 第4序列 1.0 0.30 0.30 
下载: 导出CSV
表 2 结构梁柱截面配置表
Table 2. Section configuration of the beams and columns
楼层 梁截面 柱截面 6 W24×68 W14×90 5 W24×84 W14×90 3～4 W24×84 W14×132 2 W27×102 W14×176 1 W30×116 W14×176
下载: 导出CSV
表 3 楼层加速度反应统计
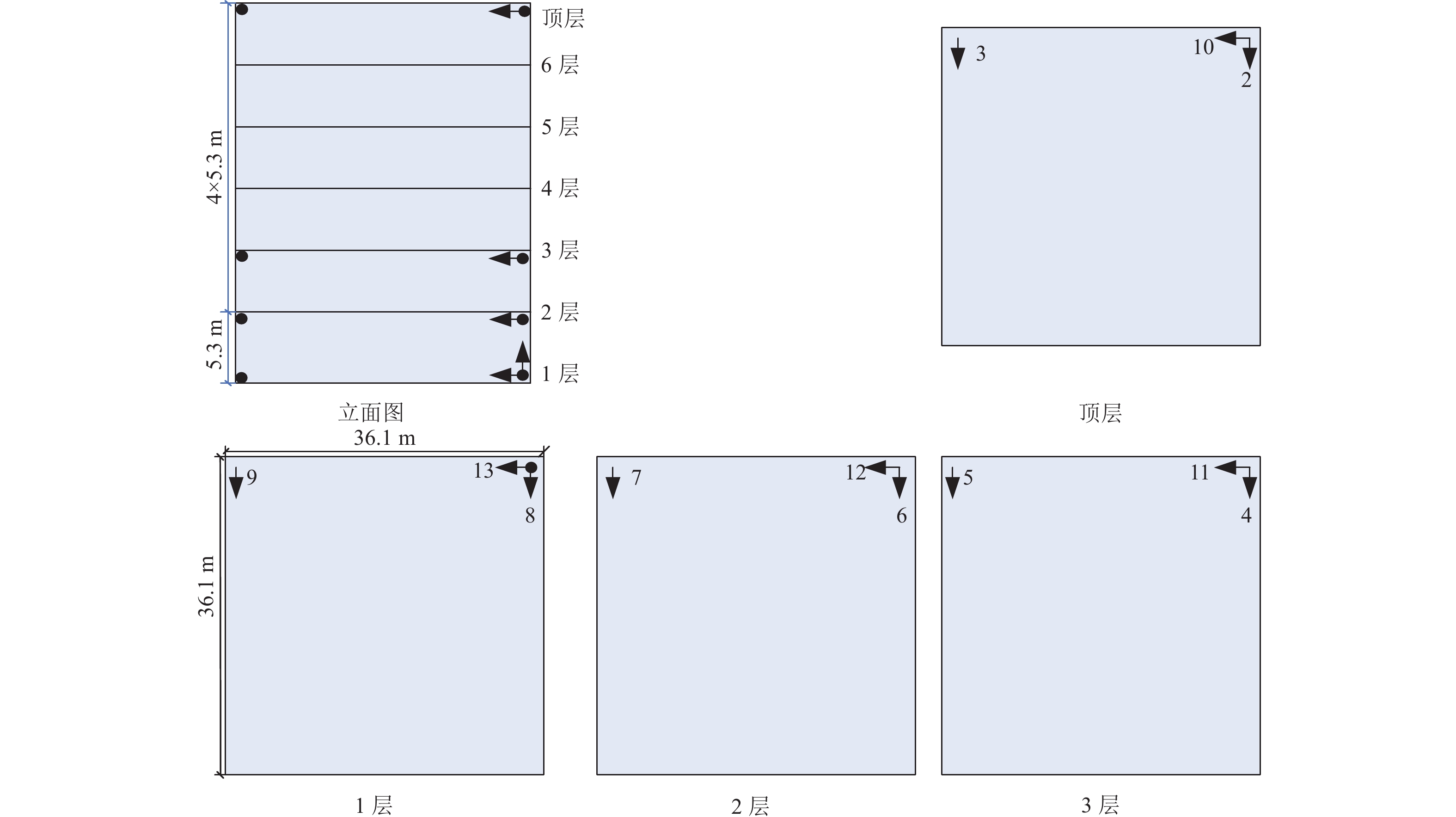
Table 3. Amplitude statistics of floor acceleration response
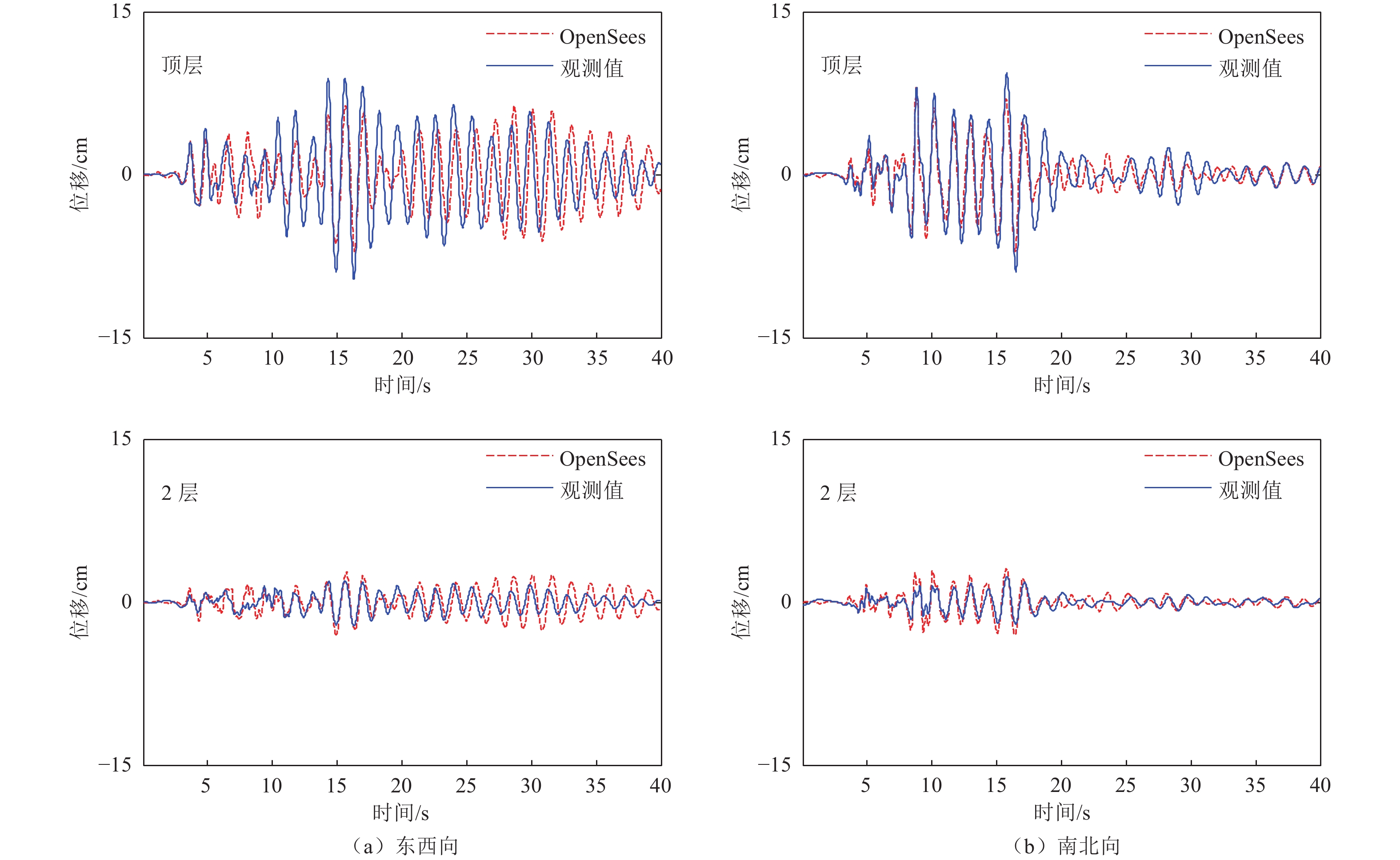
楼层 加速度/（cm·s−2） 东西向 南北向 顶层 270.7 441.1 2 195.2 279.8 1 208.6 293.0
下载: 导出CSV
表 4 结构自振频率对比分析
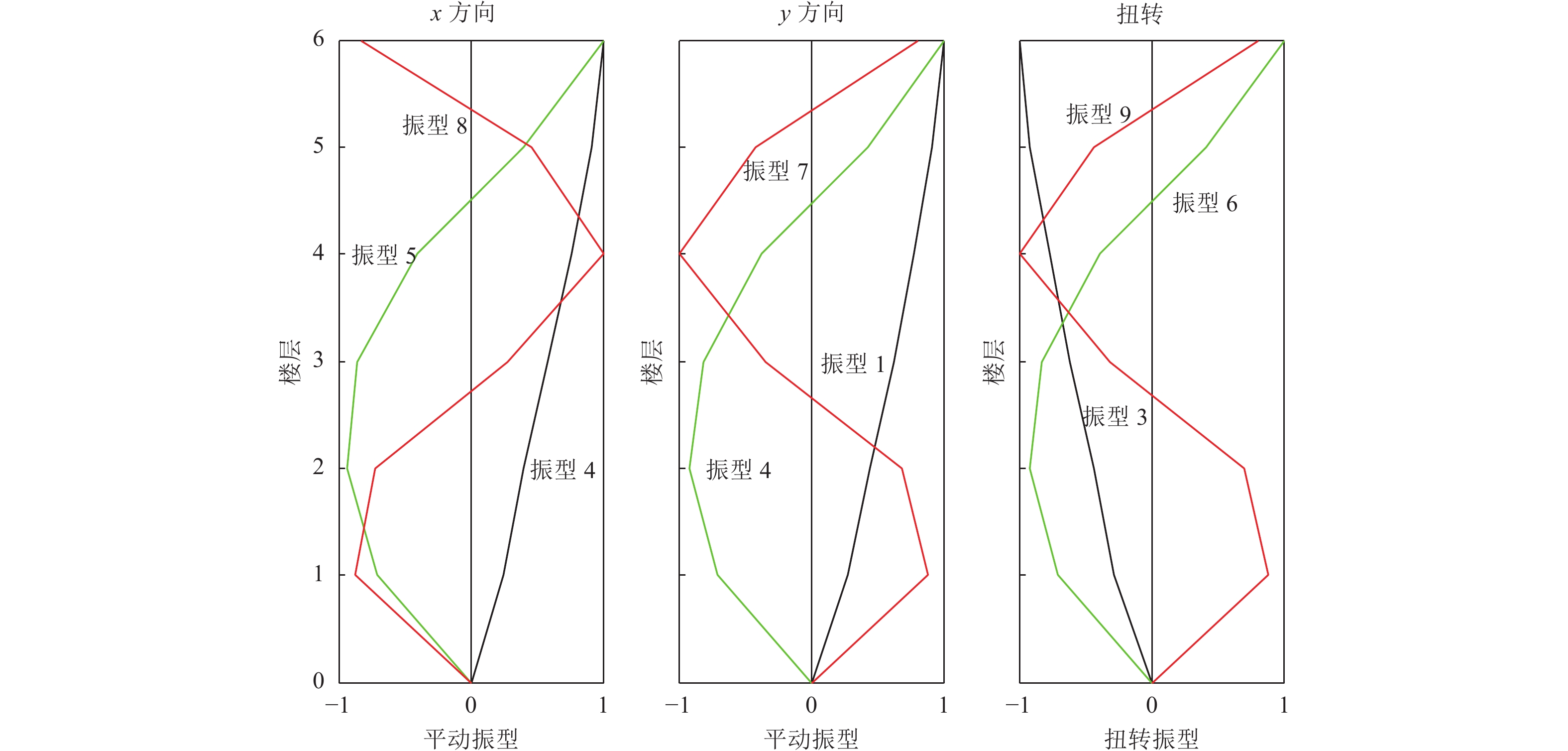
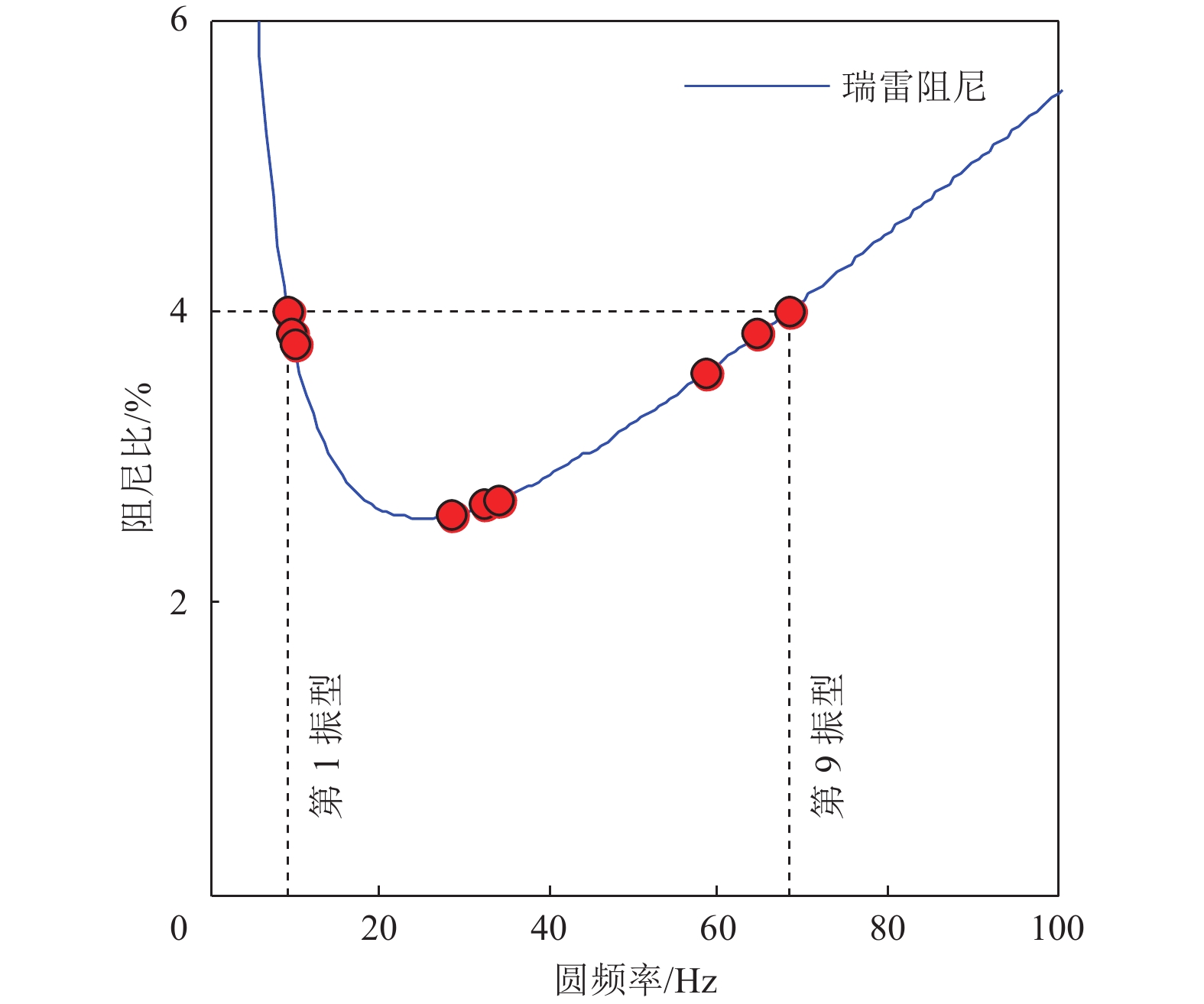
Table 4. Comparative analysis of natural frequency of the building
振型
编号振型
特性系统识别
频率/HzOpenSees计算
频率/Hz1 y方向 1.462 1.456 2 x方向 1.557 1.537 3 扭转 1.679 1.574 4 y方向 4.556 4.531 5 x方向 5.502 5.177 6 扭转 5.475 5.459 7 y方向 9.319 9.312 8 x方向 10.281 10.277 9 扭转 10.976 10.868
下载: 导出CSV
-
陈健云, 李静, 周晶等, 2003. 地震动频谱对小湾拱坝非线性响应的影响. 振动工程学报, 16（2）: 207—211Chen J. Y. , Li J. , Zhou J. , et al. , 2003. Effects of time-frequency joint non-stationariness on the response of arch dam. Journal of Vibration Engineering, 16(2): 207—211. (in Chinese) 杜修力, 王福源, 王进廷等, 2015. 地震动峰值位移对高拱坝地震反应的影响. 水力发电学报, 34（11）: 134—142Du X. L. , Wang F. Y. , Wang J. T. , et al. , 2015. Effect of peak ground displacement on seismic responses of high arch dam. Journal of Hydroelectric Engineering, 34(11): 134—142. (in Chinese) 杜修力, 许紫刚, 袁雪纯等, 2018. 地震动峰值位移和峰值速度对地下结构地震反应的影响. 震灾防御技术, 13（2）: 293—303Du X. L. , Xu Z. G. , Yuan X. C. , et al. , 2018. Influence of peak ground displacement and peak ground velocity of ground motion on dynamic response of underground structures. Technology for Earthquake Disaster Prevention, 13(2): 293—303. (in Chinese) 郝明辉, 王珊, 张郁山, 2016. 峰值速度对单自由度体系地震反应的影响分析. 地震工程与工程振动, 36（6）: 120—132Hao M. H. , Wang S. , Zhng Y. S. , 2016. Influence of ground-motion peak velocity on non-linear dynamic response of single-degree-of-freedom system. Earthquake Engineering and Engineering Vibration, 36(6): 120—132. (in Chinese) 贺秋梅, 2012. 地震动的速度脉冲对结构反应及结构减隔震性能影响研究. 北京: 中国地震局地球物理研究所.He Q. M. , 2012. Study on the influence of seismic velocity pulse on structural response and isolation properties. Beijing: Institute of Geophysics, China Earthquake Administration. (in Chinese) 胡良明, 朱军福, 孙奔博, 2019. 地震动峰值速度与峰值加速度对重力坝动力响应影响. 水力发电, 45（7）: 66—71Hu L. M. , Zhu J. F. , Sun B. B. , 2019. Influence of peak velocity and peak acceleration of seismic ground motion on dynamic response of gravity dam. Water Power, 45(7): 66—71. (in Chinese) 胡聿贤, 2006. 地震工程学. 2版. 北京: 地震出版社.Hu Y. X. , 2006. Earthquake engineering. 2 nd ed. Beijing: Seismological Press. (in Chinese) 李新乐, 朱晞, 2004. 近断层地震速度脉冲效应对桥墩地震反应的影响. 北方交通大学学报, 28（1）: 11—16Li X. L. , Zhu X. , 2004. Velocity pulse for near-fault ground motions and its effect on seismic response of pier. Journal of Northern Jiaotong University, 28(1): 11—16. (in Chinese) 陆新征, 叶列平, 缪志伟, 2009. 建筑抗震弹塑性分析——原理、模型与在ABAQUS, MSC. MARC和SAP2000上的实践. 北京: 中国建筑工业出版社.Lu X. Z. , Ye L. P. , Miao Z. W. , 2009. Elasto-plastic analysis of buildings against earthquake: theory, model and implementation on ABAQUS, MSC. MARC and SAP2000. Beijing: China Architecture & Building Press. (in Chinese) 任志林, 2004. 震害预测中结构地震破坏指数的不确定性研究. 北京: 中国地震局地球物理研究所. 孙忠贤, 2009. 地震动特性对结构地震反应的影响分析. 哈尔滨: 中国地震局工程力学研究所.Sun Z. X. , 2009. The influence of ground motion characteristics on response of structures. Harbin: Institute of Engineering Mechanics, China Earthquake Administration. (in Chinese) 王彬, 李文艺, 2000. 合成地震动方法用于研究强地震动长周期特性的探讨. 地震研究, 23（1）: 44—50Wang B. , Li W. Y. , 2000. Study on the character of long period for strong earthquake ground motion using methods of synthetic earthquake ground motion. Journal of Seismological Research, 23(1): 44—50. (in Chinese) 王飞, Reza S. , 2016. 基于振动台实验的结构损伤识别研究. 地震工程学报, 38（1）: 129—135Wang F. , Reza S. , 2016. Structural damage Identification based on shaking table tests. China Earthquake Engineering Journal, 38(1): 129—135. (in Chinese) 王国新, 李宏男, 赵真等, 2008. 结构动力反应分析中的地震动输入问题研究. 沈阳建筑大学学报（自然科学版）, 24（6）: 993—998Wang G. X. , Li H. N. , Zhao Z. , et al. , 2008. Study on ground motion input for structural dynamic analysis. Journal of Shenyang Jianzhu University (Natural Science), 24(6): 993—998. (in Chinese) 韦韬, 2005. 近断层速度脉冲对钢筋混凝土框架结构影响的研究. 北京: 中国地震局地球物理研究所.Wei T. , 2005. Study on effects of near-fault velocity pulse on RC framed structures. Beijing: Institute of Geophysics, China Earthquake Administration. (in Chinese) 杨溥, 李英民, 赖明, 2000. 结构时程分析法输入地震波的选择控制指标. 土木工程学报, 33（6）: 33—37Yang P. , Li Y. M. , Lai M. , 2000. A new method for selecting inputting waves for time-history analysis. China Civil Engineering Journal, 33(6): 33—37. (in Chinese) 尤红兵, 张郁山, 赵凤新, 2011. 地震动峰值速度对地下隧洞内力的影响研究. 震灾防御技术, 6（2）: 105—115You H. B. , Zhang Y. S. , Zhao F. X. , 2011. Influence of peak ground velocity on the internal force of underground tunnel. Technology for Earthquake Disaster Prevention, 6(2): 105—115. (in Chinese) 张斌, 俞言祥, 李小军等, 2021. 西南地区水平向峰值速度、峰值位移衰减关系研究. 地球物理学报, 64（8）: 2733—3748.Zhang B. , Yu Y. X. , Li X. J. , et al. , 2021. Ground motion attenuation relationship of horizontal component of PGV and PGD in Southwest China. Chinese Journal of Geophysics, 64（8）: 2733—2748. (in Chinese) 张新培, 2003. 钢筋混凝土抗震结构非线性分析. 北京: 科学出版社. 张郁山, 赵凤新, 2011. 地震动峰值位移对单自由度体系非线性动力反应的影响. 工程力学, 28（1）: 55—64Zhang Y. S. , Zhao F. X. , 2011. Influence of ground-motion peak displacement on non-linear dynamic response of single-degree-of-freedom system. Engineering Mechanics, 28(1): 55—64. (in Chinese) 赵凤新, 张郁山, 2006. 拟合峰值速度与目标反应谱的人造地震动. 地震学报, 28（4）: 429—437Zhao F. X. , Zhang Y. S. , 2006. Artificial ground motion compatible with specified peak velocity and target spectrum. Acta Seismologica Sinica, 28(4): 429—437. (in Chinese) 赵凤新, 韦韬, 张郁山, 2008. 近断层速度脉冲对钢筋混凝土框架结构地震反应的影响. 工程力学, 25（10）: 180—186, 193Zhao F. X. , Wei T. , Zhang Y. S. , 2008. Influence of near-fault velocity pulse on the seismic response of reinforced concrete frame. Engineering Mechanics, 25(10): 180—186, 193. (in Chinese) 中华人民共和国住房和城乡建设部, 中华人民共和国国家质量监督检验检疫总局, 2010. GB 50011—2010 建筑抗震设计规范. 北京: 中国建筑工业出版社.Ministry of Housing and Urban-Rural Development of the People's Republic of China, General Administration of Quality Supervision, Inspection and Quarantine of the People's Republic of China, 2010. GB 50011—2010 Code for seismic design of buildings. Beijing: China Architecture & Building Press. (in Chinese) 周媛, 2006. 地震动速度与位移对大跨斜拉桥地震反应影响的研究. 北京: 中国地震局地球物理研究所.Zhou Y. , 2006. Study on effects of ground velocity and ground displacement on seismic responses of long span cable-stayed bridge. Beijing: Institute of Geophysics, China Earthquake Administration. (in Chinese) Alavi B., Krawinkler H., 2000. Consideration of near-fault ground motion effects in seismic design. In: 12 th World Conference on Earthquake Engineering. Auckland: WCEE. Alavi B. , Krawinkler H. , 2004. Behavior of moment-resisting frame structures subjected to near-fault ground motions. Earthquake Engineering & Structural Dynamics, 33(6): 687—706. Anderson J. C. , Bertero V. V. , 1987. Uncertainties in establishing design earthquakes. Journal of Structural Engineering, 113(8): 1709—1724. doi: 10.1061/(ASCE)0733-9445(1987)113:8(1709) Ayan A. K., Boduroglu H., 2004. Strengthening of existing reinforced concrete buildings and near fault effects. In: 13 th World Conference on Earthquake Engineering. Vancouver: WCEE. Bertero V. V. , Mahin S. A. , Herrera R. A. , 1978. Aseismic design implications of near-fault San Fernando earthquake records. Earthquake Engineering & Structural Dynamics, 6(1): 31—42. Chopra A. K., 2001. Dynamics of structures: theory and applications to earthquake engineering. 2 nd ed. Upper Saddle River: Prentice Hall. Ghobarah A., 2004. Response of structures to near-fault ground motion. In: 13 th World Conference on Earthquake Engineering. Vancouver: WCEE. Hall J. F. , Heaton T. H. , Halling M. W. , et al. , 1995. Near-source ground motion and its effects on flexible buildings. Earthquake Spectra, 11(4): 569—605. doi: 10.1193/1.1585828 Hall J. F. , Ryan K. L. , 2000. Isolated buildings and the 1997 UBC near-source factors. Earthquake Spectra, 16(2): 393—411. doi: 10.1193/1.1586118 Jangid R. S. , Kelly J. M. , 2001. Base isolation for near-fault motions. Earthquake Engineering & Structural Dynamics, 30(5): 691—707. Katsanos E. I. , Sextos A. G. , Manolis G. D. , 2010. Selection of earthquake ground motion records: a state-of-the-art review from a structural engineering perspective. Soil Dynamics and Earthquake Engineering, 30(4): 157—169. doi: 10.1016/j.soildyn.2009.10.005 Kunnath S. K. , Nghiem Q. , El-Tawil S. , 2004. Modeling and response prediction in performance-based seismic evaluation: case studies of instrumented steel moment-frame buildings. Earthquake Spectra, 20(3): 883—915. doi: 10.1193/1.1774181 Kunnath S. K., Kalkan E., 2005. IDA capacity curves: the need for alternative intensity factors. In: Proceedings of the American Society of Civil Engineers, Structures Congress. New York: American Society of Civil Engineers, 1—9. Makris N. , Chang S. P. , 2000. Effect of viscous, viscoplastic and friction damping on the response of seismic isolated structures. Earthquake Engineering & Structural Dynamics, 29(1): 85—107. Mavroeidis G. P. , Dong G. , Papageorgiou A. S. , 2004. Near-fault ground motions, and the response of elastic and inelastic single-degree-of-freedom (SDOF) systems. Earthquake Engineering & Structural Dynamics, 33(9): 1023—1049. Reitherman R. , 1985. A review of earthquake damage estimation methods. Earthquake Spectra, 1(4): 805—847. doi: 10.1193/1.1585293 Sasani M., Bertero V. V., 2000. Importance of severe pulse-type ground motions in performance-based engineering: historical and critical review. In: 12 th World Conference on Earthquake Engineering. Auckland: WCEE. Seneviratna G. D. P. K., Krawinkler H., 1997. Evaluation of inelastic MDOF effects for seismic design. Stanford: John A. Blume Earthquake Engineering Center. Shakal A., Huang M., Darragh R., et al., 1994. CSMIP strong-motion records from the Northridge, California earthquake of 17 January 1994. Sacramento: California Office of Strong-Motion Studies. Sordo E. , Terán A. , Guerrero J. J. , et al. , 1989. The Mexico earthquake of September 19, 1985—ductility and resistance requirements imposed on a concrete building. Earthquake Spectra, 5(1): 41—50. doi: 10.1193/1.1585510 Stephens J. E. , Yao J. T. P. , 1987. Damage assessment using response measurement. Journal of Structural Engineering, 113(4): 787—801. doi: 10.1061/(ASCE)0733-9445(1987)113:4(787) Wang J. J. , Fan L. C. , Qian S. E. , et al. , 2002. Simulations of non-stationary frequency content and its importance to seismic assessment of structures. Earthquake Engineering & Structural Dynamics, 31(4): 993—1005. -
下载:
点击查看大图
计量
- 文章访问数: 97
- HTML全文浏览量: 19
- PDF下载量: 22
- 被引次数: 0
