Analysis of Monitoring Capability of Jiangsu Seismic Network Based on PMC Method
-
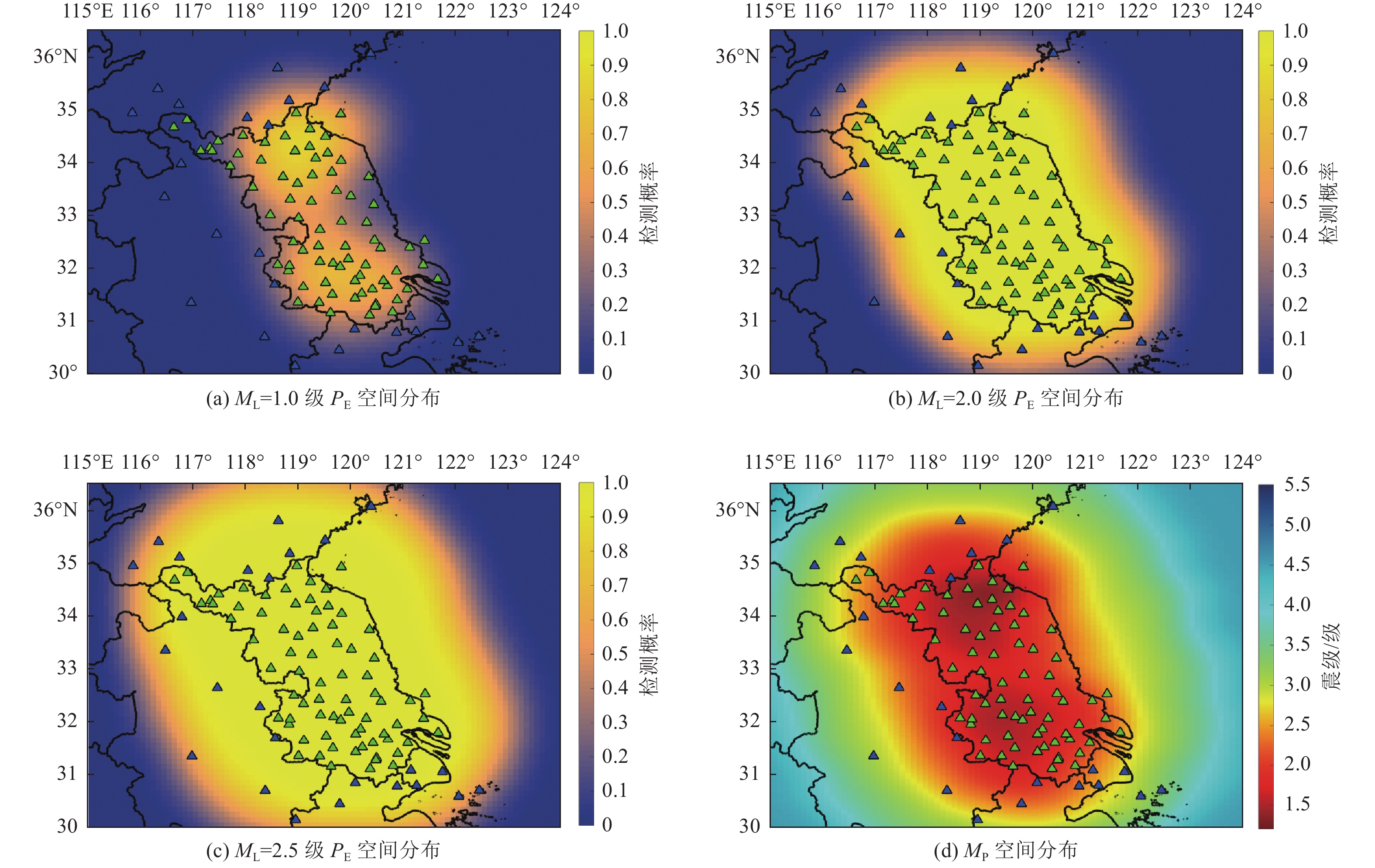
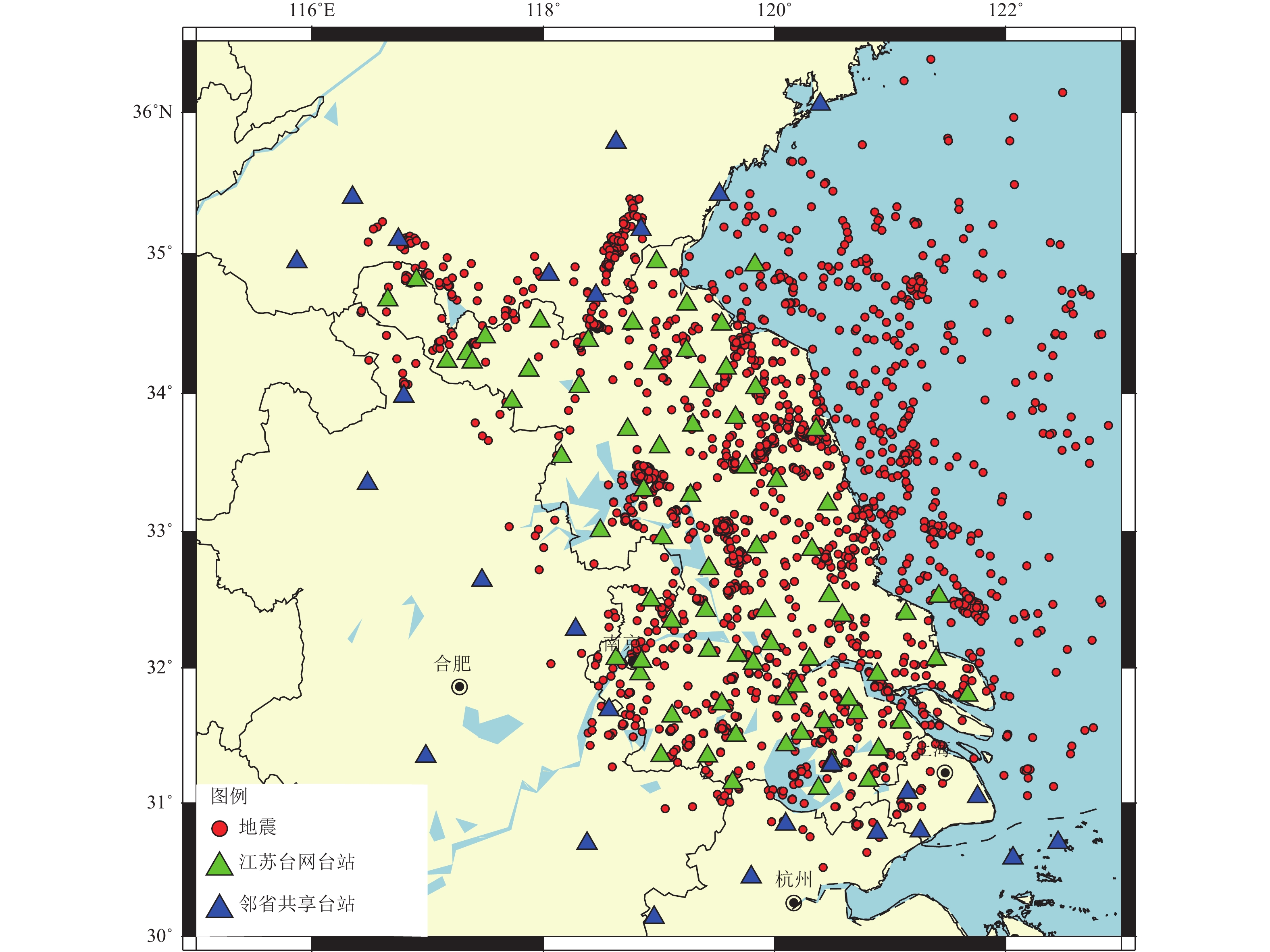
摘要: 江苏测震台网经近20年的高速发展，已拥有75个数字测震台站，针对江苏台网监测能力的评估需求，同时为进一步优化台网布局，提高江苏测震台网监测能力提供参考意见，本文使用基于概率的完整性震级方法，利用江苏台网2009—2021年正式编目观测报告数据，对江苏台网进行监测能力评估。研究结果表明，江苏台网监测能力较好的地区为北部连云港周边地区，监测能力达到了
$ {M}_{\mathrm{L}}\geqslant 1.0 $ ；其次为南部南京、镇江、常州及周边地区，监测能力达到了$ {M}_{\mathrm{L}}\geqslant 1.5 $ ；监测能力较差的地区为中部地区及近海海域，监测能力达到了$ {M}_{\mathrm{L}}\geqslant 2.0 $ 。整个江苏台网基本实现了$ {M}_{\mathrm{L}}\geqslant 2.0 $ 的监测能力，周边地区及中部近海海域基本实现了$ {M}_{\mathrm{L}}\geqslant 2.5 $ 的监测能力。-
关键词:
- 江苏测震台网 /
- 监测能力评估 /
- 完整性震级PMC方法
Abstract: After nearly two decades of rapid development, the Jiangsu Seismic Network has expanded to include 75 digital seismic stations. This study aims to evaluate the monitoring capabilities of the Jiangsu network and provide recommendations for optimizing the network layout and improving its monitoring effectiveness. The evaluation utilizes the Probability-based Magnitude of Completeness (PMC) method and examines the official cataloged observation report data from 2009 to 2021. The research findings indicate variations in monitoring capabilities across different regions of the Jiangsu network. The northern regions, particularly around Lianyungang, exhibit relatively robust monitoring capabilities, with a threshold of$ {M}_{{\rm{L}}} $ ≥1.0. The southern regions, including Nanjing, Zhenjiang, Changzhou, and the surrounding areas, demonstrate relatively stronger monitoring capabilities, reaching approximately$ {M}_{{\rm{L}}} $ ≥1.5. The central regions and nearshore areas exhibit lower monitoring capabilities, with a threshold of$ {M}_{{\rm{L}}} $ ≥2.0. Overall, the entire Jiangsu network has achieved a satisfactory level of monitoring capabilities for$ {M}_{{\rm{L}}} $ ≥2.0, while the surrounding areas and central nearshore regions have achieved a higher level of seismic monitoring capabilities, reaching$ {M}_{{\rm{L}}} $ ≥2.5. -
引言
悬索桥结构主要由锚碇、索塔、缆索系统、加劲梁和附属结构组成，具有结构简单、轻便、易于标准化、构件易运输、方便悬吊拼装、施工限制较少等特点（铁道部大桥工程局桥梁科学研究所，1996；钱炜，2007），是特大桥梁的主要类型（李光军，2012）。地震是造成桥梁结构破坏的主要原因之一，桥梁是联系外界与地震灾区的重要纽带，桥梁一旦破坏，将中断通往灾区的通道，影响救灾工作进展，造成较大损失（刘润舟，2008）。因此，对悬索桥结构进行地震响应分析具有重要意义。国内外学者已开展了大跨度桥梁多点激励地震响应研究，如许基厚等（2019）对非对称单侧混合梁斜拉桥多点激励地震响应进行研究，分析了行波效应对塔顶、主梁、塔底等关键位置动力响应的影响，并研究了不同入射角对桥梁动力响应的影响，认为多点激励下主塔内力计算结果偏小，而主梁内力计算结果偏大，地震激励对非对称大跨度斜拉桥内力产生较大影响，内力变化可达20%；张凡等（2016）对不同波速下斜拉桥地震响应进行了分析，认为波速的影响显著；王再荣等（2016）分析行波效应下斜拉桥地震响应，得出辅助墩耗能降低、桥塔损伤增大的结论；刘旭政等（2018）考虑2种地震波和3种视波速的不同组合，以一座实际工程高桩大跨度连续刚构桥为例，研究行波效应对桥梁地震响应的影响，认为随着视波速的增大，行波效应对桥梁内力的影响程度减小；梅泽洪等（2017）研究了考虑局部场地效应桥梁结构在非一致激励下地震响应的特点，认为下部结构响应所受影响较小，而上部结构所受影响明显，考虑行波效应的非一致激励对桥梁地震响应有减弱效果；黎璟等（2019）以铁路工程实际桥梁为例，研究了非一致激励下大跨度铁路斜拉桥地震响应规律，认为在非一致激励下塔顶位移响应峰值与墩底弯矩响应峰值均随着相位差呈周期性变化，且变化周期与结构一阶自振周期基本一致。
本文选取某三跨两铰连续体系悬索桥，已有研究结果表明线性分析方法和非线性分析方法得到的该桥地震响应结果相近（Fleming等，1980；李丽等，2018a）。因此利用大型通用有限元软件ANSYS建立三维弹性有限元模型，分析一致激励与多点激励下悬索桥地震响应，研究其抗震性能。
1. 桥梁结构动力响应分析方法
桥梁结构动力响应分析方法主要包括一致激励动态时程分析方法及多点激励动态时程分析方法，目前多点激励动态时程分析方法的应用较多，该方法考虑地震行波效应和局部场地效应，对各独立基础或支撑结构输入不同的设计反应谱或加速度时程进行计算（张沧海，2011），主要包括相对运动法和大质量法（范立础等，2001；Leger等，1990）。相对运动法通过支撑点的加速度时程计算非支撑点的动力响应。大质量法是对结构模型进行动力等效的分析方法，在处理多点激励问题时需解除支撑点沿地震作用方向的约束，并赋予节点大质量，其值通常远大于结构体系的总质量（苏成等，2008）。本文采用相对运动法计算悬索桥在多点激励下的响应。
2. 桥梁结构概况
三跨连续悬索桥主跨124m，主梁设计为连续加劲梁，加劲梁采用正交异性板流线形扁平钢箱梁，梁高1.88m，宽（含风嘴）10.8m；纵向分配梁宽0.16m，厚0.28m；纵向斜腹杆，横向内侧竖杆，横向内、外侧斜腹杆截面宽度均为0.16m，厚度均为0.2m；纵向上、下弦杆截面宽度均为0.3m，厚度均为0.2m；纵向竖杆截面宽0.2m，厚0.18m；横向外侧竖杆截面宽0.2m，厚0.24m；横向上弦杆截面宽0.18m，厚0.24m；横向下弦杆截面宽0.18m，厚0.2m；抗风桁架截面宽0.12m，厚0.12m。主塔为H形，塔高36m，南、北桥塔未设置上、下横梁。塔柱截面宽2m，厚4m；柱间连接件截面宽4m，厚2m。桥面以上有3根主缆，南、北边跨吊索各12根，主跨共30根，索间距4m。主缆截面直径为0.079m，吊索截面直径为0.039m。桥梁结构各构件材料物理参数见表 1。
表 1 桥梁结构材料物理参数Table 1. The material physical parameters of bridge structure构件 材料 弹性模量/Pa 泊松比 密度/kg·m-3 主缆 钢丝绳 21.0×1010 0.167 7850 吊索 钢丝绳 21.0×1010 0.167 7850 加劲桁架、纵梁 C30混凝土 3.0×1010 0.300 2500 桥塔、桥面板 C20混凝土 2.8×1010 0.300 2500 3. 输入地震动
选取常用的Kobe地震波对悬索桥进行地震响应分析，顺桥向输入的加速度时程曲线如图 1，加速度峰值为0.345g，持时为24.79s。
图 1 输入Kobe波加速度时程曲线（顺桥向）Figure 1. The input Kobe wave of acceleration time history curve (Along the bridge)对于大跨度悬索桥，输入地震动时常将水平地震系数的1/2或2/3作为竖向地震系数，组合方式通常为顺桥向与竖向组合、横桥向与竖向组合（李国豪，2003），因此，进行一致激励下的地震响应分析时，本文将竖向地震系数取为水平地震系数的1/2，并选取顺桥向与竖向组合；进行多点激励下的地震响应分析时，将竖向地震系数取为水平地震系数的1/2，先对加速度时程曲线进行2次积分，第1次积分得到速度时程曲线，并将其进行基线校正，将校正后的速度时程曲线再次积分并进行基线校正，得到位移时程曲线，如图 2。
图 2 Kobe波位移时程曲线（顺桥向）Figure 2. The input Kobe wave of displacement time history curve (Along the bridge)多点激励位移输入位置为图 3中①-④桥梁支座位置，计算时考虑不同支座输入波形相位滞后现象，采用对不同支座延迟输入位移时程的方法考虑行波效应（刘春城等，2004；王蕾等，2006；严琨等，2017），根据大跨建筑结构多点输入地震响应计算结果（李丽等，2018b；景鹏旭等，2017）与抗震设计方法研究结果（江洋，2010），取视波速为1000m/s，根据支座间距计算各支座位移输入延迟时间，见表 2。
图 3 桥梁结构立面图及荷载激励点布置Figure 3. The elevation and load excitation point layout of the bridge表 2 输入位移时程延迟时间（s）Table 2. The delay time of input displacement time history (s)支座编号 ① ② ③ ④ 时间间隔 0.000 0.050 0.174 0.224 4. 有限元模型的建立
应用大型通用有限元软件ANSYS建立悬索桥三维有限元模型（图 4），应用link10单元模拟主缆及吊索，应用beam4单元模拟加劲桁架、纵向分配梁、抗风桁架、桥塔，应用shell63单元模拟桥面板。主缆及吊索初应变设为0.0043，先对结构进行重力分析，得到结构初始应力，然后对其进行模态分析，计算桥梁结构前2阶振型圆频率，应用APDL语言编制求解瑞利阻尼系数α和β：
ALPHAD, 2*DAMPRATIO*FREQ1*2*3.1415926
BETAD, 2*DAMPRATIO/(FREQ1*2*3.1415926)
5. 地震响应分析结果
计算得到主梁位移及南、北桥塔塔柱沿柱高方向的位移、轴力、剪力及弯矩包络图，如图 5-7。
图 5 主梁顺桥向最大位移包络图Figure 5. Envelope diagram of maximum displacement of main beam along bridge direction
图 6 南桥塔纵向位移、轴力、剪力及弯矩包络图Figure 6. The envelope of displacement, axial force, shearing force and bending moment of the occurrence bridge tower longitudinal
图 7 北桥塔纵向位移、轴力、剪力及弯矩包络图Figure 7. The envelope of displacement, axial force, shearing force and bending moment of the north bridge tower longitudinal由图 5可知，多点激励下主梁最大位移略高于一致激励下主梁最大位移，总体变化不大。由图 6、图 7可知，一致激励下南、北桥塔沿塔高的位移、轴力、剪力及弯矩计算结果相同，这符合有限元计算基本原理。多点激励下南、北桥塔计算结果不对称，说明地震波输入方式对计算结果具有一定影响，由于本研究中悬索桥跨度不大，所以一致激励和多点激励下计算结果差别不大。
6. 结语
本文对某对称悬索桥在一致激励及视波速1000m/s的多点激励下的地震响应进行分析，并对计算结果进行分析。计算结果表明地震波输入方式对模拟结果具有一定影响，计算结果存在一定差异，主梁在多点激励下的位移计算结果略高于一致激励下的计算结果，桥塔最大位移发生在塔中与塔顶之间，最大弯矩、轴力、剪力均发生在塔根处。
-
图 1 江苏及共享的邻省地震台站和2009—2021年震中分布
Figure 1. Distribution of seismic stations of Jiangsu and adjacent provinces and earthquakes occurred during 2009—2021
图 2 连云港台单台监测概率分布
Figure 2. Probability diagram of single station monitoring of Lianyungang station
图 3 盐城台单台监测概率分布
Figure 3. Probability diagram of single station monitoring of Yancheng station
图 4 南京台单台监测概率分布
Figure 4. Probability diagram of single station monitoring of Nanjing station
图 5
$ {P}_{\mathrm{E}} $ 与$ {M}_{\mathrm{P}} $ 空间分布Figure 5. Composite detection probability
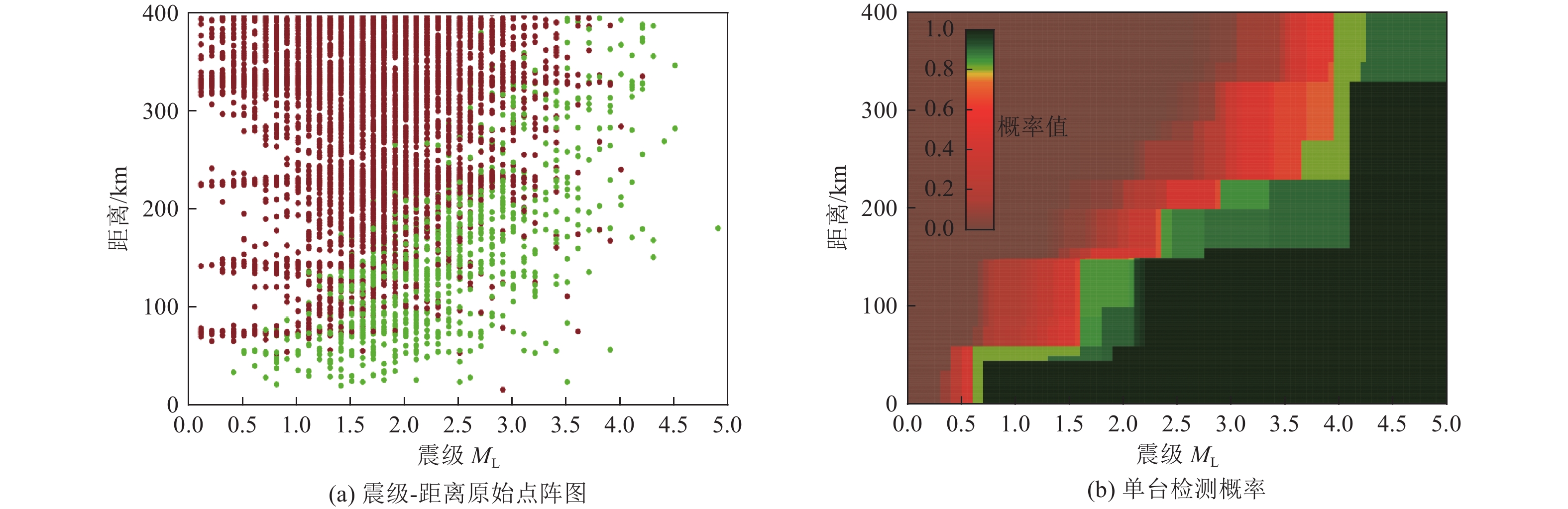
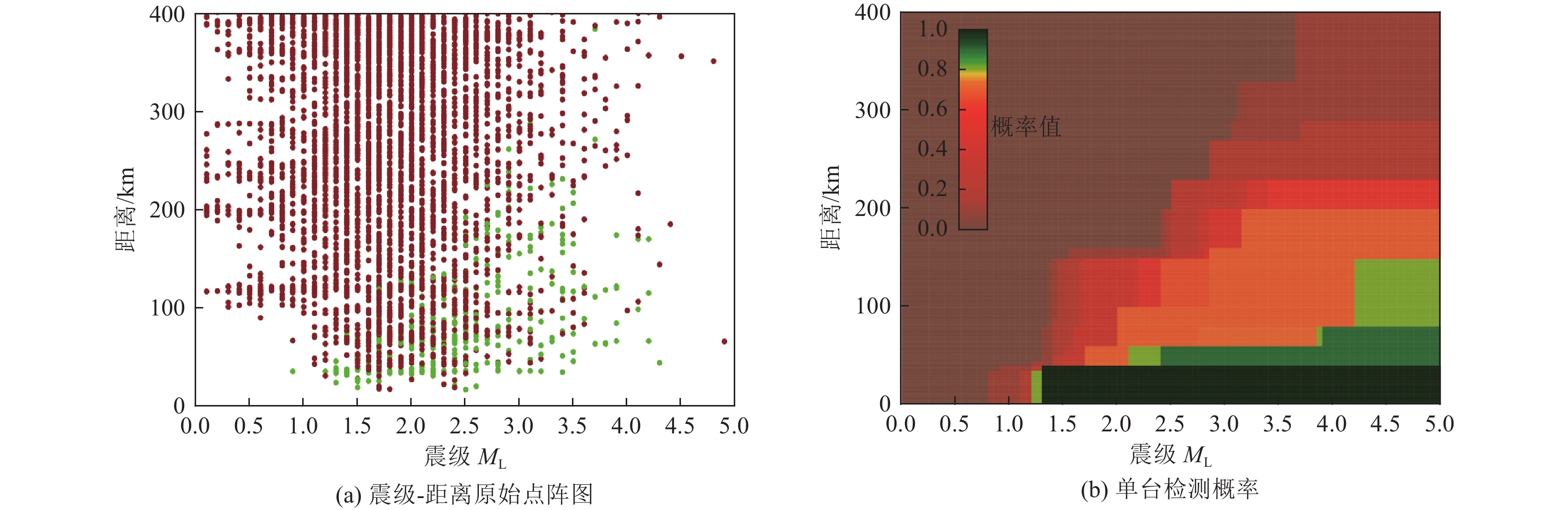
$ {{P}}_{\mathrm{E}} $ distribution map and$ {{M}}_{\mathrm{P}} $ spatial distribution map表 1 江苏台网典型台站单台检测概率
Table 1. Statistical table of single station detection probability of typical stations of Jiangsu network
台站名称/代码 L/km
（ML=1.0、PD=100%）ML/级
（L=100 km、PD=100%）L/km
（ML=3.0、PD=100%）ML/级
（L=250 km、PD=100%）噪声级别 台基
类型常熟/CS 40 1.6 150 3.5 Ⅱ 石英砂岩 常州/CZ — 1.4 150 — Ⅲ 混合花岗岩 邳州/PZ — 1.7 150 3.8 Ⅱ 石英砂岩 徐州/XZ — 2.7 150 — Ⅰ 灰岩 东海/DH 20 1.8 150 3.5 Ⅰ 混合花岗岩 赣榆/GAY 30 1.4 150 3.3 Ⅰ 混合岩 高淳/GC 30 1.6 150 3.5 Ⅰ 紫红长石砂岩 灌云/GUY 50 2.0 150 3.2 Ⅰ 花岗片麻岩 镇江/ZJ — 2.1 150 — Ⅱ 砂岩 江宁/JN — 3.4 90 3.5 Ⅱ 砂岩 浦口/PK — — 50 — Ⅲ 灰岩 靖江/JJ — 3.1 20 — Ⅲ 石英砂岩 金坛/JT — 4.6 50 — Ⅱ 砂岩 溧水/LIS 20 2.1 150 3.6 Ⅱ 片麻岩 泗洪/SH — 2.0 150 3.3 Ⅱ 安山岩 宿迁/SQ — 2.4 150 3.6 Ⅱ 安山凝灰岩 —锡/WX 20 1.7 150 3.5 Ⅱ 石英砂岩 新沂/XIY 20 2.2 150 3.3 Ⅱ 砂岩 徐圩/XW 20 2.4 150 3.7 Ⅱ 花岗片麻岩 盱眙/XY — 1.9 100 3.7 Ⅰ 灰岩 大丰（井下）/DF — — — — Ⅲ 松散沉积层 高邮（井下）/GY — 3.9 20 — Ⅱ 松散沉积层 海安（井下）/HA — 3.2 30 — Ⅱ 松散沉积层 淮安（井下）/HUA — 2.5 150 — Ⅱ 松散沉积层 涟水（井下）/LIS — — 30 — Ⅱ 石英岩 溧阳（井下）/LY 10 2.0 150 — Ⅰ 安山玄武岩 南通（井下）/NT — — 10 — Ⅱ 石英砂岩 沛县（井下）/PX — 2.4 50 — Ⅱ 石英砂岩 启东（井下）/QD — — — — Ⅱ 混合花岗岩 如东（井下）/RD — — 50 — Ⅱ 灰岩 射阳（井下）/SY — — 20 — Ⅲ 松散沉积层 泰州（井下）/TZ — 2.9 130 — Ⅲ 沉积岩 兴化（井下）/XH — — — — Ⅲ 混合花岗岩 盐城（井下）/YC — — 60 — Ⅱ 松散沉积层 阳光岛（井下）/YGD — — — — Ⅱ 基岩 宝应（井下）/BY — 3.1 40 — Ⅱ 石英砂岩 昆山/KS — 2.5 150 — Ⅲ 灰岩 六合/LH — 2.7 150 — Ⅰ 白云岩 前三岛/QSD — — — — Ⅱ 片麻岩 宜兴/YX — 2.5 150 — Ⅰ 砂岩 南京/NJ2 — 1.9 150 3.3 Ⅱ 安山玄武岩 连云港/LYG 40 1.7 150 3.1 Ⅰ 片麻岩 
下载: 导出CSV
-
安祥宇, 赵倩, 王晓睿等, 2019. 基于PMC方法的辽宁测震台网监测能力评估. 地震工程学报, 41（6）: 1545—1552 doi: 10.3969/j.issn.1000-0844.2019.06.1545An X. Y. , Zhao Q. , Wang X. R. , et al. , 2019. Assessment of earthquake monitoring capability of Liaoning seismic network based on PMC method. China Earthquake Engineering Journal, 41(6): 1545—1552. (in Chinese) doi: 10.3969/j.issn.1000-0844.2019.06.1545 黄亦磊, 周仕勇, 庄建仓, 2016. 基于地震目录估计完备震级方法的数值实验. 地球物理学报, 59（4）: 1350—1358 doi: 10.6038/cjg20160416Huang Y. L. , Zhou S. Y. , Zhuang J. C. , 2016. Numerical tests on catalog-based methods to estimate magnitude of completeness. Chinese Journal of Geophysics, 59(4): 1350—1358. (in Chinese) doi: 10.6038/cjg20160416 霍祝青, 瞿旻, 2016. 江苏地区小震精定位及构造意义分析. 地震工程学报, 38（5）: 802—807 doi: 10.3969/j.issn.1000-0844.2016.05.0802Huo Z. Q. , Qu M. , 2016. Precise relocation of small earthquakes in the Jiangsu area and associated tectonic implications. China Earthquake Engineering Journal, 38(5): 802—807. (in Chinese) doi: 10.3969/j.issn.1000-0844.2016.05.0802 蒋长胜, 房立华, 韩立波等, 2015. 利用PMC方法评估地震台阵的地震检测能力——以西昌流动地震台阵为例. 地球物理学报, 58（3）: 832—843 doi: 10.6038/cjg20150313Jiang C. S. , Fang L. H. , Han L. B. , et al. , 2015. Assessment of earthquake detection capability for the seismic array: a case study of the Xichang seismic array. Chinese Journal of Geophysics, 58(3): 832—843. (in Chinese) doi: 10.6038/cjg20150313 立凯, 陈浩, 张朋等, 2021. 江苏省测震台网背景噪声特征. 华北地震科学, 39（3）: 66—71, 76Li K., Chen H., Zhang P., et al., 2021 Background noise characteristics of Jiangsu seismological network. North China Earthquake Sciences, 39(3): 66—71, 76. (in Chinese) 李智超, 黄清华, 2014. 基于概率完备震级评估首都圈地震台网检测能力. 地球物理学报, 57（8）: 2584—2593 doi: 10.6038/cjg20140818Li Z. C. , Huang Q. H. , 2014. Assessment of detectability of the capital-circle seismic network by using the probability-based magnitude of completeness (PMC) method. Chinese Journal of Geophysics, 57(8): 2584—2593. (in Chinese) doi: 10.6038/cjg20140818 刘芳, 蒋长胜, 张帆等, 2014. 内蒙古区域地震台网监测能力研究. 地震学报, 36（5）: 919—929Liu F. , Jiang C. S. , Zhang F. , et al. , 2014. A study on detection capability of the Inner Mongolia regional seismic network. Acta Seismologica Sinica, 36(5): 919—929. (in Chinese) 王鹏, 郑建常, 李铂, 2016. 基于PMC方法的山东省测震台网监测能力评估. 地球物理学进展, 31（6）: 2408—2414 doi: 10.6038/pg20160607Wang P. , Zheng J. C. , Li B. , 2016. Analysis of detection capability of Shandong regional seismic network based on PMC method. Progress in Geophysics, 31(6): 2408—2414. (in Chinese) doi: 10.6038/pg20160607 Enescu B., Ito K., 2002. Spatial analysis of the frequency-magnitude distribution and decay rate of aftershock activity of the 2000 western tottori earthquake. Earth, Planets and Space, 54(8): 847—859. Gentili S., Sugan M., Peruzza L., et al., 2011. Probabilistic completeness assessment of the past 30 years of seismic monitoring in northeastern Italy. Physics of the Earth and Planetary Interiors, 186(1—2): 81—96. Gomberg J. , 1991. Seismicity and detection/location threshold in the southern great basin seismic network. Journal of Geophysical Research: Solid Earth, 96(B10): 16401—16414. doi: 10.1029/91JB01593 Gomberg J. , Reasenberg P. A. , Bodin P. , et al. , 2001. Earthquake triggering by seismic waves following the landers and hector mine earthquakes. Nature, 411(6836): 462—466. doi: 10.1038/35078053 Gutenberg B. , Richter C. F. , 1944. Frequency of earthquakes in California. Bulletin of the Seismological Society of America, 34(4): 185—188. doi: 10.1785/BSSA0340040185 He Y. C. , Li J. L. , Tian W. , et al. , 2021. Characterization of the near-surface shear wave attenuation in the Groningen gas field using borehole recording. Geophysical Journal International, 226(3): 2057—2072. doi: 10.1093/gji/ggab186 Knopoff L. , 2000. The magnitude distribution of declustered earthquakes in Southern California. Proceedings of the National Academy of Sciences of the United States of America, 97(22): 11880—11884. Main I., 2000. Apparent breaks in scaling in the earthquake cumulative frequency-magnitude distribution: fact or artifact? Bulletin of the Seismological Society of America, 90(1): 86—97. Nanjo K. Z. , Schorlemmer D. , Woessner J. , et al. , 2010. Earthquake detection capability of the Swiss seismic network. Geophysical Journal International, 181(3): 1713—1724 Rydelek P. A. , Sacks I. S. , 1989. Testing the completeness of earthquake catalogues and the hypothesis of self-similarity. Nature, 337(6204): 251—253. doi: 10.1038/337251a0 Schorlemmer D. , Woessner J. , 2008. Probability of detecting an earthquake. Bulletin of the Seismological Society of America, 98(5): 2103—2117. doi: 10.1785/0120070105 Schorlemmer D. , Mele F. , Marzocchi W. , 2010. A completeness analysis of the national seismic network of Italy. Journal of Geophysical Research: Solid Earth, 115(B4): B04308. Sereno T. J. Jr. , Bratt S. R. , 1989. Seismic detection capability at NORESS and implications for the detection threshold of a hypothetical network in the Soviet Union. Journal of Geophysical Research: Solid Earth, 94(B8): 10397—10414. doi: 10.1029/JB094iB08p10397 Stein R. S. , 1999. The role of stress transfer in earthquake occurrence. Nature, 402(6762): 605—609. doi: 10.1038/45144 Wiemer S. , Wyss M. , 2000. Minimum magnitude of completeness in earthquake catalogs: examples from Alaska, the western United States, and Japan. Bulletin of the Seismological Society of America, 90(4): 859—869. doi: 10.1785/0119990114 Wiemer S. , Wyss M. , 2002. Mapping spatial variability of the frequency-magnitude distribution of earthquakes. Advances in Geophysics, 45: 259—302, I-V. Woessner J. , Wiemer S. , 2005. Assessing the quality of earthquake catalogues: estimating the magnitude of completeness and its uncertainty. Bulletin of the Seismological Society of America, 95(2): 684—698. doi: 10.1785/0120040007 -
下载:
点击查看大图
计量
- 文章访问数: 161
- HTML全文浏览量: 50
- PDF下载量: 9
- 被引次数: 0
