Dimension Reduction Modeling of Near-fault Ground Motion Considering Randomness of Pulse Parameters
-
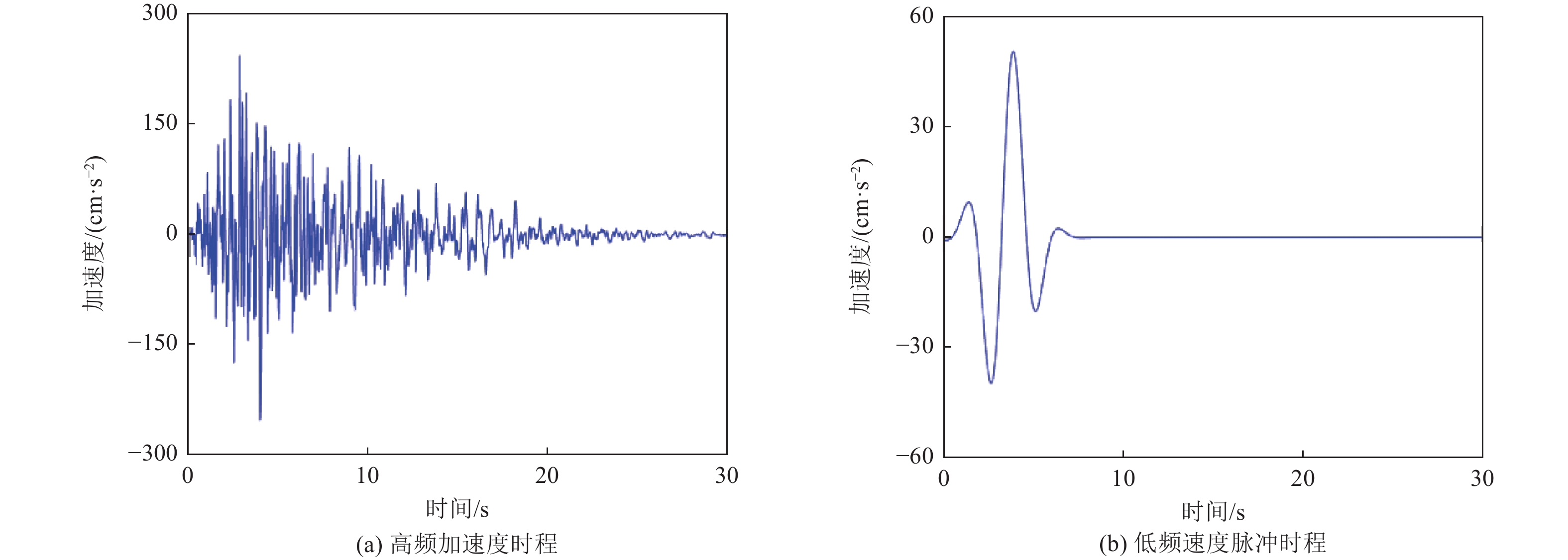
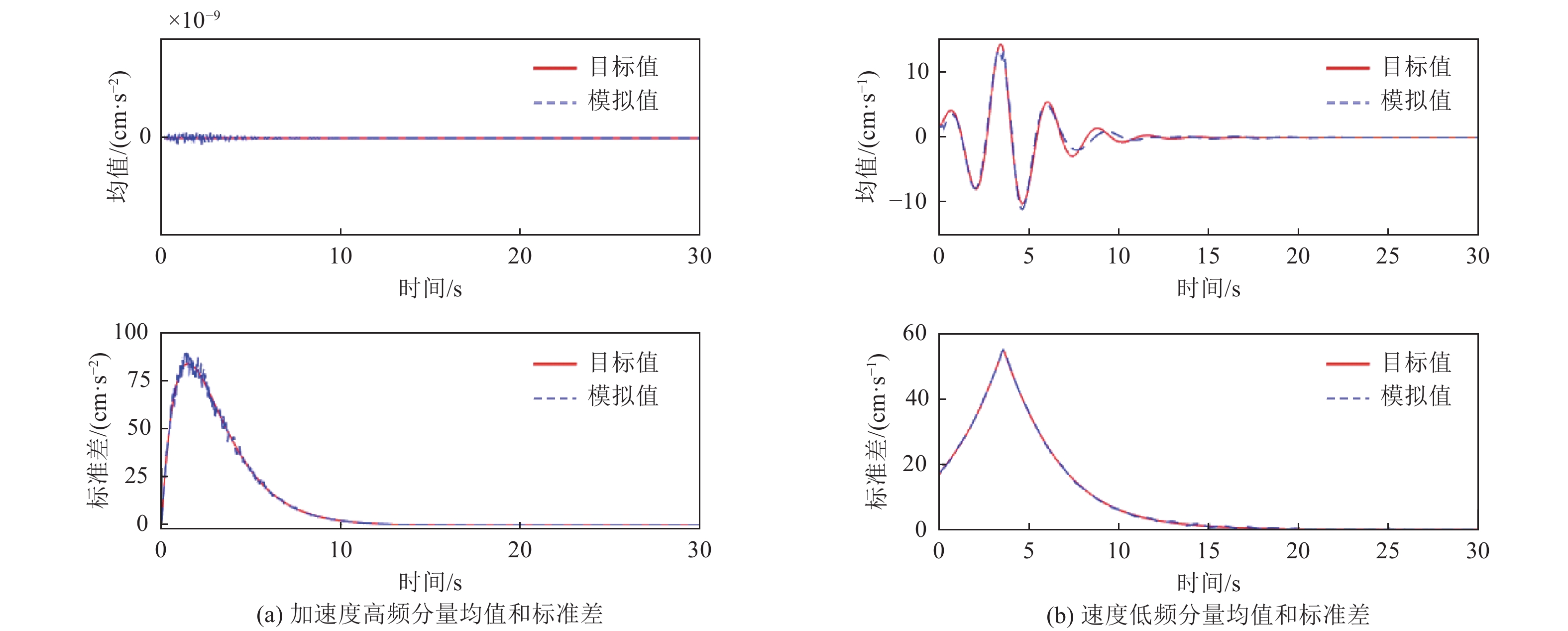
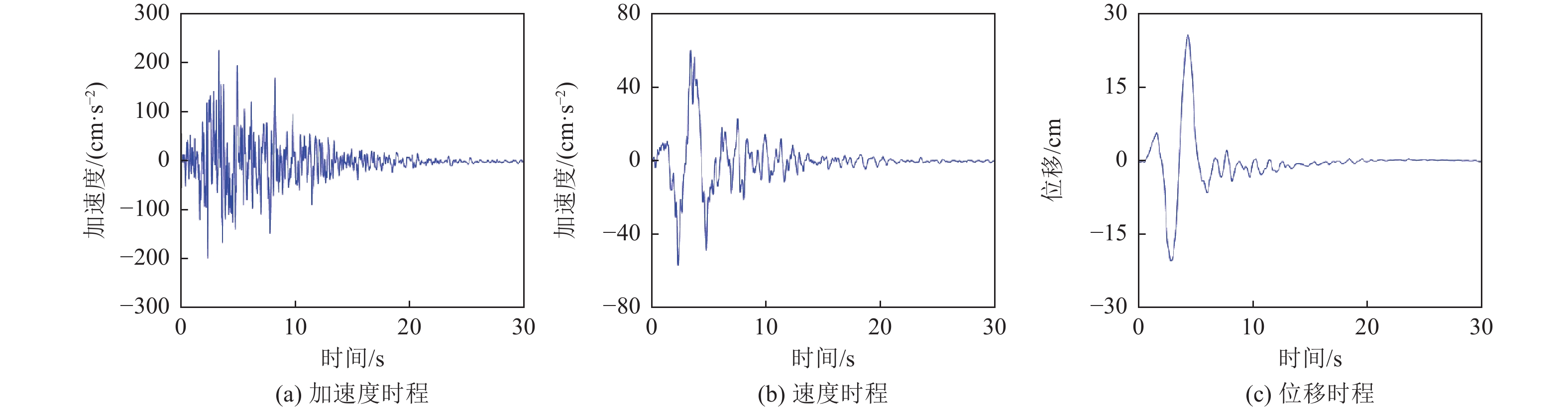
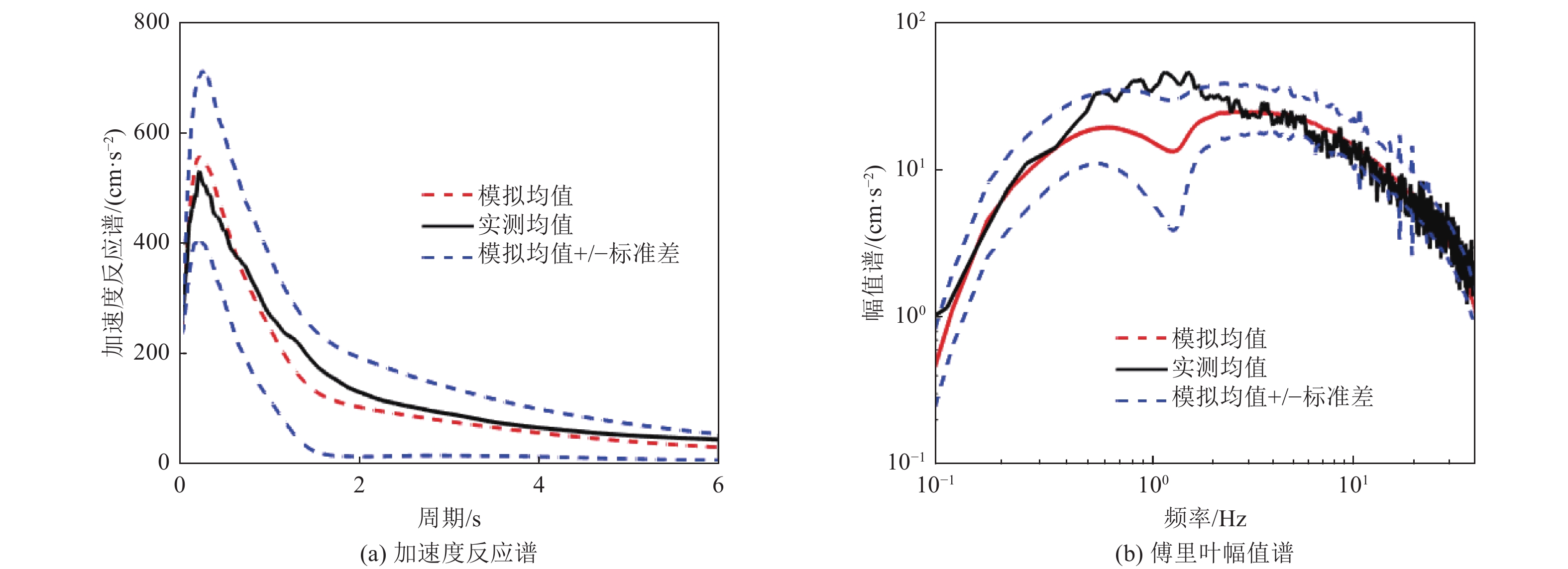
摘要: 根据50组近断层脉冲型强震动记录，采用连续小波变换提取最强速度脉冲分量，建立最强速度脉冲峰值时刻的统计模型。对近断层地震动加速度高频分量的演变功率谱模型参数进行识别，并利用谱表示-随机函数方法实现了降维模拟，进而积分得到速度高频分量。对脉冲参数进行随机化处理，并采用改进Gabor小波模型随机模拟速度低频分量。将速度高频分量与低频分量叠加得到近断层地震动速度时程。数值算例表明，近断层地震动加速度代表性时程集合的幅值谱和反应谱均与实测记录拟合一致，验证了降维模拟方法的工程适用性。近断层脉冲型地震动的降维模拟与概率密度演化理论相结合，可实现工程结构的随机地震反应与抗震可靠性精细化分析。Abstract: Based on 50 groups of near-fault pulse-like ground motion records, the strongest velocity pulse component was extracted by continuous wavelet transform, and the statistical model of the peak time of strongest velocity pulse was established. At the same time, the parameters of the evolution power spectrum model of the high frequency component of near-fault ground motion acceleration are identified, and its dimension reduction simulation is realized by spectral representation-random function method, and then the high frequency component of velocity is obtained by integrating. Secondly, the pulse parameters are randomized and the improved Gabor wavelet model is used to simulate the low-frequency component of velocity. Finally, the process of near-fault ground motion velocity is obtained by superposing them. Numerical examples show that the amplitude spectrum and response spectrum of the representative time history set of near-fault ground motion acceleration are consistent with the measured records, which verifies the engineering applicability of the proposed method. The combination of dimension reduction simulation of near-fault pulse-like ground motion and probability density evolution theory can realize the subtle analysis of random seismic response and seismic reliability of engineering structures.
-
. 引言
管理者经常面临着与决策相关信息缺失和不相关信息泛滥的问题，往往会对管理者的决策造成负面影响（Detrick，2002）。此情况在地震灾害应对过程中尤为突出，信息缺失或冗余往往造成抗震救灾指挥决策的滞后，甚至导致救援力量和资源投放重点出现偏差。
近年来，中国地震局在应急救援领域先后开展了“九五首都圈防震减灾示范项目”“十五中国数字地震观测网络项目”和“国家地震社会服务工程”。应急触发、灾情研判、快速响应及辅助决策等应急科技产出的日益丰富为国家及各省抗震救灾指挥部实施地震应急救援提供有力的科学依据和技术支持。我国虽建成了较完整的应急指挥体系及相应的指挥技术系统，但在应急信息管理方面仍存在一些问题，具体表现为：①技术产出较丰富，直接有效利用率较低；②内容重复，存放分散；③尚未建立有效的灾情管理技术。
为此国内不少专家学者对地震应急基础信息及灾情信息的收集、整理与分类编码进行了大量研究。付继华等（2009）、聂高众等（2002）从建立数据库的角度分别讨论了地震应急数据的分类。《地震学专业分类表》（梁凯利等，2011）严格按照《中国图书馆分类法》的要求，结合地震科技资料分类的自身特点，对地震学专业进行了分类；白仙富等（2010）按照信息内容的本质属性，依据发生什么事件、产生什么影响、对产生的影响人们做出什么响应、针对响应有何成效的思路对地震应急现场信息进行分类；张翼等（2016）根据地震应急信息产品管理、更新及共享的需要，针对地震应急信息产品属性、服务、时间、传递等特性，在借鉴地震应急基础理论研究及相关行业分类标准的基础上，研究地震应急信息产品的分类方法。
但对于多渠道的上传机制，加之震后大量的灾情及背景信息，使信息归类难度较大。面对紧迫的时效性压力和不同指挥决策部门对信息的不同需求，仅靠人工手动进行信息分类提取的方式难以达到令人满意的效果，因此建立条理更为清晰、标准更具实践应用意义、信息自动化程度更高的信息分类管理技术十分必要，以适应应急指挥决策部门对应急救援信息的快速获取要求。
林子雨等（2010）根据关系数据库的关键词查询问题研究背景，阐述解决该问题的基于模式图和数据图的优缺点、困难和挑战，提出利用排序函数解决关键词查询时匹配结果可能很多的情况，最终反馈给用户一个最相关信息。张晓民（2017）设计了基于关键词数据库信息检索方法及时态检索算法，主要采用时间修剪策略，同时提出时态边权重的计算方法，实现了基于关键词的关系数据库时态检索原型系统。通过借鉴关键词在信息检索中的应用，本文将关键词分类法应用于地震应急信息管理中。
1. 地震应急信息分类方法
信息分类方法主要包括线分类法、面分类法、混合分类法（耿庆斋等，2014）。现有与地震信息分类有关的标准与研究多采用线分类法，其特点是层次较清晰，易于理解；缺点是结构可塑性较差，一旦分类深度和每层级类目容量固定后，修改层级和插入新类将受限（刘若梅等，2004）。面分类法将选定的分类对象若干属性或特征视为若干个“面”，每个“面”中又可分成彼此独立的若干个类目，对于解决同种类型要素在不同应用中分类的矛盾具有优势。
参考不同分类方法（杨天青等，2016；和锐等，2011），考虑自动分类结果的时效性与实用性，本文采用线与面相结合的混合分类法，以信息服务的高效便捷为目的，按照应急信息自身的特征属性、地震发生时间线产生的直接与间接损失信息（即震前、震时与震后所造成的破坏与损失信息），针对产生的影响采取相应的应急救援信息，将地震应急信息分为震前基础背景信息、地震震情灾情信息、震后应急救援信息，如表 1所示。
表 1 地震应急信息分类定义Table 1. Definition of classification of seismic emergency information信息类别 定义 内容概述 意义 震前基础
背景信息震区客观存在的各类自然
灾害、基础社会信息震区人文、构造，救援力量背景、基础设
施设备，自然灾害等对地震应急行动起宏观的参
考价值地震震情
灾情信息地震自身具有的动态性、可
变性的各类自然属性特征与
其间接造成的破坏或损失信息地震发生的时间、地点、震级、余震监测
息、强震动监测信息、震情发展趋势判断、
成因分析及地震烈度分布、房屋破坏与人口
伤亡信息、次生灾害信息等判断地震灾害的规模和成灾
方式，直接控制地震应急救援
行动的总体规模和方式震后应急
救援信息为应对地震事件、处理地震
灾害而采取的应急救援信息应急救援决策信息、救援调度信息、指挥
部工作状态、现场工作状态、具有相关参考
性的历史救援案例信息等掌握救援行动的进展，动态
调整救援方案2. 地震应急信息自动分类方法的研究
（1）通过实地调研河北省、山西省、内蒙古自治区、四川省的基本人文地理环境信息概况，本文选择收集四川省4次地震应急资料的主要原因为：1）对同一省份的地震应急资料进行文档分词处理时，可直接忽略地名类固定性且不具实际区分意义的属性词，且同一省份文本文档之间的语义描述差异性相对较小；2）相对于地震易发的其他3个省来说，四川省地势地形地貌相对较复杂，建筑物水库大坝等公共基础设施种类结构相对复杂，且抗震救灾技术较成熟，从而使得到的信息更丰富和全面；3）四川省已建成一套独立的信息上传与协同管理体系，有助于提高资料分析和研究的准确性。
（2）应急信息资料分析统计
共收集2013年4月20日芦山7.0级地震、2014年11月22日康定6.3级地震、2017年8月8日九寨沟7级地震、2017年9月30日广元青川5.4级地震资料，由于收集到的数据较零散，且震级较小的数据资料较少，所以本文将4次地震中相同类别的信息统计在同一文件夹下，如表 2所示。
表 2 信息文档分类统计Table 2. lassification statistics of information documents类别 文档数 震前基础背景信息 12 地震震情灾情信息 405 震后应急救援信息 138 总文档量 555 （3）应急信息分类关键词的选取
中文分词(Chinese Word Segmentation）指将一个汉字序列切分成一个一个单独的词，作为文本挖掘的基础，对输入的一段中文进行中文分词，可达到自动识别语句含义的效果（赵小华，2010）。
TF词频(Term Frequency）指某一个给定的词语在该文件中出现的次数。IDF反文档频率(Inverse Document Frequency）的主要思想是：如果包含词条的文档越少，IDF越大，则说明词条具有很好的类别区分能力。TF-IDF是一种用于信息搜索和信息挖掘的常用加权技术，在搜索、文献分类和其他相关领域中的应用较为广泛（施聪莺等，2009）。
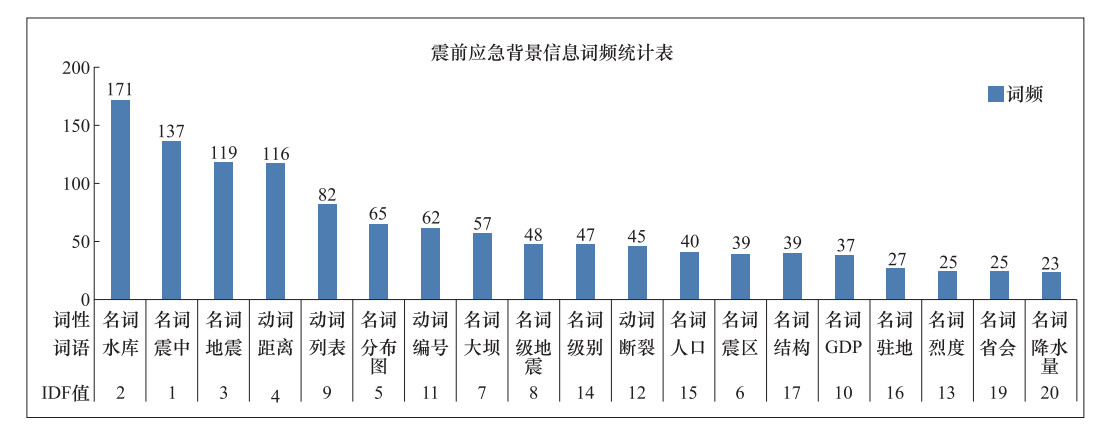
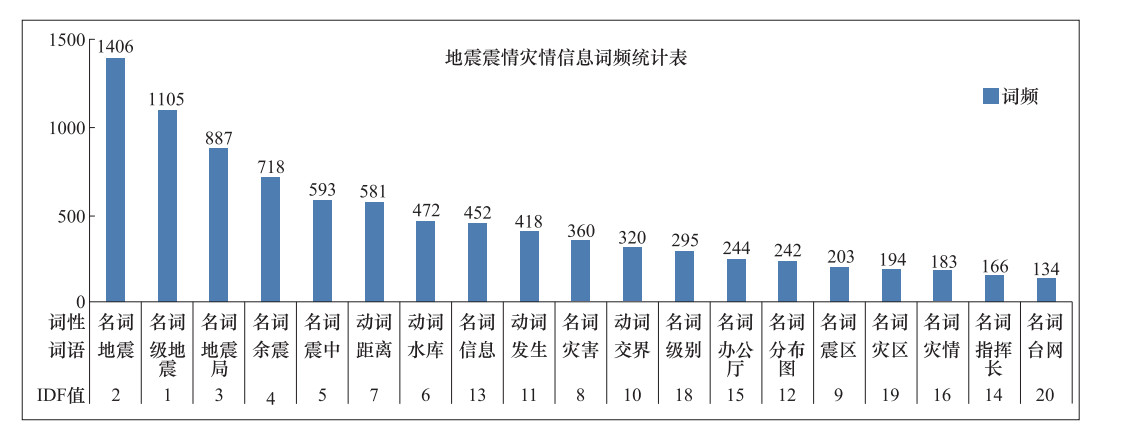
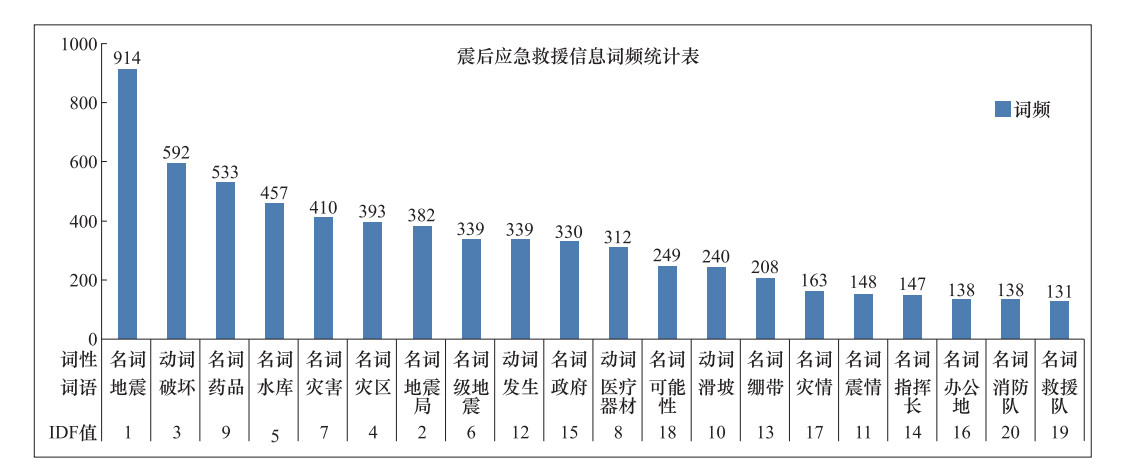
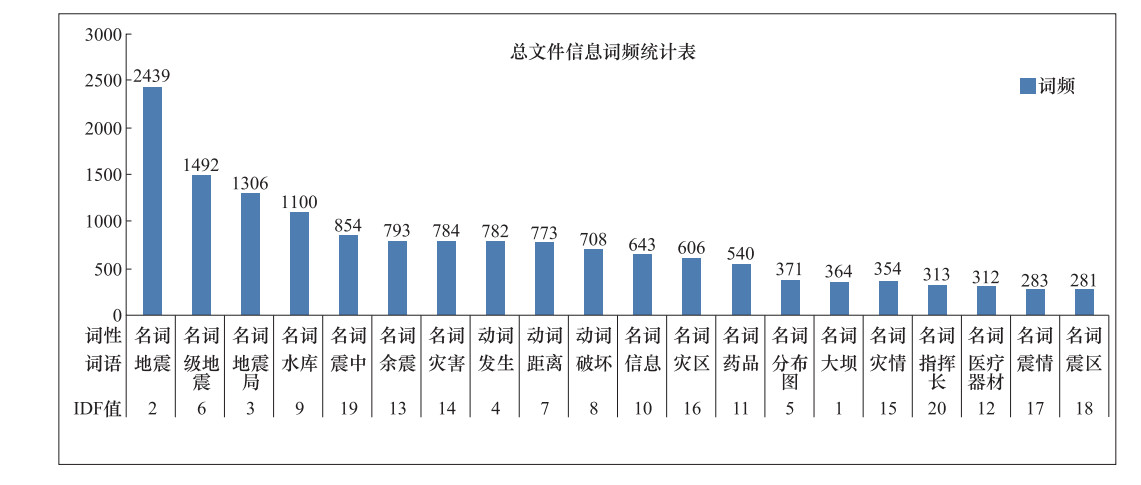
本文在对文本信息进行分析处理时，根据建立的分类标准，对收集到的信息进行分类，应用TF-IDF技术，在Excel表里对各类文本信息进行分词和词频统计。此种方法的局限是处理的文档只能是文本文档（.txt）格式。按名词和动词的词性，统计IDF和词频数排名前20的词，如图 1-3所示。
图 1 震前应急背景信息词频统计Figure 1. Frequency statistics of emergency background information before earthquake
图 3 震后应急救援信息词频统计Figure 3. Frequency statistics of emergency rescue information after earthquake由图 4可知，地震、级地震、地震局、水库4个词语的出现总频数超过1000，其中地震出现频数高达2439。各类别信息里的频数具体为：震区背景信息119次、震区震情灾情信息1105次、灾区应急救援信息914次，占各类别信息前20频数的比例分别为9%、15%、13%，在总文档里所占比例为16%，平均出现频率占12.3%。
频数为700—1000的词语共6个，分别为震中854次、余震793次、灾害784次、发生782次、距离773次、破坏708次，占所有词频的比例为4.7%—5.7%，其中发生和灾害2个词语的频数相差2，在进行词语筛选时，任选其一即可。
频数为300—700的词语共8个，其中400以上的有3个，分别为信息643次、灾区606次、药品540次；其余5个为分布图、大坝、灾情、指挥长、医疗器材，频数为300—400。8个词语从分类属性来看，主要属于应急救援信息，占总文档词语的比例为2%—4%。
整体来看，出现频率越高的词语，在分类过程中起到的作用越低，即作为关键词的代表性越不强，本文最终选取的各类别信息关键词是在各类信息词语统计里频率不高且在其他类别信息里频率较低或没有的词语。根据频数统计规律可知，本文关键词的取舍主要按以下规则：①对4个频数数据按词语词频占所有20个词语词频的比例，将频率域划分为2%以下、2%—4%、4%—6%、6%—8%、8%五个区间；②按各类信息的定义，每个区间选取一个词（选取与本类信息最相关的词语）作为3类信息的基础关键词。如第一区间地震局、第二区间水库、第三区间破坏、第四区间灾情、第五区间震情，这个组合归至震情灾情信息类；③每个区间选取2—4个固有关键词，与基础关键词重合的排除，低频率区间的词语多选，重复词语与高频词语尽量不选，最终每类信息选出15个关键词，如表 7所示。
3. 地震应急信息的自动分类
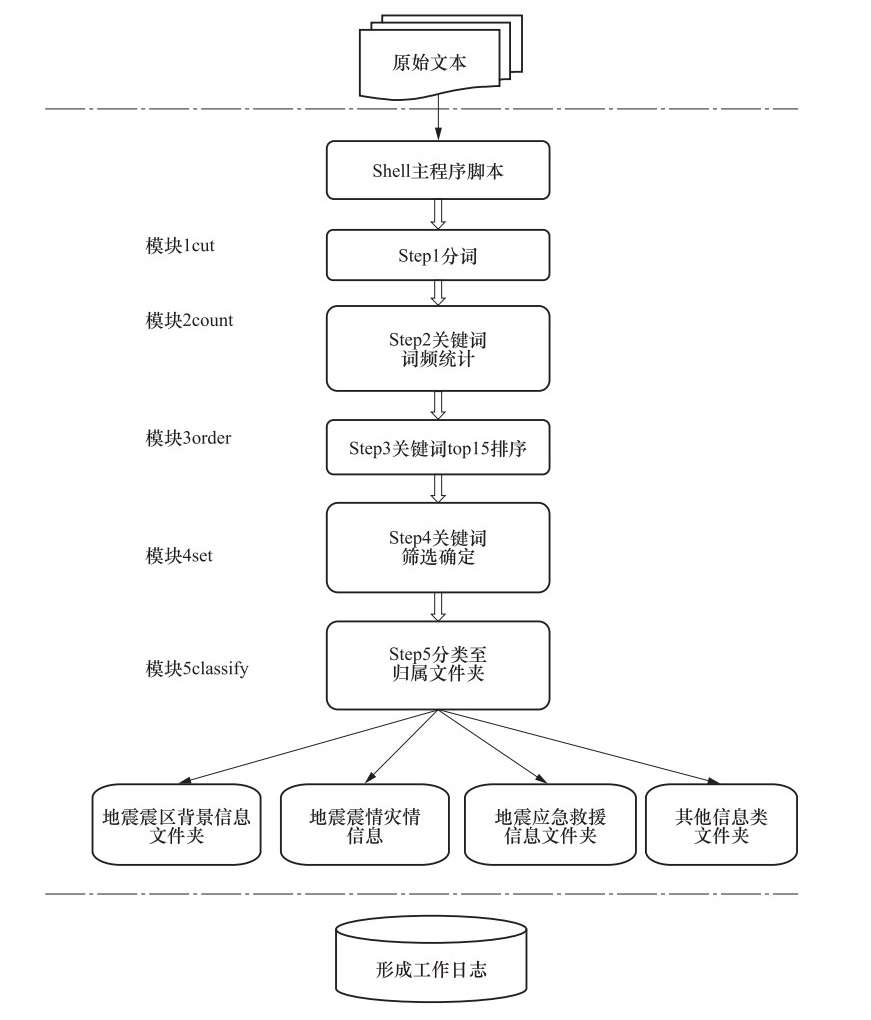
百度、谷歌等搜索引擎成功显示出关键词检索的方式已被广大用户所接受（张晓民，2017）。本文为解决应急信息的自动分类，采用“关键词分类法”，根据分类标准，对原始文本进行结构化处理，通过中文分词、词频筛选与统计实现信息关键词的提取，此阶段中的中文分词将一串连续汉字序列按动词、名词的规范重新组合成词语序列。词频统计与筛选即对分词结果进行统计，去除一些无效词后，生成关键词词库，用匹配词库的方法实现信息的自主分类，具体过程如下：①收集震后国家中心、各研究所、各省（自治区）地震局上传至应急信息共享平台、评比FTP站点、台网中心台网部FTP站点的震后产出成果，建立相对完整的产出目录；按照之前建立的地震应急信息分类标准，对收集到的条目进行梳理归类。②对所有文档按词性进行词频统计，将无效词语去除后，对每个大类建立相应的关键词词库。由于高频词语的重合度较高，因此在建立关键词词库时，需综合考虑词频和词语含义，首选该分类独有且出现频率较高的词语。③以提取的特征词作为自动分类程序中的词库，进行自动分类处理，在计算机语言的基础上，实现信息的自动分类。要求程序在震后启动，自动完成当前地震产生在各不同平台上的信息分类，并将产出成果保存至本地服务器。根据已建立的分类类别和各应急指挥部门需求，可进一步实现对产出成果的重命名（非必要）和重新分发。分类流程如图 5所示。
以九寨沟7.0级地震产出为例：
报告及图件总数如表 8所示。分类文件夹包括震前背景信息文件夹、震区灾情震情信息文件夹、震后应急救援信息文件夹和其他文件夹。
建立的分类词库较简单，结果与表 3的关键词库高度匹配。震前背景信息特征词包括构造、交通、居民点、GDP、人口等，地震震情灾情信息特征词包括截止、余震、热力图、震动、态势、数据、精密、水准、伤亡、灾害、中央电视台、设防、展开、遇难等，震后应急救援信息特征词包括救援、救援队、搜救等。
表 3 关键词选取结果Table 3. Keyword selection results震前基础背景信息 地震灾情震情信息 震后应急救援信息 地震 级地震 地震局 水库 水库 水库 距离 截止 药品 大坝 交界 政府 震中 震情 绷带 人口 震中 搜救 结构 余震 消防队 烈度 灾害 救援队 省会 灾情 医疗器材 分布图 大坝 办公地 降水量 经度 发生 断裂 灾区 可能性 GDP 防震减灾 食品 房屋 发生 震情 震源 烈度 灾区 分类标准建成后，以提取的关键词作为自动分类程序中的词库，进行自动分类，流程如图 6所示。分类过程中各环节为：①将所有格式文档转为.txt格式文件，并输出至原始文件夹；②搭建主程序运行环境（Python2.7环境、jieba程序库）；③运行shell主程序，调用Python子程序模块，将原始文件夹下的所有文件进行分类处理。模块1（cut）：获得文件对文件进行分词，并将其存至临时文件夹；模块2（count）：对原文件进行词频统计，并对统计结果进行排序；模块3（order）：分词词频统计排序前15的词进行排序；模块4（set）：根据各类关键词筛选结果，得到关键词库；模块5（classify）：将初始文档进行结构化处理后得到的前15词频作为该文档的关键词，将其与关键词库进行对比，通过文档关键词在所划分的5个频率域区间的关键词库匹配率决定文档的归属类别，将文档划分至匹配率最高的类别。判断该关键词属于哪个分类，按照文件归属，把文件归类至该目录下。某个文件可能属于多个类别，如果没有对应的目录，则把文件拷贝至其他文件夹。
表 4 报告及图件总数Table 4. Total number of reports and artworks信息文件类别 文档 图件 文件总量 震前基础背景信息 21 0 21 地震震情灾情信息 119 13 132 震后应急救援信息 7 0 7 4. 总结和应用探讨
目前我国地震应急信息是通过各省、市已建立的信息汇总渠道直接上传至相关服务平台，供指挥部及相关领导专家参阅，但大地震发生后面对的是大量灾情震情救援及背景信息，仅靠上述传输和提取方式不能达到令人满意的程度。本文的研究成果可实现多渠道应急信息的自动分类，辅助地震应急指挥控制与决策等。
（1）参考以往学者在地震应急信息分类与编号方面的研究，考虑分类信息的服务实用性，根据地震事件发生的时间轴，将地震应急信息分为震前应急背景信息、地震应急震情灾情信息和震后应急救援信息。
（2）为实现地震应急信息的自动分类，研究采用“关键词分类法”，以实现地震应急信息的自动分类，提高信息处理的目标性、针对性和有效性。
（3）通过分析，本文对应急信息进行分类、分词、词频统计，由前15位关键词信息统计结果可知，各不同类别应急信息关键词之间存在较大差异，可见与传统信息直接上传法相比，“关键词分类法”能使信息条理性更强，分析处理时更方便直接。
（4）在大数据的背景下，相比于传统的信息分类方法，实现地震应急信息的自动分类，将大大提高信息利用率，并推动地震应急救援相关技术走向智能成熟化、自动服务化。
但对于有效应用关键词分类法实现应急信息的自动分类、降低某个文件可能属于多个类别的交叉情况，仍存在以下问题：
（1）如何建立关键词之间的语义关系和逻辑关联关系，处理并不断丰富分类关系树，还需对信息自身与信息相互之间更深层次的关联关系进行探讨，如时态上或语义上。
（2）对于关键词重复和冗余问题，目前只有少数研究提出了初步解决方案，还需结合信息自身的属性、信息之间的差异及用户对信息的需求，由相关函数（如排序函数）探索建立一个权衡的标准。
-
图 1
$ {t_{{\text{pk}}}} $ 与$ {M_{\text{W}}} $ 回归模型Figure 1. Regression model between peak time
$ {t_{{\text{pk}}}} $ of the strongest velocity pulse and magnitude$ {M_{\text{W}}} $ 
图 3 低频脉冲模型参数的经验分布函数
Figure 3. Empirical distribution functions of low- frequency pulse model parameters
图 4 近断层地震动高、低频分量代表性时程
Figure 4. Representative time-history of high and low frequency components of near-fault ground motions
图 5 近断层地震动高、低频分量代表性样本集合均值、标准差的目标值与模拟值比较
Figure 5. Comparison of mean, standard deviation of representative time-history set of high and low frequency components upon near-fault ground motions with the target values
图 6 近断层地震动的代表性时程
Figure 6. The representative time-histories of near-fault ground motions
图 7 近断层地震动加速度反应谱、幅值谱模拟值与实测值的比较
Figure 7. Comparison of simulated value and measured value of response spectrum and amplitude spectrum of near-fault ground motion acceleration
表 1 近断层脉冲型地震动记录信息
Table 1. Information of the measured records of near-fault pulse-like ground motions
RSN
编号矩震级
MW断层距
R/km脉冲周期
Tp/s脉冲峰值
PGV/（cm·s−1）脉冲峰值
时刻tpk/sRSN
编号矩震级
MW断层距
R/km脉冲周期
Tp/s脉冲峰值
PGV/（cm·s−1）脉冲峰值
时刻tpk/s150 5.7 3.10 1.23 49.60 2.725 1161 7.5 10.90 5.99 53.00 6.100 159 6.5 0.70 2.34 53.50 7.650 1176 7.5 4.80 4.95 90.60 11.750 161 6.5 10.40 4.40 36.70 8.880 4040 6.6 1.70 2.02 124.20 17.860 170 6.5 7.30 4.42 70.80 7.390 4065 6.0 2.90 1.22 35.80 4.915 171 6.5 0.10 3.42 116.40 4.980 4098 6.0 3.00 1.33 51.60 3.220 173 6.5 8.60 4.52 55.20 7.445 4100 6.0 3.00 1.08 57.90 3.185 178 6.5 12.90 4.50 55.80 8.210 4101 6.0 5.50 0.52 31.00 2.720 179 6.5 7.00 4.79 80.80 6.730 4102 6.0 3.60 1.02 43.50 3.115 180 6.5 4.00 4.13 96.50 7.195 4103 6.0 4.20 0.70 38.30 2.970 181 6.5 1.40 3.77 121.60 7.300 4107 6.0 2.50 1.19 81.90 3.445 182 6.5 0.60 4.38 111.90 5.880 4113 6.0 2.90 1.13 27.00 2.930 184 6.5 5.10 6.27 73.50 7.455 4115 6.0 2.60 1.19 56.50 3.120 185 6.5 7.50 4.82 73.40 7.075 4126 6.0 3.80 0.57 43.40 2.305 316 5.9 16.70 4.39 60.80 10.090 6887 7.0 18.10 12.60 59.90 31.130 451 6.2 0.50 1.07 76.80 3.585 6897 7.0 8.50 7.83 65.90 25.460 459 6.2 9.90 1.23 37.30 5.835 6911 7.0 7.30 9.92 106.10 24.840 568 5.8 6.30 0.81 68.30 1.405 6927 7.0 7.10 7.37 116.50 25.270 723 6.5 0.90 2.39 143.90 12.200 6928 7.0 25.70 10.60 30.20 21.590 838 7.3 34.90 9.13 28.80 14.960 6942 7.0 26.80 8.04 56.50 28.090 879 7.3 2.20 5.12 132.30 12.100 6960 7.0 13.60 9.39 63.80 26.220 900 7.3 23.60 7.50 55.80 18.440 6962 7.0 1.50 7.14 85.70 25.390 1106 6.9 1.00 1.09 105.60 7.820 6966 7.0 22.30 8.76 65.70 26.520 1114 6.9 3.30 2.83 103.00 9.850 6969 7.0 20.90 9.35 64.40 27.770 1119 6.9 0.30 1.81 95.60 5.390 6975 7.0 6.10 8.93 74.10 27.480 1120 6.9 1.50 1.55 153.20 6.170 8161 7.2 11.30 8.72 72.60 39.510 
下载: 导出CSV
表 2 近断层地震动高频分量模型参数取值
Table 2. Simulation parameters of high-frequency component in near-fault ground motion
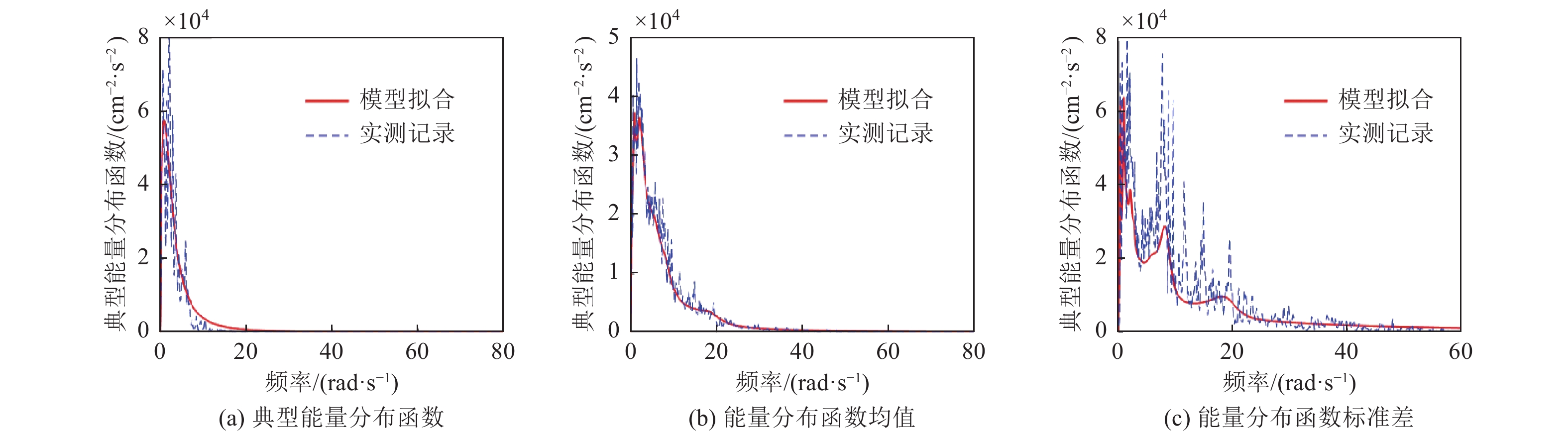
参数 取值 参数 取值 频率离散点数N 1 600 地震动峰值加速度${ \overline{A}}_{\mathrm{max}} $ 240 cm·s−2 截止频率上限$ \omega_{{\rm{u}}} $ 50π rad·s−1 峰值因子$ r $ 2.6 截止频率下限$ \omega_{1} $ 2π rad·s−1 场地土卓越圆频率$ \omega_{{\rm{g}}} $ 15.7 rad·s−1 频率离散步长$ \Delta \omega $ 0.094 rad·s−1 场地土阻尼比$ \zeta_{{\rm{g}}} $ 0.887 高、低频分界限$ f_{{\rm{r}}} $ 2π rad·s−1 基岩卓越圆频率$ \omega_{{\rm{f}}} $ 1.57 rad·s−1 地震动持时$ T $ 30 s 基岩阻尼比$ \zeta_{{\rm{f}}} $ 0.887 时间步长$ \Delta t $ 0.02 s 样本数量$ n_{{\rm{sel}}} $ 1 069
下载: 导出CSV
表 3 近断层地震动低频脉冲成分模型参数取值
Table 3. Simulation parameters of low-frequency component in near-fault ground motion
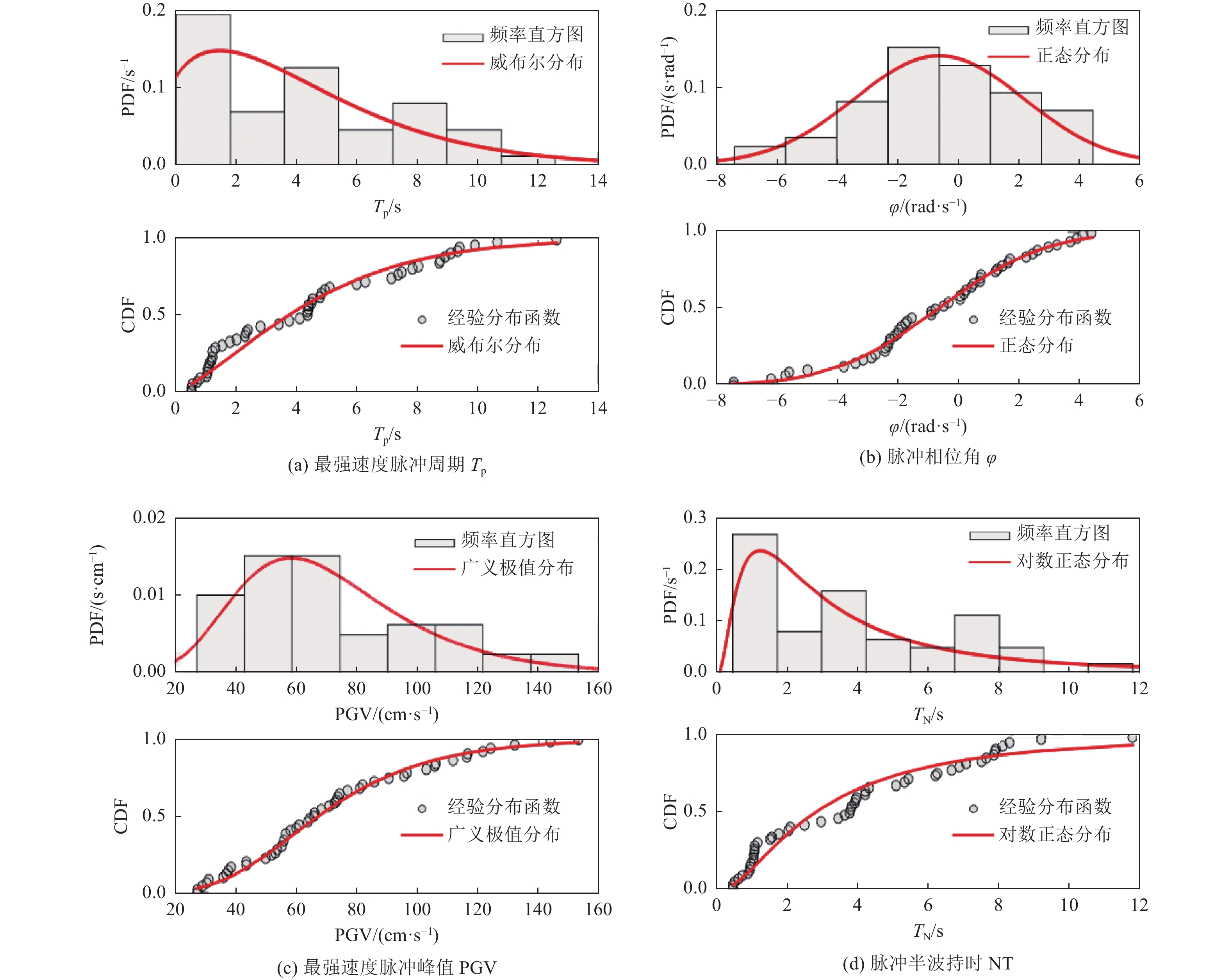
模型参数 概率分布 概率分布系数 TN 对数正态分布 M1=1.028 1，S1=0.903 4 $ \varphi $ 正态分布 M2=−0.66，S2=2.80 PGV 广义极值分布 k=0.008 7，Sigma=24.64，Mu=58.47 $T_{\rm{p}} $ 威布尔分布 Sc=4.998 4，Sa=1.405 5 注：M1、S1分别为对数正态分布的均值和标准差；M2、S2分别为正态分布的均值和标准差；Sigma、Mu、k分别为广义极值分布的尺度参数、位置参数和形状参数；Sc和Sa分别为威布尔分布的尺度参数和形状参数。
下载: 导出CSV
-
陈笑宇, 王东升, 付建宇 等, 2021. 近断层地震动脉冲特性研究综述. 工程力学, 38（8）: 1—14, 54Chen X. Y. , Wang D. S. , Fu J. Y. , et al. , 2021. State-of-the-art review on pulse characteristics of near-fault ground motions. Engineering Mechanics, 38(8): 1—14, 54. (in Chinese) 范增磊, 2017. 非平稳地震动演变功率谱的估计与建模研究. 秦皇岛: 燕山大学.Fan Z. L. , 2017. Study on evolution of non-stationary seismic ground motion estimation and modeling of power spectrum. Qinhuangdao: Yanshan University. (in Chinese) 贾路, 阮鑫鑫, 刘章军, 2019. 近断层脉冲型地震动的降维模拟. 地震研究, 42（4）: 516—522Jia L. , Ruan X. X. , Liu Z. J. , 2019. Dimension reduction simulation for near-field fault pulse-like ground motion. Journal of Seismological Research, 42(4): 516—522. (in Chinese) 姜云木, 阮鑫鑫, 刘章军, 2021. 主余震型地震动过程的降维模拟. 振动与冲击, 40（24）: 282—292Jiang Y. M. , Ruan X. X. , Liu Z. J. , 2021. Dimension-reduction simulation of main aftershock type ground motion process. Journal of Vibration and Shock, 40(24): 282—292. (in Chinese) 李华聪, 钟菊芳, 2021. 最强脉冲方向分量的周期特性及其影响因素分析. 地震研究, 44（1）: 96—104Li H. C. , Zhong J. F. , 2021. Analysis on the period characteristics of the strongest pulse direction component and its influence factors. Journal of Seismological Research, 44(1): 96—104. (in Chinese) 李启成, 杜玉春, 严冬冬 等, 2013. 基于改进的经验格林函数方法的地震动模拟. 地震研究, 36（1）: 74—80Li Q. C. , Du Y. C. , Yan D. D. , et al. , 2013. Ground motion simulation based on improved empirical Green’s function method. Journal of Seismological Research, 36(1): 74—80. (in Chinese) 刘启方, 袁一凡, 金星 等, 2006. 近断层地震动的基本特征. 地震工程与工程振动, 26（1）: 1—10Liu Q. F. , Yuan Y. F. , Jin X. , et al. , 2006. Basic characteristics of near-fault ground motion. Earthquake Engineering and Engineering Vibration, 26(1): 1—10. (in Chinese) 刘章军, 刘增辉, 刘威, 2017. 全非平稳地震动过程的概率模型及反应谱拟合. 振动与冲击, 36（2）: 32—38Liu Z. J. , Liu Z. H. , Liu W. , 2017. Probability model of fully non-stationary ground motion with the target response spectrum compatible. Journal of Vibration and Shock, 36(2): 32—38. (in Chinese) 罗全波, 陈学良, 高孟潭 等, 2018. 近断层速度脉冲与震源机制的关系浅析. 震灾防御技术, 13（3）: 646—661Luo Q. B. , Chen X. L. , Gao M. T. , et al. , 2018. Relationship between near-fault velocity pulse and focal mechanism. Technology for Earthquake Disaster Prevention, 13(3): 646—661. (in Chinese) 田玉基, 杨庆山, 卢明奇, 2007. 近断层脉冲型地震动的模拟方法. 地震学报, 29（1）: 77—84Tian Y. J. , Yang Q. S. , Lu M. Q. , 2007. Simulation method of near-fault pulse-type ground motion. Acta Seismologica Sinica, 29(1): 77—84. (in Chinese) 王宇航, 2015. 近断层区域划分及近断层速度脉冲型地震动模拟. 成都: 西南交通大学.Wang Y. H., 2015. The near-fault region zoning and near-fault velocity pulse-like ground motion simulation. Chengdu: Southwest Jiaotong University. (in Chinese) 魏勇, 崔建文, 王秋良 等, 2018. 基于合成地震动的2014年鲁甸MS6.5地震场地效应分析. 地震研究, 41（1）: 32—37Wei Y. , Cui J. W. , Wang Q. L. , et al. , 2018. Analysis on site effect of 2014 Yunnan Ludian MS6.5 earthquake based on simulating ground motion. Journal of Seismological Research, 41(1): 32—37. (in Chinese) 杨福剑, 王国新, 2019. 一种改进的近断层脉冲型地震动模拟方法. 震灾防御技术, 14（3）: 489—500 doi: 10.11899/zzfy20190303Yang F. J. , Wang G. X. , 2019. An improved approach for near-fault pulse-like ground motion simulation. Technology for Earthquake Disaster Prevention, 14(3): 489—500. (in Chinese) doi: 10.11899/zzfy20190303 杨庆山, 田玉基, 2014. 地震地面运动及其人工合成. 北京: 科学出版社.Yang Q. S., Tian Y. J., 2014. Earthquake ground motions & artificial generation. Beijing: Science Press. (in Chinese) 赵晓芬, 温增平, 陈波, 2018. 近断层地震动最强速度脉冲方向分量特性研究. 地震学报, 40（5）: 673—688 doi: 10.11939/jass.20170178Zhao X. F. , Wen Z. P. , Chen B. , 2018. Characteristics of near-fault velocity pulses in the strongest pulse orientation. Acta Seismologica Sinica, 40(5): 673—688. (in Chinese) doi: 10.11939/jass.20170178 赵晓芬, 温增平, 谢俊举等, 2021.2018年台湾花莲MW6.4地震近断层地震动方向性差异. 振动与冲击, 40（10）: 235—243Zhao X. F. , Wen Z. P. , Xie J. J. , et al. , 2021. Ground motion directionality in the 2018 Taiwan Hualien MW 6.4 earthquake. Journal of Vibration and Shock, 40(10): 235—243. (in Chinese) Bray J. D. , Rodriguez-Marek A. , 2004. Characterization of forward-directivity ground motions in the near-fault region. Soil Dynamics and Earthquake Engineering, 24(11): 815—828. doi: 10.1016/j.soildyn.2004.05.001 Clough R. W. , Penzien J. , 2003. Dynamics of structures. 2 nd ed. Berkeley: Computers and Structures, Inc. , 65—66. Deodatis G. , Shinozuka M. , 1989. Simulation of seismic ground motion using stochastic waves. Journal of Engineering Mechanics, 115(12): 2723—2737. doi: 10.1061/(ASCE)0733-9399(1989)115:12(2723) Dickinson B. W. , Gavin H. P. , 2011. Parametric statistical generalization of uniform-hazard earthquake ground motions. Journal of Structural Engineering, 137(3): 410—422. doi: 10.1061/(ASCE)ST.1943-541X.0000330 Howard J. K. , Tracy C. A. , Burns R. G. , 2005. Comparing observed and predicted directivity in near-source ground motion. Earthquake Spectra, 21(4): 1063—1092. doi: 10.1193/1.2044827 Kalkan E. , Kunnath S. K. , 2006. Effects of fling step and forward directivity on seismic response of buildings. Earthquake Spectra, 22(2): 367—390. doi: 10.1193/1.2192560 Li J. , Chen J. B. , 2006. The probability density evolution method for dynamic response analysis of non-linear stochastic structures. International Journal for Numerical Methods in Engineering, 65(6): 882—903. doi: 10.1002/nme.1479 Li J. , Chen J. B. , 2007. The number theoretical method in response analysis of nonlinear stochastic structures. Computational Mechanics, 39(6): 693—708. doi: 10.1007/s00466-006-0054-9 Liang J. W. , Chaudhuri S. R. , Shinozuka M. , 2007. Simulation of nonstationary stochastic processes by spectral representation. Journal of Engineering Mechanics, 133(6): 616—627. doi: 10.1061/(ASCE)0733-9399(2007)133:6(616) Liu Z. J. , Liu W. , Peng Y. B. , 2016. Random function based spectral representation of stationary and non-stationary stochastic processes. Probabilistic Engineering Mechanics, 45: 115—126. doi: 10.1016/j.probengmech.2016.04.004 Liu Z. J. , Liu Z. X. , Chen D. H. , 2018. Probability density evolution of a nonlinear concrete gravity dam subjected to nonstationary seismic ground motion. Journal of Engineering Mechanics, 144(1): 04017157. doi: 10.1061/(ASCE)EM.1943-7889.0001388 Mavroeidis G. P. , Papageorgiou A. S. , 2003. A mathematical representation of near-fault ground motions. Bulletin of the Seismological Society of America, 93(3): 1099—1131. doi: 10.1785/0120020100 Shahi S. K. , Baker J. W. , 2014. An efficient algorithm to identify strong‐velocity pulses in multicomponent ground motions. Bulletin of the Seismological Society of America, 104(5): 2456—2466. doi: 10.1785/0120130191 Vanmarcke E. H. , Lai S. S. P. , 1980. Strong-motion duration and RMS amplitude of earthquake records. Bulletin of the Seismological Society of America, 70(4): 1293—1307. Yang D. X. , Zhou J. L. , 2015. A stochastic model and synthesis for near-fault impulsive ground motions. Earthquake Engineering & Structural Dynamics, 44(2): 243—264. 期刊类型引用(8)
1. 白灵，黄猛，刘帅. 基于TextCNN的地震新闻标题分类方法. 信息技术与信息化. 2024(08): 91-94 . 
百度学术2. 孙鸿博，孙艺，赵楠，解惠婷，陆立，荆涛. 基于“三网一员”的震后灾情速报系统设计与应用. 华北地震科学. 2023(02): 1-7 . 百度学术3. 王斐斐，韩贞辉，樊华，宋鹏展. 基于开源GIS的地震灾情速报信息收集与处置研究——以河南省地震局为例. 震灾防御技术. 2022(01): 188-196 . 本站查看4. 李华玥，郑通彦，王尅丰，林向洋，文鑫涛. 基于地震信息的大屏可视化技术研究与应用. 中国地震. 2022(02): 293-303 . 百度学术5. 王钟浩，崔珂玮，张鑫，杨振中，刘帅. 基于fastText的地震信息文本分类方法. 现代信息科技. 2021(03): 5-8 . 百度学术6. 张翼，周妍，许娟，宴金旭，唐姝娅，陈维锋. 基于用户需求的地震应急信息服务特征研究. 震灾防御技术. 2021(01): 211-219 . 本站查看7. 李忠，杨百一，李莹，李晓丽. ALBERT与双向GRU的多标签灾情信息预测. 科学技术与工程. 2021(35): 15284-15289 . 百度学术8. 刘婉婷，杨新月，刘耀辉，魏本勇，齐文华，孙磊. 基于微博数据的西藏那曲比如县地震舆情分析. 震灾防御技术. 2021(04): 771-780 . 本站查看其他类型引用(1)
-
下载:
点击查看大图
计量
- 文章访问数: 137
- HTML全文浏览量: 20
- PDF下载量: 10
- 被引次数: 9
