Analysis of Seismic Response of Bridge across Earthquake Fault with Different Input Modes of Seismic Action
-
摘要: 大地震在近断层场地产生强烈地震动的同时,还会由于断层错动直接导致基岩甚至上覆土层破裂,在断层两侧产生显著差异性永久位移,造成位于断层附近或跨越断层的工程结构破坏。因此,跨断层桥梁面对的地震作用是断层两侧桥墩处场地的不同地震动,包括存在永久性位移的地震动。本文以垂直跨越走滑断层的多跨简支梁桥为例,基于OpenSees有限元模拟平台建立了桥梁结构的三维计算模型,计算分析了不同地震作用输入模式下桥梁结构的地震反应及其差异。考虑的地震作用模式包括:(1)断层两侧场地的地震作用视为相同的无永久位移的地震动,即无永久位移的一致地震动作用模式;(2)断层主动盘一侧场地的地震作用具有永久位移地震动,被动盘一侧采用无永久位移地震动,即具有永久位移的非一致地震动作用模式;(3)在断层主动盘一侧场地以静力方式施加断层错动位移,而被动盘一侧场地固定不动,即断层错动位移静力作用模式。计算结果分析表明,不考虑永久位移的一致地震动作用模式的地震动输入会导致严重低估桥梁反应计算结果,这也说明地震动的断层两侧永久性位移差异会显著增大桥梁结构反应;而一致地震动作用叠加断层错动永久位移静力作用的结果与非一致地震动作用模式的结果非常接近。为此,在某种程度上说,跨断层桥梁结构地震反应可采用一致地震动作用叠加断层错动位移静力作用的桥梁结构反应来近似模拟。Abstract: A large earthquake can produce strong ground motion at the near-fault site, and at the same time, it may directly break up the bedrock and even the overlying soil layers due to fault rupture, which leads to significant differential permanent displacement on both sides of the fault, and results in severe damage of the structure located near or crossing fault. Therefore, the seismic action on the bridge across fault is different on both sides of the fault, including the ground motion with permanent displacement. A simply supported girder bridge vertically across strike-slip fault is taken as an example, and a three-dimensional fnite-element model is developed using the earthquake engineering simulation software framework OpenSees (Open System for Earthquake Engineering Simulation). The seismic response of the bridge structure under different modes of seismic action is analyzed. The modes of seismic action considered include: (1) Seismic action of sites on both sides of the fault is regarded as the same ground motion without permanent displacement, that is, a consistent ground motion mode of seismic action without permanent displacement; (2) Seismic action of sites is regarded as a ground motion with permanent displacement on the active side of the fault, and a ground motion without permanent displacement on the passive side of the fault, that is, a non-consistent ground motion mode of seismic action with permanent displacement; (3) Fault dislocation displacement is applied to the site on the active side of fault, while the site on the passive side is fixed, that is, a static force mode of seismic action with fault dislocation displacement. The analysis results show that a consistent ground motion mode of seismic action without permanent displacement leads to a significant underestimation of the bridge structure responses, which indicates that the difference of permanent displacements of ground motions on both sides of the fault significantly increases the bridge structure response; The result from the superposition of the consistent ground motion mode and static force mode of fault dislocation permanent displacement is very close to the result from the non-consistent ground motion mode. Therefore, to a certain extent, the seismic response of cross-fault bridge structures can be approximately simulated by combining a consistent ground motion mode and a static force mode of fault dislocation displacement.
-
引言
1976年唐山大地震在唐山与天津地区引发了范围广、灾害重的液化震害。地震发生后,铁道部科学研究院等单位于1977、1978年对液化场地进行了钻孔勘察及静力触探测试(CPT)(刘恢先,1985)。当时使用的是单桥静力触探(以下简称“单桥CPT”)测试,在数据指标方面存在缺陷,与国际标准不接轨。单桥静力触探仅能提供比贯入阻力ps,不同的土层可能有相同的ps值,土层划分分辨率极低(孟高头等,2000)。因此,中国地震局工程力学研究所联合California Polytechnic State University及东南大学于2007年对上述唐山地区部分测点进行了现代多功能孔压静力触探(CPTU)测试(邱毅,2008;Moss等,2011)。CPTU测试在数据指标方面进行了补充,可提供锥尖阻力qc、侧壁摩阻力fs、摩阻比Rf(侧壁摩阻力fs与锥尖阻力qc的比值)及孔压u。相比单桥CPT,多了3项指标,在土层划分时分辨率较高,且有较丰富的经验和成熟的方法(刘松玉等,2013;董林等,2017,2018)。
对于CPTU测试数据,Moss等(2011)和Boulanger等(2014)先后给出了唐山地震CPT液化数据库。但是,由于CPTU测试与单桥CPT相距30年,唐山市经历了恢复重建,且改革开放后城市建设日新月异,地下水位变化及测点重新定位误差等均会影响新CPTU数据的代表性。因此,需对2007年得到的CPTU测试数据能否代表地震时的液化点与非液化点进行判断。本文对唐山大地震液化数据库进行检验,通过对比单桥CPT与CPTU测试数据沿深度的变化趋势,结合标贯击数随深度的变化趋势,判明场地力学特性是否一致。利用Robertson土质分类图,进行新CPTU数据土类分层检验,对比单桥CPT测试时钻孔柱状图,检验土层土类是否一致。通过土类筛选,并结合单桥CPT测试时标贯击数与CPTU测试锥尖阻力,选定液化层。将经过检验的液化数据库带入我国《岩土工程勘察规范》(GB 50021—2001)(中华人民共和国建设部,2004)液化判别方法,检验新CPT数据库的可信度。
1. 方法介绍
1.1 Robertson土质分类图
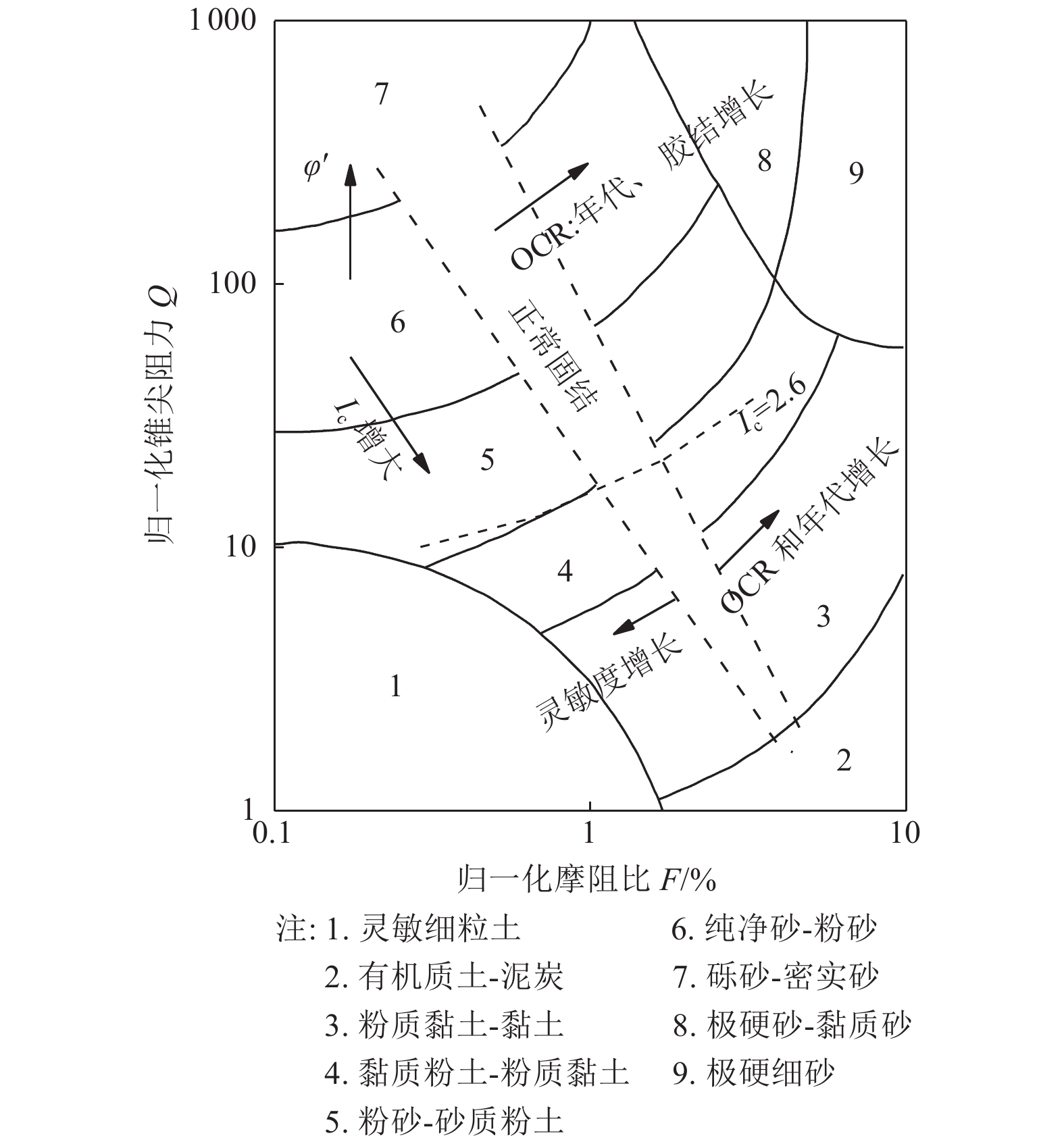
Robertson等(1998)基于大量现场实测数据与经验,建立了基于CPT指标的土质分类图(图1),根据摩阻比与土体细粒含量和塑性指数成正比的关系,构建了土类指数Ic,对于图1中土类2~7,Ic为5组同心圆的半径,按下式计算:
$$ {I_{\text{c}}} = {\left[ {{{\left( {3.47 - \log Q} \right)}^2} + {{\left( {1.22 + \log F} \right)}^2}} \right]^{0.5}} $$ (1) $$ Q = [\left( {{q_{\rm{c}}} - {\sigma _{{\rm{v}}}}} \right)/{P_{\rm{a}}}][{\left( {{P_{\rm{a}}}{\text{/}}\sigma _{{\rm{v}}}^{'}} \right)^n}] $$ (2) $$ F = \left[ {{f_{\rm{s}}}/\left( {{q_{\rm{c}}} - {\sigma _{{\rm{v}}}}} \right)} \right] \times 100\% $$ (3) 式中,Q为归一化锥尖阻力;F为归一化摩阻比;
$ {\sigma _{{\text{v}}}} $ 为总上覆压力;${\sigma '_{{\text{v}}}}$ 为有效上覆压力;Pa为1个标准大气压;n为应力指数。n值通过以下方法确定:首先假设n取为1.0,若计算得到Ic>2.6,则土为黏土,n取为1.0;若Ic<2.6,则n改取为0.5,重新计算Ic。若重新计算的Ic<2.6,则n为0.5;若Ic>2.6,则n取为0.7。
1.2 分层依据
由于CPTU测试与单桥CPT测试相距30年,考虑再次定位的误差、场地高程变化及地下各土层厚度沿水平方向变化的复杂性,本研究按照CPTU测试数据进行分层,将单桥CPT测试时钻孔柱状图与比贯入阻力沿深度的变化趋势作为参考。分层时首先利用锥尖阻力和比贯入阻力在砂层处突然变大、摩阻比变小(砂土摩阻比一般<2%,黏土摩阻比基本>3%)的特性确定砂层位置。表层填土由于土质不均、成分混杂,曲线振荡幅度较大、无明显规律,所以分层时不再考虑。同一土层中,锥尖阻力和摩阻比一般较均匀,所以本次主要根据摩阻比和锥尖阻力沿深度的变化趋势,结合单桥CPT测试比贯入阻力沿深度分层的趋势特征,对CPTU测试数据进行分层。对于分层时的超前滞后效应,均按照单桥CPT测试时的超前滞后效应确定。分层后求出各土层平均锥尖阻力、侧壁摩阻力及摩阻比,利用Robertson土质分类图进行各土层土类检验。
2. 液化数据库检验
CPTU测试在唐山地区共选取16个测点,其中液化点11个,非液化点5个。限于篇幅,本文仅介绍6个测点,主要体现检验CPTU数据的过程及与Moss等(2011)数据库、Boulanger等(2014)数据库的主要区别。 (1)T1液化点(唐山陡河桥,10度区,地下水位3.700 m)
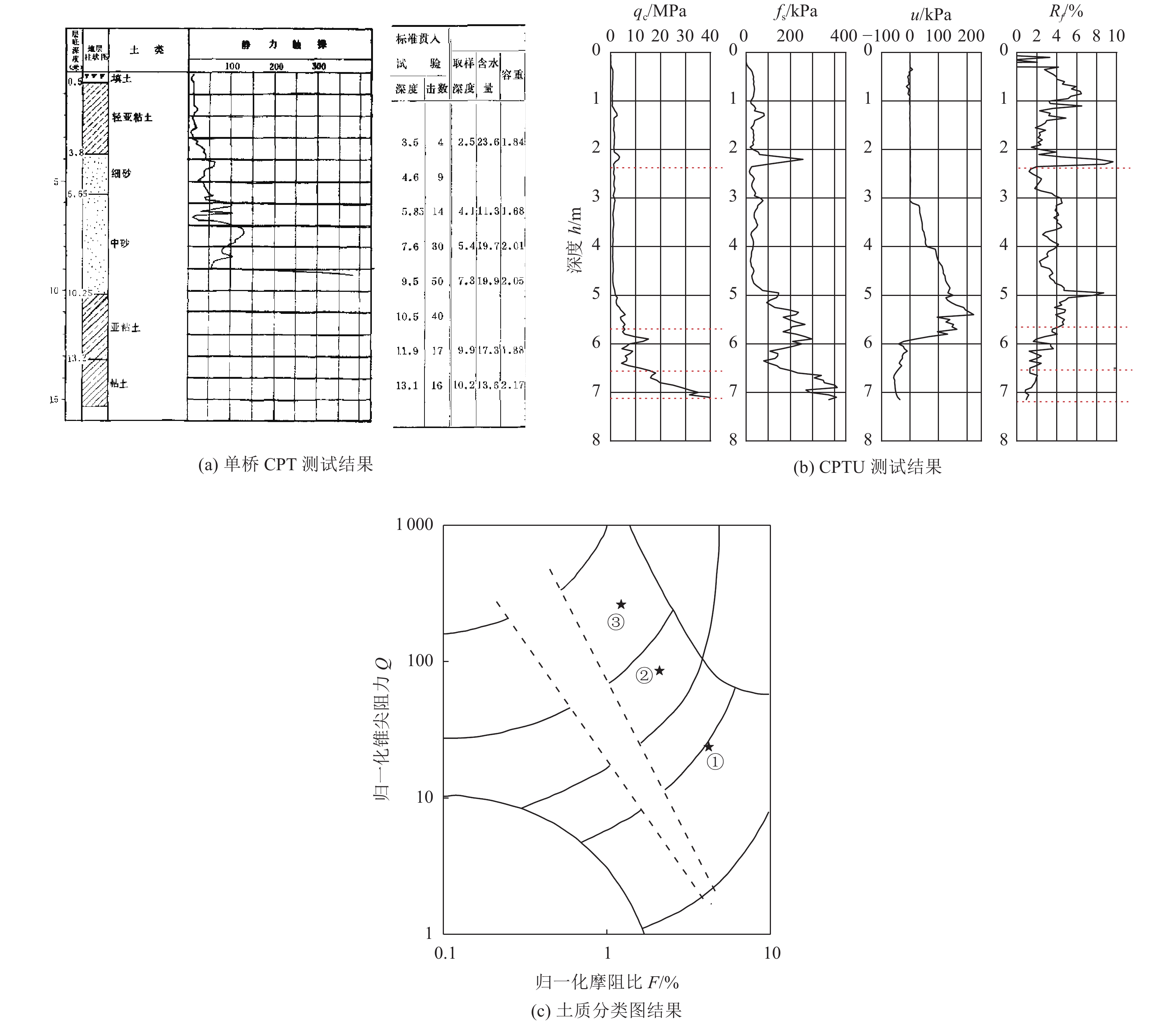
T1测点数据检验结果如图2所示。单桥CPT测试比贯入阻力-深度关系曲线(ps-h曲线)和CPTU测试锥尖阻力-深度关系曲线(qc-h曲线)趋势基本一致(qc-h曲线对应ps-h曲线0~6 m段)。根据CPTU测试摩阻比-深度关系曲线(Rf-h曲线)可知,T1测点土层自上而下可分为2类,分别为细粒土和砂土土层,与单桥CPT测试时钻孔柱状图土层土类对应程度较好。根据单桥CPT测试ps-h曲线可知,深度达3 m后,ps开始增大,但深度达3.8 m后才为砂层。根据CPTU测试qc-h曲线可知,深度达4.9 m后,锥尖阻力明显增大,所以按相同的超前滞后效应确定砂层位置从深度5.7 m处开始。确定砂层后,根据锥尖阻力和摩阻比进行分层,分层结果如表1所示。分层后将各土层数据代入Robertson土质分类图进行检验,检验结果如图2(c)所示。将图2(c)检验结果与单桥CPT测试时钻孔柱状图中的土类进行对比,结果如表2所示。由表2可知,2次测试的土层土类契合度较高,说明2次测试土层条件基本一致。深度存在的差别可能是场地填高或场地范围内土层水平向厚度不同导致的。对单桥CPT测试时的标贯击数与CPTU测试的平均锥尖阻力进行综合分析,细砂层标贯击数为9,平均锥尖阻力为8.23 MPa,中砂层标贯击数为22,平均锥尖阻力为26.67 MPa,标贯击数与平均锥尖阻力随深度变化趋势基本相符,综合判断T1测点为正确点。T1测点地下水位为3.700 m,根据2次测试得到的力学指标确定液化层为埋深5.7~6.55 m的细砂层。本研究对T1测点液化层的选取与Moss等(2011)数据库、Boulanger等(2014)数据库均一致。
表 1 T1测点CPTU分层结果Table 1. CPTU soil layer classification result of site T1分层深度/m qc/MPa fs/kPa Rf /% σv /kPa σ'v/kPa n Q F/% Ic 2.40~5.70 1.72 69.16 3.73 73.03 69.60 1.0 23.71 4.19 2.79 5.70~6.55 8.23 175.64 2.36 109.78 86.01 0.5 87.56 2.16 2.18 6.55~7.15 26.67 341.91 1.40 122.69 91.82 0.5 277.04 1.29 1.68 表 2 T1测点土层土类检验结果Table 2. Soil layer inspection results of site T1序号 单桥CPT测试钻孔土类 Robertson土质分类图土类 ① 轻亚黏土(深度0.50~3.80 m) 粉质黏土-黏土(深度2.40~5.70 m) ② 细砂(深度3.80~5.65 m) 粉砂-砂质粉土(深度5.70~6.55 m) ③ 中砂(深度5.65~10.20 m) 纯净砂-粉砂(深度6.55~7.15 m) (2)T2液化点(唐山洼里,10度区,地下水位1.250 m)
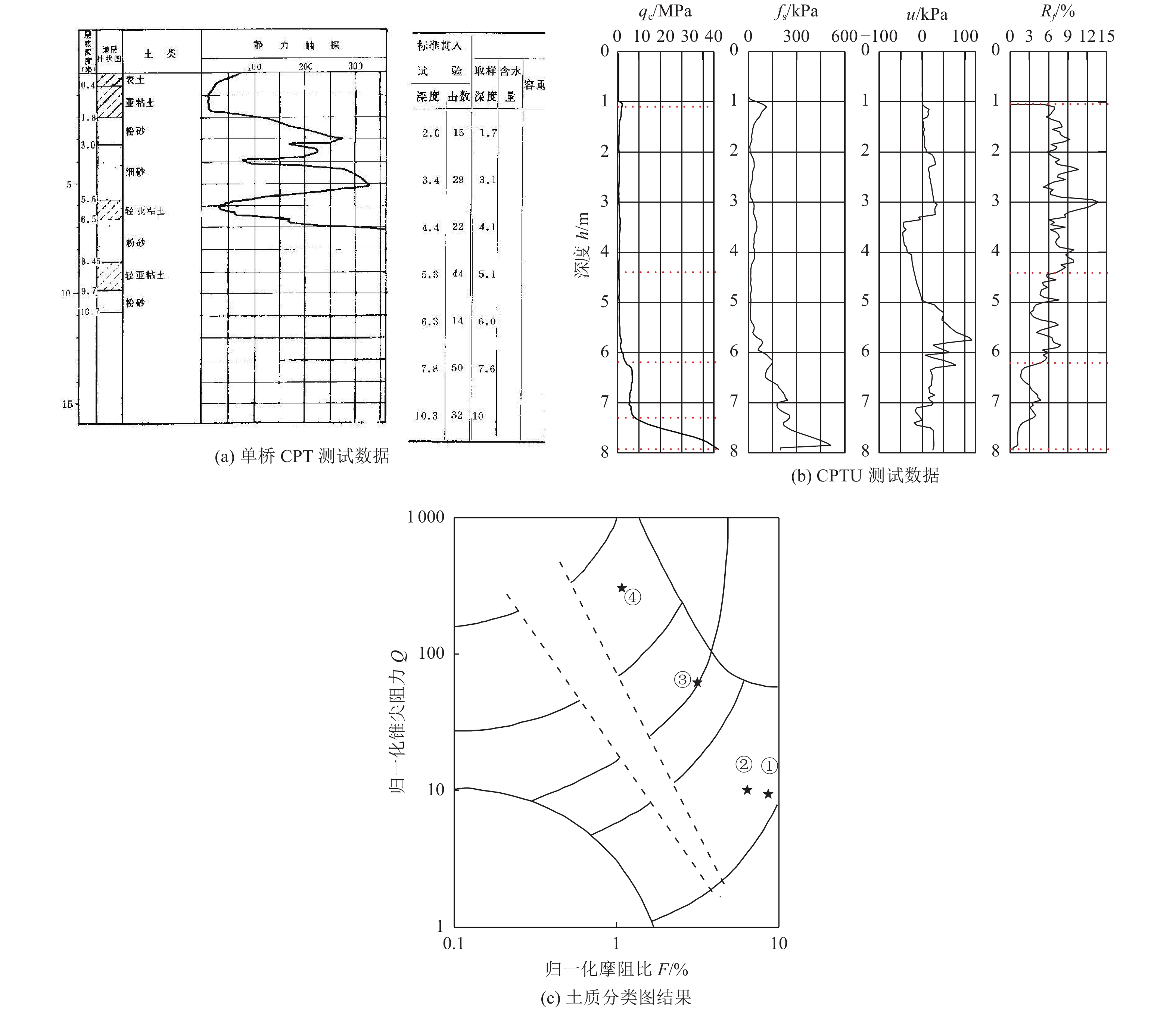
T2测点数据检验结果如图3所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势不一致。由CPTU测试Rf-h曲线可知,T2测点多为细粒土土层,仅在末端存在层厚较小的砂层,与单桥CPT测试时钻孔柱状图土层土类不符。按照CPTU测试数据重新分层,结果如表3所示,将各土层数据代入Robertson土质分类图,结果如图3(c)所示。将图3(c)土类结果与单桥CPT测试时钻孔柱状图中的土类进行对比,结果如表4所示。由表4可知,2次测试各土层土类条件不一致,推测2次测试不在同一测点。综合判断T2测点为错误点,应剔除。Moss等(2011)数据库认为T2测点为正确点,本研究与Boulanger等(2014)数据库均认定T2测点为错误点。
表 3 T2测点CPTU分层结果Table 3. CPTU soil layer classification result of site T2分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 0.35~2.20 1.09 72.25 6.57 24.62 24.37 1.0 43.65 6.79 2.75 2.20~4.20 2.97 245.18 8.36 61.78 42.67 1.0 68.10 8.44 2.70 4.20~6.90 3.99 232.30 5.86 107.15 65.01 1.0 59.73 5.98 2.62 6.90~8.30 6.52 257.71 3.75 146.73 84.50 0.5 69.30 4.05 2.45 8.30~8.45 18.63 302.42 1.79 161.75 91.93 0.5 192.58 1.64 1.86 表 4 T2测点土层土类检验结果Table 4. Soil layer inspection results of site T2序号 单桥CPT测试钻孔土类 Robertson土质分类图土类 ① 亚黏土(深度0~2.3 m) 粉质黏土-黏土(深度0.35~2.20 m) ② 细砂(深度2.3~3.7 m) 极硬细砂(深度2.20~4.20 m) ③ 中砂(深度3.7~4.4 m) 粉质黏土-黏土(深度4.20~6.90 m) ④ 淤泥质亚黏土(深度4.4~5.0 m) 黏质粉土-粉质黏土(深度6.90~8.30 m) ⑤ 粉砂(深度5.0~6.8 m) 纯净砂-粉砂(深度8.30~8.45 m) (3)T3非液化点(丰南县胥各庄,10度区,地下水位1.500 m)
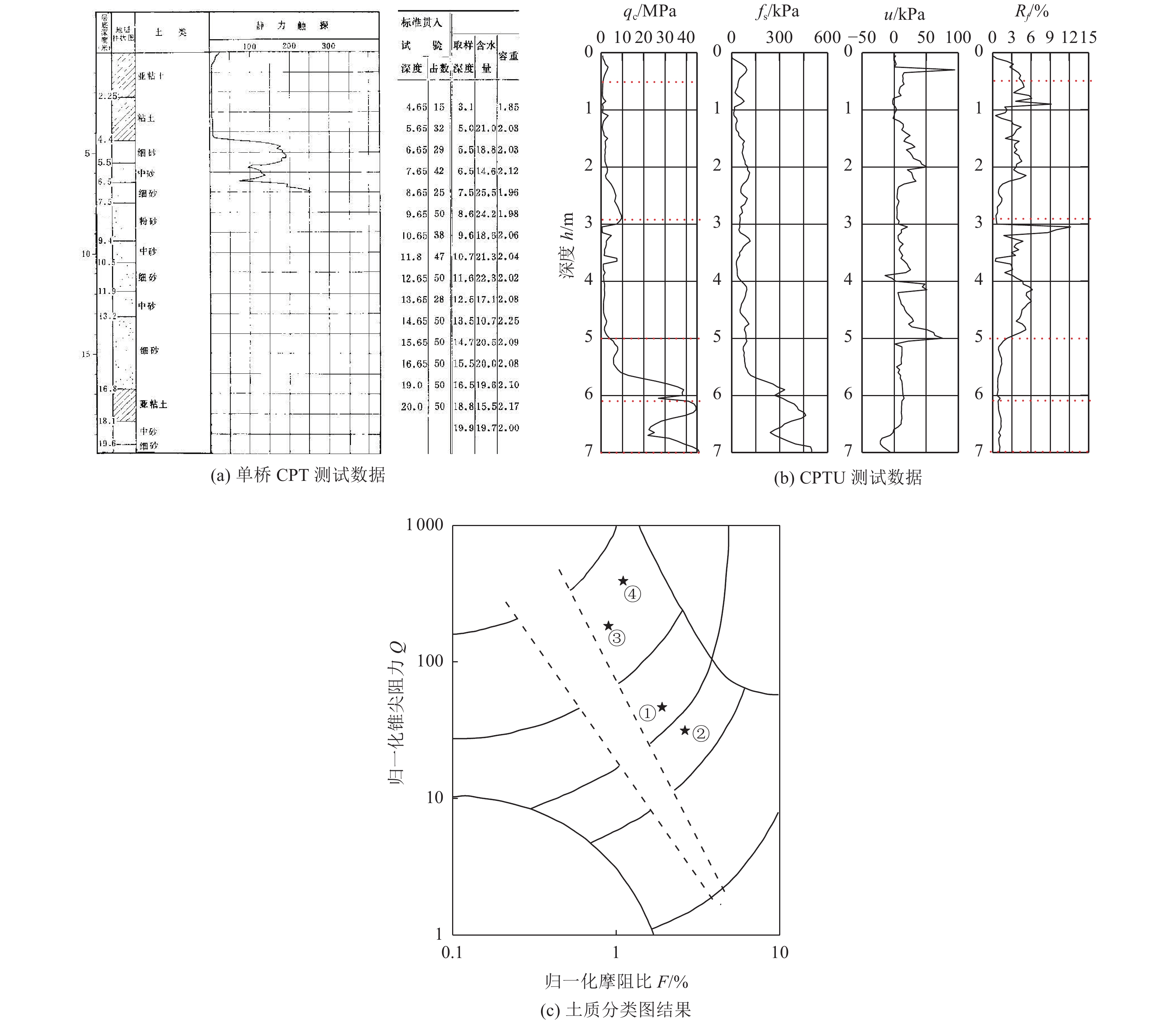
T3测点数据检验结果如图4所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势不一致。由CPTU测试Rf-h曲线可知,深度达6.2 m后出现砂土类土层,与单桥CPT测试时钻孔柱状图土层土类不相符。按照CPTU测试数据重新分层,结果如表5所示。分层后将各土层数据代入Robertson土质分类图,结果如图4(c)所示。将图4(c)土层土类结果与单桥CPT测试时钻孔柱状图中的土类进行对比,结果如表6所示。由表6可知,2次测试的土层土类不契合。单桥CPT测试时标贯击数与比贯入阻力值均较大,而CPTU测试的锥尖阻力均较小,标贯击数与平均锥尖阻力随深度的变化趋势不相符。综合判断,2次测试不在同一测点,T3测点为错误点,应剔除。本研究与Moss等(2011)数据库、Boulanger等(2014)数据库均认定T3测点为错误点。
表 5 T3测点CPTU分层结果Table 5. CPTU soil layer classification result of site T3分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 1.05~4.40 0.44 33.49 7.94 52.61 40.60 1.0 9.64 8.56 3.29 4.40~6.20 0.77 42.84 5.42 102.32 65.08 1.0 10.32 6.38 3.18 6.20~7.30 5.87 179.92 3.11 130.48 79.03 0.5 64.56 3.13 2.39 7.30~7.95 29.33 316.81 1.28 148.01 87.98 0.5 311.11 1.09 1.59 表 6 T3测点土层土类检验结果Table 6. Soil layer inspection results of site T3序号 单桥CPT测试钻孔土类 Robertson土质分类图
土类① 无 粉质黏土-黏土
(深度1.05~4.40 m)② 无 粉质黏土-黏土
(深度4.40~6.20 m)③ 亚黏土
(深度0.4~1.8 m)黏质粉土-粉质黏土
(深度6.20~7.30 m)④ 粉砂
(深度1.8~3.0 m)纯净砂-粉砂
(深度7.30~7.95 m)(4)T6液化点(唐山西大夫坨,10度区,地下水位1.500 m)
T6测点数据检验结果如图5所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势一致。由CPTU测试Rf-h曲线可知,T6测点土层自上而下可分为2类,分别为细粒土和砂土土层,与单桥CPT测试时钻孔柱状图土层土类对应程度好。按照CPTU测试数据重新分层,由单桥CPT测试ps-h曲线可知,深度达4.2 m后ps开始增大,但深度达4.4 m后才为砂层。由CPTU测试qc-h曲线可知,深度达4.8 m后锥尖阻力明显增大,所以按照相同的超前滞后效应确定砂层位置从深度5 m处开始。确定砂层深度后,根据锥尖阻力和摩阻比进行分层,分层结果如表7所示。分层后将各土层数据代入Robertson土质分类图,结果如图5(c)所示。将图5(c)土类结果与单桥CPT测试钻孔柱状图中的土类进行对比,结果如表8所示。由表8可知,2次测试的土层土类契合度较高,深度存在的差别可能是场地填高或场地范围内土层水平向厚度不同导致的。对比单桥CPT测试时的标贯击数与CPTU测试的平均锥尖阻力,细砂层标贯击数为15,平均锥尖阻力为17.71 MPa,中砂层标贯击数为32,平均锥尖阻力为35.30 MPa,标贯击数与平均锥尖阻力随深度的变化趋势基本相符,综合判断T6测点为正确点,液化层选为深度为5.0~6.1 m的细砂层。Moss等(2011)数据库对该测点液化层的选取与本研究一致。Boulanger等(2014)数据库将该测点液化层选为深度2.4~3.0 m,虽然该层摩阻比较小,属于砂土类,锥尖阻力较小,易液化,但与单桥CPT测试时钻孔柱状图中的土层土类无法对应,这样选取显然是错误的。
表 7 T6测点CPTU分层结果Table 7. CPTU soil layer classification result of site T6分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 0.50~2.85 3.04 60.75 3.04 32.33 30.62 0.5 54.36 2.02 2.31 2.85~5.00 2.48 67.54 4.16 75.77 52.01 0.5 33.36 2.81 2.56 5.00~6.10 17.71 164.39 1.07 106.50 66.81 0.5 215.47 0.93 1.65 6.10~7.00 35.30 384.7 1.11 125.42 75.94 0.5 403.68 1.09 1.53 表 8 T6测点土层土类检验结果Table 8. Soil layer inspection results of site T6序号 单桥CPT测试钻孔土类 Robertson土质分类图土类 ① 亚黏土(深度0~2.25m) 粉砂-砂质粉土(深度0.50~2.85m) ② 黏土(深度2.25~4.40m) 黏质粉土-粉质黏土(深度2.85~5.00m) ③ 细砂(深度4.40~5.50m) 纯净砂-粉砂(深度5.00~6.10m) ④ 中砂(深度5.50~6.50m) 纯净砂-粉砂(深度6.10~7.00m) (5)T15液化点(滦县佘庄,9度区,地下水位1.000 m)
T15测点数据检验结果如图6所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势基本一致。由CPTU测试qc-h曲线和Rf-h曲线可知,所测土层均为砂层,由于单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势基本一致,所以按照单桥CPT测试ps-h曲线拐点进行分层,结果如表9所示。分层后将各土层数据代入Robertson土质分类图,结果如图6(c)所示,将图6(c)检验结果与单桥CPT测试时钻孔柱状图中的土层土类进行对比,结果如表10所示。由表10可知,2次测试的土层土类契合度较高,说明2次测试的土层条件基本一致。中砂层标贯击数为11,平均锥尖阻力为17.23 MPa,粉砂层标贯击数为21,平均锥尖阻力为12.66 MPa,更深的砂层标贯击数与锥尖阻力均变的较大,不易液化,标贯击数与平均锥尖阻力变化趋势基本一致。综合判断T15测点为正确点,液化层选为深度为1.4~4.8 m的粉砂层。Moss等(2011)数据库、Boulanger等(2014)数据库均将T15测点作为错误点剔除是不对的。
表 9 T15测点CPTU分层结果Table 9. CPTU soil layer classification result of site T15分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 0~0.3 5.35 36.79 0.80 2.98 2.98 0.5 309.53 0.69 1.44 0.3~1.4 17.23 126.53 0.80 17.02 17.02 0.5 417.42 0.73 1.38 1.4~4.8 12.66 97.74 0.81 60.39 39.81 0.5 199.69 0.78 1.61 4.8~6.5 23.57 212.09 0.89 109.784 64.21 0.5 292.79 0.90 1.55 6.5~6.9 34.00 319.35 0.93 130.90 75.04 0.5 390.93 0.94 1.48 表 10 T15测点土层土类检验结果Table 10. Soil layer inspection results of site T15序号 单桥CPT测试钻孔土类 Robertson土质分类图土类 ① 细砂(深度0~1.1 m) 纯净砂-粉砂(深度0~0.3 m) ② 中砂(深度1.1~2.6 m) 纯净砂-粉砂(深度0.3~1.4 m) ③ 粉砂(深度2.6~6.2 m) 纯净砂-粉砂(深度1.4~4.8 m) ④ 细砂(深度6.2~9.3 m) 纯净砂-粉砂(深度4.8~6.5 m) ⑤ 中砂(深度9.3~10.5 m) 纯净砂-粉砂(深度6.5~6.9 m) (6)T16非液化点(滦县东坨子头,9度区,地下水位3.500 m)
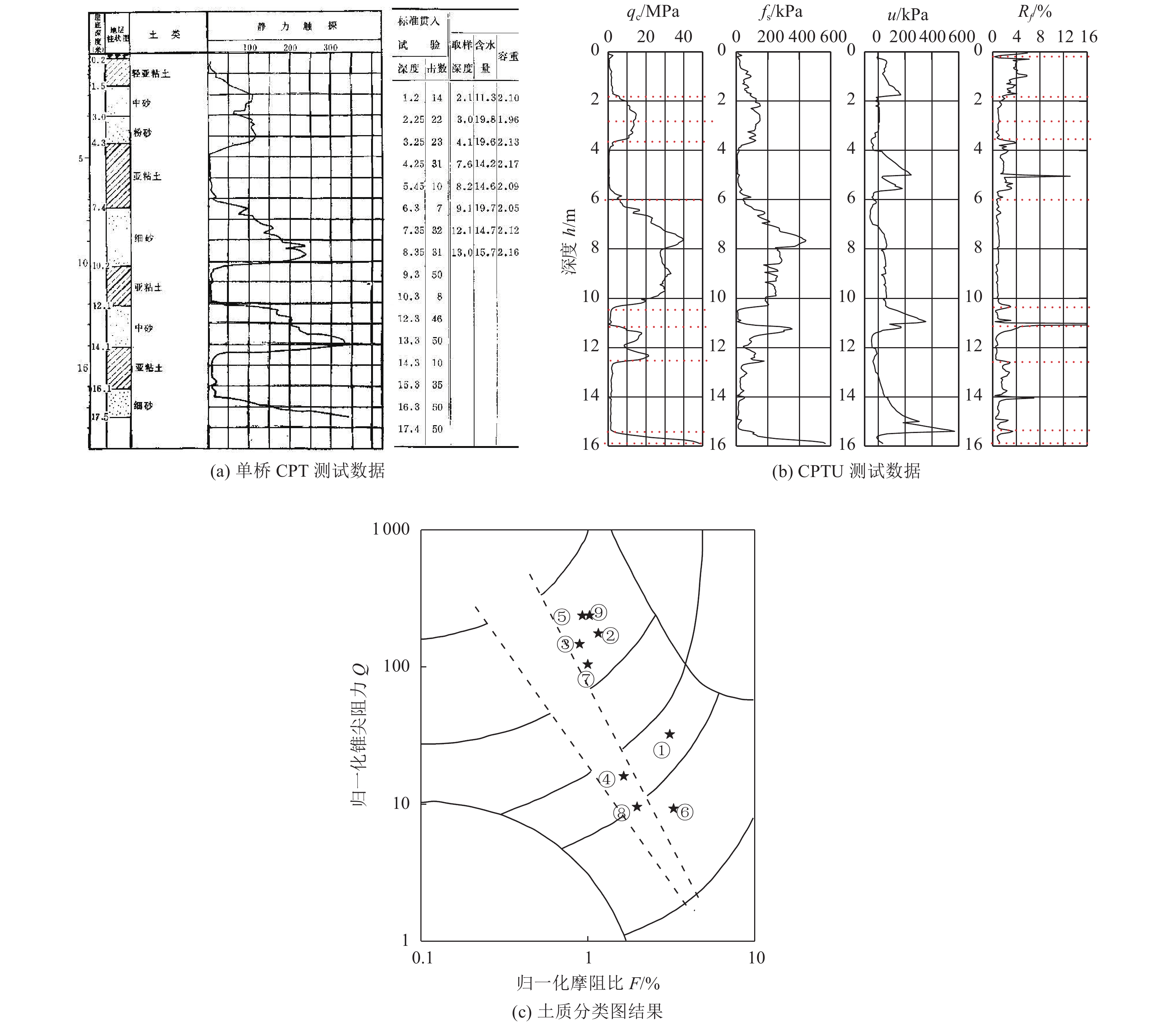
T16测点数据检验结果如图7所示。单桥CPT测试ps-h曲线和CPTU测试qc-h曲线趋势高度一致,所以按照单桥CPT测试土层曲线拐点对CPTU测试数据进行分层,结果如表11所示。分层后将各层数据代入Robertson土质分类图,结果如图7(c)所示。将图7(c)检验结果与单桥CPT测试时钻孔柱状图中的土层土类进行对比,结果如表12所示。由表12可知,2次测试的土层土类对应程度较高,说明2次测试的土层条件基本一致。深度存在的差别可能是场地范围内土层水平向厚度不同导致的。综合判断T16测点为正确点,临界液化层选为深度6.0~10.4 m的细砂层。Boulanger等(2014)数据库将液化层选为深度6.0~7.2 m的土层,本研究选为深度6.0~10.4 m的土层,该层细砂实际锥尖阻力较大,两端增大和减小段是出亚黏土层和进入亚黏土层的超前滞后效应造成的。
表 11 T16测点CPTU分层结果Table 11. CPTU soil layer classification result of site T16分层深度/m qc/MPa fs/kPa Rf /% σv/kPa σ'v/kPa n Q F/% Ic 0.25~1.80 1.65 55.03 3.67 18.98 18.98 0.5 37.62 3.36 2.58 1.80~2.80 11.57 132.98 1.21 42.94 42.94 0.5 175.99 1.15 1.77 2.80~3.60 11.85 113.82 1.00 60.89 60.89 0.5 151.18 0.96 1.77 3.60~6.00 1.37 22.76 2.23 91.47 78.73 1.0 16.35 1.77 2.69 6.00~10.40 25.55 234.77 0.97 160.48 114.42 0.5 237.38 0.92 1.61 10.40~11.10 1.53 43.13 4.26 213.75 142.70 1.0 9.28 3.26 3.04 11.10~12.50 14.29 138.92 1.09 235.05 153.71 0.5 113.38 0.99 1.87 12.50~15.50 1.91 35.20 1.74 277.65 174.75 1.0 9.37 2.15 2.94 15.50~15.90 33.09 334.69 0.97 309.59 190.03 0.5 237.85 1.02 1.65 表 12 T16测点土层土类检验结果Table 12. Soil layer inspection results of site T16序号 单桥CPT测试钻孔土类 Robertson土质分类图
土类① 轻亚黏土(0.2~1.5 m) 黏质粉土-粉质黏土(深度0.25~1.8 m) ② 中砂(1.5~3.0 m) 纯净砂-粉砂(深度1.8~2.8 m) ③ 粉砂(3.0~4.3 m) 纯净砂-粉砂(深度2.8~3.6 m) ④ 亚黏土(4.3~7.4 m) 黏质粉土-粉质黏土(深度3.6~6 m) ⑤ 细砂(7.4~10.2 m) 纯净砂-粉砂(深度6~10.4 m) ⑥ 亚黏土(10.2~12.1 m) 粉质黏土-黏土(深度10.4~11.1 m) ⑦ 中砂(12.1~14.1 m) 纯净砂-粉砂(深度11.1~12.5 m) ⑧ 亚黏土(14.1~16.1 m) 粉质黏土-黏土(深度12.5~15.5 m) ⑨ 细砂(12.1~14.1 m) 纯净砂-粉砂(深度15.5~15.9 m) 通过上述检验过程,本文剔除2个错误点T2、T3,剩下14个测点。其中,液化点10个,非液化点4个。
3. 液化数据库分析
根据《岩土工程勘察规范》(GB 50021—2001)(中华人民共和国建设部,2004)规定:当实测计算比贯入阻力或锥尖阻力小于液化比贯入阻力临界值pscr或液化锥尖阻力临界值qccr时,应判别为液化土,并按下列公式计算:
$$ p_{{\rm{s c r}}}=p_{{\rm{s}} 0} \alpha_{w} \alpha_{u} \alpha_{p}$$ (4) $$ q_{{\rm{c c r}}}=q_{{\rm{c}} 0} \alpha_{w} \alpha_{u} \alpha_{p}$$ (5) $$ \alpha_{w}=1-0.065\left(d_{w}-2\right) $$ (6) $$ \alpha_{\mathrm{u}}=1-0.05\left(d_{\mathrm{u}}-2\right) $$ (7) 式中,pscr、qccr分别为饱和土静力触探液化比贯入阻力临界值及锥尖阻力临界值;ps0、qc0分别为地下水深度dw=2 m、上覆非液化土层厚度(计算时应将淤泥和淤泥质土层厚度扣除)du=2 m时,饱和土液化判别比贯入阻力基准值和液化判别锥尖阻力基准值(MPa),可按表13取值,10度区基准值按规范原始文献取值(周神根,1980);αw为地下水位埋深修正系数,地面常年有水且与地下水有水力联系时取1.13;αu为上覆非液化土层厚度修正系数,对于深基础取1.0;αp为与静力触探摩阻比有关的土性修正系数,可按表14取值。
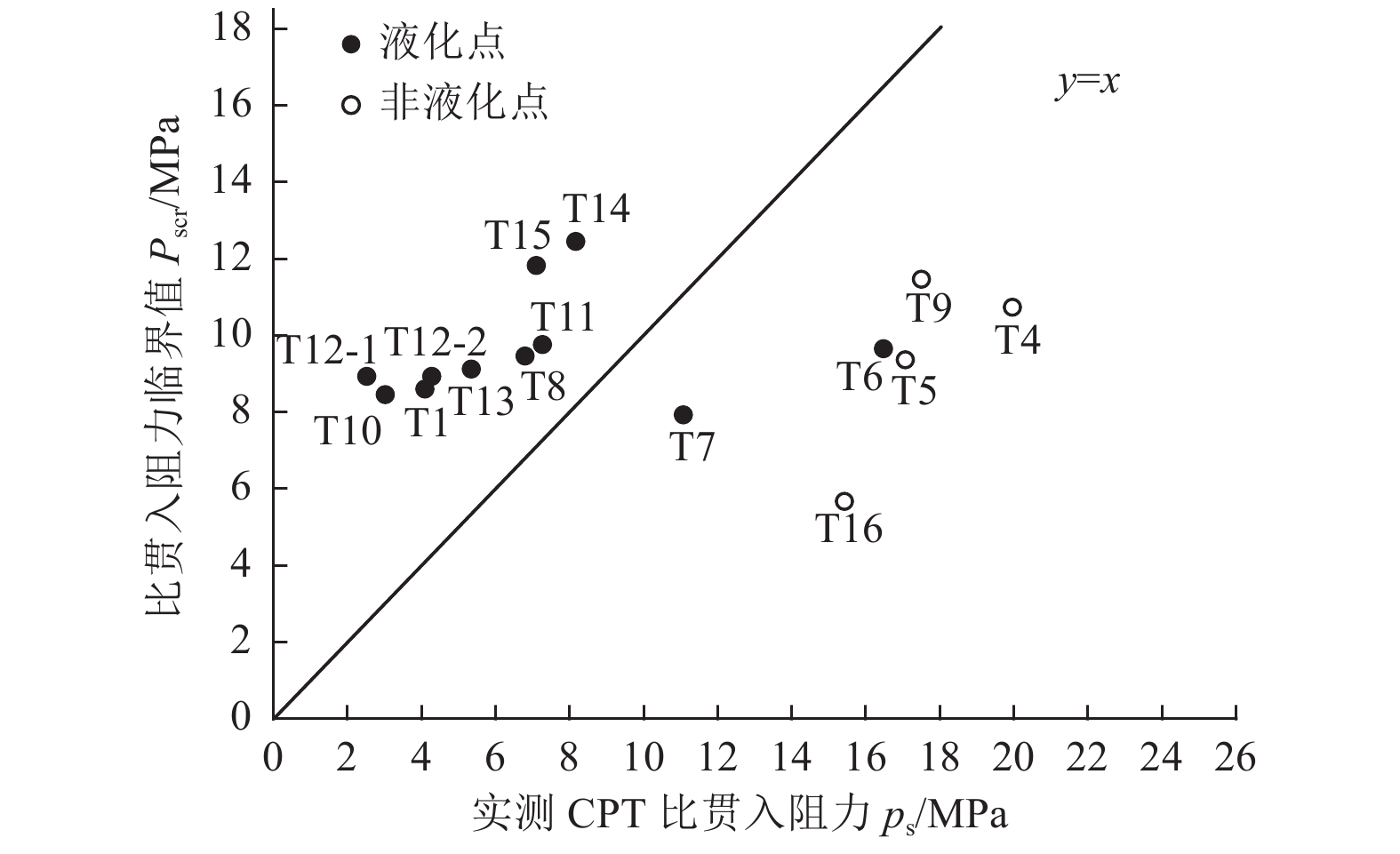
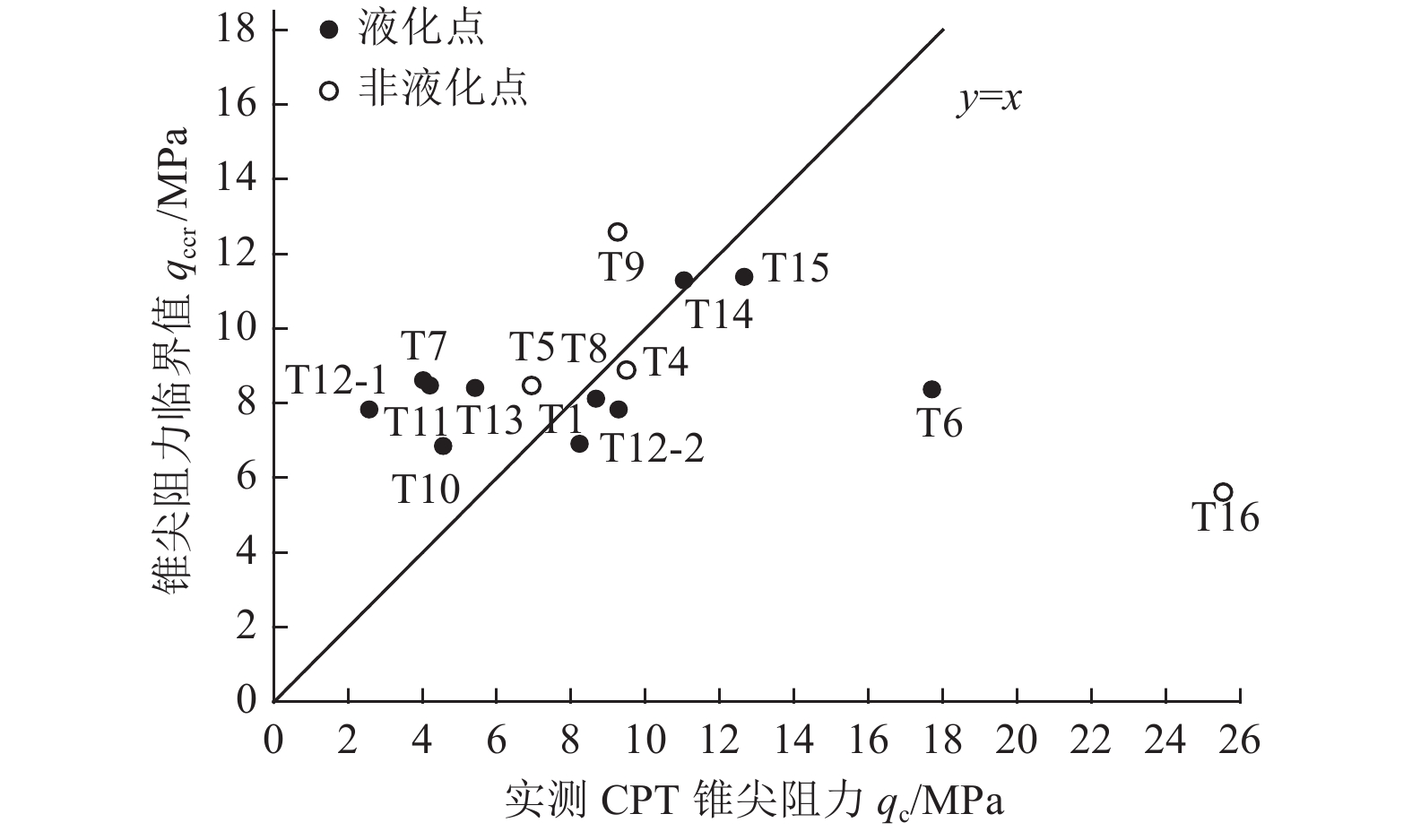
表 13 比贯入阻力和锥尖阻力基准值ps0、qc0Table 13. Liquefied reference value of specific penetration resistance and cone tip resistance参数 抗震设防烈度 7度 8度 9度 ps0/MPa 5.0~6.0 11.5~13.0 18.0~20.0 qc0/MPa 4.6~5.5 10.5~11.8 16.4~18.2 表 14 土性修正系数αp值Table 14. Values of soil property correction factor αp参数 土类 砂土 粉土 摩阻比Rf Rf≤0.4 0.4<Rf≤0.9 Rf>0.9 αp 1. 00 0.60 0.45 对于本研究通过检验的14个测点,分别针对单桥CPT测试比贯入阻力与CPTU测试锥尖阻力,分析液化可能性,建立液化数据库,如表15、16所示,液化判别结果如图8、9所示。
表 15 基于单桥CPT测试的唐山地震液化数据库Table 15. Database of ps-based liquefaction case histories in Tangshan earthquake测点 液化
情况地震
烈度/度土层
深度/mPs0/MPa dw/m du/m αw αu αp Pscr/MPa 实测Ps/MPa 液化判别 T1 液化 10 3.80~5.65 23.5 3.70 3.80 0.89 0.91 0.45 8.56 4.05 是 T4 非液化 10 2.90~3.50 23.5 1.10 2.90 1.06 0.96 0.45 10.69 19.92 否 T5 非液化 10 3.15~5.20 23.5 3.00 3.15 0.94 0.94 0.45 9.32 17.02 否 T6 液化 10 4.40~5.50 23.5 1.50 4.40 1.03 0.88 0.45 9.61 16.44 否 T7 液化 10 6.05~7.05 23.5 3.00 6.05 0.94 0.80 0.45 7.89 11.03 否 T8 液化 10 3.95~7.00 23.5 2.20 3.95 0.99 0.90 0.45 9.42 6.75 是 T9 非液化 10 6.70~8.20 23.5 1.10 6.70 1.06 0.77 0.60 11.42 17.46 否 T10 液化 9 3.00~5.50 19.0 1.45 3.00 1.04 0.95 0.45 8.41 2.98 是 T11 液化 9 0.85~3.40 19.0 0.85 0.85 1.07 1.06 0.45 9.71 7.22 是 T12-1 液化 9 1.80~3.20 19.0 1.55 1.80 1.03 1.01 0.45 8.89 2.48 是 T12-2 液化 9 3.20~10.20 19.0 1.55 1.80 1.03 1.01 0.45 8.89 4.24 是 T13 液化 9 2.00~3.80 19.0 1.05 2.00 1.06 1.00 0.45 9.08 5.30 是 T14 液化 9 1.25~2.10 19.0 1.25 1.25 1.05 1.04 0.60 12.40 8.13 是 T15 液化 9 2.60~6.20 19.0 1.00 2.60 1.07 0.97 0.60 11.78 7.06 是 T16 非液化 9 7.40~10.20 19.0 3.50 7.40 0.90 0.73 0.45 5.63 15.38 否 表 16 基于CPTU测试的唐山地震液化数据库Table 16. Database of qc-based liquefaction case histories in Tangshan earthquake测点 液化
情况地震
烈度/度土层
深度/mqc0/MPa dw/m du/m αw αu αp qccr/MPa 实测qc/MPa 液化判别 T1 液化 10 5.70~6.55 21.2 3.70 5.70 0.89 0.8150 0.45 6.92 8.23 否 T4 非液化 10 4.40~5.00 21.2 1.10 4.40 1.06 0.8800 0.45 8.89 9.49 否 T5 非液化 10 3.00~4.20 21.2 3.00 3.00 0.94 0.9500 0.45 8.47 6.94 是 T6 液化 10 5.00~6.10 21.2 1.50 5.00 1.03 0.8500 0.45 8.37 17.71 否 T7 液化 10 3.00~4.00 21.2 3.00 3.00 0.94 0.9500 0.45 8.47 4.20 是 T8 液化 10 4.75~7.40 21.2 2.20 4.75 0.99 0.8630 0.45 8.12 8.67 否 T9 非液化 10 3.30~4.80 21.2 1.10 3.30 1.06 0.9350 0.60 12.59 9.25 是 T10 液化 9 5.00~6.70 17.3 1.45 5.00 1.04 0.8500 0.45 6.85 4.93 是 T11 液化 9 1.40~2.60 17.3 0.85 1.40 1.07 1.0300 0.45 8.62 4.02 是 T12-1 液化 9 2.45~4.80 17.3 1.55 2.45 1.03 0.9775 0.45 7.83 2.57 是 T12-2 液化 9 4.80~9.40 17.3 1.55 2.45 1.03 0.9775 0.45 7.83 9.28 否 T13 液化 9 1.65~3.00 17.3 1.05 1.65 1.06 1.0175 0.45 8.41 5.42 是 T14 液化 9 1.25~2.10 17.3 1.25 1.25 1.05 1.0375 0.60 11.29 11.04 是 T15 液化 9 1.40~4.80 17.3 1.00 1.40 1.07 1.0300 0.60 11.39 12.66 否 T16 非液化 9 6.00~10.40 17.3 3.50 6.00 0.90 0.8000 0.45 5.62 25.55 否 对比表15、16可知,CPTU测试qc大于单桥CPT测试ps的测点有T1、T6、T8、T10、T12-1、T12-2、T14、T15、T16,CPTU测试测点液化层深度大于单桥CPT测试的测点有T1、T4、T6、T8、T10、T11、T12-1、T12-2。从液化判别结果来看,基于ps指标的液化判别方法判别成功率较高,因为我国规范CPT液化判别方法是利用这些数据构建的。而基于qc指标的液化判别方法判别成功率较低,将液化判别为不液化的有测点T1、T6、T8、T12-2、T15,将不液化判别为液化的测点有T5、T9,液化点整体有向右移动的趋势。
综合来看,经过30年的时间,土层液化可能性已发生较大改变,利用CPTU测试数据建立的液化数据库可靠性较低,并不能代表1976年唐山地震时的液化情况。
4. 结论
本文通过对比2次静力触探数据,利用Robertson土质分类图,进行新CPTU数据土类分层检验,将检验结果与单桥CPT测试时钻孔柱状图进行对比,发现大部分测点土层土类均能较好对应,现场测试力学指标沿深度的变化趋势较相符,仅剔除了错误点T2、T3。
对所有测点选定液化层,分别建立了基于单桥CPT测试ps指标和基于CPTU测试qc指标的液化数据库。利用我国规范CPT液化判别方法,检验了2个数据库的数据,发现基于单桥CPT测试ps指标的数据库液化点和非液化点得到了很好的区分,而基于CPTU测试qc指标的数据库判别效果较差,说明经过30年的时间,土层液化可能性已发生较大改变。因此,基于CPTU测试建立的液化数据库可靠性较低,基于该数据库对液化判别方法进行改进意义较小。
-
表 1 原始地震动时程信息
Table 1. Original ground motion parameters
方向 PGA /g PGV/(cm·s−1) PGD/cm 横桥向 0.73 133.33 113.87 顺桥向 0.79 28.09 25.52 表 2 合成的地震动时程信息
Table 2. Synthetic ground motion parameters
类别 PGA /g PGV /(cm·s−1) PGD /cm 横桥向不考虑永久位移 0.60 111.97 105.39 横桥向考虑永久位移 0.60 117.79 138.88 顺桥向不考虑永久位移 0.60 118.89 110.84 表 3 横桥向P3和P4墩顶相对位移最大值和残余值
Table 3. Maximum and residual relative displacement of the pier top at P3 and P4 in the transverse direction
工况 最大值/cm
(残余值/cm)P2 P3 P4 P5 P6 1 9.0580
(−0.1654)4.6155
(−0.0405)3.8598
(−0.0254)6.4269
(−0.1254)5.5834
(−0.1095)2 9.8470
(0.2830)4.4210
(−1.2270)4.1927
(1.1377)6.3951
(−0.0789)5.5772
(−0.0757)3 9.2286
(0.1558)4.7349
(−1.4285)4.7175
(1.2923)6.4440
(−0.1007)5.5611
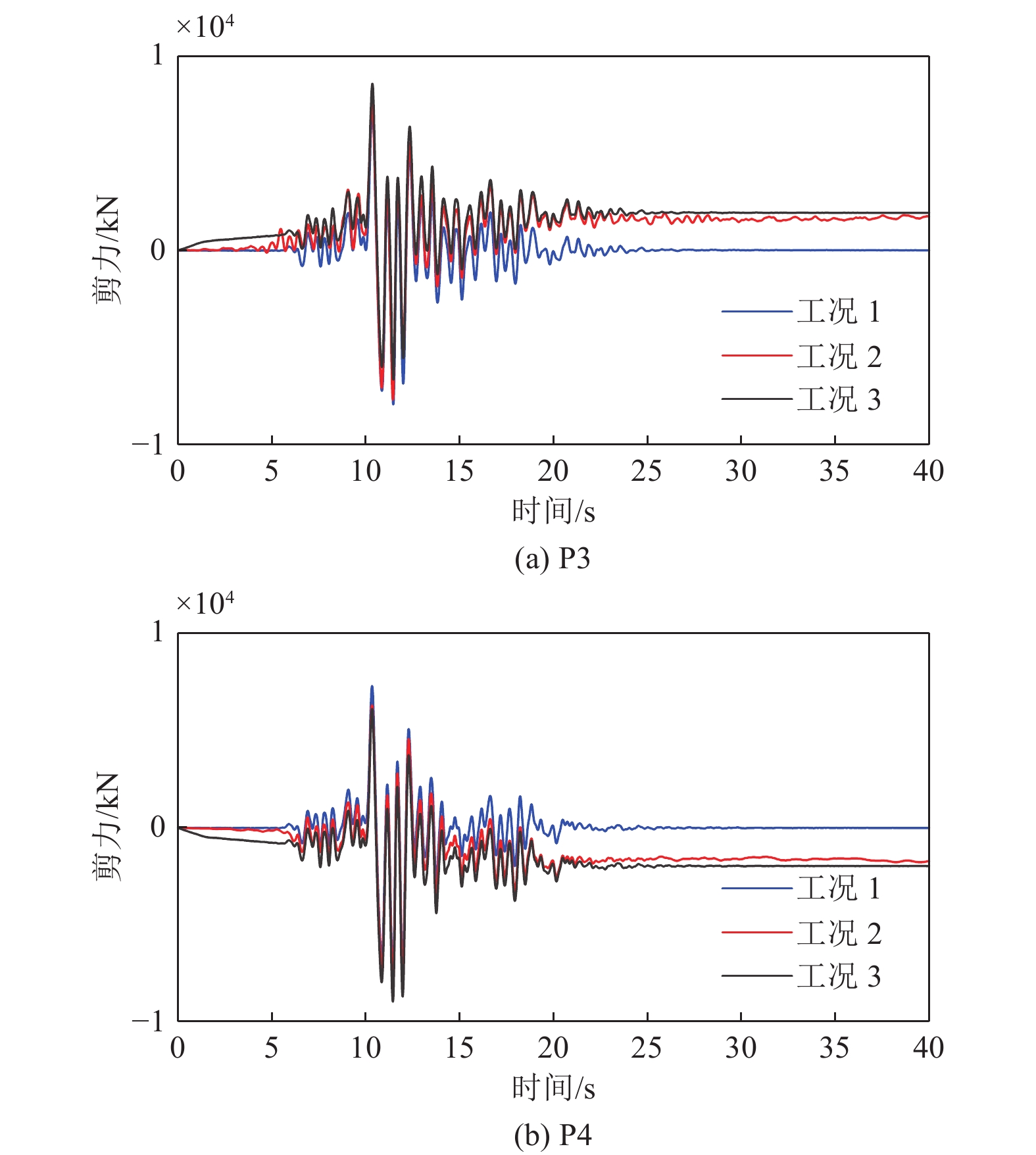
(−0.1431)表 4 横桥向P3和P4墩底剪力最大值和残余值
Table 4. The maximum and residual shear force at the bottom of pier P3 and P4 in the transverse direction
工况 剪力最大值/kN 残余剪力/kN P3 P4 P3 P4 1 7.9340$ \times {10}^{3} $ 7.6760$ \times {10}^{3} $ 0.0044$ \times {10}^{3} $ −0.0084$ \times {10}^{3} $ 2 7.6930$ \times {10}^{3} $ 8.1211$ \times {10}^{3} $ 1.7479$ \times {10}^{3} $ −1.7280$ \times {10}^{3} $ 3 8.5876$ \times {10}^{3} $ 8.9535$ \times {10}^{3} $ 1.9306$ \times {10}^{3} $ −1.9704$ \times {10}^{3} $ 表 5 横桥向P3和P4墩底弯矩最大值和残余值
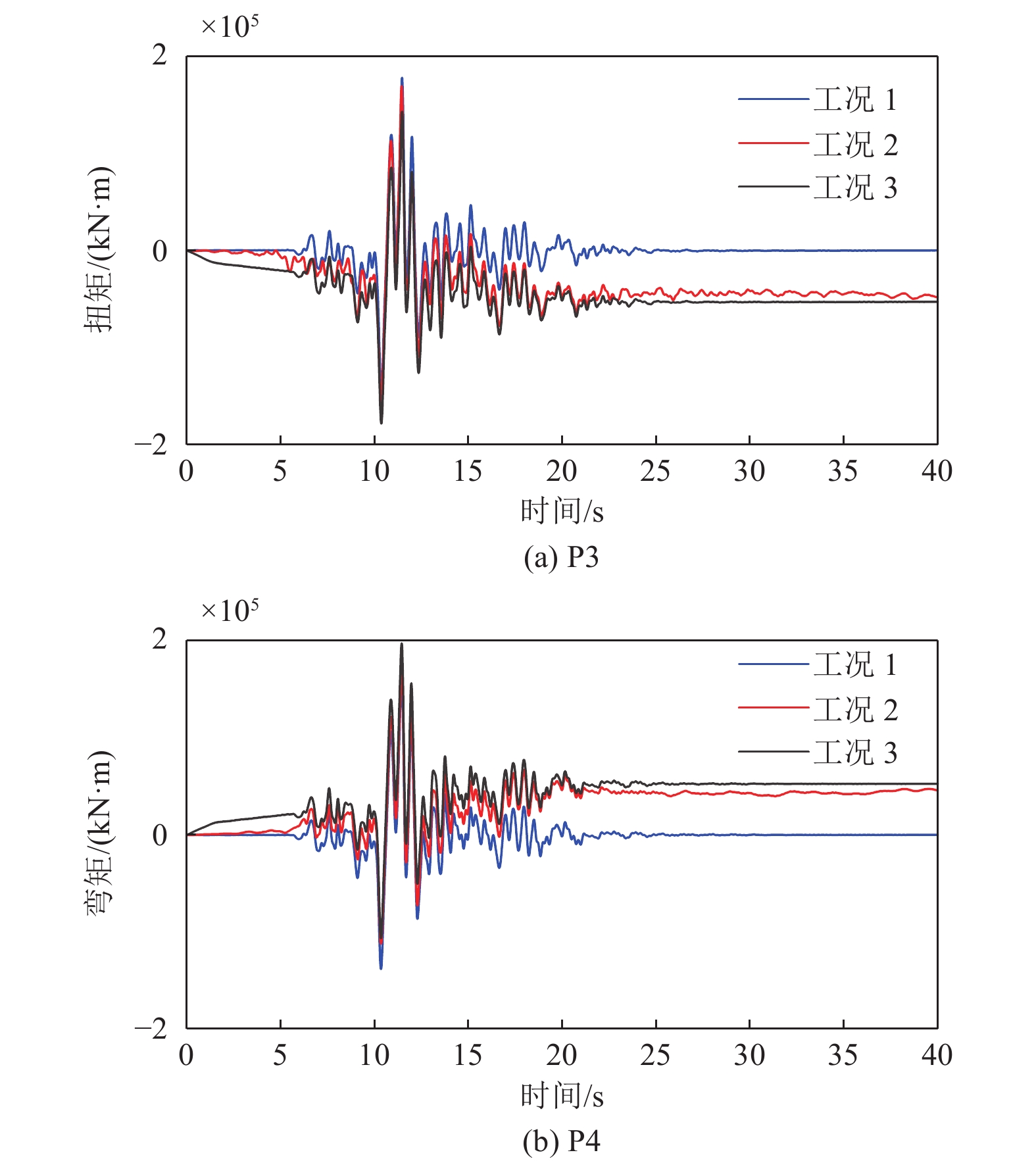
Table 5. The maximum and residual bending moment at the bottom of pier P3 and P4 in the transverse direction
工况 弯矩最大值 /(kN∙m) 残余弯矩 /(kN∙m) P3 P4 P3 P4 1 1.7790$ \times {10}^{5} $ 1.6317$ \times {10}^{5} $ 0.0016$ \times {10}^{4} $ 0.0328$ \times {10}^{4} $ 2 1.6921$ \times {10}^{5} $ 1.7596$ \times {10}^{5} $ 4.7504$ \times {10}^{4} $ 4.6370$ \times {10}^{4} $ 3 1.7830$ \times {10}^{5} $ 1.9733$ \times {10}^{5} $ 5.2868$ \times {10}^{4} $ 5.2781$ \times {10}^{4} $ 表 6 P3和P4墩底扭矩最大值和残余值
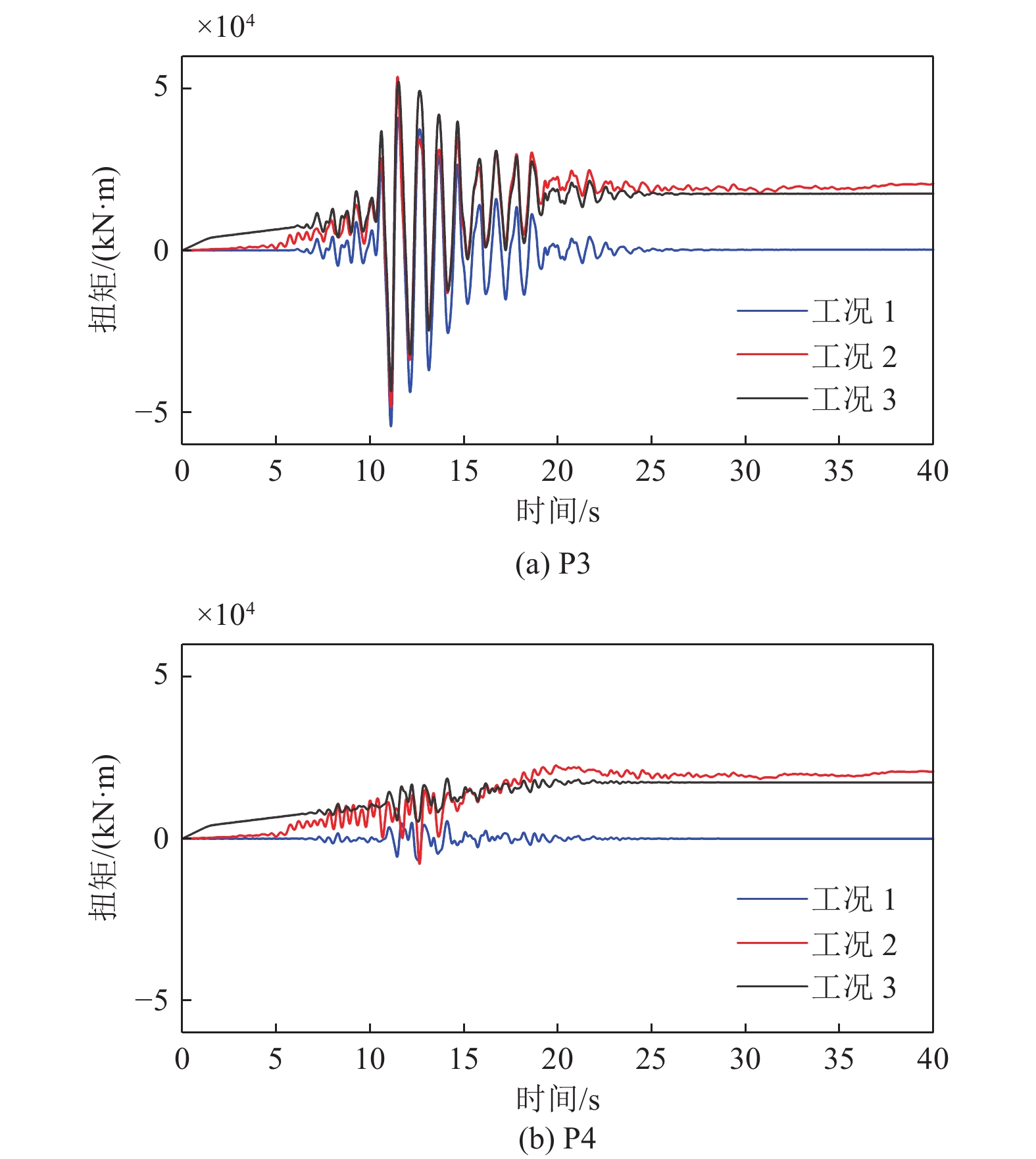
Table 6. The maximum and residual torque at the bottom of pier P3 and P4 in the transverse direction
工况 扭矩最大值 /(kN∙m) 残余扭矩 /(kN∙m) P3 P4 P3 P4 1 5.4362$ \times {10}^{4} $ 0.6780$ \times {10}^{4} $ 0.0225$ \times {10}^{4} $ 0.0054$ \times {10}^{4} $ 2 5.3635$ \times {10}^{4} $ 2.2612$ \times {10}^{4} $ 2.0436$ \times {10}^{4} $ 2.0624$ \times {10}^{4} $ 3 5.2122$ \times {10}^{4} $ 1.8589$ \times {10}^{4} $ 1.7523$ \times {10}^{4} $ 1.7337$ \times {10}^{4} $ -
陈令坤, 张楠, 夏禾, 2016. 方向脉冲及竖向效应对高铁桥梁地震响应影响. 振动工程学报, 29(4): 704—713Chen L. K. , Zhang N. , Xia H. , 2016. Effects of directivity pulse and vertical earthquake on seismic response of high-speed railway bridge. Journal of Vibration Engineering, 29(4): 704—713. (in Chinese) 杜修力, 韩强, 李忠献等, 2008.5. 12汶川地震中山区公路桥梁震害及启示. 北京工业大学学报, 34(12): 1270—1279Du X. L. , Han Q. , Li Z. X. , et al. , 2008. The seismic damage of bridges in the 2008 Wenchuan earthquake and lessons from its damage. Journal of Beijing University of Technology, 34(12): 1270—1279. (in Chinese) 管仲国, 游瀚, 郭河, 2016. 近断层斜拉桥弹塑性索与阻尼器组合横向减震. 同济大学学报(自然科学版), 44(11): 1653—1659Guan Z. G. , You H. , Guo H. , 2016. Responses of cable-stayed bridge transversely isolated with elasto-plastic cable pairs and fluid viscous damper subjected to near fault ground motions. Journal of Tongji University (Natural Science), 44(11): 1653—1659. (in Chinese) 惠迎新, 王克海, 李冲, 2014. 跨断层地表破裂带桥梁震害研究及抗震概念设计. 公路交通科技, 31(10): 51—57 doi: 10.3969/j.issn.1002-0268.2014.10.009Hui Y. X. , Wang K. H. , Li C. , 2014. Study of seismic damage and seismic conceptual design of bridges across fault surface rupture zones. Journal of Highway and Transportation Research and Development, 31(10): 51—57. (in Chinese) doi: 10.3969/j.issn.1002-0268.2014.10.009 惠迎新, 王克海, 2015 a. 基于多点激励位移输入模型的跨断层桥梁地震动输入方法. 东南大学学报(自然科学版), 45(3): 557—562Hui Y. X. , Wang K. H. , 2015 a. Earthquake motion input method for bridges crossing fault based on multi-support excitation displacement input model. Journal of Southeast University (Natural Science Edition), 45(3): 557—562. (in Chinese) 惠迎新, 王克海, 2015 b. 跨断层桥梁地震响应特性研究. 桥梁建设, 45(3): 70—75Hui Y. X. , Wang K. H. , 2015 b. Study of seismic response features of bridges crossing faults. Bridge Construction, 45(3): 70—75. (in Chinese) 惠迎新, 王克海, 吴刚等, 2015 c. 跨断层桥梁地震响应分析及合理跨越角度研究. 振动与冲击, 34(13): 6—11, 17Hui Y. X. , Wang K. H. , Wu G. , et al. , 2015 c. Seismic responses of bridges crossing faults and their best crossing angles. Journal of Vibration and Shock, 34(13): 6—11, 17. (in Chinese) 惠迎新, 台玉吉, 王克海等, 2017. 跨断层桥梁抗震若干问题探讨. 地震工程学报, 39(5): 870—875, 889Hui Y. X. , Tai Y. J. , Wang K. H. , et al. , 2017. Discussion about earthquake resistance of the bridges crossing the active fault. China Earthquake Engineering Journal, 39(5): 870—875, 889. (in Chinese) 江辉, 朱晞, 倪永军, 2011. 基于谱分析与RC梁桥检验的脉冲型近场地震动强度度量指标. 铁道学报, 33(3): 91—99 doi: 10.3969/j.issn.1001-8360.2011.03.016Jiang H. , Zhu X. , Ni Y. J. , 2011. Intensity indice of impulsive near-fault earthquake ground motion based on spectra analysis and RC bridge check. Journal of the China Railway Society, 33(3): 91—99. (in Chinese) doi: 10.3969/j.issn.1001-8360.2011.03.016 李鸿晶, 陆鸣, 温增平等, 2009. 汶川地震桥梁震害的特征. 南京工业大学学报(自然科学版), 31(1): 24—29Li H. J. , Lu M. , Wen Z. P. , et al. , 2009. Characteristics of bridge damages in Wenchuan earthquake. Journal of Nanjing University of Technology (Natural Science Edition), 31(1): 24—29. (in Chinese) 李建中, 管仲国, 2011. 基于性能桥梁抗震设计理论发展. 工程力学, 28(S2): 24—30, 53Li J. Z. , Guan Z. G. , 2011. Performance-based seismic design for bridges. Engineering Mechanics, 28(S2): 24—30, 53. (in Chinese) 李帅, 张凡, 颜晓伟等, 2017. 近断层地震动合成方法及其对超大跨斜拉桥地震响应影响. 中国公路学报, 30(2): 86—97, 106Li S. , Zhang F. , Yan X. W. , et al. , 2017. Synthetic method for near-fault ground motions and its influence on seismic response of super-span cable-stayed bridge. China Journal of Highway and Transport, 30(2): 86—97, 106. (in Chinese) 李小军, 1992. 场地土层对地震地面运动影响的分析方法. 世界地震工程, 8(2): 49—60. 李小军, 彭青, 2001. 不同类别场地地震动参数的计算分析. 地震工程与工程振动, 21(1): 29—36Li X. J. , Peng Q. , 2001. Calculation and analysis of earthquake ground motion parameters for different site categories. Earthquake Engineering and Engineering Vibration, 21(1): 29—36. (in Chinese) 李小军, 贺秋梅, 亓兴军, 2012. 地震动速度脉冲对大跨斜拉桥减震控制的影响. 应用基础与工程科学学报, 20(2): 272—285Li X. J. , He Q. M. , Qi X. J. , 2012. Seismic mitigation control effects of long-span cable-stayed bridges to ground motions with velocity pulse. Journal of Basic Science and Engineering, 20(2): 272—285. (in Chinese) 刘爱文, 夏珊, 徐超, 2008. 汶川地震交通系统震害及震后抢修. 震灾防御技术, 3(3): 243—450.Liu A. W., Xia S., Xu C., 2008. Damage and emergency recovery of the transportation systems after Wenchuan earthquake. Technology for Earthquake Disaster Prevention, 3(3): 243–250. (in Chinese) 刘启方, 袁一凡, 金星等, 2006. 近断层地震动的基本特征. 地震工程与工程振动, 26(1): 1—10Liu Q. F. , Yuan Y. F. , Jin X. , et al. , 2006. Basic characteristics of near-fault ground motion. Earthquake Engineering and Engineering Vibration, 26(1): 1–10. (in Chinese) 石岩, 王东升, 孙治国, 2014. 近断层地震动下减隔震桥梁地震反应分析. 桥梁建设, 44(3): 19—24Shi Y. , Wang D. S. , Sun Z. G. , 2014. Analysis of seismic response of seismically mitigated and isolated bridge subjected to near-fault ground motion. Bridge Construction, 44(3): 19—24. (in Chinese) 王东升, 杨海红, 王国新, 2005. 考虑邻梁碰撞的多跨长简支梁桥落梁震害分析. 中国公路学报, 18(3): 54—59Wang D. S. , Yang H. H. , Wang G. X. , 2005. Seismic analysis of girders falling down in multi-span long simply supported bridges with adjacent pounding effects. China Journal of Highway and Transport, 18(3): 54—59. (in Chinese) 王东升, 郭迅, 孙治国等, 2009. 汶川大地震公路桥梁震害初步调查. 地震工程与工程振动, 29(3): 84—94Wang D. S. , Guo X. , Sun Z. G. , et al. , 2009. Damage to highway bridges during Wenchuan earthquake. Journal of Earthquake Engineering and Engineering Vibration, 29(3): 84—94. (in Chinese) 王东升, 孙治国, 李晓莉等, 2010. 汶川大地震曲线梁桥震害及破坏机理分析. 防灾减灾工程学报, 30(5): 572—579Wang D. S. , Sun Z. G. , Li X. L. , et al. , 2010. Seismic damage investigation and failure mechanism analysis of curved bridges in Wenchuan earthquake. Journal of Disaster Prevention and Mitigation Engineering, 30(5): 572—579. ( in Chinese 杨怀宇, 李建中, 2015. 断层地震动对隔震桥梁地震响应的影响. 同济大学学报(自然科学版), 43(8): 1144—1152Yang H. Y. , Li J. Z. , 2015. Response analysis of seismic isolated bridge under influence of fault-crossing groundmotions. Journal of Tongji University (Natural Science), 43(8): 1144—1152. (in Chinese) 袁一凡, 田启文, 2012. 工程地震学. 北京: 地震出版社. 中华人民共和国交通运输部, 2014. JTG B02—2013 公路工程抗震规范. 北京: 人民交通出版社.Ministry of Transport of the People's Republic of China, 2014. JTG B02—2013 Specification of seismic design for highway engineering. Beijing: China Communications Press. (in Chinese) 中华人民共和国交通运输部, 2020. JTG/T 2231-01—2020 公路桥梁抗震设计规范. 北京: 人民交通出版社.Ministry of Transport of the People's Republic of China, 2020. JTG/T2231-01—2020 Specifications for seismic design of highway bridges. Beijing: China Communications Press. (in Chinese) 中华人民共和国住房和城乡建设部, 中华人民共和国国家质量监督检验检疫总局, 2010. GB 50011—2010 建筑抗震设计规范. 北京: 中国建筑工业出版社.Ministry of Housing and Urban-Rural Development of the People’s Republic of China, General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China, 2010. GB 50011—2010 Code for seismic design of buildings. Beijing: China Architecture & Building Press. (in Chinese) 周正华, 张艳梅, 孙平善等, 2003. 断层场地震害研究综述. 地震工程与工程振动, 23(5): 38—41 doi: 10.3969/j.issn.1000-1301.2003.05.006Zhou Z. H. , Zhang Y. M. , Sun P. S. , et al. , 2003. A summary of damage study on fault site. Earthquake Engineering and Engineering Vibration, 23(5): 38—41. (in Chinese) doi: 10.3969/j.issn.1000-1301.2003.05.006 Byers W. G. , Edwards C. , Tang A. , et al. , 2000. Performance of transportation systems after the 1999 Kocaeli earthquake. Earthquake Spectra, 16(S1): 403—410. Caltrans, 2012. Bridge design practice . Sacramento: California Department of Transportation. Caltrans, 2019. Seismic design criteria: version 2.0. Sacramento: California Department of Transportation. CEN, 2004. EN 1998-5—2004 Eurocode 8: design of structures for earthquake resistance Part 5: foundation, retaining structures and geotechnical aspects. Brussels: European Committee for Standardization, 14—20. Chang K. C. , Chang D. W. , Tsai M. H. , et al. , 2000. Seismic performance of highway bridges. Earthquake Engineering and Engineering Seismology, 2(1): 55—77. Elgamal A., Lu J., Mackie K., 2014. MSBrindge: OpenSees pushover and earthquake analysis of multi-span bridges - User Manual. San Diego: Department of Structural Engineering, University of California. Ghasemi H., Cooper J. D., Imbsen R., et al., 2000. The November 1999 Duzce earthquake: post-earthquake investigation of the structures on the TEM. Washington: Federal Highway Administration. Goel R. , Qu B. , Tures J. , et al. , 2014. Validation of fault rupture-response spectrum analysis method for curved bridges crossing strike-slip fault rupture zones. Journal of Bridge Engineering, 19(5): 06014002. doi: 10.1061/(ASCE)BE.1943-5592.0000602 Goel R. K., Chopra A. K., 2008. Analysis of ordinary bridges crossing fault-rupture zones. In: The 14 th World Conference on Earthquake Engineering. Beijing. Goel R. K. , Chopra A. K. , 2009 a. Linear analysis of ordinary bridges crossing fault-rupture zones. Journal of Bridge Engineering, 14(3): 203—215. doi: 10.1061/(ASCE)1084-0702(2009)14:3(203) Goel R. K. , Chopra A. K. , 2009 b. Nonlinear analysis of ordinary bridges crossing fault-rupture zones. Journal of Bridge Engineering, 14(3): 216—224. doi: 10.1061/(ASCE)1084-0702(2009)14:3(216) Güney D. , Acar M. , Özlüdemir M. T. , et al. , 2010. Investigation of post-earthquake displacements in viaducts using Geodetic and Finite Element Methods. Natural Hazards and Earth System Sciences, 10(12): 2579—2587. doi: 10.5194/nhess-10-2579-2010 He W. L. , Agrawal A. K. , 2008. Analytical model of ground motion pulses for the design and assessment of seismic protective systems. Journal of Structural Engineering, 134(7): 1177—1188. doi: 10.1061/(ASCE)0733-9445(2008)134:7(1177) Hsu Y. T. , Fu C. C. , 2004. Seismic effect on highway bridges in Chi Chi earthquake. Journal of Performance of Constructed Facilities, 18(1): 47—53. doi: 10.1061/(ASCE)0887-3828(2004)18:1(47) Imbsen R. A. , Roblee C. J. , Yashinsky M. , et al. , 2000. Impact on highway structures. Earthquake Spectra, 16(S1): 411—435. Kitagawa Y. , Hiraishi H. , 2004. Overview of the 1995 Hyogo-Ken Nanbu earthquake and proposals for earthquake mitigation measures. Journal of Japan Association for Earthquake Engineering, 4 (3): 1—29. doi: 10.5610/jaee.4.3_1 Li S. , Zhang F. , Wang J. Q. , et al. , 2017. Effects of near-fault motions and artificial pulse-type ground motions on super-span cable-stayed bridge systems. Journal of Bridge Engineering, 22(3): 04016128. doi: 10.1061/(ASCE)BE.1943-5592.0001008 Mackie K. R. , Lu J. C. , Elgamal A. , 2012. Performance-based earthquake assessment of bridge systems including ground-foundation interaction. Soil Dynamics and Earthquake Engineering, 42: 184—196. doi: 10.1016/j.soildyn.2012.05.023 Makris N. , Chang S. P. , 2000. Effect of viscous, viscoplastic and friction damping on the response of seismic isolated structures. Earthquake Engineering & Structural Dynamics, 29(1): 85—107. Mavroeidis G. P. , Papageorgiou A. S. , 2003. A mathematical representation of near-fault ground motions. Bulletin of the Seismological Society of America, 93(3): 1099—1131. doi: 10.1785/0120020100 Park S. W. , Ghasemi H. , Shen J. , et al. , 2004. Simulation of the seismic performance of the Bolu Viaduct subjected to near‐fault ground motions. Earthquake Engineering & Structural Dynamics, 33(13): 1249—1270. Roussis P. C. , Constantinou M. C. , Erdik M. , et al. , 2003. Assessment of performance of seismic isolation system of bolu viaduct. Journal of Bridge Engineering, 8(4): 182—190. doi: 10.1061/(ASCE)1084-0702(2003)8:4(182) Sehhati R. , Rodriguez-Marek A. , ElGawady M. , et al. , 2011. Effects of near-fault ground motions and equivalent pulses on multi-story structures. Engineering Structures, 33(3): 767—779. doi: 10.1016/j.engstruct.2010.11.032 Sengupta A. , Quadery L. , Sarkar S. , et al. , 2016. Influence of bidirectional near-fault excitations on RC bridge piers. Journal of Bridge Engineering, 21(7): 04016034. doi: 10.1061/(ASCE)BE.1943-5592.0000836 Somerville P. G., 2003. Magnitude scaling of the near fault rupture directivity pulse. Physics of the Earth and Planetary Interiors, 137(1—4): 201—212. Wang N. , Elgamal A. , Shantz T. , 2017. Recorded seismic response of the Samoa Channel Bridge-foundation system and adjacent downhole array. Soil Dynamics and Earthquake Engineering, 92: 358—376. doi: 10.1016/j.soildyn.2016.09.034 Yan G. Y. , Chen F. Q. , 2015. Seismic performance of midstory isolated structures under near-field pulse-like ground motion and limiting deformation of isolation layers. Shock and Vibration, 2015: 730612. Yang S. , Mavroeidis G. P. , 2018. Bridges crossing fault rupture zones: a review. Soil Dynamics and Earthquake Engineering, 113: 545—571. doi: 10.1016/j.soildyn.2018.03.027 期刊类型引用(5)
1. 张婷. 基于微信小程序的江苏地震应急联络通讯录设计与实现. 地震科学进展. 2024(02): 159-163 .  百度学术
百度学术2. 符捷,陈梅,刘霞. 基于微信小程序的医院设施设备报修系统设计. 电子设计工程. 2024(19): 58-61+66 .  百度学术
百度学术3. 程树岐,王西宝,刘海刚,王帅合,赵小贺. 地震中心站观测设备实时跟踪管理系统设计与开发. 防灾减灾学报. 2023(03): 52-57 .  百度学术
百度学术4. 刘彦会. 基于自适应遗传算法的微信小程序界面信息布局设计方法. 湖北科技学院学报. 2023(06): 135-140 .  百度学术
百度学术5. 王渊博,李晶,马世鹏. 基于微信小程序的评价工具设计. 湘潭大学学报(自然科学版). 2022(04): 110-117 .  百度学术
百度学术其他类型引用(2)
-





 下载:
下载:

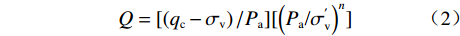

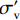






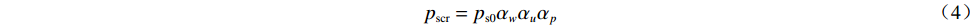
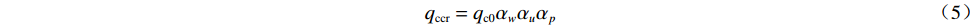
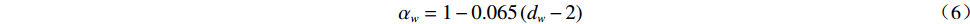
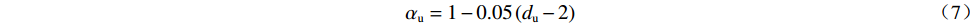



 下载:
下载: