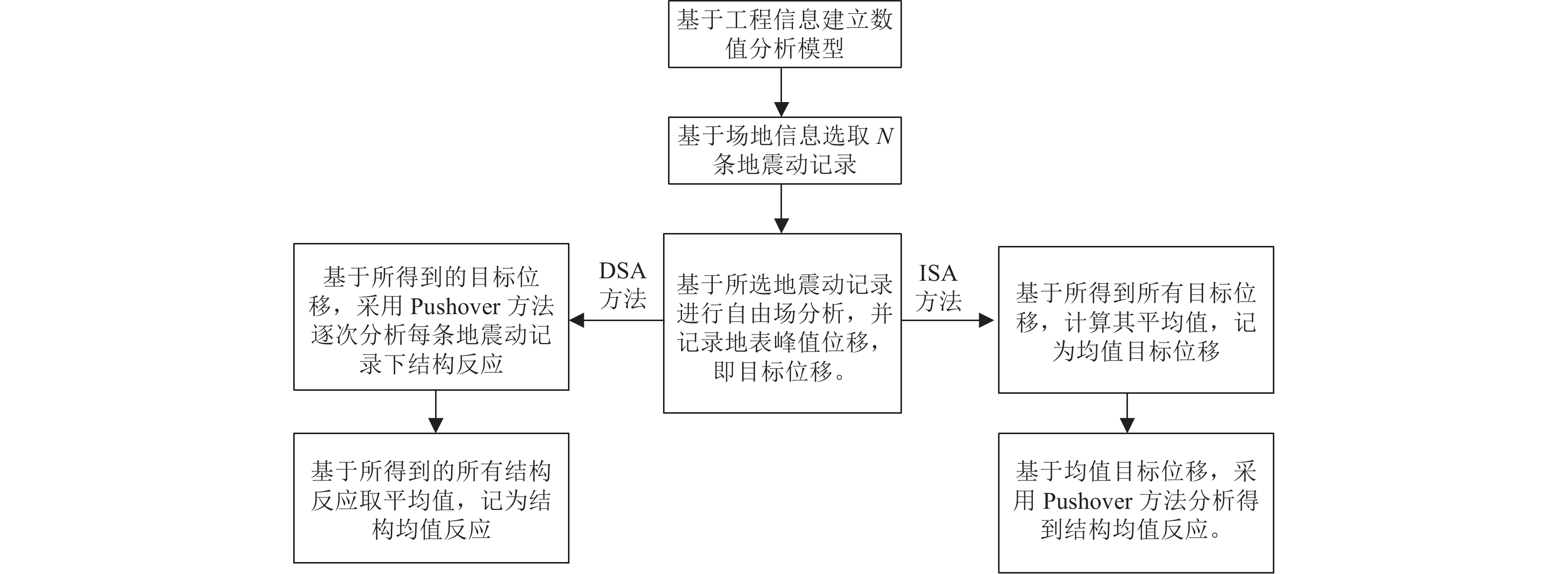
Pushover Analysis Method of Underground Structures Subjected to Multiple Earthquake Records
-
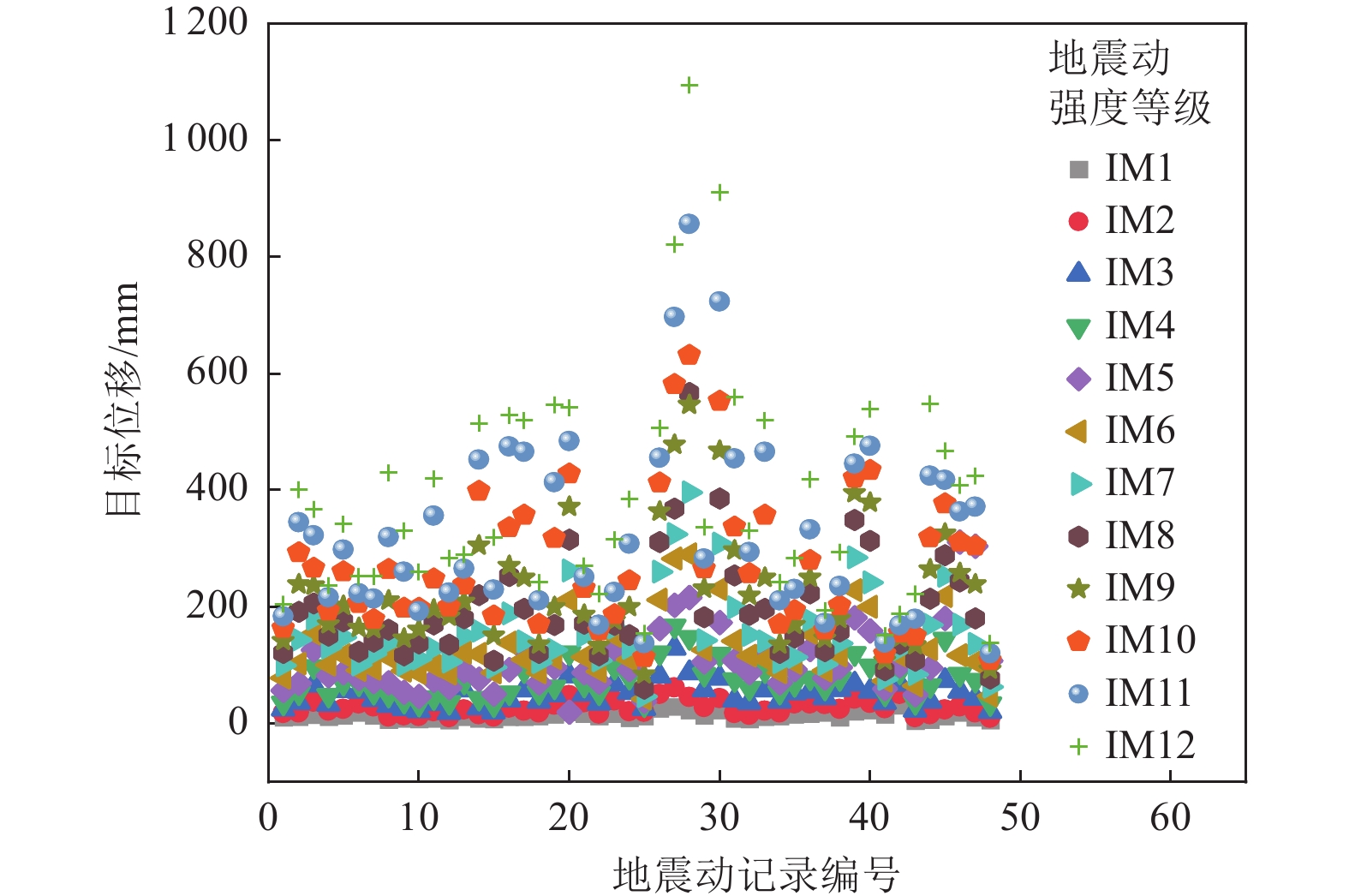
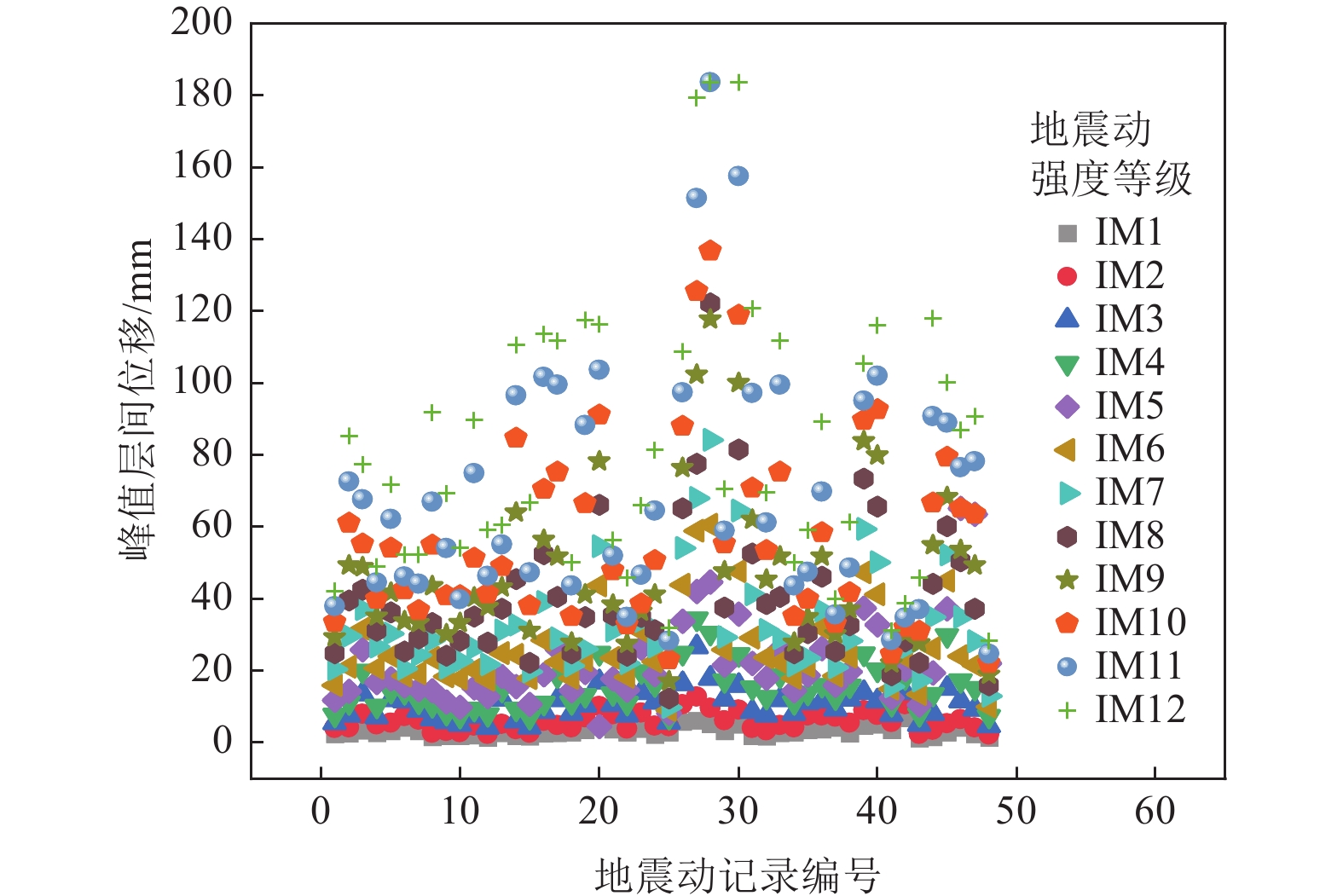
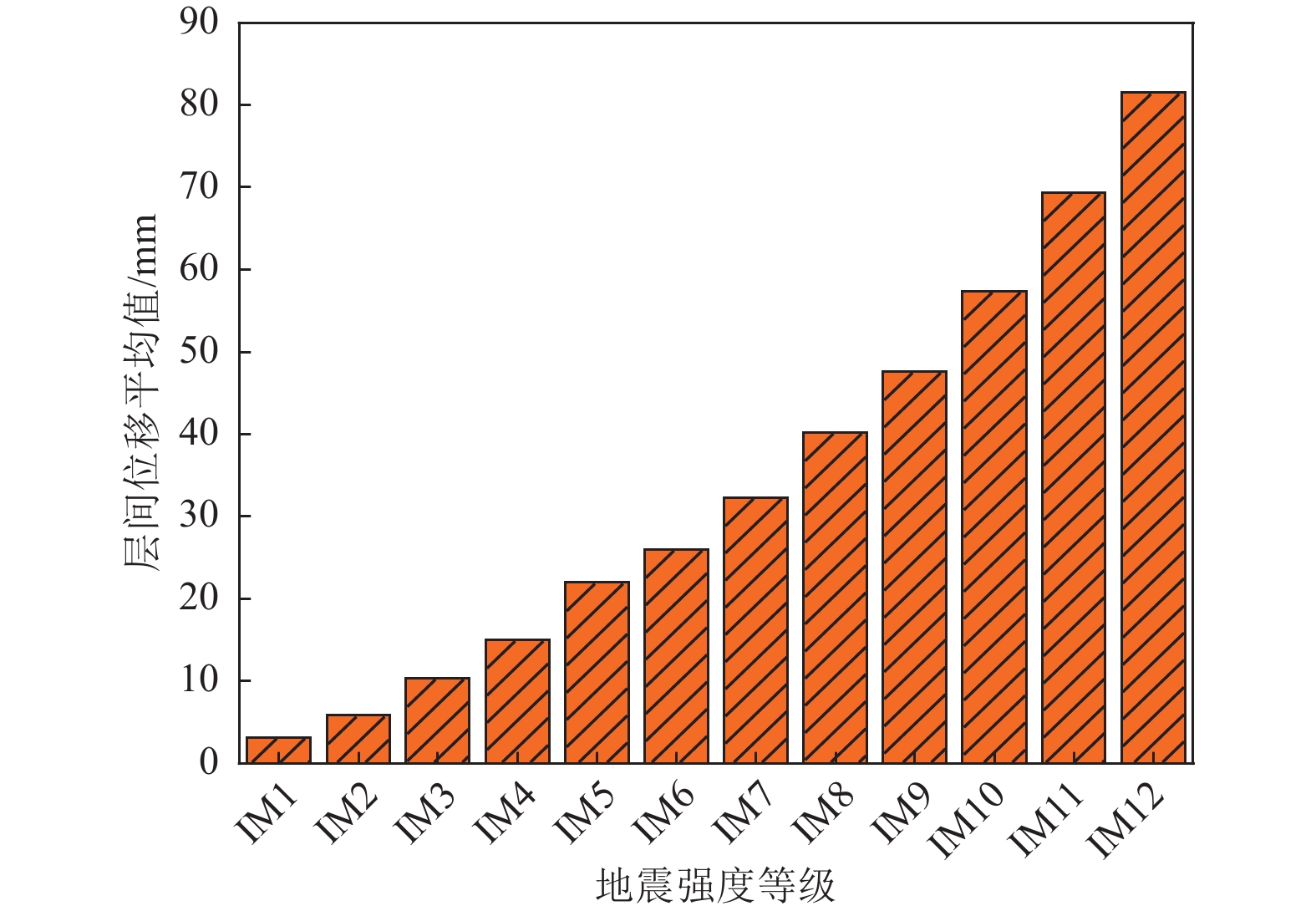
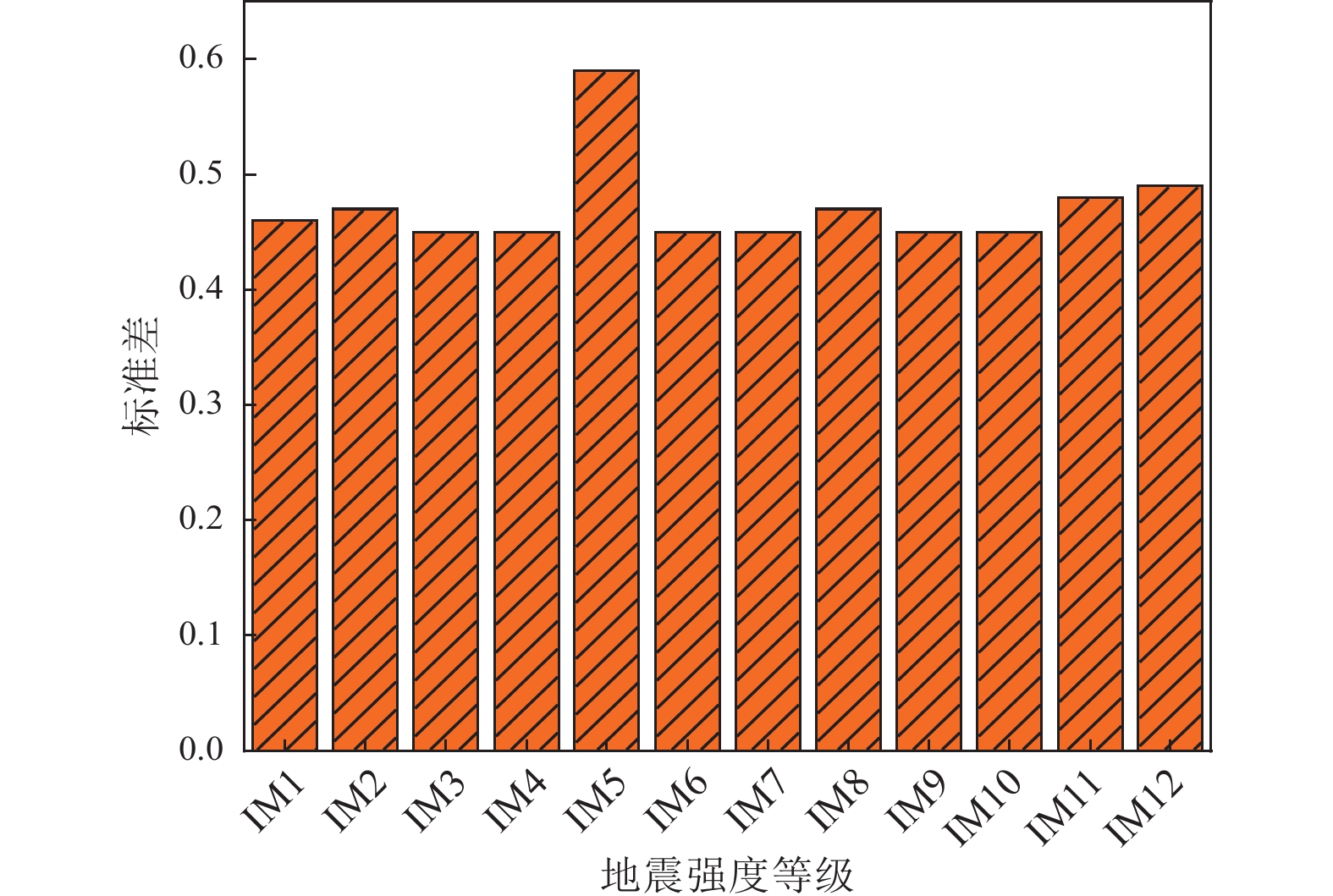
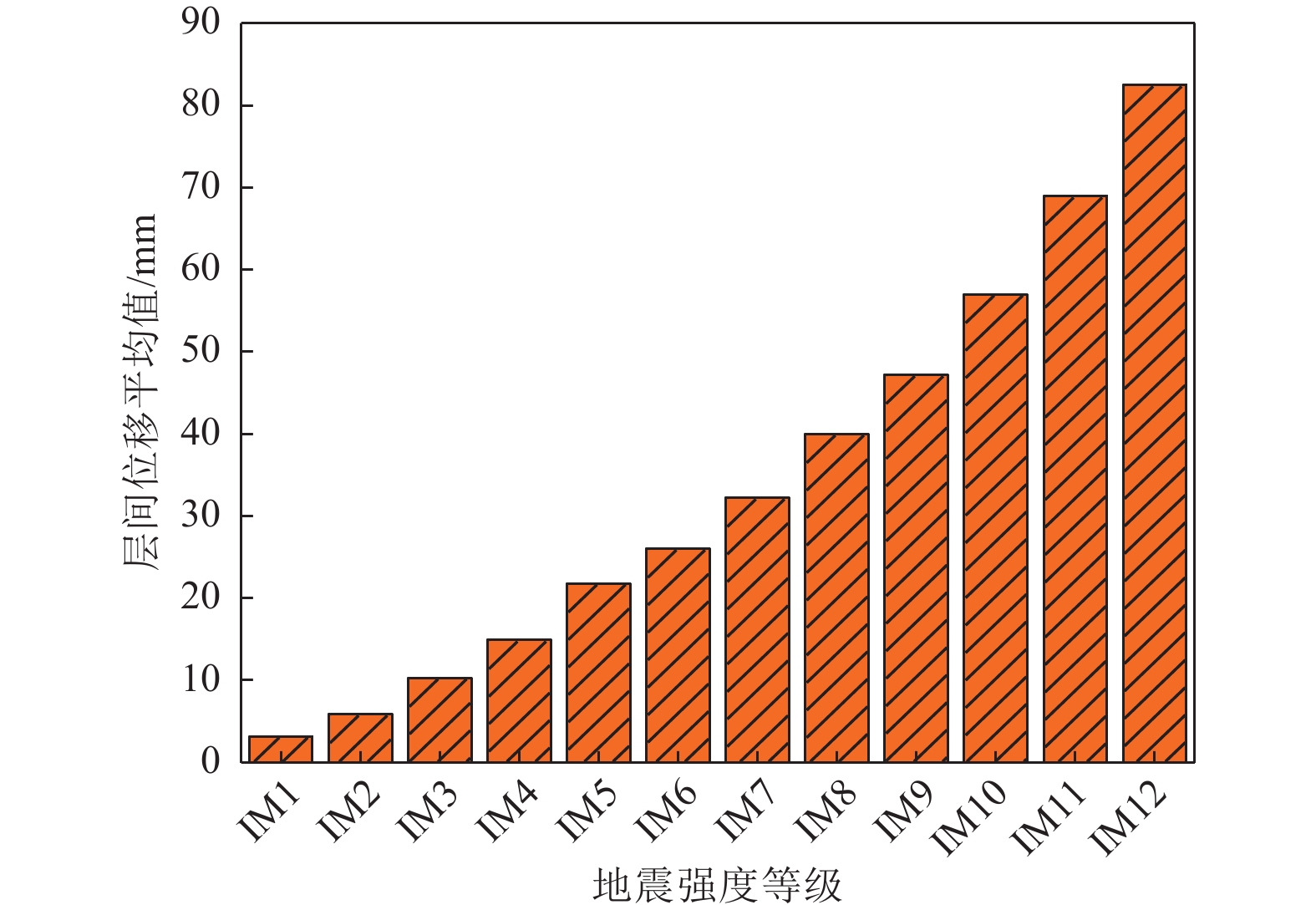
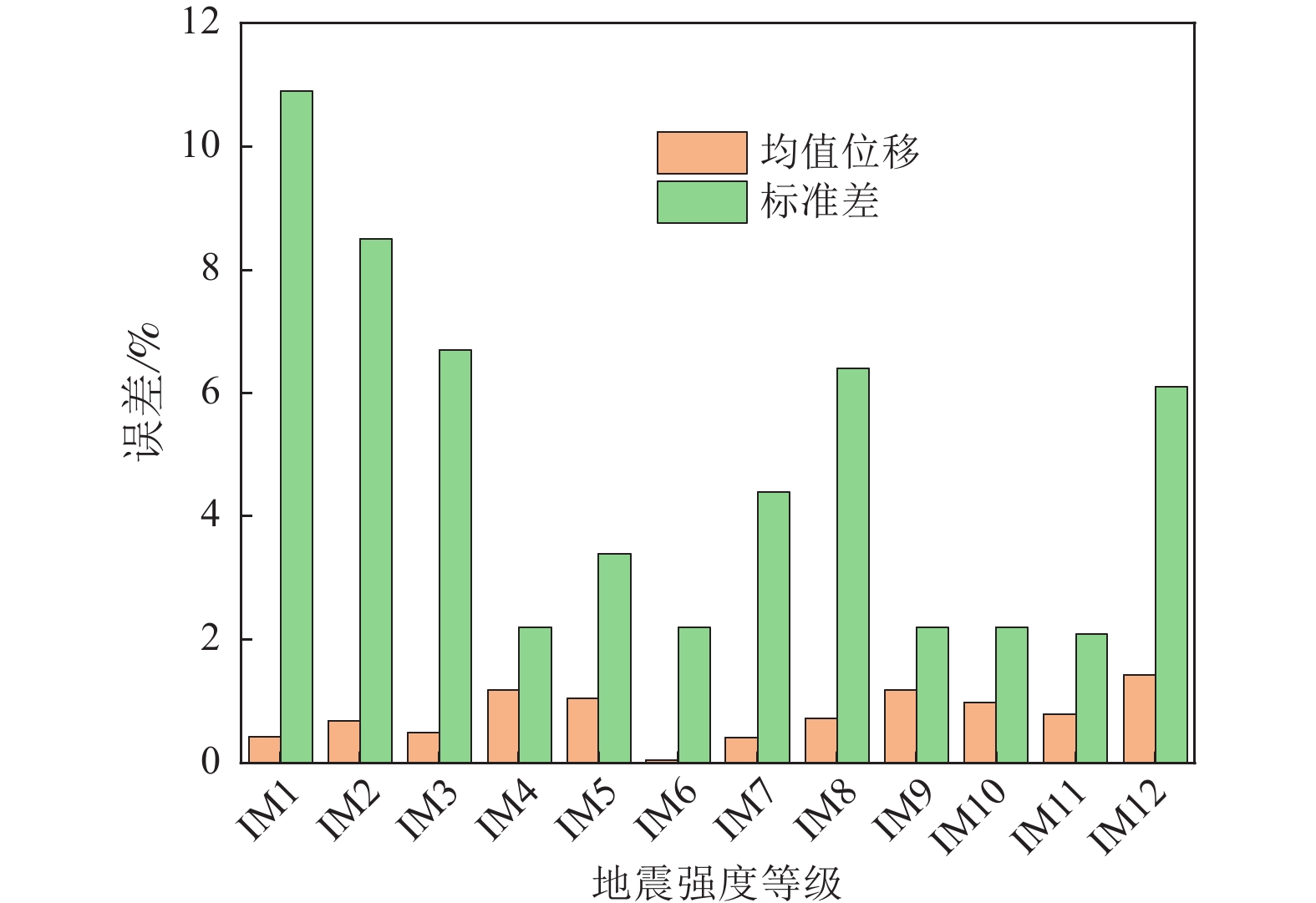
摘要: 求解多地震动作用下结构响应均值与标准差是地震易损性分析的基础工作。在地下结构Pushover分析法的基础上,提出间接计算结构在多地震动作用下均值响应的求解方法,即间接求解法(Indirectly Solution Average,ISA )。该方法以多地震动作用下自由场响应均值为目标位移,采用倒三角荷载分布模式,经1次Pushover分析得到结构响应均值。采用ISA计算得到不同地震动强度(调幅12个强度等级)下地铁车站结构层间位移响应均值与标准差。将ISA计算结果与先进行地下结构Pushover分析,然后求取响应均值与标准差的直接求解法计算结果进行比较,可知2种方法得到的结构层间位移响应均值误差<2%,标准差误差基本<10%,验证了ISA计算精度,可为地下结构基于性能的抗震设计概率地震需求模型计算提供快速分析方法。
-
关键词:
- 地下结构 /
- Pushover分析 /
- 地震动 /
- 地震易损性 /
- 层间位移
Abstract: The computed average and standard deviation of the structural seismic response subjected multiple ground motion records is significant for the seismic vulnerability analysis. Based on the Pushover method of underground structures, this paper proposes a solution method (ISA) to indirectly calculate the average response of structure as it is subjected to multiple ground motion. The ISA method takes the average value of the free field response as the target displacement under the excitation of multiple ground motion records, and uses the inverted triangular load distribution model to calculate the structural average response employing one time Pushover analysis. Based on the ABAQUS software platform, the ISA method is used to calculate the average and standard deviation of the inter-story displacement of the subway station structure under the excitation of 48 ground motion records with 12 levels intensity of ground motion. By comparing with the results obtained from the Pushover analysis conducting the ground motion records one by one, the maximum value of the average error of the inter-story displacement is only 2%, and the standard deviation error is basically less than 10%, which verifies the calculation accuracy of the proposed ISA method. The above conclusions can provide a fast numerical analysis method to establish the probabilistic seismic demand model for performance-based seismic design of underground structures. -
引言
体积式应变仪(简称体应变仪)因工作原理简单、易获取连续可靠的观测资料, 成为国内外主要的钻孔应变观测仪器(中国地震局监测预报司, 2008)。青岛地震台体应变仪采用中国地震局地壳应力研究所研制的TJ-2型体应变仪(苏恺之等, 2003)。2015年以来, 青岛地震台周边相继出现两处较显著的工程施工干扰。2018年需在地震台院内安装CZB钻孔倾斜仪, 进行钻孔施工。2015—2016年降雨偏少, 导致青岛地震台钻孔水位较往年偏低。上述干扰因素对青岛体应变观测资料产生显著影响(高福旺等, 2004;苏恺之等, 2005;李光科等, 2015), 甚至可能掩盖前兆异常信息, 造成异常信息提取困难。本文研究青岛体应变仪长趋势和周期特性, 并分时段分析降雨、钻孔水位、周围工程施工等因素对体应变资料的影响, 得到有益结论, 可为研究地下水位等因素对钻孔体应变观测的影响及今后异常信息的提取提供参考。
1. 台站及观测系统概况
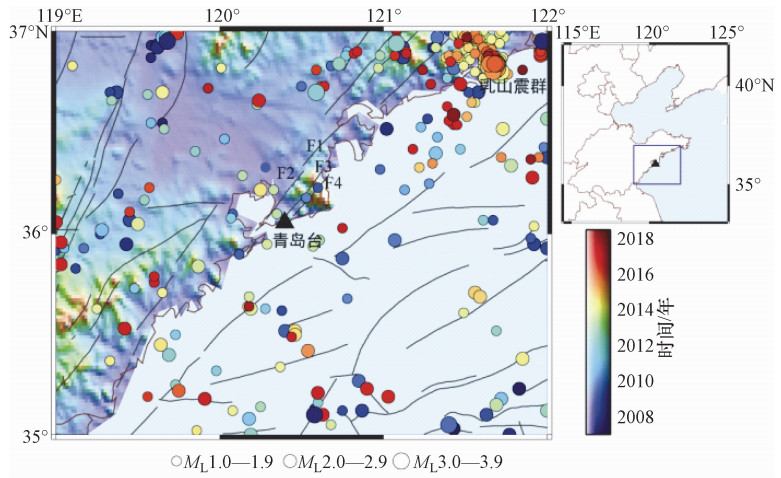
青岛地震台位于山东省青岛市主城区, 青岛市在大地构造上位于华北狼林地块与扬子京畿地块的对接带——胶南超高压变质带上(王红霞等, 2005)。区内主导断裂包括即墨断裂、沧口断裂、劈石口断裂、王哥庄断裂等NNE向构造(见图 1)。青岛地震台紧邻劈石口断裂和王哥庄断裂(张继红等, 2010), 最后一次断错地表活动发生于中更新世末至晚更新世初, 晚更新世以来没有发生活动(栾光忠等, 2009;潘元生等, 2004)。台站基底为破碎花岗岩, 覆盖层厚3m。《山东省历史地震目录》中关于青岛地区史料记载到的历史地震较少, 历史上未发生过ML5.0以上地震。据山东地震台网记录, 青岛地区现代地震活动呈现活动微弱、分布零散、低频度、低强度等特点, 属于少震、弱震地区(王红霞等, 2005;潘元生等, 2004)。地震台周边区域2007年以来主要以ML<4.0地震活动为主, 地震活动较弱, 除东北方向的乳山震群较集中外, 其他地区分布较零散(见图 1)。
 图 1 青岛地震台周围地区断裂分布及地震活动Figure 1. Fault distribution and seismic activity around Qingdao seismic station注:右上方插图表示左侧大图在华北地区的位置, 其中蓝色矩形框为左侧大图所指区域, 黑色三角形表示青岛地震台位置;填色圆圈为2007年以来的地震分布, 圆圈大小表示震级(参考图中下侧图例), 填充颜色表示发震时刻(参考图中右侧图例);黑色实线为断裂分布(晁洪太等, 1997);F1为即墨断裂;F2为沧口断裂;F3为劈石口断裂;F4为王哥庄断裂
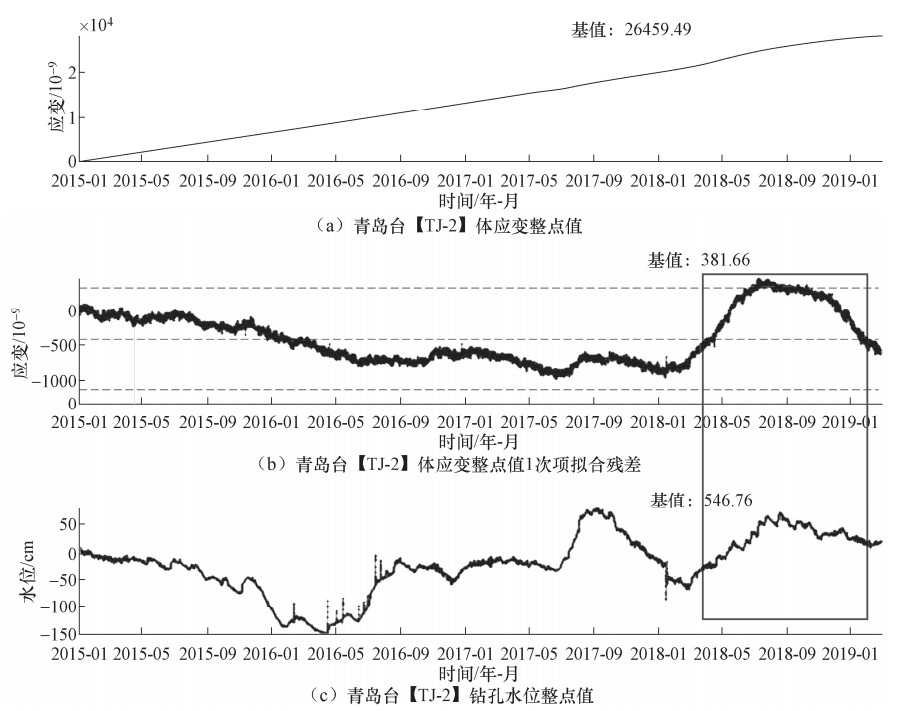
图 1 青岛地震台周围地区断裂分布及地震活动Figure 1. Fault distribution and seismic activity around Qingdao seismic station注:右上方插图表示左侧大图在华北地区的位置, 其中蓝色矩形框为左侧大图所指区域, 黑色三角形表示青岛地震台位置;填色圆圈为2007年以来的地震分布, 圆圈大小表示震级(参考图中下侧图例), 填充颜色表示发震时刻(参考图中右侧图例);黑色实线为断裂分布(晁洪太等, 1997);F1为即墨断裂;F2为沧口断裂;F3为劈石口断裂;F4为王哥庄断裂青岛地震台TJ-2型体应变仪于2006年4月13日安装运行, 可进行辅助钻孔水位观测。仪器所在钻孔孔径130mm, 钻孔深度66m, 仪器安装深度55m。钻孔岩石性质为花岗岩(见表 1), 岩体十分坚硬, 但破碎仅在58—59m、54—55m深度处相对完整。岩石节理面与裂隙的倾角近乎垂直, 受地下水位干扰严重(苏恺之, 2006), 如图 2所示。
表 1 青岛体应变钻孔地层分布Table 1. Distribution of strata in Qingdao volumetric strain borehole层号 层底深度/m 地层描述 1 12.0 强中风化花岗岩, 岩石松软破碎, 矿物成分以石英、长石为主, 岩石结构清楚 2 26.2 硅化石英正长岩, 坚硬破碎, 矿物成分以石英为主 3 34.8 微风化花岗岩, 节理裂隙发育, 较破碎, 矿物成分以石英、长石为主 4 41.7 硅化石英正长岩, 坚硬, 破碎, 矿物成分以石英为主, 接触带有糜棱岩化 5 51.9 微风化花岗岩, 节理裂隙发育, 较破碎, 矿物成分以石英、长石为主 6 56.8 微风化花岗岩, 节理少, 较完整, 矿物成分以石英、长石为主 7 66.2 微风化花岗岩, 硅化石英正长岩, 坚硬, 较破碎, 矿物成分以石英为主 2. 降雨对钻孔水位的影响
降雨是造成钻孔水位变化的常见因素, 由于青岛地震台无降雨观测手段, 所以从青岛市水文局收集降雨观测数据。该观测站与青岛地震台直线距离约250m, 可反映青岛地震台体应变仪所在区域的降水情况。降雨观测属于非连续型数据, 直接观测无法反映降雨对体应变连续的累积影响机制。对降雨资料做以下预处理:
① 将原始雨段观测值转化为每日降雨量序列${P_n}(n = 1, 2, \quad 3, \ldots, m)$, m为序列长度;
② 对每日降雨量序列做累加处理, 即
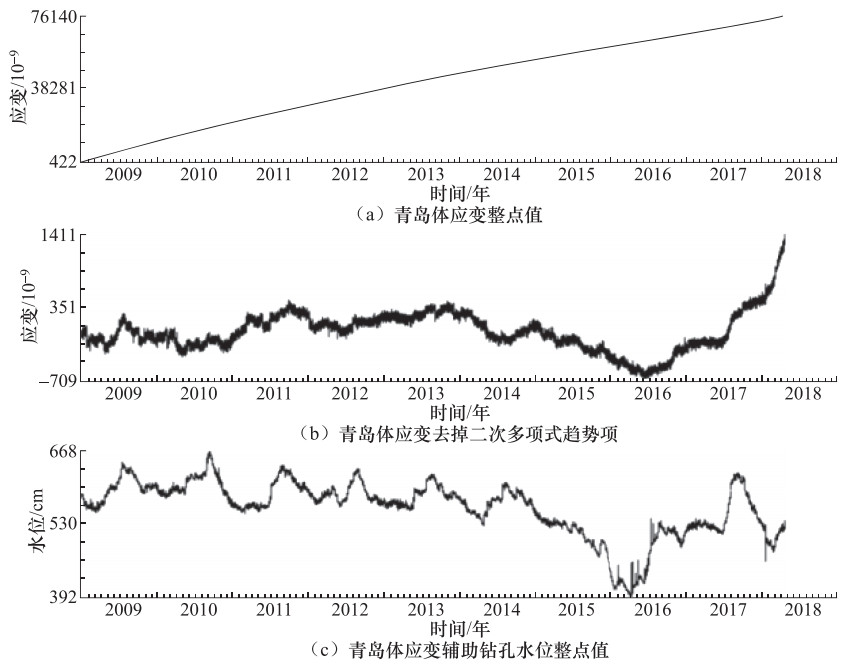
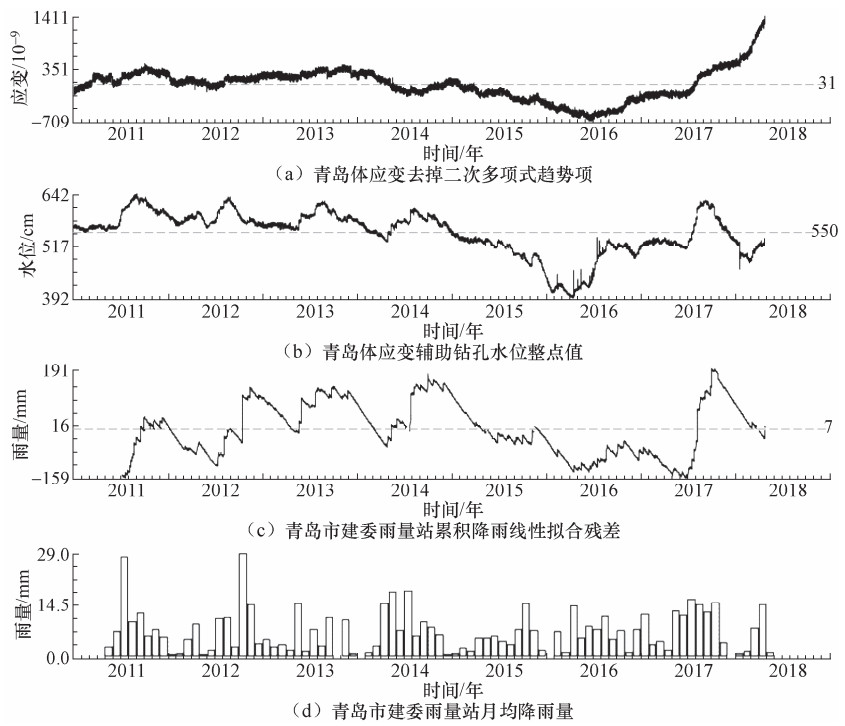
$$ P_n^\prime = \sum\limits_{i = 1}^n {{P_n}} (n = 1, 2, 3, \ldots, m) $$ (1) ③ 将$P_n^\prime $去除线性趋势, 获得累积降雨线性拟合残差序列$P_{n}^{r}$。可见, $P_{n}^{r}$考虑了累积降雨的连续影响, 并反映了各时刻降雨量与正常雨量的偏离程度。$P_{n}^{r}$序列与体应变及钻孔水位观测的对比(见图 3)表明, 青岛地震台钻孔水位与降雨情况(通过累积降雨线性拟合残差$P_{n}^{r}$表示)具有明显的同步特征, 其中2015—2016年降雨偏少, 导致青岛地震台钻孔水位较往年低。可见青岛地震台地下水主要由降雨补给, 降雨下渗改变了钻孔水位, 并影响体应变资料的变化。
3. 工程施工对钻孔应变的影响
岩石孔隙中富含流体时, 会产生孔隙流体压力效应, 岩石孔隙内流体压力称为孔隙压力, 孔隙压力可抵消岩石构造围压作用(曾佐勋等, 2013)。岩石中的有效应力(佘成学等, 2010)可描述为:
$$ \sigma^{\prime}=\sigma-\beta P_{\mathrm{w}} $$ (2) 其中, σ为岩石中的构造应力;β为孔隙压力作用系数;Pw为孔隙水压力。台站周围区域地震活动(见图 1)表明青岛地震台近期地震活动和构造应力较平稳, 而钻孔水位和钻孔环境的变化对β、Pw产生影响, 因此可认为青岛体应变所反映的应力变化主要由式(2)中的βPw项产生。
2015年后青岛地震台周边开始两处施工:一处为台站向北150m处的青岛市立医院住院大楼基坑开挖, 另一处为台站向北850m处的青岛市地铁2号线(距青岛地震台最近的地铁线)燕儿岛站施工。青岛市立医院住院大楼工程总建筑面积86000m2, 设计地上15层、地下3层, 于2015—2016年进行基坑开挖。青岛市地铁2号线呈东西走向, 根据青岛新闻网记者2015年5月30日的报道(吴帅等, 2015), 地铁2号线浮燕区间采用盾构机施工, 该区间地质条件复杂, 存在富水砂层、破碎带及上软下硬地层等不良地质, 于2016年7月29日完成土建施工。这两处大规模的工程施工涉及基坑开挖, 改变台站周边的岩石应力应变状态, 影响岩石孔隙压力作用系数, 对应变仪观测造成影响。
2018年1月17—18日进行青岛地震台距体应变观测井8m的CZB钻孔倾斜仪钻孔, 先用XY-3-500型岩芯钻机装备金刚石钻头钻进, 但由于台站岩石坚硬, 钻进速度缓慢, 后改用400型潜孔锤钻机负责钻孔钻进和护井管安装。该钻机利用压缩空气作为循环介质, 并作为驱动孔低冲击器的能源, 使之上下往复运动, 并连续不断地对下部钻具施加一定频率的冲击载荷, 进行冲击回转钻进(王达等, 2014)。钻进过程中, 潜孔锤利用对钻头的往复冲击作用破碎岩石, 并将岩屑排出至地表。潜孔锤钻机较岩芯钻机钻进速率快, 但钻孔孔径大, 且对钻孔岩体具有更大的破坏性。CZB钻孔和体应变钻孔均穿过了含水层, 钻孔施工可能对区域岩石孔隙环境造成影响, 引起体应变钻孔水位的永久阶变(见图 4)。当岩石孔隙裂缝增多, 岩石渗透率增大时, 直接扩大孔隙压力作用系数, 同样的水位上升变化引起更大的孔隙水压力, 导致体应变观测出现加速上升, 可解释2018年3月以来水位小幅上升引起体应变显著压性上升的现象。最新的观测数据(见图 5)表明, 2018年1月17日(钻孔后)至2019年1月(青岛体应变仪工作状态开始改变)受近距离钻井的影响, 钻孔应变井周边岩石孔隙环境及水位对体应变的影响作用显著增强(见图 5), 验证了上述推测。
4. 工程施工对钻孔水位与体应变相关性的影响
青岛体应变存在稳定的长趋势变化特征(见图 2), 分离长趋势变化对资料趋势异常识别至关重要。通常以多项式(3)确定仪器的长趋势项:
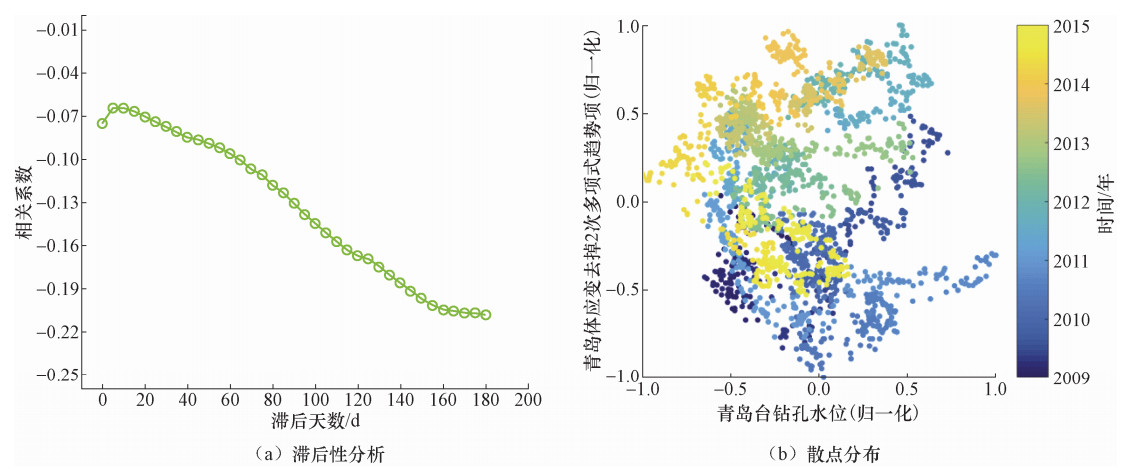
$$ Y = {a_n}{x^n} + {a_{n - 1}}{x^{n - 1}} +, \ldots, + {a_2}{x^2} + {a_1}{x^1} + {a_0}\left({{a_n} \ne 0} \right) $$ (3) 已有研究表明, 钻孔水位是体应变常见的趋势性干扰因素(马震等, 2011;卢双苓等, 2012), 可改变观测的短时趋势, 将体应变观测资料的长趋势项正确分离, 可提高体应变与钻孔水位的相关性。因此, 可利用钻孔水位与体应变的相关性检验确定多项式的阶数。考虑长期特征及稳定特性, 尽量通过低阶多项式进行描述, 当低阶多项式不能描述时增加阶数。通过取n=1, 2, 3, 4, 5逐次对观测数据进行多项式拟合, 并分析分离长趋势项后的体应变与钻孔水位的相关性, 通过最优相关系数确定多项式阶数。考虑2015年1月以来青岛体应变辅助钻孔水位出现显著变化, 分2009—2018年和2015—2018年两个时段对体应变长趋势项进行研究。由于长趋势项不考虑固体潮因素, 为减少数据量, 将整点值观测数据转为日值数据进行相关性分析。本文利用线性相关系数表示相关性, 并对其进行T检验, 计算结果(见表 2)表明, 青岛体应变2009年以来长趋势项呈二次多项式分布, 而2015年以来的长趋势项呈一次多项式分布, 可利用式(3)对青岛体应变分离长趋势项, 并研究钻孔水位的影响。
表 2 青岛体应变去除趋势后与钻孔水位的相关系数Table 2. Correlation coefficients between the detrended Qingdao volumetric strain and borehole water level多项式阶数 2009年1月1日至2018年4月25日(样本数3394) 2015年1月1日至2018年4月25日(样本数1210) 相关系数 T检验显著性/% 相关系数 T检验显著性/% 1 0.12 100.0 0.35 100.0 2 0.43 100.0 0.07 98.5 3 0.27 100.0 0.33 100.0 4 0.20 100.0 0.29 100.0 5 0.20 100.0 0.29 100.0 由图 2、表 2中的相关性分析可知, 青岛体应变受钻孔水位的影响。2015年后, 青岛体应变与钻孔水位的相关性出现显著变化, 开始呈现具有滞后特征的相关性(见表 3)。
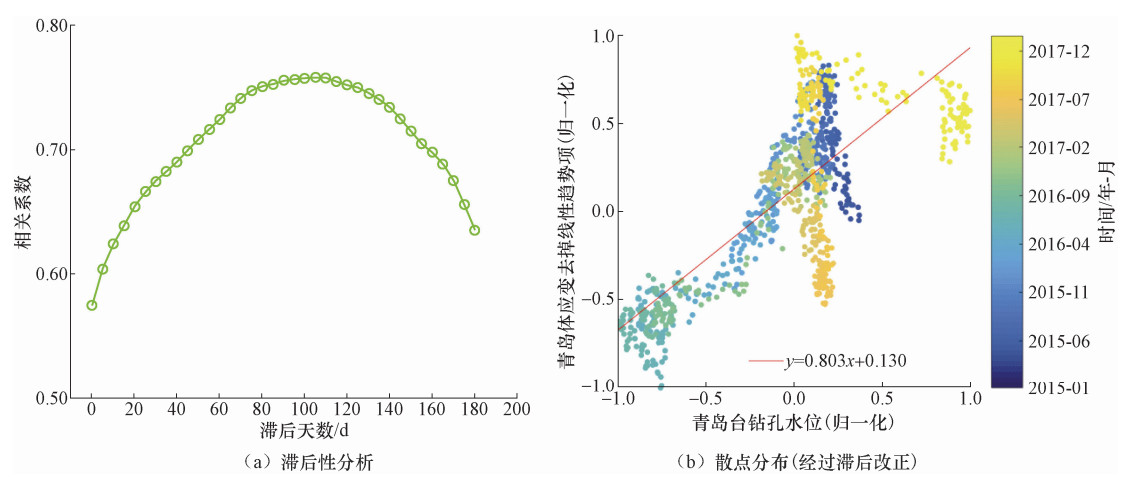
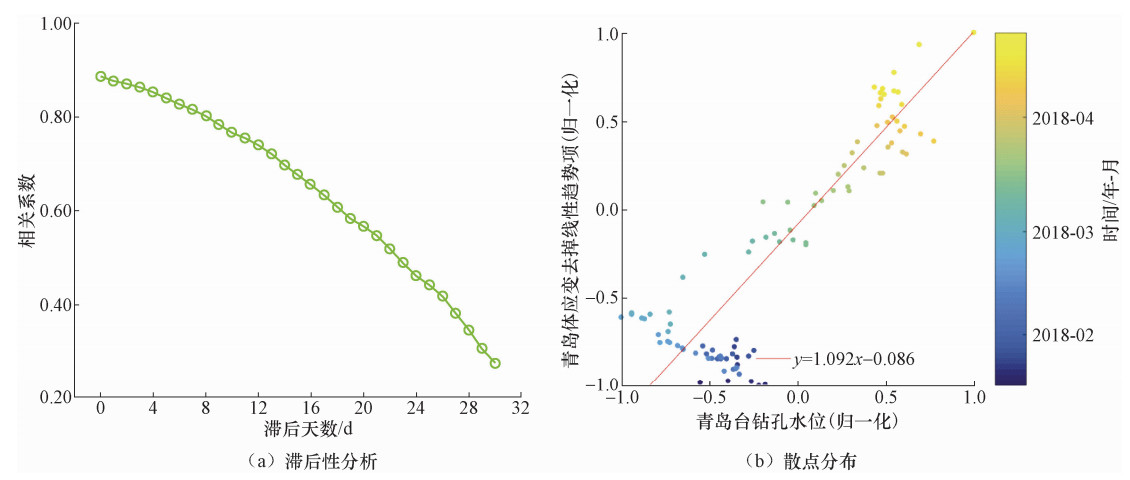
表 3 青岛体应变与钻孔水位滞后相关性Table 3. Lag correlation between Qingdao volumetric strain and borehole water level序号 时间段 相关系数 样本数 滞后特征 最优相关系数 1 2009-01-01—2015-01-01 -0.09 2191 不显著 — 2 2015-01-02—2018-01-17 0.57 1112 显著(105d) 0.76 3 2018-01-18—2018-04-25 0.89 97 不显著 — 为研究滞后性, 对于钻孔水位观测数据, 进行一定的滞后改正后分析相关性, 即在一定时间范围内不断调整滞后改正时间, 通过收敛至最优相关系数确定最优滞后时间。如果给定时间范围内未收敛至最优相关系数, 则认为滞后特征不显著。考虑季节性周期因素, 滞后时间范围设定为0—180d。考虑2015年1月以来青岛体应变辅助钻孔水位出现显著变化、2015—2017年青岛降雨量减少及台站周边存在工程施工干扰、2018年1月17日在距体应变钻孔8m处存在显著的钻孔施工干扰等情况, 分3个时间段进行分析计算, 时间段划分及计算结果如表 3所示。
2009年1月1日至2015年1月1日青岛体应变与钻孔水位相关性相对较弱(相关系数为-0.09), 在给定的时间范围内调整滞后改正时间未显著收敛至最优相关系数(见图 6(a)), 未表现出显著的滞后特性, 相关性较低, 使得两者的散点分布非常零散(见图 6(b))。2015年1月2日至2018年1月17日青岛体应变与钻孔水位呈现显著的线性相关性(相关系数为0.57), 但具有滞后特征(见图 7(a)), 在给定的时间范围内调整滞后改正105d时显著收敛至最优相关系数, 此时, 两者的散点分布呈线性分布(见图 7(b)), 可用线性函数y=0.803x+0.130拟合, 相关系数提高至0.76。2018年1月18日至2018年4月25日两者依然显著相关(相关系数为0.89), 且滞后特征消失(见图 8(a)), 两者几乎同步变化(见图 8(b)), 可用线性函数y=1.092x+0.086拟合。上述结果表明青岛体应变与钻孔水位的相关性在2015年前后出现变化, 2015年以来青岛地震台钻孔水位对体应变的影响作用趋于增强。
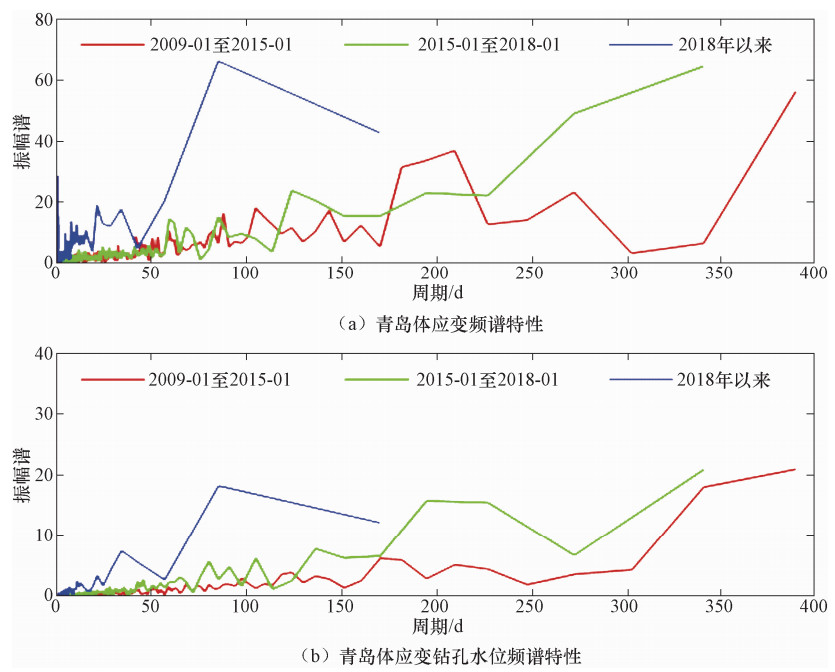
利用傅里叶变换分析表 3中3个时段青岛体应变及钻孔水位周期特性(见图 9), 周期特性基于整点值数据进行计算。2009年1月1日至2015年1月1日, 青岛体应变除具有固体潮引起的0.5d、1d周期特性外, 还表现出显著的年、半年周期特性。该时段内钻孔水位仅有年周期特性较明显, 两者400d周期以内的振幅谱曲线相关性较低。2015年1月2日至2018年1月17日, 青岛体应变半年周期特性有所衰弱, 半年至一年周期特性增强, 两者400d周期以内的振幅谱曲线相关性显著提高。2018年1月18日以来, 由于观测时间较短, 未能展示完整的周期特性。青岛体应变及钻孔水位的周期特性分析结果也表明两者的相关性在2015年前后出现显著变化。
5. 结论
通过分析降雨、工程施工、钻孔水位等因素对青岛体应变的影响, 得出以下结论:
(1) 青岛地震台钻孔水位反映的区域地下水主要由降雨补给, 降雨下渗改变地下水状态, 进而影响青岛体应变的资料变化。
(2) 青岛体应变与钻孔水位的相关性在2015年前后出现变化, 2015年1月2日至2018年1月17日表现出滞后相关性。两者的周期特性在2015年前后也出现变化, 可能与2015—2016年受降雨偏少引起的低钻孔水位及周边工程施工干扰有关。
(3) 青岛体应变与钻孔水位在CZB钻孔倾斜仪钻孔施工前后几乎同步变化, 很可能是由于青岛地震台CZB钻孔倾斜仪钻孔施工对区域岩石孔隙环境造成了影响。当岩石孔隙裂缝增多, 岩石渗透率增大, 直接扩大孔隙压力作用系数, 同样的水位上升变化将引起更大的孔隙水压力, 导致体应变观测出现加速上升, 也可解释2018年3月以来水位小幅上升引起体应变显著压性上升的现象。
致谢: 感谢青岛市水文局提供的降雨资料和山东省地震台网中心提供的地震目录数据。 -
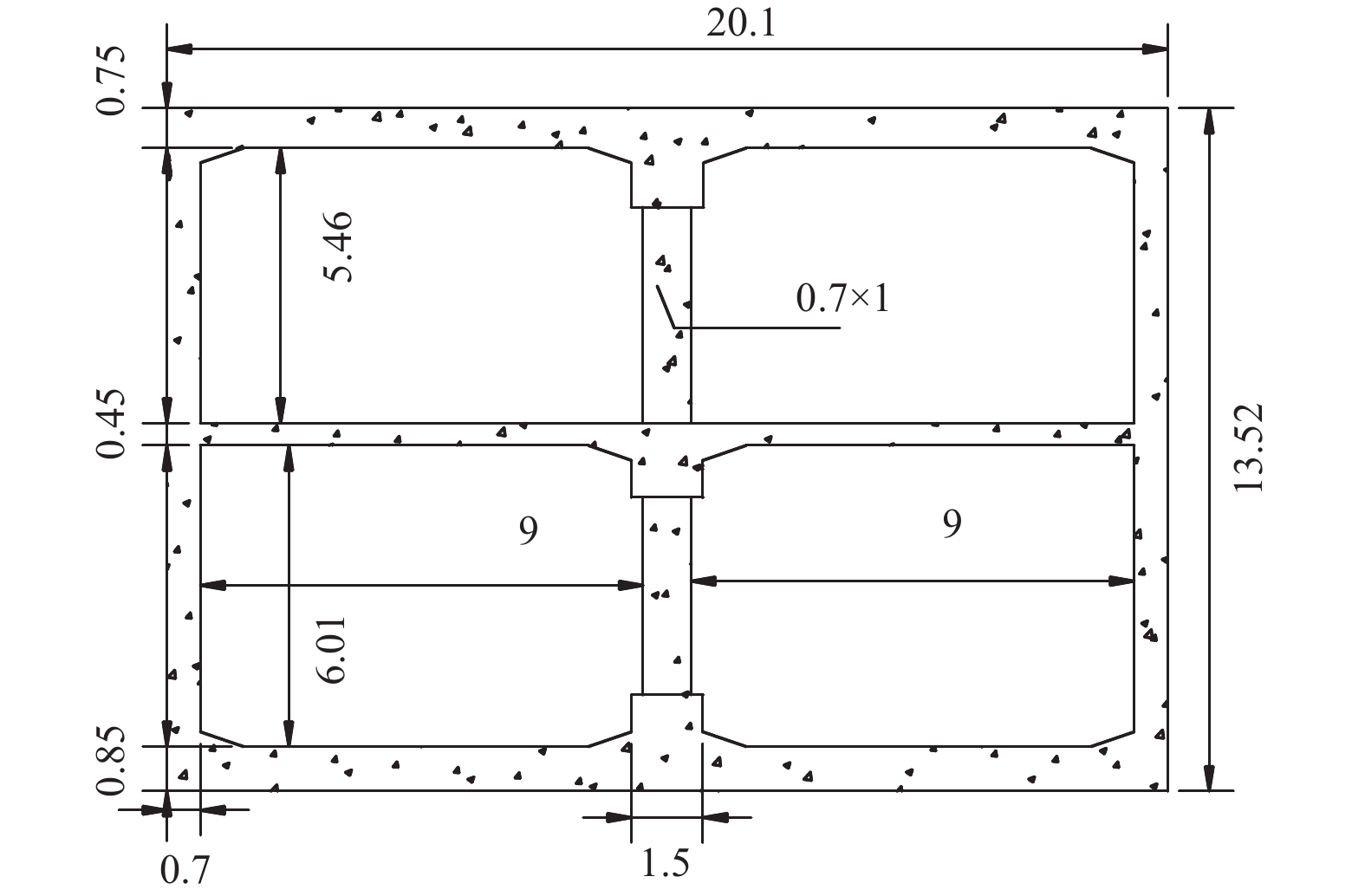
表 1 车站场地基本信息
Table 1. The basic site information of caofang subway station
编号 类型 厚度/m 密度/(kg·m−3) 剪切波速/(m·s−1) 泊松比 1 回填土 2 1 700 147 0.35 2 粉土 6 2 020 190 0.35 3 黏土 7 2 000 193 0.36 4 砂土 2 2 050 274 0.33 5 黏土 10 2 020 300 0.36 6 砂土 3 2 050 328 0.33 7 黏土 2 1 990 331 0.36 8 砂土 6 2 050 368 0.33 9 粉土 7 2 000 382 0.35 10 基岩 — 2 500 — — -
陈国兴, 陈苏, 杜修力等, 2016. 城市地下结构抗震研究进展. 防灾减灾工程学报, 36(1): 1—23 doi: 10.13409/j.cnki.jdpme.2016.01.001Chen G. X. , Chen S. , Du X. L. , et al. , 2016. Review of seismic damage, model test, available design and analysis methods of urban underground structures: retrospect and prospect. Journal of Disaster Prevention and Mitigation Engineering, 36(1): 1—23. (in Chinese) doi: 10.13409/j.cnki.jdpme.2016.01.001 还毅, 方秦, 柳锦春等, 2011. 考虑时空耦联的等效惯性加速度加载的地下结构pushover分析. 地震工程与工程振动, 31(4): 64—70 doi: 10.13197/j.eeev.2011.04.015Huan Y. , Fang Q. , Liu J. C. , et al. , 2011. Pushover analysis of underground structures by the time-space coupled equivalent inertial acceleration. Journal of Earthquake Engineering and Engineering Vibration, 31(4): 64—70. (in Chinese) doi: 10.13197/j.eeev.2011.04.015 刘晶波, 李彬, 刘祥庆, 2007. 地下结构抗震设计中的静力弹塑性分析方法. 土木工程学报, 40(7): 68—76 doi: 10.3321/j.issn:1000-131x.2007.07.012Liu J. B. , Li B. , Liu X. Q. , 2007. A static elasto-plastic analysis method in seismic design of underground structures. China Civil Engineering Journal, 40(7): 68—76. (in Chinese) doi: 10.3321/j.issn:1000-131x.2007.07.012 刘晶波, 刘祥庆, 李彬, 2008. 地下结构抗震分析与设计的Pushover分析方法. 土木工程学报, 41(4): 73—80 doi: 10.3321/j.issn:1000-131X.2008.04.011Liu J. B. , Liu X. Q. , Li B. , 2008. A pushover analysis method for seismic analysis and design of underground structures. China Civil Engineering Journal, 41(4): 73—80. (in Chinese) doi: 10.3321/j.issn:1000-131X.2008.04.011 刘晶波, 王文晖, 赵冬冬等, 2013. 循环往复加载的地下结构Pushover分析方法及其在地震损伤分析中的应用. 地震工程学报, 35(1): 21—28 doi: 10.3969/j.issn.1000-0844.2013.01.0021Liu J. B. , Wang W. H. , Zhao D. D. , et al. , 2013. Pushover analysis method of underground structures under reversal load and its application in seismic damage analysis. China Earthquake Engineering Journal, 35(1): 21—28. (in Chinese) doi: 10.3969/j.issn.1000-0844.2013.01.0021 许成顺, 许紫刚, 杜修力等, 2017. 地下结构抗震简化分析方法比较研究. 地震工程与工程振动, 37(2): 65—80 doi: 10.13197/j.eeev.2017.02.65.xucs.008Xu C. S. , Xu Z. G. , Du X. L. , et al. , 2017. Comparative study of simplified methods for seismic analysis of underground structure. Earthquake Engineering and Engineering Dynamics, 37(2): 65—80. (in Chinese) doi: 10.13197/j.eeev.2017.02.65.xucs.008 Chen G. X. , Wang Y. Z. , Zhao D. F. , et al. , 2021. A new effective stress method for nonlinear site response analyses. Earthquake Engineering & Structural Dynamics, 50(6): 1595—1611. Chen Z. Y., Chen W., Zhang W., 2014. Seismic performance evaluation of multi-story subway structure based on pushover analysis. In: Linag R. Y., Qian J. G., Tao J. L., eds., Advances in Soil Dynamics and Foundation Engineering. Shanghai, China: ASCE, 444—454. FEMA, 2009. Quantification of building seismic performance factors. Washington: Federal Emergency Management Agency. FEMA, 2018. Seismic performance assessment of buildings (volume 1-Methodology). 2 nd ed. Washington: Federal Emergency Management Agency. Gao Z. D. , Zhao M. , Du X. L. , 2022. Modal pushover analysis for seismic performance assessment of underground structures. Journal of Earthquake Engineering, 1—19. Hashash Y. M. A. , Hook J. J. , Schmidt B. , et al. , 2001. Seismic design and analysis of underground structures. Tunnelling and Underground Space Technology, 16(4): 247—293. doi: 10.1016/S0886-7798(01)00051-7 Hillerborg A. , Modéer M. , Petersson P. E. , 1976. Analysis of crack formation and crack growth in concrete by means of fracture mechanics and finite elements. Cement and Concrete Research, 6(6): 773—781. doi: 10.1016/0008-8846(76)90007-7 Jiang J. W. , Xu C. S. , El Naggar H. M. , et al. , 2021. Improved pushover method for seismic analysis of shallow buried underground rectangular frame structure. Soil Dynamics and Earthquake Engineering, 140: 106363. doi: 10.1016/j.soildyn.2020.106363 Qiu D. P. , Chen J. Y. , Xu Q. , 2020. Improved pushover analysis for underground large-scale frame structures based on structural dynamic responses. Tunnelling and Underground Space Technology, 103: 103405. doi: 10.1016/j.tust.2020.103405 Vamvatsikos D. , Cornell C. A. , 2002. Incremental dynamic analysis. Earthquake Engineering & Structural Dynamics, 31(3): 491—514. Xu Z. G. , Du X. L. , Xu C. S. , et al. , 2019. Numerical research on seismic response characteristics of shallow buried rectangular underground structure. Soil Dynamics and Earthquake Engineering, 116: 242—252. doi: 10.1016/j.soildyn.2018.10.030 Zhuang H. Y. , Yang J. , Chen S. , et al. , 2021. Statistical numerical method for determining seismic performance and fragility of shallow-buried underground structure. Tunnelling and Underground Space Technology, 116: 104090. doi: 10.1016/j.tust.2021.104090 Zou Y. , Liu H. B. , Jing L. P. , et al. , 2017. A pseudo-static method for seismic responses of underground frame structures subjected to increasing excitations. Tunnelling and Underground Space Technology, 65: 106—120. doi: 10.1016/j.tust.2017.02.006 期刊类型引用(6)
1. 朱成林,甘卫军,贾媛,徐芳芳,张正帅,鞠佳斌,冯志军,刘克辉. 工程降水对钻孔应变的干扰特征及干扰机制研究. 地球物理学报. 2024(04): 1398-1411 .  百度学术
百度学术2. 郑家军,李铂,朱成林,黄金水,杨立涛,董敏,熊玮,韩博,刘海林,池国民,吴婧. 2022年青州M_L4.1地震前后的重力场变化分析. 地震. 2023(03): 102-119 .  百度学术
百度学术3. 郑家军,朱成林,熊玮,李铂,杨立涛,董敏,韩博,闫德桥,刘海林,池国民. 累计去趋势法在分析降水与形变关系中的应用. 内陆地震. 2023(04): 392-398 .  百度学术
百度学术4. 宫杰,张朋,张扬,张敏,王佳,居海华. 江苏省测震台网井下地震计方位角检测. 震灾防御技术. 2022(01): 181-187 .  本站查看
本站查看5. 张录,张剑锋,周康云,候赛因·赛买提,木巴拉克·江布尔拜. 2020年3月20日蒙古M_S 5.9地震前地倾斜资料异常特征分析. 地震地磁观测与研究. 2022(03): 109-117 .  百度学术
百度学术6. 张录,周丹,陈鲁刚. 气象要素对巴里坤水平摆倾斜观测的影响. 地震地磁观测与研究. 2022(06): 107-115 .  百度学术
百度学术其他类型引用(0)
-




 下载:
下载:








 下载:
下载: