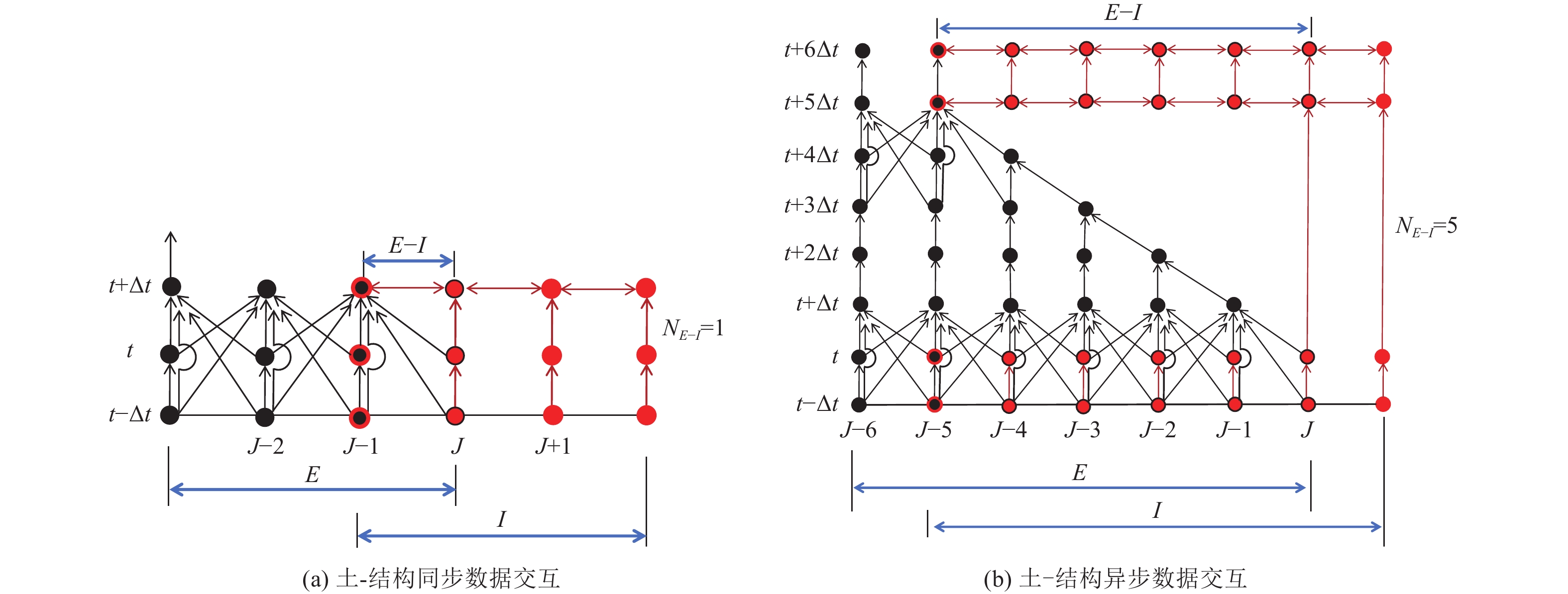
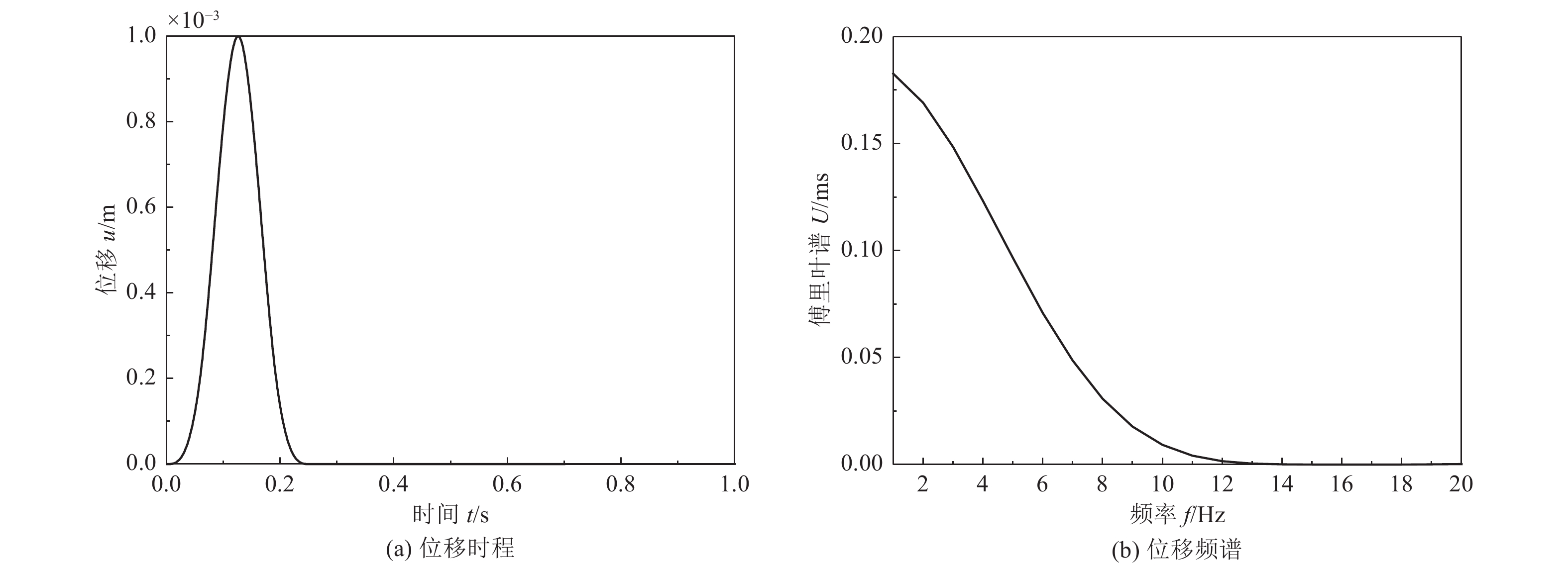
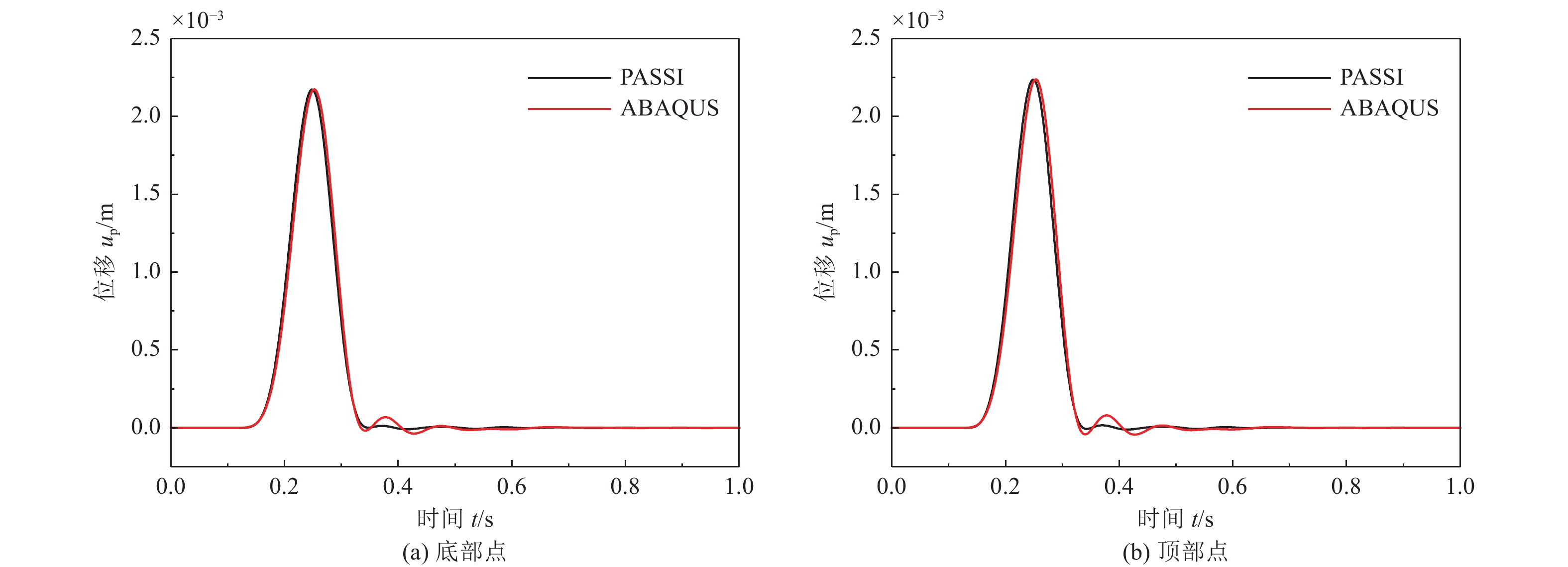
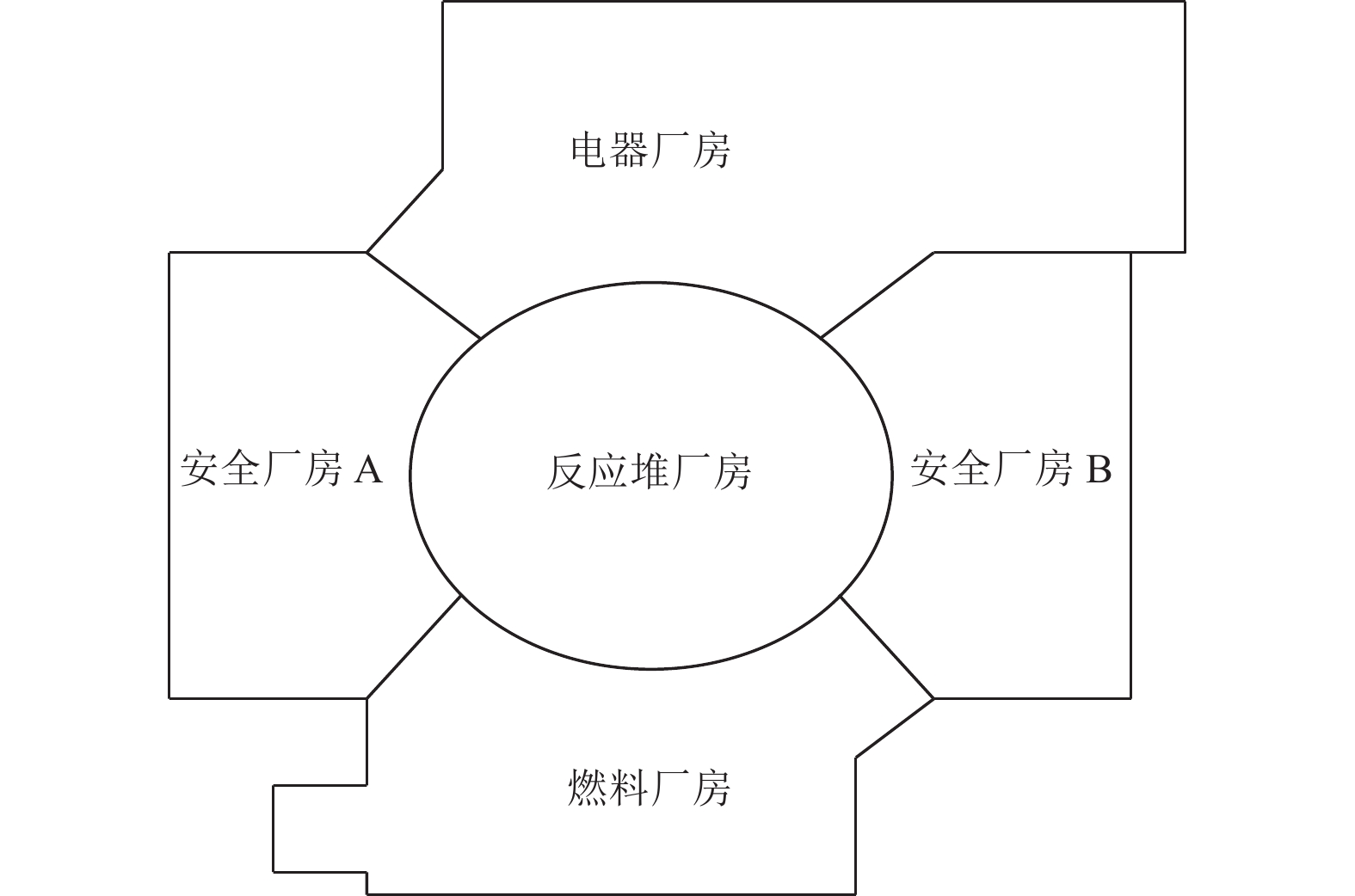
Earthquake Response Analysis of Soil-reactor Plant-nuclear power Auxiliary Plant Interaction System
-
摘要: 土-结构相互作用分析是核电结构抗震设计的重要环节，考虑到附属厂房可能导致反应堆厂房处于最不利工况状态，对地基土-反应堆厂房-核电辅助厂房结构相互作用体系地震响应进行研究。基于PASSI算法，提出显-隐式单元层计算方法，实现显、隐式交替计算，保持高效性的同时，提高计算稳定性。通过场地分析算例和土-结构相互作用分析算例，与ABAQUS软件计算结果进行对比，验证计算方法的可行性。以某核电站为对象，分析同一基础上相邻厂房对反应堆厂房地震响应的影响。研究结果表明，在基岩场地上，安全厂房通过基础和场地对反应堆厂房的作用较小，对反应堆厂房地震响应的影响较小；地震动输入下，燃料厂房和电器厂房加大了反应堆厂房位移峰值，减小了反应堆厂房加速度反应谱峰值，并使反应堆厂房顶部点加速度反应谱峰值向高频移动；与反应堆厂房共用同一基础的辅助厂房，应与反应堆厂房作为整体进行地震响应分析，至少应将与反应堆厂房相连的辅助厂房作为整体进行分析。Abstract: Soil-structure interaction analysis is an important step in seismic design, Considering that the existence of the auxiliary plant may cause the reactor plant in the most unfavorable condition, In the paper the coupled dynamic response of soil- reactor plant-auxiliary plant structure system to earthquake excitation is studied. Based on PASSI, a computation scheme of explicit and implicit element layer is proposed, which can realize explicit and implicit computation alternately, and improve the computation stability with high efficiency. The site analysis and soil-structure interaction analysis examples were compared with the results of ABAQUS commercial software, and the feasibility of the calculation scheme was verified. On this basis, the influence of adjacent plants on the seismic response of a nuclear power plant on the same basis was analyzed. The results show that on the bedrock site, the safety plant has little effect on the reactor plant through the foundation and site, It also has little effect on the seismic response of the reactor plant. Under the seismic wave input, the fuel and electrical plant increased the displacement peak of the reactor plant, reduced the peak of the acceleration response spectrum, and made the peak of the acceleration response spectrum of the top point of the reactor plant move to high frequency. The auxiliary plant sharing the same foundation with the reactor plant should be analyzed holistically, and at least the auxiliary plant connected with the reactor plant should be analyzed holistically.
-
Key words:
- Nuclear power plant /
- SSI /
- Explicit-implicit element method /
- PASSI /
- Adjacent structure
-
引言
陆地与大气紧密相连，地震前大气的异常情况受到地震学者们的重视。前人进行了大量探索性研究工作，以寻求地震前兆（徐国钧等，1993；李贵福等，1996；曹新来等，1997；张铁宝等，2013）。在这些地震研究中，长期连续完整且均一性较好的气象资料是研究地震前气温异常的数据基础。但是，由于各种原因（如环境干扰、硬件故障等），历史气温资料缺测现象时有发生，导致气温观测资料缺测，造成历史资料的不连续（王建国等，2010；2013；姚会琴等，2012）。
中国许多学者开展了对日、月、年时间尺度的气象资料缺测插补研究，并利用一维车贝雪夫多项式展开、线性回归、标准序列法、基于SVD的迭代等方法对中国部分地区的气象日、月、年值资料进行了恢复性实验（张秀芝等, 1996a, 1996b；涂诗玉等，2001；张永领等，2006；王海军等，2008；余予等，2012），但对整点气温值进行缺测插补鲜见文献报道。在国外，Huth等（1995）建立回归模型来插补缺测的日气温数据，Eischeid等（2000）采用空间差值法，插补后建立美国西部40年逐日气温和降水数据集，但这些插补模型只用于1个或数个缺测日数据的插补，不合适用于连续几个月数据缺测的情况。DeGaetano等（1995）引用改进的标准序列法，对美国东北部近400个站的日最高、最低气温缺测值进行了插补。标准序列法和线性回归法解决了插补长期连续缺测数据的问题。整点气温值的缺测插补方法可借鉴日平均、最高、最低气温值的缺测插补方法。由于线性回归法具有更好的统计性能和稳健性，本文对线性回归法进行改进，考虑了距离因素，采用线性回归模型参数求解法，解决了连续数日甚至数月造成的气温缺测问题，为地震前后气温变化特征的研究提供长期连续完整可靠的数据资料，也为今后开展卫星遥感红外亮温与卫星过境时刻气温的对比研究奠定了数据基础。
1. 研究区及数据资料
1.1 研究区介绍
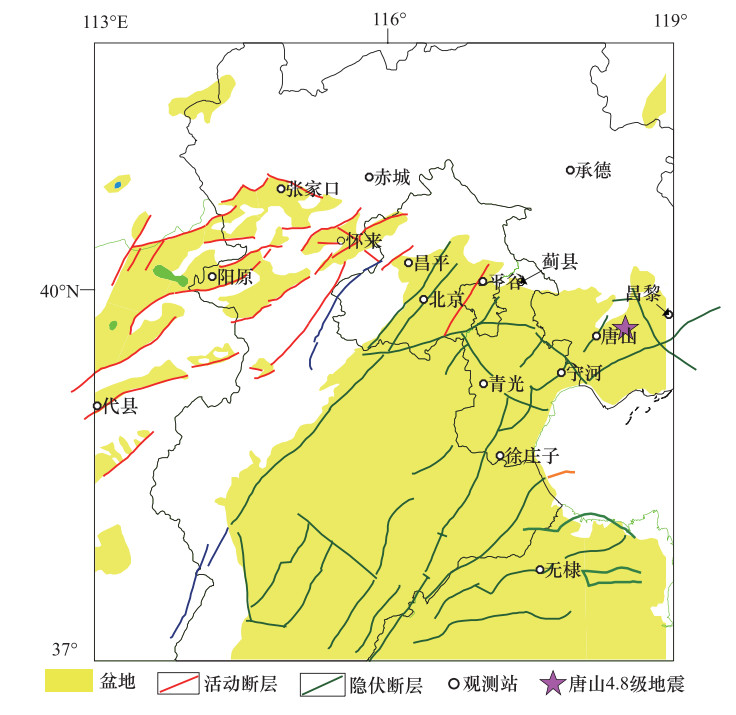
本文选取的研究区范围为37°—42°N、113°—119°E，在研究区内收集到15个地震观测站（气温观测站）的气温整点值数据，观测站分布情况如图 1所示。
1.2 气温资料
本文收集了15个地震观测站气温数据，气温指地面以上1.5m处百叶窗测得的空气温度。太阳的热能被地面吸收后，地面再通过辐射、传导和对流把热传给空气，这是空气中热量的主要来源。气温的观测范围-30℃—70℃，精度0.1℃。由于各观测站安装时间、停测时间不同，导致观测时间的长度不等，多数观测数据起止时间为2007年1月1日，截止时间为2014年12月31日。
1.3 气温数据的缺测情况
由于观测环境、仪器设备故障等原因，部分观测站的观测数据缺测，包括仅缺测1个值、缺测1天的值（即24个观测值）、连续缺测几天甚至1个多月导致长达数千个观测值连续缺测等情况。基于震例研究，本文仅讨论唐山站气温数据的缺测情况（表 1），可以看出该站缺测情况较严重，如2008年8月18日—9月15日连续缺测696个整点值，2009年4月2日—5月13日连续缺测1000个整点值，2012年6月2日—24日连续缺测552个整点值，2013年1月21日—2月5日连续缺测384个整点值。唐山站数据连续缺测时间较长，连续缺测几十个值的频率较高，同时该站还存在错误值，如2010年11月22日18时的观测气温整点值是59.701，类似的错误值在每年均有出现。2010、2011年的观测数据较完整。
表 1 唐山站整点气温数据的缺测统计Table 1. Missing data in integral point temperatures from the Tangshan site数据起止时间 年份 数据缺测情况 2007-01-01 — 2013-12-18 2007 7月14—15日、7月25日 2008 3月8—11日、11月17—24日、11月26日、12月3日、12月7—11日 2009 3月18日、3月26日、4月2日—5月13日、6月27—29日、7月25日、11月10日—11日8时、11月19—21日、11月25—27日、11月30日、12月1—5日、12月13日 2012 6月1—24日、8月30日—9月1日、9月8日、12月24—29日 2013 1月21日—2月5日、6月25日、8月1—3日、7月27—30日、9月5—6日、9月23—24日 1.4 气温数据的日变情况
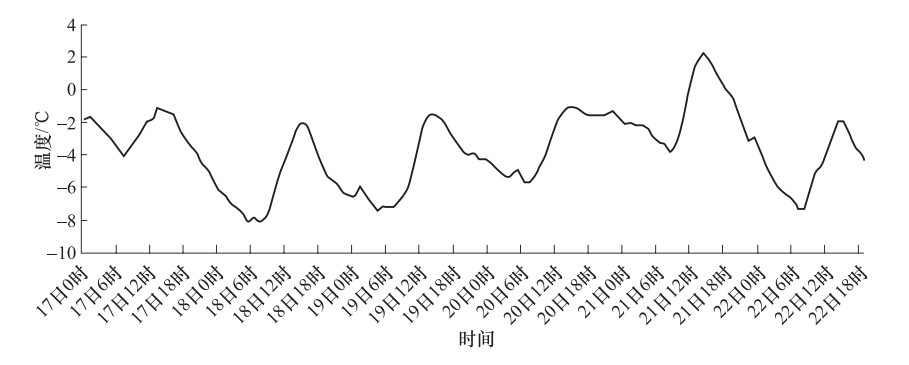
图 2为唐山站2012年12月17日—22日气温整点值变化曲线，可以看出气温日变明显，最高温一般出现在14时左右，最低温出现在8时左右，符合日变规律。
图 2 唐山站气温变化曲线Figure 2. Daily variation of interpolated integral point temperatures for Tangshan site1.5 气温数据的月变情况
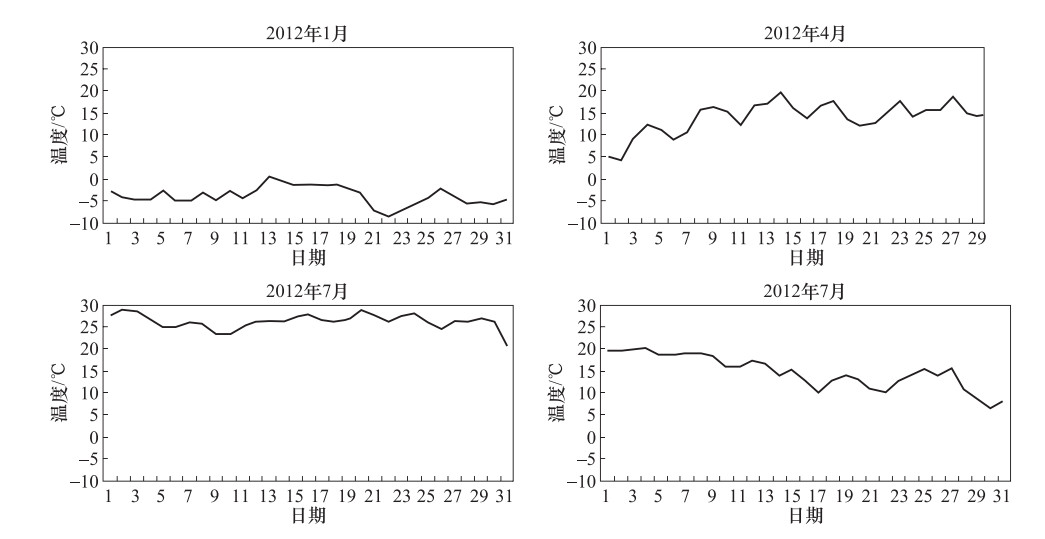
对唐山站2012年1、4、7、10月的日均值进行分析，绘制了相关曲线，如图 3所示。由图可以看出，气温变化整体表现出明显的夏高冬低的年变规律；1月、7月气温变化平稳，4月气温表现为升温过程，10月气温表现为明显的降温过程。
图 3 唐山站气温日均值变化曲线Figure 3. Monthly variation of interpolated daily mean temperatures for Tangshan site1.6 气温数据的年变情况
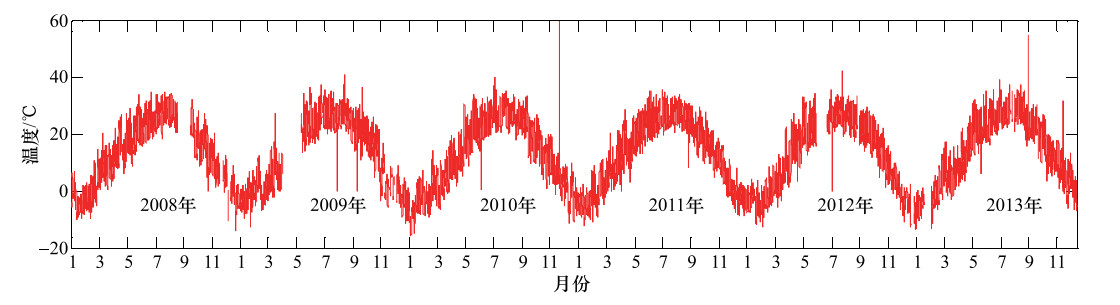
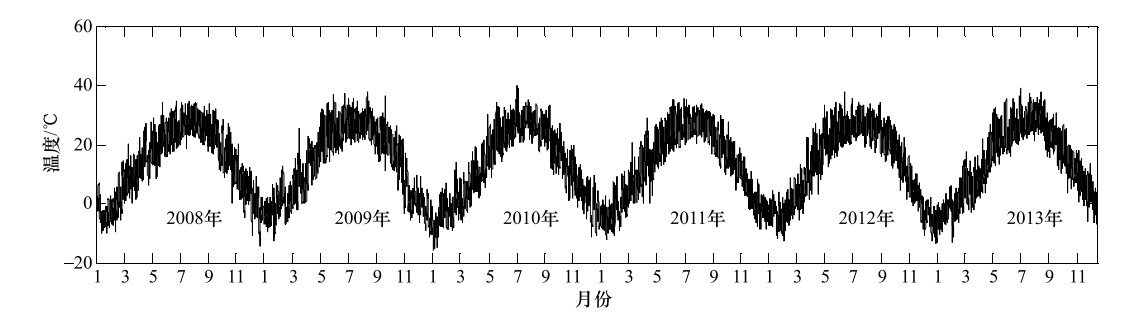
唐山站2008—2013年连续的气温整点值数据变化曲线如图 4所示。由图可以看出，气温表现出明显的夏高冬低的年变特征，夏季最高日平均温度接近31℃，冬季最低日平均温度接近-14℃；气温在冬季和夏季处于稳定的状态，气温从3月开始上升，1月—7月处于升温过程，气温上升快，7月、8月气温达到最高，9月后气温开始迅速降低，进入降温阶段，符合季节变化规律；此外，数据缺测明显。
图 4 唐山站气温整点值年变曲线Figure 4. Annual variation of integral point temperature of Tangshan site2. 研究方法
应用线性回归法解决连续几日甚至数月的整点气温值缺测问题，并采用交叉验证方法对插补结果进行误差分析。
2.1 线性回归模型的建立
本文对线性回归方法进行改进，考虑了参考站和缺测站之间的距离。改进后该方法更科学，可以更好地去除距离因素的影响。
利用邻近站资料对距离进行加权，建立回归模型，插补缺测站资料的方程式为：
$$ {\hat y_i} = \frac{{{a_{1i}}{x_{1i}}}}{{{d_{1i}}}} + \frac{{{a_{2i}}{x_{2i}}}}{{{d_{2i}}}} + \cdots + \frac{{a{}_{mi}{x_{mi}}}}{{{d_{mi}}}} + {a_{m + 1}} $$ (1) 其中，${\hat y_i}$为插补值，${x_{mi}}$为临近站数据，${a_{mi}}$为回归模型的参数，${d_{mi}}$为邻近站与缺测站之间的距离，m为临近站站数。利用最小二乘原理求解回归模型的参数，即使观测值和插补值之间差值的平方和（Q）最小：
$$ \min Q = {\rm{min}}\sum\limits_{i = 1}^n {{{({y_i} - \hat y{}_i)}^2}} $$ (2) 其中${y_i}$为插补站观测值。
因历史同期各要素时空变化规律通常比较相似，选择缺测整点值前后若干整点值的历史同期（不包括缺测值所在的年份）数据，作为拟合回归模型的样本数据，建立线性回归模型，并利用附近站资料，计算缺测记录插补值。
2.2 误差检验方法
本文采用交叉验证的方法对缺测记录的插补结果进行分析，即假设某个站的记录缺测，首先利用插补模型插补整点气温数据，然后对插补值与实际观测资料进行对比和误差分析，并用平均绝对误差（Mean Absolute Error，MAE）代表插补精度（王海军等，2008），其表达式为：
$$ MAE = \frac{1}{N}\sum\limits_{i = 1}^N {\left| {{x_{io}} - {x_{ei}}} \right|} $$ (3) 其中，${x_{oi}}$为第i个实际整点观测值，${x_{ei}}$为第i个插补整点值，N为插补整点值的个数。
3. 插补结果分析
3.1 邻近参考站及时间窗选择
参考站的选择不仅与观测站密度有关，也与插补站及其邻近站所处的地理环境有关（如平原、丘陵、山区等）。同时，时间窗大小也对缺测数据的插补精度有影响。本文采用滑动优选法确定时间窗，时间窗的宽度为气温整点值个数，高度为年数。以选择缺测整点值所在的年份为中心，其前后若干点值历史同期若干年的数据作为样本数据，对于前后无资料的年份，则使用靠近插补年份的资料。
唐山站位于华北平原，周围地势平坦，气温变化相近，故采用最短距离的原则选取临近参考站。根据距离及地形因素，选取了该站周边的北京、昌黎、蓟县、宁河、青光和徐庄子6个观测站，年数为7年。采用15个整点值作为样本资料，建立线性回归模型，插补唐山站的缺测值（包括连续和不连续的单点缺测值）。插补站及其邻近站的基本信息见表 2。
表 2 唐山插补站及其邻近台站信息Table 2. Information of interpolation site and its neighboring sites台站 经度/°E 纬度/°N 海拔/m 与唐山站距离/km 唐山 116.6 40.4 30 0 北京 116 40 60 156 昌黎 119 39.7 18.4 83 蓟县 117.5 40.1 65 82 宁河 117.7 39.4 2.5 45 青光 117 39.2 3 110 徐庄子 117.2 38.7 2 142 3.2 误差检验
采用交叉验证的方法评估上述插补方法，统计了2010年3月的31天实际观测整点值与相应插补值的相关系数，统计结果见表 3。从表中可以看出，3月8日、14日、15日、19日和20日的相关系数相对较低。王海军等（2008）经过对比研究，在平原地区选取了4个参考站，选取年数为8年、天数为15天，插补误差最小。唐山站也位于平原地区，考虑与唐山站的距离及地形因素，选取昌黎、宁河、青光、徐庄子4个参考站，并选取年数为7年、15个整点值的优化模型。通过对比发现，4个参考站的相关系数偏高（表 3）。
表 3 观测值与插补值相关系数Table 3. Correlation coefficients between interpolated and observed data日期 优化模型相关系数 相关系数 2010-03-01 0.922018 0.916277 2010-03-02 0.962184 0.967497 2010-03-03 0.934722 0.94439 2010-03-04 0.986854 0.973136 2010-03-05 0.97029 0.931489 2010-03-06 0.925587 0.958141 2010-03-07 0.962133 0.96263 2010-03-08 0.72234 0.652517 2010-03-09 0.896098 0.915444 2010-03-10 0.980709 0.977074 2010-03-11 0.928618 0.952106 2010-03-12 0.892358 0.900113 2010-03-13 0.950986 0.959182 2010-03-14 0.799879 0.753849 2010-03-15 0.881494 0.792166 2010-03-16 0.974627 0.968444 2010-03-17 0.972681 0.970844 2010-03-18 0.976031 0.973557 2010-03-19 0.596366 0.618699 2010-03-20 0.846827 0.745552 2010-03-21 0.951358 0.951009 2010-03-22 0.932557 0.951905 2010-03-23 0.901255 0.919988 2010-03-24 0.897624 0.920017 2010-03-25 0.974534 0.981077 2010-03-26 0.958726 0.979361 2010-03-27 0.976428 0.948801 2010-03-28 0.987332 0.993851 2010-03-29 0.927046 0.962993 2010-03-30 0.841163 0.902429 2010-03-31 0.968156 0.977347 利用式（3）对优化后的模型得到的插补结果计算平均绝对误差，并统计其误差的比例分布（表 4）。从表 4可以看出，插补误差在±0.5℃范围内的比例为60.5%，在±0.8℃范围内的比例为80.6%，其误差绝对值大于1.0℃的为9.4%，平均绝对误差为0.82℃。
表 4 唐山站整点气温缺测插补误差比例Table 4. Proportion of error for interpolated integral point temperature误差范围 比例/% （-∞，-1） 7.8 [-1，-0.8） 5.4 [-0.8，-0.5） 9.5 [-0.5，0） 31.2 [-0.5，0.5] 60.5 [0，0.5） 29.3 [0.5，0.8） 11.6 [0.8，1） 3.6 [1，∞） 1.6 [-0.8，0.8] 81.6 3.3 插补结果
针对唐山站2008年1月1日—2013年12月18日的气温整点值缺测数据及错误数据，利用唐山邻近站的同期数据和线性回归模型，对缺测数据插补完整，并修正错误数据，绘制气温整点值的年变曲线，如图 5所示。从图中可以看出，在长时间序列缺测的部位，插补值与前后正确的数据衔接吻合，没有出现突升或突降变化。插补后完整连续的数据符合夏高冬低的年变规律，气温6年的变化形态一致。
图 5 插补后的唐山台气温整点值年变曲线Figure 5. Annual variation of interpolated integral point temperature for Tangshan site4. 震例应用
据中国地震台网中心测定，2012年5月28日10时22分在河北省唐山市辖区、滦县交界处发生4.8级地震，震源深度8km。
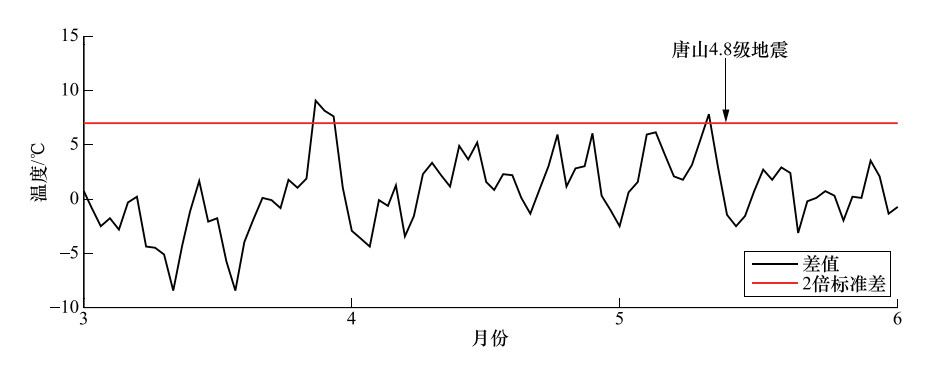
利用插补完整的连续数据，分析2012年3—5月震前气温数据的变化情况。首先，选取无震年份（2008—2011年）同期（3—5月）整点值气温，并计算历年同期气温日均值，以此作为3—5月正常的背景值；其次，将2012年3—5月日均值与历年同期背景值做差值，得到2012年3—5月份数据与历年同期均值的偏移程度；最后，以无震年份同期气温波动范围作为基准，即2008—2011年3—5月所有气温值的标准差作为判断标准。根据以往经验将差值大于2倍标准差视为气温前兆异常。
从2012年3—5月当年日均值与历年同期（2008—2011年）多年日均值的差值及标准差（图 6）中可以看出，从3月27日开始，唐山站数据出现大幅度增温异常现象，4月增温天数也较多，5月1日—11日的气温日均值仍然高于历年同期，特别是5月10日（即震前2天）增幅达到约8℃，且大于2倍标准差；震后差值开始变小，并逐步恢复至平静。
图 6 观测值与同期均值的差值及标准差Figure 6. Standard deviation and difference between mean and observed value5. 结论
长期连续完整的历史气象资料是震前气温异常判别研究的重要数据基础，但由于观测环境、仪器故障等原因，造成气温观测数据缺测或错误数据，且部分数据缺测的时间较长。为此，本文利用线性回归模型，插补缺测和错误的气温整点值数据，较好地解决了长期连续缺测的情况。
通过对唐山观测站2008年1月1日—2013年12月18日的气温整点值缺测数据及错误数据进行插补，使得数据完整连续，并应用插补完整的气温整点值数据，分析研究了2012年5月28日唐山4.8级地震的气温前兆异常现象，主要得出以下结论：
（1）唐山观测站的插补值与其前后的观测数据衔接吻合，插补后完整连续的数据符合夏高冬低的年变规律。
（2）插补误差在±0.5℃范围内的比例为60.2%，在±0.8℃范围内的比例为80.3%，其误差绝对值大于1.0℃的为9.6%，平均绝对误差为0.84℃。插补值与观测值的相关系数大部分在0.9以上，可见插补结果真实可靠。
（3）从3月27日起，唐山观测站数据出现增温异常，震前2天增温幅度约8℃。
-
图 4 ABAQUS软件中黏弹性人工边界及监测点示意
Figure 4. Viscoelastic artificial boundary and monitoring points in ABAQUS
图 10 地震动加速度时程与反应谱曲线
Figure 10. Ground motion acceleration time history and response spectrum
图 12 反应堆厂房监测点位移时程曲线
Figure 12. Displacement time history of each monitoring point on the reactor plant
图 13 反应堆厂房监测点加速度反应谱
Figure 13. Acceleration response spectrum of monitoring point on the reactor plant
图 14 反应堆厂房监测点加速度反应谱
Figure 14. Acceleration response spectrum of monitoring point on the reactor plant
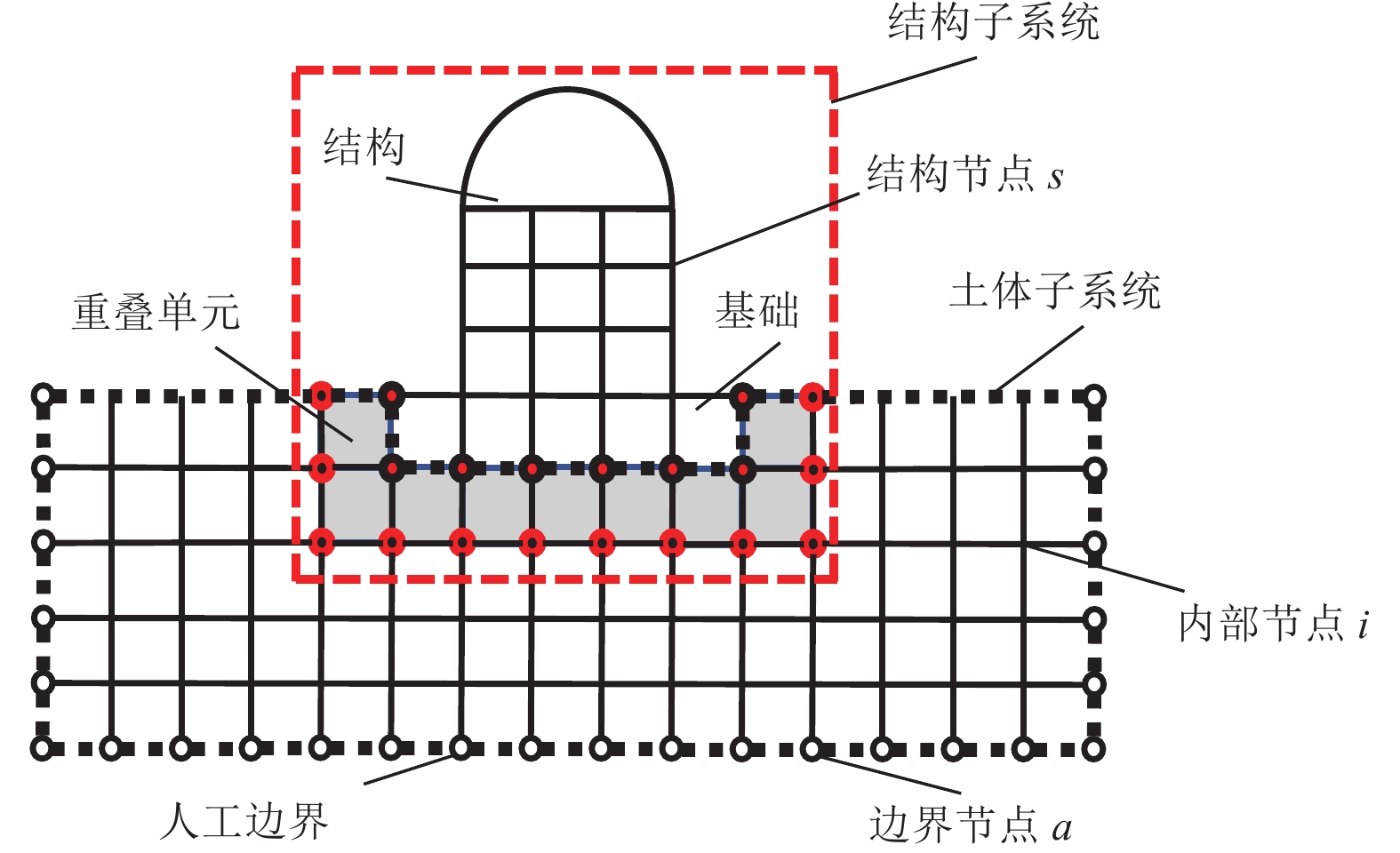
表 1 土体参数
Table 1. Soil parameters
材料 厚度/m 弹性模量/GPa 泊松比 密度/kg·m−3 剪切波速/m·s−1 压缩波速/m·s−1 软土 50 0.108 0.35 1 000 200 416 
下载: 导出CSV
表 2 显-隐式单元层计算方法和ABAQUS软件计算效率
Table 2. PASSI and ABAQUS calculation efficiency
算例 单元数/个 节点数/个 自由度数/个 显-隐式单元层计算方法
计算时间/minABAQUS软件
计算时间/min场地 80 000 85 731 257 193 4 106 土-结构相互作用 80 010 85 771 257 313 33 106 注：自由度数=节点数*3（每个节点自由度个数）
下载: 导出CSV
表 3 核电站结构材料参数
Table 3. Material parameters of nuclear power plant
编号 材料 结构 弹性模量/GPa 泊松比 密度/kg·m−3 1 C30 厂房/基础 32.5 0.2 2 400 2 C40 内/外壳 36.0 0.2 2 450
下载: 导出CSV
表 4 核电站厂址参数
Table 4. Soil parameters of nuclear power plant site
材料 厚度/m 弹性模量/GPa 泊松比 密度/kg·m−3 剪切波速/m·s−1 压缩波速/m·s−1 岩石 60 46.9 0.26 2 650 2 673 4 639
下载: 导出CSV
表 5 模态分析
Table 5. Modality analysis
振型 Case1 Case2 Case3 Case4 频率/Hz 振型参与系数 频率/Hz 振型参与系数 频率/Hz 振型参与系数 频率/Hz 振型参与系数 1 3.006 69 0.186 26 3.006 69 0.186 19 4.016 96 1.000 00 3.726 48 0.426 64 2 3.007 49 1.000 00 3.007 49 1.000 00 4.068 74 0.277 89 3.861 54 0.413 38 3 4.078 57 0.540 83 3.725 20 0.677 04 4.114 50 0.094 62 3.885 43 0.002 48 4 4.096 08 0.381 57 3.858 00 0.683 88 4.210 24 0.482 59 4.017 18 1.000 00 5 5.488 44 0.002 04 3.885 28 0.009 75 4.739 49 0.029 30 4.068 75 0.275 37 6 5.513 01 0.001 43 4.078 57 0.540 69 5.472 46 0.169 67 4.114 53 0.097 12 7 5.540 46 0.003 31 4.096 08 0.381 50 5.566 56 0.002 17 4.210 38 0.480 55 8 5.572 03 0.004 84 4.559 21 0.068 57 5.610 47 0.012 11 4.560 35 0.045 69 9 5.629 19 0.003 16 5.439 43 0.064 35 5.762 07 0.080 10 4.739 62 0.029 71 10 5.658 22 0.010 71 5.488 44 0.002 05 6.163 02 0.037 35 5.441 09 0.042 47 11 6.426 34 0.004 54 5.513 01 0.001 43 6.470 85 0.006 88 5.472 66 0.167 66 12 6.471 34 0.015 75 5.540 46 0.003 31 6.496 26 0.389 72 5.566 56 0.002 12 13 6.496 20 0.598 36 5.572 03 0.004 82 6.615 10 0.003 04 5.610 47 0.011 96 14 6.614 21 0.001 58 5.629 19 0.003 17 6.875 02 0.134 96 5.702 48 0.044 00 15 6.875 13 0.207 35 5.658 22 0.010 72 7.121 85 0.000 71 5.762 18 0.079 50 16 7.117 11 0.000 87 5.698 29 0.070 07 7.183 56 0.005 66 6.163 16 0.037 35 17 7.171 63 0.005 92 6.426 34 0.004 55 7.186 37 0.071 54 6.470 85 0.006 79 18 7.186 59 0.070 52 6.471 34 0.015 75 7.228 46 0.359 01 6.496 26 0.386 15 19 7.218 46 0.014 67 6.496 20 0.598 31 7.349 15 0.546 56 6.615 10 0.003 03 20 7.235 54 0.000 05 6.614 21 0.001 59 7.778 06 0.042 94 6.875 02 0.133 73 21 7.778 05 0.071 15 6.875 13 0.207 34 7.984 62 0.026 00 7.121 85 0.000 71 22 7.984 60 0.042 40 7.117 11 0.000 87 8.112 55 0.020 30 7.183 56 0.005 58 23 8.112 54 0.033 21 7.171 63 0.005 93 8.241 69 0.004 93 7.186 37 0.070 85 24 8.241 67 0.008 19 7.186 59 0.070 51 8.355 48 0.012 78 7.228 55 0.356 06 25 8.355 47 0.020 32 7.218 46 0.014 67 8.603 98 0.003 84 7.349 13 0.541 36 26 8.457 00 0.005 00 7.235 54 0.000 04 8.879 18 0.000 60 7.778 06 0.042 54 27 8.603 97 0.006 27 7.778 05 0.071 15 8.980 48 0.011 40 7.984 62 0.025 76 28 8.977 70 0.014 41 7.984 60 0.042 39 9.092 81 0.031 82 8.112 55 0.020 11 29 9.092 80 0.051 76 8.112 54 0.033 21 9.161 85 0.066 65 8.241 69 0.004 88 30 9.229 79 0.118 33 8.241 67 0.008 19 9.229 87 0.071 67 8.355 48 0.012 67 31 9.414 48 0.015 02 8.355 47 0.020 32 9.414 48 0.009 21 8.603 98 0.003 80 32 9.518 82 0.077 79 8.457 00 0.005 04 9.450 98 0.026 21 8.879 18 0.000 58 33 9.698 92 0.086 25 8.603 97 0.006 27 9.518 87 0.045 52 8.980 48 0.011 29 34 9.776 22 0.456 36 8.977 70 0.014 42 9.787 15 0.014 03 9.092 81 0.031 53 35 9.841 21 0.024 00 9.092 80 0.051 76 9.841 17 0.020 96 9.161 81 0.065 98
下载: 导出CSV
-
曹金凤, 王旭春, 孔亮, 2011. Python语言在Abaqus中的应用. 北京: 机械工业出版社. 韩冰, 陈少林, 梁建文, 2019. 结构-土-结构动力相互作用对结构系统频率的影响. 地震工程学报, 41（6）: 1574—1580Han B. , Chen S. L. , Liang J. W. , 2019. Effects of structure-soil-structure dynamic interaction on the frequency of structural systems. China Earthquake Engineering Journal, 41(6): 1574—1580. (in Chinese) 何涛, 姜南, 2020. 土-相邻结构相互作用子结构振动台试验研究. 振动与冲击, 39（4）: 207—214He T. , Jiang N. , 2020. A substructure shaking table test of soil-adjacent structure interaction. Journal of Vibration and Shock, 39(4): 207—214. (in Chinese) 李培振, 严克非, 徐鹏, 2014. 地震下考虑群体效应的高层建筑土-结构相互作用研究. 土木工程学报, 47（S1）: 1—5Li P. Z. , Yan K. F. , Xu P. , 2014. Study on dynamic interaction between soil and group of high-rise buildings under seismic excitation. China Civil Engineering Journal, 47(S1): 1—5. (in Chinese) 廖振鹏, 2002. 工程波动理论导论. 2版. 北京: 科学出版社.Liao Z. P. , 2002. Introduction to wave motion theories in engineering. 2 nd ed. Beijing: Science Press. (in Chinese) 刘晶波, 王振宇, 杜修力等, 2005. 波动问题中的三维时域粘弹性人工边界. 工程力学, 22（6）: 46—51 doi: 10.3969/j.issn.1000-4750.2005.06.008Liu J. B. , Wang Z. Y. , Du X. L. , et al. , 2005. Three-dimensional visco-elastic artificial boundaries in time domain for wave motion problems. Engineering Mechanics, 22(6): 46—51. (in Chinese) doi: 10.3969/j.issn.1000-4750.2005.06.008 刘晶波, 杜义欣, 闫秋实, 2007. 粘弹性人工边界及地震动输入在通用有限元软件中的实现. 见: 第三届全国防震减灾工程学术研讨会论文集. 南京: 中国土木工程学会, 43—48. 刘晶波, 宝鑫, 李述涛等, 2022. 采用黏弹性人工边界时显式算法稳定性条件. 爆炸与冲击, 42（3）: 034201Liu J. B. , Bao X. , Li S. T. , et al. , 2022. Stability conditions of explicit algorithms when using viscoelastic artificial boundaries. Explosion and Shock Waves, 42(3): 034201. (in Chinese) 柳玉印, 尹训强, 2018. 考虑结构—土—结构动力相互作用的重力坝地震响应分析. 水利水电技术, 49（10）: 52—58Liu Y. Y. , Yin X. Q. , 2018. Structure-soil-structure dynamic interaction-considered seismic response analysis of gravity dam. Water Resources and Hydropower Engineering, 49(10): 52—58. (in Chinese) 苏景鹤, 江丙云, 2016. ABAQUS Python二次开发攻略. 北京: 人民邮电出版社. 田彼得, 俞载道, 1987. 结构-土-结构相互作用体系的动力分析. 同济大学学报, 15（2）: 157—168Tian B. D. , Yu Z. D. , 1987. Dynamic analysis of structure-soil-structure interaction. Journal of Tongji University, 15(2): 157—168. (in Chinese) 王飞, 宋志强, 刘昱杰等, 2018. 基于ABAQUS无限元的静-动力统一人工边界研究. 水资源与水工程学报, 29（6）: 170—177Wang F. , Song Z. Q. , Liu Y. J. , et al. , 2018. Research on static-dynamic unified artificial boundary based on ABAQUS infinite element. Journal of Water Resources and Water Engineering, 29(6): 170—177. (in Chinese) 王国波, 袁明智, 苗雨, 2018. 结构-土-结构相互作用体系地震响应研究综述. 岩土工程学报, 40（5）: 837—847Wang G. B. , Yuan M. Z. , Miao Y. , 2018. Review of seismic response of structure-soil-structure interaction system. Chinese Journal of Geotechnical Engineering, 40(5): 837—847. (in Chinese) 应稼年, 王荣昌, 俞载道, 1995. 地基土-桩基础-核电站辅助厂房结构相互作用体系的地震响应分析. 地震工程与工程振动, 15（1）: 44—52 doi: 10.13197/j.eeev.1995.01.006Ying J. N. , Wang R. C. , Yu Z. D. , 1995. Earthquake response analysis of soil-pile-nuclear power station auxiliary workshop structure interaction system. Earthquake Engineering and Engineering Vibration, 15(1): 44—52. (in Chinese) doi: 10.13197/j.eeev.1995.01.006 中华人民共和国住房和城乡建设部, 国家市场监督管理总局, 2019. GB 50267—2019 核电厂抗震设计标准. 北京: 中国计划出版社.Ministry of Housing and Urban Rural Development of the People's Republic of China, State Administration of Market Supervision and Administration, 2019. GB 50267—2019 Standard for seismic design of nuclear power plants. Beijing: China Planning Press. (in Chinese) Aldaikh H. , Alexander N. A. , Ibraim E. , et al. , 2015. Two dimensional numerical and experimental models for the study of structure–soil–structure interaction involving three buildings. Computers & Structures, 150: 79—91. Aldaikh H. , Alexander N. A. , Ibraim E. , et al. , 2016. Shake table testing of the dynamic interaction between two and three adjacent buildings (SSSI). Soil Dynamics and Earthquake Engineering, 89: 219—232. doi: 10.1016/j.soildyn.2016.08.012 ASCE, Seismic analysis of safety-related nuclear structures, ASCE/SEI 4-16. 2016, American Society of Civil Engineers: New York Belytschko T. , Mullen R. , 1978. Stability of explicit-implicit mesh partitions in time integration. International Journal for Numerical Methods in Engineering, 12(10): 1575—1586. doi: 10.1002/nme.1620121008 Belytschko T., Yen H. J., Mullen R., 1979. Mixed methods for time integration. Computer Methods in Applied Mechanics and Engineering, 17—18: 259—275. Belytschko T. , Smolinski P. , Liu W. K. , 1985. Stability of multi-time step partitioned integrators for first-order finite element systems. Computer Methods in Applied Mechanics and Engineering, 49(3): 281—297. doi: 10.1016/0045-7825(85)90126-4 Chen S. L. , Lv H. , Zhou G. L. , 2022. Partitioned analysis of soil-structure interaction for Nuclear Island Buildings. Earthquake Engineering & Structural Dynamics, 51(10): 2220—2247. Ghandil M. , Behnamfar F. , Vafaeian M. , 2016. Dynamic responses of structure–soil–structure systems with an extension of the equivalent linear soil modeling. Soil Dynamics and Earthquake Engineering, 80: 149—162. doi: 10.1016/j.soildyn.2015.10.014 Kitada Y., Hirotani T., Iguchi M., 1999. Models test on dynamic structure–structure interaction of nuclear power plant buildings. Nuclear Engineering and Design, 192(2—3): 205—216. Lee T. H. , Wesley D. A. , 1973 a. Soil-structure interaction of nuclear reactor structures considering through-soil coupling between adjacent structures. Nuclear Engineering and Design, 24(3): 374—387. doi: 10.1016/0029-5493(73)90007-1 Lee T. H., Wesley D. A., 1973b. Influence of through-soil coupling between adjacent structures on seismic response of nuclear reactors. In: Proceedings of the Second International Conference on Structural Mechanics in Reactor Technology. Berlin: IASMiRT, 2—9. Lehmann L. , Antes H. , 2001. Dynamic structure-soil-structure interaction applying the Symmetric Galerkin Boundary Element Method (SGBEM). Mechanics Research Communications, 28(3): 297—304. doi: 10.1016/S0093-6413(01)00177-X Lou M. L. , Wang H. F. , Chen X. , et al. , 2011. Structure-soil-structure interaction: literature review. Soil Dynamics and Earthquake Engineering, 31(12): 1724—1731. doi: 10.1016/j.soildyn.2011.07.008 Luco J. E. , Contesse L. , 1973. Dynamic structure-soil-structure interaction. Bulletin of the Seismological Society of America, 63(4): 1289—1303. doi: 10.1785/BSSA0630041289 Matthees W. , Magiera G. , 1982. A sensitivity study of seismic structure-soil-structure interaction problems for nuclear power plants. Nuclear Engineering and Design, 73(3): 343—363. doi: 10.1016/0029-5493(82)90011-5 Murakami H. , Luco J. E. , 1977. Seismic response of a periodic array of structures. Journal of the Engineering Mechanics Division, 103(5): 965—977. doi: 10.1061/JMCEA3.0002286 Wang J. S. , Guo T. , Du Z. Y. , 2022. Experimental and numerical study on the influence of dynamic structure-soil-structure interaction on the responses of two adjacent idealized structural systems. Journal of Building Engineering, 52: 104454. doi: 10.1016/j.jobe.2022.104454 Warburton G. B. , Richardson J. D. , Webster J. J. , 1971. Forced vibrations of two masses on an elastic half space. Journal of Applied Mechanics, 38(1): 148—156. doi: 10.1115/1.3408735 Whitman R. V. , 1969. The current status of soil dynamics. Applied Mechanics Reviews, 22(1): 1—8. Wong H. L. , Trifunac M. D. , 1975. Two-dimensional, antiplane, building-soil-building interaction for two or more buildings and for incident planet SH waves. Bulletin of the Seismological Society of America, 65(6): 1863—1885. -
下载:
点击查看大图
计量
- 文章访问数: 202
- HTML全文浏览量: 40
- PDF下载量: 17
- 被引次数: 0
