Primary Exploration of the Basin Effect on Significant Duration
-
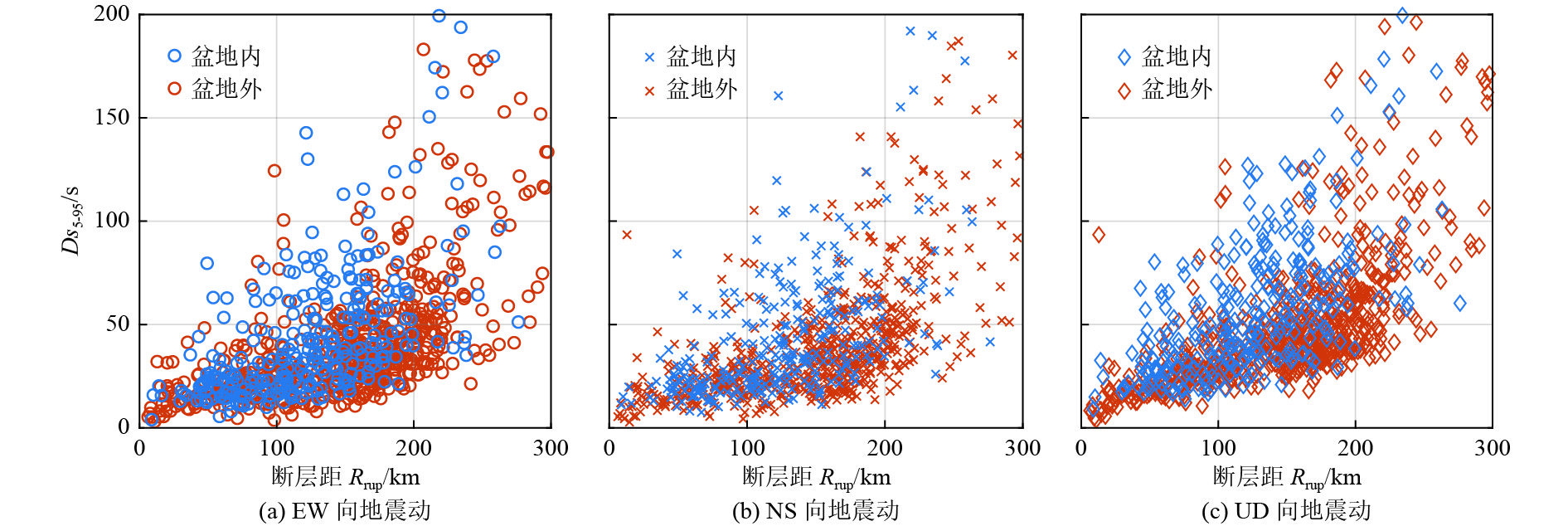
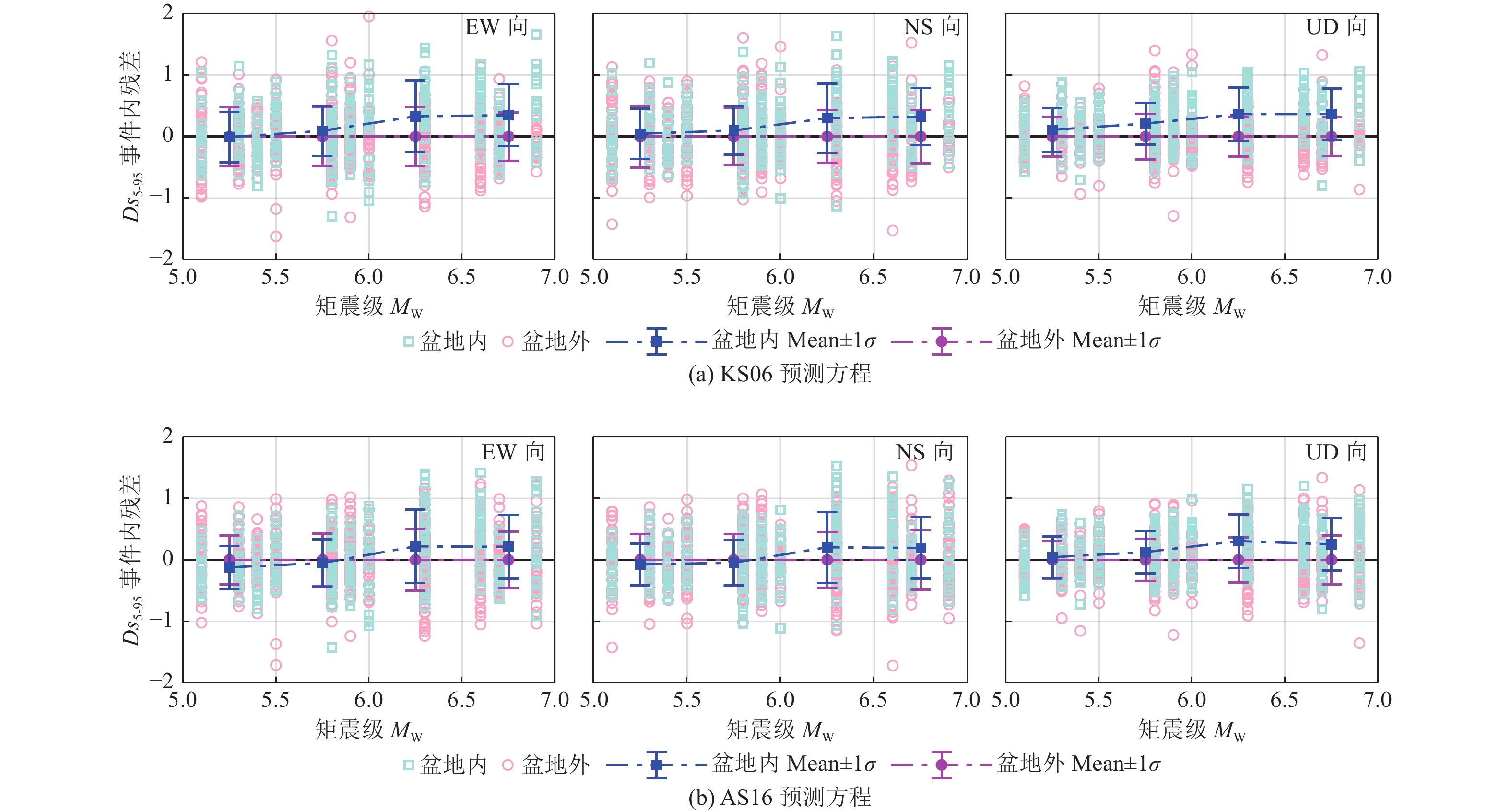
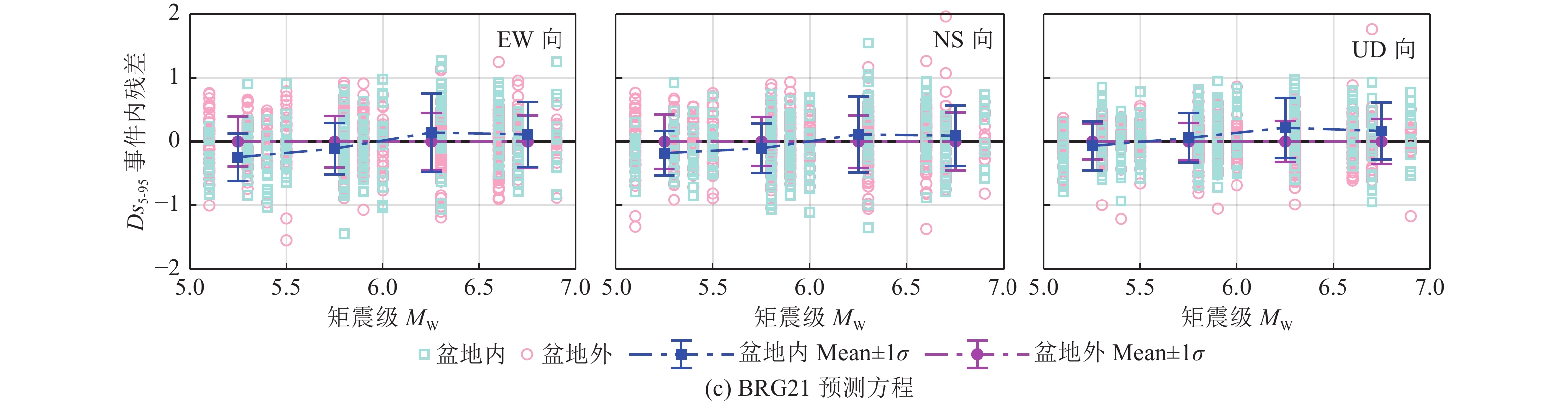
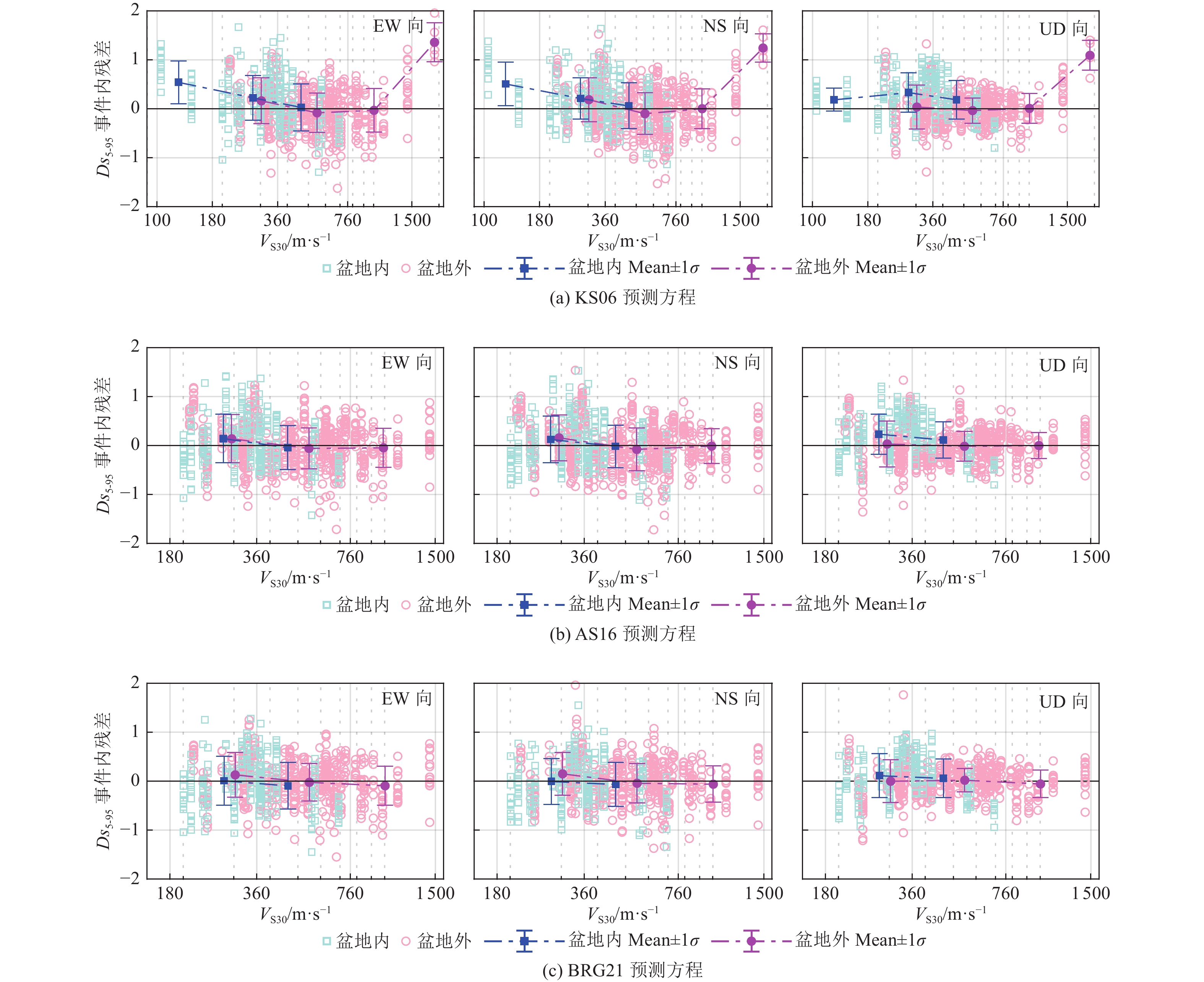
摘要: 利用关东盆地及其周边KiK-net台网井上台站记录的2004—2017年15次中强地震（矩震级为5.1～6.9级）构建三分量记录显著持时Ds5-95数据库。针对该数据库，基于残差分析方法和3种水平向地震动持时参数预测方程，计算并给出事件间残差和事件内残差及其随不同类别参数的变化。在此基础上，初步探讨了水平向地震动持时预测方程应用于预测竖向地震动持时的可行性及盆地对三分量地震动持时的影响。研究结果表明，对于震源距和场地VS30相当的情况，盆地内台站持时普遍大于盆地外台站持时，盆地内、外台站竖向地震动持时均大于水平向地震动持时；3种预测方程均可实现对盆地外台站水平向地震动Ds5-95的合理估计，但在一定程度上低估了盆地内台站的水平向地震动Ds5-95；3种预测方程均无法直接应用于竖向地震动持时预测。Abstract: Based on the three-component records of MW 5.1～MW6.9 earthquake events recorded by KiK-net of Japan Strong Motion Observatory Network from 2004 to 2017, this paper constructs the Ds5-95 duration database of 15 strong crustal earthquakes in the Kanto basin. Further, the inter-event residuals and intra-event residuals of the duration database are calculated based on the residual analysis method and three kinds of duration prediction equations, and their changes with different parameters are also discussed. The prediction level of vertical earthquake ground motion duration based on duration prediction equation for horizontal ground motion and the influence of basin on three component earthquake records duration are analyzed. The results show that the duration of earthquake ground motion for inside-basin stations is greater than that of outside-basin stations, and the duration of vertical earthquake ground motion is greater than that of horizontal ground motion, thus, the influence of the basin effect on the duration can not be ignored; Three kinds of duration prediction equations can realize the reasonable estimation of horizontal earthquake ground motion Ds5-95 duration for outside-basin stations, but to a certain extent, horizontal earthquake ground motion Ds5-95 duration is underestimated for inside-basin stations; Three kinds of duration prediction equations can not be directly applied to the estimation of vertical earthquake ground motion duration.
-
引言
1989年日本学者中村（Nakamura）首次提出谱比法（水平与竖向观测信号谱之比，即H/V谱比法），该方法计算过程简单且具有较强的灵活度，现已在地震工程研究的各领域中得到广泛应用。H/V谱比法最早应用于地脉动记录及微震领域，可较好地反映场地特征，后由Yamazaki等（1997）推广到强震动加速度记录中，验证了强震动记录的S波谱比不受震级、震中距和震源深度的影响，为利用强震动记录的谱比分析台站场地特征、场地分类提供理论基础。随着全球强震动观测网络的迅速发展，强震动记录数量迅猛增加，H/V谱比法得到广泛应用，如Lermo等（1993）基于H/V谱比法估算了场地经验传递函数；Zare等（1999）和Lee等（2001）分别完成了基于强震动记录的伊朗与中国台湾自由场地强震动台站场地类别划分；Satoh等（2001）分析了地脉动、P波、S波及尾波间场地特征的差异。Yadab等（2017）利用谱比法识别K-NET台站海底地震台站场地的非线性反应；Dimitriu等（1998）和Bonilla等（2002）通过对比H/V谱比法与传统场地特征研究方法，证明不同方法得到的场地卓越周期结果基本一致。我国学者也应用H/V谱比法对场地特性进行了广泛研究，如温瑞智等（2015a）基于谱比法统计了我国三类场地标准谱比曲线，并对113个强震动台站进行场地分类；王伟君等（2009）应用单台谱比法获取了北京市详细的场地响应和浅层结构速度；荣棉水等（2016）通过对比场地水平、垂直谱比曲线与传递函数曲线间的差异，提出在可忽略场地竖向放大的频段内，谱比曲线可用于场地效应研究；冀昆（2014）、任叶飞等（2014）利用H/V谱比法分别对芦山地区及汶川地区场地特征开展研究。近年来还有研究人员将H/V谱比法拓展运用到建筑结构对环境噪声的响应研究上，如罗桂纯（2015）采用该方法识别结构的自振频率。
上述研究说明H/V谱比法具有较高的实用性。在实际使用过程中，对强震动记录进行必要的数据处理不可或缺，通常需依次进行S波窗口的截取、Taper处理、傅里叶振幅谱计算、谱进行平滑、合成H/V谱比等。如果不对其中的几个关键环节谨慎处理，H/V谱比计算结果的准确性和可靠性将大幅降低。如S波截取不当会影响场地卓越周期的判断结果；未进行Taper处理的截取数据进行傅里叶变换时将产生边瓣效应；傅里叶谱未平滑或平滑过渡则导致谱比峰值过多或削弱重要峰值点等，但目前还没有相关文献给出上述关键环节的处理方法或注意细节。因此，本文试图给出解决办法，首先筛选汶川余震记录并截取S波，对其进行Taper预处理，对比分析不同带宽Parzen窗对S波傅里叶振幅谱比曲线平滑效果的影响，给出满足傅里叶振幅谱比曲线平滑要求的较为合理的Parzen窗带宽。研究结果可为其他学者在计算强震动记录H/V谱比时提供技术帮助和理论支持。
1. 数据选取
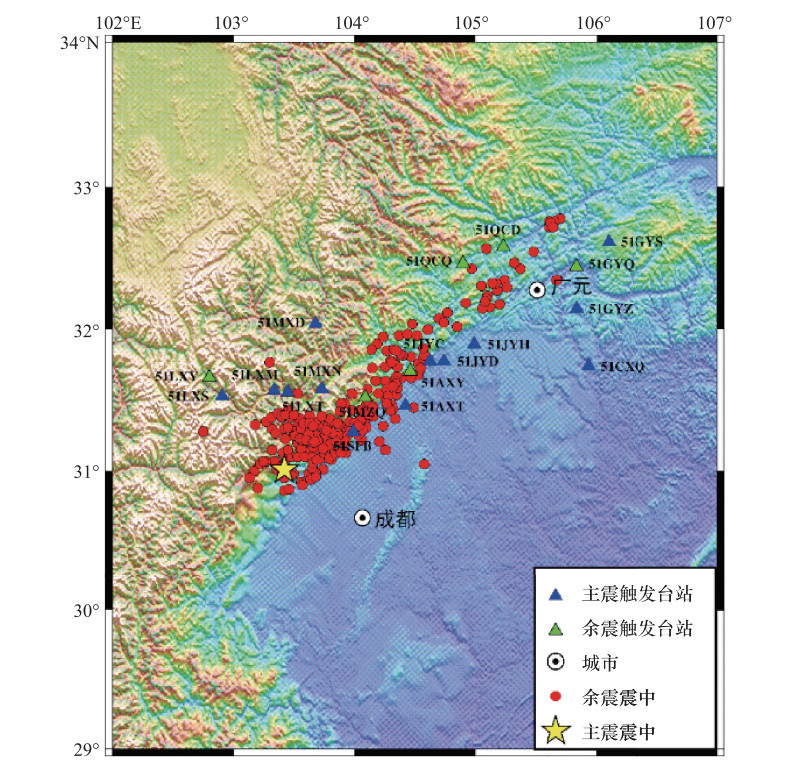
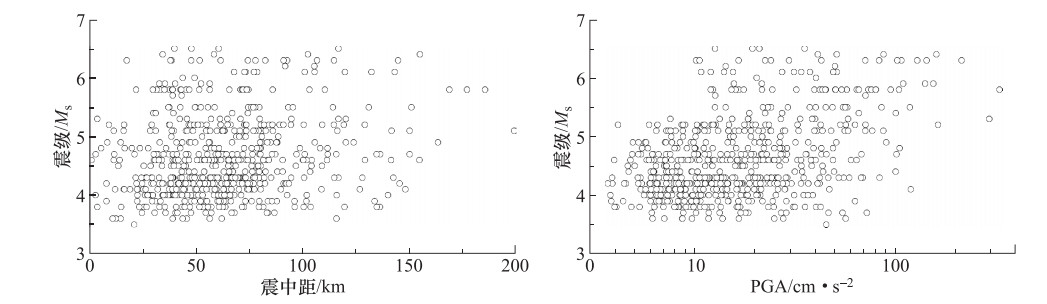
本文对汶川地震余震中获取的强震动记录开展相关研究，选取原则为：①选取3个方向的峰值地面加速度（PGA）均不低于3cm/s2的记录，以减小背景噪声对强震动记录的干扰；②对筛选后的记录，按照下文S波拾取方法逐一处理后，选择具有完整S波震相的记录；③为降低谱比计算结果的离散性，保证每个台站得到的谱比平均值曲线具有统计意义上的代表性，选择余震记录不少于5条的强震动台站进行分析。根据上述原则，本文最终选取19个台站在249次地震中获取的642组强震动记录，台站位置及震中分布如图 1所示，震级-震中距和震级-PGA分布情况如图 2所示。由图 2可知，最终选取的记录震级主要分布在MS3.6—5.5，震中距大部分集中于20—150km，PGA以3—100cm/s2为主。
图 2 震级-震中距和震级-PGA分布Figure 2. Distribution of magnitude and epicentral distance and peak ground acceleration2. S波的震相拾取
H/V谱比法的基本假设为地震动在水平向放大的频段内，竖向没有显著放大。但有些情况下记录到的面波在竖向存在放大效应（Satoh等，2001），如果利用地表强震动记录的全时程计算H/V谱比，可能引起不合理的场地效应分析结果。因此在利用H/V谱比进行场地效应分析时，S波提取是尤为关键的环节。
通常拾取S波到时采用自动拾取技术。目前已有多种拾取S波到时的有效方法，常用的有长短时平均（STA/LTA）法（Baer等，1987）、AIC法（Akaike，1992）、基于高阶统计量偏斜度和峰度的PAI-S/K法（Saragiotis等，2002）及综合多种自动拾取方法特点的多步自动拾取法等（何先龙等，2016），这些自动拾取方法操作相对复杂，过程不易掌握。实际上，对于H/V谱比分析场地效应而言，S波拾取的精度需求并不高，简单实用更为重要。
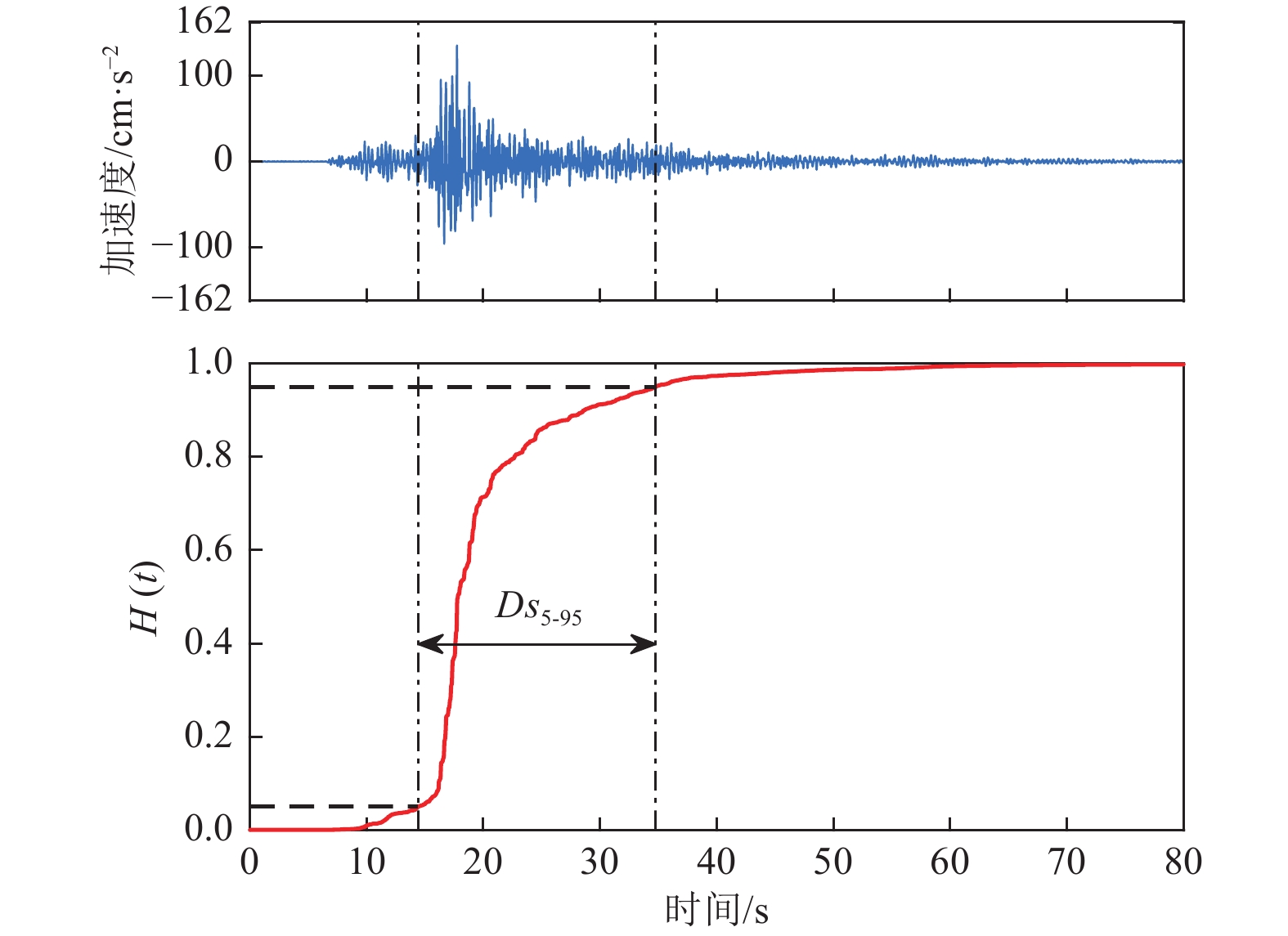
本文以能反映整个加速度时程内能量分布的Husid函数确定S波震相初至时间，将地震能量开始急剧增加时点tS1定义为S波的到达时间，Husid函数表示为（Husid，1967）：
$${H_n}(t) = \frac{{\int_0^t {{{[a(t)]}^2}} {\rm{d}}t}}{{\int_0^\infty {{{[a(t)]}^2}} {\rm{d}}t}}$$ (1) S波的结束时间根据累计均方根（CRMS）函数（Mccann等，1979）确定，以累计均方根函数开始下降的点tS2定义为S波的结束时间，CRMS函数如下：
$$CRMS = \sqrt {\frac{1}{T}\int_0^T {{{\left\| {a(t)} \right\|}^2}{\rm{d}}t} } $$ (2) 式中a(t)表示加速度时程；T表示计算持时。S波结束时间与S波到达时间的差值为S波窗口（Td-S，见图 3阴影部分），计算如下：
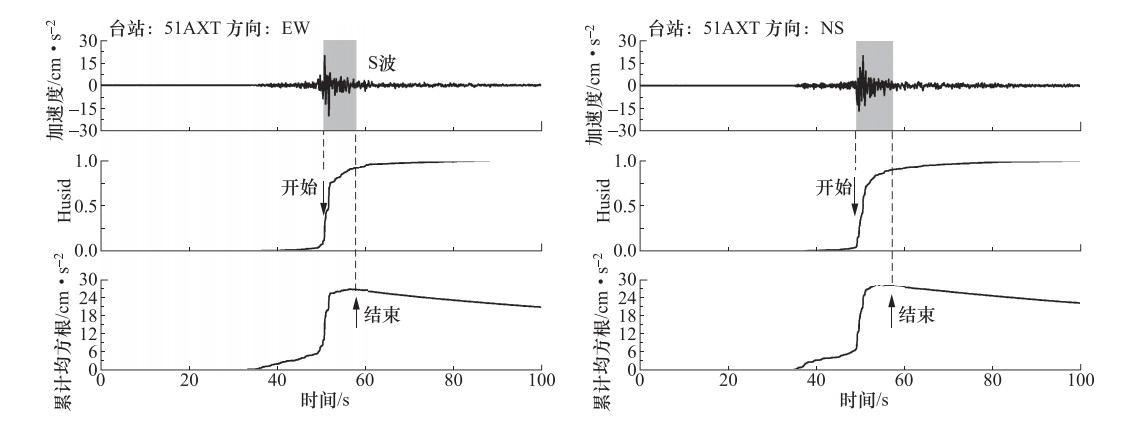
$${T_{d - S}}{\rm{ = }}{t_{S2}} - {\rm{ }}{t_{S1}}$$ (3) 
图 3 强震动记录S波窗口拾取示例Figure 3. Illustration of selecting S wave window in strong motion recordings国内外研究学者已证实上述拾取方法具有实用性（温瑞智等，2015b；Hassani等，2011；Wang等，2018），且该方法在强震动记录的震源效应、路径衰减和场地反应的广义反演及H/V谱比相关研究中得到有效应用。以安县塔水台站一条汶川地震余震记录（编号051AXT080513044531）为例，根据上述2种函数的计算方法识别此记录东西向（EW）和北南向（NS）加速度时程的S波时段（见图 3）。由图 3可知，两方向S波时段差异甚微，拾取效果较理想。
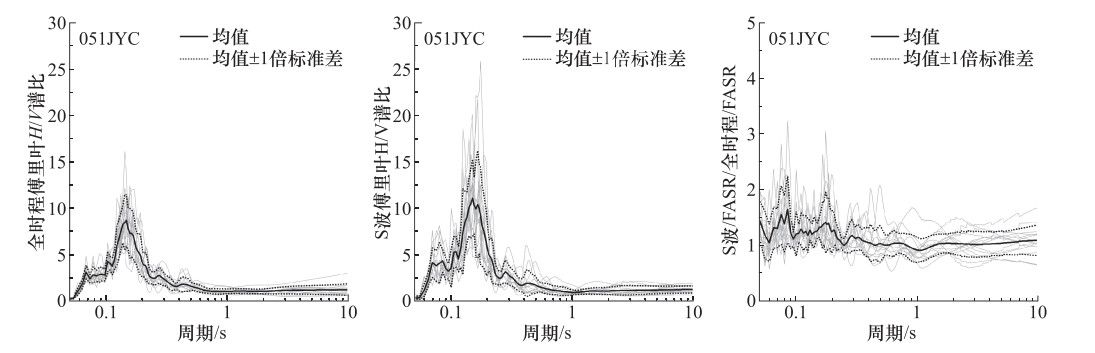
本文以数据集中江油重华台站（编号051JYC）的8个记录为例，分别计算全时程、S波傅里叶H/V谱比及二者的比值，用以对比二者对地震动放大效应的影响，计算结果如图 4所示。由图 4可知，S波H/V谱比曲线平均振幅总体上高于全时程，当周期 < 1s时S波的场地放大效应更显著，体现了S波截取的必要性。同时，工程界更多关注S波对上部结构的作用，研究S波的场地放大效应也更具工程意义。因此，本文建议在相关的研究工作中应尽可能截取合适的S波窗口用于计算强震动记录的H/V谱比，从而提高场地效应分析的适用性。
图 4 强震动记录全时程、S波H/V谱比对比Figure 4. H/V spectral ratio of whole-record and S wave window and the ratio of FASR3. Taper预处理对数据的影响
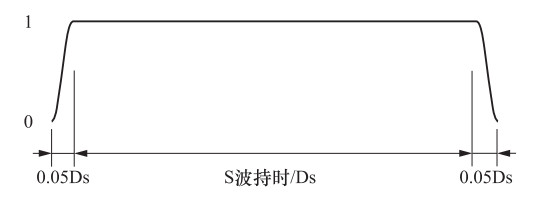
实际观测的地震动记录是有限且离散的数据点，在进行离散傅里叶变换的时域和频域上均呈现出离散的形式，这种离散性往往造成频谱分析中存在一些特殊现象，如频谱泄露、频谱混叠、栅栏现象（程佩青，2001）。而截断数字信号，必然会引起能量泄露，为减少泄露，数字信号中常采用窗函数进行截断。进行强震动记录的H/V谱比计算时，强震动记录中截取S波时会出现数据首尾两端不为零且数值较大的现象，因而对其进行傅里叶变换时将产生边瓣效应（王勤彩等，2005），如果不加以处理，必然影响H/V谱比的计算结果。因此，本文在截取S波窗口后的强震动记录首尾两端各加5%S波时长的余弦窗进行Taper预处理，能有效消除边瓣效应的影响，图 5所示为Taper预处理计算示意（Ren等，2013）。
本文中S波窗口首端Taper处理函数为：
$${W_i} = \frac{1}{2}\left[ {1{\rm{ + }}\cos \left({{\rm{ \mathit{ π} }}\frac{{{n_{\rm{b}}} + i - 1}}{{{n_{\rm{b}}}}}} \right)} \right], i = 1, ...{n_{\rm{b}}}$$ (4) S波窗口尾端Taper处理函数为：
$${W_i} = \frac{1}{2}\left[ {1{\rm{ + }}\cos \left({{\rm{ \mathit{ π} }}\frac{{i - 1}}{{{n_{\rm{e}}}}}} \right)} \right], i = 1, ...{n_{\rm{e}}}$$ (5) 式中nb、ne分别表示Taper处理中首尾端余弦窗长所含数据点数量。
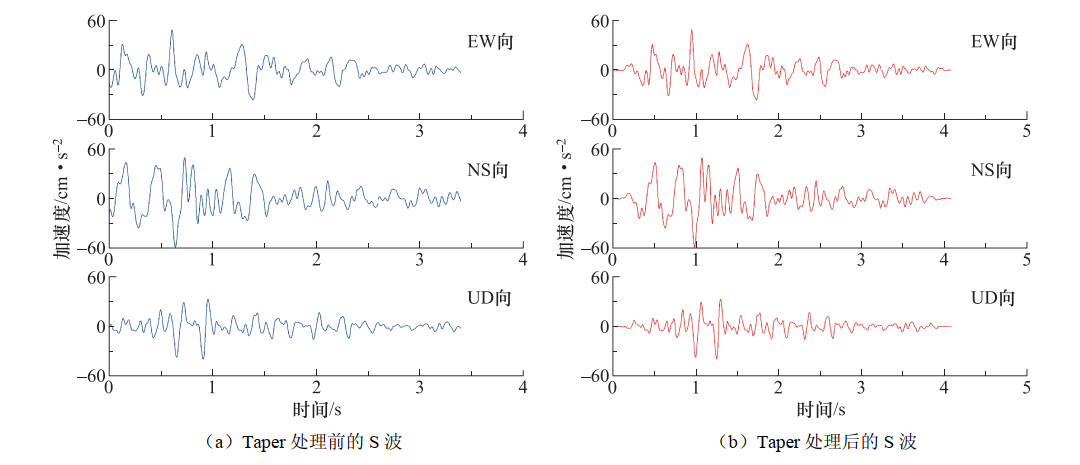
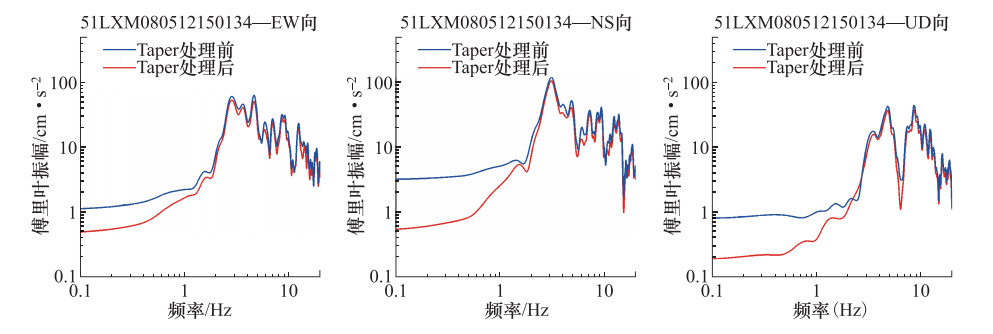
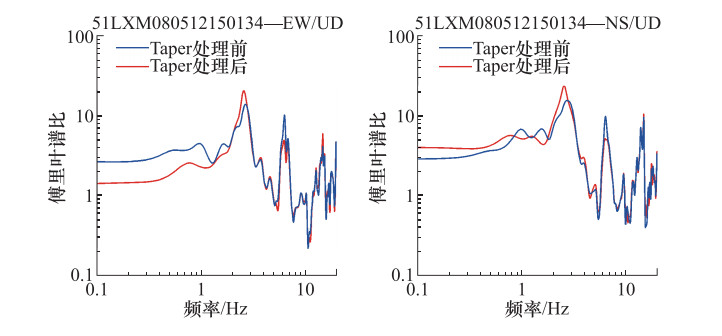
本文以理县木卡台（编号051LXM）捕获的一组记录051LXM080512150134为例，说明Taper处理对截取后记录的影响。图 6所示为截取的S波在Taper处理前后的加速度时程，由图 6可知，S波首尾并不为零，尤其是EW向和NS向的S波首端幅值为40cm/s2左右，Taper处理后3个方向的时程首尾均归零。分别计算记录S波Taper处理前后三分量的傅里叶幅值谱，计算结果如图 7所示，可知在低频部分二者的差异非常明显。图 8所示为该记录EW、NS水平方向S波时程与UD垂直方向在Taper处理前后计算的H/V谱比曲线，可知在 < 3Hz频带范围内Taper处理对H/V谱比计算的影响非常明显，若不进行Taper处理，将直接误导场地效应的分析结果，证实了对截取后的S波数据两端进行加窗处理的必要性。后文将对上述强震动记录数据集计算傅里叶H/V谱比前，统一对所有S波截取的数据进行Taper预处理。
图 6 Taper处理前后的时程对比Figure 6. The difference of S wave time recording between tapering(b) and without tapering(a)
图 7 Taper处理前后的傅氏谱对比Figure 7. The difference of S wave window Fourier spectrum between tapering and without tapering
图 8 Taper处理对H/V谱比计算的影响Figure 8. The influence of calculating H/V spectral ratio with tapering S wave window4. Parzen窗带宽对记录平滑的影响
对强震动记录进行截取和Taper处理后，记录仍以离散数据点的形式存在，此时进行傅里叶变换得到的强震动记录傅里叶振幅谱通常呈锯齿状，若直接将转换后的数据应用到H/V谱比计算中，频带分布范围和能量分布情况难以确定，如果不进行平滑，会增加场地卓越频率的识别难度。实际操作过程中，平滑通过加窗实现，平滑窗带宽取值关系到平滑的强弱。如果带宽过大导致平滑过度，将低估场地放大幅值，而带宽过小致使平滑太弱，又将影响卓越频率的识别，所以带宽取值是权衡谱平滑度的关键性问题。本文以常用的帕曾（Parzen）窗函数为例（大崎顺彦，2008），研究H/V谱比计算中合理的带宽取值。为不改变原波形具有的功率，先将傅里叶振幅谱转换为功率谱，对功率谱进行平滑；然后从平滑后的功率谱再转换回傅里叶振幅谱（罗桂纯，2015）。在平滑过程中，带宽在功率谱的首尾附近会超出谱的范围，故本文将功率谱两端连接起来，循回使用数据；然后分别选取带宽0.1Hz、0.3Hz、0.5Hz、0.7Hz、1.0Hz的Parzen窗，对比平滑效果。
本文采用H/V谱比平均值和标准差评价平滑效果的强弱，以广元石井台站（编号051GYS）为例，通过该台站汶川余震记录的S波在不同带宽Parzen窗下的H/V谱比曲线（见图 9），说明不同带宽Parzen窗的平滑效果。由图 9可知，随着Parzen窗带宽的增加，H/V谱比的平均值和标准差均逐渐减小；H/V谱比曲线平滑效果逐渐加强；曲线卓越周期处的峰值降低，但卓越周期无明显变化。
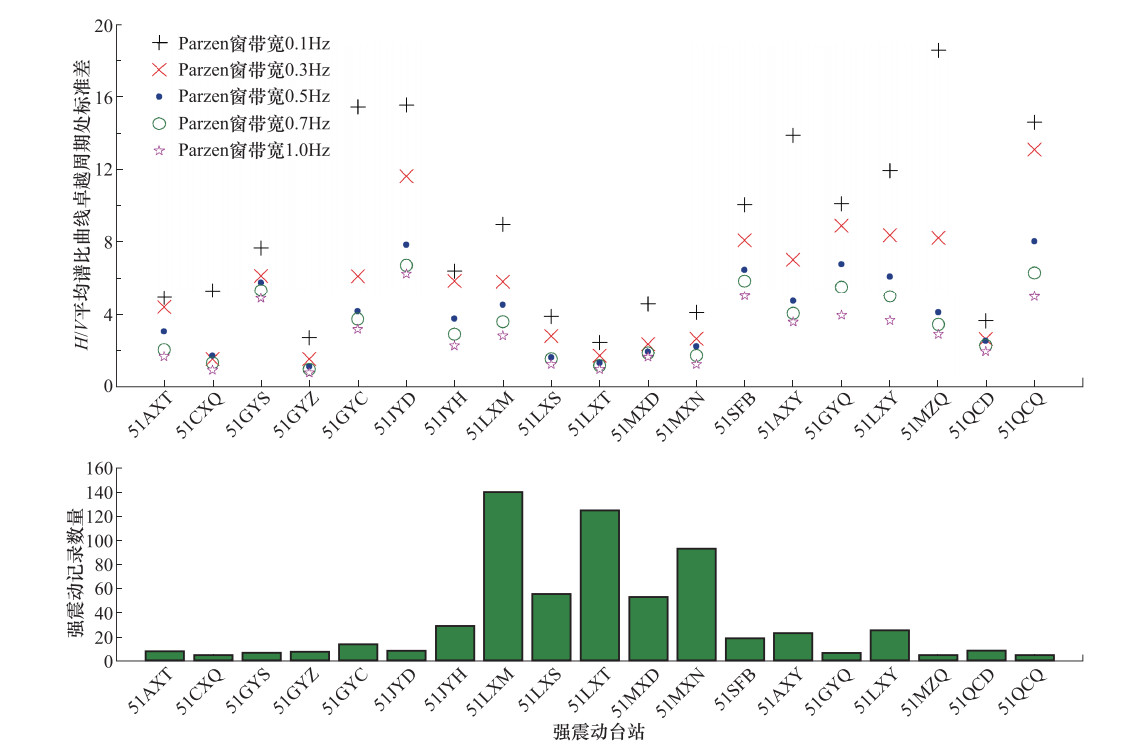
图 9 不同带宽Parzen窗平滑后的S波H/V谱比曲线对比Figure 9. The distinctness of H/V spectral ratio caves of S wave window smoothed with different band Parzen window除单个台站外，图 10给出不同台站强震动记录S波H/V平均谱比曲线在卓越周期处的标准差，图 11给出各台站在0.05—3s周期段内H/V平均谱比曲线在所有周期点标准差的平均值。标准差能反映数据集的离散程度，其值越大则数据集越离散。因此，由图 10、11可知，无论是卓越周期处的标准差，还是0.05—3s周期段内标准差的平均值，均随着Parzen窗带宽的增大而减小，反映了H/V谱比曲线随Parzen窗带宽增大而逐渐平滑的变化趋势。此外，分析图 10、11中的标准差值不难发现，带宽0.1Hz、0.3Hz的H/V谱比曲线离散性相对较大，带宽0.5Hz、0.7Hz、1.0Hz的H/V谱比曲线离散程度相对较低且标准差值相近。同时还可以发现，对于强震动记录数量较多的台站，卓越周期处的H/V谱比标准差普遍相对较低。究其原因，对每条记录的H/V谱比曲线进行平均实际上也是一种平滑过程，记录数量越多，在一定意义上增加了平滑次数，加强了平滑效果。
图 10 S波H/V平均谱比曲线在卓越周期处的标准差及所采用的强震动记录数量Figure 10. The standard deviation of average curve of S wave H/V spectral ratio in predominant period and recording number in different stations
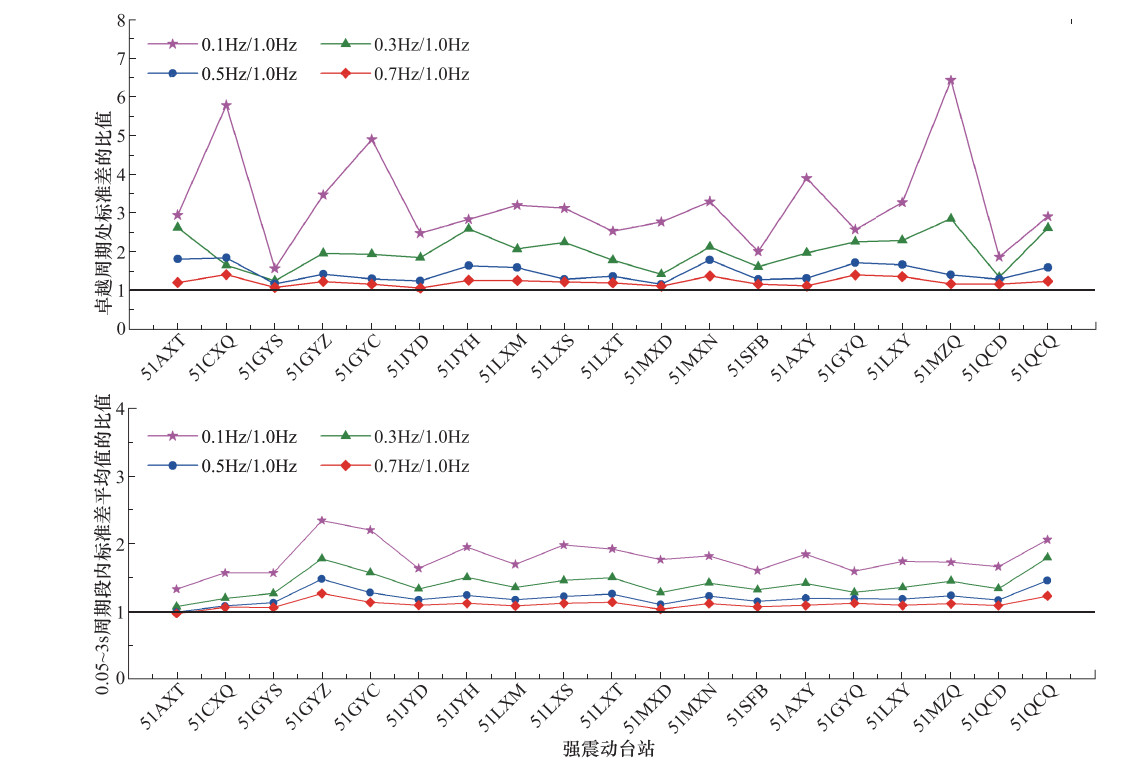
图 11 S波H/V平均谱比曲线在0.05—3s周期段内标准差的平均值Figure 11. The mean value of standard deviation of S wave H/V spectral ratio in 0.05s-3s period由图 9—11可知，经1.0Hz带宽的Parzen窗平滑后，H/V平均谱比的标准差相对较小，平滑效果较好。为更直观地判断其他带宽Parzen窗的平滑效果是否合适，以带宽1.0Hz的Parzen窗下H/V平均谱比曲线在卓越周期处的标准差和0.05—3s周期段内标准差的平均值为参考，分别计算0.1Hz、0.3Hz、0.5Hz、0.7Hz带宽Parzen窗下的结果与比值，如图 12所示。由图 12可知，无论是卓越周期处的标准差，还是全周期内标准差的平均值，0.1Hz带宽下的比值不稳定，不同台站结果差异显著，部分台站的比值偏大；0.3Hz的结果略优于前者，但也不尽如人意；而0.5Hz、0.7Hz带宽下的比值均在2以内，且比值曲线也相对平缓，大部分台站的结果非常接近1.0Hz。尽可能降低平滑对H/V谱比幅值的削弱影响，同时兼顾卓越周期的可识别性，本文认为0.5Hz的Parzen窗带宽较适用于平滑H/V傅里叶谱比曲线。
图 12 H/V平均谱比曲线标准差与1.0Hz带宽下结果的比值Figure 12. The ratio of standard deviation of H/V spectral ratio smoothed by Parzen window in different band to the result under 1.0Hz5. 结论
本文针对地震工程相关领域中常用的强震动记录H/V谱比法开展研究，选取642组汶川余震记录作为研究对象，重点关注数据处理的几个关键环节，主要工作和结论如下：
（1）以江油重华台站为例，对比全时程和S波的H/V谱比，结果表明S波窗口截取对H/V谱比计算有影响，当周期 < 1s时，其影响不容忽视，进而对场地放大效应分析产生影响，建议在计算H/V谱比时截取适当的S波窗口。
（2）对比Taper处理前后的S波时程、傅里叶振幅谱及谱比，以示例说明S波窗口截断引起傅里叶振幅谱的边瓣效应，不加以处理将显著影响低频部分的谱比结果，以示例证明对截取的S波时程首尾进行Taper预处理可消除这种截断误差。
（3）对比19个台站在不同带宽（0.1Hz、0.3Hz、0.5Hz、0.7Hz、1.0Hz）Parzen窗平滑下，S波H/V平均谱比曲线在卓越周期处的标准差及全周期内标准差均值，兼顾平滑效果及卓越周期识别的准确度，认为0.5Hz带宽的Parzen窗宜用于平滑H/V傅里叶谱比曲线。
致谢: 感谢中国地震局工程力学研究所“国家强震动台网中心”为本研究提供数据支持。 -
图 1 震中-台站方位的分布
Figure 1. Orientation distribution of earthquake epicenters and stations
图 2 构建持时数据库中各参数的分布情况
Figure 2. Distribution of parameters in constructed duration database
图 3 Ds5-95计算示意及Husid函数
Figure 3. Significant duration Ds5-95 using the Husid plot for acceleration time history
图 4 三分量地震动记录显著持时Ds5-95随断层距Rrup的分布
Figure 4. Distribution of significant duration Ds5-95 for three-component ground motion records versus rupture distance Rrup
图 5 不同持时预测方程显著持时Ds5-95的事件间残差随矩震级的变化
Figure 5. The inter-event residual and of significant duration Ds5-95 versus moment magnitude MW in different duration ground motion prediction equations
图 6 不同持时预测方程显著持时Ds5-95的事件内残差及分组均值标准差随断层距的变化
Figure 6. The intra-event residual and their binned means and ±1 standard deviations of significant duration Ds5-95 versus rupture distance Rrup in different duration ground motion prediction equations
7 不同持时预测方程显著持时Ds5-95的事件内残差及分组均值标准差随矩震级的变化。
7. The intra-event residual and their binned means and ±1 standard deviations of significant duration Ds5-95 versus moment magnitude Mw in different duration ground motion prediction equations
图 8 不同持时预测方程显著持时Ds5-95的事件内残差及分组均值标准差随VS30的变化
Figure 8. The intra-event residual and their binned means and ±1 standard deviations of significant duration Ds5-95 versus VS30 in different duration ground motion prediction equations
图 9 不同持时预测方程显著持时Ds5-95的事件内残差及同一Z2.5值对应的残差均值随Z2.5的变化
Figure 9. The intra-event residual and mean residual of the same Z2.5 value of significant duration Ds5-95 versus Z2.5 in different duration ground motion prediction equations
表 1 地震基本信息
Table 1. Information of selected crustal earthquakes
发震时间 矩震级MW 震中纬度/ ° 震中经度/ ° 断层距范围/ km 事件台站数量/ 个 事件记录总数/ 条 2004年10月23日17时56分 6.6 37.289 5 138.870 3 41.8～258.4 62 186 2004年10月23日18时34分 6.3 37.303 3 138.933 2 48.0～263.3 61 183 2007年07月16日10时13分 6.6 37.556 8 138.609 5 72.8～297.7 63 189 2011年03月12日03时59分 6.3 36.986 0 138.597 8 22.6～248.4 62 186 2011年03月16日12时52分 5.8 35.837 0 140.906 5 10.0～220.8 60 180 2011年03月19日18时56分 5.8 36.783 7 140.571 5 7.7～201.4 64 192 2011年04月11日17时16分 6.7 36.945 7 140.672 7 12.8～227.7 65 195 2011年04月11日20时42分 5.5 36.966 0 140.635 0 20.8～233.7 64 192 2011年04月12日14时07分 5.9 37.052 5 140.643 5 25.1～239.0 65 195 2011年04月13日10时08分 5.3 36.915 0 140.709 7 16.6～229.3 64 192 2012年03月14日21时05分 6.0 35.747 7 140.932 0 8.7～226.1 61 183 2013年09月20日02时25分 5.4 37.051 3 140.695 3 17.6～231.8 59 177 2016年11月22日05时59分 6.9 37.354 7 141.604 2 79.9～296.0 64 192 2016年12月28日21时38分 5.9 36.720 2 140.574 2 6.2～203.4 64 192 2017年08月02日02时02分 5.1 36.803 5 140.535 2 8.3～202.6 61 183 
下载: 导出CSV
表 2 台站基本信息
Table 2. Information of selected stations inside and outside Kanto basin
台站编码 位于盆地内外情况 纬度/ ° 经度/ ° 台站编码 位于盆地内外情况 纬度/ ° 经度/ ° CHBH06 盆地内 35.721 5 140.504 6 GNMH12 盆地外 36.144 0 138.912 9 CHBH10 盆地内 35.545 8 140.241 7 GNMH13 盆地外 36.862 0 139.062 7 CHBH13 盆地内 35.830 7 140.298 0 GNMH14 盆地外 36.493 1 139.321 9 CHBH14 盆地内 35.734 2 140.823 0 IBRH06 盆地外 36.880 9 140.654 5 GNMH05 盆地内 36.314 3 139.184 7 IBRH11 盆地外 36.370 1 140.140 1 GNMH11 盆地内 36.286 2 138.921 0 IBRH12 盆地外 36.836 9 140.318 1 IBRH07 盆地内 35.952 1 140.330 1 IBRH13 盆地外 36.795 5 140.575 0 IBRH10 盆地内 36.111 2 139.988 9 IBRH14 盆地外 36.692 2 140.548 4 IBRH17 盆地内 36.086 4 140.314 0 IBRH15 盆地外 36.556 6 140.301 3 IBRH18 盆地内 36.363 1 140.619 8 IBRH16 盆地外 36.640 5 140.397 6 IBRH19 盆地内 36.213 7 140.089 3 KNGH11 盆地外 35.404 0 139.353 9 IBRH20 盆地内 35.828 4 140.732 3 KNGH18 盆地外 35.643 7 139.128 3 KNGH10 盆地内 35.499 1 139.519 5 KNGH19 盆地外 35.417 3 139.043 6 SITH03 盆地内 35.899 0 139.384 3 KNGH20 盆地外 35.366 3 139.126 0 SITH04 盆地内 35.802 8 139.535 3 KNGH21 盆地外 35.462 8 139.214 6 SITH06 盆地内 36.113 1 139.289 4 KNGH22 盆地外 35.358 3 139.091 0 TCGH06 盆地内 36.445 8 139.950 9 NGNH17 盆地外 36.142 5 138.550 4 TCGH10 盆地内 36.857 8 140.022 5 NGNH19 盆地外 35.973 5 138.584 5 TCGH12 盆地内 36.695 9 139.984 2 NIGH19 盆地外 36.811 4 138.784 9 TCGH13 盆地内 36.734 2 140.178 1 SITH05 盆地外 36.150 9 139.050 4 TCGH15 盆地内 36.559 5 139.863 7 SITH07 盆地外 35.911 8 139.148 5 TCGH16 盆地内 36.548 0 140.075 1 SITH08 盆地外 36.027 4 138.969 1 CHBH11 盆地外 35.286 7 140.152 9 SITH09 盆地外 36.071 5 139.099 3 CHBH12 盆地外 35.344 5 139.855 4 SITH10 盆地外 35.996 4 139.219 1 CHBH15 盆地外 34.959 1 139.788 5 SITH11 盆地外 35.863 7 139.272 6 CHBH16 盆地外 35.138 4 139.964 9 TCGH07 盆地外 36.881 7 139.453 4 CHBH17 盆地外 35.171 4 140.339 8 TCGH08 盆地外 36.882 8 139.645 9 CHBH20 盆地外 35.088 2 140.099 7 TCGH09 盆地外 36.862 5 139.836 4 FKSH05 盆地外 37.254 4 139.872 5 TCGH11 盆地外 36.708 4 139.769 4 FKSH06 盆地外 37.172 3 139.519 9 TCGH14 盆地外 36.550 9 139.615 4 FKSH10 盆地外 37.161 6 140.093 0 TCGH17 盆地外 36.985 3 139.692 2 FKSH13 盆地外 36.995 1 140.585 3 TKYH12 盆地外 35.670 1 139.265 0 GNMH07 盆地外 36.699 8 139.210 4 TKYH13 盆地外 35.701 7 139.127 5 GNMH08 盆地外 36.491 7 138.524 4 YMNH11 盆地外 35.624 7 138.977 7 GNMH09 盆地外 36.621 2 138.906 8 YMNH14 盆地外 35.511 5 138.967 5 GNMH10 盆地外 36.235 6 138.729 1
下载: 导出CSV
表 3 3类显著持时预测方程概述
Table 3. The summary of three significant duration prediction equations
项目 KS06方程 AS16方程 BRG21方程 方程、基础数据
库及适用范围$ \ln Ds = \ln ({D_{{\rm{source}}}} + {D_{{\rm{path}}}} + {D_{{\rm{site}}}}) $，
NGA-West1水平向地震动数据库，
MW为5～7.6级，Rrup为0～200 km$ \ln Ds = \ln ({D_{{\rm{source}}}} + {D_{{\rm{path}}}}) + {D_{{\rm{site}}}} $，NGA-West2水平向地震动数据库，MW为3～8级（其中走滑和逆断层为3～8级，正断层为3～7级），断层距Rrup为0～300 km，VS30为150～1 500 m/s，Z1.0为0～3 km $ \ln Ds = \ln ({D_{{\rm{source}}}} + {D_{{\rm{path}}}}) + {D_{{\rm{site}}}} $，日本KiK-net水平向地震动数据库，MW为4～7.5级，断层距Rrup为0～200 km，VS30为150～1 500 m/s，Z1.0为0～400 km 震源项 $ \,{M_0} = {10^{1.5 M + 16.05\;}} $
$ \,\Delta \sigma = \exp [{b_1} + {b_2}(M - {M^*})] $
${f_{\rm{c}}} = 4.9 \times {10^6} \times \beta {(\Delta \sigma /{M_0})^{1/3} }$
${D_{ {\rm{source} } } } = f_{\rm{c}}^{ - 1}$$ \,{M_0} = {10^{1.5 M + 16.05\;}} $
$ \Delta \sigma = \left\{ \begin{gathered} \exp [{b_1} + {b_2}(M - {M^*})],\,\;M \leqslant {M_2} \\ \exp [{b_1} + {b_2}({M_2} - {M^*}) \\ + {b_3}(M - {M_2})],\,\,\,M > {M_2}\; \\ \end{gathered} \right. $
${f_{\rm{c}}} = 4.9 \times {10^6} \times \beta {(\Delta \sigma /{M_0})^{1/3} }$
${D_{\rm{source} } } = \left\{ \begin{gathered} \;\;\;1/{f_{\rm{c}}},\;\;M > {M_1} \\ \,\,\,\,\,\,\,\,{b_0},\;\;\;\,M \leqslant {M_1}\;\; \\ \end{gathered} \right.$$ \,{M_0} = {10^{1.5 M + 16.05\;}} $
$ \Delta \sigma = \exp ({b_1} + {b_2}M) $
${f_{\rm{c}}} = 4.9 \times {10^6} \times \beta {(\Delta \sigma /{M_0})^{1/3} }$
$ \ln {D_{{\rm{source}}}} = {10^{{m_1}(M - {m_2})}} + {m_3} $路径项 $ {D_{{\rm{path}}}} = {c_2}{R_{{\rm{rup}}}} $ $ {D_{{\rm{path}}}} = \left\{ \begin{gathered} {c_1}{R_{\rm{rup}}},\,{R_{\rm{rup}}} \leqslant {R_1} \\{c_1}{R_1} + {c_2}({R_{\rm{rup}}} - {R_1}),\;{R_1}\; < {R_{\rm{rup}}} \leqslant {R_2}\; \\ {c_1}{R_1} + {c_2}({R_2} - {R_1}) \\ + {c_3}({R_{\rm{rup}}} - {R_2}),\;{R_{\rm{rup}}}\; > {R_2} \\ \end{gathered} \right. $ ${D_{path} } = \left\{ \begin{gathered}{r_1} \cdot {R_{rup} },\;{R_{rup} } \leqslant {R_1} \\{r_1} \cdot [{R_1} + MSE({R_{rup} } - {R_1})],\;{R_{rup} } > {R_1}\end{gathered} \right.$
$ MSE = \left\{ \begin{gathered}0,\; M \leqslant {M_1} \\\frac{ {M - {M_1} } }{ { {M_2} - {M_1} } },\;{M_1} < M \leqslant {M_2} \\1,\;M > {M_2} \\\end{gathered} \right. $场地项 二元场地模型：$ {D_{{\rm{site}}}} = {c_1}S $，S取值为
0或1；VS30模型：$ {D_{{\rm{site}}}} = {c_4} + {c_5}{V_{{\rm{S}}30}} $；
VS30与盆地深度的综合模型：$ {D_{{\rm{site}}}} = {c_4} + {c_5}{V_{{\rm{S}}30}} + {c_6} + {c_7}{Z_{1.5}} $${D_{{\rm{site}}} } = \left\{ \begin{gathered} {c_4}\ln \left( {\frac{ { {V_{{\rm{S}}30} } } }{ { {V_{{\rm{ref}}} } } }} \right) + {F_{\delta {Z_1} } }\;\;\;{V_{{\rm{S}}30} } \leqslant {V_1} \\ {c_4}\ln \left( {\frac{ { {V_1} } }{ { {V_{{\rm{ref}}} } } }} \right) + {F_{\delta {Z_1} } }\;\;\;{V_{{\rm{S}}30} } > {V_1}\;\; \\ \end{gathered} \right.$
${F_{\delta {Z_1} } } = \left\{ \begin{gathered} \;{c_5}\delta {Z_1}\;\;\;\;\;\delta {Z_1} \leqslant \delta {Z_{1,{\rm{ref}}} } \\ {c_5}\delta {Z_{1,{\rm{ref}}} }\;\;\;\delta {Z_1} > \delta {Z_{1,{\rm{ref}}} } \\ \end{gathered} \right.$
$ \delta {Z_1} = {Z_1} - {\mu _{Z1}} $
$\begin{gathered} \ln ({\mu _{Z1} }) \\ = \frac{ { - 5.23} }{2}\ln \left( {\frac{ {V_{{\rm{S}}30}^2 + { {412.39}^2} } }{ { { {1360}^2} + { {412.39}^2} } } } \right) - \ln 1000 \\ \end{gathered}$$\begin{gathered} {D_{{\rm{site}}} } = {s_1}\ln \left( {\frac{ {\min ({V_{{\rm{S}}30} },600)} }{ {600} } } \right) \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, + {s_2}\min (\delta {Z_1},250)\, + {s_3} \\ \end{gathered}$
${Z_{1,P} } = \exp \left[ - \dfrac{ {5.23} }{2}\ln \left( {\dfrac{ {V_{ {\rm{S} }30}^2 + { {412}^2} } }{ { { {1360}^2} + { {412}^2} } } } \right) - 0.9 \right]$$ \delta {Z_1} = {Z_1} - {Z_{1,P}} $方程系数 $ {b_1},{b_2},{c_1},{c_2},{c_4},{c_5},{c_6},{c_7},{M^*} $系数参见Kempton等（2006）的研究 $ {b_0},{b_1},{b_2},{b_3},{c_1},{c_2},{c_3},{c_4},{c_5} $和
${M^*},{M_1},{M_2},{R_1},{R_2},{V_1},\delta {Z_{1,{\rm{ref}}} }$系数参见Afshari等（2016）的研究$ {b_1},{b_2},{m_1},{m_2},{r_1},{R_1},{s_1},{s_2},{s_3} $系数参见Bahrampouri等（2021）的研究 注：为表述统一，3个持时预测方程中震源、路径、场地项符号与原文略有差异。M为震级，一般取矩震级MW，注意KS06方程中，当无可用的矩震级时，6级以上使用面波震级MS，6级以下使用地方震级ML；Rrup为断层距，为场点或台站到断层的最近距离，单位km；VS30为地面以下30 m平均剪切波速，单位m/s；z1为地面到剪切波速为1 km/s等值面的深度，单位km；Z1.5为地面到剪切波速为1.5 km/s等值面的深度，单位km；μZ1和Z1,P均为根据VS30预测的Z1值，其在AS16方程中的单位为km，在BRG21方程中的单位为m； fc为拐角频率，单位Hz；Δσ为应力降指标，单位为bar；M0为地震矩，单位为dyne-cm；β为震源处剪切波速，单位km/s，本研究取3.2 km/s。
下载: 导出CSV
-
刘浪, 李小军, 彭小波, 2011. 汶川地震中强震动相对持时的空间变化特性研究. 地震学报, 33（6）: 809—816 doi: 10.3969/j.issn.0253-3782.2011.06.011Liu L. , Li X. J. , Peng X. B. , 2011. Study on relative duration of strong motions during the great Wenchuan earthquake. Acta Seismologica Sinica, 33(6): 809—816. (in Chinese) doi: 10.3969/j.issn.0253-3782.2011.06.011 中华人民共和国住房和城乡建设部, 中华人民共和国国家质量监督检验检疫总局, 2010. GB 50011—2010（2016年版） 建筑抗震设计规范. 北京: 中国建筑工业出版社, 19—21Ministry of Housing and Urban-Rural Development of the People’s Republic of China, General Administration of Quality Supervision, Inspection and Quarantine of the People's Republic of China, 2010. GB 50011—2010 Code for seismic design of buildings. Beijing: China Architecture & Building Press, 19—21. (in Chinese) Abraham J. R. , Smerzini C. , Paolucci R. , et al. , 2016. Numerical study on basin-edge effects in the seismic response of the Gubbio valley, Central Italy. Bulletin of Earthquake Engineering, 14(6): 1437—1459. doi: 10.1007/s10518-016-9890-y Afshari K. , Stewart J. P. , 2016. Physically parameterized prediction equations for significant duration in active crustal regions. Earthquake Spectra, 32(4): 2057—2081. doi: 10.1193/063015EQS106M Aochi H. , Douglas J. , 2006. Testing the validity of simulated strong ground motion from the dynamic rupture of a finite fault, by using empirical equations. Bulletin of Earthquake Engineering, 4(3): 211—229. doi: 10.1007/s10518-006-0001-3 Bahrampouri M. , Rodriguez-Marek A. , Green R. A. , 2021. Ground motion prediction equations for significant duration using the KiK-net database. Earthquake Spectra, 37(2): 903—920. doi: 10.1177/8755293020970971 Baltay A. S. , Hanks T. C. , Abrahamson N. A. , 2017. Uncertainty, variability, and earthquake physics in ground-motion prediction equations. Bulletin of the Seismological Society of America, 107(4): 1754—1772. Bijelić N. , Lin T. , Deierlein G. G. , 2019. Quantification of the influence of deep basin effects on structural collapse using SCEC CyberShake earthquake ground motion simulations. Earthquake Spectra, 35(4): 1845—1864. doi: 10.1193/080418EQS197M Bommer J. J. , Martínez-Pereira A. , 1999. The effective duration of earthquake strong motion. Journal of Earthquake Engineering, 3(2): 127—172. Boore D. M. , 2003. Phase derivatives and simulation of strong ground motions. Bulletin of the Seismological Society of America, 93(3): 1132—1143. doi: 10.1785/0120020196 Boore D. M. , Sisi A. A. , Akkar S. , 2012. Using pad-stripped acausally filtered strong-motion data. Bulletin of the Seismological Society of America, 102(2): 751—760. doi: 10.1785/0120110222 Boore D. M. , Thompson E. M. , 2014. Path durations for use in the stochastic-method simulation of ground motions. Bulletin of the Seismological Society of America, 104(5): 2541—2552. doi: 10.1785/0120140058 Hancock J. , Bommer J. J. , 2006. A state-of-knowledge review of the influence of strong-motion duration on structural damage. Earthquake Spectra, 22(3): 827—845. doi: 10.1193/1.2220576 Kaklamanos J. , Baise L. G. , Boore D. M. , 2011. Estimating unknown input parameters when implementing the NGA ground-motion prediction equations in engineering practice. Earthquake Spectra, 27(4): 1219—1235. doi: 10.1193/1.3650372 Kallioras S. , Graziotti F. , Penna A. , et al. , 2022. Effects of vertical ground motions on the dynamic response of URM structures: comparative shake-table tests. Earthquake Engineering & Structural Dynamics, 51(2): 347—368. Kamarroudi S. H. , Hosseini M. , Hosseini K. , 2021. Influence of earthquake vertical excitations on Sloshing-Created P-Δ effect in elevated water Tanks: experimental Validation, numerical simulation and proposing a modification for Housner model. Engineering Structures, 246: 112995. doi: 10.1016/j.engstruct.2021.112995 Kempton J. J. , Stewart J. P. , 2006. Prediction equations for significant duration of earthquake ground motions considering site and near-source effects. Earthquake Spectra, 22(4): 985—1013. doi: 10.1193/1.2358175 Kolli M. K. , Bora S. S. , 2021. On the use of duration in random vibration theory (RVT) based ground motion prediction: a comparative study. Bulletin of Earthquake Engineering, 19(4): 1687—1707. doi: 10.1007/s10518-021-01052-w Lee S. J. , Chen H. W. , Liu Q. Y. , et al. , 2008. Three-dimensional simulations of seismic-wave propagation in the Taipei basin with realistic topography based upon the spectral-element method. Bulletin of the Seismological Society of America, 98(1): 253—264. doi: 10.1785/0120070033 Liang J. W. , Chaudhuri S. R. , Shinozuka M. , 2007. Simulation of nonstationary stochastic processes by spectral representation. Journal of Engineering Mechanics, 133(6): 616—627. doi: 10.1061/(ASCE)0733-9399(2007)133:6(616) Loghman V. , Khoshnoudian F. , Banazadeh M. , 2015. Effect of vertical component of earthquake on seismic responses of triple concave friction pendulum base-isolated structures. Journal of Vibration and Control, 21(11): 2099—2113. doi: 10.1177/1077546313503359 Marafi N. A. , Eberhard M. O. , Berman J. W. , et al. , 2017. Effects of deep basins on structural collapse during large subduction earthquakes. Earthquake Spectra, 33(3): 963—997. doi: 10.1193/071916eqs114m Meimandi-Parizi A. , Mahdavian A. , Saffari H. , 2022. New equations for determination of shaping window in stochastic method of simulating ground motion. Journal of Earthquake Engineering, 26(7): 3506—3522. doi: 10.1080/13632469.2020.1809560 Muscolino G. , Genovese F. , Biondi G. , et al. , 2021. Generation of fully non-stationary random processes consistent with target seismic accelerograms. Soil Dynamics and Earthquake Engineering, 141: 106467. doi: 10.1016/j.soildyn.2020.106467 Olsen K. B. , Mayhew J. E. , 2010. Goodness-of-fit criteria for broadband synthetic seismograms, with application to the 2008 Mw 5.4 Chino Hills, California, earthquake. Seismological Research Letters, 81(5): 715—723. doi: 10.1785/gssrl.81.5.715 Ruiz S. , Ojeda J. , Pastén C. , et al. , 2018. Stochastic strong-motion simulation in borehole and on surface for the 2011 Mw 9.0 Tohoku-Oki Megathrust Earthquake considering P, SV, and SH amplification transfer functions. Bulletin of the Seismological Society of America, 108(5 A): 2333—2346. doi: 10.1785/0120170342 Semblat J. F., Kham M., Parara E., et al., 2005. Seismic wave amplification: basin geometry vs soil layering. Soil Dynamics and Earthquake Engineering, 25(7—10): 529—538. Somerville P. G. , Smith N. F. , Graves R. W. , et al. , 1997. Modification of empirical strong ground motion attenuation relations to include the amplitude and duration effects of rupture directivity. Seismological Research Letters, 68(1): 199—222. doi: 10.1785/gssrl.68.1.199 Stewart J. P. , Blake T. F. , Hollingsworth R. A. , 2003. A screen analysis procedure for seismic slope stability. Earthquake Spectra, 19(3): 697—712. doi: 10.1193/1.1597877 Trifunac M. D. , Brady A. G. , 1975. A study on the duration of strong earthquake ground motion. Bulletin of the Seismological Society of America, 65(3): 581—626. Zhao J. X. , Zhou S. L. , Gao P. J. , et al. , 2015. An earthquake classification scheme adapted for Japan determined by the goodness of fit for ground-motion prediction equations. Bulletin of the Seismological Society of America, 105(5): 2750—2763. doi: 10.1785/0120150013 期刊类型引用(0)
其他类型引用(2)
-
下载:
点击查看大图
计量
- 文章访问数: 231
- HTML全文浏览量: 58
- PDF下载量: 25
- 被引次数: 2
