Three-dimensional Large-scale Marine Seismic Response Analysis Based on the Unified Computational Framework of Fluid-solid Interaction−A Case Study of Tokyo Bay
-
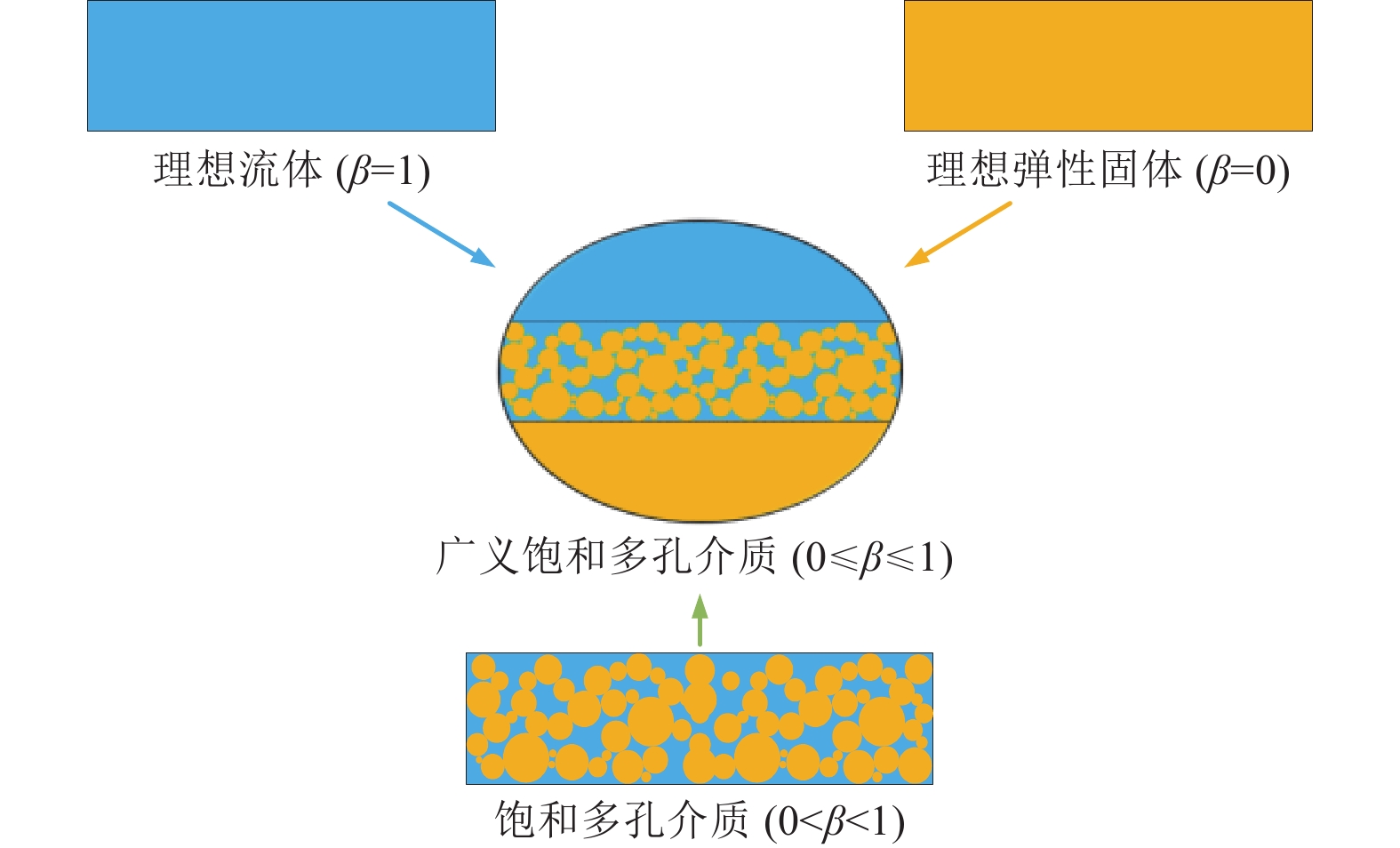
摘要: 海域场地地震响应分析是确定海洋工程结构抗震设计地震动输入的重要环节。然而，针对海水、饱和土、基岩之间的流固耦合分析，目前一般通过对3种介质方程进行离散，然后整体求解或分区耦合求解的方式进行，过程复杂而低效。因此，大规模海域场地地震反应分析仍是一个挑战性问题。本文基于流固耦合统一计算框架求解海域近场波动问题，采用透射边界模拟无限域，通过将海水和基岩视为孔隙率分别等于1和0的广义饱和多孔介质，使得海水、饱和土、基岩之间的相互耦合可在统一计算框架中实现，避免不同介质求解器之间的数据交换。采用集中质量显式有限元并行计算，不同进程之间采用MPI进行数据交换，提高计算效率；采用逐元技术，按单元类别存储单元刚度，大大节省了内存，便于大规模计算。通过自编程，输入界面高程数据和材料参数，实现建模-自由场-三维地震动模拟全流程自动化。以东京湾为例，使用该方法和程序在超级计算机上模拟SV波垂直入射时的地震响应，证实了该方法用于三维大规模海域地震波场模拟的高效性和可行性。Abstract: The seismic response analysis of marine sites plays an important role in determining the ground motion input for the seismic design of marine engineering structures. However, for the fluid-solid coupling analysis between seawater, saturated soil, and bedrock, the three media equations are generally discretized, and then the overall solution or partition coupling solution is performed, which is complex and inefficient. Therefore, the seismic response analysis of large-scale marine sites is difficult at present. In this paper, an efficient unified approach is proposed to solve the near-field seismoacoustic scattering problem, and the influence of the infinite domain is simulated by the transmitting boundary. Seawater and dry bedrock are considered as generalized saturated porous media with porosity equals to one and zero respectively, and the coupling between seawater, saturated seabed and dry bedrock can be analyzed in the unified framework of generalized saturated porous media and avoid interaction between different solvers. In order to improve the computing efficiency, the concentrated mass explicit finite element parallel computing is adopted, and MPI is used for data exchange between different processes. Through self-programming, users only need to input the interface elevation data and material parameters to realize the whole process automatic operation of modeling-free field-3D ground motion simulation. Finally, this method and program is used to simulate the seismic response of SV waves vertically incident on the Tokyo Bay area on high performance computer. The numerical results confirm the high efficiency and feasibility of the method for 3D large-scale sea seismic wave field simulation.
-
引言
为了确定工程的场地相关反应谱，需要考虑场地条件对地震动的影响。对于横向性质变化较小的工程场地，通常采用一维模型进行土层地震反应分析。核设施土层地震反应分析工作对地震动输入和土层模型的要求较高，如自由基岩表面地震动反应谱应按确定性和概率方法的计算结果分别确定、地震动输入界面的剪切波速不小于700m/s、应根据土力学性能测定结果确定模型参数等，这些技术要求通过限制模型参数的不确定性，提高了土层地震反应分析结果的可靠性。
考虑到土层地震反应分析中的不确定性，我国的地震安全性评价规范要求进行多个钻孔场地力学模型和多个地震动时程样本的组合地震反应分析计算，再综合评判多个钻孔场地力学模型和输入多个地震动组合时的计算结果，以确定场地的地震动参数。综合评判通常采用对地震动反应谱值的平均拟合方法或者外包络法（卢寿德，2006）。美国核电厂地震危险性分析工作基于工程场地的钻孔、原位测试和室内实验结果，根据动力特性和波速分布的研究成果，随机生成土层模型，表征土层模型参数的不确定性；采用调整天然地震动或利用随机振动理论方法，确定自由地表基岩地震动输入进行土层地震反应分析；对计算出的放大系数进行统计，再以放大系数分布的均值乘以基岩地震动反应谱，确定工程的场地相关谱（Silva等，1996；USNRC，2007）。
综上所述，中美两国核设施土层地震反应分析的总体思路是一致的，都是采用地震动-土层动力学模型组合的方式来考虑土层地震反应分析结果的不确定性。可能影响分析结果的参数包括基岩地震动、土层动力特性和土层剪切波速。
石玉成等（1999）采用Monte Carlo方法对影响土层地震反应分析结果的不确定因素进行了分析；王恒知等（2009）分析了时程拟合参数对土层地震反应分析结果的影响；施春花等（2009）统计分析了北京地区不同深度粘土的动力特性，采用等效线性化方法分析了统计结果的代表性，为北京地区难以取得原状土的工程场地地震安评工作提供了参考；王玉石等（2016）总结了强震动条件下土体非线性动力特性研究的发展历程和方向；沈建文等(2010, 2011)分析了土体剪应变折减系数对土层地震反应分析结果的影响，提出了采用震级和距离参数修正土层反应的等效线性化方法，建议使用设定地震定义震级和距离；Robinson等（2006）考虑了土层厚度和波速结构的不确定性，基于实测数据，随机生成土层模型，分析了悉尼港口地区的地震危险性及其不确定性。这些研究工作的地震动输入均为与目标谱匹配的地震动时程，加速度时程是地震动随机过程的实现。由于地震动随机过程的变异性较大，为了给出土层地表加速度反应谱的稳定估计值，需要采用多条时程进行多次分析。美国核管会（U. S. Nuclear Regulatory Commission，USNRC）的管理导则中推荐了随机振动理论（Random Vibration Theory，RVT）方法，该方法以幅值谱和持时作为输入，降低了分析工作的计算量和对地震动时程的依赖，可以快速准确地估计工程场地的地表地震动（Schneider等，1991；Silva等，1996；Boore，2003；Rathje等，2006；Ozbey，2006；USNRC，2007；Rathje等，2010；Boore等，2015）。
本文以某核电厂址为例，采用随机振动理论方法确定基岩地震动输入，基于工程场地土力学和剪切波速测试数据，随机生成土层模型，进行土层地震反应分析，讨论土层波速剖面、动力特性、基岩地震动输入界面不确定性对土层地震反应分析结果的影响。
1. 基岩地震危险性和土层模型
本文重点在于土层地震反应分析，因此不再详述厂址基岩地震危险性的评价过程，评价结果见图 1。图 1中的数据为厂址特定基岩地表地震动（SL-2），是概率法和确定论方法评价结果的包络值，其中概率论方法的年平均超越概率为1×10-4。
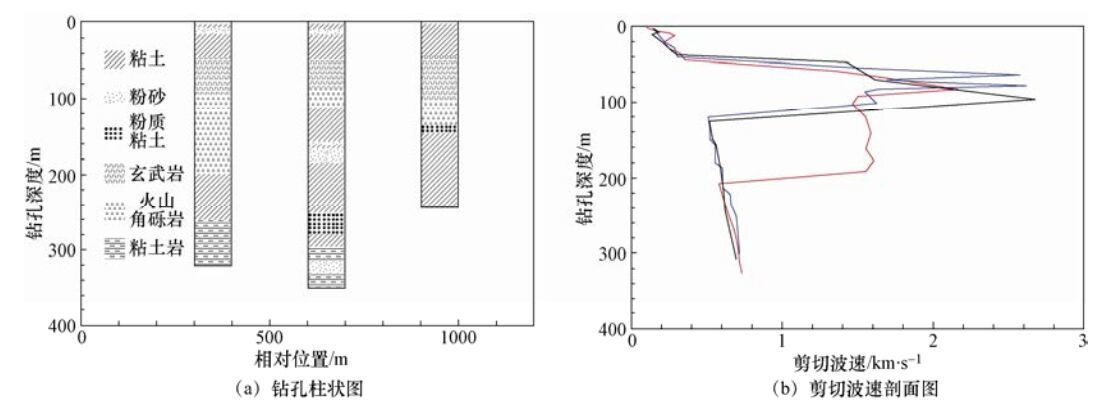
依据我国地震安全性评价工作的规范要求，在不同位置对工程场地进行了多个钻孔测试，将其中的控制孔分别命名为zk1、zk2和zk3。钻孔岩石地层柱状图和剪切波速剖面图见图 2。
图 2 工程场地钻孔柱状图和剪切波速剖面图Figure 2. Log columns and profiles of shear wave velocity of site图 2（a）中从左至右依次为zk1、zk2和zk3的剖面。从图 2（a）可知，工程场地的主要地层包括粘土、粉质粘土、粉砂、玄武岩、火山角砾岩、粘土岩，其中zk3未见粘土岩。图 2（b）中的红色、蓝色和黑色曲线分别代表zk1、zk2和zk3中介质剪切波速随深度的变化。由图 2（b）可以看出，粘土和砂土中的剪切波速随深度的增加逐渐变大，玄武岩和火山角砾岩中的剪切波速与土壤中的剪切波速相比，存在明显的突变，其中玄武岩的剪切波速又明显高于火山角砾岩中的剪切波速。3个钻孔位于同一场地中，相互距离处在百米量级上。土壤中剪切波速随深度的变化趋势基本一致，玄武岩和火山角砾岩中剪切波速随着风化程度的变化而变化，但是都远远高于美国西部强地面运动记录中基岩的定义值（760m/s），因此可以据此对zk3的剖面进行波速延拓，至国标定义的基岩地震动输入界面。
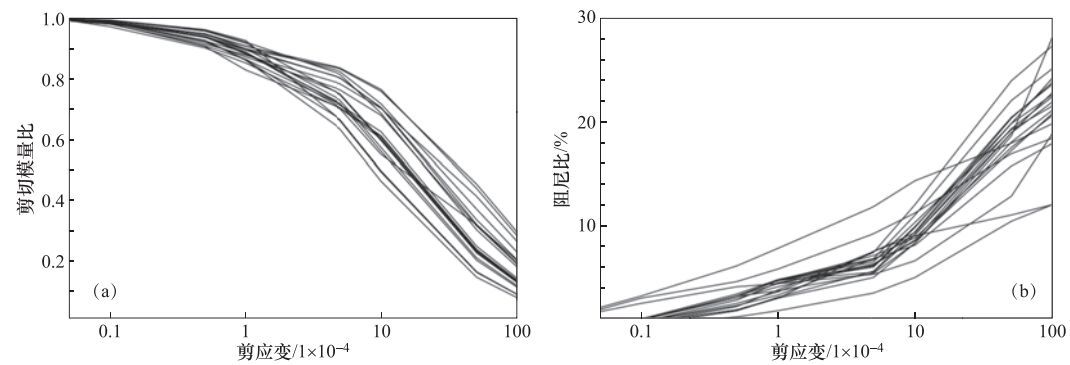
为了确定土层的动力非线性特性，对钻孔内各土层的典型样品分别进行了共振柱和动三轴实验，综合确定了土层样品的动剪切模量比（G/Gmax）和阻尼比（λ）随动剪应变（γ）的变化，土层样品动力非线性特征见表 1，剪切模量比和阻尼比与剪应变关系曲线如图 3所示。
表 1 土层样品动力非线性特性Table 1. The shear modulus and damping ratio vs. strain of soil samples序号 土层名称 剪切模量比与阻尼比 剪应变γ/10-4 0.05 0.1 0.5 1 5 10 50 100 1 粉质粘土 G/Gmax 0.993 0.987 0.937 0.881 0.718 0.56 0.203 0.113 λ 0.004 0.007 0.028 0.045 0.06 0.098 0.204 0.236 2 粉土 G/Gmax 0.993 0.986 0.936 0.879 0.749 0.599 0.23 0.13 λ 0.004 0.007 0.023 0.033 0.068 0.11 0.22 0.251 3 粉质粘土 G/Gmax 0.996 0.992 0.96 0.924 0.661 0.493 0.163 0.089 λ 0.005 0.009 0.032 0.047 0.065 0.103 0.192 0.215 4 粉质粘土 G/Gmax 0.991 0.983 0.919 0.87 0.762 0.678 0.316 0.188 λ 0.005 0.009 0.032 0.047 0.055 0.091 0.193 0.224 5 粉质粘土 G/Gmax 0.99 0.98 0.905 0.827 0.702 0.601 0.232 0.131 λ 0.005 0.009 0.032 0.047 0.061 0.099 0.198 0.226 6 粉砂 G/Gmax 0.989 0.978 0.947 0.906 0.822 0.716 0.371 0.227 λ 0.005 0.009 0.032 0.037 0.054 0.083 0.186 0.282 7 粉砂 G/Gmax 0.991 0.982 0.936 0.892 0.802 0.704 0.336 0.202 λ 0.005 0.009 0.032 0.047 0.067 0.091 0.189 0.219 8 粉质粘土 G/Gmax 0.992 0.985 0.938 0.896 0.801 0.703 0.321 0.191 λ 0.005 0.009 0.032 0.044 0.063 0.085 0.172 0.208 9 粉质粘土 G/Gmax 0.992 0.984 0.923 0.884 0.703 0.611 0.239 0.136 λ 0.005 0.009 0.032 0.047 0.071 0.088 0.175 0.206 10 粉质粘土 G/Gmax 0.993 0.986 0.935 0.886 0.713 0.624 0.249 0.142 λ 0.002 0.005 0.022 0.038 0.075 0.093 0.198 0.242 11 粉质粘土 G/Gmax 0.99 0.98 0.907 0.862 0.712 0.607 0.236 0.134 λ 0.021 0.032 0.061 0.078 0.118 0.143 0.179 0.198 12 粉质粘土 G/Gmax 0.992 0.984 0.924 0.859 0.633 0.463 0.147 0.079 λ 0.019 0.03 0.046 0.058 0.092 0.112 0.169 0.184 13 粉质粘土 G/Gmax 0.996 0.991 0.958 0.919 0.813 0.685 0.303 0.179 λ 0.002 0.004 0.018 0.03 0.05 0.083 0.18 0.211 14 粉质粘土 G/Gmax 0.989 0.978 0.913 0.876 0.782 0.701 0.413 0.262 λ 0.017 0.025 0.041 0.044 0.053 0.066 0.128 0.189 15 粉砂 G/Gmax 0.992 0.984 0.925 0.861 0.664 0.497 0.165 0.09 λ 0.004 0.007 0.025 0.036 0.074 0.12 0.239 0.273 16 粉质粘土 G/Gmax 0.994 0.989 0.946 0.897 0.728 0.572 0.211 0.118 λ 0.005 0.009 0.03 0.041 0.062 0.086 0.189 0.228 17 粉质粘土 G/Gmax 0.993 0.986 0.943 0.905 0.834 0.763 0.439 0.281 λ 0.005 0.01 0.034 0.048 0.068 0.081 0.157 0.179 18 粉质粘土 G/Gmax 0.995 0.991 0.955 0.915 0.746 0.595 0.227 0.128 λ 0.002 0.004 0.018 0.031 0.056 0.094 0.203 0.238 19 粉细砂 G/Gmax 0.992 0.985 0.934 0.897 0.831 0.757 0.453 0.293 λ 0.001 0.003 0.012 0.018 0.035 0.05 0.104 0.12 
图 3 剪切模量-剪应变关系（a）和阻尼比-剪应变关系（b）Figure 3. Plot of shear modulus vs. strain (a) and plot of damping ratio vs. strain (b)图 3给出了表 1中土样动力非线性特征测量值的拟合曲线，由图 3（a）可知，随着剪应变的增大，剪切模量比逐渐减小。由图 3（b）可知，随着剪应变的增大，阻尼比逐渐增大。需要注意的是，由于工程场地内采集的样品进行了动三轴和共振柱实验，剪应变的变化范围较大，使得阻尼比的最大值可达20%以上。
2. 随机振动理论方法（RVT）
Schneider等于1991年首次提出了采用随机振动理论方法进行场地响应分析的思路，RVT方法的输入为傅立叶幅值谱（FAS）和持时（Tgm），不需要地震动时程，可以通过一次分析就给出场地响应的分布特征（Schneider等，1991）。Silva等（1996）将RVT方法应用于核设施土层地震反应分析中，研究了土层动力特性曲线不确定性的表征方式。Rathje等（2006）验证了RVT方法对美国东部地区的适用性，Ozbey（2006）进行了RVT方法和传统时程方法的对比研究。
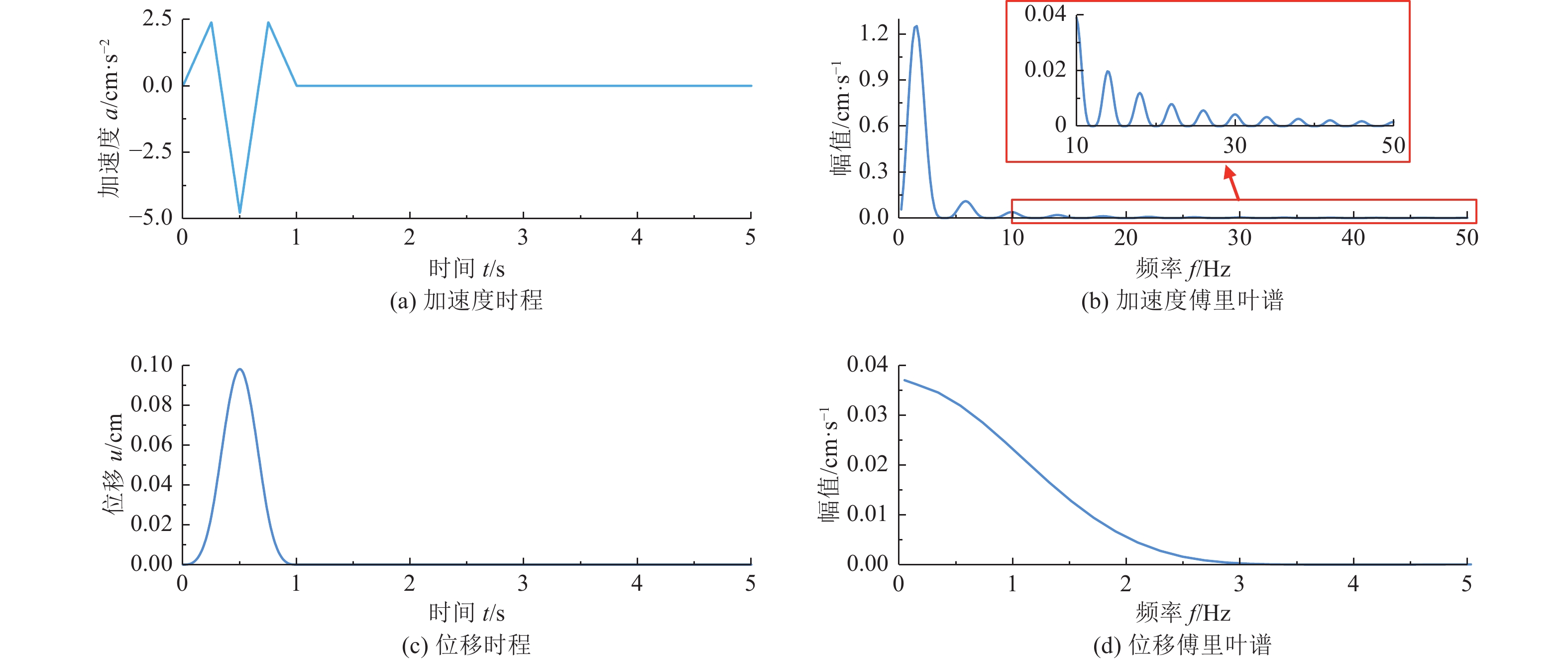
RVT方法中地震动输入的FAS可以根据地震学的震源理论给出，也可以通过目标反应谱反算来确定（Brune，1970；Gasparini等，1976）。Tgm的定义可以采用震源理论计算或经验模型，Boore（2003）给出了适用于美国西部的经验公式，Boore等（2015）给出了适用于稳定大陆区域的地壳放大模型和持时模型。本文采用反应谱-幅值谱的相互关系，计算幅值谱，作为基岩地震动输入，进行土层地震反应分析。
3. 不确定性影响分析
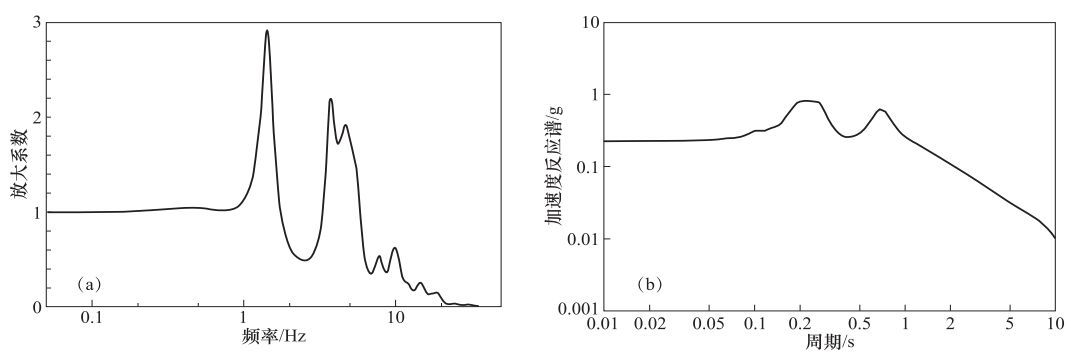
为了分析土层模型中动力特性、剪切波速、基岩地震动输入界面位置对分析结果的影响，将变量分离，研究单个变量对评价结果的影响。首先，固定所有参数，以第2章中给出的基岩地震动和zk1的土层剖面模型作为输入，采用RVT方法进行土层地震反应分析，钻孔剖面模型zk1的加速度传递函数和土层地表加速度反应谱如图 4（a）和（b）所示。
图 4 固定参数模型条件下钻孔zk1的加速度传递函数（a）和土层地表加速度反应谱（b）Figure 4. Acceleration transfer function (a) and soil surface acceleration spectra (b) of zk1由图 4（a）可知，zk1的土层剖面模型改变了地震动的频谱特征，1.4Hz和3.8Hz左右的放大效应非常明显，尤其是1.4Hz处，幅值被放大了接近3倍；高于6Hz部分的幅值降低明显，低于1.3Hz的频段内的幅值基本没有变化，说明zk1剖面模型的特征周期在0.7s左右，0.26s附近频谱的放大主要是近地表砂土层的影响。
由图 4（b）可知，土层地表加速度反应谱在2个周期段内数值较高，一个是0.19—0.27s，表现为较宽的平台段，一个是0.68s附近，呈尖峰状，二者之间则是一个显著的“谷地”。加速度反应谱的特征与加速度传递函数的特征相似，都说明了zk1所代表的土层模型对基岩地震动在2个频段内有明显的放大作用。
3.1 剪切波速
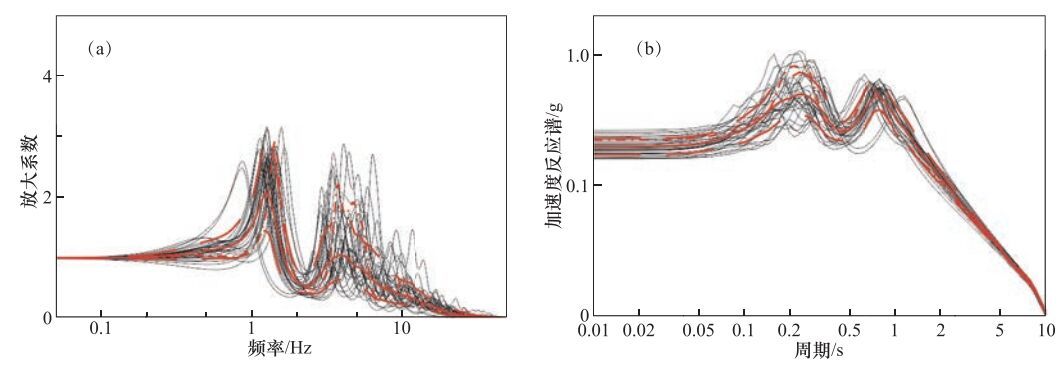
以zk1的剪切波速剖面为均值，采用Robinson等（2006）提出的土层剪切波速不确定性分布作为前提假设，随机生成30个土层模型，采用RVT方法进行土层地震反应分析。钻孔zk1的加速度传递函数和土层地表地震动反应谱如图 5（a）和（b）所示。
图 5 剪切波速随机变化条件下zk1的加速度传递函数（a）和地表加速度反应谱（b）Figure 5. Acceleration transfer function (a) and soil surface acceleration spectrum (b) of zk1 through randomly variationon shear wave velocity图 5（a）中的灰色曲线为每个随机模型的计算结果，黑色实线为所有结果的中值，黑色虚线为所有传递函数曲线的中值加减1倍标准差。将随机模型计算结果的幅值放大系数中值和中值加1倍标准差与剪切波速不变前提下的放大系数进行对比可知，二者的整体趋势一致，基岩上覆土层对低频部分基本没有影响，明显地放大了特征周期频段附近的幅值，降低了高频部分的幅值。不同的是，随机模型的中值在1.6Hz之后迅速降低了基岩地震动的傅立叶幅值谱，没有反映出近地表砂土对基岩地震动的影响。传递函数中值加1倍标准差的结果反映出了2个明显的放大频段，与固定参数模型的传递函数相比，2个放大频段之间的放大系数虽然也相对较小，但是其绝对值大于1，放大频段的上限也扩展到8Hz左右。
图 5（b）中的灰色曲线为每个随机模型的计算结果，黑色实线为所有结果的中值，黑色虚线为中值加减1倍标准差，黑色点虚线为固定参数模型给出的土层地表加速度反应谱。与固定参数模型分析结果相比，剪切波速随机变化模型给出的反应谱平台段较宽，除了放大效应明显的顶峰频段以外，中值加减1倍标准差反应谱基本能够包络固定参数模型分析给出的结果。
3.2 土层动力特性曲线
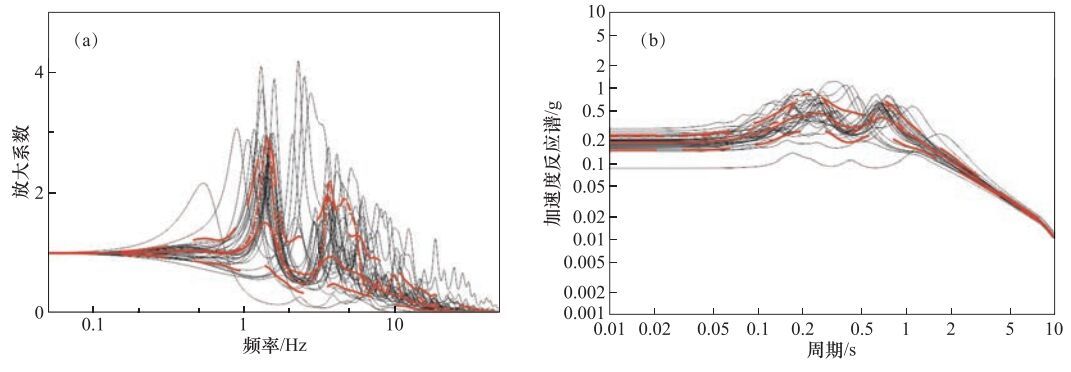
固定其他参数不变，以图 3给出的剪切模量比-剪应变和阻尼比-剪应变关系曲线作为中值，采用Silva等（1996）提出的土层动力特性曲线不确定性分布作为前提假设，随机生成30个土层剖面模型，采用RVT方法进行土层地震反应分析。加速度传递函数和土层地表加速度反应谱如图 6所示。
图 6 随机生成土层动力特性曲线条件下的加速度传递函数（a）和土层地表加速度反应谱（b）Figure 6. Acceleration transfer function (a) and soil surface acceleration spectrum (b) of zk1 through randomly variation on soil dynamic character图 6（a）中的黑色曲线为土层动力特性曲线随机变化模型的加速度传递函数，红色实线为传递函数的中值，红色虚线为中值加减1倍标准差，红色点虚线为固定参数模型的传递函数。从图中可以看出，土层动力特性曲线随机变化模型的统计结果可以反映土层模型对基岩地震动的影响，放大频段特征与固定参数的结果相似，曲线形状非常相似。固定参数模型的传递函数与参数随机变化模型相比，除了放大频段以外，与两者的中值相近；1.4Hz附近明显高于中值，略低于中值加1倍标准差；3.8—4.8Hz范围内介于中值和中值加1倍标准差之间。
图 6（b）中的黑色曲线为每个土层动力特性曲线随机变化模型的土层地表加速度反应谱，红色实线为反应谱的中值，红色虚线为中值加减1倍标准差，红色点虚线为固定参数模型的土层地表加速度反应谱。从图中可以看出，随机模型的统计结果可以反映土层地表加速度反应谱的形状特征，同样都是在0.18—0.28s范围内表现为明显的平台段，在0.70s附近表现为明显的尖峰。数值上固定参数的反应谱在周期低于0.36s时介于中值和中值加1倍标准差之间，高于0.36s时，固定参数的反应谱基本上与随机模型反应谱的中值一致。
3.3 基岩地震动输入界面
本小节的思路与前2小节相同，随机模拟基岩地震动输入界面的位置，即固定土层波速结构模型，令输入界面的深度在给定范围内均匀分布。加速度传递函数和土层地表加速度反应谱的对比结果如图 7所示。
图 7 基岩地震动输入界面深度随机变化条件下的加速度传递函数（a）和土层地表加速度反应谱（b）Figure 7. Acceleration transfer function (a) and soil surface spectrum (b) of zk1 under randomly variation on depth of hard rock surface图 7中的黑色曲线为基岩地震动输入界面深度随机变化模型的加速度传递函数和土层地表加速度反应谱，红色实线为计算结果的中值，红色虚线为中值加减1倍标准差，红色点虚线为固定参数模型的传递函数。从加速度传递函数图中的对比情况可知，固定参数模型的传递函数与地震动输入界面深度随机变化模型传递函数统计结果的形状相似，在1.5Hz附近和3.4—5.4Hz之间高于随机模型传递函数统计结果的中值加1倍标准差。
通过对比土层地表加速度反应谱可知，固定参数模型的土层地表加速度反应谱与地震动输入界面深度随机变化模型反应谱统计结果的形状相似，自振周期低0.34s时，前者介于后者的中值和中值加1倍标准差之间，高于0.34s时，前者基本与后者的中值相一致。
3.4 综合影响
综合土层剖面模型的不确定性，即参数随机变化模型中剪切波速、动力特性、基岩界面位置同时变化，土层地震反应分析的结果如图 8所示。
图 8 土层剖面随机模型与固定参数模型加速度传递函数（a）和地表加速度反应谱对比图（b）Figure 8. Acceleration transfer function (a) and soil surface acceleration spectrum (b) of zk1 through randomly variation on shear wave velocity, soil dynamic character and depth of hard rock surface图 8中的黑色曲线为剪切波速、动力特性、基岩界面位置同时随机变化的加速度传递函数和反应谱，红色实线为计算结果的中值，红色虚线为中值加减1倍标准差，红色点虚线为固定参数模型的传递函数和反应谱。从图 8（a）中可以看出，参数随机变化模型分析结果的中值加1倍标准差可以反映场地对基岩地震动的影响，在1.4Hz附近和3.6Hz附近明显放大了地震动幅值，放大倍数与固定参数模型分析结果相一致。其余频段中，固定模型放大倍数与随机模型分析结果的中值基本一致。
通过图 8（b）可知，参数随机变化模型分析结果统计特征的平台段较宽，中值加1倍标准差可以包络固定参数模型的分析结果。
4. 结论
本文介绍了随机振动理论方法在核设施一维土层地震反应分析工作中的应用，基于某核电厂工程场地实测数据和土层剖面模型参数的不确定性分布，随机生成土层模型，采用随机振动理论方法分析了剪切波速、土层动力特性和基岩地震动输入界面位置的不确定性对核设施一维土层地震反应分析结果的影响。
加速度传递函数结果表明，与固定参数模型相比，采用土层参数随机变化模型和随机振动理论方法可以反映工程场地对地震动的影响。
土层地表加速度反应谱结果表明，土层剖面模型的不确定性中，剪切波速的不确定性对评价结果影响最大，使得加速度反应谱的平台段较宽，随机模型评价结果的中值加1倍标准差，除尖峰位置外，可以包络固定参数模型的评价结果。
土层动力特性曲线和基岩地震动输入界面位置的不确定性对评价结果的影响相当，固定参数模型的分析结果介于土层参数随机变化模型分析结果的中值和中值加1倍标准差之间。
-
图 3 逐元并行FEM地震波场模拟流程图
Figure 3. Flow chart showing the processes of seismic wave modeling using element-by-element parallel FEM
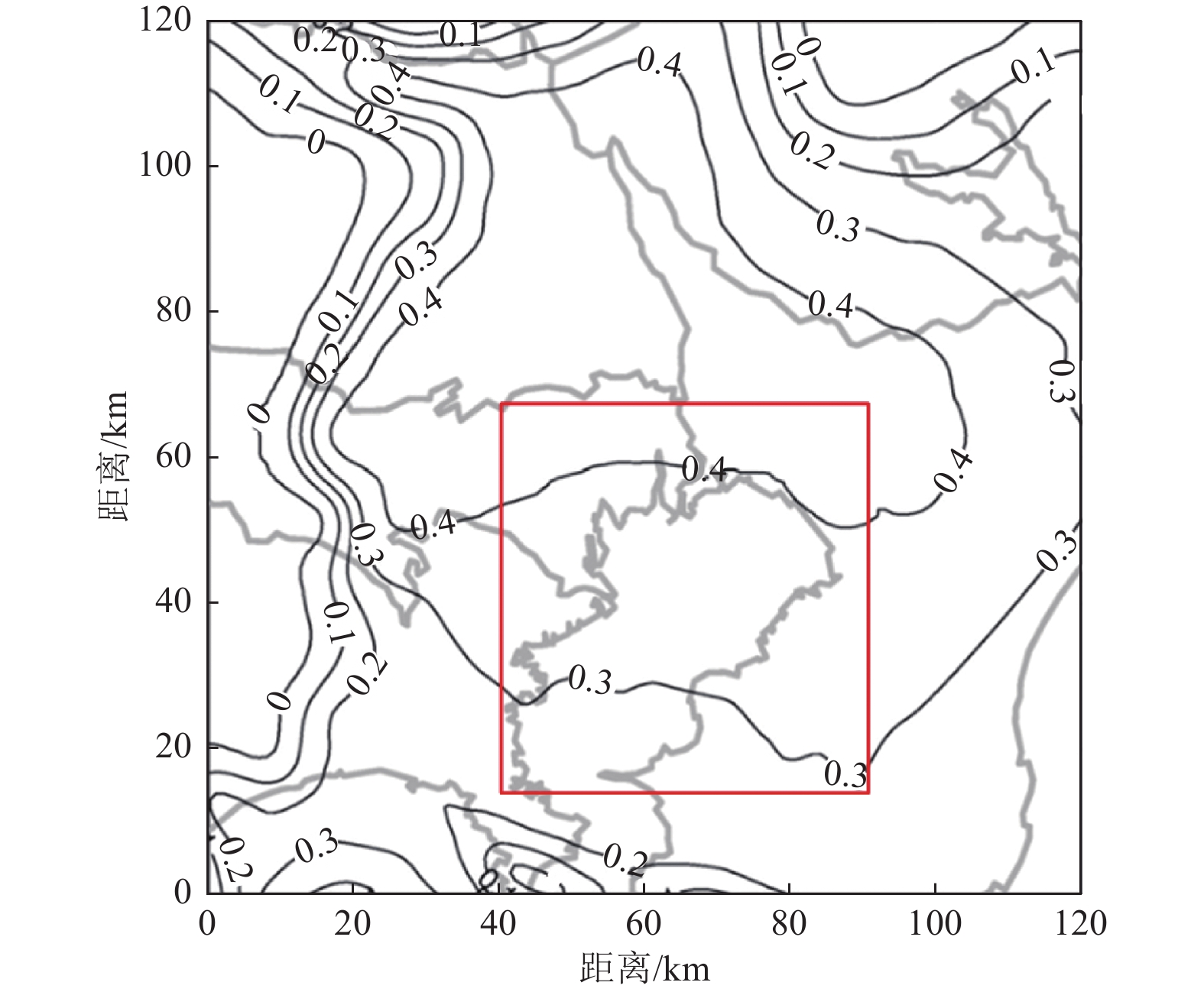
图 5 Koketsu等（2009）确定的第一界面深度
Figure 5. The first interface depth determined by Koketsu et al.（2009）
图 7 观测点的位移、加速度时程曲线和加速度傅里叶谱
Figure 7. Displacement, acceleration time history curve and acceleration Fourier spectrum of observation points
图 9 x方向位移的波场快照
Figure 9. Snapshot of the wavefield with displacement in the x-direction
表 1 东京湾材料参数（Koketsu 等, 2009）
Table 1. Parameters of materials used in Tokyo bay （Koketsu et al., 2009）
材料 孔隙率/β μ0 ρs /kg·m−3 Ρw/kg·m−3 ν G /GPa Ew /GPa M /GPa α k0/μm2 海水 1 0 0 1000 0.020 0 2.25 2.25 1 1 基岩1 0 0 1850 0 0.437 0.666 0 — 0 0 基岩2 0 0 2080 0 0.395 2.080 0 — 0 0 
下载: 导出CSV
-
陈少林, 柯小飞, 张洪翔, 2019 a. 海洋地震工程流固耦合问题统一计算框架. 力学学报, 51（2）: 594—606Chen S. L. , Ke X. F. , Zhang H. X. , 2019 a. A unified computational framework for fluid-solid coupling in marine earthquake engineering. Chinese Journal of Theoretical and Applied Mechanics, 51(2): 594—606. (in Chinese) 陈少林, 程书林, 柯小飞, 2019 b. 海洋地震工程流固耦合问题统一计算框架——不规则界面情形. 力学学报, 51（5）: 1517—1529Chen S. L. , Cheng S. L. , Ke X. F. , 2019 b. A unified computational framework for fluid-solid coupling in marine earthquake engineering: irregular interface case. Chinese Journal of Theoretical and Applied Mechanics, 51(5): 1517—1529. (in Chinese) 杜修力, 2009. 工程波动理论与方法. 北京: 科学出版社, 215—216Du X. L. , 2009. Theories and methods of wave motion for engineering. Beijing: China Science Press, 215—216. (in Chinese) 柯小飞, 陈少林, 张洪翔, 2019. P-SV波入射时海水-层状海床体系的自由场分析. 振动工程学报, 32（6）: 966—976Ke X. F. , Chen S. L. , Zhang H. X. , 2019. Free-field analysis of seawater-seabed system for incident plane P-SV waves. Journal of Vibration Engineering, 32(6): 966—976. (in Chinese) 李小军, 2006. 海域工程场地地震安全性评价的特殊问题. 震灾防御技术, 1（2）: 97—104Li X. J. , 2006. Special problems on evaluation of seismic safety for offshore engineering site. Technology for Earthquake Disaster Prevention, 1(2): 97—104. (in Chinese) 李小军, 陈苏, 任治坤等, 2020. 海域地震区划关键技术研究项目及研究进展. 地震科学进展, 50（1）: 2—19Li X. J. , Chen S. , Ren Z. K. , et al. , 2020. Project plan and research progress on key technologies of seismic zoning in sea areas. Progress in Earthquake Sciences, 50(1): 2—19. (in Chinese) 王笃国, 尤红兵, 张合等, 2021. 海域不同类别场地地震动参数变化规律研究. 震灾防御技术, 16（1）: 116—122Wang D. G. , You H. B. , Zhang H. , et al. , 2021. Study on the change of earthquake ground motion parameters for different classification sites of ocean areas. Technology for Earthquake Disaster Prevention, 16(1): 116—122. (in Chinese) 朱镜清, 1988. 地震作用下海水与海床土的耦合运动. 地震工程与工程振动, 8（2）: 37—43Zhu J. Q. , 1988. Coupled motion between sea water and sea bed-soil under earthquake action. Earthquake Engineering and Engineering Vibration, 8(2): 37—43. (in Chinese) Bao X. , Liu J. B. , Chen S. , et al. , 2022. Seismic analysis of the reef-seawater system: comparison between 3 D and 2 D models. Journal of Earthquake Engineering, 26(6): 3109—3122. doi: 10.1080/13632469.2020.1785976 Biot M. A. , 1956. Theory of propagation of elastic waves in a fluid-saturated porous solid. I. low-frequency range. The Journal of the Acoustical Society of America, 28(2): 168—191. Biot M. A. , 1962. Mechanics of deformation and acoustic propagation in porous media. Journal of Applied Physics, 33(4): 1482—1498. doi: 10.1063/1.1728759 Brekhovskikh L. M., 1980. Waves in layered media. 2 nd ed. New York: Academic Press. Deresiewicz H. , Rice J. T. , 1964. The effect of boundaries on wave propagation in a liquid-filled porous solid: V. Transmission across a plane interface. Bulletin of the Seismological Society of America, 54(1): 409—416. doi: 10.1785/BSSA0540010409 Dhakal Y. P., Aoi S., Kunugi T., et al., 2017. Assessment of nonlinear site response at ocean bottom seismograph sites based on S-wave horizontal-to-vertical spectral ratios: a study at the Sagami Bay area K-NET sites in Japan. Earth, Planets and Space, 69(1): 29. Hu J. J. , Tan J. Y. , Zhao J. X. , 2020. New GMPEs for the Sagami bay region in Japan for moderate magnitude events with emphasis on differences on site amplifications at the seafloor and land seismic stations of K-NET. Bulletin of the Seismological Society of America, 110(5): 2577—2597. doi: 10.1785/0120190305 Ichimura T. , Hori M. , Kuwamoto H. , 2007. Earthquake motion simulation with multiscale finite-element analysis on hybrid grid. Bulletin of the Seismological Society of America, 97(4): 1133—1143. doi: 10.1785/0120060175 Koketsu K., Miyake H., Afnimar, et al., 2009. A proposal for a standard procedure of modeling 3-D velocity structures and its application to the Tokyo metropolitan area, Japan. Tectonophysics, 472(1—4): 290—300. Li C. , Hao H. , Li H. N. , et al. , 2017. Modeling and simulation of spatially correlated ground motions at multiple onshore and offshore sites. Journal of Earthquake Engineering, 21(3): 359—383. doi: 10.1080/13632469.2016.1172375 Liao Z. P. , Wong H. L. , 1984. A transmitting boundary for the numerical simulation of elastic wave propagation. International Journal of Soil Dynamics and Earthquake Engineering, 3(4): 174—183. doi: 10.1016/0261-7277(84)90033-0 Link G., Kaltenbacher M., Breuer M., et al., 2009. A 2 D finite-element scheme for fluid-solid-acoustic interactions and its application to human phonation. Computer Methods in Applied Mechanics and Engineering, 198(41—44): 3321—3334. Liu S. L. , Yang D. H. , Dong X. P. , et al. , 2017. Element-by-element parallel spectral-element methods for 3-D teleseismic wave modeling. Solid Earth, 8(5): 969—986. doi: 10.5194/se-8-969-2017 Maeda T., Takemura S., Furumura T., 2017. OpenSWPC: an open-source integrated parallel simulation code for modeling seismic wave propagation in 3 D heterogeneous viscoelastic media. Earth, Planets and Space, 69(1): 102. Moczo P. , Kristek J. , Halada L. , 2000. 3 D fourth-order staggered-grid finite-difference schemes: stability and grid dispersion. Bulletin of the Seismological Society of America, 90(3): 587—603. doi: 10.1785/0119990119 Nakamura T. , Takenaka H. , Okamoto T. , et al. , 2012. FDM Simulation of seismic-wave propagation for an aftershock of the 2009 Suruga Bay earthquake: effects of ocean-bottom topography and seawater layer. Bulletin of the Seismological Society of America, 102(6): 2420—2435. doi: 10.1785/0120110356 Oba A. , Furumura T. , Maeda T. , 2020. Data assimilation‐based early forecasting of long‐period ground motions for large earthquakes along the Nankai trough. Journal of Geophysical Research: Solid Earth, 125(6): e2019 JB019047. Okamoto T. , Takenaka H. , 1999. A reflection/transmission matrix formulation for seismoacoustic scattering by an irregular fluid–solid interface. Geophysical Journal International, 139(2): 531—546. doi: 10.1046/j.1365-246x.1999.00959.x Okamoto T., Takenaka H., Nakamura T., et al., 2010. Accelerating large-scale simulation of seismic wave propagation by multi-GPUs and three-dimensional domain decomposition. Earth, Planets and Space, 62(12): 939—942. Okamoto T., Takenaka H., Nakamura T., et al., 2017. FDM simulation of earthquakes off western Kyushu, Japan, using a land-ocean unified 3 D structure model. Earth, Planets and Space, 69(1): 88. Takemura S. , Kubo H. , Tonegawa T. , et al. , 2019. Modeling of long-period ground motions in the Nankai subduction zone: model simulation using the accretionary prism derived from oceanfloor local S-wave velocity structures. Pure and Applied Geophysics, 176(2): 627—647. doi: 10.1007/s00024-018-2013-8 Takemura S. , Okuwaki R. , Kubota T. , et al. , 2020. Centroid moment tensor inversions of offshore earthquakes using a three-dimensional velocity structure model: slip distributions on the plate boundary along the Nankai trough. Geophysical Journal International, 222(2): 1109—1125. doi: 10.1093/gji/ggaa238 Takemura S., Yoshimoto K., Shiomi K., 2021. Long-period ground motion simulation using centroid moment tensor inversion solutions based on the regional three-dimensional model in the Kanto Region, Japan. Earth, Planets and Space, 73(1): 15. Wang X. , Zhan Z. W. , 2020. Moving from 1-D to 3-D velocity model: automated waveform-based earthquake moment tensor inversion in the Los Angeles region. Geophysical Journal International, 220(1): 218—234. doi: 10.1093/gji/ggz435 -
下载:
点击查看大图
计量
- 文章访问数: 308
- HTML全文浏览量: 82
- PDF下载量: 33
- 被引次数: 0
