基于单自由度等效线性化模型的RC结构地震易损性分析

耿飞; 徐超; 温增平

doi:10.11899/zzfy20220212

基于单自由度等效线性化模型的RC结构地震易损性分析

doi: 10.11899/zzfy20220212

中国地震局地球物理研究所, 北京 100081

基金项目: 国家自然基金（51378477）；中国地震局地球物理研究所基本科研业务专项（DQJB19A0133）

详细信息

作者简介:
耿飞，男，生于1986年。博士。主要从事地震工程研究。Email：gf-6325007@163.com

通讯作者:
温增平，男，生于1963年。研究员。主要从事地震工程研究。Email：wenzp@cea-igp.ac.cn

计量
- 文章访问数: 187
- HTML全文浏览量: 58
- PDF下载量: 24
- 被引次数: 0
出版历程
- 收稿日期: 2019-11-22
- 网络出版日期: 2022-08-13
- 刊出日期: 2022-06-30

Fragility Analysis of RC Frame Structures Based on Equivalent Linearization Method of SDOF System

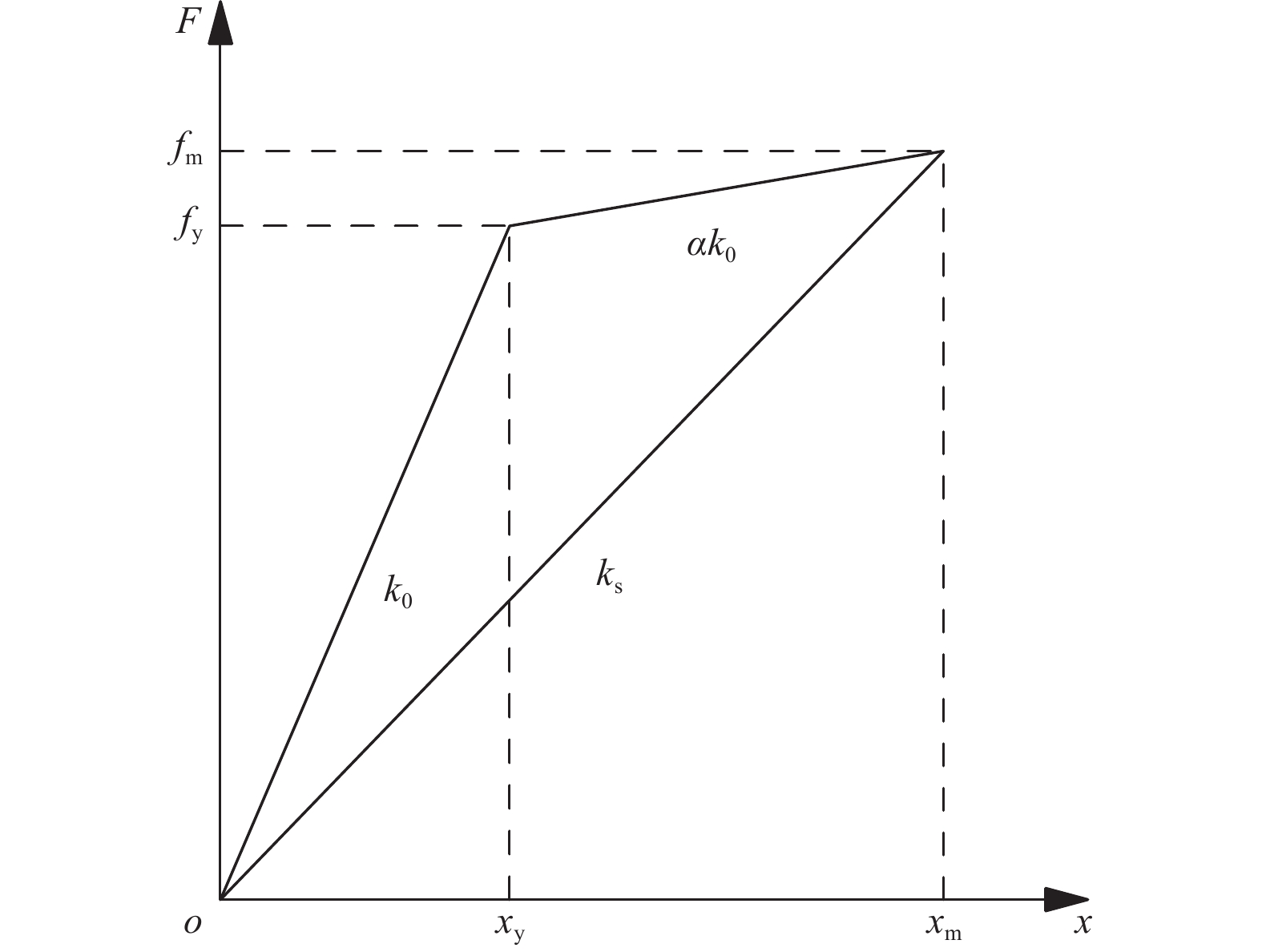
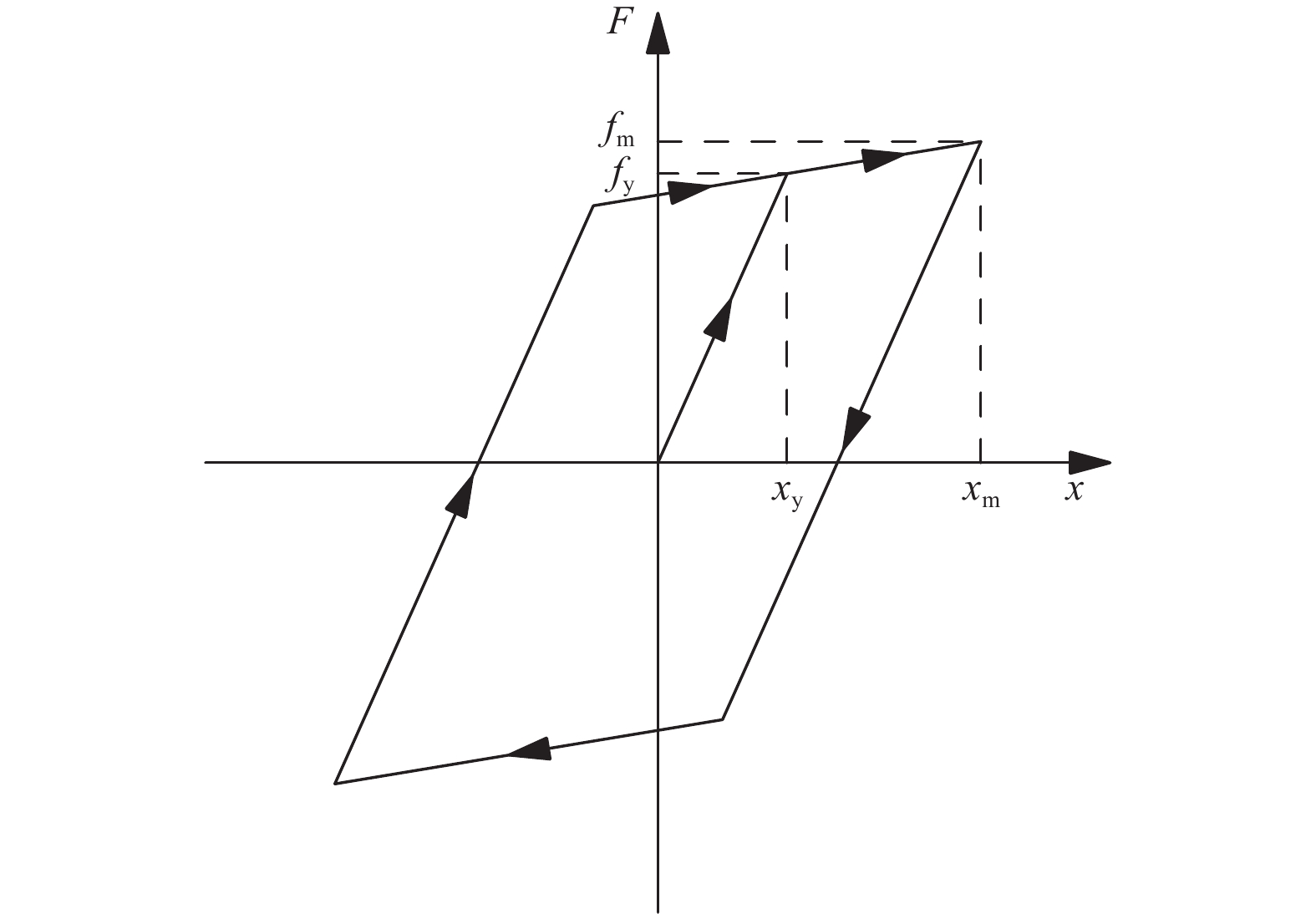
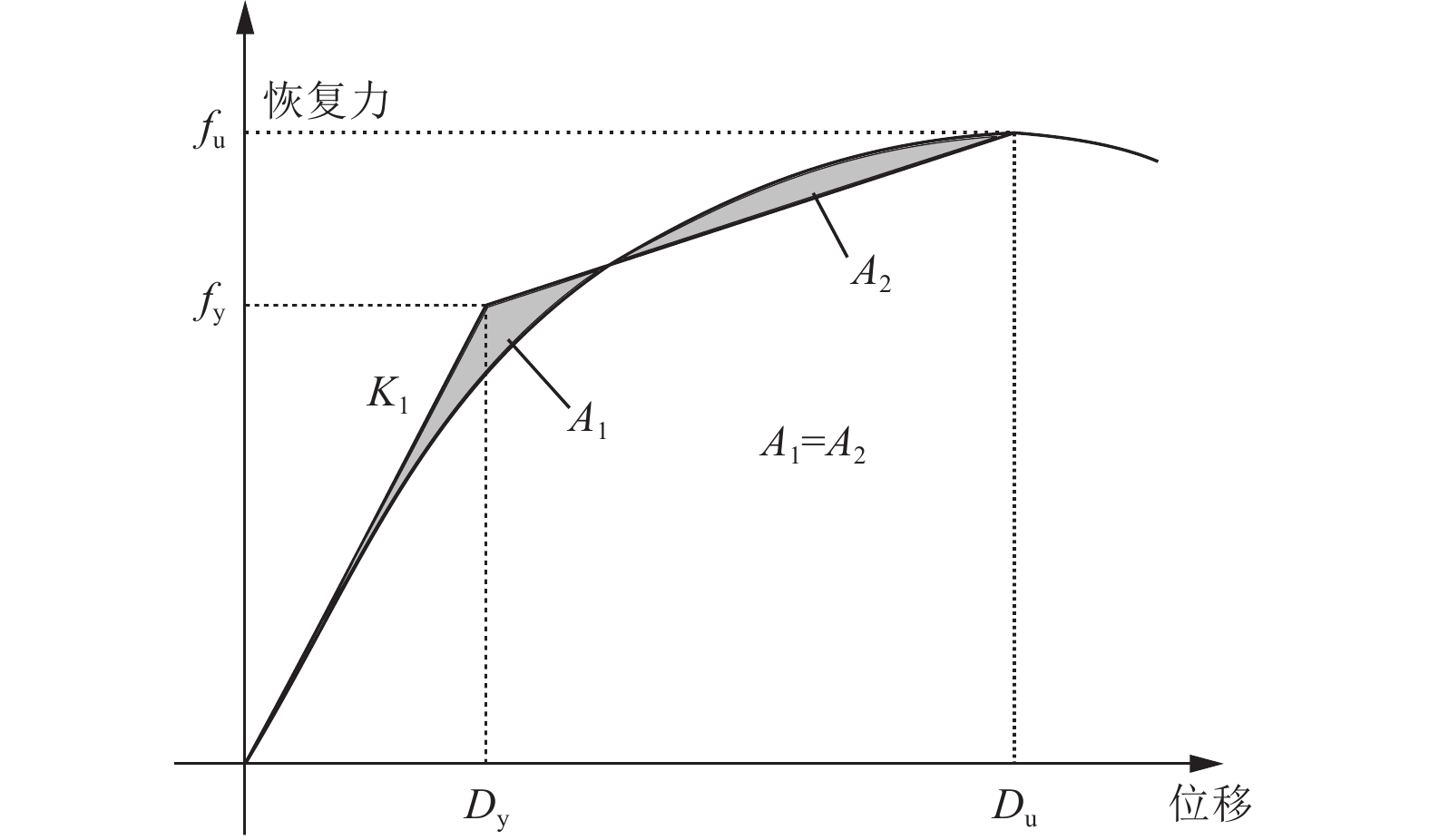
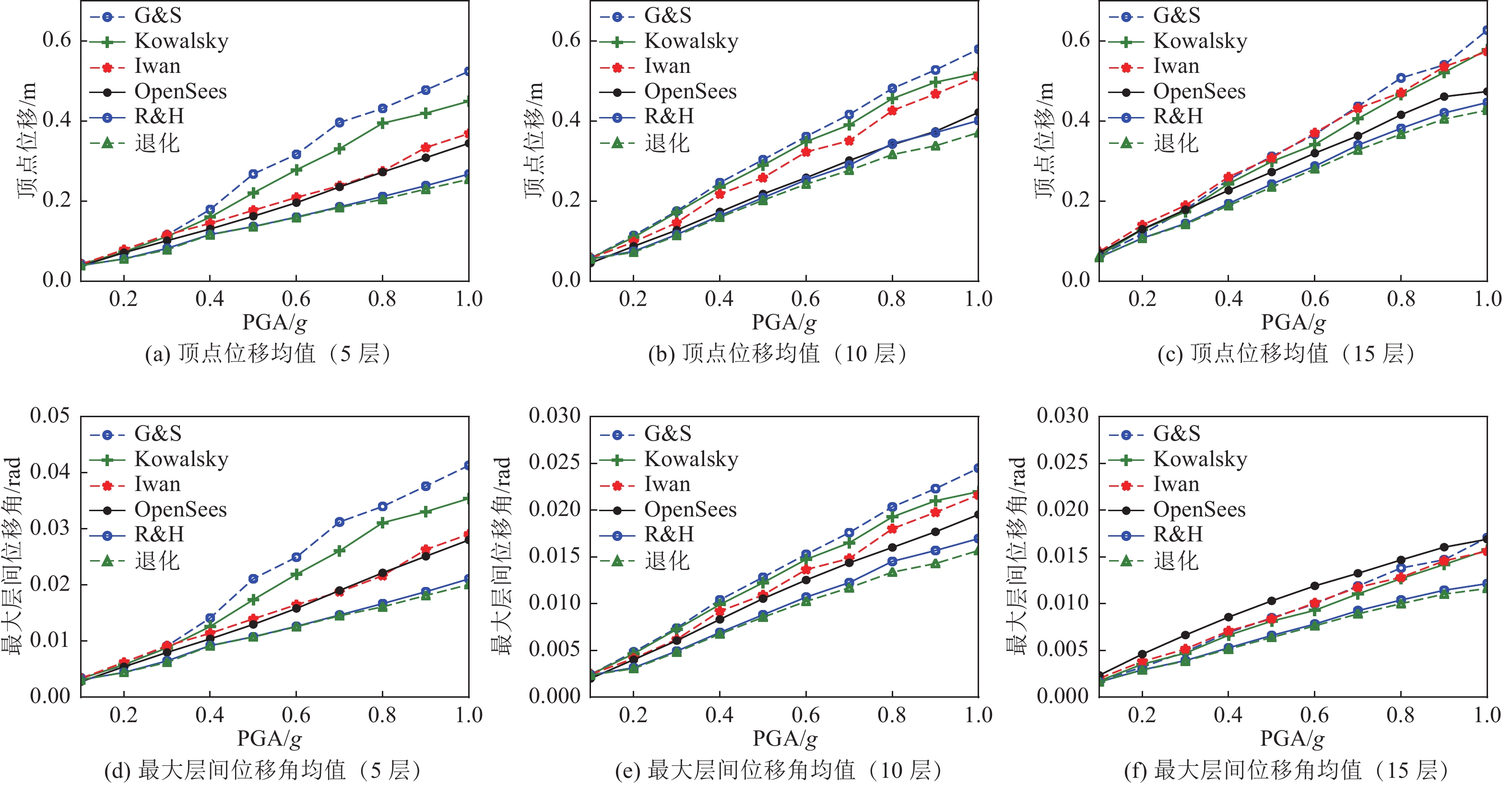
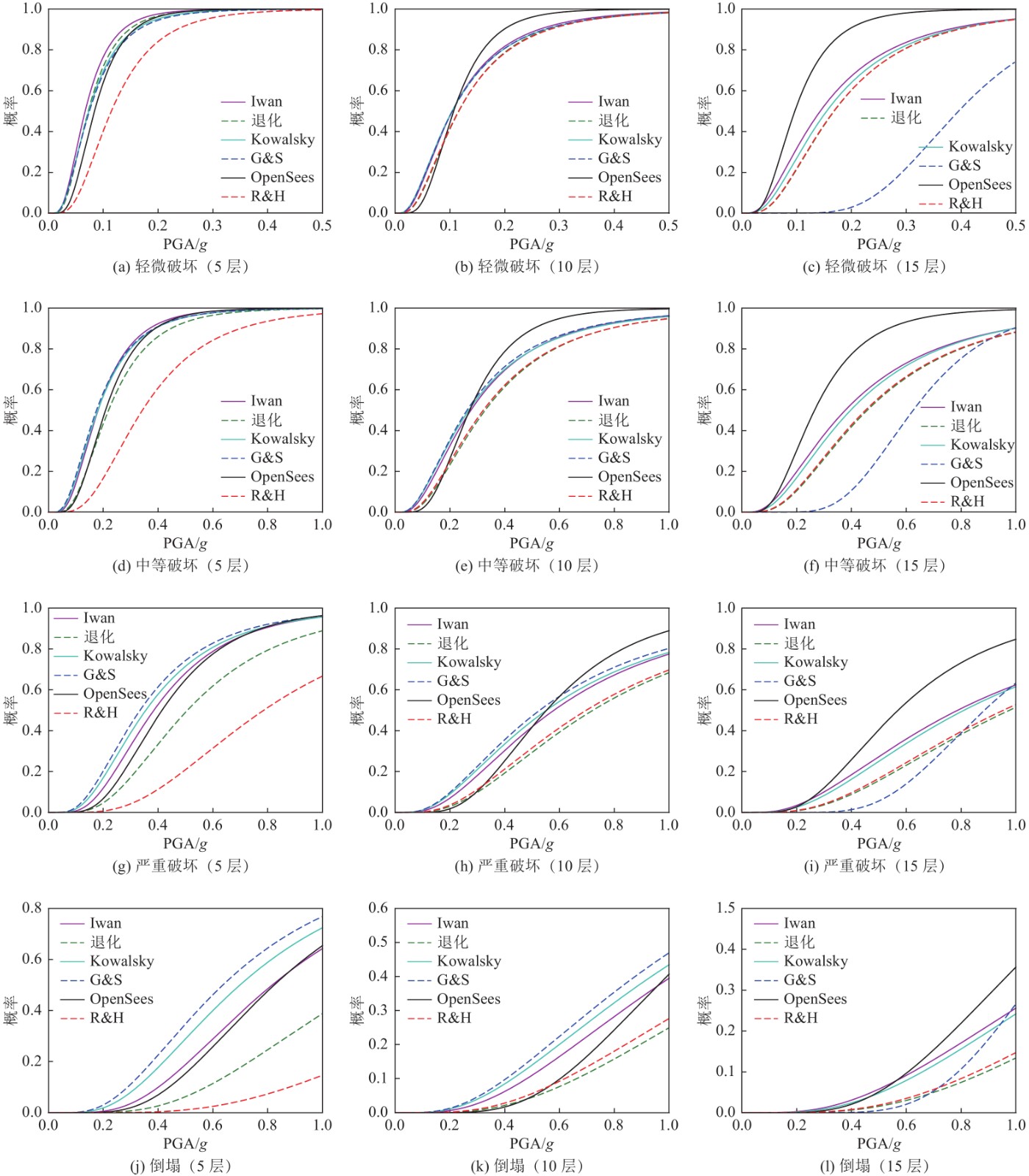
Institute of Geophysics, China Earthquake Administration, Beijing 100081, China

摘要

摘要: 通过有限元方法进行非线性动力时程分析获取解析的易损性曲线，计算量大且耗时。本文采用一种简化的计算方法，即基于单自由度的等效线性化模型，对钢筋混凝土框架结构进行地震易损性分析，并研究了该方法在结构高度上的适用性。通过选用5种典型的等效线性化模型对3栋不同高度的钢筋混凝土框架结构进行增量动力分析（IDA），得到了不同高度的结构在不同强度地震作用下结构的反应和易损性，并与OpenSees程序的计算结果进行对比，研究了等效线性化模型应用于RC框架结构易损性分析在高度上的适用性。分析结果表明：对于10层及以下的框架结构，基于单自由度的等效线性化模型在结构地震易损性分析中具有较好的适用性；对于更高层数的结构，由于高阶振型反应对整体结构反应的影响增大，基于单自由度等效线性化模型的易损性分析结果会出现明显的偏差。
- 钢筋混凝土 /
- 单自由度 /
- 等效线性化 /
- IDA /
- 易损性
Abstract: Obtaining the analytical fragility curves through nonlinear dynamic time history analysis of FEA model is computationally intensive and time-consuming. In this paper, we used a simplified calculation method, namely the equivalent linearization method based on single degree of freedom, to analyze the seismic vulnerability of reinforced concrete frame structures and the applicability of this method for structures with different heights. The incremental dynamic analysis (IDA) for three reinforced concrete frame structures with different heights was carried out by using five typical equivalent linearization methods. The response and vulnerability of structures with different heights under different earthquakes were obtained. Compared with the results of OpenSees program, the applicability of the equivalent linearization method to the vulnerability analysis of RC frame structure is investigated. The result shows that for RC structures with height less than 10 stories, the equivalent linearization method based on single degree of freedom has good applicability for structural seismic vulnerability prediction. For higher structures, the influence of the high-order mode response on the overall structural response increases and the vulnerability analysis result based on the single-degree-of-freedom equivalent linearization method shows a significant deviation.
- RC frame structure /
- Equivalent linearization /
- Single degree of freedom /
- IDA /
- Fragility

HTML全文

图 1 单自由度系统

Figure 1. Single degree of freedom system

下载: 全尺寸图片幻灯片

图 2 割线刚度

Figure 2. Secant stiffness

下载: 全尺寸图片幻灯片

图 3 双线性滞回曲线

Figure 3. Bilinear hysteretic curve

下载: 全尺寸图片幻灯片

图 4 广义力-位移关系的双折线化

Figure 4. Bilinear model of generalized load-response curve

下载: 全尺寸图片幻灯片

图 5 结构平面及侧立面示意图

Figure 5. Structural plane and side elevation diagram

下载: 全尺寸图片幻灯片

图 6 顶点位移统计均值和最大层间位移角统计均值关系图

Figure 6. The relationship between mean values of roof displacement and mean values of inter-story drift ratio

下载: 全尺寸图片幻灯片

图 7 3栋不同高度结构的易损性曲线

Figure 7. Fragility curves of 3 structures with different heights

下载: 全尺寸图片幻灯片

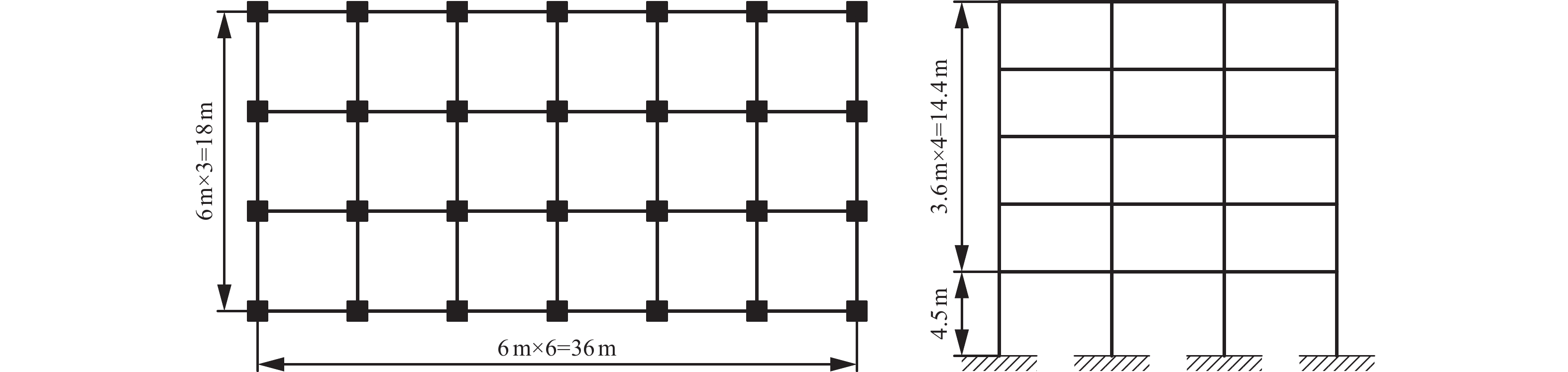
表 1 柱尺寸及配筋

Table 1. Sizes and reinforcement of columns

5层结构	1层	2层	3～5层

	500×500 mm	500×500 mm	500×500 mm
10层结构	1～3层	4～5层	6～8层	9～10层

	700×700 mm	700×700 mm	650×650 mm	650×650 mm
15层结构	1层	2～7层	8～15层

	700×700 mm	700×700 mm	650×650 mm

下载: 导出CSV

表 2 对数回归分析的参数结果

Table 2. Results of parameters from logarithmic regression analysis

层数	方法	a	b	$\beta $
5 层	R&H	0.83676	−4.0223	0.4665
	退化	0.82756	−4.0423	0.4591
	G&S	1.0745	−3.4258	0.6621
	Kowalsky	1.0226	−3.5449	0.6149
	Iwan	0.92018	−3.7250	0.5102
	OpenSees	1.0078	−3.7162	0.4922
10 层	R&H	0.89648	−4.2811	0.6235
	退化	0.88171	−4.3166	0.5990
	G&S	0.99393	−3.9680	0.7445
	Kowalsky	0.96367	−4.0314	0.7292
	Iwan	0.93816	−4.0919	0.6768
	OpenSees	0.97903	−4.0231	0.4738
15 层	R&H	0.92646	−4.5607	0.6187
	退化	0.91607	−4.5822	0.6051
	G&S	0.99907	−4.3595	0.7181
	Kowalsky	0.97238	−4.3966	0.6967
	Iwan	0.94396	−4.3783	0.7116
	OpenSees	0.90681	−4.0942	0.4959

下载: 导出CSV

表 3 结构破坏状态对应的最大层间位移角

Table 3. The maximum inter-story drift ratio corresponding to the damage states of the structure

破坏状态	轻微破坏	中等破坏	严重破坏	倒塌
层间位移角$\theta $	0.002	0.005	0.01	0.02

下载: 导出CSV

参考文献(22)

[1]	耿飞, 2013. 基于单自由度等效线性化方法的RC框架结构易损性分析. 北京: 中国地震局地球物理研究所. Geng F. , 2013. Fragility analysis of RC frame structures based on equivalent linearization method of SDOF system. Beijing: Institute of Geophysics, China Earthquake Administration. (in Chinese)
[2]	李妍, 吴斌, 欧进萍, 2005. 弹塑性结构等效线性化方法的对比研究. 工程抗震与加固改造, 27（1）: 1—6 doi: 10.3969/j.issn.1002-8412.2005.01.001 Li Y. , Wu B. , Ou J. P. , 2005. Comparison of equivalent linearization methods for inelastic structures. Earthquake Resistant Engineering and Retrofitting, 27(1): 1—6. (in Chinese) doi: 10.3969/j.issn.1002-8412.2005.01.001
[3]	李应斌, 刘伯权, 史庆轩, 2003. 结构的性能水准与评价指标. 世界地震工程, 19（2）: 132—137 doi: 10.3969/j.issn.1007-6069.2003.02.024 Li Y. B. , Liu B. Q. , Shi Q. X. , 2003. Performance levels and estimation indices of structures. World Earthquake Engineering, 19(2): 132—137. (in Chinese) doi: 10.3969/j.issn.1007-6069.2003.02.024
[4]	曲哲, 叶列平, 2010. 建筑结构弹塑性地震响应计算的等价线性化法研究. 建筑结构学报, 31（9）: 95—102 Qu Z. , Ye L. P. , 2010. Equivalent linear analysis in estimating nonlinear seismic responses of building structures. Journal of Building Structures, 31(9): 95—102. (in Chinese)
[5]	曲哲, 叶列平, 2011. 计算结构非线性地震峰值响应的等价线性化模型. 工程力学, 28（10）: 93—100 Qu Z. , Ye L. P. , 2011. An equivalent linear model to estimate maximum inelastic seismic responses of structural systems. Engineering Mechanics, 28(10): 93—100. (in Chinese)
[6]	苏亮, 王毅, 2011. 等效线性化方法中系统参数求解的优化算法. 工程力学, 28（9）: 23—29 Su L. , Wang Y. , 2011. New optimization algorithm for determining system parameters of equivalent linearization method. Engineering Mechanics, 28(9): 23—29. (in Chinese)
[7]	吴巧云, 朱宏平, 樊剑, 2012. 基于性能的钢筋混凝土框架结构地震易损性分析. 工程力学, 29（9）: 117—124 doi: 10.6052/j.issn.1000-4750.2010.12.0944 Wu Q. Y. , Zhu H. P. , Fan J. , 2012. Performance-based seismic fragility analysis of rc frame structures. Engineering Mechanics, 29(9): 117—124. (in Chinese) doi: 10.6052/j.issn.1000-4750.2010.12.0944
[8]	中华人民共和国住房和城乡建设部, 中华人民共和国国家质量监督检验检疫总局, 2010. GB 50011—2010 建筑抗震设计规范. 北京: 中国建筑工业出版社. Ministry of Housing and Urban-Rural Development of the People's Republic of China, General Administration of Quality Supervision, Inspection and Quarantine of the People's Republic of China, 2010. GB 50011—2010 Code for seismic design of buildings. Beijing: China Architecture & Building Press. (in Chinese)
[9]	中华人民共和国住房和城乡建设部, 2011. JGJ 3—2010 高层建筑混凝土结构技术规程. 北京: 中国建筑工业出版社. Ministry of Housing and Urban-Rural Development of the People's Republic of China, 2011. JGJ 3—2010 Technical specification for concrete structures of tall building. Beijing: China Architecture & Building Press. (in Chinese)
[10]	周奎, 李伟, 余金鑫, 2011. 地震易损性分析方法研究综述. 地震工程与工程振动, 31（1）: 106—113 Zhou K. , Li W. , Yu J. X. , 2011. Review of seismic fragility analysis methods. Journal of Earthquake Engineering and Engineering Vibration, 31(1): 106—113. (in Chinese)
[11]	ATC, 1996. Seismic evaluation and retrofit of concrete buildings. Redwood City, California: Applied Technology Council.
[12]	Ellingwood B. R. , Celik O. C. , Kinali K. , 2007. Fragility assessment of building structural systems in Mid-America. Earthquake Engineering & Structural Dynamics, 36(13): 1935—1952.
[13]	FEMA, 2005. FEMA 440-improvement of nonlinear static seismic analysis procedures. Washington, D. C. : Department of Homeland Security, Federal Emergency Management Agency.
[14]	Goda K. , Atkinson G. M. , 2010. Non-iterative equivalent linearization of inelastic SDOF systems for earthquakes in Japan and California. Earthquake Engineering & Structural Dynamics, 39(11): 1219—1238.
[15]	Gulkan P. , Sozen M. A. , 1974. Inelastic responses of reinforced concrete structures to earthquake motions. ACI Journal, 71(12): 604—610.
[16]	Iwan W. D. , 1980. Estimating inelastic response spectra from elastic spectra. Earthquake Engineering & Structural Dynamics, 8(4): 375—388.
[17]	Kowalsky M. J., 1994. Displacement based design: a methodology for seismic design applied to RC bridge columns. San Diego: University of California.
[18]	Lin Y. Y. , Miranda E. , 2008. Noniterative equivalent linear method for evaluation of existing structures. Journal of Structural Engineering, 134(11): 1685—1695. doi: 10.1061/(ASCE)0733-9445(2008)134:11(1685)
[19]	Miranda E. , Ruiz-García J. , 2002. Evaluation of approximate methods to estimate maximum inelastic displacement demands. Earthquake Engineering & Structural Dynamics, 31(3): 539—560.
[20]	Miranda E. , Lin Y. Y. , 2004. Non-iterative equivalent linear method for displacement-based design. In: Proceedings of the 13th World Conference on Earthquake Engineering. Vancouver, B. C. : WCEE.
[21]	Rosenblueth E. , Herrera I. , 1964. On a kind of hysteretic damping. Journal of the Engineering Mechanics Division, 90(4): 37—48. doi: 10.1061/JMCEA3.0000510
[22]	Shibata A. , Sozen M. A. , 1976. Substitute-structure method for seismic design in R/C. Journal of the Structural Division, 102(1): 1—18. doi: 10.1061/JSDEAG.0004250