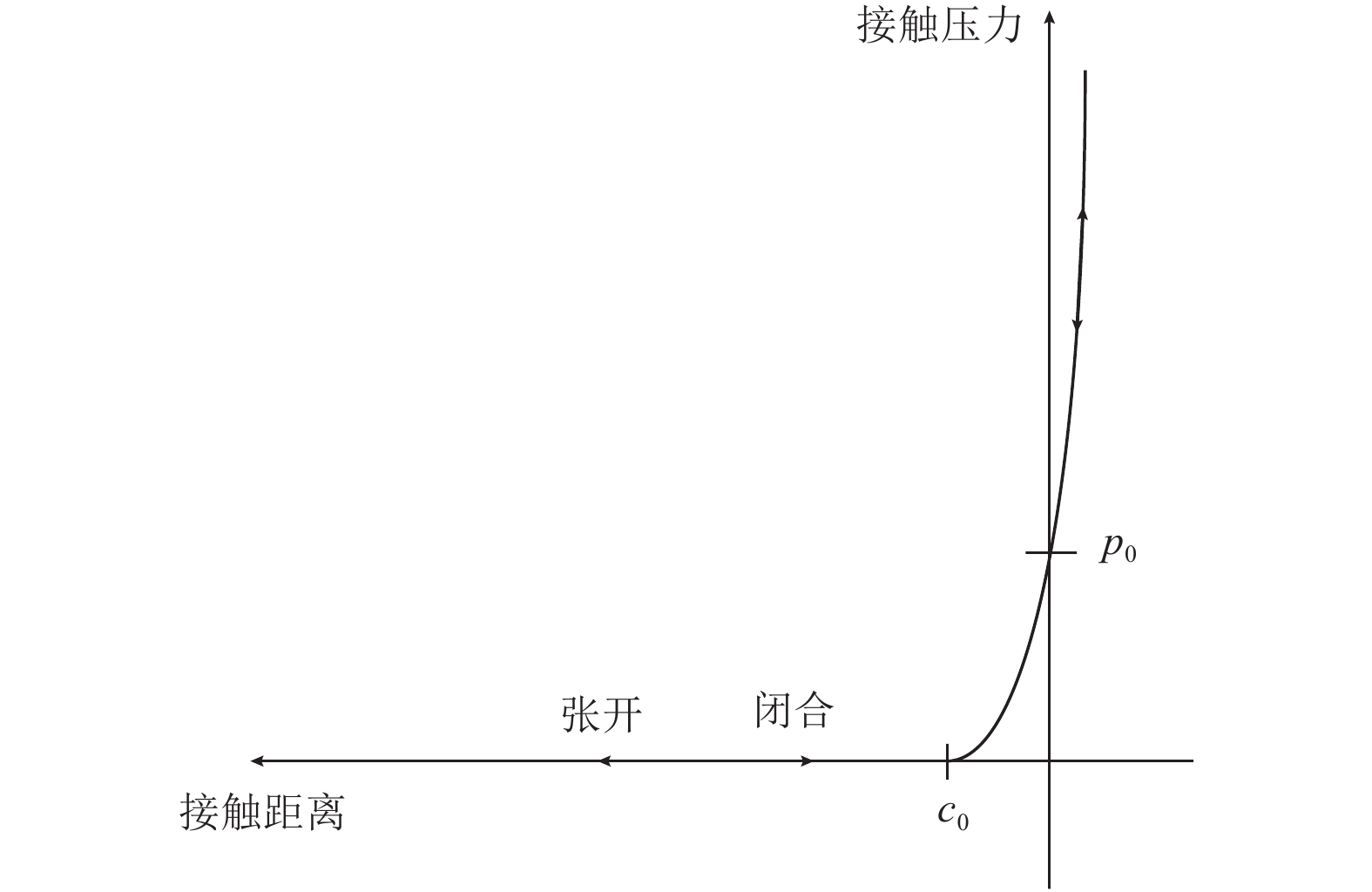
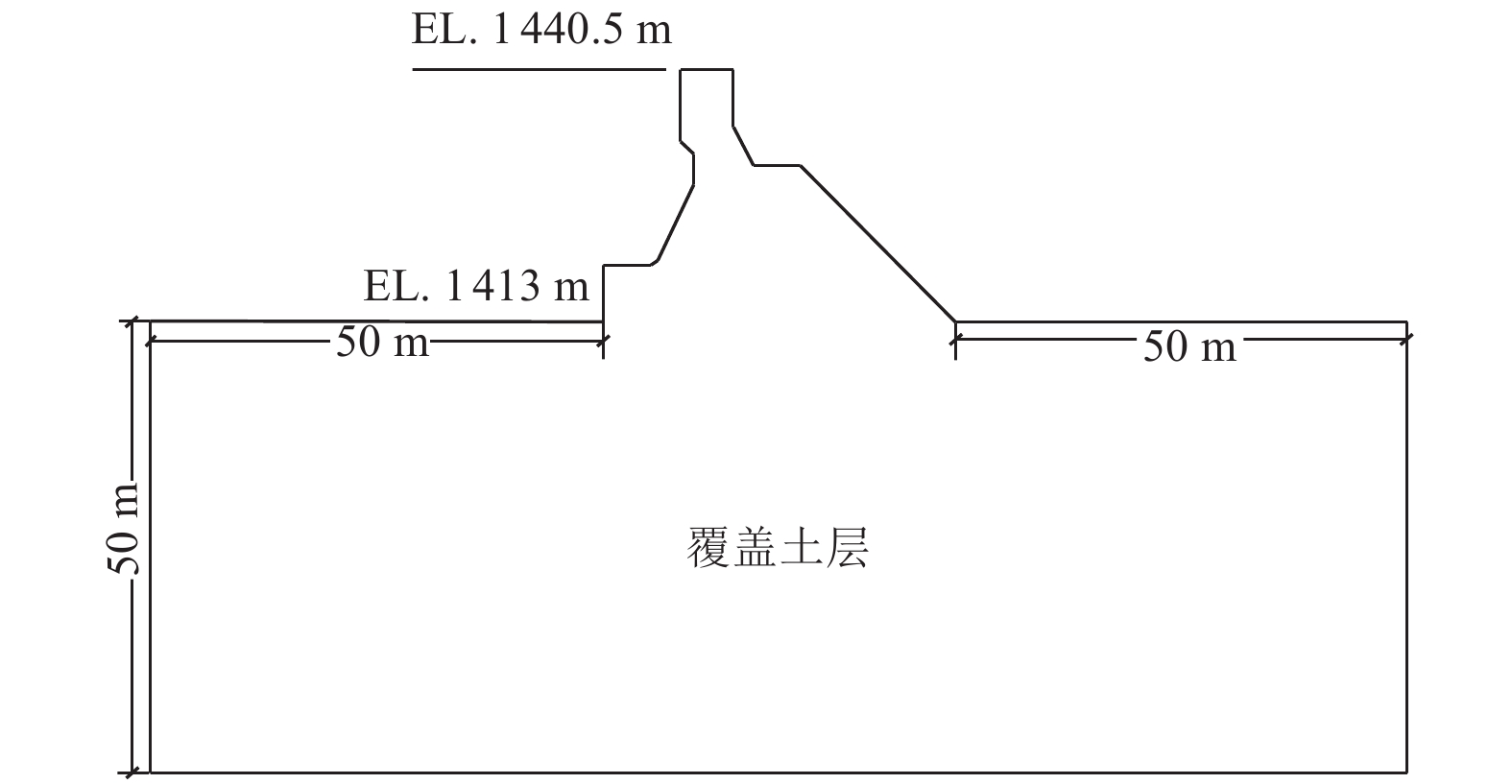
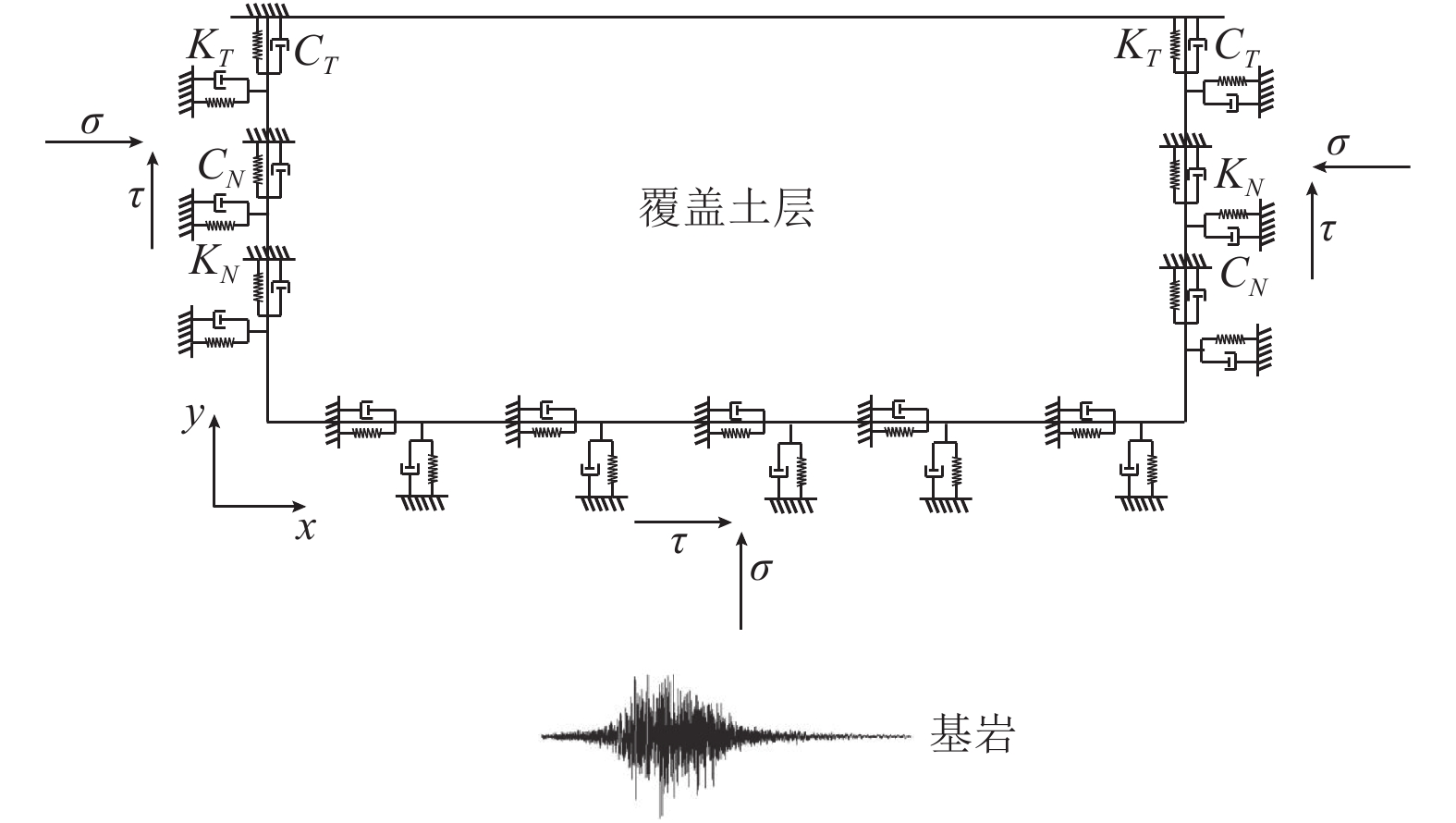
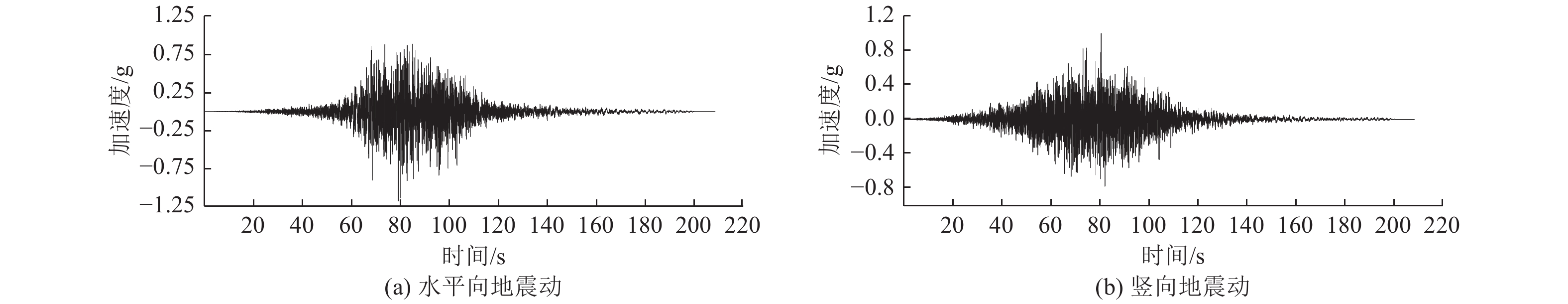
Seismic Safety Evaluation of a Gravity Dam in Deep Overburden Site Under Super Strong Earthquakes
-
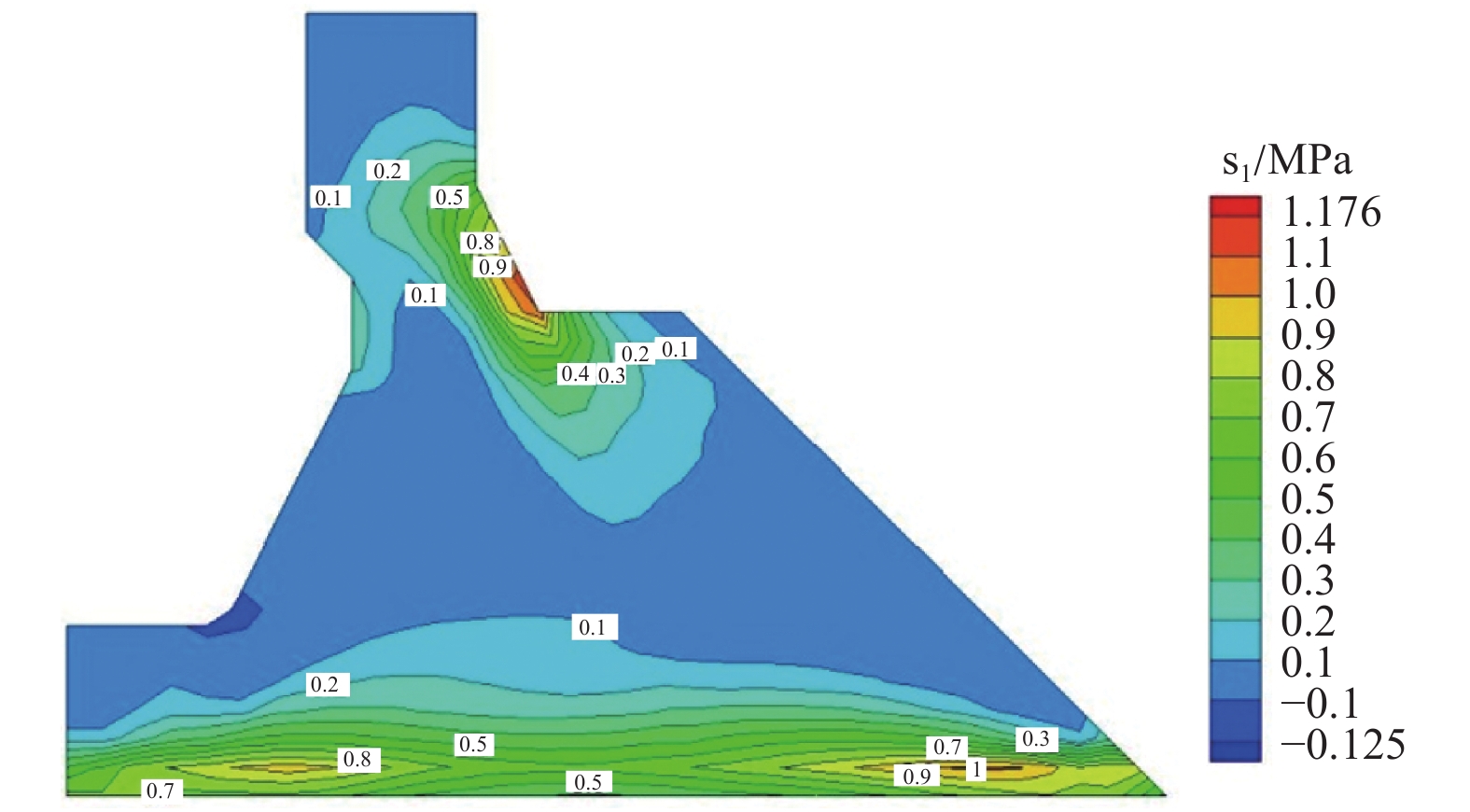
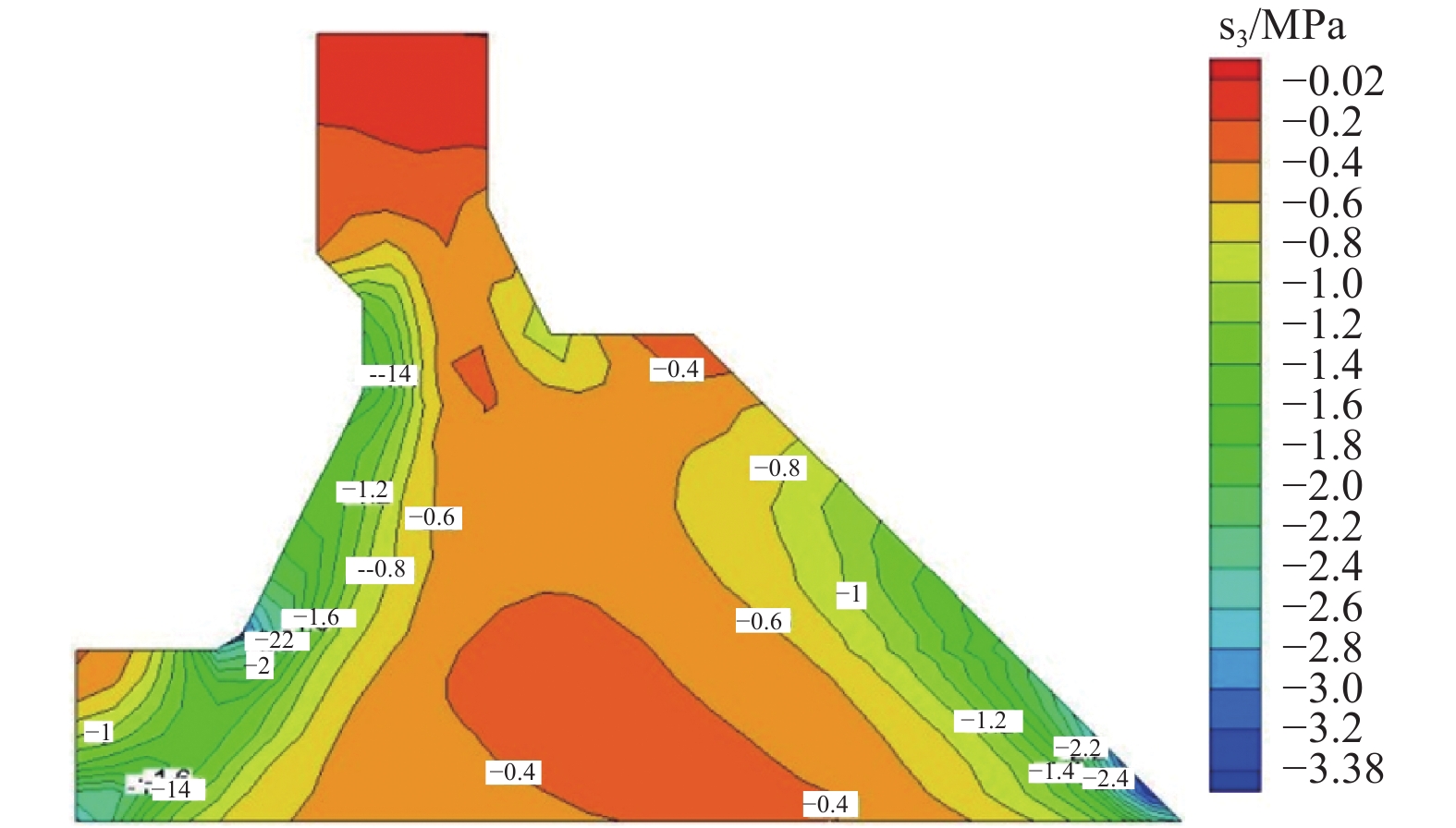
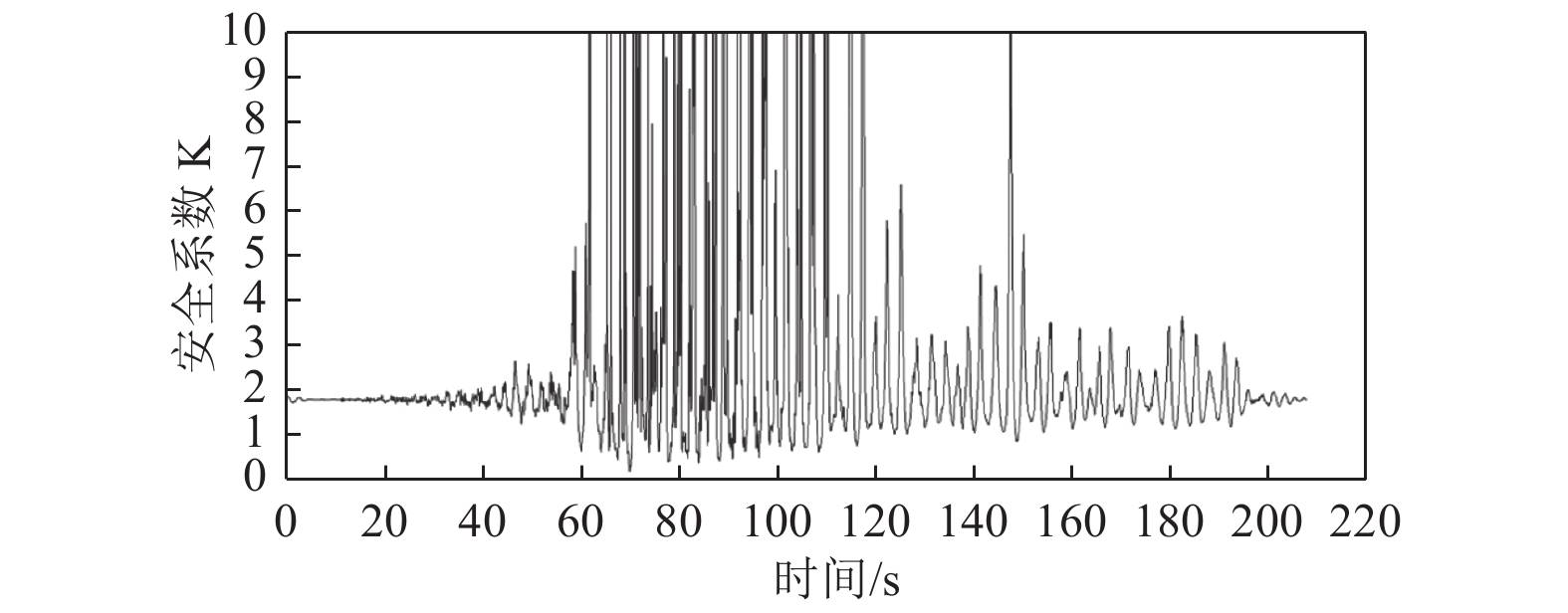
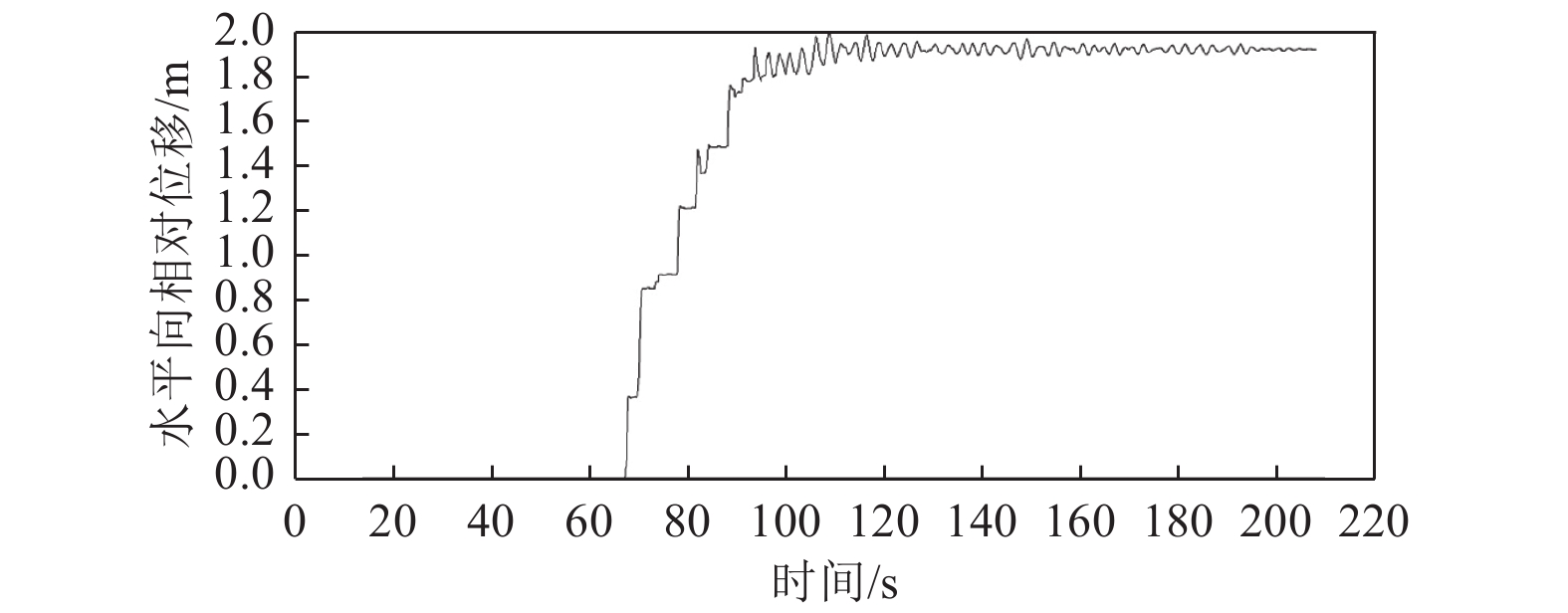
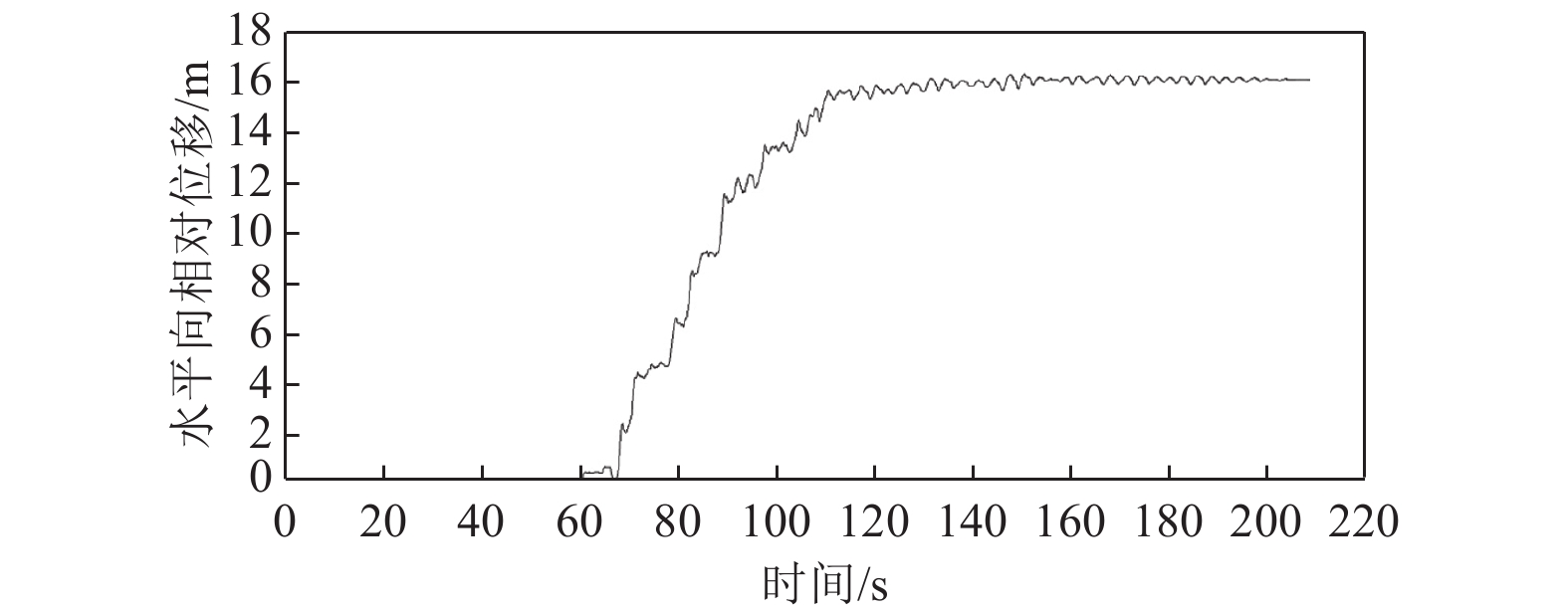
摘要: 本文通过成层状地基地震动输入计算方法得到覆盖层边界自由场运动，采用粘弹性边界，考虑地基辐射阻尼效应及坝体和地基的接触非线性，针对强震区深厚覆盖层场地重力坝开展线性和非线性动力时程分析研究，结合需求能力比DCR评估其抗震性能。由线弹性动力时程分析可知，在运行基准地震OBE作用下，重力坝坝体应力均在允许范围内，其抗滑稳定安全系数不能满足要求；由非线性动力分析可知，在OBE和最大设计地震MDE作用下，重力坝发生较大滑动位移。通过在重力坝坝体下游坝后回填土加强重力坝抗震稳定性，结果表明，下游坝后回填土可有效减小坝体滑动位移，加强其抗震稳定性。本文针对深厚覆盖层场地重力坝开展的抗震安全研究为抗震设计提供了科学依据，为强震区深厚覆盖层场地重力坝的抗震分析提供参考。
-
关键词:
- 重力坝 /
- 覆盖层场地 /
- 地基辐射阻尼效用 /
- DCR /
- 线性和非线性动力时程
Abstract: Based on the method for calculating the seismic input of a layered foundation, the free field motion is obtained. The viscoelastic boundary is adopted to consider the radiation damping effect and the contact nonlinearity between dam and foundation is considered. The linear and nonlinear dynamic time history analysis of a gravity dam in strong earthquake area is carried out, and its seismic performance is evaluated based on demand capacity ratio. The linear elastic dynamic time history analysis shows that the stress of gravity dam body is within the allowable range under OBE, while its seismic stability safety coefficient cannot meet the requirements; the nonlinear dynamic analysis shows that under OBE and MDE, the gravity dam appears obvious sliding. Furthermore, the backfill behind the gravity dam downstream is considered to enhance the seismic stability of the gravity dam. The results show that the backfill behind the downstream dam can effectively reduce the sliding, and enhance its seismic stability. In this paper, the research on seismic safety of the gravity dam under overburden site provides a scientific basis for its seismic design, and also provides a reference for seismic analysis of gravity dams under overburden sites in strong earthquake area.-
Key words:
- Gravity dam /
- Overburden /
- Radiation damping effect /
- DCR /
- Linear and nonlinear dynamic time history
-
引言
2017年4月12日2时25分，浙江省杭州市临安市（现为杭州市临安区，考虑地震发生时行政区划名称为临安市，本文沿用临安市名称）发生4.2级地震，震中（30.08°N，119.34°E）位于临安市河桥镇与潜川镇交界附近的九龙坑，震源深度16.2km。震中附近的潜川镇、河桥镇震感强烈，於潜镇、太阳镇、湍口镇等地也有较明显的震感。地震发生后，浙江省地震局启动了Ⅲ级响应，组织工作队赶赴地震现场，在对震区地质构造概况、震源机制解、实际震情等情况进行分析的基础上，开展了烈度调查，获取了震区震害分布基本资料，并绘制了地震烈度分布图。
1. 震区地形地貌及地质构造概况
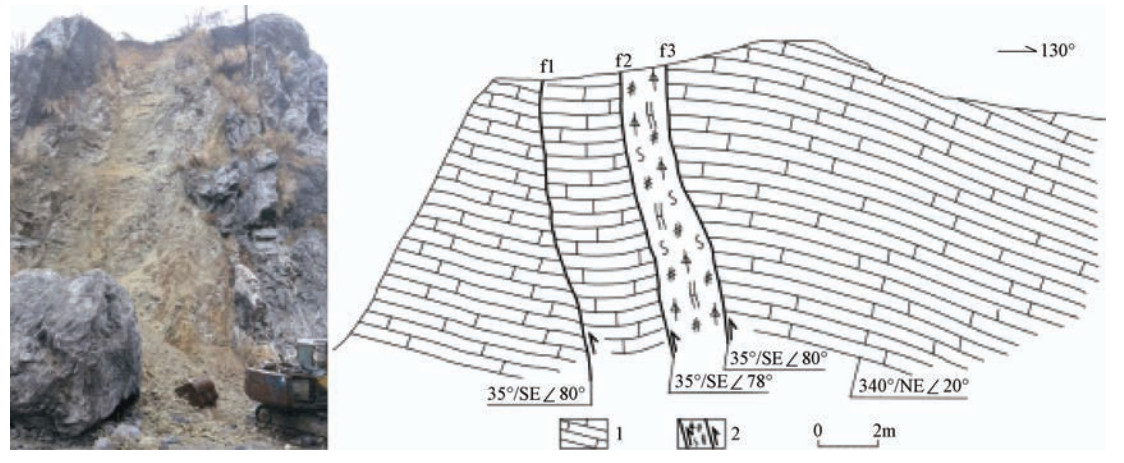
震区在地貌上属于浙西低山丘陵区，地势自西北、西南向东部倾斜。震区在大地构造上属于扬子准地台的钱塘台褶带，新构造运动主要表现为大面积的间歇性升降运动，以整体抬升为主，震区发育第四系早期的坡洪积物、侏罗系凝灰岩、志留系、奥陶系粉砂岩、泥岩及燕山期花岗岩等地层。震中附近发育区域性北东走向的马金-乌镇断裂带（图 1）。马金-乌镇断裂带是浙江省内1条重要的区域性断层（浙江省地质矿产局，1989），控制前新生代地层分区，总体走向约40°，倾向南东，倾角约80°，震区一带断层带宽12km、由多条分支组成，在东山畈采石场观察点见其中一主要分支断层的出露剖面（图 2），剖面上断层切割寒武纪厚层灰色灰岩，剖面中见3个断面（f1、f2、f3），性质皆逆断。其中，f1为单一断面，f2与f3则构成宽约2m的黄褐色粗碎裂岩；f2、f3延伸到冲沟对面，断面及黄褐色粗碎裂岩仍出露清楚（图 3），且沿2个断面还形成1—2cm的灰绿色断层泥，断层泥ESR样品测试结果为（151±30）ka BP，表明断层中更新世有过活动迹象。
图 2 东山畈附近调查照片及清绘剖面1Figure 2. Fault profile #1 near Dongshanfan1：厚层灰色灰岩；2：逆断层及黄褐色粗碎裂岩
图 3 东山畈附近断层照片及清绘剖面2Figure 3. Fault profile #2 near Dongshanfan1：厚层灰色灰岩；2：逆断层及黄褐色粗碎裂岩；3：逆断层及灰绿色断层泥；4：ESR样点2. 震源机制
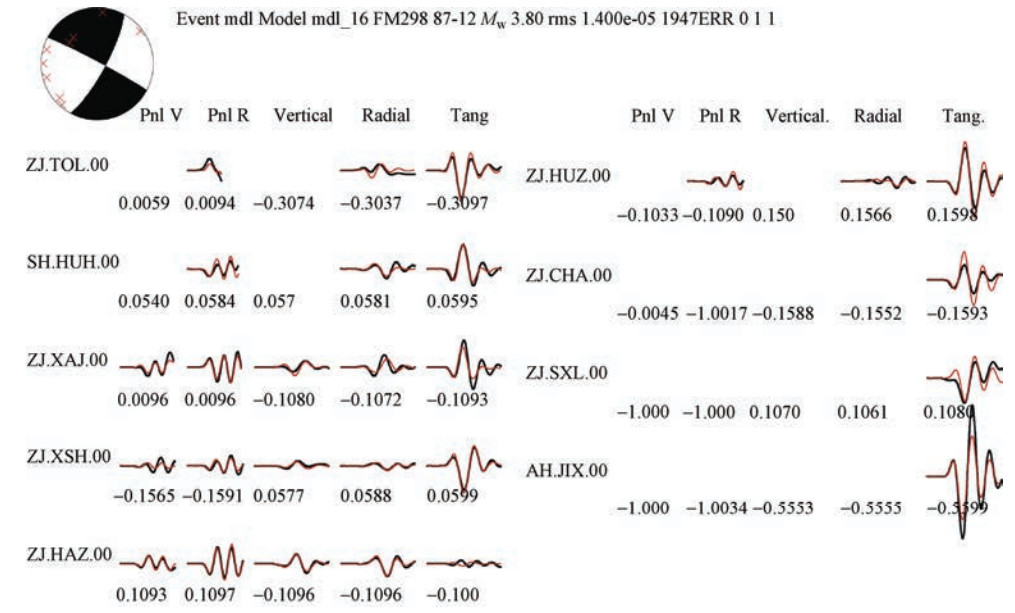
采用CAP方法（Zhao等，1994；Zhu等，1996；Wang，2011），计算获得了此次地震的震源机制解。其中，节面Ⅰ走向为298°，倾向87°，滑动角-12°；节面Ⅱ走向为29°，倾向78°、滑动角为-177°；主压应力轴（P轴）方位角平均为253°；主张应力轴（T轴）方位角平均为344°。震源机制解具体数值见表 1，拟合波形如图 4所示，图中红色曲线为理论地震波形，黑色曲线为观测地震波形。
表 1 临安4.2级地震震源机制解参数Table 1. The parameters of focal mechanism solution of Lin'an M 4.2 earthquake最佳双力偶解 节面Ⅰ/° 节面Ⅱ/° 走向 倾向 滑动角 走向 倾向 滑动角 298 87 -12 29 78 -177 应力轴 T轴/° B轴/° P轴/° 方位角 倾角 方位角 倾角 方位角 倾角 344 6 104 78 253 11 
图 4 临安4.2级地震震源机制解参数Figure 4. The focal mechanism solution of the Lin'an M 4.2 earthquakeCAP方法求解震源机制解受震源深度影响较大。通过设置步长1km，搜索不同深度对应的震源机制解，拟合误差越小，深度越准确。最终，得到误差最小的16.2km为此次临安M 4.2地震最佳震源深度（图 5）。
震区现代构造应力场的特征以北东东向水平压应力、北北西向水平张应力为主，北东向断层容易发生右旋走滑错动，震源机制解显示本次地震受大范围构造应力的控制。
3. 现场调查与震害特征
3.1 现场调查
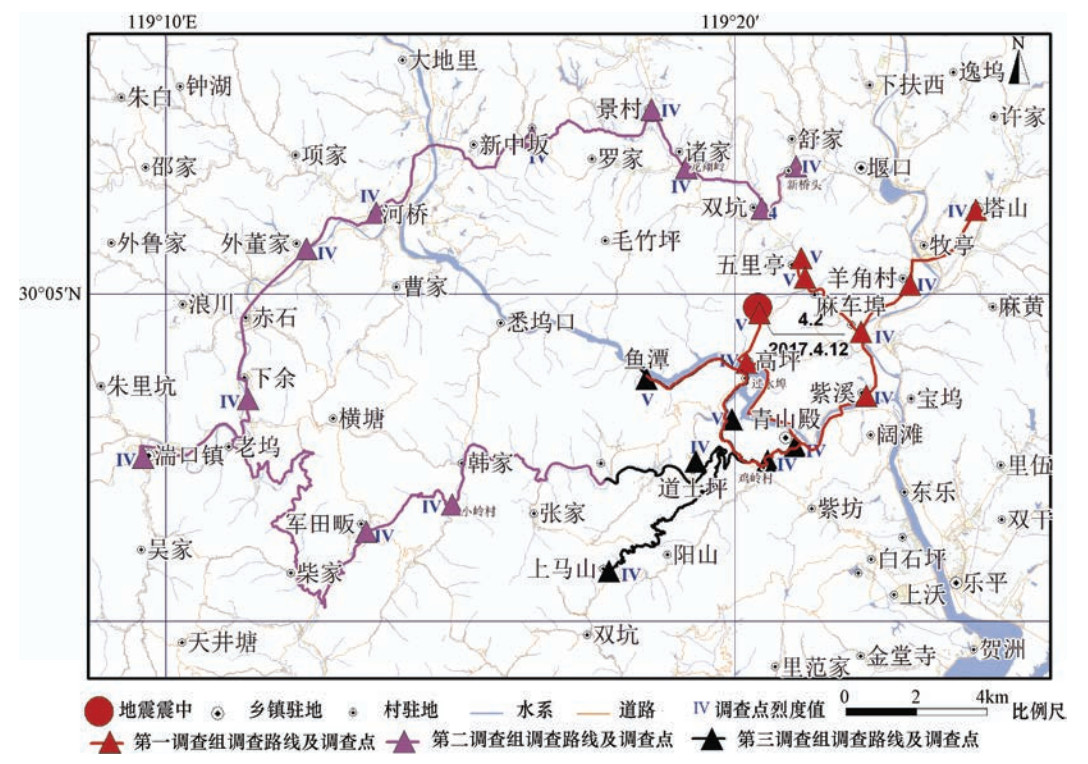
依据《地震现场工作（第3部分）：调查规范》、《地震现场工作（第4部分）：灾害直接损失评估》（中华人民共和国国家质量监督检验检疫总局等, 2012a, 2012b）及《中国地震烈度表》（GB/T 17742—2008）（中华人民共和国国家质量监督检验检疫总局等，2009）中的技术要求，主要根据震区人的感觉、器物反应、房屋震害程度等（夏坤等，2011）开展地震烈度调查与评定工作。根据当地政府反馈的震感情况，分别在潜川镇、河桥镇、於潜镇、太阳镇和湍口镇安排调查路线，共25个调查点，取得了震区大量的基础数据和第一手资料，调查范围基本上覆盖了本次地震震区。地震现场调查路线及调查点分布见图 6。
3.2 震区烈度分布情况
通过对25个调查点的震害评估与科学考察，同时收集了当地政府提供的人员伤亡、房屋受损排查情况以及山塘水库基础设施和地质灾害点排查情况，4个调查点的烈度评定为Ⅴ度，分别为潜川镇的五里亭、茶叶山、鱼潭和过水埠，主要分布在震中的东北和西南，Ⅴ度区面积约22.6km2；其余21个调查点烈度评定为Ⅳ度（图 7）。本次地震未造成人员伤亡和较大经济损失。
Ⅴ度区人和器物的反应特征主要为：①震区主要建筑为3层房屋，绝大多数调查点居民居住在2楼，反映震感强烈，多数人梦中惊醒，少数逃出户外，个别居民在室外过夜直至天明；②绝大多数被调查居民听到地声，且反映声音很响，如同打雷的轰轰声或放炮声，部分居民能明确指出声音由震源方向传来；③大多数被调查居民听到门窗、屋顶、屋架颤动作响，且门窗响声较大；④多数被调查居民感觉床和悬挂物有较大幅度的晃动。
Ⅴ度区房屋震害特征主要为：①个别土木、砖木结构房屋有老裂缝加宽、加长及墙皮脱落现象；②个别砌体结构房屋出现细微裂缝和老裂缝加宽、加长及灰土掉落现象（图 8）。
图 8 震中区个别老旧房屋震害特征Figure 8. Earthquake damage characteristics of some old houses at the epicenter area（a）五里亭某户砖木结构房屋二楼窗口下墙皮掉落；（b）新桥头某户土木结构房屋墙体老裂缝加长、加宽；（c）鱼潭村某户砌体房屋老缝加长，瓷砖出现细小裂纹通过调查分析本次地震的房屋破坏情况，认为造成破坏的主要因素有以下方面：
（1）房屋质量
震区内产生破坏的个别房屋属于老旧房屋，建造时基本没有采取任何抗震措施，而且房龄长，一般多在20多年以上，有的甚至30、40年，这些房屋多为夯土（砖、石）墙木结构，或者某一面承重墙为空斗砖墙，由于房龄长、年久失修且普遍存在裂缝，有的墙体已倾斜，特别是房屋的屋顶、屋内木架构，梁木多有腐烂现象；另外，震区内部分建造于2000年以前的砌体房屋中，仅有少数有地梁或圈梁，多数无地梁、圈梁和构造柱，抗震性能较差，加之地基基础的不均匀，个别房屋墙体出现细微裂缝或抹灰缝。
（2）场地效应
震区位于山区，多数自然村分布于陡坡、边坡等地，导致部分调查点上的个别房屋在建造时为天然地基加垒石形成的不均匀基础（属于抗震不利地段），地震时容易产生沉降差异，抗震稳定性差，易出现轻微破坏。
4. 结语
通过对临安4.2级地震开展现场调查，得出如下认识：
（1）震源机制解显示长轴方向为北东向，与马金-乌镇断裂带走向基本一致；地震现场地震烈度调查结果表明，本次地震的影响烈度最高为Ⅴ度，面积22.6km2，烈度圈长轴方向为北东向，与地震震源机制解及北东向马金-乌镇断裂带方向一致。
（2）本次地震震中附近的潜川镇、河桥镇震感强烈，但未造成人员伤亡和较大经济损失，震中区少数房屋有轻微开裂和老裂缝加长、加宽现象。
（3）与浙江省内其它类似震级大小的地震相比，此次地震震源深度较深（16km），地面的地震破坏影响相对较小，因此虽然震感强烈，但震害相对较轻。
-
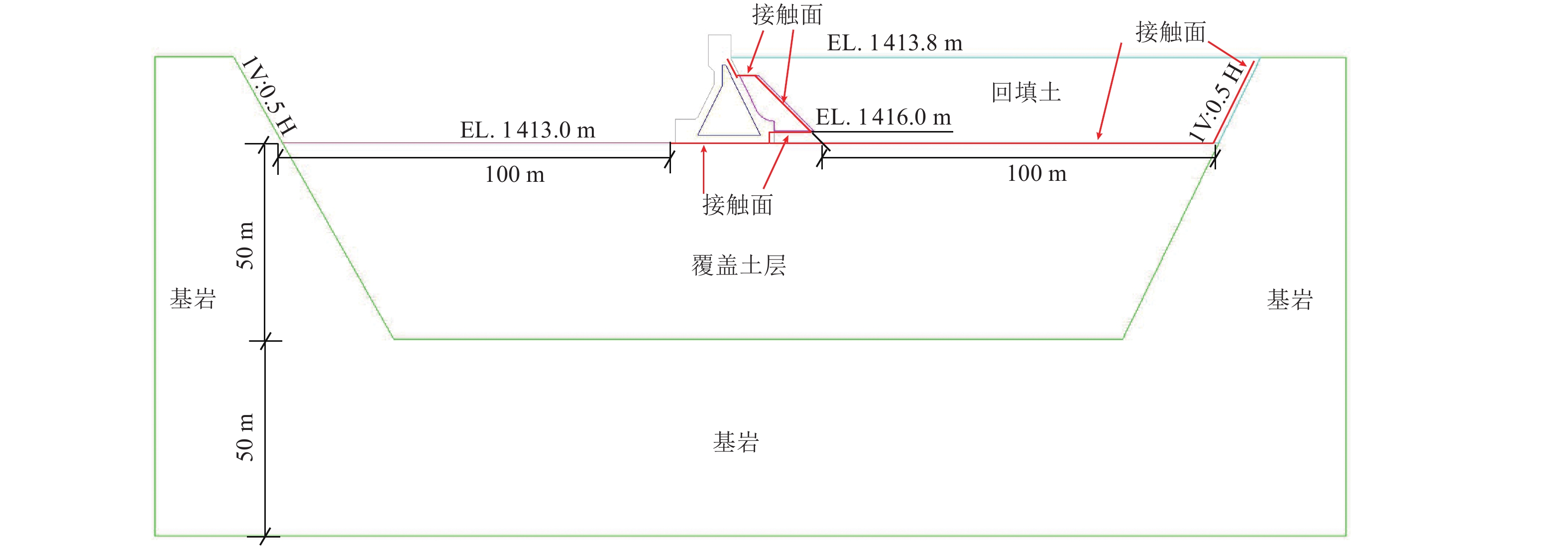
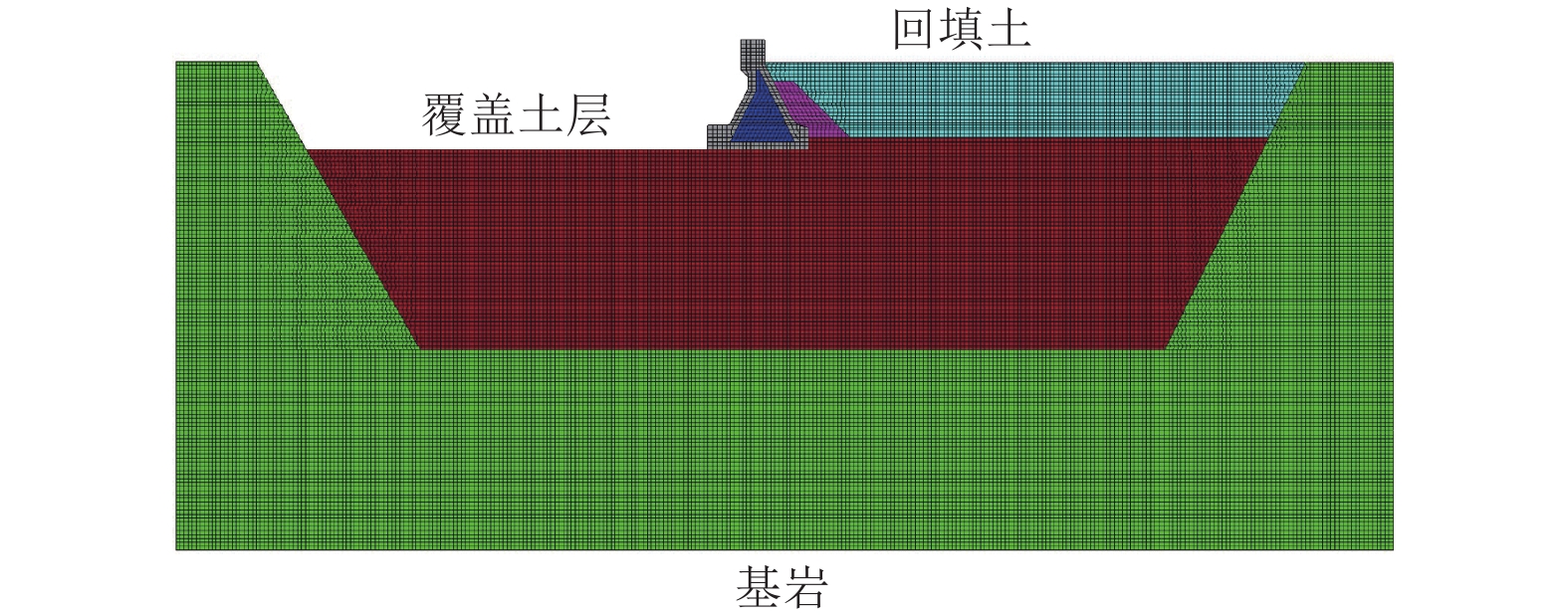
表 1 混凝土及地基材料参数
Table 1. Material parameters of concrete and foundation
材料 容重γ/kN·m−3 剪切
模量G/MPa泊松比$ \mu $ 弹性模量E/MPa 摩擦角φ/° 容许承载力/kPa 抗压/抗拉强度/MPa 砼C20 24.0 12000 0.167 28000 － 12500 20/2.40 毛石砼
C1224.0 9500 0.167 22000 － 7500 12/1.71 覆盖层 19.0 － 0.200 25 32.5 400 － 岩石 26.5 1800 0.275 4500 － － － 
下载: 导出CSV
表 2 各类接触面参数
Table 2. Parameters of contact surfaces
接触面 粘聚力c/kPa 摩擦角φ/° 砼-砼 0 45.0 砼-毛石砼 0 40.0 砼-覆盖层 0 28.8 毛石砼-覆盖层 0 28.8
下载: 导出CSV
-
[1] 杜修力, 袁雪纯, 黄景琦, 等, 2017. 典型土层场地随机地震反应规律分析. 震灾防御技术, 12(3): 574—588 doi: 10.11899/zzfy20170314Du X. L. , Yuan X. C. , Huang J. Q. , et al. , 2017. Analysis of stochastic seismic response in typical soil sites. Technology for Earthquake Disaster Prevention, 12(3): 574—588. (in Chinese) doi: 10.11899/zzfy20170314 [2] 刘晶波, 吕彦东, 1998. 结构-地基动力相互作用问题分析的一种直接方法. 土木工程学报, 31(3): 55—64Liu J. B. , Lv Y. D. , 1998. A direct method for analysis of dynamic soil-structure interaction. China Civil Engineering Journal, 31(3): 55—64. (in Chinese) [3] 潘坚文, 张楚汉, 徐艳杰, 2010. 强震输入方式与地基模型对重力坝反应的影响. 岩土工程学报, 32(1): 82—88Pan J. W. , Zhang C. H. , Xu Y. J. , 2010. Influence of seismic input mechanism and foundation models on response of gravity dams. Chinese Journal of Geotechnical Engineering, 32(1): 82—88. (in Chinese) [4] 殷琳, 楼梦麟, 康帅, 2019. 水平分层土层系统等效阻尼比的简化计算方法. 震灾防御技术, 14(1): 10—23 doi: 10.11899/zzfy20190102Yin L. , Lou M. L. , Kang S. , 2019. Simplified method for determining equivalent damping ratio of horizontal multi-layered soil stratum. Technology for Earthquake Disaster Prevention, 14(1): 10—23. (in Chinese) doi: 10.11899/zzfy20190102 [5] 郑晓东, 刘云贺, 马静, 2016. 考虑强震持续时间的混凝土重力坝损伤累积研究. 水利水电技术, 47(4): 18—23Zheng X. D. , Liu Y. H. , Ma J. , 2016. Study on accumulated damage of concrete gravity dam under consideration of strong-motion duration. Water Resources and Hydropower Engineering, 47(4): 18—23. (in Chinese) [6] ABAQUS. 2010. ABAQUS 6.10: Analysis User’s Manual. Providence: ABAQUS, Inc. [7] Alembagheri M. , 2016. Earthquake damage estimation of concrete gravity dams using linear analysis and empirical failure criteria[J]. Soil Dynamics and Earthquake Engineering, 90: 327—339. doi: 10.1016/j.soildyn.2016.09.005 [8] Bhattacharjee S. S. , Léger P. , 1993. Seismic cracking and energy dissipation in concrete gravity dams. Earthquake Engineering & Structural Dynamics, 22(11): 991—1007. [9] Cervera M. , Oliver J. , Faria R. , 1995. Seismic evaluation of concrete dams via continuum damage models. Earthquake Engineering & Structural Dynamics, 24(9): 1225—1245. [10] Chopra A. K. , Wang J. T. , 2010. Earthquake response of arch dams to spatially varying ground motion. Earthquake Engineering & Structural Dynamics, 39(8): 887—906. [11] Chopra A. K. , 2012. Earthquake analysis of arch dams: factors to be considered. Journal of Structural Engineering, 138(2): 205—214. doi: 10.1061/(ASCE)ST.1943-541X.0000431 [12] Deeks A. J. , Randolph M. F. , 1994. Axisymmetric time-domain transmitting boundaries. Journal of Engineering Mechanics, 120(1): 25—42. doi: 10.1061/(ASCE)0733-9399(1994)120:1(25) [13] El-Aidi B. , Hall J. F. , 1989. Non-linear earthquake response of concrete gravity dams part 1: modelling. Earthquake Engineering & Structural Dynamics, 18(6): 837—851. [14] Fenves G. , Chopra A. K. , 1985. Effects of reservoir bottom absorption and dam-water-foundation rock interaction on frequency response functions for concrete gravity dams. Earthquake Engineering & Structural Dynamics, 13(1): 13—31. [15] Fenves G. L. , Mojtahedi S. , Reimer R. B. , 1992. Effect of contraction joints on earthquake response of an arch dam. Journal of Structural Engineering, 118(4): 1039—1055. doi: 10.1061/(ASCE)0733-9445(1992)118:4(1039) [16] Hall J. F. , Chopra A. K. , 1982. Hydrodynamic effects in the dynamic response of concrete gravity dams. Earthquake Engineering & Structural Dynamics, 10(2): 333—345. [17] Hariri-Ardebili M. A. , Mirzabozorg H. , Ghasemi A. , 2013. Strain-based seismic failure evaluation of coupled dam-reservoir-foundation system. Coupled Systems Mechanics, 2(1): 85—110. doi: 10.12989/csm.2013.2.1.085 [18] Idriss I. M. , Sun J. I. , 1992. User's manual for SHAKE91-A computer program for conducting equivalent linear seismic response analysis of horizontally layered soil deposits. Davis: University of California. [19] Løkke A. , Chopra A. K. , 2018. Direct finite element method for nonlinear earthquake analysis of 3-dimensional semi-unbounded dam-water-foundation rock systems. Earthquake Engineering & Structural Dynamics, 47(5): 1309—1328. [20] Pan J. W. , Zhang C. H. , Xu Y. J. , et al. , 2011. A comparative study of the different procedures for seismic cracking analysis of concrete dams. Soil Dynamics and Earthquake Engineering, 31(11): 1594—1606. doi: 10.1016/j.soildyn.2011.06.011 [21] Tan H. C. , Chopra A. K. , 1996. Dam-foundation rock interaction effects in earthquake response of arch dams. Journal of Structural Engineering, 122(5): 528—538. doi: 10.1061/(ASCE)0733-9445(1996)122:5(528) [22] US Army Corps of Engineers. 1995. EM 1110-2-2200 Gravity dam design. Washington: US Army Corps of Engineers. [23] US Army Corps of Engineers. 2003. EM 1110-2-6051 Time-history dynamic analysis of concrete hydraulic structures. Washington: US Army Corps of Engineers. [24] US Army Corps of Engineers. 2005. EM 1110-2-2100 Stability analysis of concrete structures. Washington: US Army Corps of Engineers. [25] US Army Corps of Engineers. 2007. EM 1110-2-6053 Earthquake design and evaluation of concrete hydraulic structures. Washington: US Army Corps of Engineers. [26] Zhang C. H. , Pan J. W. , Wang J. T. , 2009. Influence of seismic input mechanisms and radiation damping on arch dam response. Soil Dynamics and Earthquake Engineering, 29(9): 1282—1293. doi: 10.1016/j.soildyn.2009.03.003 -
下载:
点击查看大图
计量
- 文章访问数: 238
- HTML全文浏览量: 82
- PDF下载量: 14
- 被引次数: 0
