Influence of Bi-directional Horizontal Ground Motion on the Vulnerability of a Reinforced Concrete Continuous Beam Bridge
-
摘要: 以双向水平地震动作为输入,对钢筋混凝土连续箱梁高架桥开展非线性动力时程分析。建立基于双向水平地震动强度参数的桥梁结构易损性曲面,比较单向及双向水平地震动输入下桥梁结构易损性差异,分析双向水平地震动输入下横桥向地震动强度对桥梁整体易损性的影响规律。研究结果表明,双向水平地震动输入下的桥梁结构易损性明显高于单向地震动输入的情况,且随着横桥向输入地震动强度的增加,结构各破坏状态的超越概率明显增大。Abstract: The nonlinear dynamic time history analysis of reinforced concrete continuous box girder bridge is carried out with bidirectional horizontal ground motion as input. The vulnerability surface of bridge structure is established based on bidirectional horizontal ground motion intensity parameters. The difference of vulnerability of bridge structure under unidirectional and bidirectional horizontal ground motion input is compared, and the influence law of transverse seismic intensity on overall vulnerability of bridge under bidirectional horizontal ground motion input is analyzed. The results show that: the vulnerability of bridge structure under bidirectional horizontal ground motion input is significantly higher than that under unidirectional ground motion input, and with the increase of transverse ground motion input intensity, the exceeding probability of each failure state of the structure also increases significantly.
-
引言
随着我国城市化进程的不断加快和基础设施建设的不断完善,以城市高架桥为依托的城市交通网络逐渐建立。以往经验表明,城市高架桥作为生命线系统的重要组成部分,易在地震中遭到破坏,从而造成严重后果。桥梁地震易损性分析作为地震风险评估中的重要环节及基于性能的地震工程的重要组成部分,可从概率层面对桥梁抗震性能进行有效评估,为桥梁结构抗震设防提供参考。
近年来,桥梁结构地震易损性分析受到国内外学者的广泛关注,并取得了一系列研究成果。Yamazaki等(2000)对Kobe地震中216座桥梁震害数据进行了调查与统计分析,通过最小二乘法回归分析得到了以地震动峰值加速度PGA为地震动强度参数的经验易损性曲线。Shinozuka等(2000)基于1995年神户地震中观测到的桥梁损伤数据,假设易损性函数呈双参数的对数正态分布,采用极大似然方法对未知参数进行估计,从而得到了桥梁墩柱经验易损性曲线。陈力波等(2012)基于对汶川地震桥梁震害的调查,针对分类后的桥梁样本子集建立了各自独立的地震易损性曲线。林庆利等(2017)在现有研究的基础上,对汶川地震高烈度区桥梁震害数据加以补充,分析了桥型和桥梁规模对易损性的影响。
由于能够较真实地反映结构在地震激励下的响应,基于非线性动力时程分析方法获得理论易损性曲线的方法被广泛研究。Hwang等(2004)使用SAP2000软件建立了连续梁桥有限元模型,并采用位移延性比作为损伤指标,通过非线性时程分析分别得到了以加速度反应谱值Sa和PGA作为地震动强度指标的理论易损性曲线。张菊辉(2006)通过数值模拟,系统地研究了墩柱高度、截面尺寸 、配箍率变化、支座形式等对桥梁易损性曲线的影响。刘洋等(2016)和李勇等(2018)研究了考虑脉冲近场地震动对桥梁易损性的影响。李立峰等(2016)和谷音等(2019)综合考虑了氯离子的侵蚀作用,对桥梁时变地震易损性进行了评估和分析。宋帅等(2020)基于串并联体系的桥梁系统易损性分析方法,对中小跨径梁桥地震易损性进行了研究。
在以往的研究中,多数学者在进行非线性时程分析时采用单向地震动输入的方法,这明显不符合结构在地震中的真实受力情况。易方民等(2003)和姬淑艳等(2006)的研究表明结构构件地震响应在2个主轴方向上存在耦合关系,仅考虑单向地震动输入可能会低估地震动对结构的破坏程度。周长东等(2017)分别输入单向、双向水平和三向地震动作用,得到了钢筋混凝土双曲线冷却塔的易损性曲线,研究结果表明,双向水平或三向地震动作用下的冷却塔损伤概率较单向地震动作用下显著增加。
综上所述,虽然诸多学者对桥梁地震易损性已进行了较多研究,但考虑双向水平地震动输入对钢筋混凝土桥梁易损性影响的研究较少。基于此,本文以规则钢筋混凝土连续梁桥为研究对象,采用增量动力分析法,通过双向水平地震动输入的非线性时程分析建立基于不同地震动强度指标的易损性曲线,进一步研究双向水平地震动输入对桥梁易损性的影响。
1. 地震易损性分析原理与方法
结构易损性表示在给定地震动强度水平的地震动作用下,工程结构达到或超越一定破坏状态的条件概率,概率表达式为:
$$ P_{\mathrm{f}}=P(D \geqslant C \mid I M)$$ (1) 式中,Pf表示结构达到某极限状态的概率;D表示结构能力需求,C表示结构抗震能力指标,D≥C表示结构达到或超越某种极限状态,IM表示地震动强度参数。
概率地震需求模型表示结构地震需求与地震动强度之间的关系,依据Shome(1999)的研究结果,在给定地震动强度IM的前提下,可认为结构地震需求D服从对数正态分布。在此基础上,Cornell等(2002)给出了结构地震需求参数与地震动强度参数IM的关系表达式:
$$ \mu_{{\rm{d}}}=a(I M)^{b}$$ (2) 将式(2)两端取自然对数得:
$$ \ln \left(\mu_{\mathrm{d}}\right)=\ln {a}+{b} \ln (I M)$$ (3) 式中,μd为给定地震动强度IM下的地震需求中值;a、b为待定常数,可通过对数回归拟合得到。假定结构地震需求D服从对数正态分布,则概率地震需求模型可表示为:
$$ P(D \geqslant C \mid I M)=1-\varPhi\left[\frac{\ln C-\ln \left(\mu_{\mathrm{d}}\right)}{\beta_{\mathrm{d}}}\right]$$ (4) $$ \beta_{\mathrm{d}}=\sqrt{\frac{\displaystyle\sum_{{i}=1}^{{n}}\left[\ln C-\left(\ln {a}+{b\ln} I M_{{i}}\right)\right]^{2}}{{n}-2}}$$ (5) 式中,Φ(x)为标准正态累计分布函数,βd为地震需求的对数标准差(徐超等,2017)。
进一步假定结构抗震能力服从对数正态分布,特定阶段的失效概率Pf可表示为:
$$ P_{\mathrm{f}}=\varPhi\left[\frac{\ln \left(\mu_{\mathrm{d}}\right)-\ln \left(\mu_{\mathrm{c}}\right)}{\sqrt{\beta_{{\rm{c}}}{ }^{2}+\beta_{{\rm{d}}}{ }^{2}}}\right]=\varPhi\left[\frac{\ln {a}+{b} \ln (I M)-\ln \left(\mu_{\mathrm{c}}\right)}{\sqrt{\beta_{{\rm{c}}}{ }^{2}+\beta_{{\rm{d}}}{ }^{2}}}\right]$$ (6) 式中,μc为某种极限状态下桥梁抗力的中值,βc为桥梁抗力的对数标准差。
根据美国联邦应急管理局( Federal Emergency Management Agency,FEMA)和国家建筑科学研究所(National Institute of Building Sciences)合作开发的地震损失估计方法 HAZUS 99(Federal Emergency Management Agency (FEMA),2001),当易损性曲线以Sa为自变量时,
$\sqrt{{{\beta }_{{\rm{c}}}}^{2}+{{\beta }_{{\rm{d}}}}^{2}}$ =0.4;当易损性曲线以PGA为自变量时,$\sqrt{{{\beta }_{{\rm{c}}}}^{2}+{{\beta }_{{\rm{d}}}}^{2}}$ =0.5。2. 地震动-桥梁系统建立
2.1 模型建立
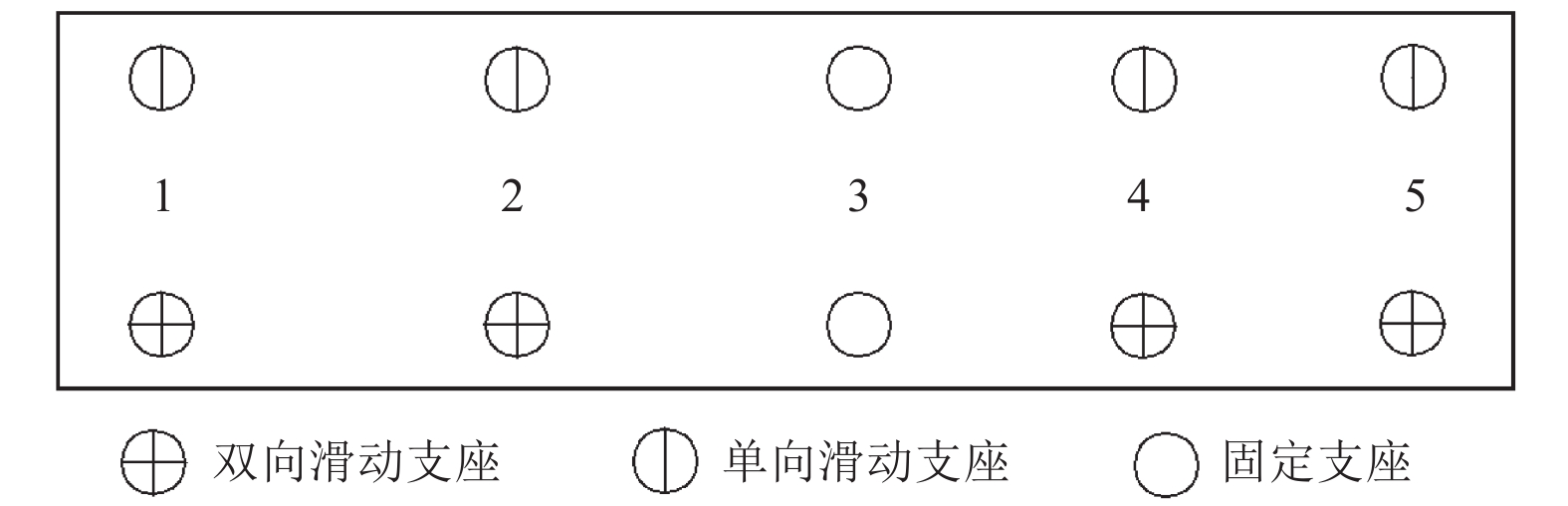
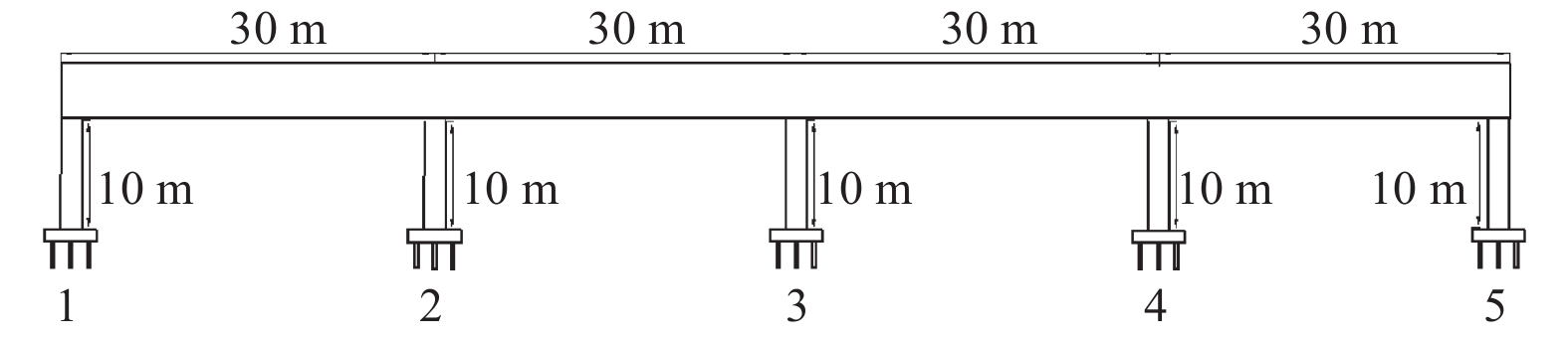
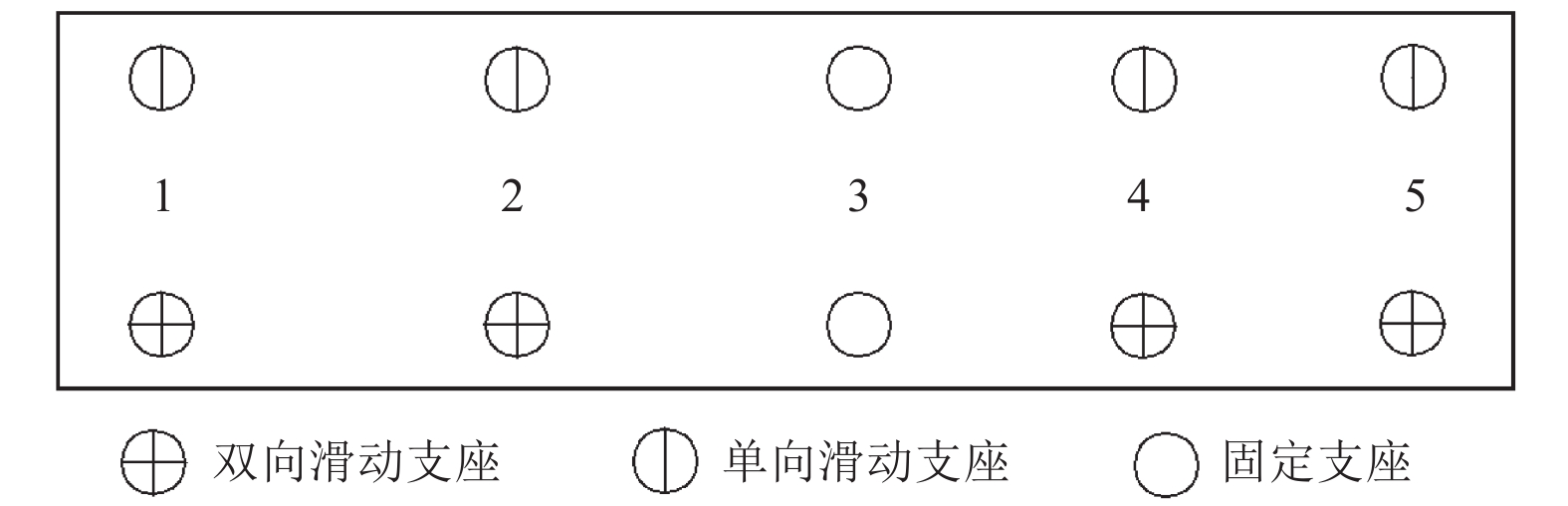
本文以某市钢筋混凝土高架连续箱梁桥为研究对象,选取连续梁桥中的一联。桥梁上部结构采用常见的等截面混凝土单箱梁,根据《公路桥涵设计通用规范》(JTG D60—2015)(中华人民共和国交通运输部,2015)规定的各级公路桥设计速度及车道宽度标准,桥面按照一级公路设计,采用单向三车道,上桥面宽为(3.75+3.75+3.5+0.25×2)m=11.5 m,下桥面宽为9.1 m,截面高度为1.6 m,选用C50混凝土。桥梁下部墩柱采用直径为1.2 m的圆形双柱墩,墩高6 m,单跨跨径为30 m,如图1所示。桥墩采用C40混凝土浇筑,最外层保护层厚度为50 mm,纵筋选用直径25 mm的HRB335钢筋,箍筋选用直径12 mm的HPB300钢筋,箍筋间距为100 mm,箍筋形式为环形。桥梁支座为中墩设置固定墩,墩顶设置固定盆式支座,其余连接处均在一侧设置单向滑动支座,在另一侧设置双向滑动支座,如图2所示。盖梁采用矩形截面,高1.2 m,宽1.6 m,采用C40混凝土浇筑。桥梁基础采用钻孔灌注桩,桩径为1.2 m。桥梁抗震设防烈度为8度,设计地震分组为第二组,场地类型为Ⅱ类,结构一阶自振周期为1.27 s (纵桥向振动)。
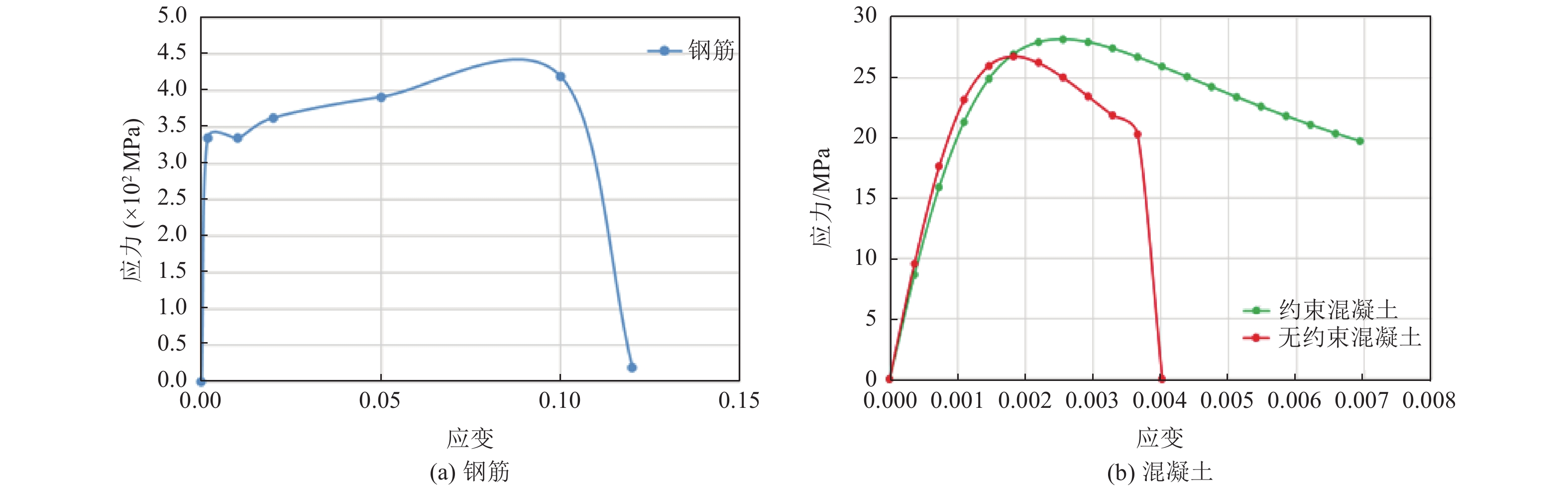
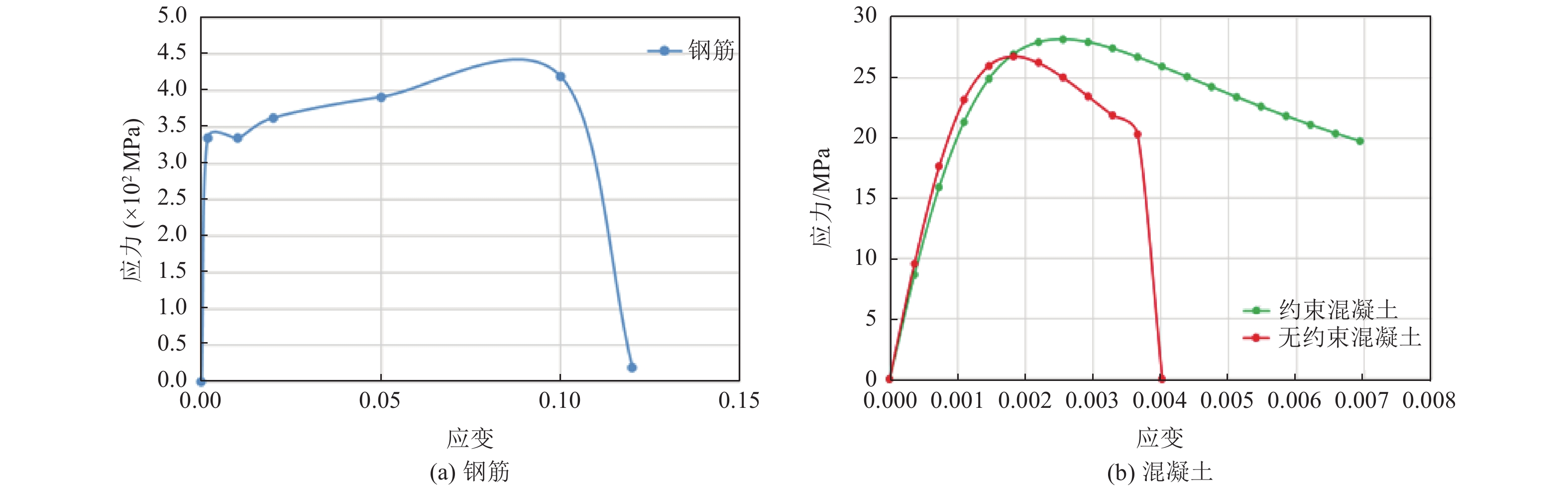
基于CSiBridge软件建立结构分析三维有限元计算模型。主梁和盖梁在地震动作用下一般处于弹性状态,桥梁体系的非线性仅出现在墩柱和支座中。因此,主梁和盖梁采用弹性梁单元模拟,而桥墩弹塑性通过轴力-弯矩铰(PMM)模拟。桥墩塑性铰一般出现在墩柱顶部和底部,塑性铰骨架曲线采用较适合钢筋混凝土的Takeda三折线退化滞回曲线表示,约束混凝土采用Mander本构模型模拟,钢筋、混凝土本构关系如图3所示。将桥墩按1~5从左至右依次编号,支座均采用Wen塑性单元模拟,各项参数均按照《公路桥梁盆式支座》(JT/T 391—2019)(中华人民共和国交通运输部,2019)要求进行设置,屈服力根据支座型号取其水平承载力。对于盆式固定支座和单向滑动支座的非滑移方向,取其屈服刚度无穷大,支座布置及支座参数如表1所示。桥墩下的桩基础采用等效弹簧单元模拟。
表 1 支座参数Table 1. Parameter of bearings支座类型 支座型号 支座位置 盆式固定支座 GPZ4000GX 3 盆式单向滑动支座 GPZ4000DX 1、2、4、5(上) 盆式双向滑动支座 GPZ4000SX 1、2、4、5(下) 2.2 桥梁破坏准则
在HAZUS 99(Federal Emergency Management Agency(FEMA),2001)中将地震对桥梁的破坏分为5种,即基本完好、轻微破坏、中等破坏、严重破坏和完全破坏,并使用桥墩位移延性比作为桥梁破坏状态的指标。目前关于桥梁墩柱易损性研究中,Hwang等(2004)提出的基于位移延性比μ的延性指标是应用最广泛的物理量之一。张菊辉(2006)在参考以上研究的基础上,考虑我国桥梁设计实际情况,提出以0.004的混凝土剥落应变作为中等破坏和严重破坏的限值,如表2所示。其中,μcy1为首次屈服时位移延性比,μcy为等效屈服位移延性比,μc4为柱截面边缘钢筋混凝土压应变达0.004时的位移延性比,μcmax为柱截面边缘钢筋混凝土达极限压应变时的位移延性比,且认为μcmax=μc4+3。
表 2 损伤破坏准则Table 2. Damage failure criterion损伤状态 基本完好 轻微破坏 中等破坏 严重破坏 完全破坏 破坏准则 0<μ≤μcy1 μcy1<μ≤μcy μcy<μ≤μc4 μc4<μ≤μcmax μcmax≤μ 本文使用UCFYBER进行弯矩-曲率计算,从而得到确定损伤指标所需的重要参数,即首次屈服曲率фy1、等效屈服曲率фy、混凝土应变εc=0.004时的曲率фc4、极限曲率фu,如表3所示,由此确定的损伤指标与损伤等级之间的关系如表4所示。
表 3 桥墩截面弯矩-曲率关系(单位:rad·m−1)Table 3. Moment- curvature relationship of pier section(Unit: rad·m−1)分析参数 фy1 фy фc4 фu 桥墩截面曲率 2.353 3.045 18.390 50.630 表 4 损伤指标与损伤等级关系Table 4. Relationship between damage index and damage grade损伤状态 基本完好 轻微破坏 中等破坏 严重破坏 完全破坏 破坏准则 0<μ≤1.00 1.00<μ≤1.29 1.29<μ≤3.34 3.34<μ≤6.34 6.34<μ 2.3 地震记录的选取
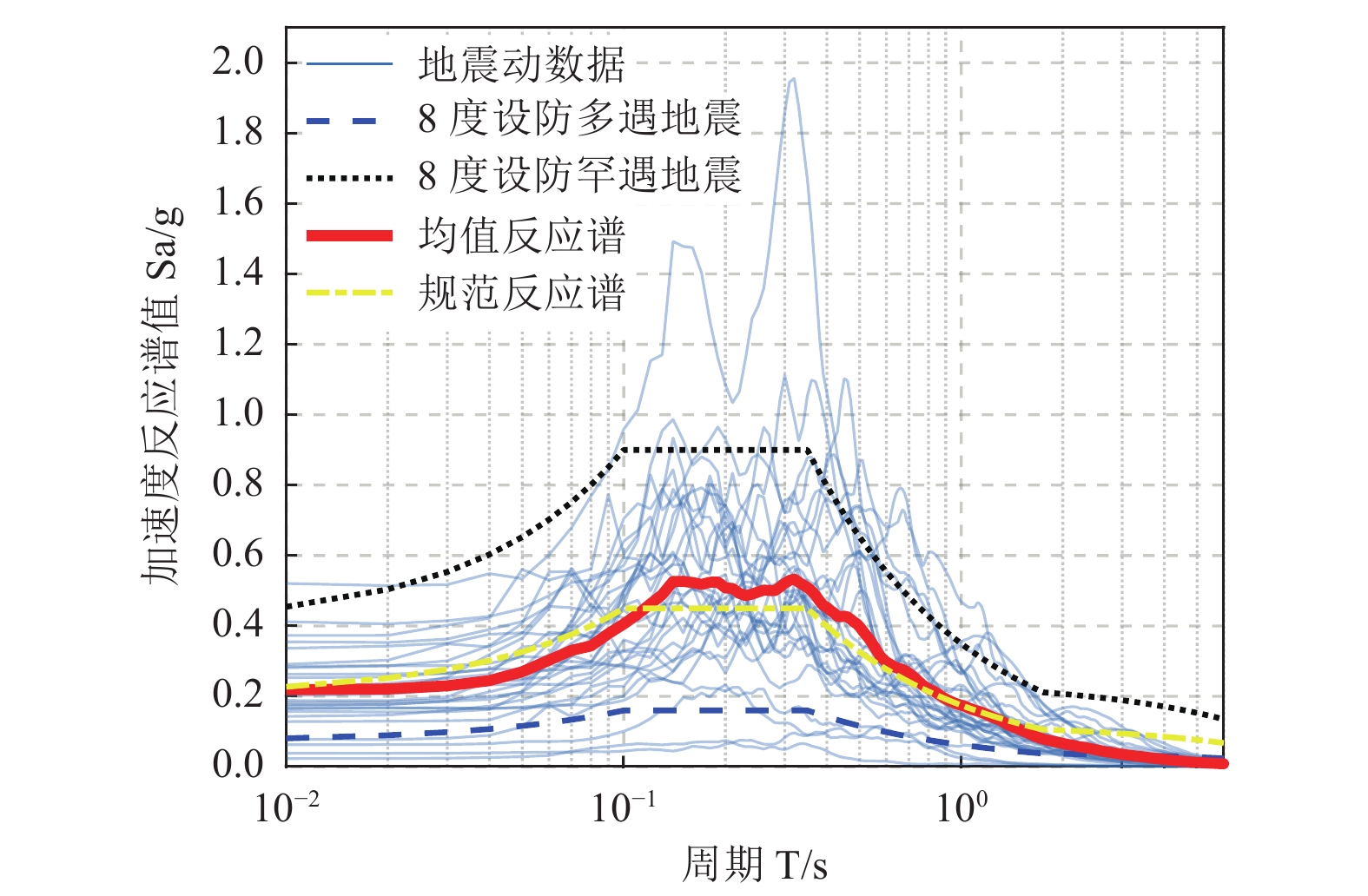
地震动具有很强的随机性,为充分考虑地震动的不确定性给非线性时程分析带来的影响,从美国太平洋地震工程研究中心(PEER)强震数据库中选择30组原始双向水平地震动记录。为保证地震动记录的一般性,所选择的30组地震动记录基于不同的地震事件,震中距分布范围为0.75~59.52 km,震级按照不同数量的比例进行选取,其中震级为5~6的地震动有15组,震级为6~7的地震动有10组,震级>7的地震动有5组。
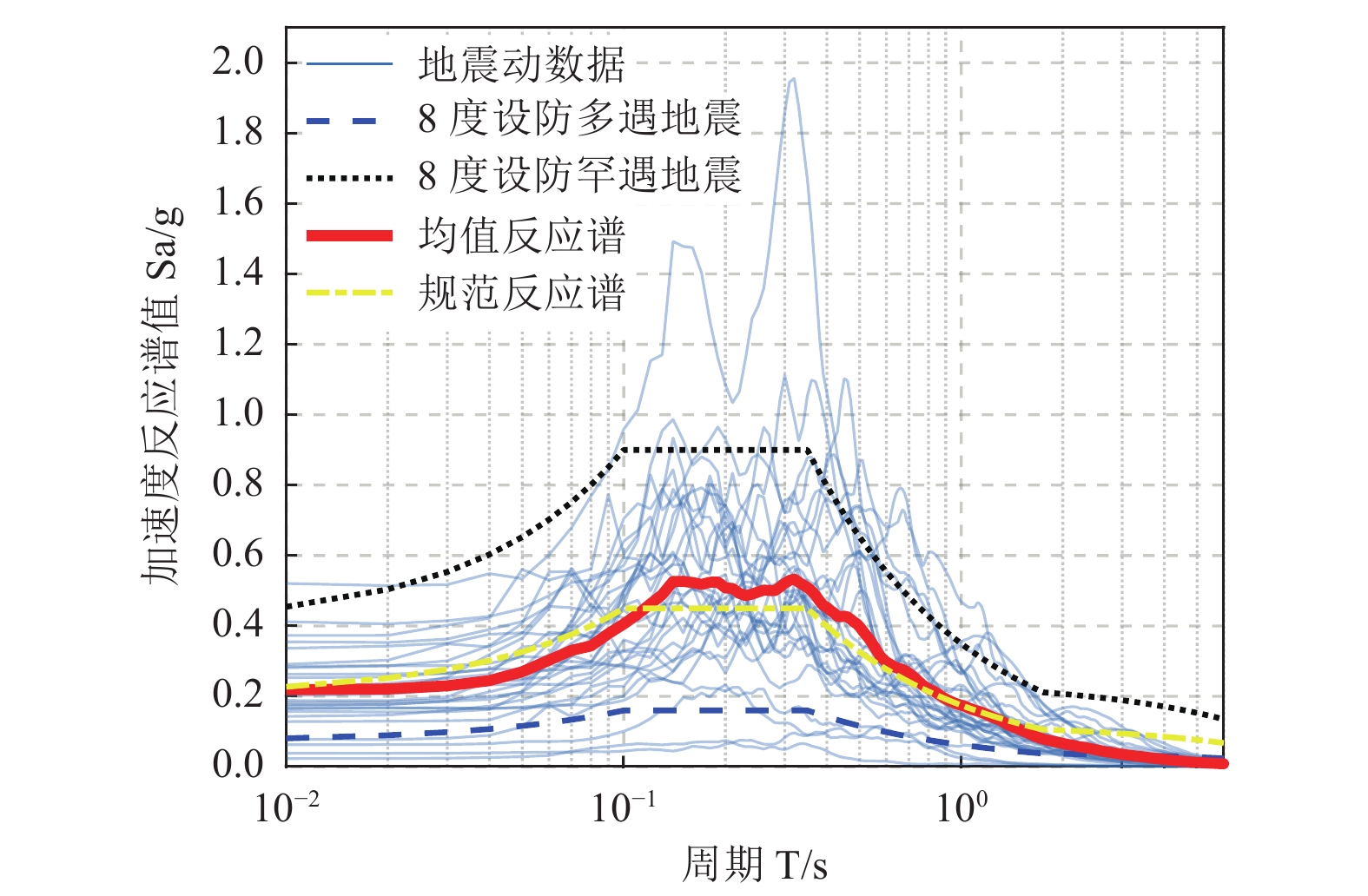
《建筑抗震设计规范》(GB 50011—2010)(中华人民共和国住房和城乡建设部等,2010)规定,在采用非线性时程分析方法进行抗震设计计算时,所选地震动记录的均值反应谱和设计规范谱应在统计意义上具有一致性。地震波均值谱与标准谱的对比如图4所示。
2.4 地震动的调幅与输入
本文基于增量动力分析法(IDA),将选取的30组地震动记录以峰值加速度为参数进行等间距调幅。将2条原始水平地震动分量中峰值加速度较大的分量定义为主分量,在研究双向水平地震动对桥梁地震易损性的影响时,将地震动调幅及输入分为单向、双向水平地震动时程输入。单向水平地震动时程输入时,首先将主分量从0.2 g到1.0 g进行等间距调幅,增量步长为0.2 g;然后逐级将调至不同峰值的单条加速度时程沿纵桥向输入,对桥梁进行结构需求分析计算,共150条工况。双向水平地震动时程输入时,首先将2条水平地震动分量从0.2 g到1.0 g进行等间距调幅,增量步长为0.2 g;然后固定每次沿横桥向输入地震动幅值,纵桥向地震动同单向水平地震动时程输入时,共750条工况。
3. 桥梁地震易损性分析
3.1 概率地震需求分析
沿横桥向每个墩柱均布置了单向滑动支座,其沿横桥向相当于固定支座的作用,因此横桥向地震惯性力由各墩共同承担。而沿纵桥向仅有中墩墩柱支座为固接,因此主梁纵向地震惯性力主要由中墩承担。由此可知,中墩墩柱截面是桥梁获得最大位移的控制截面。将经过调幅后的地震动分别沿纵桥向和横桥向输入,通过非线性时程分析得到桥梁地震需求反应,并提取控制截面峰值位移。
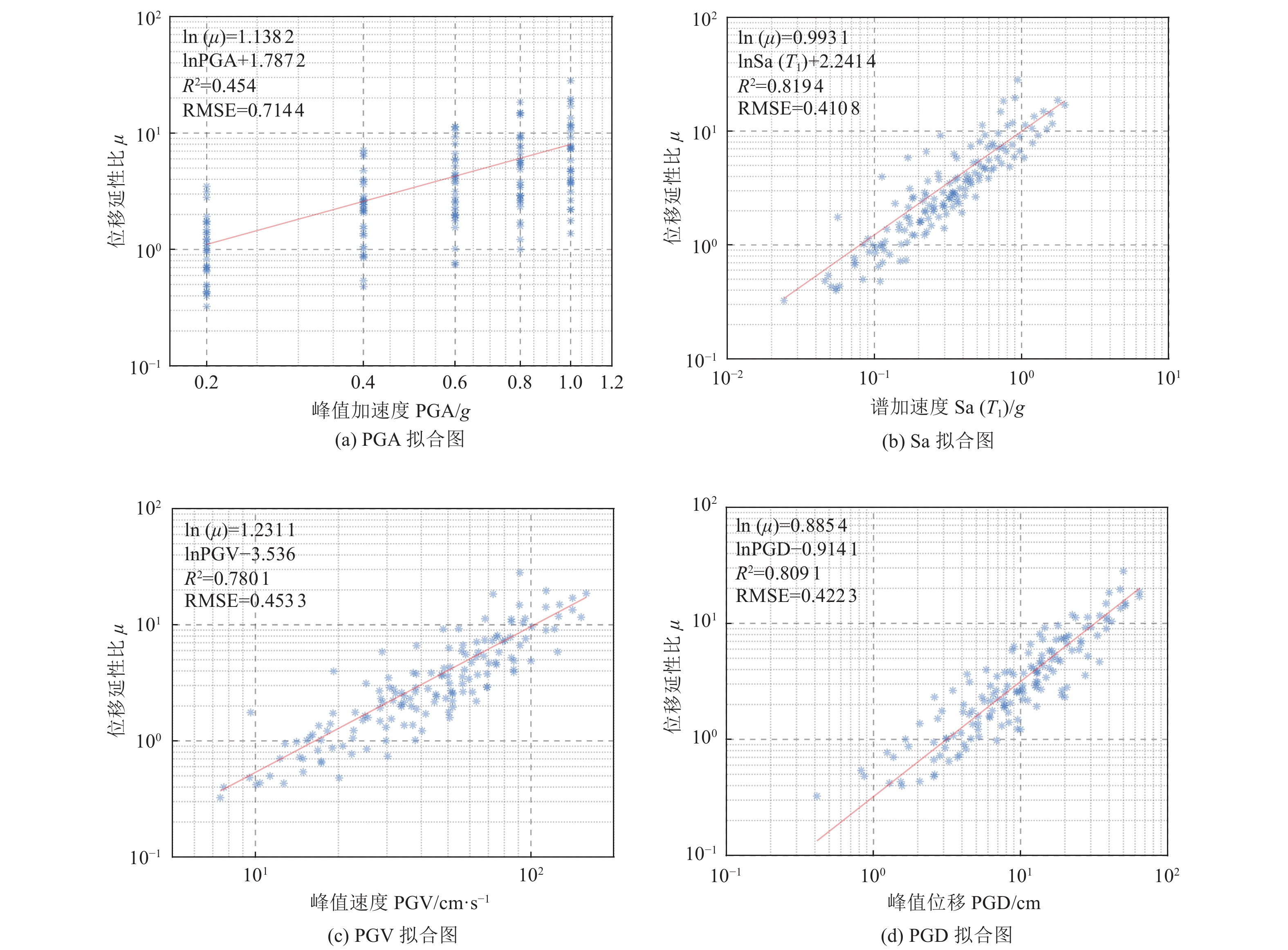
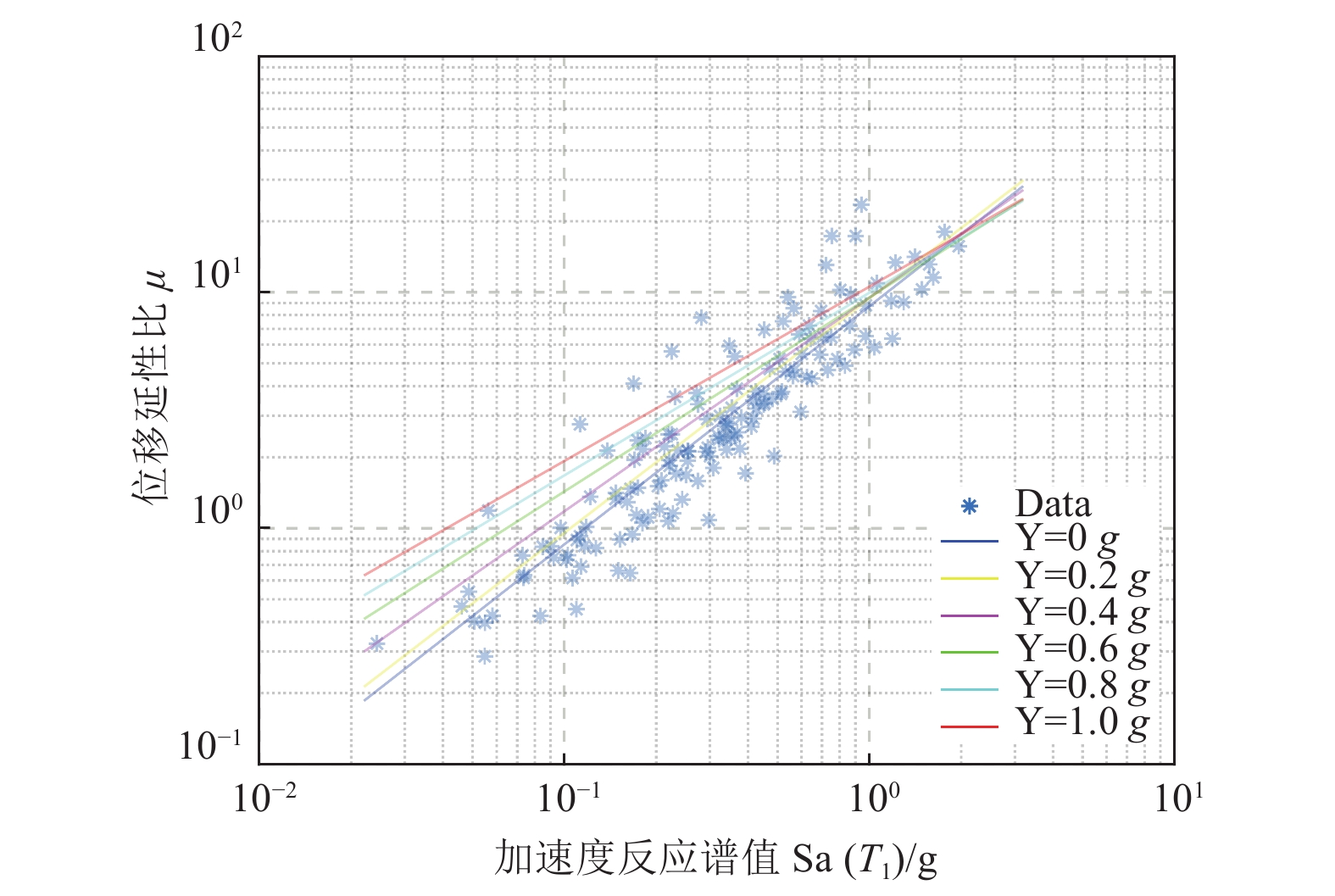
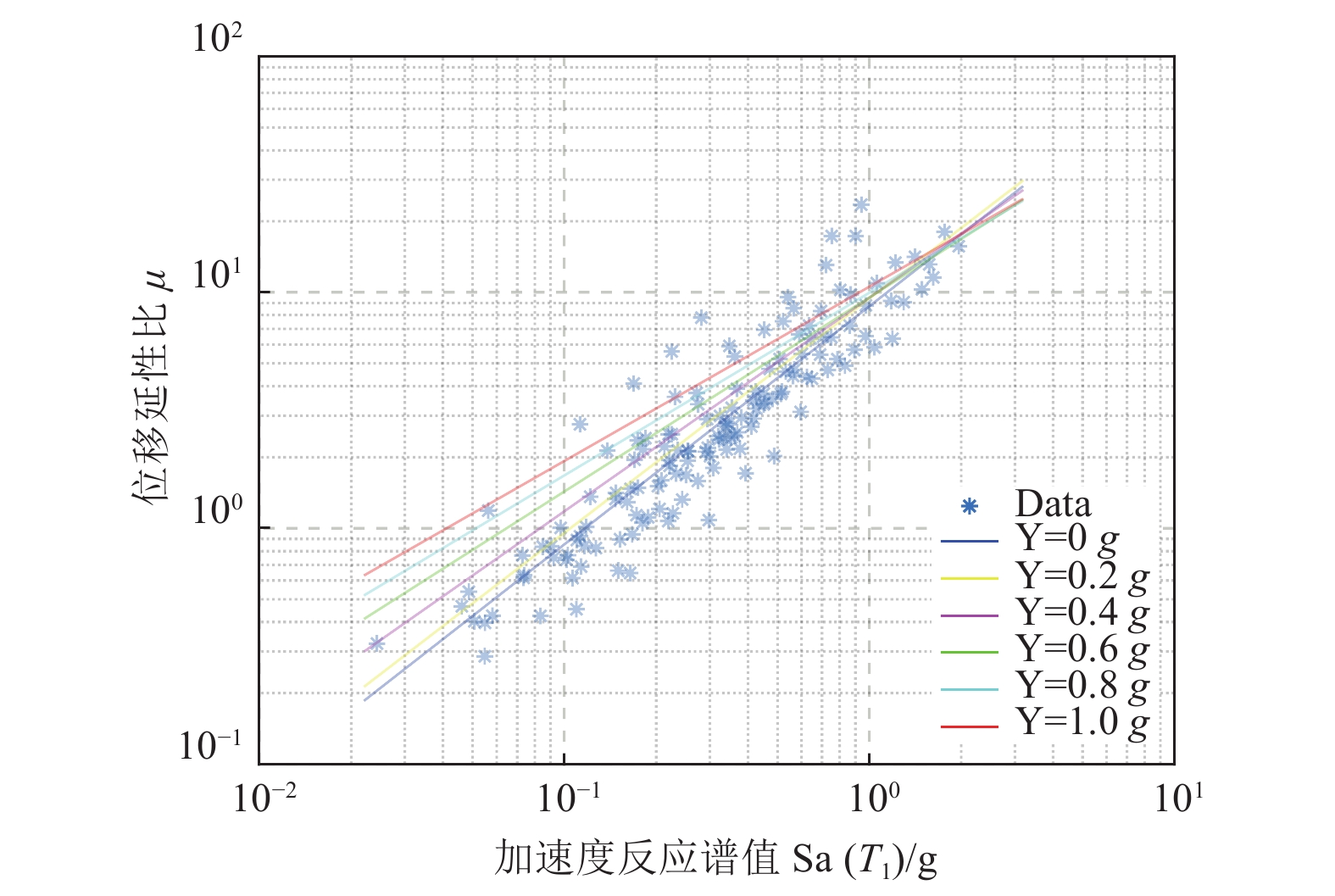
分别以地震动峰值加速度PGA、结构一阶周期对应的加速度反应谱值Sa(T1)、地震动峰值速度PGV、地震动峰值位移PGD作为地震动强度参数进行对数线性回归分析,得到地震动-桥梁系统的回归拟合结果,如图5所示。限于篇幅,图5中仅列举了双向水平地震动输入下Y=0.2 g(Y表示调幅后沿横桥向输入地震动峰值加速度)时的概率地震需求模型,其中μ为位移延性比,R2为相关系数,RMSE为均方根误差,其余工况下的拟合回归结果如表5、6所示。由表5、6可知,随着横桥向地震动的逐渐增大,不同强度参数的相关性系数不断减小,且均方根误差不断增大,这是由于随着横向荷载的增加,桥梁逐渐进入塑性阶段,保护层混凝土剥落后发生较大的塑性变形,造成离散程度增大;PGA相关性系数最小,回归均方根误差最大;以PGD和Sa(T1)为参数的回归曲线与得到的反应数据拟合最好,且离散程度最小。相对来说,目前以PGD为强度参数的应用相对较少;而对于Sa(T1)而言,反应谱加速度是单自由度结构体系在地震动作用下的最大响应,不仅反映了地震动特性,又反映了结构自身特性,本研究桥梁结构几何形状、固有频率和振型为已知,结构基本周期对应的加速度反应谱成为能够结合地震动特性和结构属性的地震动强度指标,因此,综合考虑地震动强度参数相关性和实用性,本文选取Sa(T1)作为地震动强度指标。
表 5 不同地震动强度参数的相关系数Table 5. Determination coefficients of different ground motion intensity parameters强度参数 Y=0 g Y=0.2 g Y=0.4 g Y=0.6 g Y=0.8 g Y=1.0 g Sa(T1) 0.837 5 0.819 4 0.763 8 0.716 5 0.683 1 0.657 5 PGA 0.485 7 0.454 0 0.419 8 0.373 6 0.336 9 0.301 7 PGV 0.813 5 0.780 1 0.714 6 0.659 5 0.611 8 0.572 2 PGD 0.813 7 0.809 1 0.769 8 0.739 1 0.714 0 0.693 9 表 6 不同地震动强度参数的均方根误差Table 6. Root mean square error of different ground motion intensity parameters强度参数 Y=0 g Y=0.2 g Y=0.4 g Y=0.6 g Y=0.8 g Y=1.0 g Sa(T1) 0.391 8 0.410 8 0.443 7 0.455 9 0.464 7 0.469 9 PGA 0.697 0 0.714 4 0.695 3 0.677 7 0.672 3 0.670 9 PGV 0.419 7 0.453 3 0.487 7 0.499 7 0.514 4 0.525 1 PGD 0.419 5 0.423 3 0.438 0 0.437 4 0.441 4 0.444 2 3.2 双向水平地震动输入易损性分析
将双向水平地震动分别沿纵桥向和横桥向输入,通过非线性时程分析得到桥梁最大结构响应,通过对桥梁地震需求参数和地震强度参数进行对数线性回归拟合,得到桥梁在双向水平地震动输入下的概率地震需求模型,由于数据点过多,仅展示Y=0 g的数据点,如图6所示。
基于桥梁的概率需求分析及破坏准则,根据易损性定义计算桥梁在不同地震水平下的损伤概率,形成桥梁构件易损性关系,如图7所示,图中Sa(T1a)和Sa(T1b)分别表示调幅后沿纵桥向和横桥向结构自振周期对应的加速度反应谱值,P表示桥梁在特定地震动强度下发生不同破坏状态的概率。
(1) 在Y=0 g的条件下(仅沿纵桥向输入水平地震动),桥梁发生不同破坏状态的超越概率最小,说明双向水平地震动作用下桥梁响应大于单向地震动作用下。
(2) 在Y=1 g的条件下,桥梁发生不同破坏状态的超越概率最大,表明2个主轴方向上的耦合关系对桥梁在地震作用下的响应有放大作用,随着横桥向输入地震动的不断增加,在某一相同破坏状态下桥梁的超越概率不断增大。
(3) 通过将不同破坏状态的地震易损性曲线进行对比,可知轻微破坏曲线和中等破坏曲线之间的区别较小,严重破坏曲线和中等破坏曲线之间存在较大差距,这是由于轻微破坏和中等破坏损伤指标较接近(μcy1=1,μcy=1.294),而桥墩在进入中等破坏后产生了塑性铰,使桥墩具有一定延性,因此二者之间的差距变大。
4. 结语
本文以城市交通网络中常见的规则钢筋混凝土连续箱梁桥为例,选取30组不同地震事件的天然水平地震动,通过增量动力分析法进行非线性时程分析,研究了双向水平地震动输入对桥梁地震易损性的影响,得到以下结论:
(1) 桥梁结构地震响应与参数Sa(T1)、PGA、PGV、PGD的回归分析结果表明,结构地震响应与Sa(T1)之间关系的离散性相对较小,拟合优度最好,即Sa(T1)作为地震动强度指标可相对较好地揭示地震动对桥梁结构的破坏作用,以Sa(T1)作为易损性分析的输入参数可增加分析结果的可靠性。
(2) 在单向和双向水平地震动输入下,对桥梁进行非线性时程分析,分别建立了桥梁结构基于双向输入加速度反应谱值的概率地震需求模型及易损性曲面。结果表明,双向水平地震动输入下的桥梁结构响应及易损性明显大于纵桥向单向地震动输入的情况。随着横桥向输入地震动峰值加速度由0增至1 g,轻微破坏状态的超越概率最大增加76%,中等破坏状态的超越概率最大增加70%,严重破坏状态的超越概率最大增加40%,完全破坏状态的超越概率最大增加32%。
(3) 本研究结果表明双向水平地震动输入对桥梁易损性影响较大,因此,为更好地反映结构真实损伤状态,在进行桥梁抗震设计与抗震性能评估时,须考虑双向或三向地震作用对桥梁的破坏,否则可能会低估地震对桥梁的损伤水平。
-
表 1 支座参数
Table 1. Parameter of bearings
支座类型 支座型号 支座位置 盆式固定支座 GPZ4000GX 3 盆式单向滑动支座 GPZ4000DX 1、2、4、5(上) 盆式双向滑动支座 GPZ4000SX 1、2、4、5(下) 表 2 损伤破坏准则
Table 2. Damage failure criterion
损伤状态 基本完好 轻微破坏 中等破坏 严重破坏 完全破坏 破坏准则 0<μ≤μcy1 μcy1<μ≤μcy μcy<μ≤μc4 μc4<μ≤μcmax μcmax≤μ 表 3 桥墩截面弯矩-曲率关系(单位:rad·m−1)
Table 3. Moment- curvature relationship of pier section(Unit: rad·m−1)
分析参数 фy1 фy фc4 фu 桥墩截面曲率 2.353 3.045 18.390 50.630 表 4 损伤指标与损伤等级关系
Table 4. Relationship between damage index and damage grade
损伤状态 基本完好 轻微破坏 中等破坏 严重破坏 完全破坏 破坏准则 0<μ≤1.00 1.00<μ≤1.29 1.29<μ≤3.34 3.34<μ≤6.34 6.34<μ 表 5 不同地震动强度参数的相关系数
Table 5. Determination coefficients of different ground motion intensity parameters
强度参数 Y=0 g Y=0.2 g Y=0.4 g Y=0.6 g Y=0.8 g Y=1.0 g Sa(T1) 0.837 5 0.819 4 0.763 8 0.716 5 0.683 1 0.657 5 PGA 0.485 7 0.454 0 0.419 8 0.373 6 0.336 9 0.301 7 PGV 0.813 5 0.780 1 0.714 6 0.659 5 0.611 8 0.572 2 PGD 0.813 7 0.809 1 0.769 8 0.739 1 0.714 0 0.693 9 表 6 不同地震动强度参数的均方根误差
Table 6. Root mean square error of different ground motion intensity parameters
强度参数 Y=0 g Y=0.2 g Y=0.4 g Y=0.6 g Y=0.8 g Y=1.0 g Sa(T1) 0.391 8 0.410 8 0.443 7 0.455 9 0.464 7 0.469 9 PGA 0.697 0 0.714 4 0.695 3 0.677 7 0.672 3 0.670 9 PGV 0.419 7 0.453 3 0.487 7 0.499 7 0.514 4 0.525 1 PGD 0.419 5 0.423 3 0.438 0 0.437 4 0.441 4 0.444 2 -
[1] 陈力波, 郑凯锋, 庄卫林等, 2012. 汶川地震桥梁易损性分析. 西南交通大学学报, 47(4): 558—566 doi: 10.3969/j.issn.0258-2724.2012.04.004Chen L. B. , Zheng K. F. , Zhuang W. L. , et al. , 2012. Analytical investigation of bridge seismic vulnerability in wenchuan earthquake. Journal of Southwest Jiaotong University, 47(4): 558—566. (in Chinese) doi: 10.3969/j.issn.0258-2724.2012.04.004 [2] 谷音, 李晓芳, 2019. 考虑氯离子侵蚀的近海桥梁结构地震易损性分析. 应用基础与工程科学学报, 27(5): 1019—1032Gu Y. , Li X. F. , 2019. Seismic fragility analysis of offshore bridge structure considering chloride erosion. Journal of Basic Science and Engineering, 27(5): 1019—1032. (in Chinese) [3] Hwang H, 刘晶波, 2004. 地震作用下钢筋混凝土桥梁结构易损性分析. 土木工程学报, 37(6): 47—51 doi: 10.3321/j.issn:1000-131X.2004.06.009Hwang H, 刘晶波, 2004. 地震作用下钢筋混凝土桥梁结构易损性分析. 土木工程学报, 37(6): 47—51. Hwang H. , Liu J. B. , 2004. Seismic fragility analysis of reinfo钢筋混凝土ed concrete bridges. China Civil Engineering Journal, 37(6): 47—51. (in Chinese) doi: 10.3321/j.issn:1000-131X.2004.06.009 [4] 姬淑艳, 李英民, 刘立平等, 2006. 考虑双向水平地震作用的结构设计问题研究. 地震工程与工程振动, 26(5): 68—72 doi: 10.3969/j.issn.1000-1301.2006.05.011Ji S. Y. , Li Y. M. , Liu L. P. , et al. , 2006. Study on bi-directional seismic action in structural seismic design. Earthquake Engineering and Engineering Vibration, 26(5): 68—72. (in Chinese) doi: 10.3969/j.issn.1000-1301.2006.05.011 [5] 李立峰, 吴文朋, 胡思聪等, 2016. 考虑氯离子侵蚀的高墩桥梁时变地震易损性分析. 工程力学, 33(1): 163—170 doi: 10.6052/j.issn.1000-4750.2014.06.0530Li L. F. , Wu W. P. , Hu S. C. , et al. , 2016. Time-dependent seismic fragility analysis of high pier bridge based on chloride ion induced corrosion. Engineering Mechanics, 33(1): 163—170. (in Chinese) doi: 10.6052/j.issn.1000-4750.2014.06.0530 [6] 李勇, 闫维明, 刘晶波等, 2018. 近断层高架连续梁桥地震易损性与振动台试验研究. 工程力学, 35(4): 52—60, 86 doi: 10.6052/j.issn.1000-4750.2017.03.0236Li Y. , Yan W. M. , Liu J. B. , et al. , 2018. Seismic vulnerability analysis and a shaking table test of a near-fault continuous viaduct. Engineering Mechanics, 35(4): 52—60, 86. (in Chinese) doi: 10.6052/j.issn.1000-4750.2017.03.0236 [7] 林庆利, 林均岐, 刘金龙, 2017. 汶川地震公路桥梁易损性研究. 振动与冲击, 36(4): 110—118, 126Lin Q. L. , Lin J. Q. , Liu J. L. , 2017. A study on the fragility of highway bridges in the Wenchuan earthquake. Journal of Vibration and Shock, 36(4): 110—118, 126. (in Chinese) [8] 刘洋, 吕大刚, 于晓辉, 2016. 近场地震作用下型钢-混凝土组合结构桥易损性分析. 土木工程学报, 49(S1): 56—60, 77Liu Y. , Lv D. G. , Yu X. H. , 2016. Seismic fragility analysis of steel-concrete composite bridges excited by near-fault ground motions. China Civil Engineering Journal, 49(S1): 56—60, 77. (in Chinese) [9] 宋帅, 王帅, 吴刚等, 2020. 中小跨径梁桥地震易损性研究. 振动与冲击, 39(9): 118—125Song S. , Wang S. , Wu G. , et al. , 2020. Seismic vulnerability analysis of small and medium span girder bridges. Journal of Vibration and Shock, 39(9): 118—125. (in Chinese) [10] 徐超, 温增平, 2017. 不同设防标准钢筋混凝土框架结构基于易损性分析的抗震性能评估. 震灾防御技术, 12(4): 845—857 doi: 10.11899/zzfy20170413Xu C. , Wen Z. P. , 2017. Seismic performance evaluation of 钢筋混凝土 frames with different seismic precautionary intensity based on vulnerability analysis. Technology for Earthquake Disaster Prevention, 12(4): 845—857. (in Chinese) doi: 10.11899/zzfy20170413 [11] 易方民, 高小旺, 张维嶽等, 2003. 高层建筑钢结构在多维地震动输入作用下的反应. 建筑结构学报, 24(3): 33—43 doi: 10.3321/j.issn:1000-6869.2003.03.005Yi F. M. , Gao X. W. , Zhang W. Y. , et al. , 2003. Response of tall building steel structure to multi dimensional seismic action. Journal of Building Structures, 24(3): 33—43. (in Chinese) doi: 10.3321/j.issn:1000-6869.2003.03.005 [12] 张菊辉, 2006. 基于数值模拟的规则梁桥墩柱的地震易损性分析. 上海: 同济大学.Zhang J. H., 2006. Study on seismic vulnerability analysis of normal beam bridge piers based on numerical simulation. Shanghai: Tongji University. (in Chinese) [13] 中华人民共和国交通运输部, 2015. JTG D60-2015 公路桥涵设计通用规范. 北京: 人民交通出版社.Ministry of Transport of the People's Republic of China, 2015. JTG D60-2015 General specifications for design of highway bridges and culverts. Beijing: China Communications Press. (in Chinese) [14] 中华人民共和国交通运输部, 2019. JT/T 391—2019 公路桥梁盆式支座. 北京: 人民交通出版社.Ministry of Transport of the People's Republic of China, 2019. JT/T 391—2019 Pot bearing for highway bridge. Beijing: China Communications Press. (in Chinese) [15] 中华人民共和国住房和城乡建设部, 中华人民共和国国家质量监督检验检疫总局, 2010. GB 50011—2010 建筑抗震设计规范. 北京: 中国建筑工业出版社.Ministry of Housing and Urban-Rural Development of the People's Republic of China, General Administration of Quality Supervision, Inspection and Quarantine of the People's Republic of China, 2010. GB 50011—2010 Code for seismic design of buildings. Beijing: China Architecture & Building Press. (in Chinese) [16] 周长东, 田苗旺, 张许等, 2017. 考虑多维地震作用的高耸钢筋混凝土烟囱结构易损性分析. 土木工程学报, 50(3): 54—64Zhou C. D. , Tian M. W. , Zhang X. , et al. , 2017. Seismic fragility analysis for high-rise 钢筋混凝土 chimney considering multi-dimensional seismic actions. China Civil Engineering Journal, 50(3): 54—64. (in Chinese) [17] Cornell C. A. , Jalayer F. , Hamburger R. O. , 2002. Probabilistic basis for 2000 SAC federal emergency management agency steel moment frame guidelines. Journal of Structural Engineering, 128(4): 526—533. doi: 10.1061/(ASCE)0733-9445(2002)128:4(526) [18] Federal Emergency Management Agency (FEMA), 2001. HAZUS 99 Estimated annualized earthquake losses for the United States. Washington: FEMA. [19] Shinozuka M. , Feng M. Q. , Lee J. , et al. , 2000. Statistical analysis of fragility curves. Journal of Engineering Mechanics, 126(12): 1224—1231. doi: 10.1061/(ASCE)0733-9399(2000)126:12(1224) [20] Shome N., 1999. Probabilistic seismic demand analysis of nonlinear structures. Stanford: Stanford University. [21] Yamazaki F., Motomura H., Hamada T., 2000. Damage assessment of expressway networks in Japan based on seismic monitoring. In: Proceeding of the 12th World Conference on Earthquake Engineering, Upper Hutt, New Zealand: WCEE, 0551. -




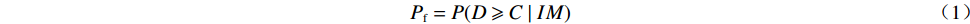
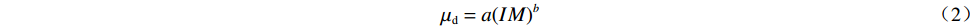
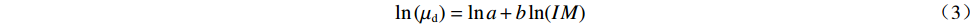
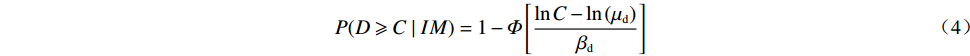
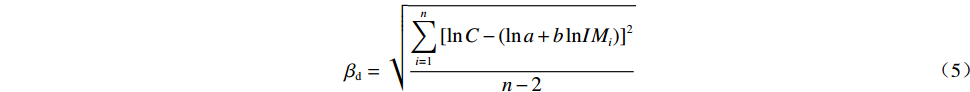
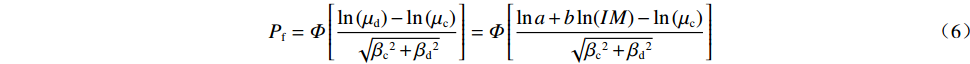
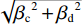
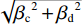

 下载:
下载:








 下载:
下载: