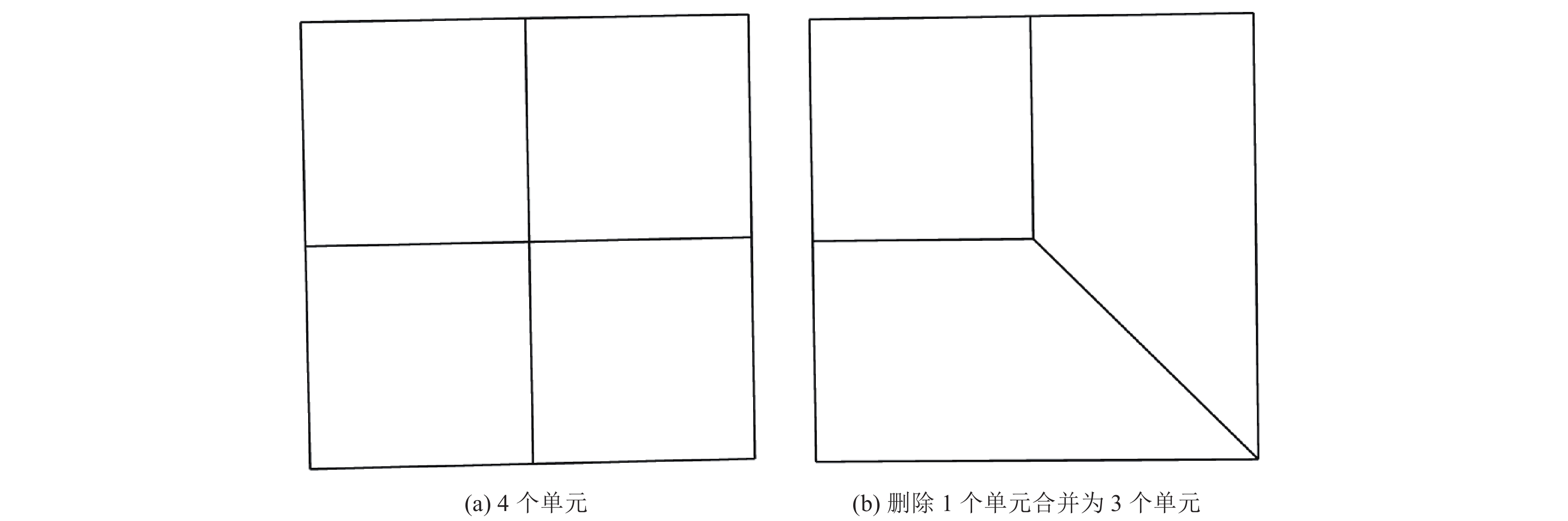
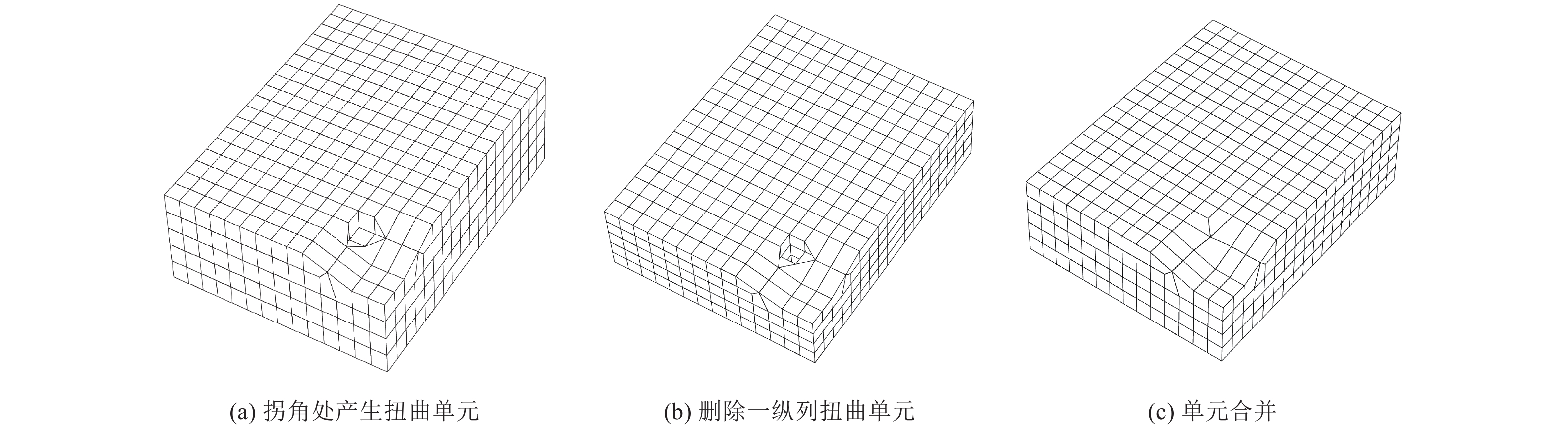
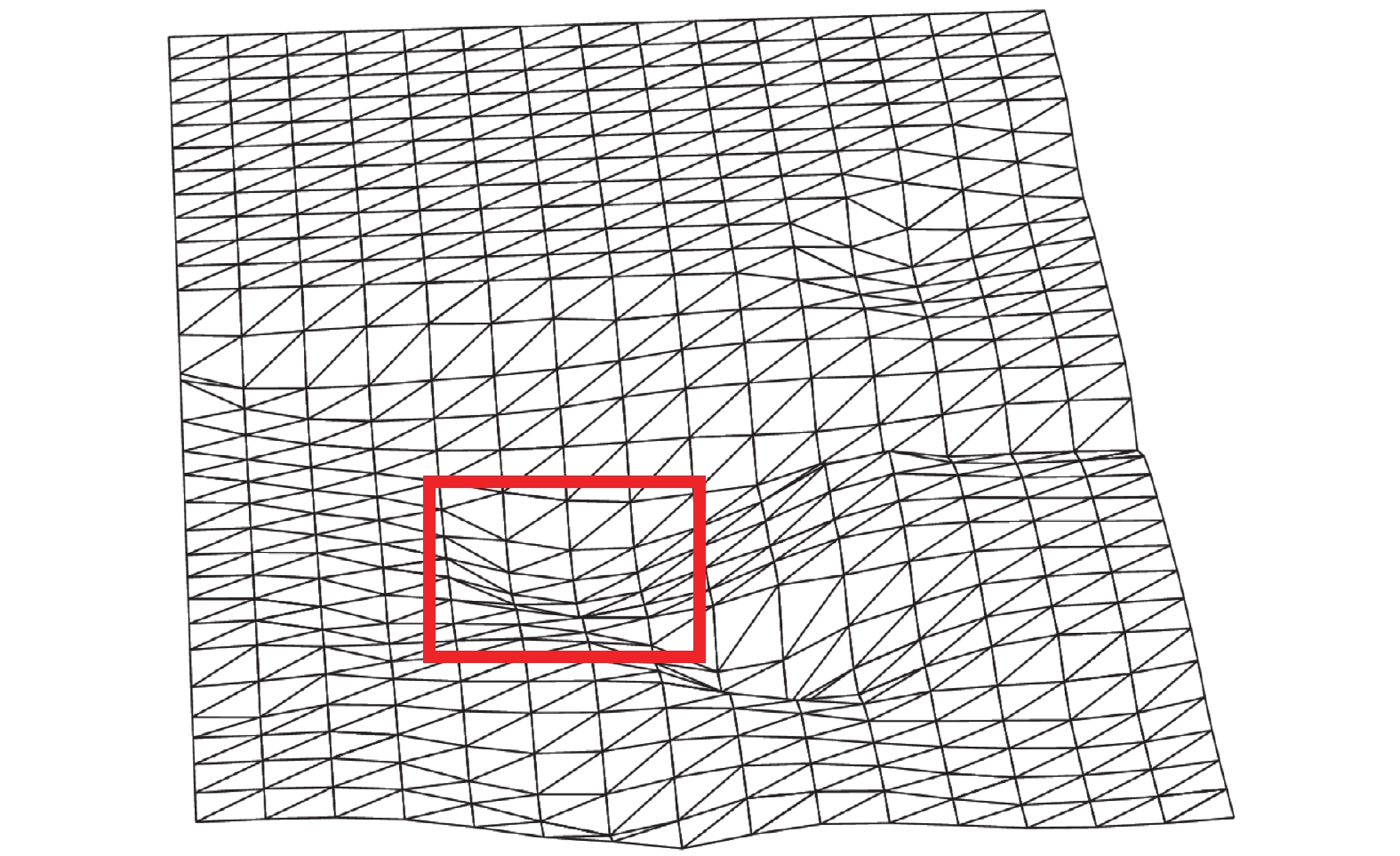
Accurate Mesh Generation of Undulating Interface Based on Truegrid and the Ground Motion Simulation
-
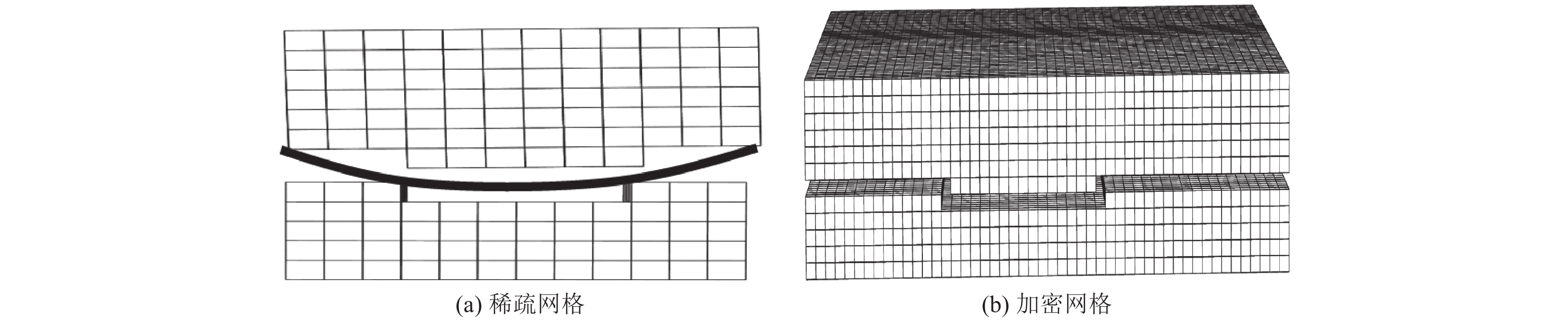
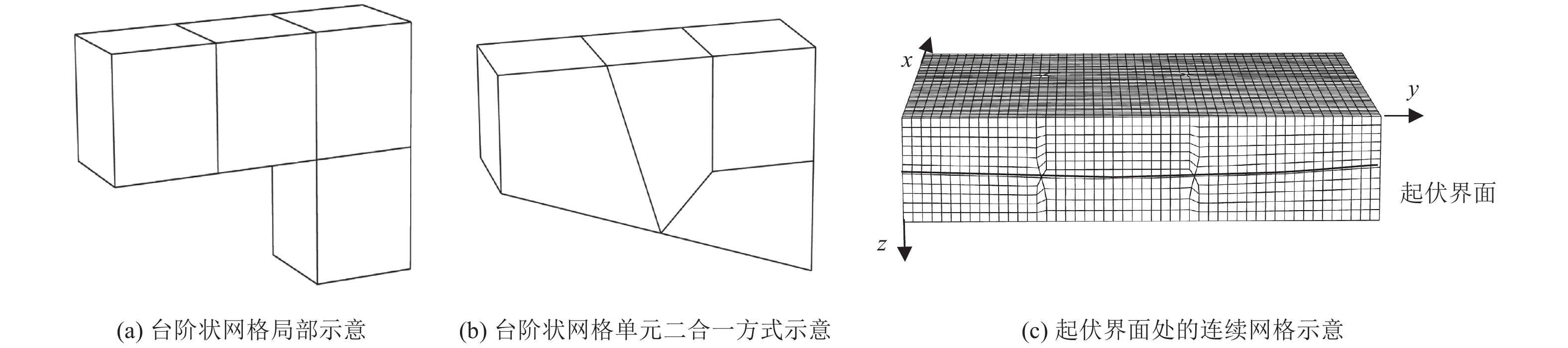
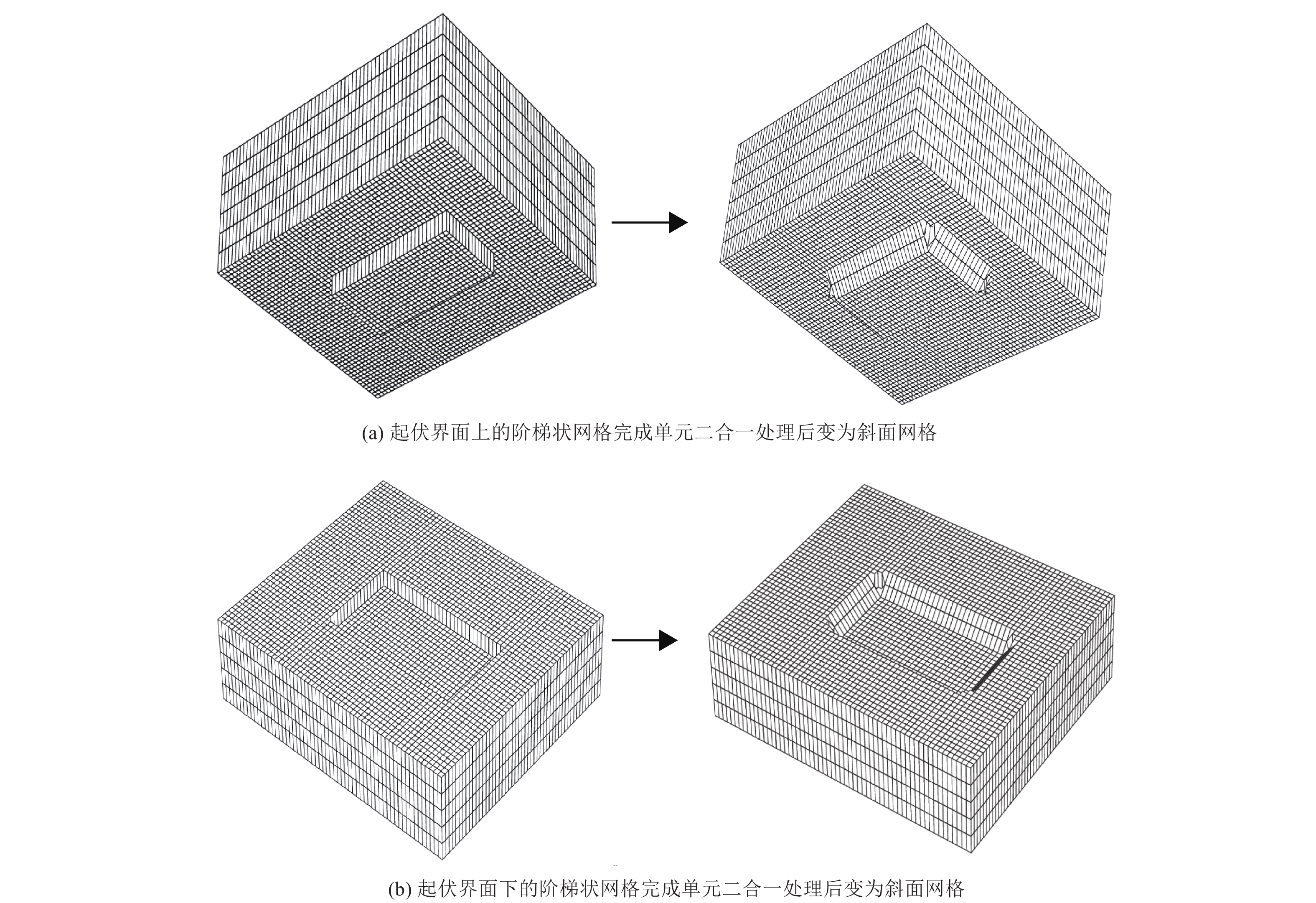
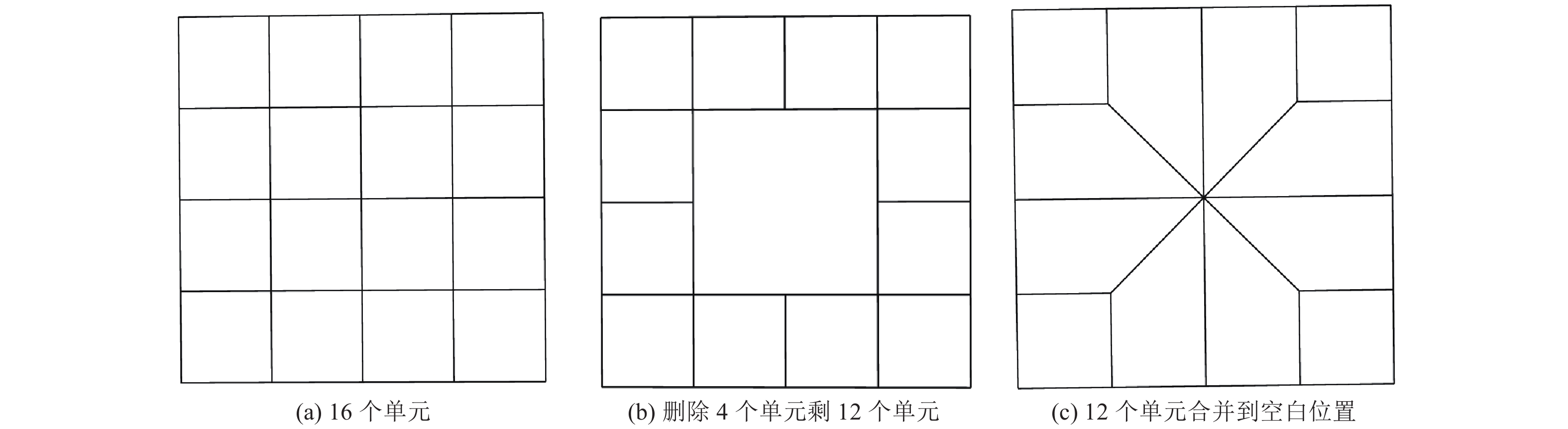
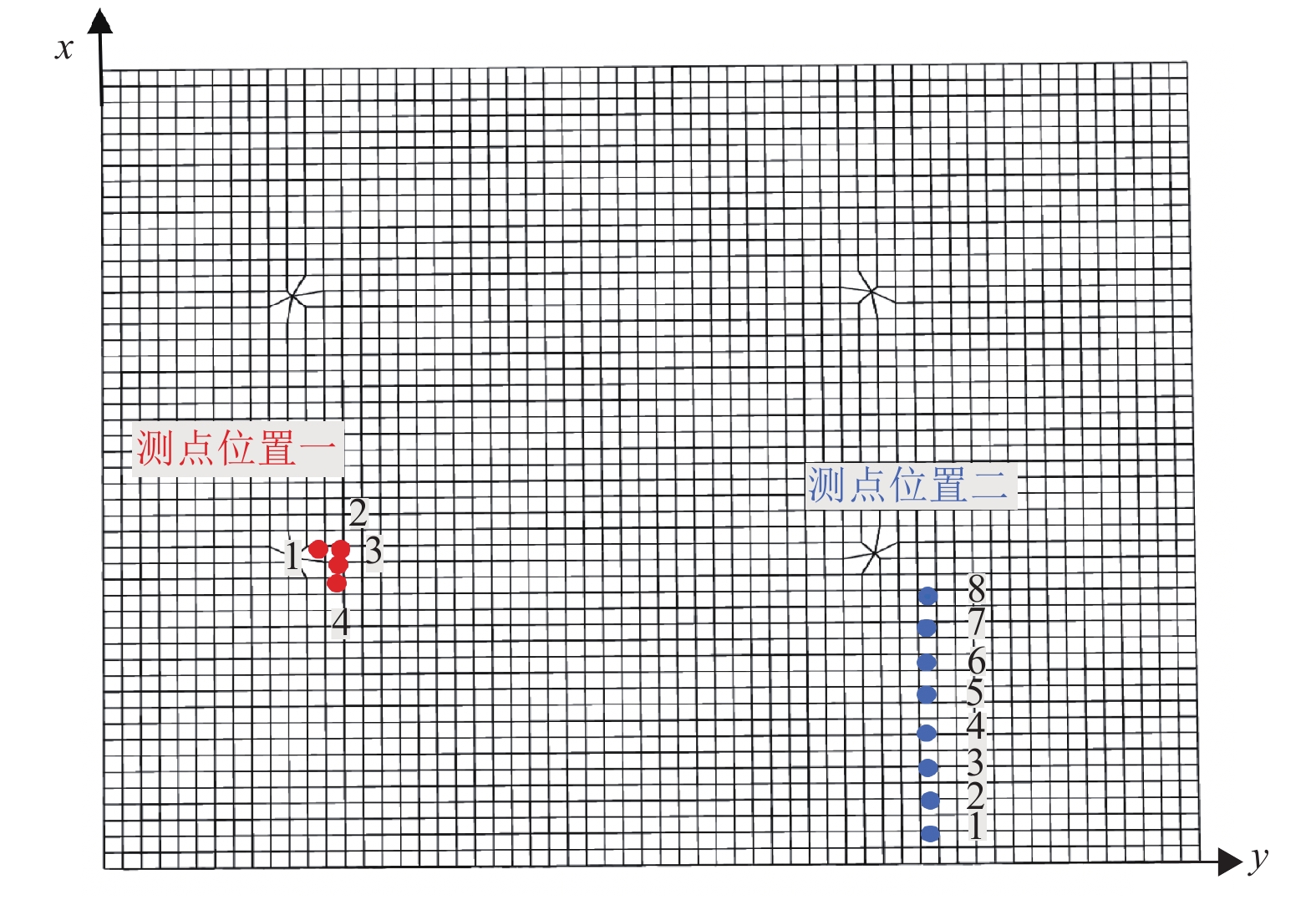
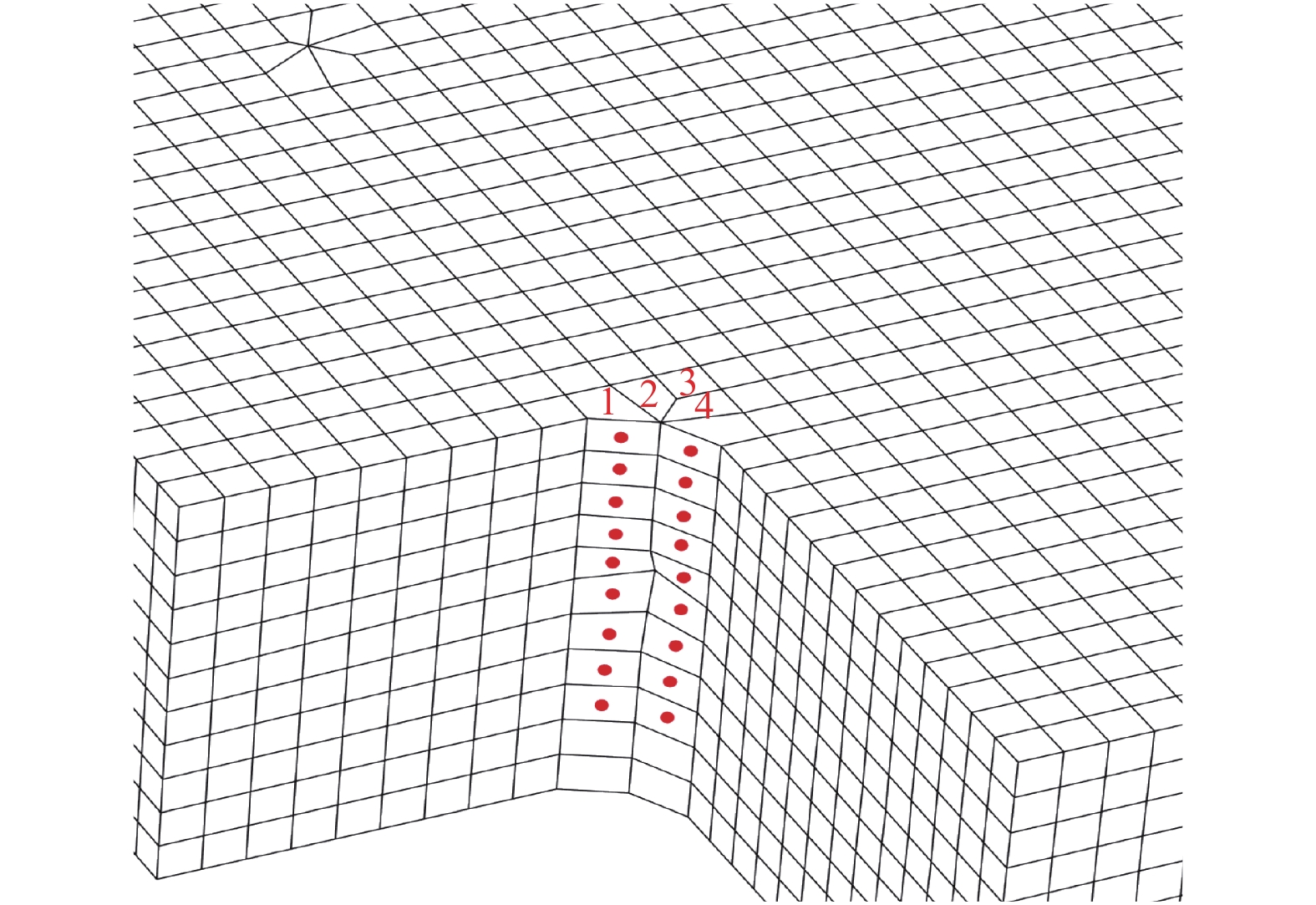
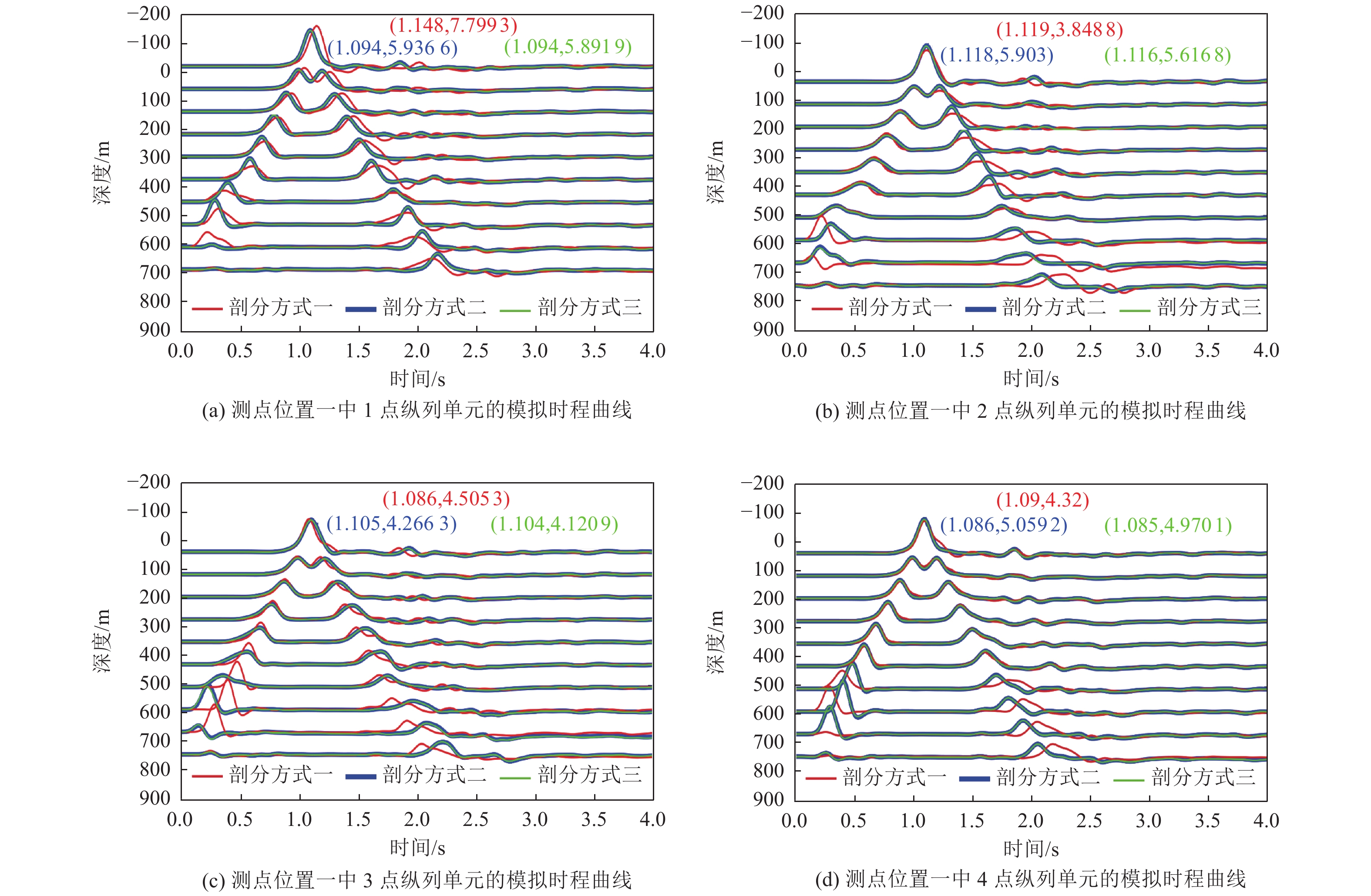
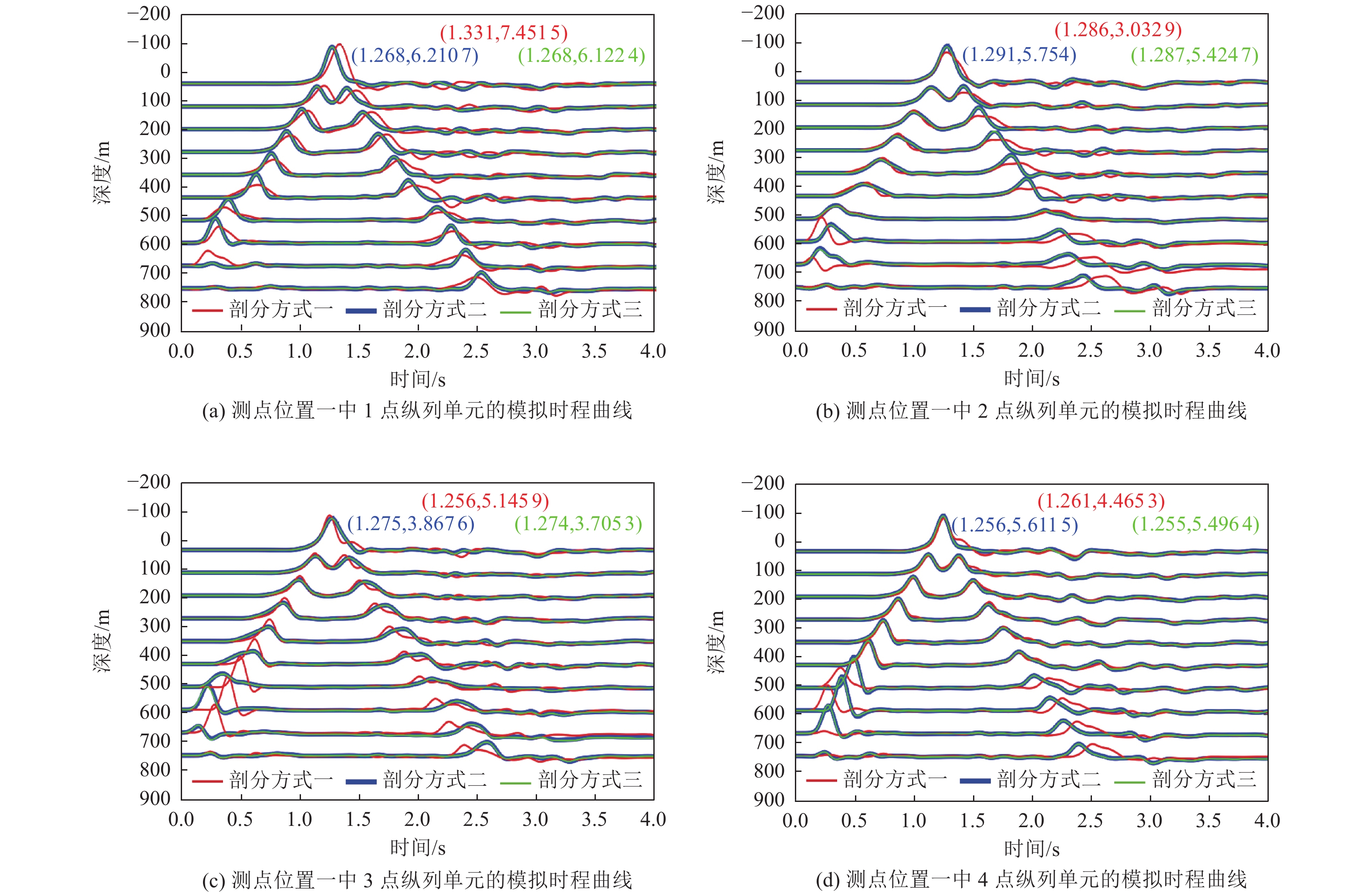
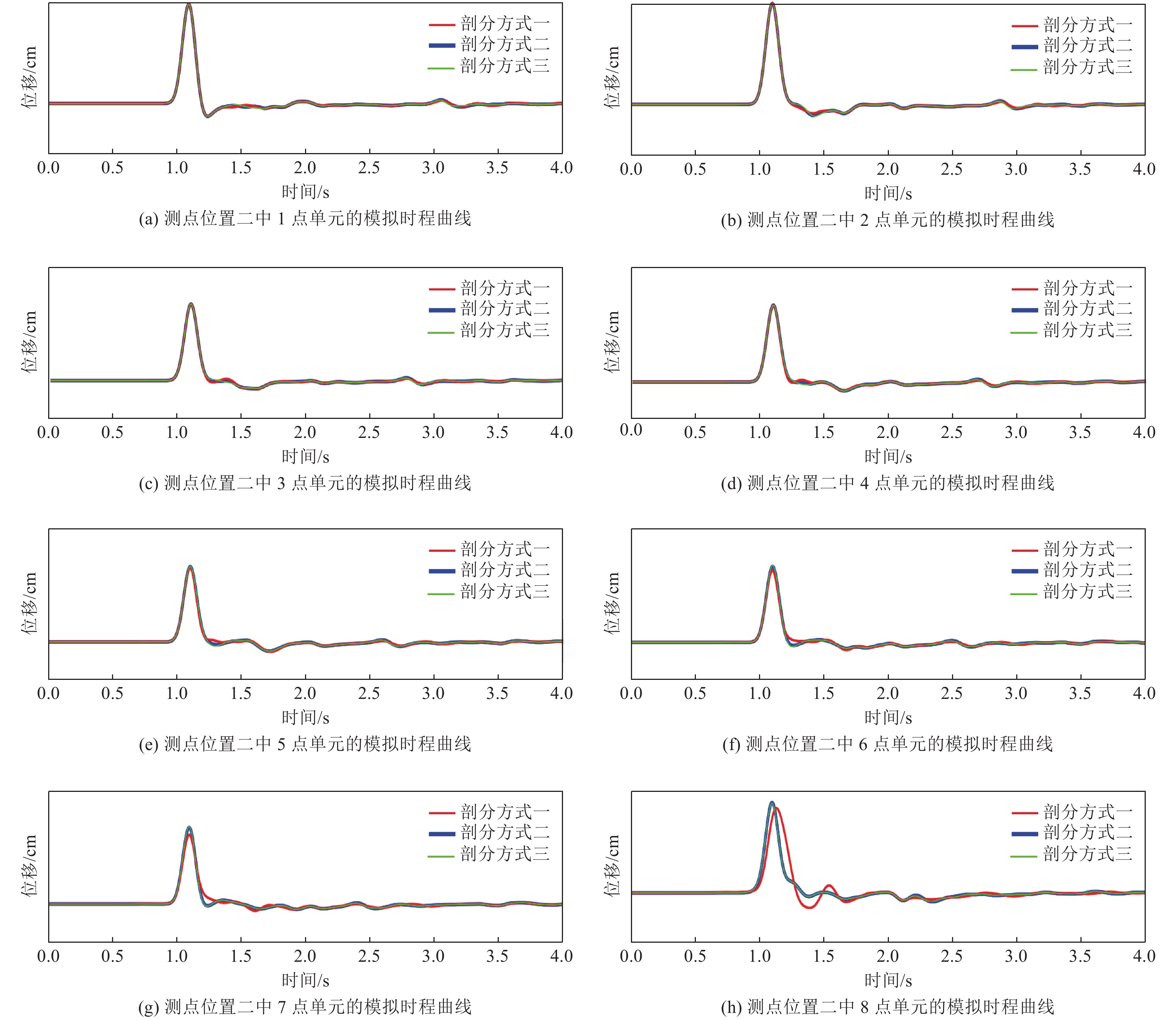
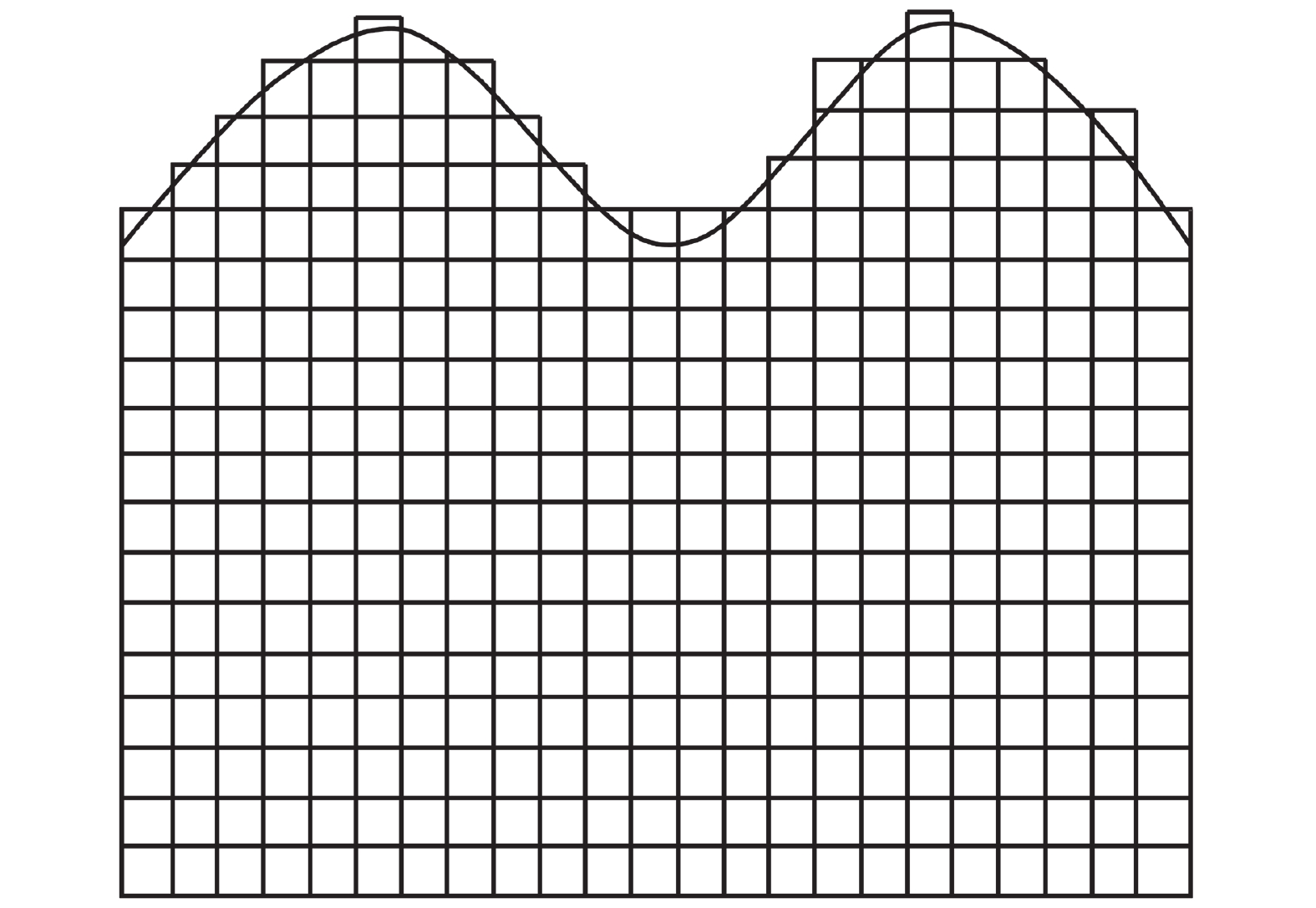
摘要: 利用谱元法的规则六面体单元进行网格剖分时，界面起伏较大处会出现阶梯状网格而导致模拟时产生数值散射。为消除阶梯状网格对起伏界面地震动模拟计算的影响，本文基于TrueGrid软件编写了应用程序，提出了起伏界面处六面体单元网格剖分方式，通过该程序可快速建立起伏界面处均匀的六面体网格模型。本文采取了删除四纵列拐角单元、删除一纵列拐角单元以及构造单元过渡环3种剖分方式，解决两个方向上（x-z与y-z方向）单元二合一过渡后拐角处产生扭曲单元的问题。将阶梯状网格经二合一处理后变为斜面网格，并投影至起伏界面，使得网格完全贴合起伏界面，改善了用台阶状网格近似描绘起伏界面的问题。将3种模型通过谱元法进行数值模拟计算验证了该剖分方式的正确性,对比结果发现删除拐角处一纵列单元方式与设置过渡环的方式均可使用，删除四纵列拐角单元方式不推荐使用，本文提出的方案有助于提高谱元法处理起伏界面问题的灵活性。Abstract: When the regular hexahedral element of the spectral element method is used for meshing, a stepped grid will appear where the interface fluctuates greatly, which leads to numerical scattering during simulation. In order to eliminate the influence of the stepped grid on the ground motion simulation calculation of the undulating interface, an application program is written based on the TrueGrid software, and a method of meshing hexahedral element at the undulating interface is proposed. By using this program, a uniform hexahedral mesh model at the undulating interface can be quickly established. This paper adopts three meshing methods: deleting four-column corner elements, deleting one-column corner elements, and constructing element transition rings to solve the problem of twisting elements at the corners after the two-in-one transition of the elements in the two directions (x-z and y-z directions). The stepped grid is transformed into an inclined grid after two-in-one processing, and projected to the undulating interface, so that the grid fits the undulating interface completely, and the problem of using a stepped grid to approximate the undulating interface is improved. Numerical simulations of the three models verify the correctness of the subdivision method. Comparing the results, it is found that both the method of deleting one column of elements at the corner and the method of setting a transition ring are effective,but the method of deleting four columns of corner elements is not recommended. The schemes proposed in this paper can help to improve the flexibility of the spectral element method to deal with undulating interface problems.
-
Key words:
- Mesh generation /
- Hexahedral element /
- Spectral element method /
- Numerical simulation
-
1. 2020年中国地震概况
2020年，中国境内共发生5.0级及以上地震28次（中国大陆地区发生20次，台湾地区及海域地区发生8次），其中5.0～5.9级地震25次，6.0～6.9级地震3次，最大地震为2020年7月23日发生在西藏那曲市尼玛县的6.6级地震（表1、图1）。2020年中国大陆发生的20次5.0级以上地震中有10次发生在新疆、6次发生在西藏，6.0级以上地震中有2次发生在新疆、1次发生在西藏。
表 1 2020年中国5.0级及以上地震事件目录及成灾事件Table 1. Catalogue of earthquakes (MS≥5.0) and disaster - causing events in China, 2020序号 时间 纬度/° 经度/° 震中位置 震级/M 成灾事件 1 2020-01-16 16:32:38 41.21 83.6 新疆阿克苏地区库车市 5.6 (1) 2 2020-01-18 00:05:50 39.83 77.18 新疆喀什地区伽师县 5.4 3 2020-01-19 21:27:55 39.83 77.21 新疆喀什地区伽师县 6.4 (2) 4 2020-01-19 22:23:01 39.89 77.46 新疆克孜勒苏州阿图什市 5.2 5 2020-01-25 06:56:05 31.98 95.09 西藏昌都市丁青县 5.1 6 2020-01-29 07:39:29 27.16 126.6 东海海域 5.3 7 2020-02-03 00:05:41 30.74 104.46 四川成都市青白江区 5.1 8 2020-02-15 19:00:07 23.95 121.49 台湾花莲县 5.4 9 2020-02-21 02:01:40 34.56 85.68 西藏阿里地区改则县 5.0 10 2020-02-21 23:39:14 39.87 77.47 新疆喀什地区伽师县 5.1 11 2020-03-10 02:12:11 32.84 85.52 西藏阿里地区改则县 5.0 12 2020-03-12 23:44:03 32.88 85.55 西藏阿里地区改则县 5.1 13 2020-03-20 09:33:15 28.63 87.42 西藏日喀则市定日县 5.9 14 2020-03-23 03:21:39 41.75 81.11 新疆阿克苏地区拜城县 5.0 15 2020-04-01 20:23:27 33.04 98.92 四川甘孜州石渠县 5.6 (3) 16 2020-05-03 11:24:40 23.29 121.6 台湾台东县海域 5.4 17 2020-05-06 18:51:00 39.71 74.1 新疆克孜勒苏州乌恰县 5.0 18 2020-05-09 23:35:59 40.77 78.76 新疆阿克苏地区柯坪县 5.2 19 2020-05-18 21:47:59 27.18 103.16 云南昭通市巧家县 5.0 (4) 20 2020-06-14 04:18:59 24.29 122.41 台湾宜兰县海域 5.5 21 2020-06-26 05:05:20 35.73 82.33 新疆和田地区于田县 6.4 (5) 22 2020-07-12 06:38:25 39.78 118.44 河北唐山市古冶区 5.1 23 2020-07-13 09:28:02 44.42 80.82 新疆伊犁州霍城县 5.0 24 2020-07-23 04:07:20 33.19 86.81 西藏那曲市尼玛县 6.6 25 2020-07-26 20:52:27 24.27 122.48 台湾花莲县海域 5.5 26 2020-09-29 04:50:53 22.29 121.1 台湾台东县海域 5.0 27 2020-09-30 12:37:18 24.85 122.14 台湾宜兰县海域 5.0 28 2020-12-10 21:19:58 24.74 121.99 台湾宜兰县海域 5.8 注：“（）”中表示为地震灾害事件。 
图 1 2020年中国5.0级及以上地震事件及成灾事件空间分布图Figure 1. Spatial distribution of earthquakes (MS≥5.0) in China, 20202. 2020年中国大陆地震灾害情况
2020年，中国大陆地区共发生地震灾害事件5次，造成5人死亡，30人受伤，直接经济损失约18.47亿元（表2）。其中，灾害损失最严重的地震为1月19日新疆伽师6.4级地震，造成1人死亡，2人受伤，直接经济损失15.26亿元。人员伤亡最严重的地震为5月18日云南巧家5.0级地震，造成4人死亡，28人受伤，直接经济损失约1.04亿元。
表 2 2020年中国大陆地震灾害损失Table 2. Losses caused by earthquake disasters in China mainland, 2020序号 时间 震中位置 震级/M 死亡人数/人 受伤人数/人 直接经济损失/万元 1 2020-01-16 16:32 新疆阿克苏地区库车市 5.6 0 0 712 2 2020-01-19 21:27 新疆喀什地区伽师县 6.4 1 2 152 642 3 2020-04-01 20:23 四川甘孜州石渠县 5.6 0 0 19 242.69 4 2020-05-18 21:47 云南昭通市巧家县 5.0 4 28 10 430 5 2020-06-26 05:05 新疆和田地区于田县 6.4 0 0 1 650 3. 2020年中国大陆地区地震灾害特点
（1）地震成灾事件总体偏少
2020年共发生5次地震灾害事件，低于2000年以来平均水平。
（2）地震灾害相对集中
5次地震灾害事件中有3次发生在新疆维吾尔自治区，共造成1人死亡，2人受伤，直接经济损失约15.5亿元。地震造成的人员伤亡主要集中在云南省，其中云南省巧家县5.0级地震虽未造成巨大的直接经济损失，但造成4人死亡，28人受伤。
发生在新疆维吾尔自治区的3次地震灾害事件分别为：①2020年1月16日16时32分阿克苏地区库车市发生的5.6级地震，震源深度16 km，未造成人员伤亡，直接经济损失712万元。震中位于库车市，极震区烈度为Ⅵ度，Ⅵ度区面积813.6 km2（表3）。灾区场地位于塔里木盆地腹地，场地对地震动有显著的放大作用，易产生不均匀沉降，加重了建筑物震害。灾区农居房屋抗震能力普遍提高，震中“安居富民房”与农村安居房均未出现破坏，有效保护灾区群众生命财产安全，同时缓解了抗震救灾和转移安置压力，仅少数建造年代较早的抗震安居房出现破坏。②2020年1月19日21时27分喀什地区伽师县发生的6.4级地震，震源深度16 km，造成1人死亡，2人受伤，直接经济损失152 642万元。Ⅵ度及以上区总面积为7 599 km2，其中，Ⅵ度区面积4 945 km2；Ⅶ度区面积为2 397 km2；Ⅷ度区面积为257 km2，主要涉及伽师县西克尔库勒镇、古勒鲁克乡。极震区烈度为Ⅷ度，农村安居房设防烈度为8度，安居房主要承重构件未发生明显破坏，有效抵御了本次地震灾害，在保障人民群众生命财产安全及震后转移安置、余震防范中发挥了重要作用。本次地震为前震-主震-余震型，前震为5.4级，主震为6.4级，最大余震为5.2级，对震中区产生多次震害影响，自建砖木结构房屋墙体为黏土砖砌筑，震害叠加效应显著。当地群众防震减灾意识在历次地震灾害实践中不断得到加强，防震减灾意识、应急避险知识和自救互救能力等得到明显提升。③2020年6月26日5时5分，和田地区于田县发生的6.4级地震，震源深度10 km，未造成人员伤亡，直接经济损失1 650万元。于田县阿羌乡3.5 km道路出现塌方，1座中桥出现多处裂缝，1座自建木桥受损，1个涵洞严重受损，1座拱桥受损。本次地震有感范围较广，但破坏性较小。震区附近乡镇村庄居民普遍反映地震造成的晃动强度一般，大部分区域房屋抗震设防水平高，“富民安居工程”房屋和早期的抗震安居房屋基本完好，仅个别民居院内的自建砖木结构房屋出现轻微程度破坏。
表 3 2020年中国大陆地震灾区范围统计Table 3. Statistics of range of earthquake disaster area in China mainland, 2020序号 时间 震中位置 震级/M 极震区烈度/度 震源深度/km 乡镇
/个人口
/人各烈度区面积/km2 Ⅴ Ⅵ Ⅶ Ⅷ 1 2020-01-16 16:32 新疆阿克苏地区库车市 5.6 Ⅵ 16 12 685 — 813.6 0 0 2 2020-01-19 21:27 新疆喀什地区伽师县 6.4 Ⅷ 16 12 279 388 — 4 945 2 397 257 3 2020-04-01 20:23 四川甘孜州石渠县 5.6 Ⅶ 10 6 — — 360 3 140 0 4 2020-05-18 21:47 云南昭通市巧家县 5.0 Ⅵ 8 6 67 083 — 330 0 0 5 2020-06-26 05:05 新疆和田地区于田县 6.4 Ⅷ 10 — — — — — — 注：新疆伽师地震Ⅵ度区之外的部分地区也受到波及，个别老旧房屋出现破坏受损现象，为Ⅵ度异常点；四川甘孜州石渠县地震位于Ⅵ度区之外色达县泥朵镇和其他地区也受到波及，零星房屋有破坏现象。 （3）地震次生灾害突出
今年发生的地震灾害中，次生灾害多发，如新疆伽师地震造成震区1座水库出现险情，当地政府紧急疏散安置受影响群众，云南巧家地震次生地质灾害造成2人死亡，数人受伤，震区交通等基础设施受损，再次为各级政府敲响警钟，应对西部地区地震次生灾害引起足够重视，才能进一步减轻地震灾害。
4. 1991—2020年主要震害情况
1991—2020年主要震害统计数据如表4所示，3个主要统计数据分布情况（许永江等，1999；郑通彦等，2012，2015a，2015b；陈通等，2016；文鑫涛等，2018；林向洋等，2018，2020a，2020b）如图2所示。由表4和图2可知，2011—2020年共造成2 063.08亿元的经济损失，其中2013、2014年地震造成的灾害是2011年以来最严重的，这两年造成灾害最重的地震分别为2013年4月20日四川芦山7.0级地震和2014年8月3日云南鲁甸6.5级地震，这2年合计的经济损失、人员死亡（失踪）和人员受伤数量分别占2011—2020年总数的82.9%、81.8%和65.5%。
表 4 1991—2020年主要震害统计数据Table 4. The statistics of the annual damage caused by earthquakes from 1991 to 2020年份 成灾次数/次 死亡人数/人 受伤人数/人 直接经济损失/亿元 2011 15 32 506 60.11 2012 11 86 1 331 82.88 2013 14 294 15 671 995.36 2014 10 736 3 688 355.64 2015 12 33 1 217 180.00 2016 16 2 103 66.80 2017 11 37 638 217.40 2018 11 0 81 27.30 2019 13 17 411 59.12 2020 5 5 30 18.47 2011—2020 118 1242 23 676 2 063.08 2001—2010 108 72372 398 917 8 984.90 1991—2000 130 564 52 668 120.18 
图 2 1991—2020年中国大陆成灾地震年度震害分布图Figure 2. Distribution of annual earthquakes losses in China mainland from 1991 to 2020近10年中国大陆年均成灾地震频次较1991年以来的总体水平略低，近10年期间地震灾害造成的经济损失、人员死亡（失踪）和人员受伤数量分别占30年来的18.47%、1.67%和4.98%，可见地震灾害造成的经济损失、人员伤亡数量均低于1991年以来的平均水平。
5. 结语
2020年我国大陆未发生特重大地震灾害事件，地震灾害损失总体偏轻，成灾地震次数较少，低于2000年以来的平均水平。地震灾害相对集中，5次成灾地震中3次发生在新疆维吾尔自治区，四川和云南各发生1次。地震次生灾害多发，小震致灾致亡现象突出，云南省巧家县5.0级地震造成4人死亡，28人受伤，其中次生地质灾害造成2人死亡。各级政府应对西部地区地震次生灾害引起足够重视，进而减轻人员伤亡。
-
图 5 起伏界面上与下的阶梯状网格完成单元二合一处理后变为斜面网格示意图
Figure 5. The stepped mesh above and below the undulating interface become inclined grids using two-in-one unit method
图 6 台阶状网格处使用单元二合一的方式处理过程示意图
Figure 6. process of using two-in-one unit method on stepped mesh
图 7 起伏界面上与下的阶梯状网格完成单元二合一处理后变为斜面网格的模型示意图
Figure 7. The stepped mesh above and below the undulating interface become inclined grids using two-in-one unit method
图 14 研究区域第四系沉积地层地形
Figure 14. Topographic map of quaternary sedimentary strata in the study area
图 18 均匀介质中3种剖分方式在测点位置一处四纵列单元的模拟时程曲线结果对比
Figure 18. Comparison of the simulation time history curves of three mesh generation methods in a uniform medium with four rows of elements at the test point one
图 19 非均匀介质中3种剖分方式在测点位置一处四纵列单元的模拟时程曲线结果对比
Figure 19. Comparison of the simulation time history curves of three mesh generation methods in a four-row unit at a test point in heterogeneous medium
图 20 非均匀介质中直接使用台阶状网格在测点位置一处四纵列单元的模拟时程曲线结果对比
Figure 20. Comparison of simulation time history curve results of four rows of units at a test point directly using stepmesh in non-uniform medium
图 21 3种剖分方式在测点位置二的时程曲线对比
Figure 21. Comparison of the time history curves of the three mesh generation methods at the second test point
表 1 模型基本参数
Table 1. Basic parameters of the model
模型尺寸/m 总步长/步 格点间距/m 时间步长/s 震源时间函数主频/Hz 震源时间函数 6 000×5 000×1 100（长×宽×深） 4 000 100 0.001 4 主频为4 Hz的Ricker子波 
下载: 导出CSV
-
[1] 董良国, 马在田, 曹景忠等, 2000. 一阶弹性波方程交错网格高阶差分解法. 地球物理学报, 43(3): 411—419 doi: 10.3321/j.issn:0001-5733.2000.03.015Dong L. G. , Ma Z. T. , Cao J. Z. , et al. , 2000. A staggered-grid high-order difference method of one-order elastic wave equation. Chinese Journal of Geophysics, 43(3): 411—419. (in Chinese) doi: 10.3321/j.issn:0001-5733.2000.03.015 [2] 董良国, 李培明, 2004. 地震波传播数值模拟中的频散问题. 天然气工业, 24(6): 53—56 doi: 10.3321/j.issn:1000-0976.2004.06.016Dong L. G. , Li P. M. , 2004. Dispersive problem in seismic wave propagation numerical modeling. Natural Gas Industry, 24(6): 53—56. (in Chinese) doi: 10.3321/j.issn:1000-0976.2004.06.016 [3] 付长华, 高孟潭, 陈鲲, 2012. 北京盆地结构对长周期地震动反应谱的影响. 地震学报, 34(3): 374—382 doi: 10.3969/j.issn.0253-3782.2012.03.009Fu C. H. , Gao M. T. , Chen K. , 2012. A study on long-period response spectrum of ground motion affected by basin structure of Beijing. Acta Seismologica Sinica, 34(3): 374—382. (in Chinese) doi: 10.3969/j.issn.0253-3782.2012.03.009 [4] 高孟潭, 俞言祥, 张晓梅等, 2002. 北京地区地震动的三维有限差分模拟. 中国地震, 18(4): 356—364 doi: 10.3969/j.issn.1001-4683.2002.04.005Gao M. T. , Yu Y. X. , Zhang X. M. , et al. , 2002. Three-dimensional finite-difference simulations of ground motions in the Beijing area. Earthquake Research in China, 18(4): 356—364. (in Chinese) doi: 10.3969/j.issn.1001-4683.2002.04.005 [5] 国家地震局, 1986. 中国地壳上地幔地球物理探测成果. 北京: 地震出版社. [6] 金安蜀, 刘福田, 孙永智, 1980. 北京地区地壳和上地幔的三维P波速度结构. 地球物理学报, 23(2): 172—182 doi: 10.3321/j.issn:0001-5733.1980.02.006Jin A. S. , Liu F. T. , Sun Y. Z. , 1980. Three-dimensional P velocity structure of the crust and upper mantle under Beijing region. Chinese Journal of Geophysics, 23(2): 172—182. (in Chinese) doi: 10.3321/j.issn:0001-5733.1980.02.006 [7] 罗宏伟, 郭鹏, 王树山等, 2013. 基于TrueGrid软件的射孔弹有限元仿真模型参数化建立方法. 测井技术, 37(2): 219—222 doi: 10.3969/j.issn.1004-1338.2013.02.022Luo H. W. , Guo P. , Wang S. S. , et al. , 2013. Parametric modeling method for finite element simulation of perforating charge based on TrueGrid software. Well Logging Technology, 37(2): 219—222. (in Chinese) doi: 10.3969/j.issn.1004-1338.2013.02.022 [8] 蒙茂洲, 2010. 功能强大的网格生成软件——TrueGrid. CAD/CAM与制造业信息化, (1): 57—60. [9] 孙建国, 2007. 复杂地表条件下地球物理场数值模拟方法评述. 世界地质, 26(3): 345—362 doi: 10.3969/j.issn.1004-5589.2007.03.015Sun J. G. , 2007. Methods for numerical modeling of geophysical fields under complex topographical conditions: a critical review. Global Geology, 26(3): 345—362. (in Chinese) doi: 10.3969/j.issn.1004-5589.2007.03.015 [10] 孙若昧, 赵燕来, 吴丹, 1996. 京津唐地区地壳结构与强震的发生──Ⅱ. S波速度结构. 地球物理学报, 39(3): 347—355Sun R. M. , Zhao Y. L. , Wu D. , 1996. Crust structures and strong earthquakes in the Beijing, Tianjin, Tangshan area: Ⅱ. S wave velocity structure. Acta Geophysica Sinica, 39(3): 347—355. (in Chinese) [11] 王夫运, 张先康, 陈棋福等, 2005. 北京地区上地壳三维细结构层析成像. 地球物理学报, 48(2): 359—366 doi: 10.3321/j.issn:0001-5733.2005.02.018Wang F. Y. , Zhang X. K. , Chen Q. F. , et al. , 2005. Fine tomographic inversion of the upper crust 3-D structure around Beijing. Chinese Journal of Geophysics, 48(2): 359—366. doi: 10.3321/j.issn:0001-5733.2005.02.018 [12] 王瑞, 高曙明, 吴海燕, 2020. 六面体网格生成和优化研究进展. 计算机辅助设计与图形学学报, 32(5): 693—708Wang R. , Gao S. M. , Wu H. Y. , 2020. Progress in hexahedral mesh generation and optimization. Journal of Computer-Aided Design & Computer Graphics, 32(5): 693—708. [13] 赵金仁, 张先康, 张成科等, 1999. 香河-北京-涿鹿及其相邻地区壳幔构造与速度结构特征. 地震地质, 21(1): 29—36 doi: 10.3969/j.issn.0253-4967.1999.01.004Zhao J. R. , Zhang X. K. , Zhang C. K. , et al. , 1999. The crust-mantle tectonics and velocity structure characteristics in Xianghe-Beijing-Zhulu and its adjacent areas. Seismology and Geology, 21(1): 29—36. doi: 10.3969/j.issn.0253-4967.1999.01.004 [14] Alford R. M. , Kelly K. R. , Boore D. M. , 1974. Accuracy of finite-difference modeling of the acoustic wave equation. Geophysics, 39(6): 834—842. doi: 10.1190/1.1440470 [15] Alterman Z. , Karal Jr F. C. , 1968. Propagation of elastic waves in layered media by finite difference methods. Bulletin of the Seismological Society of America, 58(1): 367—398. [16] Crase E. , Pica A. , Noble M. , et al. , 1990. Robust elastic nonlinear waveform inversion; application to real data. Geophysics, 55(5): 527—538. doi: 10.1190/1.1442864 [17] Dablain M. A. , 1986. The application of high-order differencing to the scalar wave equation. Geophysics, 51(1): 54—66. doi: 10.1190/1.1442040 [18] Furumura T. , Kennett B. L. N. , Furumura, M. , 1998. Seismic wavefield calculation for laterally heterogeneous whole earth models using the pseudospectral method. Geophysical Journal International, 135(3): 845—860. doi: 10.1046/j.1365-246X.1998.00682.x [19] Graves R. W. , 1996. Simulating seismic wave propagation in 3D elastic media using staggered-grid finite differences. Bulletin of the Seismological Society of America, 86(4): 1091—1106. [20] Hestholm S. , Ruud B. , 1994. 2D finite-difference elastic wave modelling including surface topography. Geophysical Prospecting, 42(5): 371—390. doi: 10.1111/j.1365-2478.1994.tb00216.x [21] Hestholm S. , Ruud B. , 2002. 3D free-boundary conditions for coordinate-transform finite-difference seismic modelling. Geophysical Prospecting, 50(5): 463—474. doi: 10.1046/j.1365-2478.2002.00327.x [22] Jastram C. , Tessmer E. , 1994. Elastic modelling on a grid with vertically varying spacing. Geophysical Prospecting, 42(4): 357—370. doi: 10.1111/j.1365-2478.1994.tb00215.x [23] Koketsu K. , Fujiwara H. , Ikegami Y. , 2004. Finite-element simulation of seismic ground motion with a voxel mesh. Pure and Applied Geophysics, 161(11—12): 2183—2198. [24] Komatitsch D. , Vilotte J. P. , 1998. The spectral element method: an efficient tool to simulate the seismic response of 2D and 3D geological structures. Bulletin of the Seismological Society of America, 88(2): 368—392. [25] Komatitsch D. , Tromp J. , 1999. Introduction to the spectral element method for three-dimensional seismic wave propagation. Geophysical Journal International, 139(3): 806—822. doi: 10.1046/j.1365-246x.1999.00967.x [26] Komatitsch D. , Barnes C. , Tromp J. , 2000. Simulation of anisotropic wave propagation based upon a spectral element method. Geophysics, 65(4): 1251—1260. doi: 10.1190/1.1444816 [27] Komatitsch D. , Tromp J. , 2002. Spectral-element simulations of global seismic wave propagation—I. Validation. Geophysical Journal International, 149(2): 390—412. doi: 10.1046/j.1365-246X.2002.01653.x [28] Komatitsch D., Tsuboi S., Tromp J., 2005. The spectral-element method in seismology. In: Levander A, Nolet G, eds., Seismic Earth: Array Analysis of Broadband Seismograms. Washington: American Geophysical Union, 157. [29] Lan, H. Q. , Zhang, Z. J. , 2011. Three-dimensional wave-field simulation in heterogeneous transversely isotropic medium with irregular free surface. Bulletin of the Seismological Society of America, 101(3): 1354—1370. doi: 10.1785/0120100194 [30] Levander A. R. , 1988. Fourth-order finite-difference P-SV seismograms. Geophysics, 53(11): 1425—1436. doi: 10.1190/1.1442422 [31] Madariaga R. , 1976. Dynamics of an expanding circular fault. Bulletin of the Seismological Society of America, 66(3): 639—666. doi: 10.1785/BSSA0660030639 [32] Moczo P. , 1989. Finite-difference technique for SH-waves in 2-D media using irregular grids-application to the seismic response problem. Geophysical Journal International, 99(2): 321—329. doi: 10.1111/j.1365-246X.1989.tb01691.x [33] Moczo P. , Kristek J. , Bystrický E. , 2001. Efficiency and optimization of the 3-D finite-difference modeling of seismic ground motion. Journal of Computational Acoustics, 9(2): 593—609. doi: 10.1142/S0218396X01000681 [34] Olsen K. B. , Archuleta R. J. , Matarese J. R. , 1995. Three-dimensional simulation of a magnitude 7.75 earthquake on the San Andreas fault. Science, 270(5242): 1628—1632. doi: 10.1126/science.270.5242.1628 [35] Olsen K. B. , Madariaga R. , Archuleta R. J. , 1997. Three-dimensional dynamic simulation of the 1992 landers earthquake. Science, 278(5339): 834—838. doi: 10.1126/science.278.5339.834 [36] Olsen K. B. , 2000. Site amplification in the Los Angeles basin from three-dimensional modeling of ground motion. Bulletin of the Seismological Society of America, 90(6B): S77—S94. doi: 10.1785/0120000506 [37] Patera A. T. , 1984. A spectral element method for fluid dynamics: laminar flow in a channel expansion. Journal of Computational Physics, 54(3): 468—488. doi: 10.1016/0021-9991(84)90128-1 [38] Pitarka A. , 1999. 3D elastic finite-difference modeling of seismic motion using staggered grids with nonuniform spacing. Bulletin of the Seismological Society of America, 89(1): 54—68. doi: 10.1785/BSSA0890010054 [39] Priolo E. , Carcione J. M. , Seriani G. , 1994. Numerical simulation of interface waves by high-order spectral modeling techniques. The Journal of the Acoustical Society of America, 95(2): 681—693. doi: 10.1121/1.408428 [40] Robertsson J. O. A, 1996. A numerical free-surface condition for elastic/viscoelastic finite-difference modeling in the presence of topography. Geophysics, 61(6): 1921—1934. doi: 10.1190/1.1444107 [41] Seriani G. , 1998. 3-D large-scale wave propagation modeling by spectral element method on Cray T3E multiprocessor. Computer Methods in Applied Mechanics and Engineering, 164(1—2): 235—247. doi: 10.1016/S0045-7825(98)00057-7 [42] Smith W. D. , 1975. The application of finite element analysis to body wave propagation problems. Geophysical Journal International, 42(2): 747—768. [43] Tarrass I. , Giraud L. , Thore P. , 2011. New curvilinear scheme for elastic wave propagation in presence of curved topograph. Geophysical Prospecting, 59(5): 889—906. doi: 10.1111/j.1365-2478.2011.00972.x [44] Tessmer E. , Kosloff D. , Behle A. , 1992. Elastic wave propagation simulation in the presence of surface topography. Geophysical Journal International, 108(2): 621—632. doi: 10.1111/j.1365-246X.1992.tb04641.x [45] Xue Z. , Dong L. G. , Li X. B. , et al. , 2014. Discontinuous galerkin finite-element method for elastic wave modeling including surface topography. Chinese Journal of Geophysics, 57(4): 1209—1223. [46] Zahradník J. , Moczo P. , Hron F. , 1993. Testing four elastic finite-difference schemes for behavior at discontinuities. Bulletin of the Seismological Society of America, 83(1): 107—129. [47] Zhang W. , Chen X. F. , 2006. Traction image method for irregular free surface boundaries in finite difference seismic wave simulation. Geophysical Journal International, 167(1): 337—353. doi: 10.1111/j.1365-246X.2006.03113.x [48] Zhang W. Q. , Zhang Z. G. , Fu H. H. , et al. , 2019. Importance of spatial resolution in ground motion simulations with 3-D basins: an example using the Tangshan earthquake. Geophysical Research Letters, 46(21): 11915—11924. doi: 10.1029/2019GL084815 [49] Zhou H. , Chen X. F. , 2010. A new technique to synthesize seismography with more flexibility: the Legendre spectral element method with overlapped elements. Pure and Applied Geophysics, 167(11): 1365—1376. doi: 10.1007/s00024-010-0106-0 -
下载:
点击查看大图
计量
- 文章访问数: 193
- HTML全文浏览量: 83
- PDF下载量: 10
- 被引次数: 0
