Study on Nonlinear Statistical Characteristics of Surface/Downhole Response Spectrum Ratio and Influencing Factors
-
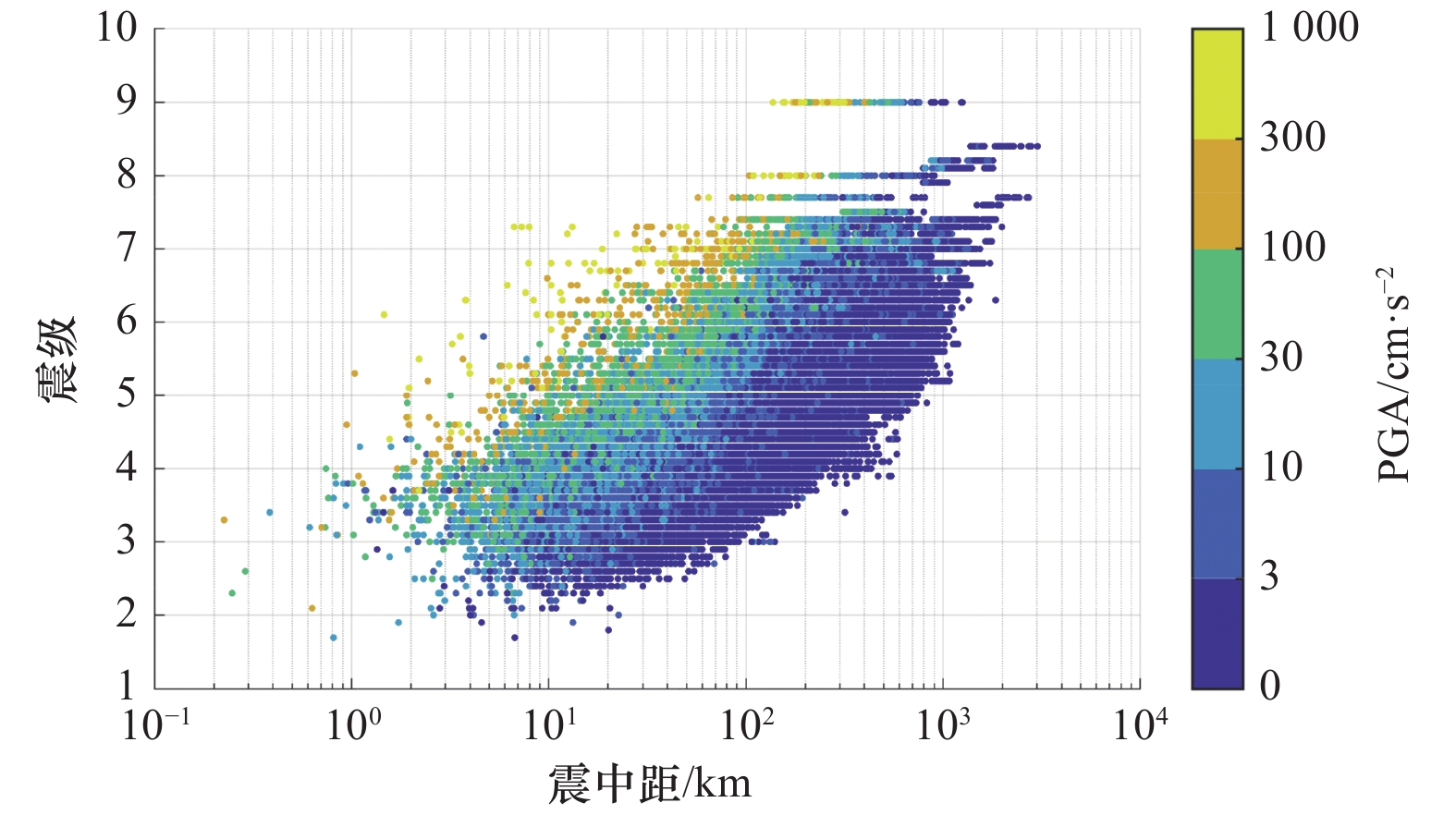
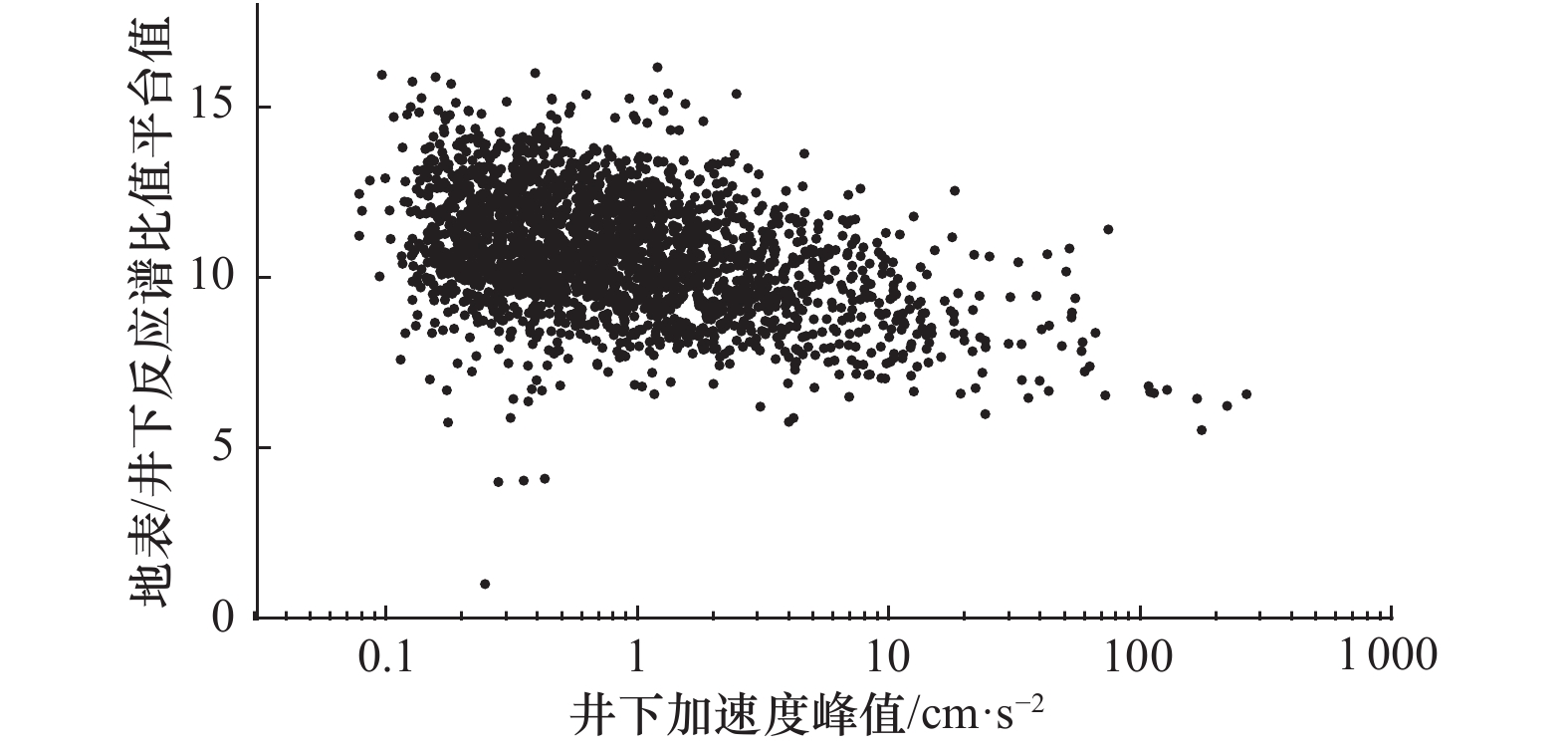
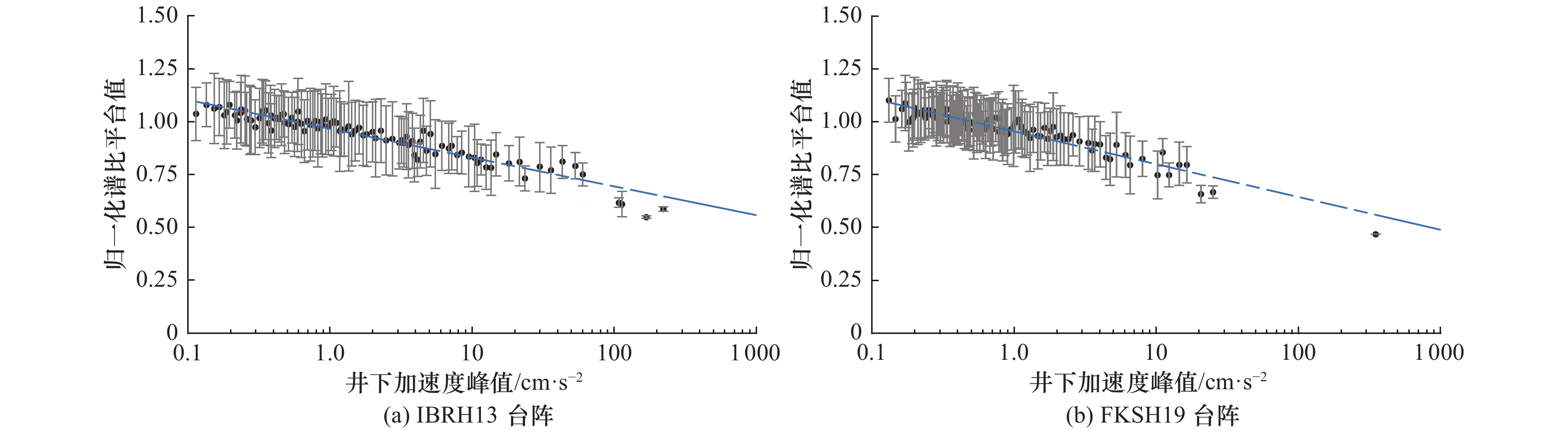
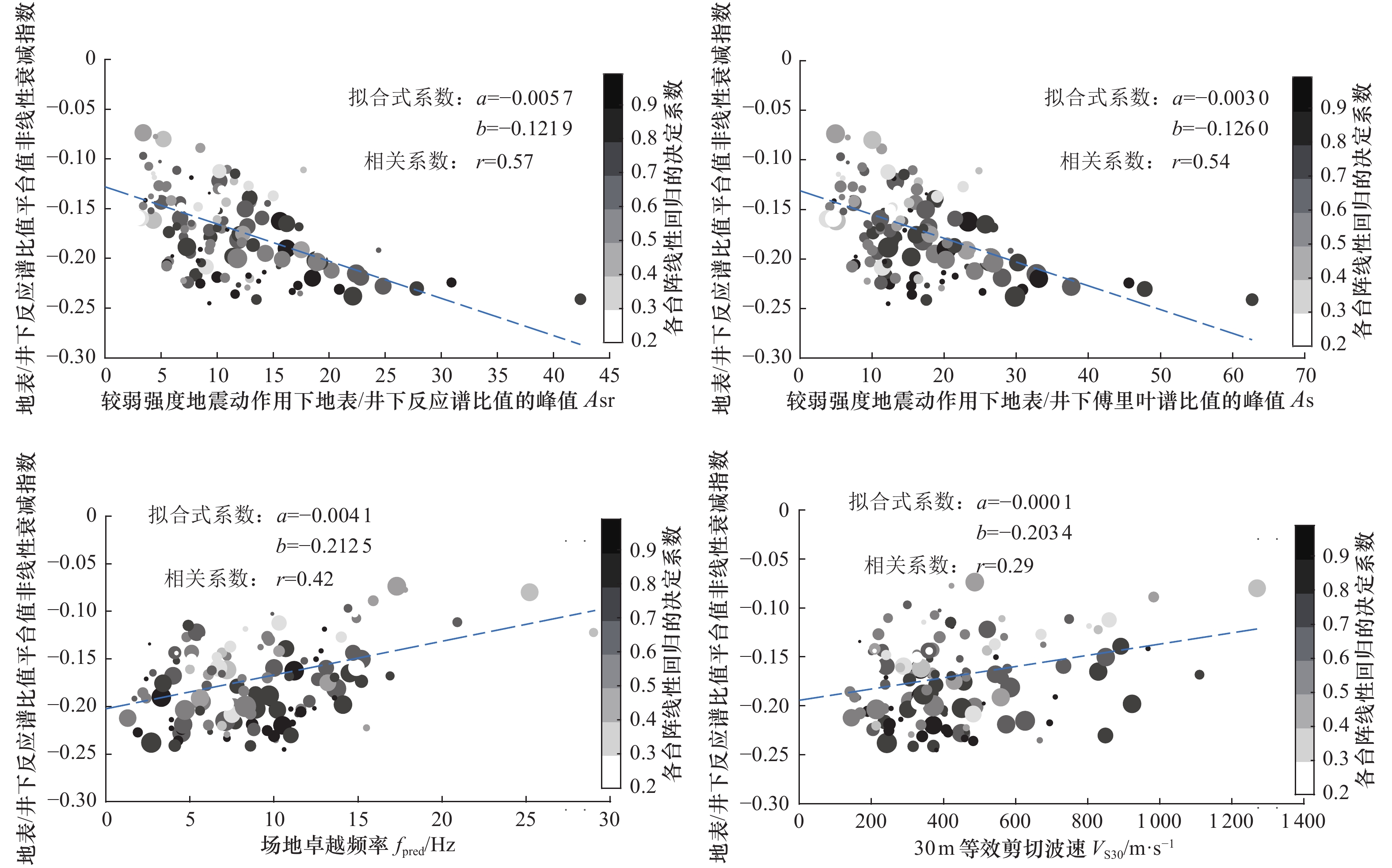
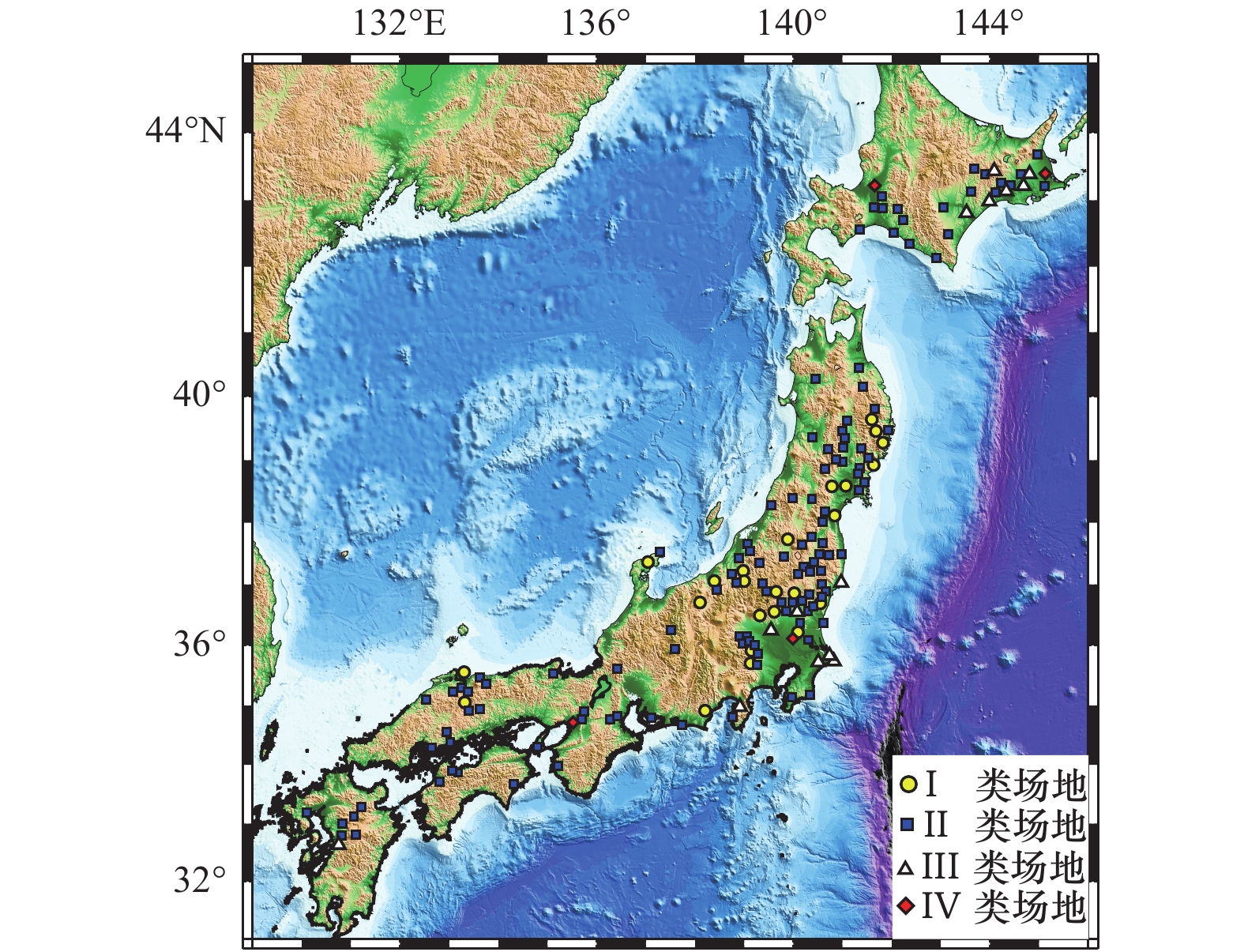
摘要: 浅地表软弱覆盖层对地震动的影响具有强非线性,但因观测记录样本量过小,难以通过参考谱比法等直接方法予以可靠分析。本文基于日本KiK-net台网136个竖向钻井台阵获取的141881组加速度记录的统计分析,研究了地表/井下反应谱比值随地震动强度变化的非线性变化规律与主要影响因素。利用变窗口尺度的滑动窗口平均法加强数据线性度,并确定了与地震动强度相关的台站最小记录样本量,统计结果表明地表/井下反应谱比值平台值随地震动强度变化的非线性衰减指数为−0.24~−0.08。利用套索(Lasso)算法统计回归发现,本研究12个场地特性表征参数中,30 m平均剪切波速(
$ {V}_{\mathrm{s}30} $ )、场地卓越频率与地表/井下反应谱比值非线性衰减指数具有较好的正相关性;较弱强度地震动作用下地表/井下傅里叶谱比值峰值、地表/井下反应谱比值峰值与地表/井下反应谱比值非线性衰减指数具有较好的负相关性。Abstract: The influence of shallow soft overburden on ground motion is strongly nonlinear, but it is difficult to make reliable analysis by direct methods such as reference spectral ratio method because of the small sample size of observation records. In this study, based on the statistical analysis of 141881 sets of acceleration records obtained from 136 vertical borehole array in Japan’s KiK-net network, the nonlinear variation of surface/downhole response spectrum ratio and its main influencing factors of field motion intensity were studied. The sliding window average method with variable window scale was used to enhance the linearity of the data, and the minimum sample size related to the ground motion intensity was determined. The statistical results show that the nonlinear attenuation index of ground motion intensity variation is between −0.24 and −0.08 for the platform value of the surface/underground response spectrum ratio. According to Lasso regression statistics, the average shear wave velocity of 30 m ($ {V}_{S30} $ ) and site predominant frequency have a good positive correlation with the nonlinear attenuation index of surface/downhole response spectrum ratio among the 12 site characteristic parameters studied. However, the peak values of the surface/downhole fourier spectrum ratio and the surface/downhole response spectrum ratio are negatively correlated with the nonlinear attenuation index of the surface/downhole response spectrum ratio under the weak ground motion.-
Key words:
- Strong motion /
- Site effect /
- Response spectrum /
- Nonlinear /
- Vertical borehole arrays
-
引言
应急装备是处理突发事件、实施应急工作的物质基础。地震应急装备是指震灾发生后在应急工作中所使用的专业设备,包括感知探测装备、生命救援装备、交通运输和工程保障等物资(高建国,2004;李磊,2006)。我国是受地震灾害最为严重的国家之一,地震给人们的生命财产安全和社会稳定带来巨大威胁。地震应急是为最大限度地减轻地震灾害损失这一核心目标所做的重要工作,其工作重心在震区,而破坏性地震往往会使震区环境发生异常,灾区生存环境将变得非常恶劣。因此,专业化的应急装备保障是顺利开展地震应急工作所不可或缺的重要保障。
地震应急装备的重要性在历次地震现场得到了实践检验,并得到了各级政府的高度重视。目前,我国地震应急装备的数量、质量、性能和种类均得到了大幅度的提高,且在各地均设立了独立的地震应急装备库,基本可以满足当前应急工作的现实需要,但在地震应急装备管理方面仍然存在不足。各地基本还是沿袭传统的物资仓库管理方式,以卡片、纸张文件为基础来记录、追踪进出的物资,完全依靠人工实施管理。这种管理模式速度慢、效率低、准确性差,一旦发生地震灾害,不能迅速掌握装备的需求、定位和调配,将极大地影响应急工作的时效性,延误最佳的应急时机(范灵春等,2011;陈良冬,2014;孙路强等,2016)。要解决这一问题,就必须充分利用计算机的强大信息处理和运算能力这一优势,结合地震应急装备库不同于一般物资仓库这一特点,开发地震应急装备库信息管理系统,实现信息接收及处理、方案生成和打印输出的自动化,代替以手工为主的作业方式和经验式的装备保障方式,提高地震应急工作装备保障工作的准确性、可靠性和时效性。
本文采用PowerScript语言进行开发,应用MySQL数据库,以地震应急装备库信息为基础数据,设计与实现了可供多部门、多用户共享访问的地震应急装备信息管理系统。该系统可以及时呈现出每个装备的所属信息,实现应急装备的高效、智能化、科学化管理,保证装备资源的安全性和使用时的高效性,使地震应急时装备分配效率得到很大提升。
1. 系统分析
1.1 系统需求分析
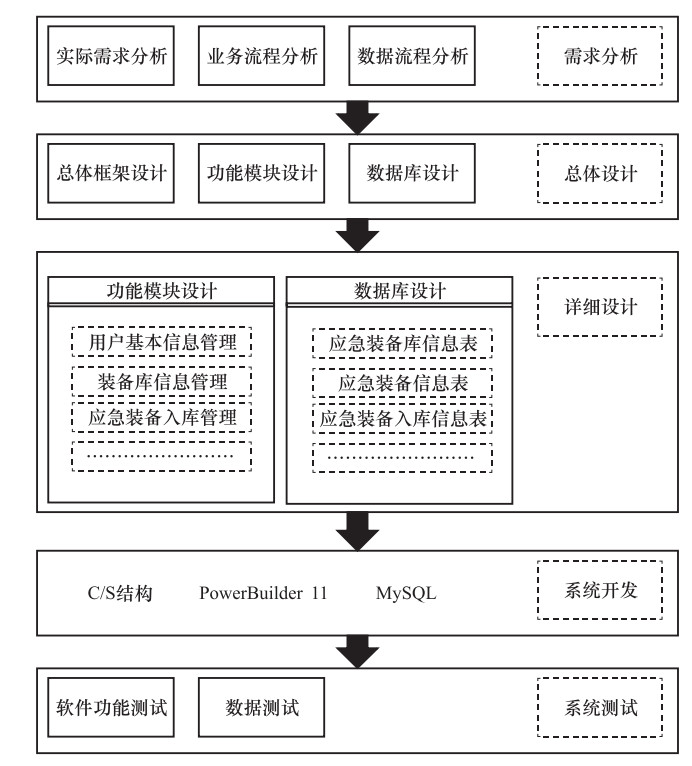
目前我国的地震应急装备管理工作主要还是依靠传统管理模式和手段,面临着信息共享性和监管力度差等问题,比如在地震应急装备的使用情况记录上还是采用普通的人工记录,缺乏可靠的记录和监管。各部门之间也缺少良性的沟通,信息化共享水平有限,缺乏统一的数据标准化和信息化管理平台,从而导致地震应急装备在平时存放无序、管理混乱,极大降低日常的工作效率。地震应急装备是整个地震应急工作的前提和基础,针对目前地震应急装备管理存在的工作方法和技术手段相对落后、装备处置时效性差和维护成本大等问题,现结合地震应急装备库不同于一般传统仓储的特点,以中国地震局第一监测中心地震应急装备库数据为基础,根据实际需求构建地震应急装备信息管理系统,对地震应急装备的入库、出库、盘点、预警管理等进行统一的信息化管理,以便在震时及时、准确地对应急装备进行管理和调度,为地震应急工作的顺利开展提供高效、安全可靠的装备保障。
地震应急装备信息管理系统不仅是简单的业务处理系统,其为管理者提供了全局的、实时准确的应急装备信息。本文系统根据应急装备管理工作技术性强、准确性差、手工处理慢等特点,提出了统一规划的信息监管平台,通过数据库来实现地震应急装备的高效、智能化、可视化管理,实现基础数据集中维护,从而保证应急装备资源的安全性和使用时的高效性。
1.2 系统业务流程分析
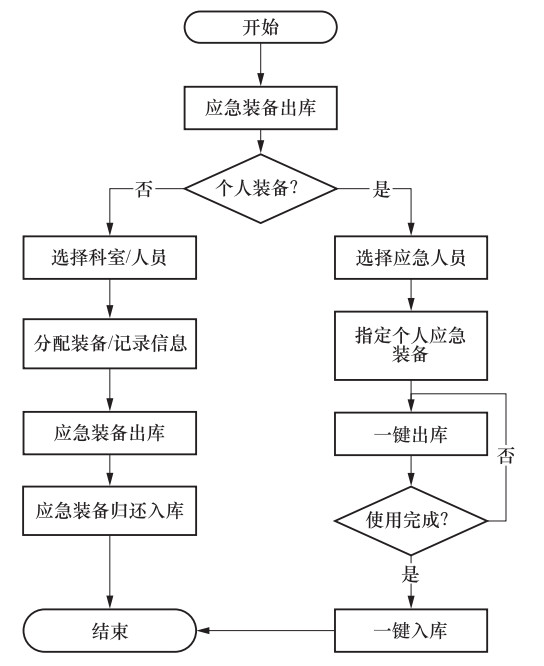
系统业务流程分析不仅可以帮助我们了解某一项业务的具体处理过程,还可以在分析过程中发现和处理系统调研工作中的疏漏,从而修改原业务不合理之处,优化日常业务的处理流程。通过对地震应急装备管理工作模式的分析,本文系统抽取出4个业务流程,主要包括应急装备入库、出库分配、盘点和预警业务。应急装备入库业务流程是指在装备使用完毕后需要对其进行入库、清点,并在登记入库后更新库存现状表。应急装备出库分配业务流程是指发生地震应急响应时,针对现有已入库的应急装备,根据地震级别对应急装备进行分配出库。分配过程和装备现有的库存具有互动关系,一般应急装备现有库存大于一定的需求量,当应急人员临时出现装备使用需求时,首先对应急装备库进行库存量查询,若有库存,则进行应急装备分配,否则需要补足库存。应急装备分配业务主要包括一般应急装备分配和个人应急装备分配两种。一般应急装备主要指一些大型应急装备,如测震仪、钢筋扫描仪、发电机、GPS定位仪、帐篷、睡袋等。个人应急装备是指个人可随身携带的应急装备,平时就放在背包里,遇到应急任务可以领包出库,如对讲机、医用应急包、卫星电话等,这部分装备可以根据需求一键出库,归还时可以一键入库,其地震应急装备分配业务流程图如图 1所示。应急装备盘点业务流程是指对装备进行定期盘点,把当前库存数量与最低库存和最高库存进行对比,对装备的保质期进行检查。应急装备预警业务流程是指在定期盘点后,若装备数量不满安全库存或超出保质期范围时,系统就会发出预警信息,并提示需要处理的相关信息。此时管理人员根据预警选择合适的筹措方式进行处理,以保证装备的存储状态一直在安全库存和保质期范围内;若此时管理员不及时处理则会再次预警,直至库存和保质期达到要求。
2. 系统设计
2.1 系统总体结构设计
考虑到存取安全性、快捷性、交互性以及业务需求等特点,本系统选用客户机/服务器(Client/Server)结构,简称C/S结构。在客户机终端上实现具体的业务逻辑,负责数据的输入与输出,在服务器端架设数据库应用程序,负责对来自Client端的数据进行处理。C/S配备的是点对点的结构模式,适用于局域网,其安全性较为可靠。C/S结构提供了更安全的存取模式,而且交互性强。由于MySQL数据库是一种开源的关联数据库,具备访问速度快、数据体积小、总体运行成本低等特点,因此本文选用其作为系统的数据库。开发工具选用PowerBuilder,它是一个功能超群、使用方便、易于开发复杂应用系统的数据库前端开发工具,尤其是其数据窗口功能强大、使用方便。系统整体框架图如图 2所示。
2.2 系统数据设计
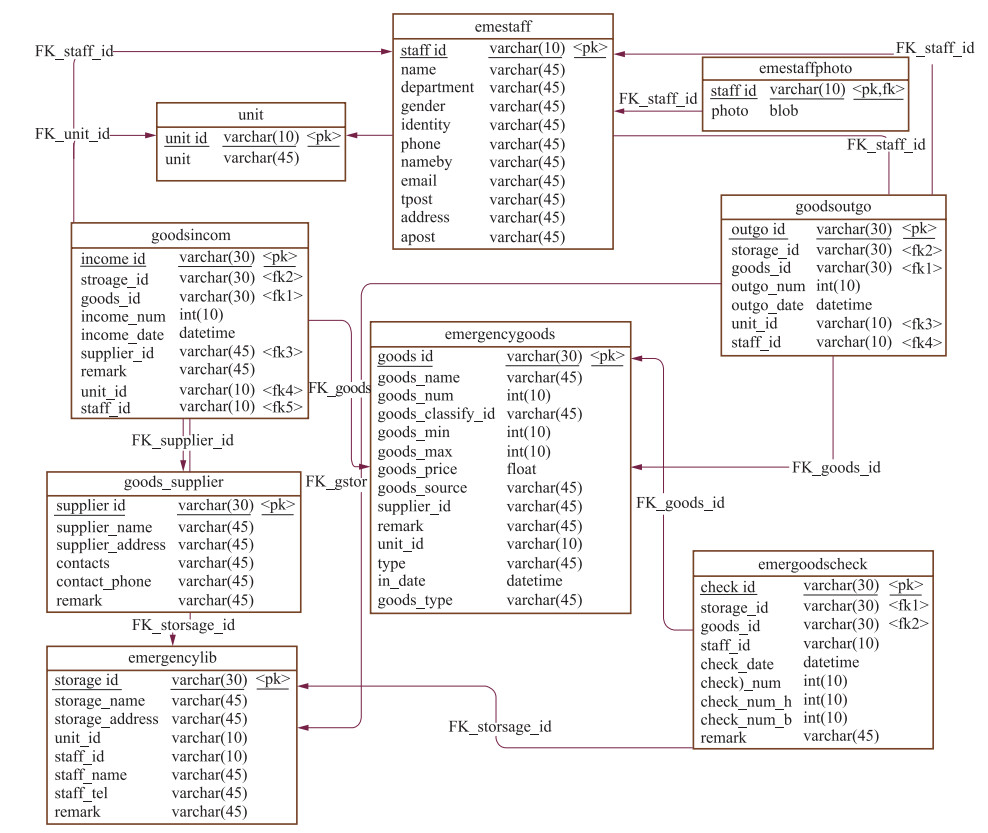
地震应急装备信息管理系统的设计应用了关系数据库的理论,基于MySQL数据库对应急装备相关信息进行相应的数据管理和维护。本系统所涉及的实体主要包括了应急装备、应急人员、各部门、仓库、供应商等,其中应急装备与应急人员之间具有分配、出入库等业务关联关系,而各部门及应急人员之间具有从属关系,库与装备之间具有从属关系。主要涉及的数据库表包括应急人员信息表、人员照片信息表、部门信息表、应急装备信息表、应急库信息表、应急装备入库信息表、应急装备出库信息表、应急装备盘点信息表等,其系统数据库物理数据模型如图 3所示,地震应急装备信息管理系统所有数据库表如表 1所示。
表 1 应急装备信息管理系统关系表Table 1. List of attributes in earthquake emergency equipment information management system数据表名称 数据属性 应急装备库信息表 应急装备库编号、应急装备库名称、应急装备库地址、管理人编号、姓名、联系方式、管理人所在部门编号、备注等 应急装备信息表 应急装备编号、应急装备名称、应急装备数量、应急装备分类、应急装备最低数量值、应急装备最高数量值、应急装备价格、应急装备来源、应急装备类别、备注等 应急装备入库信息表 应急装备入库编号、应急库编号、应急装备编号、入库数量、入库时间、供应商编号、应急人员编号、部门编号、备注等 应急装备出库信息表 应急装备出库编号、应急库编号、应急装备编号、出库数量、出库时间、供应商编号、应急人员编号、部门编号、备注等 应急装备盘点信息表 应急装备盘点编号、应急库编号、应急装备编号、盘点数、盘点时间、合格应急装备数、不合格的应急装备数、盘点人编号、备注等 应急装备供应商信息表 应急装备供应商编号、供应商名称、地址、联系人、联系电话、备注等 应急人员信息表 应急人员编号、姓名、部门、性别、身份证编号、手机号、姓名缩写、Email、职称、职务、地址 应急人员相片信息表 应急人员编号、照片 应急部门信息表 部门编号、部门名称 2.3 系统主要功能设计
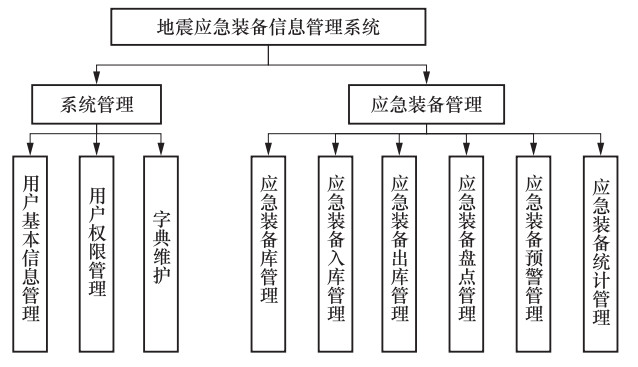
地震应急装备信息管理系统对应急装备在整个应急过程中的传递进行信息化管理,主要功能设计如图 4所示。
(1)系统管理
该模块主要包括用户基本信息管理、用户权限管理、字典维护3部分。用户基本信息管理模块主要是对应急人员基本信息的管理和维护,以及对应急装备信息管理系统中管理人员的管理,具有新增用户、对已有用户的基础信息以及密码等进行修改及删除用户等功能。用户权限管理是为保障系统数据库操作安全性,对用户权限实现分配与回收功能的管理,即对不同用户分配不同的菜单权限和数据权限。字段维护主要是对系统中涉及到的数据字典进行日常的管理和维护。
(2)应急装备信息管理模块
该部分是本系统的核心功能模块,下面对其具体功能进行介绍:
应急装备库信息管理:可以实时查询应急装备的使用状态和使用类型,从而方便用户及时掌握应急装备的分配动态。对应急装备库存信息进行实时更新及维护,包括应急装备库和装备基本信息的管理及维护。系统管理员可以增加、修改应急装备库相关数据信息,同时可以对库中装备的相关数据项进行实时更新和管理。
应急装备入库管理:是应急装备信息系统的主要业务,主要包括个人应急装备一键入库和一般应急装备入库两部分,能够对将要入库的装备进行信息录入、编辑以及检索等。这里的应急装备信息包括应急人员基本信息、所入库的仓库信息、供应商信息、应急装备的特征信息以及数量等。
应急装备出库管理:主要包括个人应急装备一键出库和一般应急装备出库两部分。能够对将要出库的装备进行信息录入、编辑以及检索等。
应急装备库存盘点:可以及时地掌握应急装备的使用情况,还可以通过对应急装备的具体分配情况进行查询。管理人员需要定期对应急装备进行盘点,检查应急物资的保质期、破损情况等,一旦发现不合格应及时进行处理,从而避免因此引起的损失。
应急装备预警管理:可以对系统中的应急装备进行实时的监控,从而保障应急装备的存储状态一直在安全库存和保质期范围内。
应急装备统计管理:主要是对系统中的相关数据,如每年应急装备出入库情况、现有应急装备信息进行统计等。
3. 系统实现
本系统采用PowerScript作为开发语言,采用了MySQL数据库,应用Powerbuilder开发环境,设计并实现了地震应急装备信息管理系统。为提高系统的智能性,本系统采用了程序动态自动更新技术。系统程序可以自动判断当前版本号,自动下载并更新为最新版本,从而保证了程序及时稳定更新。
3.1 应急装备信息管理模块的实现
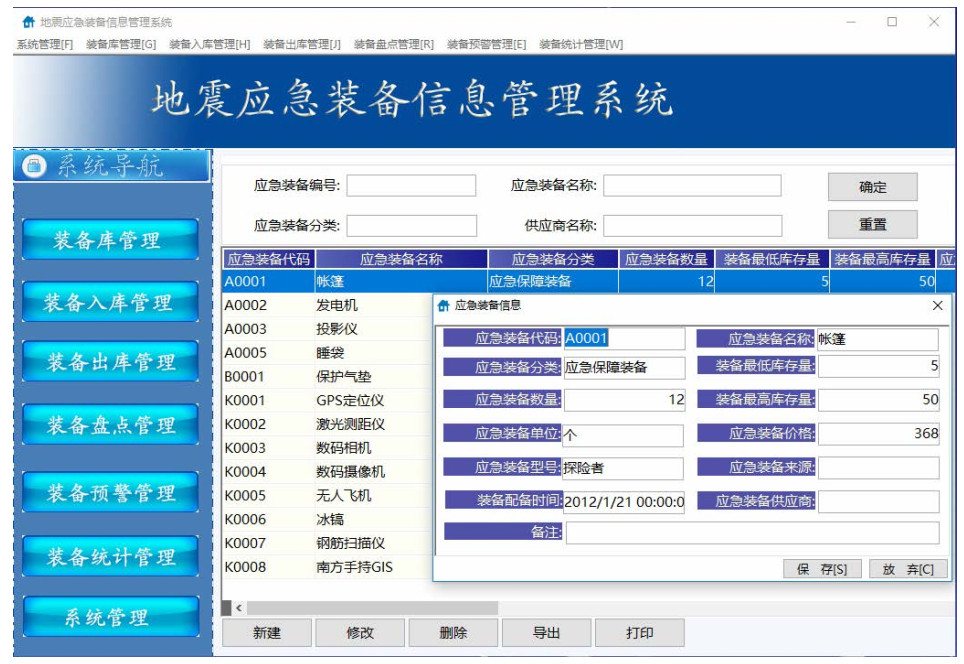
本窗体主要实现的功能包括根据应急装备编号或名称查询其基本信息、某一类别下所有的装备情况及某供应商提供的所有应急装备的情况等,同时可对应急装备信息进行实时更新和维护,包括新增、修改和删除功能。为了便于领导审阅或存档,本窗体还实现了数据记录导出功能,用户可以直接导出excel文件或在线打印文件。该模块界面如图 5所示。
3.2 个人应急装备一键出库模块的实现
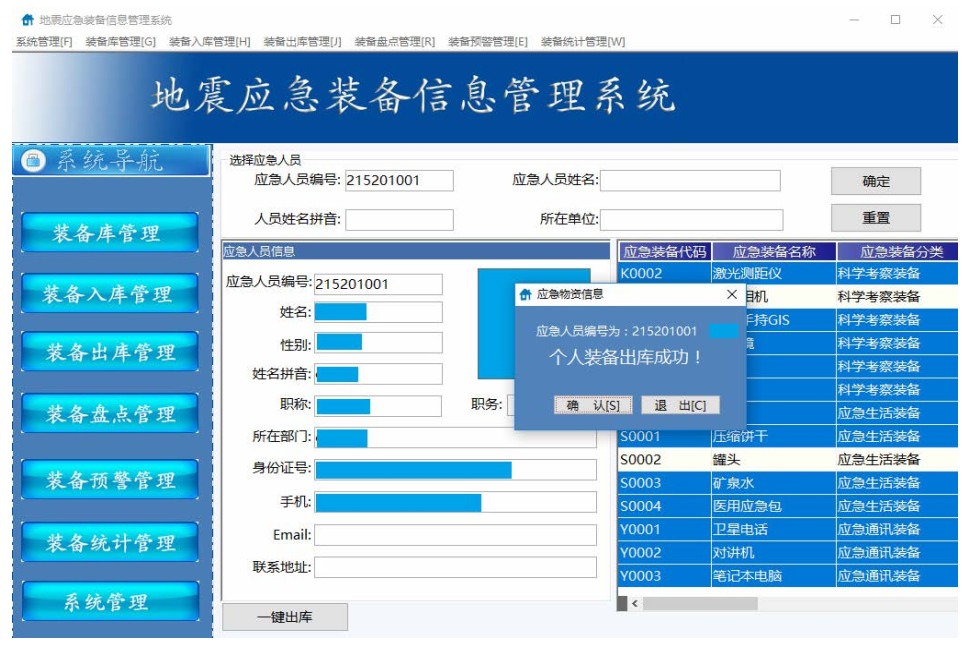
本窗体主要实现了个人应急装备一键出库的功能,用户可以通过查询模块获取应急人员的相关应急装备信息,应急人员的编号、姓名、性别、姓名拼音、所在部门、联系方式、联系地址及照片等,然后在个人应急装备窗口选取要出库的装备,也可以一次性多选,之后点击按钮“一键出库”即可完成个人应急装备出库,其界面如图 6所示。
4. 结论
本文所述系统的设计主要针对当前地震应急装备管理工作的特点,通过分析现有操作方法存在的问题,提出了应用信息化技术解决的策略,旨在提高应急装备的管理水平,进一步促进相关工作的开展,从而提高工作效率。该地震应急装备信息管理系统将日常复杂的应急装备管理工作分解,使其简单化、层次化,同时具有很强的通用性。该系统保证了底层数据的统一性和一致性,通过C/S结构设计增强了访问数据的安全性。随着地震应急信息管理系统投入使用,不仅可以提升日常应急装备管理的水平及工作效率,同时也实现了应急装备科学化、规范化、信息化管理,为我国地震灾后应急工作提供便捷服务。本系统在功能设计上还需要进一步拓展,同时在技术的实现上仍需要进一步的完善,在今后的工作中还需要考虑基于跨平台系统的移植方案等。
地震应急装备信息管理不仅是对现有存储装备场所及作业的管理,更是一种信息化支撑服务,保障了应急装备处置的高效性和科学性。应用测试表明,其有效提高了应急装备管理的工作效率和质量。
-
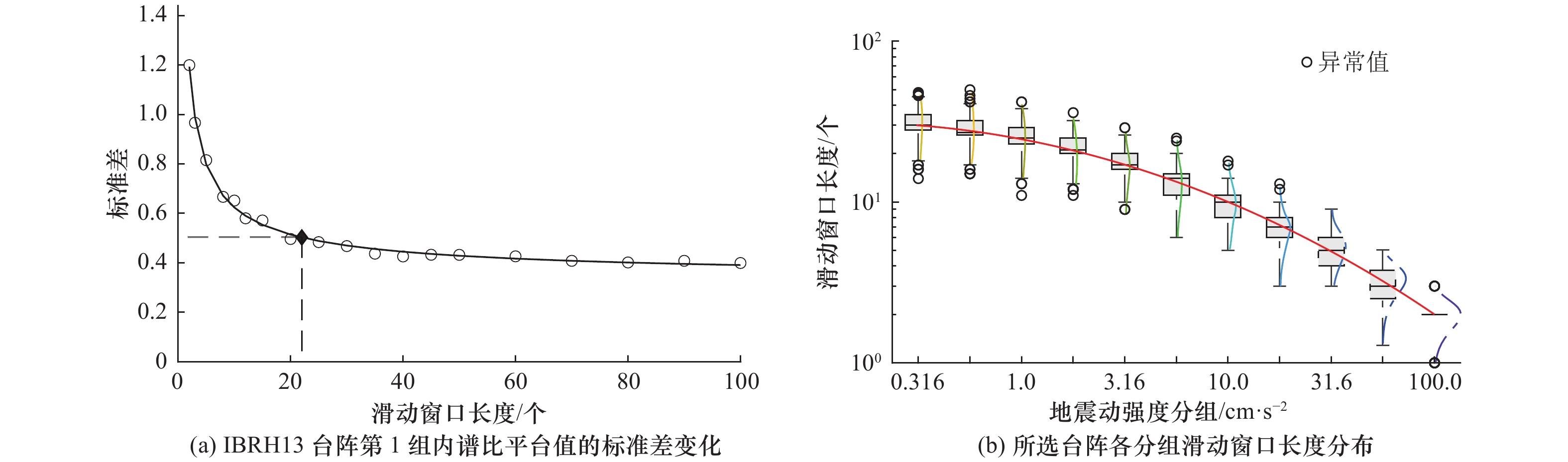
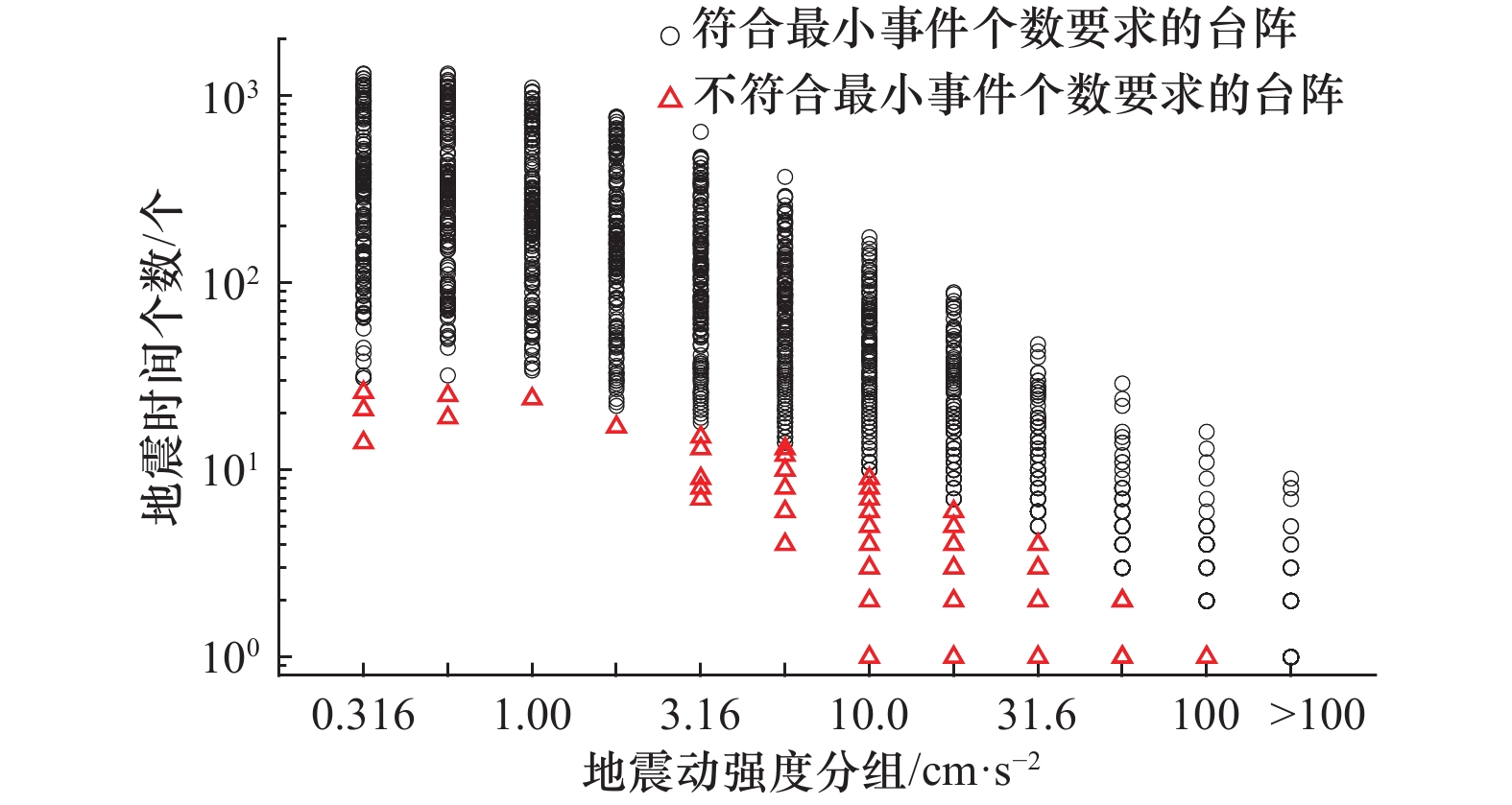
表 1 不同地震动强度分组下的最小可信样本量
Table 1. Minimum reliable sample size for different ground motion intensity groups
组别 1 2 3 4 5 6 7 8 9 10 11 12 分组标准/cm·s−2 0.178~
0.5620.316~
1.0000.562~
1.7801.000~
3.1601.780~
5.6203.160~
10.0005.620~
17.80010.000~
31.60017.800~
56.20031.600~
100.00056.200~
177.800>100.000 n0平均值/个 30 27 25 21 17 14 10 7 5 3 2 1 表 2 场地条件表征参数
Table 2. The site characterization parameters selected in this paper
参数 含义 定义 参考文献 $ {V}_{\rm{se}} $ 我国规范定义的等效剪切波速 ${V}_{\rm{se}}={d}_{0}/{\displaystyle\sum }_{i=1}^{n}\left({d}_{i}/{V}_{\mathrm{s}i}\right)$ 中华人民共和国住房和城乡建设部等,2010 $ {V}_{\mathrm{S}30} $ 美国规范定义的平均剪切波速 ${V}_{\mathrm{S}30}=30/{\displaystyle\sum }_{i=1}^{n}\left({d}_{i}/{V}_{\mathrm{s}i}\right)$ BSSC,2001(美国NEHRP规范) $ {B}_{30} $ 剪切波速剖面梯度 $ {\mathrm{log}}_{10}{V}_{\mathrm{s}}\left(z\right)={B}_{Z\mathrm{m}\mathrm{a}\mathrm{x}}{\mathrm{log}}_{10}\left(z\right)+{A}_{Z\mathrm{m}\mathrm{a}\mathrm{x}}\pm {\sigma }_{Z\mathrm{m}\mathrm{a}\mathrm{x}} $ Régnier等,2013 $ {Z}_{0.5} $ 0.5 km/s覆盖层厚度 剪切波速达0.5 km/s时的土层深度 中华人民共和国住房和城乡建设部等,2010 $ {Z}_{0.8} $ 0.8 km/s覆盖层厚度 剪切波速达0.8 km/s时的土层深度 Zhu等,2020 $ {Z}_{1.0} $ 1.0 km/s覆盖层厚度 剪切波速达1.0 km/s时的土层深度 Zhu等,2020 $ {V}_{\mathrm{s}\mathrm{a}\mathrm{v}} $ 整个土层沉积平均剪切波速 地表到$ {V}_{\mathrm{s}}>800 $ m/s的“地震基岩”
土层厚度内的走时平均剪切波速Pitilakis等,2013 $ {f}_{\mathrm{p}\mathrm{r}\mathrm{e}\mathrm{d}} $ 场地卓越频率 弱震平均傅里叶谱放大系数峰值对应的频率 Régnier等,2013 $ {f}_{\mathrm{s}} $ 场地基本频率 弱震HVSR曲线峰值对应的频率 陈国兴等,2020 $ {T}_{\mathrm{s}} $ 场地基本周期 $ {f}_{\mathrm{s}} $的倒数 陈国兴等,2020 $ {A}_{\mathrm{s}} $ 弱震平均傅里叶谱放大系数峰值 — Régnier等,2013 $ {A}_{\mathrm{s}\mathrm{r}} $ 弱震平均反应谱放大系数峰值 — -
[1] 陈国兴, 谢君斐, 张克绪, 1995. 土的动模量和阻尼比的经验估计. 地震工程与工程振动, 15(1): 73-84.Chen G. X., Xie J. F., Zhang K. X., 1995. The empirical evaluation of soil moduli and damping ratio for dynamic analysis. Earthquake Engineering and Engineering Vibration, 15(1): 73-84. (in Chinese) [2] 陈国兴, 丁杰发, 方怡等, 2020. 场地类别分类方案研究. 岩土力学, 41(11): 3509-3522, 3582.Chen G. X., Ding J. F., Fang Y., et al., 2020. Investigation of seismic site classification scheme. Rock and Soil Mechanics, 41(11): 3509-3522, 3582. (in Chinese) [3] 杜修力, 路德春, 2011. 土动力学与岩土地震工程研究进展. 岩土力学, 32(S2): 10-20.Du X. L., Lu D. C., 2011. Advances in soil dynamics and geotechnical earthquake engineering. Rock and Soil Mechanics, 32(S2): 10-20. (in Chinese) [4] 刘红帅, 2009. 基于小生境遗传算法的设计地震动反应谱标定方法. 岩土工程学报, 31(6): 975-979. doi: 10.3321/j.issn:1000-4548.2009.06.025Liu H. S., 2009. Calibrating method for seismic response spectra based on niche genetic algorithm. Chinese Journal of Geotechnical Engineering, 31(6): 975-979. (in Chinese) doi: 10.3321/j.issn:1000-4548.2009.06.025 [5] 罗桂纯, 2016. 基于强震动观测的场地效应非线性特性研究. 国际地震动态, (1): 46-48. [6] 王海云, 谢礼立, 2010. 自贡市西山公园地形对地震动的影响. 地球物理学报, 53(7): 1631-1638. doi: 10.3969/j.issn.0001-5733.2010.07.014Wang H. Y., Xie L. L., 2010. Effects of topography on ground motion in the Xishan park, Zigong city. Chinese Journal of Geophysics, 53(7): 1631-1638. (in Chinese) doi: 10.3969/j.issn.0001-5733.2010.07.014 [7] 王玉石, 李小军, 兰日清等, 2016. 强震动作用下土体非线性动力特征研究发展与展望. 震灾防御技术, 11(3): 480-492. doi: 10.11899/zzfy20160305Wang Y. S., Li X. J., Lan R. Q., et al., 2016. Development and prospect of study on soil nonlinear dynamic characteristics under strong-motion. Technology for Earthquake Disaster Prevention, 11(3): 480-492. (in Chinese) doi: 10.11899/zzfy20160305 [8] 夏江, 陈清军, 2006. 基于遗传算法的设计地震反应谱标定方法. 力学季刊, 27(2): 317-322. doi: 10.3969/j.issn.0254-0053.2006.02.021Xia J., Chen Q. J., 2006. Calibrating method of seismic response spectrum based on genetic algorithm. Chinese Quarterly of Mechanics, 27(2): 317-322. (in Chinese) doi: 10.3969/j.issn.0254-0053.2006.02.021 [9] 叶鹏, 2013. 四川地区强震动台站的场地反应研究. 哈尔滨: 中国地震局工程力学研究所.Ye P., 2013. Study on site responses of strong motion stations in Sichuan area. Harbin: Institute of Engineering Mechanics, China Earthquake Administration. (in Chinese) [10] 袁晓铭, 孙锐, 孙静等, 2000. 常规土类动剪切模量比和阻尼比试验研究. 地震工程与工程振动, 20(4): 133-139. doi: 10.3969/j.issn.1000-1301.2000.04.020Yuan X. M., Sun R., Sun J., et al., 2000. Laboratory experimental study on dynamic shear modulus ratio and damping ratio of soils. Earthquake Engineering and Engineering Vibration, 20(4): 133-139. (in Chinese) doi: 10.3969/j.issn.1000-1301.2000.04.020 [11] 张立宝, 2018. 基于钻井台阵数据的场地非线性效应研究. 北京: 中国地震局地球物理研究所.Zhang L. B., 2018. Study on nonlinearity of site effects on ground motion based on vertical borehole array data. Beijing: Institute of Geophysics, China Earthquake Administration. (in Chinese) [12] 中华人民共和国住房和城乡建设部, 中华人民共和国国家质量监督检验检疫总局, 2010. GB 50011—2010 建筑抗震设计规范(附条文说明)(2016年版). 北京: 中国建筑工业出版社.Ministry of Housing and Urban-Rural Development of the People’s Republic of China, General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China. 2010. GB 50011—2010 Code for seismic design of buildings. Beijing: China Architecture & Building Press. (in Chinese) [13] Aguirre J., Irikura K., 1997. Nonlinearity, liquefaction, and velocity variation of soft soil layers in Port Island, Kobe, during the Hyogo-ken Nanbu earthquake. Bulletin of the Seismological Society of America, 87(5): 1244-1258. [14] Aki K., 1993. Local site effects on weak and strong ground motion. Tectonophysics, 218(1-3): 93-111. doi: 10.1016/0040-1951(93)90262-I [15] Andrews D. J., 1986. Objective determination of source parameters and similarity of earthquakes of different size. In: Das S., Boatwright J., Scholz C. H., eds., Earthquake Source Mechanics. Washington: American Geophysical Union, 259—267. [16] Beresnev I. A., Field E. H., Van Den Abeele K., et al., 1998. Magnitude of nonlinear sediment response in Los Angeles basin during the 1994 Northridge, California, earthquake. Bulletin of the Seismological Society of America, 88(4): 1079-1084. [17] Bernardie S., Foerster E., Modaressi H., 2006. Non-linear site response simulations in Chang-Hwa region during the 1999 Chi–Chi earthquake, Taiwan. Soil Dynamics and Earthquake Engineering, 26(11): 1038-1048. doi: 10.1016/j.soildyn.2006.02.004 [18] Bonilla L. F., Steidl J. H., Gariel J. C., et al., 2002. Borehole response studies at the garner valley downhole array, Southern California. Bulletin of the Seismological Society of America, 92(8): 3165-3179. doi: 10.1785/0120010235 [19] Bonilla L. F., Tsuda K., Pulido N., et al., 2011. Nonlinear site response evidence of K-NET and KiK-net records from the 2011 off the Pacific coast of Tohoku Earthquake. Earth, Planets and Space, 63(7): 785-789. doi: 10.5047/eps.2011.06.012 [20] Borcherdt R. D., 1970. Effects of local geology on ground motion near San Francisco Bay. Bulletin of the Seismological Society of America, 60(1): 29-61. [21] Building Seismic Safety Council (BSSC), 2001. NEHRP recommended provisions for seismic regulations for new buildings and other structures, 2000 Edition. Washington, DC: Federal Emergency Management Agency. [22] Castro-Cruz D., Régnier J., Bertrand E., et al., 2020. A new parameter to empirically describe and predict the non-linear seismic response of sites derived from the analysis of Kik-Net database. Soil Dynamics and Earthquake Engineering, 128: 105833. doi: 10.1016/j.soildyn.2019.105833 [23] Darragh R. B., Shakal A. F., 1991. The site response of two rock and soil station pairs to strong and weak ground motion. Bulletin of the Seismological Society of America, 81(5): 1885-1899. [24] Field E. H., Johnson P. A., Beresnev I. A., et al., 1997. Nonlinear ground-motion amplification by sediments during the 1994 Northridge earthquake. Nature, 390(6660): 599-602. doi: 10.1038/37586 [25] Frankel A. D., Carver D. L., Williams R. A., 2002. Nonlinear and linear site response and basin effects in Seattle for the M 6.8 Nisqually, Washington, earthquake. Bulletin of the Seismological Society of America, 92(6): 2090-2109. doi: 10.1785/0120010254 [26] Hardin B. O., Drnevich V. P., 1972. Shear modulus and damping in soils: measurement and parameter effects. Journal of the Soil Mechanics and Foundations Division, 98(6): 603-624. doi: 10.1061/JSFEAQ.0001756 [27] Hartzell S., 1998. Variability in nonlinear sediment response during the 1994 Northridge, California, earthquake. Bulletin of the Seismological Society of America, 88(6): 1426-1437. [28] Héloïse C., Bard P. Y., Rodriguez-Marek A., 2012. Site effect assessment using KiK-net data: part 1. A simple correction procedure for surface/downhole spectral ratios. Bulletin of Earthquake Engineering, 10(2): 421-448. doi: 10.1007/s10518-011-9283-1 [29] Huang H. C., Shieh C. S., Chiu H. C., 2001. Linear and nonlinear behaviors of soft soil layers using Lotung downhole array in Taiwan. Terrestrial, Atmospheric and Oceanic Sciences, 12(3): 503-524. doi: 10.3319/TAO.2001.12.3.503(T) [30] Noguchi S., Sasatani T., 2008. Quantification of degree of nonlinear site response. In: The 14th World Conference on Earthquake Engineering. Beijing, China: WCEE. [31] Pavlenko O., Irikura K., 2002. Changes in shear moduli of liquefied and nonliquefied soils during the 1995 Kobe earthquake and its aftershocks at three vertical-array sites. Bulletin of the Seismological Society of America, 92(5): 1952-1969. doi: 10.1785/0120010143 [32] Pavlenko O. V., Wen K. L., 2008. Estimation of nonlinear soil behavior during the 1999 Chi-Chi, Taiwan, Earthquake. Pure and Applied Geophysics, 165(2): 373-407. doi: 10.1007/s00024-008-0309-9 [33] Pender M. J., 1997. Recent developments in earthquake geotechnical engineering. Bulletin of the New Zealand Society for Earthquake Engineering, 30(2): 167-173. doi: 10.5459/bnzsee.30.2.167-173 [34] Pitilakis K., Riga E., Anastasiadis A., 2013. New code site classification, amplification factors and normalized response spectra based on a worldwide ground-motion database. Bulletin of Earthquake Engineering, 11(4): 925-966. doi: 10.1007/s10518-013-9429-4 [35] Régnier J., Cadet H., Bonilla L. F., et al., 2013. Assessing nonlinear behavior of soils in seismic site response: statistical analysis on kik-net strong-motion data. Bulletin of the Seismological Society of America, 103(3): 1750-1770. doi: 10.1785/0120120240 [36] Régnier J., Cadet H., Bard P. Y., 2016. Empirical quantification of the impact of nonlinear soil behavior on site response. Bulletin of the Seismological Society of America, 106(4): 1710-1719. doi: 10.1785/0120150199 [37] Seed H. B., Idriss I. M., Kiefer F. W., 1969. Characteristics of rock motions during earthquakes. Journal of the Soil Mechanics and Foundations Division, 1969, 95(15): 1199-1218. [38] Steidl J. H., Tumarkin A. G., Archuleta R. J., 1996. What is a reference site?. Bulletin of the Seismological Society of America, 86(6): 1733-1748. [39] Tibshirani R., 2011. Regression shrinkage and selection via the lasso: a retrospective. Journal of the Royal Statistical Society: Series B (Statistical Methodology), 73(3): 273-282. doi: 10.1111/j.1467-9868.2011.00771.x [40] Trifunac M. D., Todorovska M. I., 1996. Nonlinear soil response-1994 Northridge, California, earthquake. Journal of Geotechnical Engineering, 122(9): 725-735. doi: 10.1061/(ASCE)0733-9410(1996)122:9(725) [41] Tsuda K., Archuleta R. J., Koketsu K., 2006. Quantifying the spatial distribution of site response by use of the Yokohama high-density strong-motion network. Bulletin of the Seismological Society of America, 96(3): 926-942. doi: 10.1785/0120040212 [42] Wen K. L., Beresnev I. A., Yeh Y. T., 1994. Nonlinear soil amplification inferred from downhole strong seismic motion data. Geophysical Research Letters, 21(24): 2625-2628. doi: 10.1029/94GL02407 [43] Wen K. L., Lin C. M., Chiang H. J., et al., 2008. Effect of surface geology on ground motions: the case of station TAP056-Chutzuhu site. Terrestrial, Atmospheric and Oceanic Sciences, 19(5): 451-462. doi: 10.3319/TAO.2008.19.5.451(T) [44] Zhu C. B., Pilz M., Cotton F., 2020. Which is a better proxy, site period or depth to bedrock, in modelling linear site response in addition to the average shear-wave velocity?. Bulletin of Earthquake Engineering, 18(3): 797-820. doi: 10.1007/s10518-019-00738-6 -







 下载:
下载:





 下载:
下载: