DEM分辨率对提取东北地区坡度的影响研究

刘佳轩; 高瑞; 师黎静

doi:10.11899/zzfy20210202

DEM分辨率对提取东北地区坡度的影响研究

doi: 10.11899/zzfy20210202

刘佳轩^1,,
高瑞^2, ,,
师黎静¹

1.
中国地震局工程力学研究所，地震工程与工程振动重点实验室，哈尔滨 154200
2.
黑龙江科技大学，建筑工程学院，哈尔滨 154200

基金项目: 国家重点研发计划（2018YFC1504601）；国家自然科学基金面上项目（51978635）

详细信息

作者简介:
刘佳轩，男，生于1995年。硕士研究生。主要从事工程抗震方面的研究。E-mail：liujiaxuanhit@163.com

通讯作者:
高瑞，男，生于1993年。硕士研究生。主要从事工程抗震方面的研究。E-mail：gaoruihy@163.com

计量
- 文章访问数: 154
- HTML全文浏览量: 107
- PDF下载量: 12
- 被引次数: 0
出版历程
- 收稿日期: 2021-04-26
- 刊出日期: 2021-06-30

Research on the Influence of DEM Resolution on the Slope Extraction in Northeast, China

Liu Jiaxuan^1
,,
Gao Rui^{2
, ,},
Shi Lijing¹

1.
Key Laboratory of Earthquake Engineering and Engineering Vibration, Institute of Engineering Mechanics, China Earthquake Administration, Harbin 154200, China
2.
Department of Civil Engineering, Heilongjiang University of Science and Technology, Harbin 154200, China

摘要

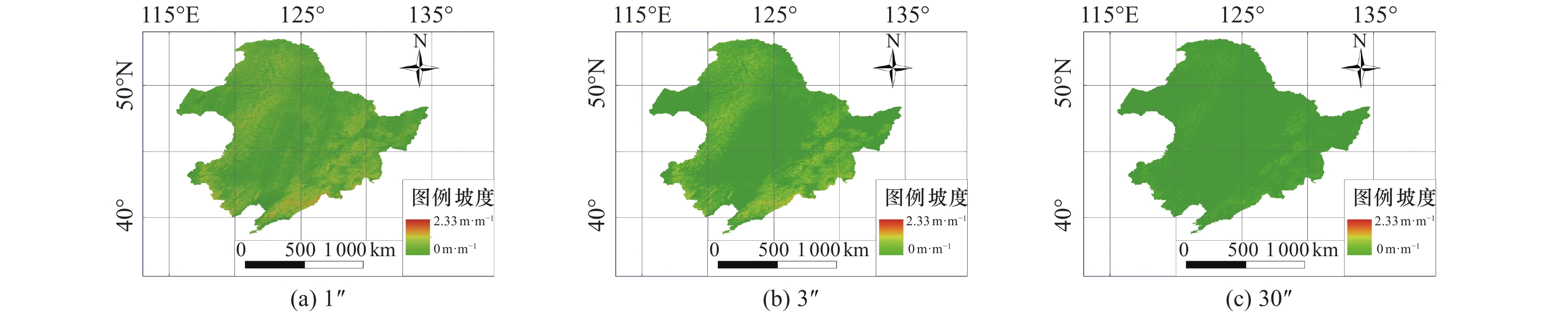
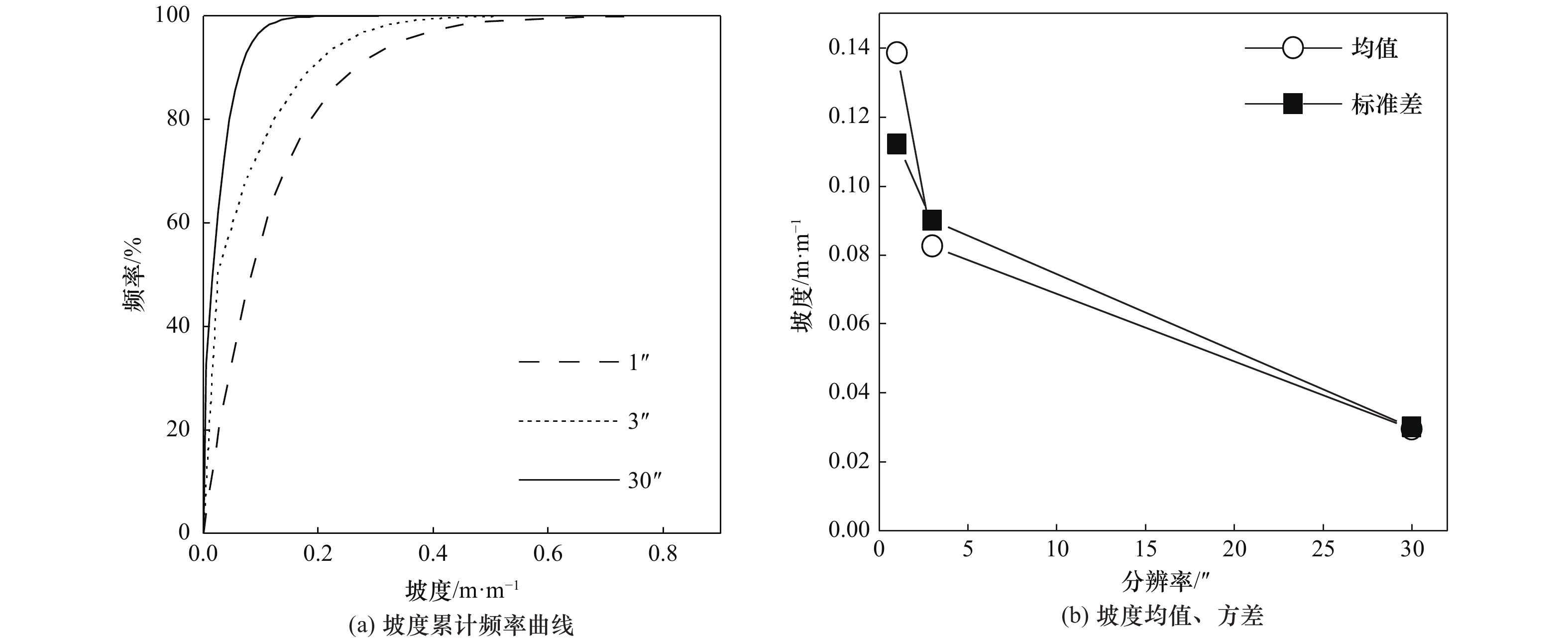
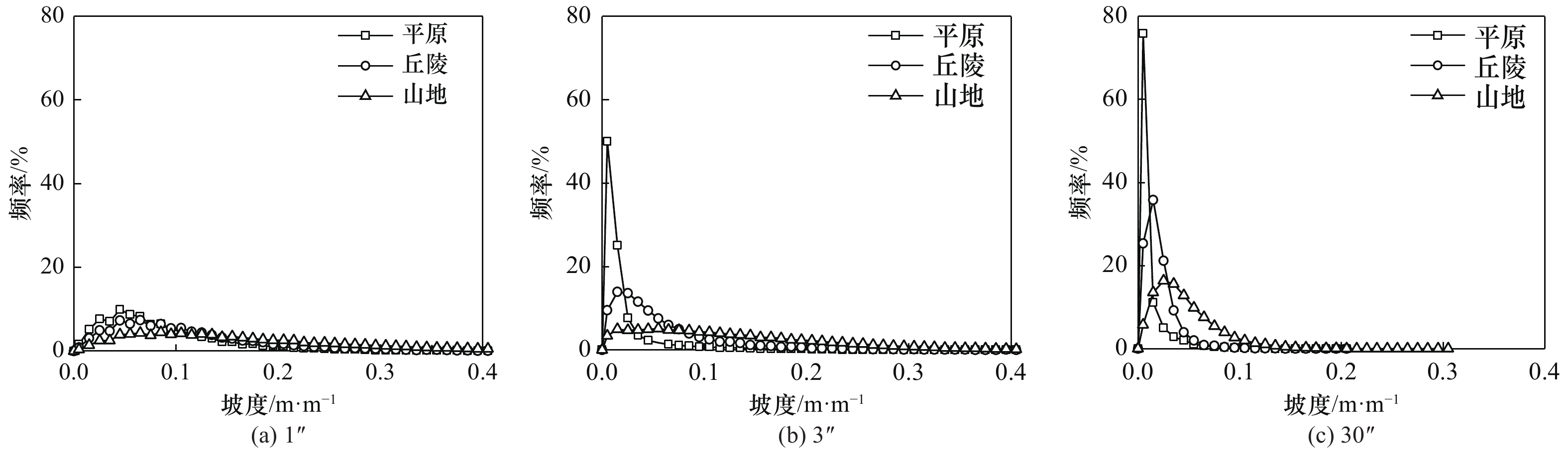
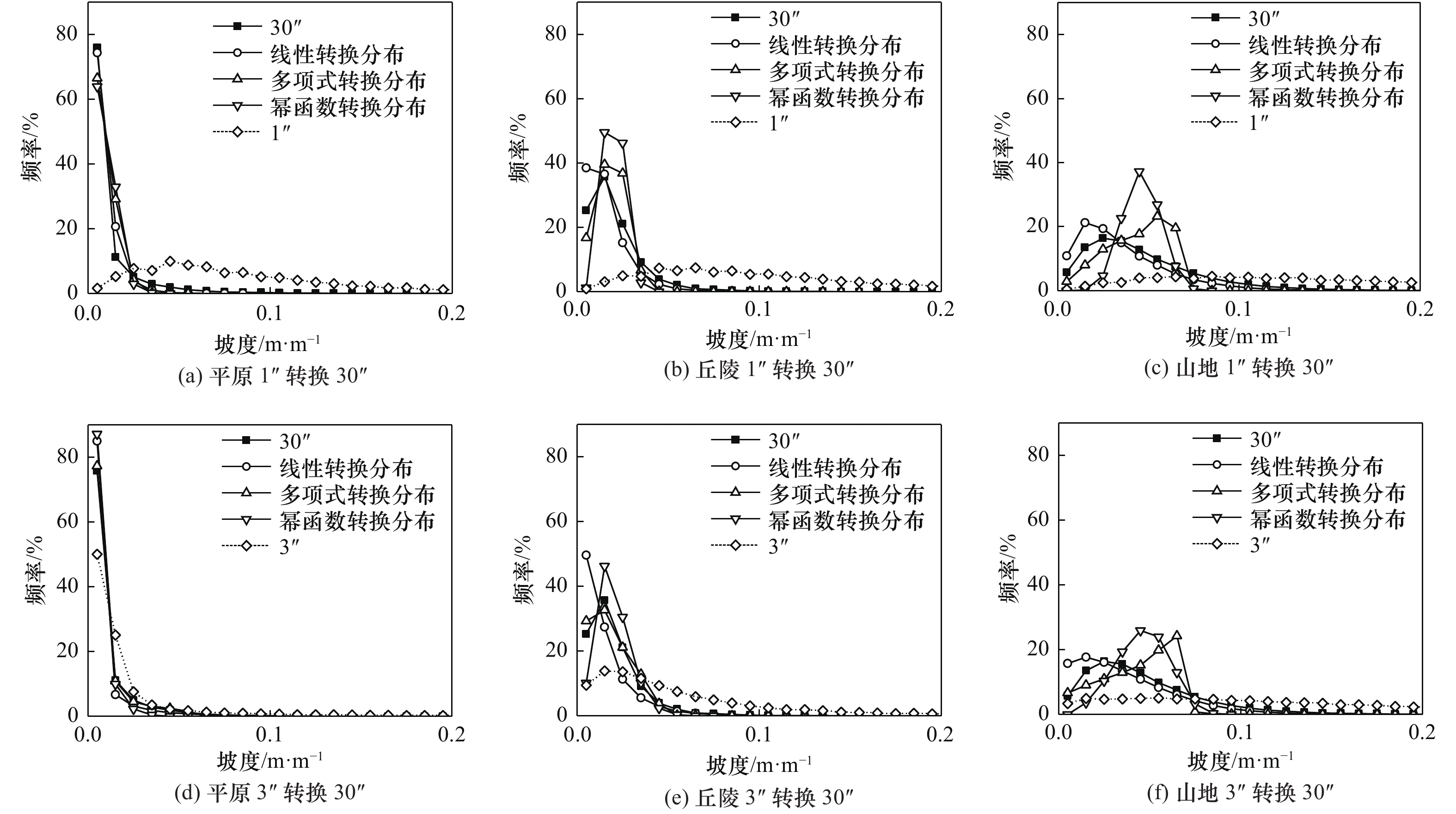
摘要: 目前国内外广泛采用的坡度-等效剪切波速关系模型中，坡度主要基于30″分辨率数字高程数据的提取。DEM分辨率对坡度的提取具有重要影响，且对于不同地区、地貌单元的影响不同。本文以我国东北地区1″、3″、30″分辨率DEM为基础，分别提取研究区域内公里网格点的百分比坡度，分析不同分辨率下提取坡度差异的原因。区分平原、丘陵和山地统计分析坡度随分辨率变化特征，通过比较多种拟合模型的拟合优度和坡度分级平均相对差值，给出不同分辨率下的最佳坡度转换关系。研究结果表明，坡度体现计算面积的平均特性，随着分辨率的增大，栅格计算面积减小，导致坡度有所差异，区域坡度变化越大差异越大；在我国东北地区，随着DEM分辨率的降低，坡度-频率分布曲线向低坡度段移动，且均值、方差及值域变小，不同地貌的坡度-频率分布曲线向低坡度方向移动，且峰值区域变窄，其中平原地貌变化趋势性显著，丘陵次之，山地最小；平原及山地地貌最佳坡度拟合为线性拟合，丘陵地貌下1″、3″与30″分辨率下最佳坡度拟合分别为线性拟合和多项式拟合。
- DEM分辨率 /
- 地貌 /
- 坡度 /
- 坡度转换 /
- 场地分类
Abstract: The regional site classification method based on the slope has been successfully applied. The relationship model of slope-equivalent shear wave velocity based on 30 arc-second DEM is widely used at home and abroad. DEM resolution influences the extraction of the slope, and this influence is different in varying regions and landforms. Based on 1 arc-second, 3 arc-second, and 30 arc-second resolution DEM in Northeast China, this paper extracts the percentage slopes of the kilometer grid points and analyzes the reasons for the different slopes. Analyzing the characteristics of slope change with resolution in varying landforms, comparing the goodness of fit and the average relative difference, the best grade conversion relationship for different resolutions is selected. The results indicate the slope reflects the average characteristics of the calculated area. As the resolution increases, the grid area for slope calculation becomes smaller, which is the main reason for the different slopes. And the greater the slope of the area, the more significant the difference. With the decrease of DEM resolution, the frequency distribution curve of slope shifts to the low slope section, and the mean, variance, and range of values become smaller. The frequency distribution curves of varying landforms also move to the low slopes section, and the peak area becomes narrower. Among them, the changing trend of plain landforms is significant, followed by hills and mountains. The best fits for the slopes of plain and mountainous landforms are linear fitting. The best fits for slopes of 1 arc-second, 3 arc-seconds and 30 arc-seconds of hilly landforms are linear fitting and polynomial fitting, respectively.
- DEM resolution /
- Landform /
- Slope /
- Slope conversion /
- Site classification

HTML全文

1. 2020年中国地震概况

2020年，中国境内共发生5.0级及以上地震28次（中国大陆地区发生20次，台湾地区及海域地区发生8次），其中5.0～5.9级地震25次，6.0～6.9级地震3次，最大地震为2020年7月23日发生在西藏那曲市尼玛县的6.6级地震（表1、图1）。2020年中国大陆发生的20次5.0级以上地震中有10次发生在新疆、6次发生在西藏，6.0级以上地震中有2次发生在新疆、1次发生在西藏。

表 1 2020年中国5.0级及以上地震事件目录及成灾事件

Table 1. Catalogue of earthquakes (M_S≥5.0) and disaster - causing events in China, 2020

序号	时间	纬度/°	经度/°	震中位置	震级/M	成灾事件
1	2020-01-16 16:32:38	41.21	83.6	新疆阿克苏地区库车市	5.6	(1)
2	2020-01-18 00:05:50	39.83	77.18	新疆喀什地区伽师县	5.4
3	2020-01-19 21:27:55	39.83	77.21	新疆喀什地区伽师县	6.4	(2)
4	2020-01-19 22:23:01	39.89	77.46	新疆克孜勒苏州阿图什市	5.2
5	2020-01-25 06:56:05	31.98	95.09	西藏昌都市丁青县	5.1
6	2020-01-29 07:39:29	27.16	126.6	东海海域	5.3
7	2020-02-03 00:05:41	30.74	104.46	四川成都市青白江区	5.1
8	2020-02-15 19:00:07	23.95	121.49	台湾花莲县	5.4
9	2020-02-21 02:01:40	34.56	85.68	西藏阿里地区改则县	5.0
10	2020-02-21 23:39:14	39.87	77.47	新疆喀什地区伽师县	5.1
11	2020-03-10 02:12:11	32.84	85.52	西藏阿里地区改则县	5.0
12	2020-03-12 23:44:03	32.88	85.55	西藏阿里地区改则县	5.1
13	2020-03-20 09:33:15	28.63	87.42	西藏日喀则市定日县	5.9
14	2020-03-23 03:21:39	41.75	81.11	新疆阿克苏地区拜城县	5.0
15	2020-04-01 20:23:27	33.04	98.92	四川甘孜州石渠县	5.6	(3)
16	2020-05-03 11:24:40	23.29	121.6	台湾台东县海域	5.4
17	2020-05-06 18:51:00	39.71	74.1	新疆克孜勒苏州乌恰县	5.0
18	2020-05-09 23:35:59	40.77	78.76	新疆阿克苏地区柯坪县	5.2
19	2020-05-18 21:47:59	27.18	103.16	云南昭通市巧家县	5.0	(4)
20	2020-06-14 04:18:59	24.29	122.41	台湾宜兰县海域	5.5
21	2020-06-26 05:05:20	35.73	82.33	新疆和田地区于田县	6.4	(5)
22	2020-07-12 06:38:25	39.78	118.44	河北唐山市古冶区	5.1
23	2020-07-13 09:28:02	44.42	80.82	新疆伊犁州霍城县	5.0
24	2020-07-23 04:07:20	33.19	86.81	西藏那曲市尼玛县	6.6
25	2020-07-26 20:52:27	24.27	122.48	台湾花莲县海域	5.5
26	2020-09-29 04:50:53	22.29	121.1	台湾台东县海域	5.0
27	2020-09-30 12:37:18	24.85	122.14	台湾宜兰县海域	5.0
28	2020-12-10 21:19:58	24.74	121.99	台湾宜兰县海域	5.8
注：“（）”中表示为地震灾害事件。

下载: 导出CSV

| 显示表格

图 1 2020年中国5.0级及以上地震事件及成灾事件空间分布图

Figure 1. Spatial distribution of earthquakes (M_S≥5.0) in China, 2020

下载: 全尺寸图片幻灯片

2. 2020年中国大陆地震灾害情况

2020年，中国大陆地区共发生地震灾害事件5次，造成5人死亡，30人受伤，直接经济损失约18.47亿元（表2）。其中，灾害损失最严重的地震为1月19日新疆伽师6.4级地震，造成1人死亡，2人受伤，直接经济损失15.26亿元。人员伤亡最严重的地震为5月18日云南巧家5.0级地震，造成4人死亡，28人受伤，直接经济损失约1.04亿元。

表 2 2020年中国大陆地震灾害损失

Table 2. Losses caused by earthquake disasters in China mainland, 2020

序号	时间	震中位置	震级/M	死亡人数/人	受伤人数/人	直接经济损失/万元
1	2020-01-16 16:32	新疆阿克苏地区库车市	5.6	0	0	712
2	2020-01-19 21:27	新疆喀什地区伽师县	6.4	1	2	152 642
3	2020-04-01 20:23	四川甘孜州石渠县	5.6	0	0	19 242.69
4	2020-05-18 21:47	云南昭通市巧家县	5.0	4	28	10 430
5	2020-06-26 05:05	新疆和田地区于田县	6.4	0	0	1 650

下载: 导出CSV

| 显示表格

3. 2020年中国大陆地区地震灾害特点

（1）地震成灾事件总体偏少

2020年共发生5次地震灾害事件，低于2000年以来平均水平。

（2）地震灾害相对集中

5次地震灾害事件中有3次发生在新疆维吾尔自治区，共造成1人死亡，2人受伤，直接经济损失约15.5亿元。地震造成的人员伤亡主要集中在云南省，其中云南省巧家县5.0级地震虽未造成巨大的直接经济损失，但造成4人死亡，28人受伤。

发生在新疆维吾尔自治区的3次地震灾害事件分别为：①2020年1月16日16时32分阿克苏地区库车市发生的5.6级地震，震源深度16 km，未造成人员伤亡，直接经济损失712万元。震中位于库车市，极震区烈度为Ⅵ度，Ⅵ度区面积813.6 km²（表3）。灾区场地位于塔里木盆地腹地，场地对地震动有显著的放大作用，易产生不均匀沉降，加重了建筑物震害。灾区农居房屋抗震能力普遍提高，震中“安居富民房”与农村安居房均未出现破坏，有效保护灾区群众生命财产安全，同时缓解了抗震救灾和转移安置压力，仅少数建造年代较早的抗震安居房出现破坏。②2020年1月19日21时27分喀什地区伽师县发生的6.4级地震，震源深度16 km，造成1人死亡，2人受伤，直接经济损失152 642万元。Ⅵ度及以上区总面积为7 599 km²，其中，Ⅵ度区面积4 945 km²；Ⅶ度区面积为2 397 km²；Ⅷ度区面积为257 km²，主要涉及伽师县西克尔库勒镇、古勒鲁克乡。极震区烈度为Ⅷ度，农村安居房设防烈度为8度，安居房主要承重构件未发生明显破坏，有效抵御了本次地震灾害，在保障人民群众生命财产安全及震后转移安置、余震防范中发挥了重要作用。本次地震为前震-主震-余震型，前震为5.4级，主震为6.4级，最大余震为5.2级，对震中区产生多次震害影响，自建砖木结构房屋墙体为黏土砖砌筑，震害叠加效应显著。当地群众防震减灾意识在历次地震灾害实践中不断得到加强，防震减灾意识、应急避险知识和自救互救能力等得到明显提升。③2020年6月26日5时5分，和田地区于田县发生的6.4级地震，震源深度10 km，未造成人员伤亡，直接经济损失1 650万元。于田县阿羌乡3.5 km道路出现塌方，1座中桥出现多处裂缝，1座自建木桥受损，1个涵洞严重受损，1座拱桥受损。本次地震有感范围较广，但破坏性较小。震区附近乡镇村庄居民普遍反映地震造成的晃动强度一般，大部分区域房屋抗震设防水平高，“富民安居工程”房屋和早期的抗震安居房屋基本完好，仅个别民居院内的自建砖木结构房屋出现轻微程度破坏。

表 3 2020年中国大陆地震灾区范围统计

Table 3. Statistics of range of earthquake disaster area in China mainland, 2020

序号	时间	震中位置	震级/M	极震区烈度/度	震源深度/km	乡镇 /个	人口 /人	各烈度区面积/km²
序号	时间	震中位置	震级/M	极震区烈度/度	震源深度/km	乡镇 /个	人口 /人	Ⅴ	Ⅵ	Ⅶ	Ⅷ
1	2020-01-16 16:32	新疆阿克苏地区库车市	5.6	Ⅵ	16	12	685	—	813.6	0	0
2	2020-01-19 21:27	新疆喀什地区伽师县	6.4	Ⅷ	16	12	279 388	—	4 945	2 397	257
3	2020-04-01 20:23	四川甘孜州石渠县	5.6	Ⅶ	10	6	—	—	360	3 140	0
4	2020-05-18 21:47	云南昭通市巧家县	5.0	Ⅵ	8	6	67 083	—	330	0	0
5	2020-06-26 05:05	新疆和田地区于田县	6.4	Ⅷ	10	—	—	—	—	—	—
注：新疆伽师地震Ⅵ度区之外的部分地区也受到波及，个别老旧房屋出现破坏受损现象，为Ⅵ度异常点；四川甘孜州石渠县地震位于Ⅵ度区之外色达县泥朵镇和其他地区也受到波及，零星房屋有破坏现象。

下载: 导出CSV

| 显示表格

（3）地震次生灾害突出

今年发生的地震灾害中，次生灾害多发，如新疆伽师地震造成震区1座水库出现险情，当地政府紧急疏散安置受影响群众，云南巧家地震次生地质灾害造成2人死亡，数人受伤，震区交通等基础设施受损，再次为各级政府敲响警钟，应对西部地区地震次生灾害引起足够重视，才能进一步减轻地震灾害。

4. 1991—2020年主要震害情况

1991—2020年主要震害统计数据如表4所示，3个主要统计数据分布情况（许永江等，1999；郑通彦等，2012，2015a，2015b；陈通等，2016；文鑫涛等，2018；林向洋等，2018，2020a，2020b）如图2所示。由表4和图2可知，2011—2020年共造成2 063.08亿元的经济损失，其中2013、2014年地震造成的灾害是2011年以来最严重的，这两年造成灾害最重的地震分别为2013年4月20日四川芦山7.0级地震和2014年8月3日云南鲁甸6.5级地震，这2年合计的经济损失、人员死亡（失踪）和人员受伤数量分别占2011—2020年总数的82.9%、81.8%和65.5%。

表 4 1991—2020年主要震害统计数据

Table 4. The statistics of the annual damage caused by earthquakes from 1991 to 2020

年份	成灾次数/次	死亡人数/人	受伤人数/人	直接经济损失/亿元
2011	15	32	506	60.11
2012	11	86	1 331	82.88
2013	14	294	15 671	995.36
2014	10	736	3 688	355.64
2015	12	33	1 217	180.00
2016	16	2	103	66.80
2017	11	37	638	217.40
2018	11	0	81	27.30
2019	13	17	411	59.12
2020	5	5	30	18.47
2011—2020	118	1242	23 676	2 063.08
2001—2010	108	72372	398 917	8 984.90
1991—2000	130	564	52 668	120.18

下载: 导出CSV

| 显示表格

图 2 1991—2020年中国大陆成灾地震年度震害分布图

Figure 2. Distribution of annual earthquakes losses in China mainland from 1991 to 2020

下载: 全尺寸图片幻灯片

近10年中国大陆年均成灾地震频次较1991年以来的总体水平略低，近10年期间地震灾害造成的经济损失、人员死亡（失踪）和人员受伤数量分别占30年来的18.47%、1.67%和4.98%，可见地震灾害造成的经济损失、人员伤亡数量均低于1991年以来的平均水平。

5. 结语

2020年我国大陆未发生特重大地震灾害事件，地震灾害损失总体偏轻，成灾地震次数较少，低于2000年以来的平均水平。地震灾害相对集中，5次成灾地震中3次发生在新疆维吾尔自治区，四川和云南各发生1次。地震次生灾害多发，小震致灾致亡现象突出，云南省巧家县5.0级地震造成4人死亡，28人受伤，其中次生地质灾害造成2人死亡。各级政府应对西部地区地震次生灾害引起足够重视，进而减轻人员伤亡。

图 1 东北地区不同分辨率下高程数据模型图

Figure 1. Elevation maps of 1 arc second, 3 arc second, and 30 arc second resolution in the Northeast, China

下载: 全尺寸图片幻灯片

图 2 计算高程梯度变化率的像元窗口

Figure 2. 3×3 unit schematic diagram of slope calculation point

下载: 全尺寸图片幻灯片

图 3 东北地区不同分辨率下坡度图

Figure 3. Slope maps of 1 arc second, 3 arc second, and 30 arc second resolution in the Northeast, China

下载: 全尺寸图片幻灯片

图 4 不同DEM分辨率下的坡度统计

Figure 4. Slope statistics of different DEM resolutions

下载: 全尺寸图片幻灯片

图 5 地貌分布图

Figure 5. Landform distribution map

下载: 全尺寸图片幻灯片

图 6 不同分辨率下不同地貌单元坡度-频率分布曲线

Figure 6. Slope-frequency distribution curves of different topography at different resolutions

下载: 全尺寸图片幻灯片

图 7 不同地貌单元不同分辨率下坡度转换结果

Figure 7. Conversion results of DEM slopes with different resolutions under different landforms

下载: 全尺寸图片幻灯片

表 1 不同地貌单元回归模型拟合参数及评价参数（1″与30″分辨率下）

Table 1. Fitting and evaluation parameters of regression models for different landforms (1 arc second and 30 arc second)

地貌单元类型	拟合模型	拟合参数				R²	Δ_mean	K
地貌单元类型	拟合模型	a	b	c	d	R²	Δ_mean	K
平原	线性函数	0	0.085 63	—	—	0.313 40	0.535 7	0.585 0
	多项式函数	0.114 600	−0.154 80	0.090 50	0	0.322 20	0.763 9	0.421 7
	幂函数	0.047 160	0.658 50	0	—	0.080 00	0.892 3	0.089 6
丘陵	线性函数	0	0.132 57	—	—	0.552 50	0.320 8	1.722 2
	多项式函数	0.259 270	−0.736 80	0.711 46	0	0.610 81	0.486 5	1.255 5
	幂函数	0.043 249	0.329 23	0	—	0.077 8	0.842 3	0.092 3
山地	线性函数	0	0.199 24	—	—	0.598 49	0.345 1	1.734 2
	多项式函数	0.445 110	−0.996 52	0.686 59	0	0.687 30	0.714 8	0.961 5
	幂函数	0.079 990	0.287 73	0	—	0.092 45	0.965 3	0.095 7

下载: 导出CSV

表 2 不同地貌单元回归模型拟合参数及评价参数（3″与30″分辨率下）

Table 2. Fitting and evaluation parameters of regression models for different landforms (3 arc second and 30 arc second)

地貌	拟合模型	拟合参数				R²	Δ_mean	K
地貌	拟合模型	a	b	c	d	R²	Δ_mean	K
平原	线性函数	0	0.180 0	—	—	0.313 40	0.106 7	2.937 20
	多项式函数	0.485 0	−1.476 0	1.646	0	0.322 20	0.324 4	0.993 20
	幂函数	0.160 0	0.713 0	0	—	0.080 00	0.525 9	0.152 10
丘陵	线性函数	0	0.246 0	—	—	0.552 50	0.385 1	1.434 70
	多项式函数	0.449 0	−1.695 0	2.337	0	0.610 81	0.410 1	1.489 40
	幂函数	0.082 1	0.465 0	0	—	0.077 80	0.579 9	0.134 10
山地	线性函数	0	0.279 8	—	—	0.598 49	0.354 1	1.690 10
	多项式函数	0.635 0	−2.104 0	2.366	0	0.687 30	0.716 0	0.959 90
	幂函数	0.103 6	0.360 2	0	—	0.092 45	0.782 7	0.118 11

下载: 导出CSV

表 3 不同地貌单元不同分辨率下坡度转换公式

Table 3. The slope conversion formula of different resolution DEM for different landforms

地貌单元类型	与30″的转换关系
地貌单元类型	1″（i=1″）	3″（i=3″）
平原	$ {S_{30}} = {\text{0}}{\text{.085 63}}{S_{{i}}} $	$ {S_{30}} = {\text{0}}{\text{.18}}{S_{{i}}} $
丘陵	$ {S_{30}} = {\text{0}}{\text{.132 57}}{S_{{i}}} $	$ {S_{30}} = {\text{0}}{\text{.449}}{S_{{i}}}{{ - 1}}{\text{.695}}{S_{{i}}}^{\text{2}}{\text{ + 2}}{\text{.337}}{S_{{i}}}^{\text{3}} $
山地	$ {S_{30}} = {\text{0}}{\text{.199 24}}{S_{{i}}} $	$ {S_{30}} = {\text{0}}{\text{.279 8}}{S_{{i}}} $

下载: 导出CSV

参考文献(19)

[1]	李昕蕾, 2020. 基于多因素的区域场地分类方法研究. 哈尔滨: 中国地震局工程力学研究所. Li X. L., 2020. Regional site classification method based on multiple factors. Harbin: Institute of Engineering Mechanics, China Earthquake Administration. (in Chinese)
[2]	刘飞, 范建容, 崔兆岩等, 2019. 基于DEM分形特征的坡度尺度变换模型. 山地学报, 37(1): 129—136 Liu F., Fan J. R., Cui Z. Y., et al., 2019. A model of re-scaling slope based on DEM fractal feature. Mountain Research, 37(1): 129—136. (in Chinese)
[3]	刘利峰, 2018. DEM分辨率对白马小流域坡度与坡向提取的影响. 山西水土保持科技, (2): 10—12 doi: 10.3969/j.issn.1008-0120.2018.02.005 Liu L. F., 2018. DEM resolving capability influence on extract slope and aspect in Baima minor watershed. Soil and Water Conservation Science and Technology in Shanxi, (2): 10—12. (in Chinese) doi: 10.3969/j.issn.1008-0120.2018.02.005
[4]	刘晓, 赵荣, 梁勇等, 2017. 顾及地貌与DEM分辨率的坡度算法适应性研究. 测绘科学, 42(3): 29—34 Liu X., Zhao R., Liang Y., et al., 2017. Research of algorithms for deriving slope based on different geomorphic types and DEM resolution. Science of Surveying and Mapping, 42(3): 29—34. (in Chinese)
[5]	乔之轩, 2019. 基于DEM的多分辨率水文特征提取. 北京: 北京林业大学. Qiao Z. X., 2019. Multi-resolution hydrological feature extraction based on DEM. Beijing: Beijing Forestry University. (in Chinese)
[6]	师动, 朱奇峰, 杨勤科等, 2020. DEM分辨率对坡度算法选择影响的分析. 山地学报, 38(6): 935—944 Shi D., Zhu Q. F., Yang Q. K., et al., 2020. DEM resolution influence on slope algorithm selection. Mountain Research, 38(6): 935—944. (in Chinese)
[7]	史大成, 2009. 基于GIS的场地分类新方法研究. 哈尔滨: 中国地震局工程力学研究所. Shi D. C., 2009. Study on new methods of site classification based on GIS. Harbin: Institute of Engineering Mechanics, China Earthquake Administration. (in Chinese)
[8]	土祥, 王春梅, 庞国伟等, 2018. 黄土丘陵沟壑区坡度尺度效应空间分异分析. 山地学报, 36(6): 964—972 Tu X., Wang C. M., Pang G. W., et al., 2018. On the spatial differentiation of scale effect of slopes in Loess Hill and Gully Region, China. Mountain Research, 36(6): 964—972. (in Chinese)
[9]	王英, 龚家国, 贾仰文等, 2019. 基于不同分辨率DEM提取坡度值的转换关系研究. 水利水电技术, 50(8): 45—51 Wang Y., Gong J. G., Jia Y. W., et al., 2019. Study on conversion relationship of slope information extracted from different resolution DEM. Water Resources and Hydropower Engineering, 50(8): 45—51. (in Chinese)
[10]	吴效勇, 2019. 基于多因素的地震影响场研究. 北京: 中国地震局地震预测研究所. Wu X. Y., 2019. Study on seismic influence field based on multi-factors. Beijing: Institute of Earthquake Forecasting, China Earthquake Administration. (in Chinese)
[11]	Grohmann C. H., 2015. Effects of spatial resolution on slope and aspect derivation for regional-scale analysis. Computers & Geosciences, 77: 111—117.
[12]	Horn B. K. P., 1981. Hill shading and the reflectance map. Proceedings of the IEEE, 69(1): 14—47. doi: 10.1109/PROC.1981.11918
[13]	Mukherjee S., Mukherjee S., Garg R. D., et al., 2013. Evaluation of topographic index in relation to terrain roughness and DEM grid spacing. Journal of Earth System Science, 122(3): 869—886. doi: 10.1007/s12040-013-0292-0
[14]	Olaya V., 2009. Basic land-surface parameters. Developments in Soil Science, 33: 141—169.
[15]	Thompson E. M., Wald D. J., Worden C. B., 2014. A Vs30 map for California with geologic and topographic constraints. Bulletin of the Seismological Society of America, 104(5): 2313—2321. doi: 10.1785/0120130312
[16]	Thornley J. H. M., 1976. Mathematical models in plant physiology. London: Academic Press, 86—110.
[17]	Wald D. J., Earle P. E., Lin K. W., et al., 2006. Challenges in rapid ground motion estimation for the prompt assessment of global urban earthquakes. Bulletin of the Earthquake Research Institute, 81: 273—281.
[18]	Walker J. P., Willgoose G. R., 1999. On the effect of digital elevation model accuracy on hydrology and geomorphology. Water Resources Research, 35(7): 2259—2268. doi: 10.1029/1999WR900034
[19]	Wang C. M., Yang Q. K., Guo W. L., et al., 2012. Influence of resolution on slope in areas with different topographic characteristics. Computers & Geosciences, 41: 156—168.