Study on Failure Mode and Deformation Capacity of Reinforced Concrete Columns
-
摘要: 对钢筋混凝土（RC）柱在地震作用下的变形性能进行量化，本文从太平洋地震研究中心柱数据库中收集到123根RC柱抗震性能试验数据，提出基于参数剪跨比和弯剪比的RC柱破坏形态判别标准；在弯曲破坏、弯剪破坏、剪切破坏三种破坏形态下，研究了轴压比、剪跨比、配箍特征值等参数对位移角的显著性影响，通过回归分析归纳出三种破坏形态下屈服位移角和极限位移角的回归方程，回归系数显著性概率均小于0.05。结果表明：本文提出的RC柱破坏形态判别标准准确度高，适应性强；位移角线性回归方程具有合理性。Abstract: In order to quantify the deformation performance and failure characteristics of RC columns under earthquake action, this paper collected 123 reinforced concrete (RC) column test data from PEER (Pacific Earthquake Research Center) database, and proposed the standard of RC column failure morphology based on parameter shear span ratio and bending-shear ratio. Under the three failure modes of bending failure, bending shear failure and shear failure, the significant effects of parameters such as axial compression ratio, shear span ratio, hoop characteristic value, volume hoop ratio, longitudinal reinforcement ratio on the displacement angle were studied. Through regression analysis, the regression equations of yield displacement angle and ultimate displacement angle under three failure modes were summarized and the significance probability of regression coefficient is less than 0.05. The results show that the criterion of RC column damage morphology proposed in this paper has high accuracy and strong adaptability; the linear regression equation of displacement angle is reasonable.
-
Key words:
- RC frame column /
- Deformation limit /
- Damage form discrimination /
- Displacement angle
-
引言
防震减灾是国家公共安全的重要组成部分，是重要的基础性、公益性事业，事关人民生命财产安全和经济社会可持续发展。研究表明，浅层地质构造和深部地壳结构有着复杂的几何、力学关系，当地震发生时，对地壳浅部隐伏活断裂附近的地面运动具有明显的放大作用，严重破坏地表建(构)筑物，造成重大人员伤亡和经济损失(邓起东等，2007；徐锡伟等，2008；酆少英等，2010；赵成彬等，2013；朱国军等，2018)。因此，响应国家大力推动新时代防震减灾事业现代化建设号召，开展城市活动断层探测，了解隐伏断层性质、特点及空间展布特征，及时采取活动断裂带避让和建(构)筑物加固等措施，可极大减轻地震灾害对城市的影响(李倩等，2019)，对于全面建成小康社会、构建和谐社会具有十分重要的意义。
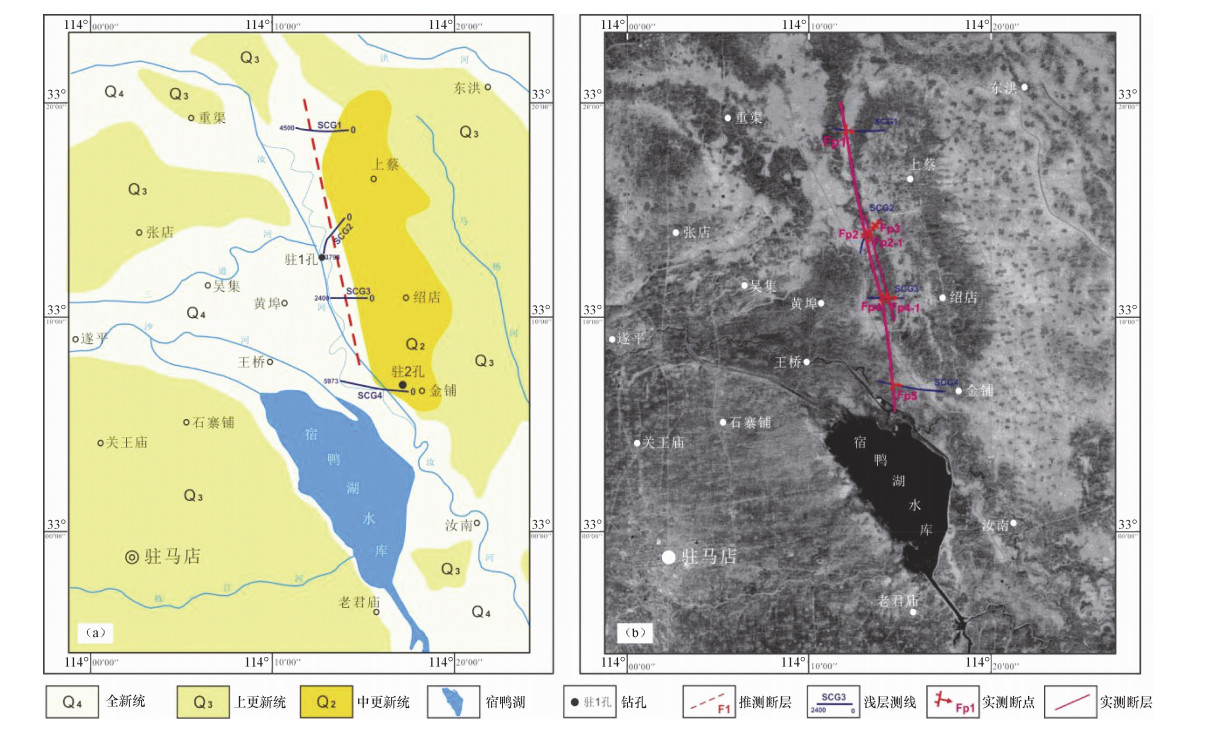
河南省驻马店市位于华北盆地和秦岭褶皱带接壤地带，构造复杂，既有华北盆地的剪切拉张构造，又有秦岭褶皱带的挤压逆冲-走滑断裂。已有地质资料显示，上蔡岗断裂为隐伏逆断层，最新活动时代为第四纪。研究区第四纪地质图和遥感解译影像如图 1所示，由图 1可知，上蔡岗断裂北东盘为岗地，岗地轮廓清晰，地表主要出露中更新统，断裂南西盘为平原，地表主要为晚更新统和全新统(中国地震局地球物理勘探中心，2019)。为研究上蔡岗断裂浅部构造特征，笔者依托《驻马店市活断层探测与地震危险性评价服务》项目，跨该断裂布设高分辨率浅层地震测线，利用获得的浅层地震剖面对上蔡岗断裂浅部特征进行分析，研究结果可为驻马店市地震危害性评价及城市规划提供地质和地球物理学依据。
图 1 研究区第四纪地质图和遥感解译影像Figure 1. Quaternary geological map and remote sensing interpretation image of the study area1. 高分辨率浅层地震测线位置与工作方法
高分辨率浅层地震勘探是目前隐伏断层的主要探测方法，该方法通过采用“小道距、小炮间距、高覆盖次数”采集技术及高精度数据处理手段，既可很好地压制干扰波、提高地震资料信噪比，又可获得直观反映地下构造形态的高分辨率反射剖面，有助于判断断层的位置与形态(何正勤等，2001；刘保金等，2002；谭雅丽等，2017)。受施工条件限制，高分辨率浅层地震测线主要沿市政道路布设，为使探测结果能准确反映断裂构造特征，布设测线时应尽量选取与断层垂直的线路。为研究上蔡岗断裂浅部构造特征，参考已有地质资料，在上蔡岗断裂目标区由北至南布设4条高分辨率浅层地震测线，编号SCG1—SCG4，具体位置如图 1所示。
地震数据采集是地震勘探工作的关键环节之一，而地震仪器设备性能影响地震数据的采集质量(何正勤等，2001；刘保金等，2002)。本次浅层地震勘探采用法国Sercel公司生产的SN388型遥测数字地震仪，该仪器具有瞬时动态范围大、谐波畸变极小、记录频带宽和带道能力强等优点，内设排列监视、数据监控和各种测试功能，现场可随时监视记录质量和设备工作状态，较好地满足了高分辨率浅层地震勘探数据采集的技术要求。地震波激发采用美国生产的M18-612型可控震源，该震源具有激发能量强、重复性好和抗干扰能力强的特点。为了尽可能地获得频带宽、主频高的地震信号，数据采集时采用既可压制低频干扰，又可拓宽记录高频上限的高频地震检波器。根据以往城市活断层探测经验，本次浅层地震探测采用4个固有频率为60Hz的高频检波器组合接收。
施工过程中，为得到高分辨率地震反射数据，根据现场试验结果采用可控震源线性扫描，扫描频率20—160Hz，扫描长度12s，采样率1ms，记录长度2s。考虑不同测线地质条件和工作环境可能不同，根据现场试验情况对每条测线采用不同的观测系统参数，如表 1所示。
表 1 浅层地震测线观测系统参数Table 1. Geometry parameters of shallow seismic lines测线名称 探测方向 道间距/m 炮间距/m 接收排列 接收道数 覆盖次数 测线长度/m SCG1 E-W 2 10 7-186 180 18 4500 SCG2 NE-SW 3 15 6-185 180 18 3798 SCG3 E-W 3 15 6-185 180 18 2400 SCG4 E-W 3 15 6-185 180 18 5973 2. 高分辨率浅层地震剖面与解释结果
2.1 SCG1测线
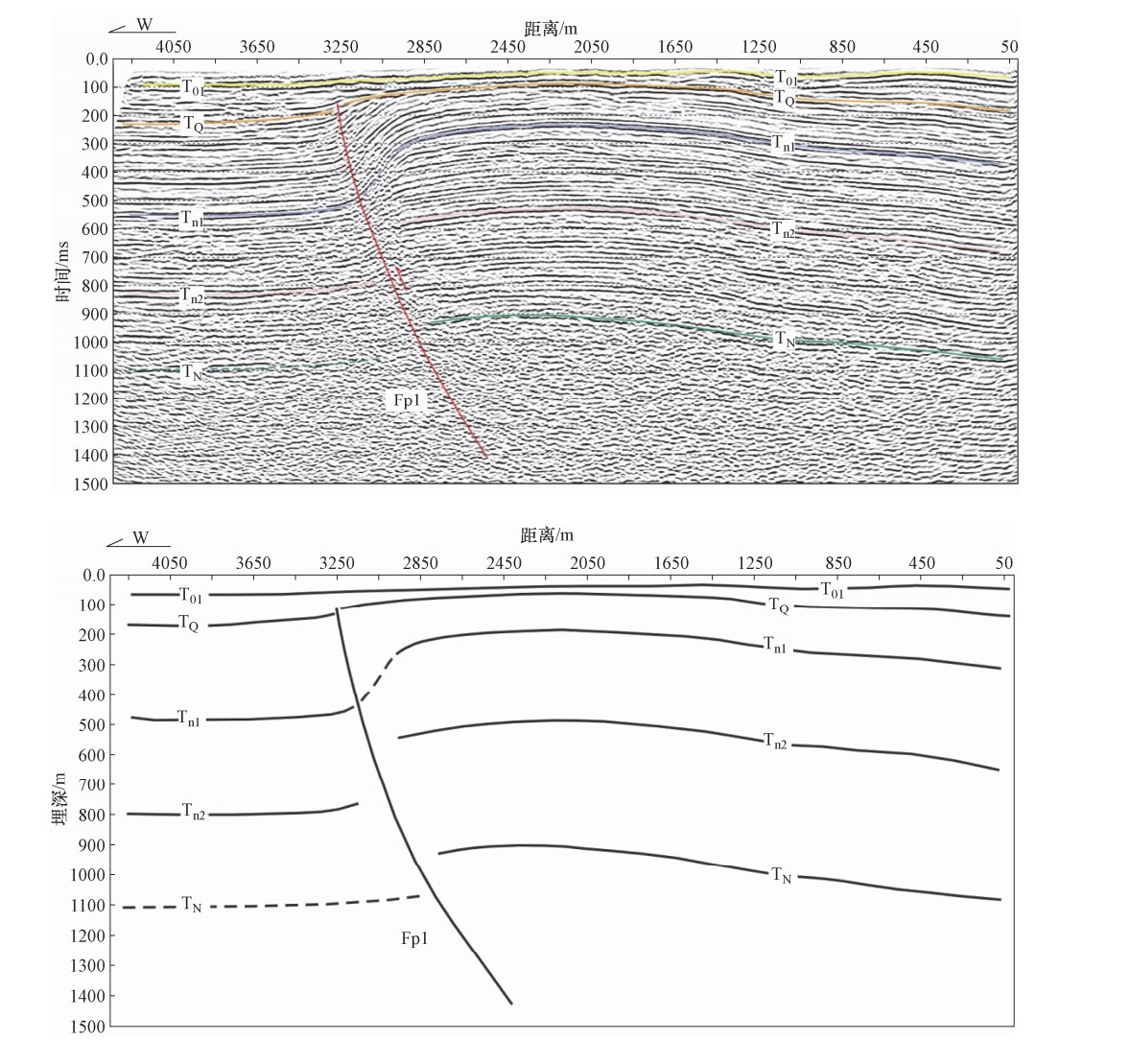
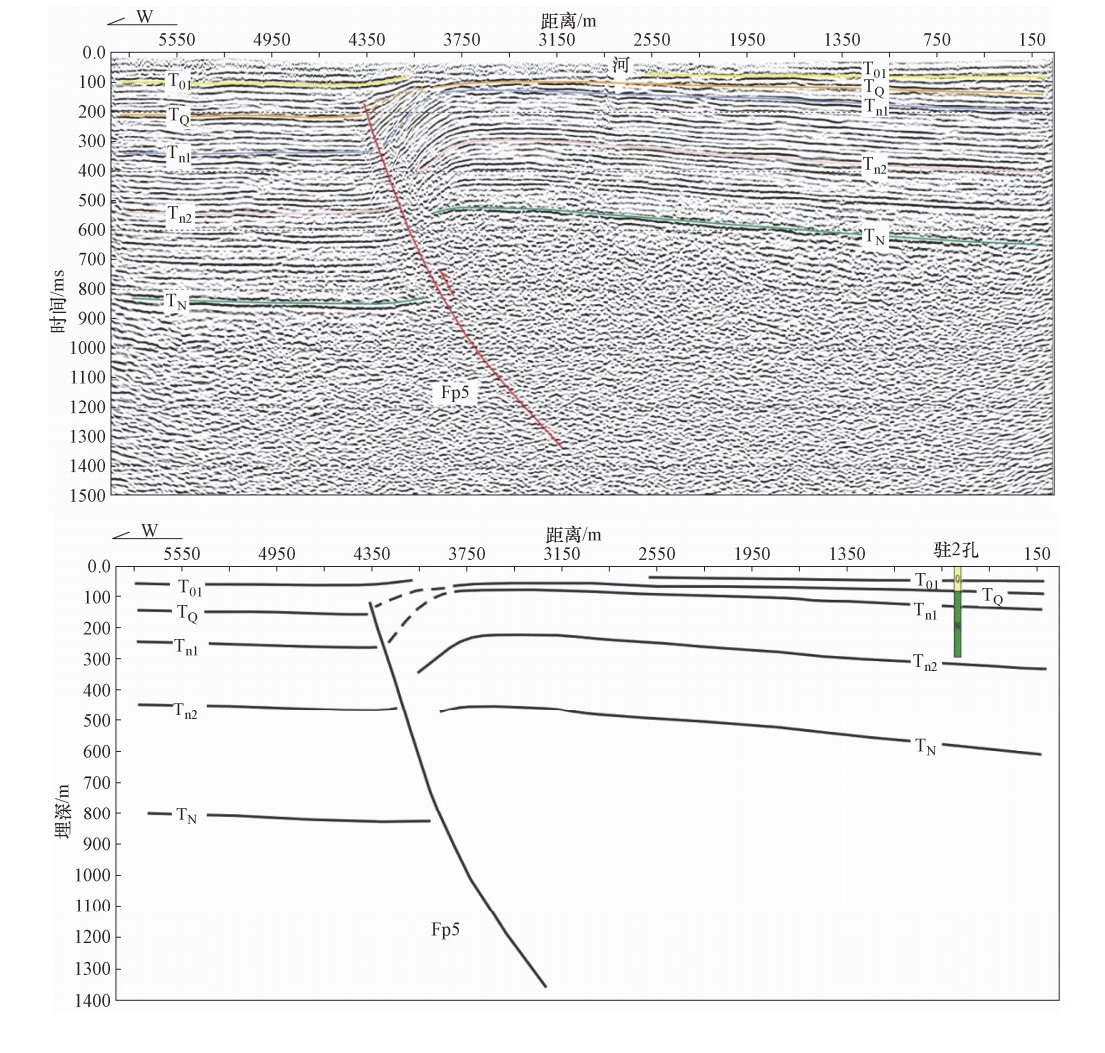
SCG1测线近东-西向布设于上蔡县北部的蔡州大道上，全长4500m。图 2所示为SCG1测线地震反射波时间剖面和地质解释，时间剖面上反射信息丰富，反射波能量较强，剖面中段反射同相轴有明显上拱特征，与地表岗地特征基本一致。根据反射波震相特征和反射波组关系，在剖面上解释了5个反射同相轴，根据已有地质资料及驻1孔、驻2孔分层数据，同相轴TQ、TN分别解释为第四系、新近系底界面反射，同相轴T01为第四系内部反射，同相轴Tn1、Tn2为新近系内部反射。在剖面桩号3270m附近，TQ及以下各反射同相轴存在明显的扭曲或错断，应为断层在剖面上的反映，在桩号3267m处解释了1个视倾向东的逆断点，用Fp1标记，可分辨上断点埋深约100—105m，向上错断了第四系底界面TQ。
图 2 SCG1测线地震反射波时间剖面及地质解释Figure 2. Seismic reflection wave time-section and geological interpretation of line SCG12.2 SCG2测线
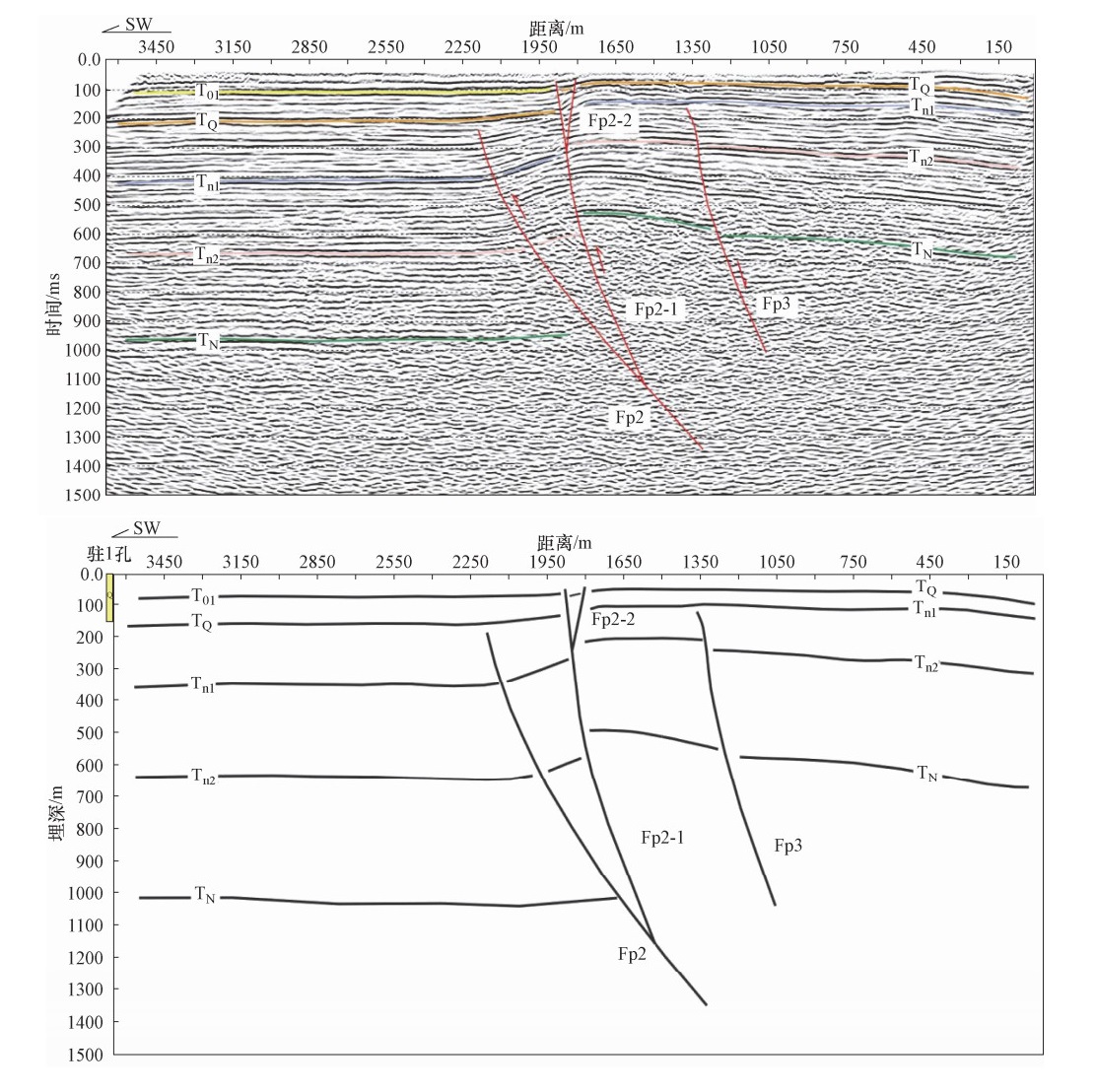
SCG2测线近北东-南西向布设于上蔡县去往驻马店市区的省道上，全长3798m。距测线东南端约100m的驻1孔深度约170m，根据测年数据及岩心分析，全新统底界埋深2.35m，上更新统底界埋深12.45m，中更新统底界埋深24.0m，未钻穿下更新统底界。图 3所示为SCG2测线地震反射波时间剖面和地质解释，时间剖面上反射信息丰富，反射波能量较强，根据反射波震相特征和反射波组关系，在剖面上解释了5个反射同相轴，全新世、晚更新世和中更新世地层埋藏较浅，在地震反射波时间剖面上无连续反射。剖面桩号1300—2250m间存在明显的同相轴缺失和错断，应是断层在剖面上的表现，在剖面上解释了4个断点，用Fp2、Fp2-1、Fp2-2和Fp3标记。Fp2为逆断层，视倾向北东，可分辨上断点位于测线桩号2188m，埋深约185—190m。Fp2-1为逆断层，视倾向北东，可分辨上断点位于测线桩号1881m，埋深约50—55m。Fp2和Fp2-1在剖面上呈反y形，其间可见反射同相轴明显扭曲。Fp2-2为正断层，视倾向南西，可分辨上断点位于测线桩号1805m，埋深约40—45m。Fp3为正断层，视倾向北东，可分辨上断点位于测线桩号1364m，埋深约120—125m。Fp2-2和Fp3应是逆断层上升盘膝折带受力作用生成的正断层。Fp2和Fp3未错断第四系底界面TQ，Fp2-1和Fp2-2错断了第四系内地层界面T01。
图 3 SCG2测线地震反射波时间剖面及地质解释Figure 3. Seismic reflection wave time-section and geological interpretation of line SCG22.3 SCG3测线
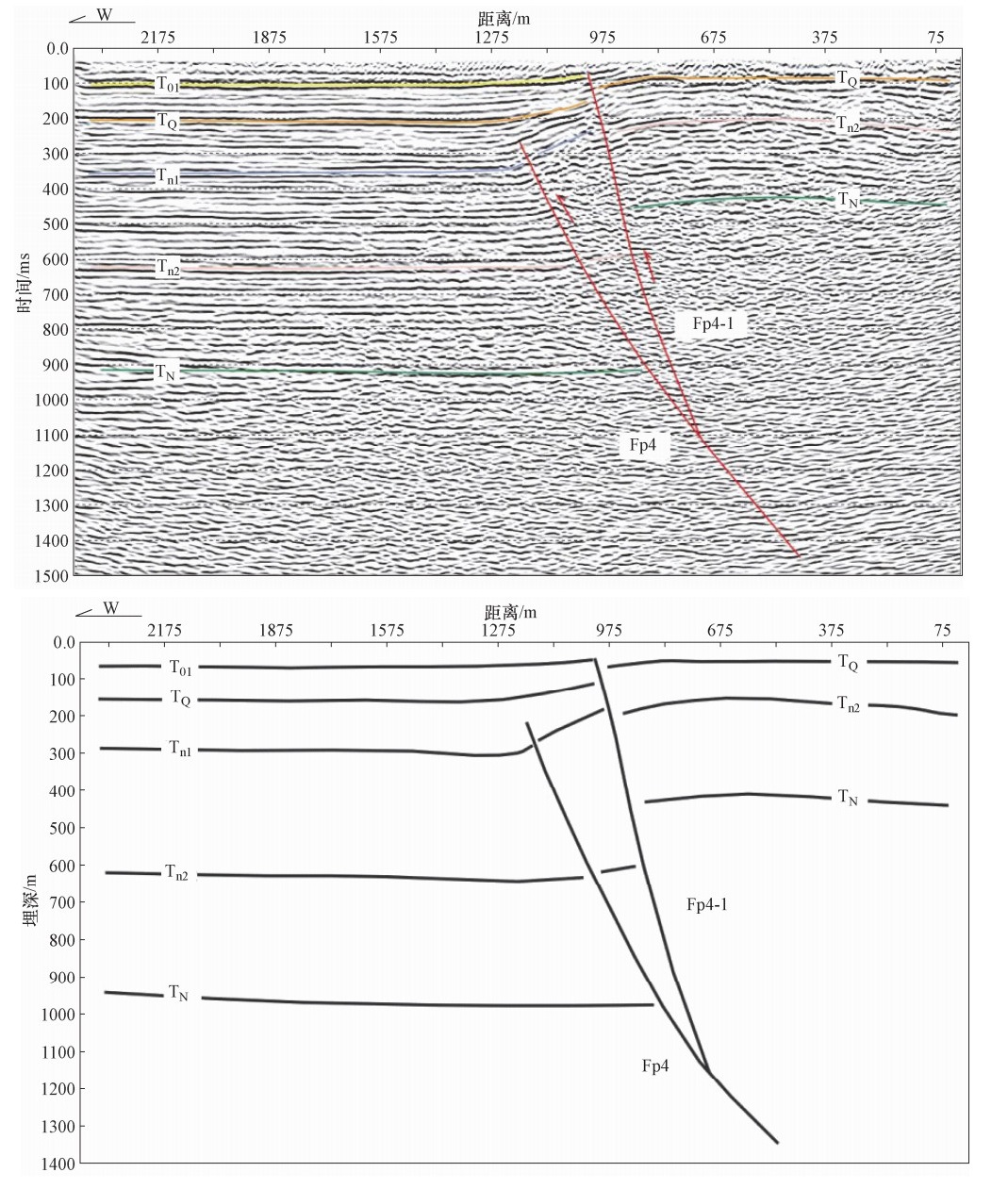
SCG3测线近东-西向布设于上蔡县邵店乡去往黄埠镇的水泥路上，全长2400m。图 4所示为SCG3测线地震反射波时间剖面和地质解释，该测线较短，剖面整体形态与SCG2剖面相似。时间剖面上反射信息丰富，反射波能量较强，解释了5个反射同相轴。反射时间剖面桩号950—1250m间存在明显的同相轴缺失和错断，应是断层的表现，在剖面上解释了2个断点，用Fp4和Fp4-1标记。Fp4为逆断层，视倾向东，可分辨上断点位于测线桩号1193m，埋深约222—227m。Fp4-1为逆断层，视倾向东，可分辨上断点位于测线桩号1011m，埋深约45—50m。Fp4和Fp4-1在剖面上呈反y形分布，其间可见反射同相轴明显扭曲和数目的减少。对比SCG2剖面，SCG3剖面上逆断层上升盘埋深明显变浅、同相轴连续性减弱，仅剩3个同相轴(TQ、Tn2和TN)可追踪，推测断点Fp4和Fp4-1应该是SCG2剖面上断点Fp2和Fp2-1在SCG3剖面上的反映。断点Fp4未错断第四系底界面TQ，断点Fp4-1错断了第四系内地层界面T01。
图 4 SCG3测线地震反射波时间剖面及地质解释Figure 4. Seismic reflection wave time-section and geological interpretation of line SCG32.4 SCG4测线
SCG4测线近东-西向布设于汝南县金铺镇去往张楼乡的县道上，全长5973m。驻2孔位于测线桩号645m北侧约400m处，深度约300m，中更新统底界埋深57.38m，第四系底界埋深86.05m，未钻穿新近系底界。图 5所示为SCG4测线地震反射波时间剖面和地质解释。时间剖面上反射信息丰富，反射波能量较强，解释了5个反射同相轴。反射同相轴T01在断层西侧近水平状沉积，而断层东侧受断层逆冲抬升影响，呈西高东低的倾斜状，近断层附近同相轴连续性较差，参考SCG2、SCG3剖面特征，解释的反射同相轴T01东西两段是否为同一地层界面反射尚无法确定，可采用钻孔取芯等方法进一步确认。在剖面桩号4370m附近，反射同相轴TQ及以下存在明显的扭曲或错断，应是断层在剖面上的反映，在桩号4365m处解释了1个视倾向东的逆断点，用Fp5标记，可分辨上断点埋深约125—130m，向上错断了第四系底界面TQ，未错断第四系内地层界面T01。
图 5 SCG4测线地震反射波时间剖面及地质解释Figure 5. Seismic reflection wave time-section and geological interpretation of line SCG42.5 上蔡岗断裂浅部构造特征
对比图 2—5可知，SCG1测线断点Fp1、SCG2测线断点Fp2、SCG3测线断点Fp4和SCG4测线断点Fp5均为视倾向东的逆断层，具有以下共同特征：逆断层下降盘地层均呈水平状分层展布，地表表现为平原；上升盘地层呈自西向东下倾的层状展布，地表表现为岗地；断点附近有明显的逆推变形特征。这4个断点属性相同、错断参数相近，所处的空间位置与推测的上蔡岗断裂位置基本一致，因此认为其为上蔡岗断裂在相应测线上的反映。
由图 3、4可知，上蔡岗断裂在SCG2、SCG3测线上逆断层断距较大，上升盘地层受力形变量大、埋深浅，形成了次级逆断点Fp2-1、Fp4-1和正断点Fp2-2、Fp3，尤其是SCG3剖面上逆断层上升盘反射同相轴连续性明显减弱、地层缺失较多。因此，上蔡岗断裂在此段形成了由断点Fp2-1和Fp4-1确定的次级断层，推测上蔡岗地区受力变形最大的位置应在SCG2、SCG3测线之间。
将解释断点展布在遥感解译图像上(见图 1)，可知上蔡岗断裂沿岗地西侧边缘延伸，为1条走向近北北西，倾向北东东的逆断层，其上断点错断了第四系底界面，并延伸至第四系内；由断点Fp2-1和Fp4-1确定的次级断层与主断层近平行，错断了所有可连续追踪的反射同相轴。
3. 结语
本研究采用高分辨率浅层地震勘探方法，获得了4条跨上蔡岗断裂的高分辨率浅层地震剖面，结合已有地质资料和钻孔数据，通过对高分辨率浅层地震剖面的分析和解释，对上蔡岗断裂位置、断层性质、空间展布特征等浅部特征有了较直观的认识。高分辨率浅层地震剖面验证了上蔡岗断裂的存在，该断裂为1条走向北北西、倾向北东东的逆断层，在测线SCG2、SCG3之间存在1条次级断层。浅层地震测线控制的上蔡岗断裂主断层延伸长度约24km，次级断层延伸长度约5km。高分辨率浅层地震剖面揭示的上蔡岗内部结构特征与岗地地表特征基本吻合：在岗地南北两端的SCG1、SCG4剖面上，第四系内部反射同相轴基本可连续追踪，新近系底界面垂直断距200—300m；而在岗地中间的SCG2、SCG3剖面上，逆断层上升盘明显抬升，反射同相轴连续性变差，新近系底界面垂直断距达500m。
从高分辨率浅层地震剖面上可以看出，逆断层断距深部较大、浅部较小。受地震纵波分辨率的限制，断层实际错断地层可能比地震剖面上判定的浅，为确定断层上断点具体埋深及断层最新活动时代，可采用钻孔联合地质剖面，通过测年等方法确定(刘保金等，2008；李倩等，2019)。
致谢: 本研究的野外数据采集由中国地震局地球物理勘探中心20余名专业技术人员共同完成，野外工作得到河南省地震局和驻马店市地震局的大力支持与配合，资料解释得到了驻马店市活断层项目组多位专家和评委的建议与指导，在此一并表示感谢！ -
图 2 破坏形态与${{{V_{\rm{p}}}} \mathord{\left/ {\vphantom {{{V_{\rm{p}}}} {({{{V_{\rm{n}}}} \mathord{\left/ {\vphantom {{{V_{\rm{n}}}} k}} \right. } k})}}} \right. } {({{{V_{\rm{n}}}} \mathord{\left/ {\vphantom {{{V_{\rm{n}}}} k}} \right. } k})}}$的关系
Figure 2. Relationship between failure form and $ {{{V_{\rm{p}}}} \mathord{\left/ {\vphantom {{{V_{\rm{p}}}} {({{{V_{\rm{n}}}} \mathord{\left/ {\vphantom {{{V_{\rm{n}}}} k}} \right. } k})}}} \right. } {({{{V_{\rm{n}}}} \mathord{\left/ {\vphantom {{{V_{\rm{n}}}} k}} \right. } k})}} $
图 3 破坏形态与弯剪比和剪跨比的关系
Figure 3. Relationship between Failure Mode and Bending-shear Ratio and Shear-to-span Ratio
表 1 ASCE/SEI 41-06标准中的柱破坏模式划分
Table 1. Column failure mode division in ASCE/SEI 41-06
折减后的弯剪比 柱中箍筋细部构造形式 135°弯钩的箍筋 90°弯钩的封闭环形箍 其他构造形式 ${V_\text{p}}/({V_\text{n}}/k) \leqslant 0.60$ 弯曲破坏 弯剪破坏 弯剪破坏 $0.60 < {V_\text{p}}/({V_\text{n}}/k) \leqslant 1$ 弯剪破坏 弯剪破坏 剪切破坏 ${V_\text{p}}/({V_\text{n}}/k) > 1$ 剪切破坏 剪切破坏 剪切破坏 
下载: 导出CSV
表 2 破坏形态与剪跨比和配箍特征值、体积配箍率、配筋率之间的关系
Table 2. Relationship between failure mode and shear span ratio and hoop characteristic value, volume hoop ratio and reinforcement ratio
破坏状态 剪跨比$\lambda $ 配箍特征值${\beta _{v}}$ 体积配箍率${\rho _{v}}$% 配筋率$\rho $% 弯曲破坏 $1.50 \leqslant \lambda \leqslant 5.50$ $0.02 \leqslant {\beta _{v}} \leqslant 0.45$ $0.30 \leqslant \rho _{v}^{} \leqslant 2.80$ $0.70 \leqslant \rho \leqslant 3.30$ 弯剪破坏 $1.50 \leqslant \lambda \leqslant 3.22$ $0.02 \leqslant {\beta _{v}} \leqslant 0.23$ $0.20 \leqslant \rho _{v}^{} \leqslant 1.60$ $1.30 \leqslant \rho \leqslant 3.80$ 剪切破坏 $1 \leqslant \lambda \leqslant 3.22$ $0.02 \leqslant {\beta _{v}} \leqslant 0.51$ $0.20 \leqslant \rho _{v}^{} \leqslant 1.80$ $1.30 \leqslant \rho \leqslant 4.10$
下载: 导出CSV
表 3 钢筋混凝土柱破坏形态判别标准
Table 3. Discrimination criteria for failure mode of reinforced concrete columns
破坏形态 判断标准 弯曲破坏 $2 < \lambda \leqslant 4$且${V_\text{p}}/({V_\text{n}}/k) \leqslant 0.7$ $\lambda > 4$且${V_\text{p}}/({V_\text{n}}/k) \leqslant 0.8$ 弯剪破坏 $\lambda \leqslant 2$且${V_\text{p}}/({V_\text{n}}/k) < 1.0$ $2 < \lambda \leqslant 4$且$0.7 < {V_\text{p}}/({V_\text{n}}/k) < 1.0$ 剪切破坏 $\lambda \leqslant 2$且${V_\text{p}}/({V_\text{n}}/k) \geqslant 1.0$ $2 < \lambda \leqslant 4$且${V_\text{p}}/({V_\text{n}}/k) \geqslant 1.0$
下载: 导出CSV
表 4 各影响因素与屈服位移角的相关系数
Table 4. Correlation coefficients between various influencing factors and yield displacement angle
影响因素 弯曲破坏柱 弯剪破坏柱 剪切破坏柱 Spearman
相关系数Sig.（双侧） Spearman
相关系数Sig.（双侧） Spearman
相关系数Sig.（双侧） 轴压比 -.822** .000 -.925** .000 -.621* .031 轴压比倒数 .822** .000 .925** .000 .621* .031 剪跨比 .063 .647 .042 .857 .120 .726 配箍特征值 .187 .094 -.279 .167 .320 .227 体积配箍率 -.017 .903 -.270 .034 .381 .247 纵筋配筋率 .360** .007 .251 .237 -.506 .112 注：**在0.01显著性水平（双侧）上显著相关；*在0.05显著性水平（双侧）上显著相关
下载: 导出CSV
表 5 各影响因素与极限位移角的相关系数
Table 5. Correlation coefficients between various influencing factors and ultimate displacement angle
影响因素 弯曲破坏柱 弯剪破坏柱 剪切破坏柱 Spearman
相关系数Sig.(双侧) Spearman
相关系数Sig.(双侧) Spearman
相关系数Sig.(双侧) 轴压比 -.773** .000 -.798** .000 -.922** .000 轴压比倒数 .773** .000 .798** .000 .922** .000 剪跨比 -.269* .037 .563** .010 .837** .000 配箍特征值 .458** .000 -.588** .006 .611 .108 体积配箍率 .348** .006 -.475* .034 .522 .184 纵筋配筋率 .509** .000 .181 .445 -.638 .015 注：**在0.01显著性水平（双侧）上显著相关；*在0.05显著性水平（双侧）上显著相关
下载: 导出CSV
表 6 不同破坏形态下位移角的回归方程
Table 6. Regression equations of displacement angles under different failure modes
破坏状态 回归方程 弯曲破坏 ${\theta _{y}} = 0.011 - 0.015n + 0.136\rho $ ${\theta _{u}} = 0.170 - 0.062n + 0.030{\beta _{v}} + 1.744\rho $ 弯剪破坏 ${\theta _{y}} = 0.011 - 0.015n + 0.155\rho $ ${\theta _{u}} = 0.029 - 0.097n + 0.029\lambda + 0.020{\beta _{v}}$ 剪切破坏 ${\theta _{y}} = 0.032 - 0.012n + 0.745\rho $ ${\theta _{u}} = 0.005 - 0.030n + 0.012\lambda - 0.007\rho $
下载: 导出CSV
表 7 不同破坏形态下位移角回归方程中的R，R2和Adjusted R2
Table 7. Correlation Coefficients R, R2 and Adjusted R2 in the Displacement Angle Regression Equation under Different Failure Modes
屈服位移角 极限位移角 弯曲破坏 弯剪破坏 剪切破坏 弯曲破坏 弯剪破坏 剪切破坏 R 0.872 0.876 0.885 0.892 0.894 0.994 R2 0.761 0.768 0.784 0.795 0.799 0.989 Adjusted R2 0.752 0.757 0.779 0.782 0.785 0.981
下载: 导出CSV
-
董正方, 王君杰, 韩鹏, 2010.工程结构柱式构件的抗震性能试验研究进展.震灾防御技术, 5(1):43-52. doi: 10.3969/j.issn.1673-5722.2010.01.006 蒋友宝, 周浩, 曹青等, 2017.不同设计配筋下大偏压RC柱承载力抗震可靠度.土木建筑与环境工程, 39(6):68-77. http://d.old.wanfangdata.com.cn/Periodical/cqjzdxxb201706009 刘良林, 王全凤, 应建中, 2016.地震作用下高强混凝土柱延性计算方法研究.世界地震工程, 32(1):261-265. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=sjdzgc201601037 李强, 金贤玉, 2015.箍筋锈蚀对轴压混凝土短柱承载力的影响.浙江大学学报(工学版), 49(10):1929-1938, 1951. http://d.old.wanfangdata.com.cn/Periodical/zjdxxb-gx201510015 戚永乐, 2012.基于材料应变的RC梁、柱及剪力墙构件抗震性能指标限值研究.广州: 华南理工大学. 苏佶智, 邢国华, 马煜东等, 2018.反复荷载作用下锈蚀钢筋混凝土柱力学性能研究.震灾防御技术, 13(03):512-523. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20180303&journal_id=zzfyjs 万海涛, 韩小雷, 季静, 2010.基于性能设计方法的钢筋混凝土柱构件分析.中南大学学报(自然科学版), 41(4):1584-1589. http://d.old.wanfangdata.com.cn/Periodical/zngydxxb201004059 杨君, 2007.钢筋混凝土框架结构基于位移的抗震设计理论和方法.西安: 西安建筑科技大学. ASCE/SEI 41-06 Seismic Rehabilitation of Existing Buildings. Reston, Virginia: American Society of Civil Engineers, 2007. Ahani E, Mousavi M N, Ahani A, et al, 2019. The effects of amount and location of openings on lateral behavior of masonry infilled RC frames. KSCE Journal of Civil Engineering: 1-13. Berry M, Parrish M, Eberhard M. PEER Structural performance database user's manual (version 1.0). University of California: Berkeley, 2004. Elwood K J, Moehle J P, 2006. Idealized backbone model for existing reinforced concrete columns and comparisons with FEMA 356 criteria. Structural Design of Tall and Special Buildings, 15(5):17. https://www.researchgate.net/publication/229471296_Idealized_backbone_model_for_existing_reinforced_concrete_columns_and_comparisons_with_FEMA_356_criteria Lee K, Yoo J K, 2014. Canonical correlation analysis through linear modeling. Australian & New Zealand Journal of Statistics, 56(1):59-72. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0232630464/ Maekawa K, An X, 2000. Shear failure and ductility of RC columns after yielding of main reinforcement. Engineering Fracture Mechanics, 65(2):335-368. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=a8adb1be393292771fb8687d3a0ab8eb Sezen H, Moehle J. P, 2004. Shear strength model for lightly reinforced concrete columns. Journal of Structural Engineering, 130(11):1692-1703. doi: 10.1061/(ASCE)0733-9445(2004)130:11(1692) -
下载:
点击查看大图
计量
- 文章访问数: 221
- HTML全文浏览量: 110
- PDF下载量: 20
- 被引次数: 0
