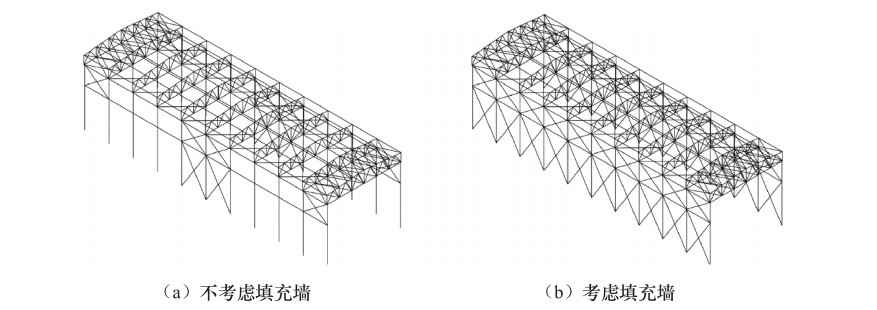
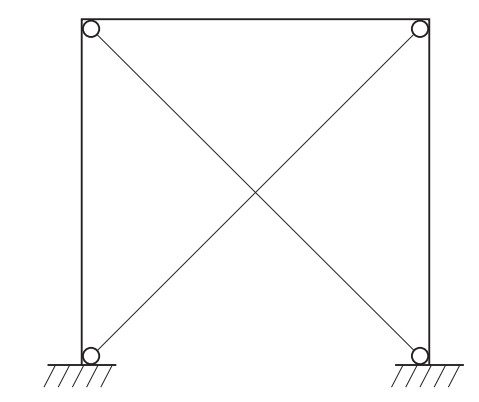
Seismic Fragility Analysis of Reinforced Concrete Plant Structure Considering the Influence of Infilled Walls
-
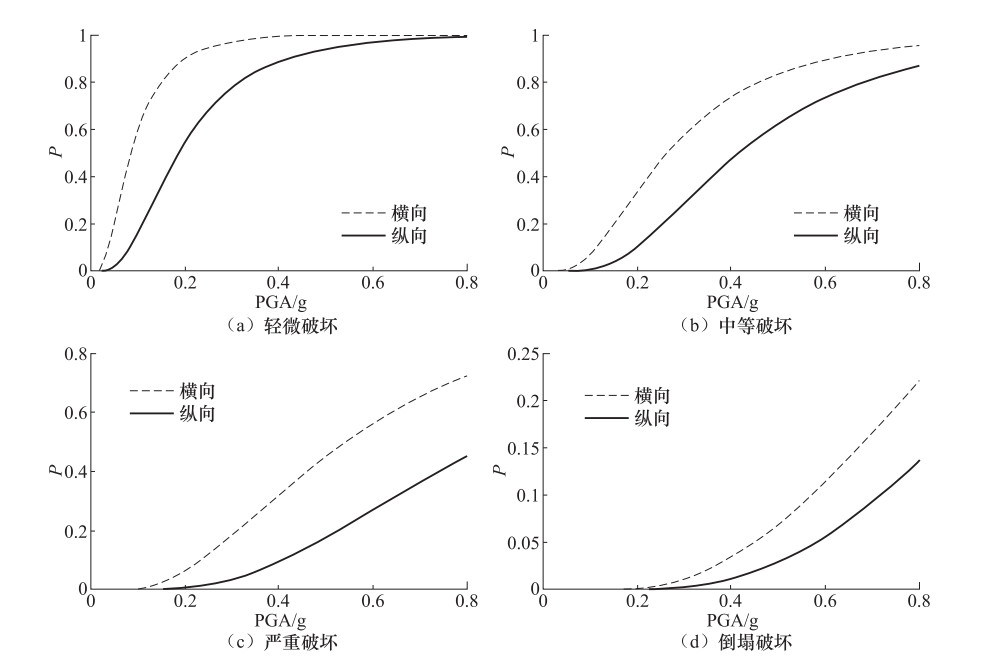
摘要: 采用对角斜撑模拟纵向填充墙的作用，建立考虑填充墙和不考虑填充墙厂房结构模型，采用拉丁超立方抽样技术建立考虑材料不确定性的结构分析样本，基于随机Pushover分析确定结构不同破坏状态下的统计参数。综合考虑结构材料强度及输入地震动不确定性的影响，通过非线性时程分析开展单层钢筋混凝土厂房结构易损性研究，在此基础上比较结构横、纵向易损性的差异，研究填充墙对结构易损性的影响。研究结果表明：钢筋混凝土厂房结构体系横向地震易损性显著大于纵向地震易损性；对纵向结构体系而言，加入填充墙会明显降低结构易损性，但在相同强度的地震动作用下，填充墙破坏程度比主体结构严重，这与厂房结构的实际震害特征相符。
-
关键词:
- 钢筋混凝土工业厂房 /
- 地震易损性 /
- 拉丁超立方抽样 /
- 随机Pushover分析填充墙 /
Abstract: The diagonal strut was used to simulate the action of the longitudinal in-filled walls, and the plant structure model with and without in-filled walls was established. The Latin Hypercube Sampling was used to establish structural analysis samples considering material uncertainties, and based on random Pushover analysis, the statistical parameters of different failure states of structures were determined. Considering the influence of the uncertainties of structural material strength and input ground motion, the fragility of single-story reinforced concrete plant structure was studied using nonlinear dynamic time history analysis. On this basis, the difference between transverse and longitudinal fragility of structure was compared and the influence of in-filled walls on the fragility of structure was studied. The results show that the transverse seismic fragility of reinforced concrete plant structure is significantly greater than the longitudinal seismic fragility, and the in-filled walls will obviously reduce the longitudinal seismic fragility of the structure. However, under the same seismic intensity, the damage of the in-filled walls is more serious than that of the main structure, which is consistent with the actual seismic damage characteristics of the plant structures. -
引言
地震小区划是对特定区域范围内可能遭遇到的地震影响进行划分，包括设计地震动参数小区划和地震地质灾害小区划。相比地震区划，地震小区划工作更重视局部场地条件的影响，更为详细地考虑周围的地震地质环境，其成果可为抗震设计、土地利用规划、震害防御等提供更精确的资料(胡聿贤，1999)。
地震小区划工作内容涉及地震活动性评价、地震构造评价、场地地震动分析等，工作量大，获取的数据也很丰富。传统地震小区划成果通常以文字报告、专题图件的形式提交，类型较单一，展示度较差，难以满足目前公共服务的需要。管理现有数据并充分利用数据推出更多服务型产品是目前包括地震小区划工作在内的地震工作信息化和现代化迫切需要。
随着GIS技术的发展，国内很多研究者逐步将GIS技术引入地震小区划研究工作中(张苏平等，2003；王庆满等，2011；李程程等，2014)，建立有关数据库和数据管理系统(田勤虎等，2011；龚磊等，2015)。但已建系统多以数据查询、展示为主，缺少分析功能和实际产出服务。本文以嘉兴科技城地震小区划工作为基础，将基础数据与地理信息系统(GIS)相结合，形成集查询、分析于一体的地震小区划成果数字化系统，可为不同用户提供不同数据服务。
1. 研究区概况
研究区位于嘉兴科技城，行政区划属于嘉兴市南湖区，面积约30km2，是浙江省四大科技平台之一。现聚集了包括浙江清华长三角研究院、浙江中科院应用技术研究院在内的一批高端院所，还建有多个国际技术合作平台、工程中心和产学研基地，是嘉兴市乃至浙江省重要的创业创新平台。
查询《中国地震动参数区划图》(GB 18306—2015)(中华人民共和国国家质量监督检验检疫总局等，2015)可知，嘉兴市大部分地区由原来的Ⅵ度设防提升为Ⅶ度设防，设防水准的提高将对区内建设工程抗震设防、国土利用规划、社会经济发展等提出更高的要求。嘉兴科技城现处于Ⅶ度设防区内，加之人才产业聚集、产值高，地震灾害风险大。在该区进行地震小区划工作，可为该区提供更精确的抗震设防和土地规划资料，有效降低地震灾害风险。
2. 基础数据
根据相关规范要求，地震小区划工作内容包括地震地质及地震活动性资料的收集、场地钻探、场地土层反应分析等，涉及地质、地震、地球物理、工程勘察等多方面的数据，根据系统建设的需要归纳如下：
(1) 地震地质及地震活动性数据
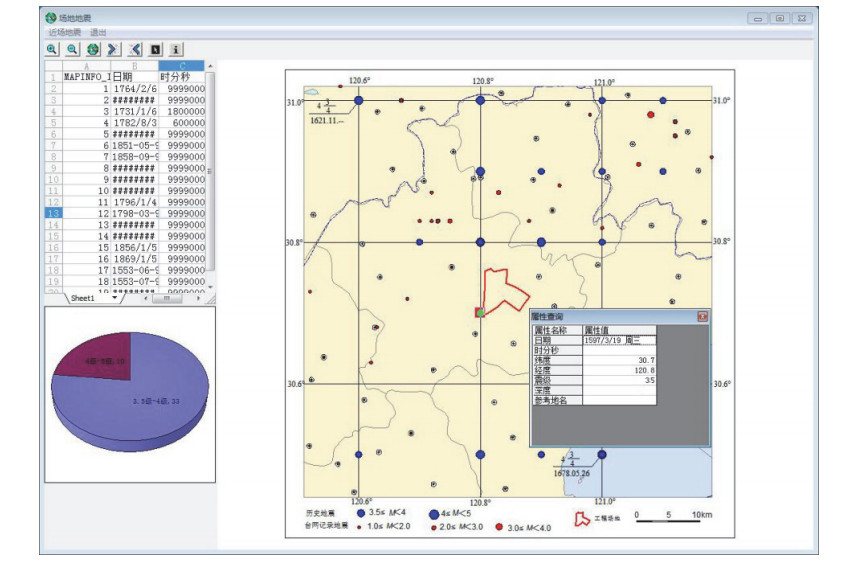
研究区内地震地质及地震活动性专题图包括区域地震分布图、场地地震分布图、历史地震等震线分布图、区域构造图、区域断层分布图、近场构造图等，均为Mapinfo格式的矢量文件。
(2) 场地地震工程地质条件勘察数据
区划范围内布设60个钻孔，其中标准钻孔1个(孔深232.8m)，地震钻孔29个(深度不小于105m)，勘察孔30个(深度小于30m)，钻探总进尺4204m，浅层人工地震探测测线共21534m。系统建设收集的数据包括60幅钻孔柱状图、18条工程地质剖面、5幅浅层人工地震探测解译图，其中钻孔资料均以柱状图的形式提供，格式为AUTO CAD矢量文件，钻孔的其他信息以Excel表格的形式提供；工程地质剖面图和地震探测测线数据为PDF文档和栅格图片；勘察报告以PDF文档的形式提供。
场地内60个钻孔均进行了波速测试，钻孔代表性土层动三轴土样80件，收集的波速数据和动三轴数据以Excel表格的形式提供。
(3) 地震动分析与参数区划数据
根据土层地震动分析的需要，建立29个场地钻孔模型，数据格式为TXT文本。收集全球范围内天然地震记录1692条，人工合成地震波522条(每个钻孔3个超越概率各6条)，以TXT文本的形式提供。
地震小区划成果图件主要为地震动参数区化图，以Mapinfo矢量文件的形式提供。另外，还包括小区划报告的文本，以PDF文档的形式提供。
3. 系统设计
3.1 面向对象实现
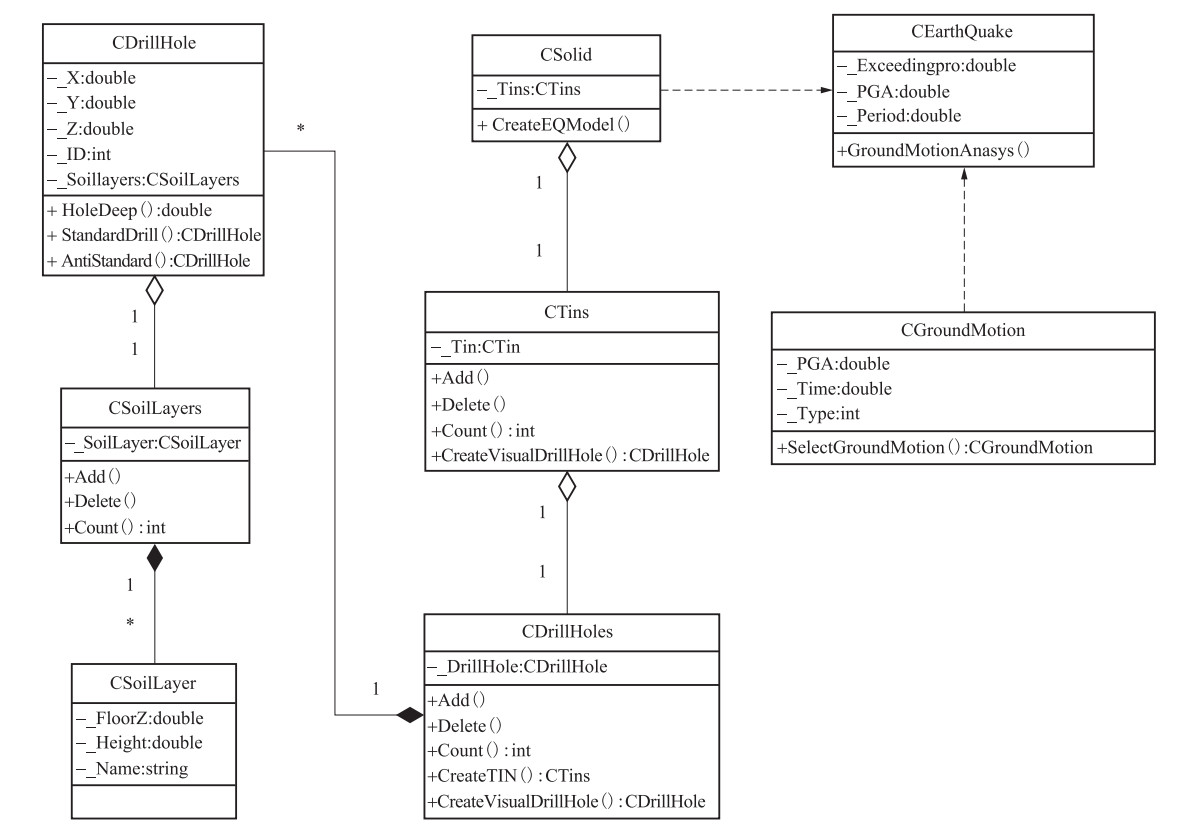
地震小区划涉及钻孔、场地、强震记录、地震灾害等概念，其对象层次清晰，易于以面向对象的方式实现，形成地震小区划数字化系统。根据需求分析，首先将地震小区划数字化涉及的问题抽象成类，并建立类之间的消息机制及类之间的关系，即进行地震小区划数字化概念设计。
根据概念模型抽象出类，相应的类关系如图 1所示，地震小区划数字化主要类有CSolid(工程地质三维实体类)、CDrillHoles(钻孔集合类)、CSoilLayers(土层集合类)、CGroundMotion(强震动观测记录类)、CEarthQuake(场地地震动类)等。CDrillHoles类由CDrillHole类组合形成，并通过CreateTIN方法实现CTins类，由CTins类实现CSolid类，利用CGroundMotion类中SelectEarthQuake方法筛选出符合地质条件的历史强震记录，导入CSolid类，进行地震动分析，计算场地地震动参数峰值加速度、特征周期，并绘制峰值加速度等值线图和特征周期等值线图，由此生成场地地震小区划成果。
3.2 数据库设计
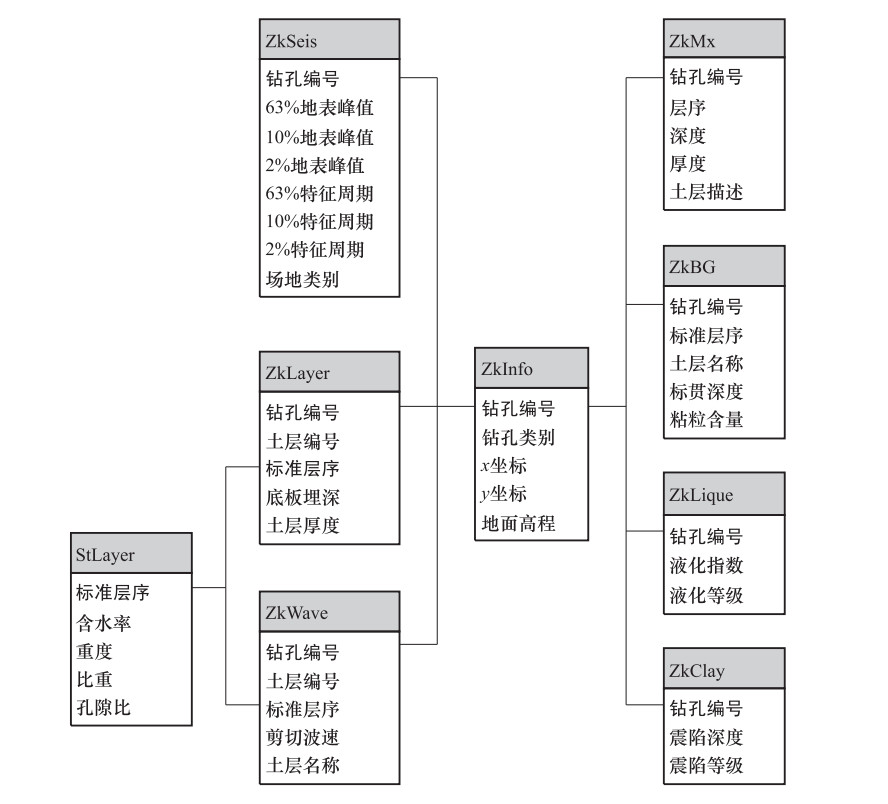
系统采用Access小型数据库实现场地基础地理信息数据、地震动数据、地震震陷数据、地震液化数据等的建库，结合GIS技术实现空间数据与属性数据的整合、矢量数据与栅格数据的整合、信息数据与分析数据的整合。数据库共包含9张数据表，分类如表 1所示。
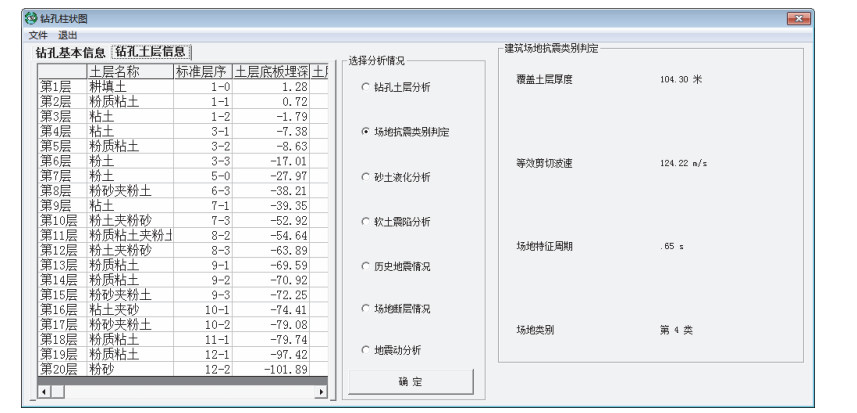
表 1 数据库汇总Table 1. Database summary序号 表名 功能说明 1 ZkInfo 钻孔基本信息 2 ZkLayer 钻孔土层信息 3 StLayer 标准层序土层信息 4 ZkLique 钻孔砂土液化信息 5 ZkClay 钻孔软土震陷信息 6 ZkWave 钻孔土层剪切波速信息 7 ZkBG 钻孔土层标贯信息 8 ZkMx 钻孔动力分析模型信息 9 ZkSeis 钻孔动参数区划信息表 数据库逻辑设计如图 2所示。
3.3 系统物理设计
系统采用MapX作为GIS开发组件，数据库采用Access，在VB环境下完成开发，可运行于多个版本的windows操作系统中。
4. 系统功能实现
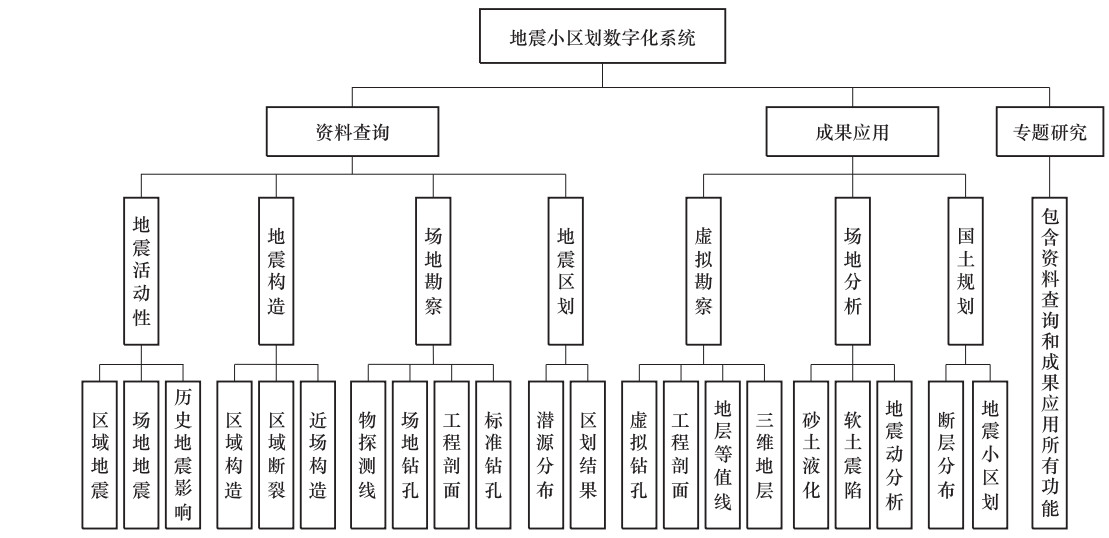
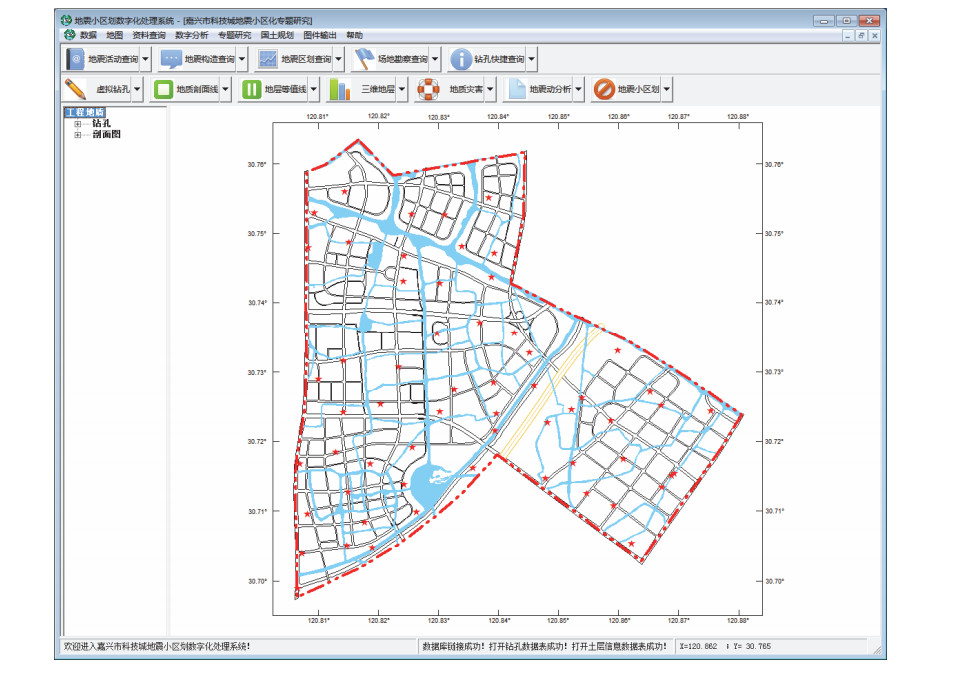
系统功能分为资料查询、成果应用、专题研究三大模块，其中专题研究整合了资料查询、成果应用模块的所有功能，详细的功能设计如图 3所示，系统主界面(专题研究模块)如图 4所示，主要对资料查询、成果应用模块进行介绍。
4.1 资料查询模块
该模块主要对地震小区划工作的基础资料及成果数据进行可视化展示，内容包括地震活动性、地震构造、场地勘察、地震区划等。由于部分功能展示的形式类似，对其中的部分功能进行介绍。
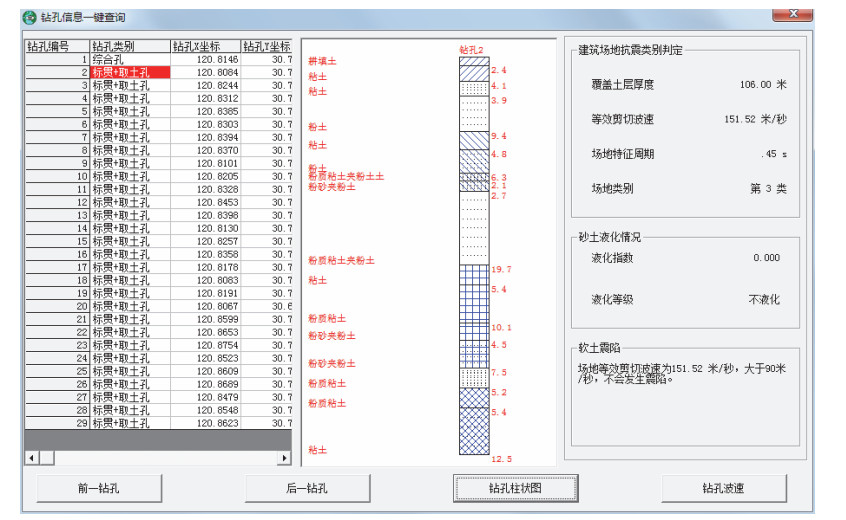
(1) 地震活动性查询：可查询区域地震、场地地震、历史地震影响烈度及场地地震综合评价。按地震震级自动统计地震活动性情况，通过地点地图及数据表的方式查询当前地震发生日期、经度、纬度、震级、震源深度等相关信息，成果展示如图 5所示。
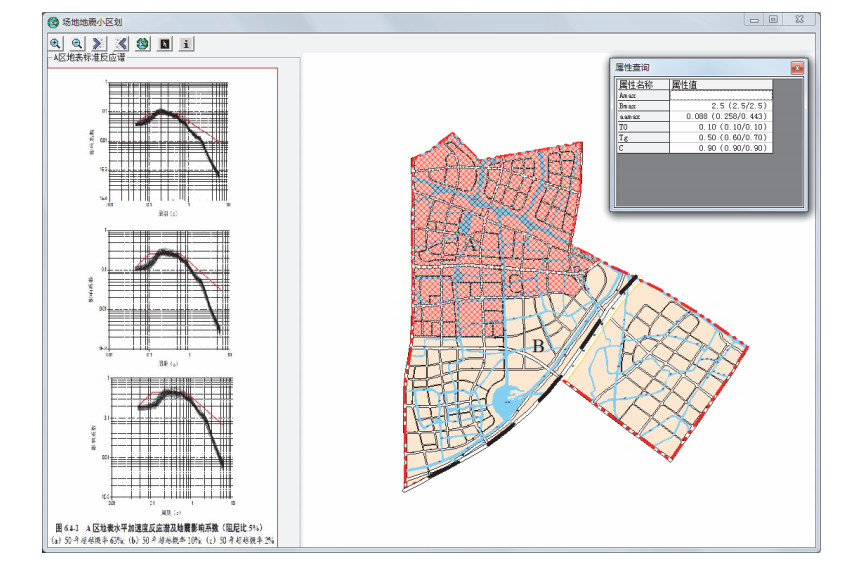
(2) 地震区划：对区内潜在震源区的划分情况及地震小区划成果进行查询，成果以可视化的形式展示，包括区划的范围、相关的地震动参数及标定的反应谱，成果展示如图 6所示。
(3) 钻孔快捷查询：可对区内所有勘察钻孔进行集中展示，包括钻孔柱状图、钻孔土层剪切波速、抗震类别判定、砂土液化和软土震陷情况判断等所有信息，成果展示如图 7所示。
4.2 成果应用
该模块基于现有基础资料，通过建立分析模型对数据进行数字化分析，生成相应的成果数据。该模块主要包括虚拟勘察、场地分析及国土规划3个子模块，其中虚拟勘察模块包括虚拟钻孔、工程地质剖面、地层等值线等，场地分析模块包括砂土液化、软土震陷分析及场地抗震类别判定，国土规划模块包括断层分布及地震小区划。
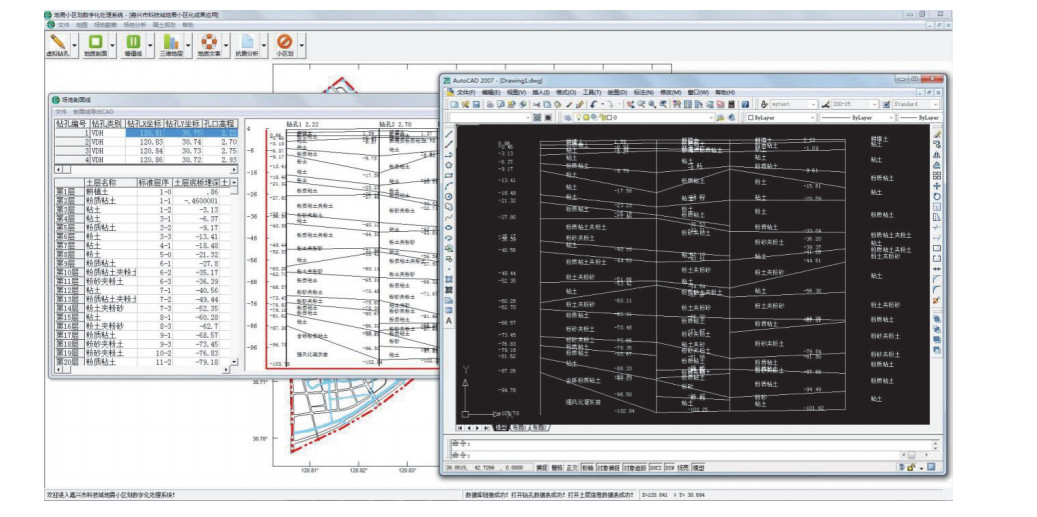
(1) 虚拟钻孔：采用delaunay三角网上的线性内插算法，根据坐标输入或地图点选获得示范区内任意位置钻孔(虚拟)的相关资料，包括钻孔柱状图、场地抗震类别判定、砂土液化和软土震陷分析、历史地震、地震动参数等。虚拟钻孔的柱状图以栅格图片或CAD矢量文件的形式导出，所有虚拟钻孔信息还可以Word文档的形式导出，内容包括场地土层信息、场地地震动参数、场地类别等，涵盖了地震工程大部分基础资料，可供相关单位参考使用，如图 8所示。
(2) 工程地质剖面：根据连续的坐标输入或在底图上绘制剖面线生成研究区内相应的工程地质剖面图(见图 9)，可将剖面图以栅格图片或CAD矢量文件的形式导出。
(3) 地震地质灾害：包括场地内的砂土液化和软土震陷。根据部分钻孔试验参数，采用建规中的判别方法对场地内钻孔进行判定，给出其液化程度和震陷情况。
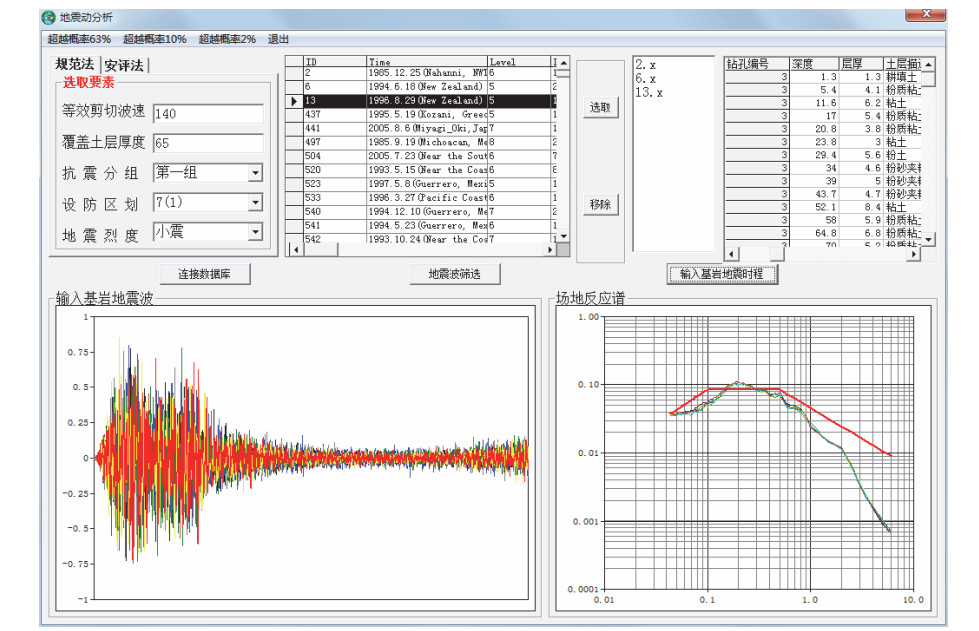
(4) 地震动分析：根据点选的坐标位置，选择距离最近的地震钻孔数据，输入筛选要素，从天然地震时程库中选取符合要求的天然地震时程；根据人工合成的基岩地震动计算得到各超越概率下的场地地表地震动参数，成果展示如图 10所示。
5. 结论与建议
通过对地震小区划数据的整理，基于GIS软件开发地震小区划成果数字化系统。在实现项目成果数据查询与可视化展示的同时，还可导出数字化分析成果。相比传统的地震小区划工作，该系统的成果更丰富，形式更多样，为地震工作服务能力的提升进行了尝试。同时，需指出的是，此次建立的系统仅为单机版，与实现公共服务仍存在一定差距，后续还需进行更多的研究，补充和完善相关功能，以期建立高效便捷的在线服务系统。
-
图 1 结构立面图及柱配筋（单位：mm）
Figure 1. Elevation of structure and reinforcement of columns(unit: mm)
图 2 结构三维有限元分析模型
Figure 2. Three-dimensional finite element analysis model of structure
图 6 结构概率地震需求模型（横向）
Figure 6. Probabilistic seismic demand model of the structure(the transverse orientation)
图 7 结构概率地震需求模型（纵向）
Figure 7. Probabilistic seismic demand model of the structure(the longitudinal orientation)
图 8 考虑填充墙的结构概率地震需求模型（纵向）
Figure 8. Probabilistic seismic demand model with considering the infilled walls(the longitudinal orientation)
图 9 基于PGA的厂房结构地震易损性曲线
Figure 9. Seismic fragility curves of plant structure based on PGA
图 10 基于PGA的厂房结构地震易损性曲线（纵向）
Figure 10. Seismic fragility curves of plant structure based on PGA(the longitudinal orientation)
图 11 填充墙与主体结构地震易损性曲线（纵向）
Figure 11. Seismic fragility curves of the infilled walls and the structure(the longitudinal orientation)
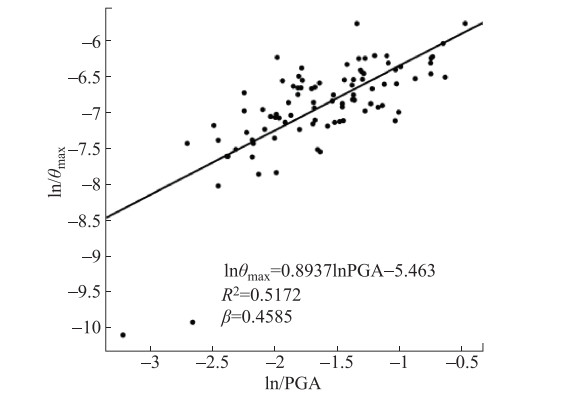
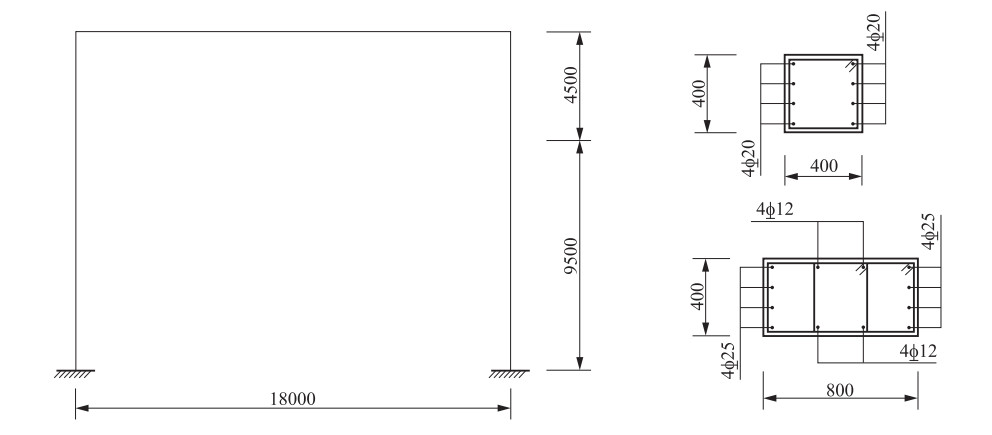
表 1 结构不确定性参数
Table 1. Considered structural random parameters
不确定性因素 随机变量 均值 变异系数 分布类型 C30混凝土 抗压强度${f_\text{c}}$ $26.1\text{MPa}$ 0.14 对数正态 弹性模量${E_\text{c}}$ $3 \times {10^4}\text{MPa}$ 0.06 对数正态 容重${\gamma _\text{c}}$ 26.5kN·m-3 0.07 正态 HRB335钢筋 抗拉强度${f_y}$ $378\text{MPa}$ 0.07 对数正态 弹性模量${E_s}$ $2.0 \times {10^5}\text{MPa}$ 0.02 对数正态 
下载: 导出CSV
表 2 结构横向各破坏状态变形指标统计参数
Table 2. Statistical parameters of deformation indexes for each damage state of the structure(The transverse orientation)
破坏状态 轻微破坏 中等破坏 严重破坏 倒塌破坏 ${m_\text{C}}$ 0.004638 0.009784 0.01545 0.02776 ${\beta _\text{C}}$ 0.1759 0.1829 0.1819 0.1147
下载: 导出CSV
表 3 结构纵向各破坏状态变形指标统计参数
Table 3. Statistical parameters of deformation indexes for each damage state of the structure(The longitudinal orientation)
破坏状态 轻微破坏 中等破坏 严重破坏 倒塌破坏 ${m_\text{C}}$ 0.002067 0.004618 0.009461 0.01687 ${\beta _\text{C}}$ 0.2546 0.0813 0.0664 0.1468
下载: 导出CSV
表 4 考虑填充墙时结构纵向各破坏状态变形指标统计参数
Table 4. Statistical parameters of deformation indexes for each damage state of the structure with considering the infilled walls(The longitudinal orientation)
破坏状态 轻微破坏 中等破坏 严重破坏 倒塌破坏 ${m_\text{C}}$ 0.001202 0.002596 0.005088 0.006547 ${\beta _\text{C}}$ 0.3318 0.2479 0.0945 0.1072
下载: 导出CSV
表 5 砌体填充墙性能水准划分
Table 5. Performance level division of the infilled walls
破坏状态 轻微破坏 中等破坏 严重破坏 倒塌破坏 ${\mu _\text{C}}$ 0.001 0.0018 0.005 0.006 ${\delta _\text{C}}$ 0.35 0.35 0.35 0.35
下载: 导出CSV
表 6 考虑填充墙和不考虑填充墙时易损性曲线校正系数
Table 6. The correction coefficient of fragility curve when considering the infilled walls and not considering the infilled walls
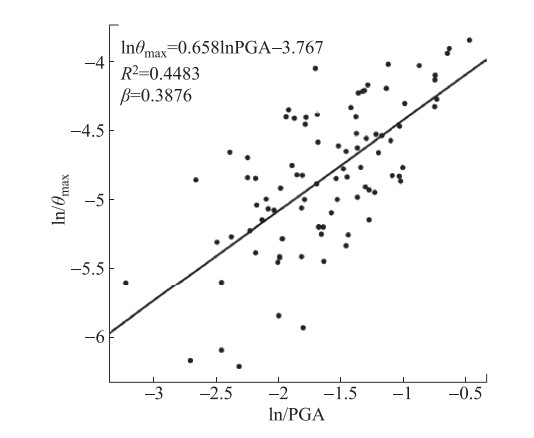
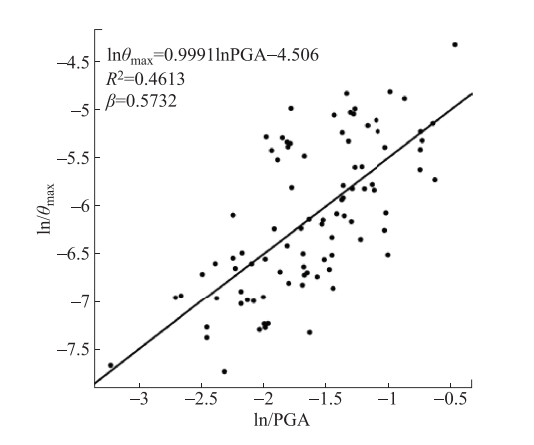
破坏状态 轻微破坏 中等破坏 严重破坏 倒塌破坏 不考虑填充墙 0.187g 0.418g 0.857g 1.528g 考虑填充墙 0.244g 0.577g 1.266g 1.626g 校正系数 1.30 1.38 1.43 1.06
下载: 导出CSV
-
刘大海, 杨翠如, 钟锡根, 1989.单层厂房抗震整体分析.世界地震工程, (2):7-14. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000004586837 刘桂秋, 2005.砌体结构基本受力性能的研究.长沙: 湖南大学. 刘阳冰, 2009.钢-混凝土组合结构体系抗震性能研究与地震易损性分析.北京: 清华大学. 裘民川, 刘大海, 1989.单层厂房抗震设计.北京:地震出版社. 童岳生, 钱国芳, 1985.砖填充墙钢筋混凝土框架的变形性能及承载能力.西安建筑科技大学学报(自然科学版), (2):1-21. http://www.cnki.com.cn/Article/CJFD1985-XAJZ198502000.htm 徐超, 温增平, 2017.不同设防标准RC框架结构基于易损性分析的抗震性能评估.震灾防御技术, 12(4):845-857. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20170413&journal_id=zzfyjs 阎红霞, 2012.楼板和填充墙对RC框架结构抗震性能的影响.北京: 北京交通大学. 于晓辉, 2012.钢筋混凝土框架结构的概率地震易损性与风险分析.哈尔滨: 哈尔滨工业大学. 张号浩, 2011.单层钢筋混凝土柱厂房地震易损性分析.哈尔滨: 中国地震局工程力学研究所. 张明远, 2017.基于构件性能的填充墙框架结构地震易损性研究.哈尔滨: 中国地震局工程力学研究所. 赵文哲, 温增平, 徐超等, 2018.楼层侧向刚度比对砌体结构地震易损性的影响分析.震灾防御技术, 13(4):878-892. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20180415&journal_id=zzfyjs 朱彦鹏, 2014.钢筋混凝土结构课程设计指南.北京:中国建筑工业出版社. 朱健, 谭平, 周福霖, 2010.基于位移的钢筋混凝土结构单层厂房易损性分析[J].振动与冲击, 29(1):207-213, 247. doi: 10.3969/j.issn.1000-3835.2010.01.045 庄一舟, 谢狄敏, 傅军, 1999.砌体结构抗震可靠度分析方法.工程力学, (a03):920-925. http://d.old.wanfangdata.com.cn/Conference/75229 Babič A., Dolšek M., 2016. Seismic fragility functions of industrial precast building classes. Engineering Structures, 118:357-370. doi: 10.1016/j.engstruct.2016.03.069 Beilic D., Casotto C., Nascimbene R., et al., 2017. Seismic fragility curves of single storey RC precast structures by comparing different Italian codes. Earthquakes and Structures, 12(3):359-374. doi: 10.12989/eas.2017.12.3.359 Bolognini D., Borzi B., Pinho R., 2008. Simplified pushover-based vulnerability analysis of traditional Italian RC precast structures.14th Wcee. Casotto C., Silva V., Crowley H., et al., 2015. Seismic fragility of Italian RC precast industrial structures. Engineering Structures, 94:122-136. doi: 10.1016/j.engstruct.2015.02.034 Cornell C. A., Jalayer F., Hamburger R. O., et al., 2002. Probabilistic basis for 2000 SAC Federal Emergency Management Agency steel moment frame guidelines. Journal of Structural Engineering, 128(4):526-533. doi: 10.1061/(ASCE)0733-9445(2002)128:4(526) Mainstone R. J., Weeks G. A., 1970. The influence of bounding frame on the racking stiffness and strength of brick walls. England: Proc., 2nd Int. Brick Masonry Conf. Palanci M., Senel S. M., Kalkan A., 2016. Assessment of one story existing precast industrial buildings in Turkey based on fragility curves. Bulletin of Earthquake Engineering, 15(1):1-19. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=f3c7b7f1209c63132dda8e004d2e8dcb Polyakov S. V., 1960. On the interaction between masonry filler walls and enclosing frame when loading in the plane of the wall. Translation in Earthquake Engineering, 36-42. Senel S. M., Kayhan A. H., 2010. Fragility based damage assessment in existing precast industrial buildings:A case study for Turkey. Structural Engineering & Mechanics, 34(1):39-60. Shome N., 1999. Probabilistic seismic demand analysis of nonlinear structures. Stanford: Stanford University. Smith B. S.1967. Methods for predicting the lateral stiffness and strength of multi-storey infilled frames. Building Science, 2(3):247-257. doi: 10.1016/0007-3628(67)90027-8 -
下载:
点击查看大图
计量
- 文章访问数: 141
- HTML全文浏览量: 67
- PDF下载量: 4
- 被引次数: 0
