Seismic Energy Response Analysis of Simple Supported Beam Bridge
-
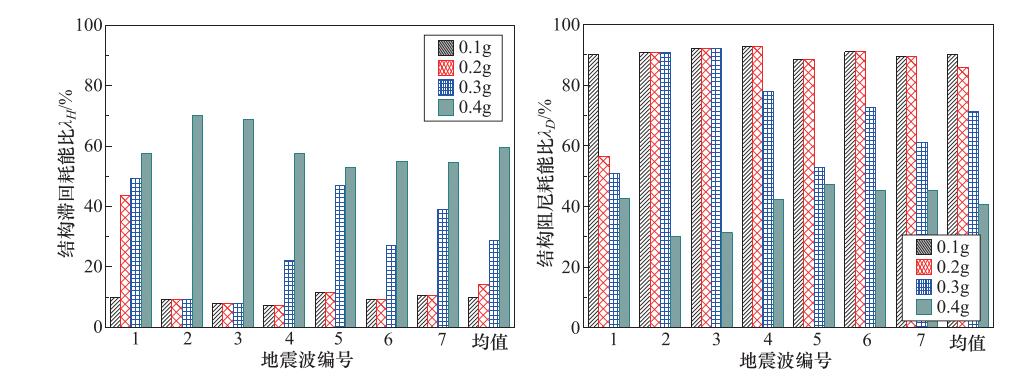
摘要: 利用有限元软件SAP2000建立了某公路简支梁桥的有限元模型,以7条典型强震记录为输入,研究了公路简支梁桥的地震能量响应及其分配规律。结果表明:①地基柔性效应对公路简支梁桥的地震能量响应及其分配规律的影响较小;②当场地土质变软时,地震总输入能、结构阻尼耗能和结构阻尼耗能比均呈递增趋势,而结构滞回耗能和结构滞回耗能比则不断减小,即地基土体作为桥梁动力系统的一部分,增大了系统阻尼,并分担了部分非弹性变形;③随着PGA增大,输入结构的地震能量也增加,导致塑性铰的非弹性变形增加,即结构滞回耗能和结构阻尼耗能增大。Abstract: SAP2000 is used to build the finite-element analysis (FEA) model of one simple supported beam bridge. By inputting seven typical ground motions, the seismic energy response and its distribution are analyzed based on seismic energy response equation. The results are obtained as followings:①Effect of flexible foundation on seismic energy response and its distribution of simple supported beam bridge is less. ②As site become soften, the input seismic energy, hysteretic energy dissipation and hysteretic energy dissipation ratio increases, but damping energy dissipation and damping energy dissipation ratio decreases. It is because that damping is increased along with the foundation soil which can share part of inelastic deformation. ③With increasing of PGA, input seismic energy increases. As the result, the inelastic deformation of plastic hinge increases. It suggests that the hysteretic energy dissipation and damping energy dissipation increases too.
-
引言
2017年5月11日,新疆维吾尔自治区喀什地区塔什库尔干县发生MS 5.5地震,地震造成8人死亡、31人受伤,造成房屋及设施破坏,直接经济损失共20.05亿元(侯建盛等,2017)。
此次地震灾区主要涉及喀什地区塔什库尔干县科克亚尔柯尔克孜民族乡、塔合曼乡、提孜那普乡、塔什库尔干乡、班迪尔乡、巴扎达什牧林场(行政隶属班迪尔乡)、瓦恰乡、达布达尔乡等9个乡镇。灾区面积3288km2,受灾人口26486人,9285户,由于房屋毁坏和较大程度破坏造成失去住所人数共计16194人,4753户。
1. 地震构造背景及基本情况
1.1 地震烈度
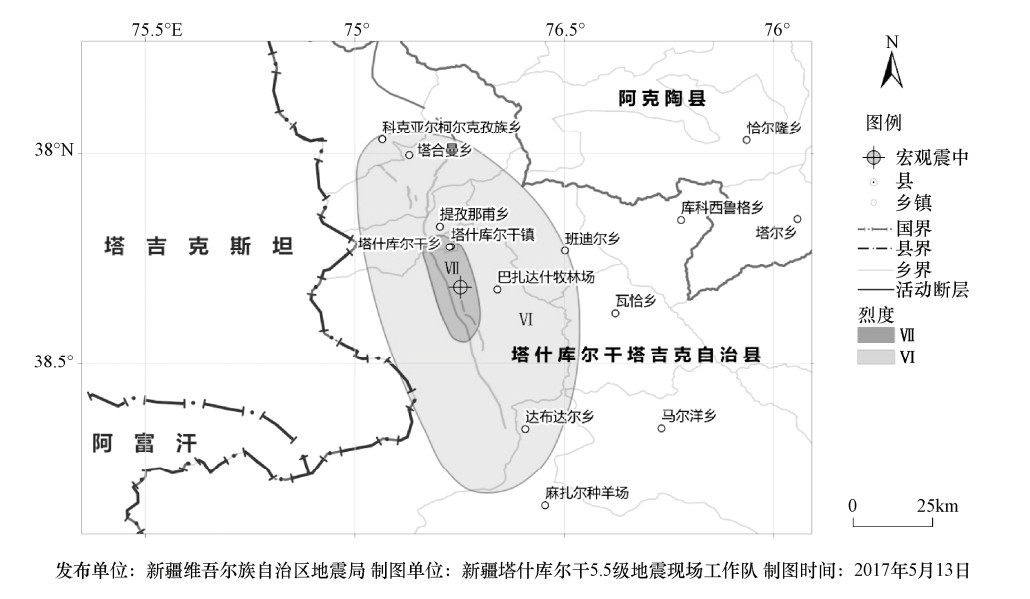
此次地震震中位于新疆喀什地区塔什库尔干县塔什库尔干乡,宏观震中位于塔什库尔干乡库孜滚村,为Ⅷ度异常点。通过对灾区9个乡(镇、场)的69个调查点展开实地调查,得到的烈度图等震线长轴呈北北西走向分布(图 1)。Ⅶ度区面积227km2,长轴28km,短轴8km,涉及塔什库尔干镇(含县城)和塔什库尔干乡;Ⅵ度区面积3061km2,长轴100km,短轴43km,涉及科克亚尔柯尔克孜民族乡、塔合曼乡、提孜那普乡、塔什库尔干乡、班迪尔乡、巴扎达什牧林场(行政隶属班迪尔乡)、瓦恰乡、达布达尔乡等8个乡(场);Ⅵ度区及以上总面积为3288km2。
1 新疆地震局,2017.新疆塔什库尔干5.5级地震灾害损失评估报告.
1.2 发震构造
震区位于帕米尔高原塔什库尔干断陷谷地,该谷地是由青藏高原西北帕米尔构造结内部塔什库尔干拉张系晚新生代以来的拉张作用形成的盆地,其南北狭长,东西分布海拔为4000—5000m的高山。
震区内塔什库尔干断裂成型于华力西时期,有长期的演化发育史。大部分在喜马拉雅期重新复活,该断裂控制着塔什库尔干盆地的形成与演化,此次塔什库尔干MS 5.5地震就发生在塔什库尔干断裂带上(图 2)。
1 新疆地震局,2017.新疆塔什库尔干5.5级地震灾害损失评估报告.
从本次地震的新构造运动分区背景来看,新近纪以来震区所处的西昆仑隆起区隆起幅度大致在2—7km,该隆起区第四纪以来的隆起幅度和速率分别为1200—1700m和10—13mm/a。
1.3 震区场地条件
震区位于帕米尔东北—西昆仑区段,西昆仑山体呈北西—南东走向,平均海拔5000—6000m,主要山峰偏于西部。主峰公格尔山海拔7649m,慕士塔格山为7509m,山体宽厚高大,南北不对称,北坡长而陡峭,与海拔1000m多的塔里木盆地相邻,高差4000m。帕米尔高原实际上并非平坦的高原面,由几组山脉和山脉之间宽阔的谷地和盆地构成。
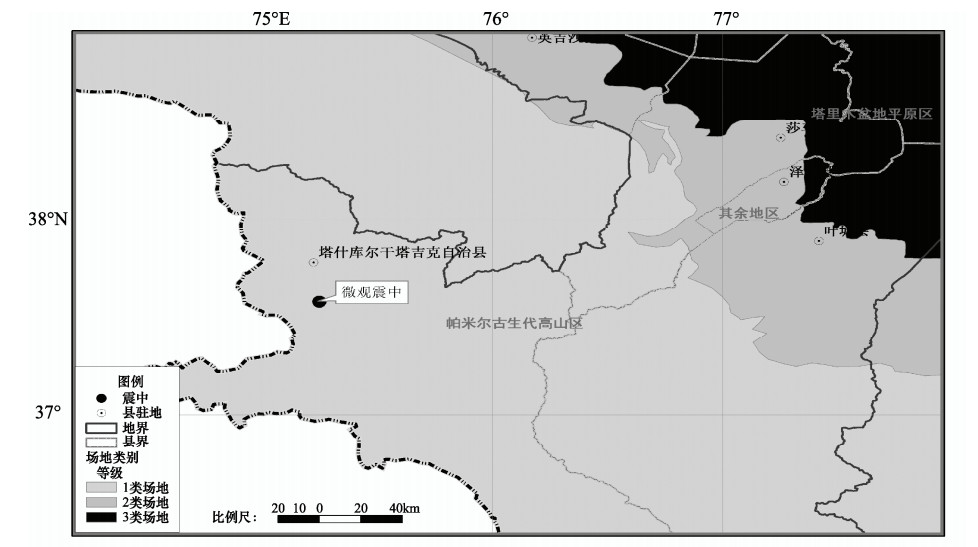
此次震中位于塔什库尔干谷地内,发育有塔什库尔干河,震区附近谷地与两侧高差700—1200m,谷地总体走向近南北,宽数千米,呈狭长状,谷地两岸冰碛物堆积及冲洪积堆积发育,村庄沿河流阶地及山前冲洪积扇分布(刘军等,2014),该地貌单元内场地类别为Ⅰ类,如图 3所示。
2. 震害特征分析
2.1 地震灾害特征分析
本次地震涉及影响范围内的房屋结构类型主要包括简易房(土石木结构)、砖混结构、砖木结构及少量的框架结构。简易房按照承重墙体可分成两类,其中一类主要分布在山前洪积扇倾斜平原地带,多为当地居民就地取材而建,屋顶结构为先搭建房梁后在其上搁置短木条作为椽子,在椽子上铺设草席后覆盖房泥,部分老旧房屋屋顶房泥较厚,墙体由卵石、粉土砌筑而成,粘结强度极差,加之施工质量和场地条件的影响,造成一定数量的毁坏和大面积破坏,是导致本次地震造成人员伤亡的主要原因;另一类主要分布在塔什库尔干河两岸阶地上,此类房屋多为土坯砌筑而成。由于该地区经济落后,交通极为不便,建造成本高,在县城存在大量2000年左右建设的土石木房屋。在地震中,第一类房屋大面积倒塌,房屋倒塌基本为整体性倾覆,第二类土坯房倒塌相对略少,多为局部倒塌,2种不具抗震性能的房屋破坏面积较严重,计算时均列入土石木结构房屋,该类房屋在乡镇Ⅶ度区毁坏达39.1%,在县城的Ⅶ度区毁坏达24.3%。震区各类结构房屋面积如表 1所示。
表 1 震区各类结构房屋面积(单位:m2)Table 1. Total areas of various kinds of structures in the earthquake area (unit: m2)行政区 土木结构面积 砖木结构面积 砖混结构面积 框架结构面积 总面积 县城 9000 114700 171135 325700 620535 乡镇 814770 46954 47636 0 909360 乡镇中的砖木结构房屋为近年新建居住用房,设有构造措施,抗震能力好;老旧砖木房屋未经抗震设防,砌筑工艺和质量较差。震区老旧砖木房屋严重破坏现象为房屋承重墙体大面积剪切裂缝或外闪,局部屋顶塌落;中等破坏现象主要为墙体斜向或竖向开裂,宽度约1mm,但延伸长度较长,由墙体顶部延伸至底部。Ⅶ度区严重破坏以上达24.3%,但无整体倒塌房屋,该类结构房屋未造成人员伤亡。
砖混结构房屋主要是乡(镇)公用房屋,或县城的居住用房。2010年后建设的砖混结构房屋抗震能力较好,地震后出现一定数量墙体细微开裂。2000年前建设砖混结构办公楼设防烈度低,在地震中造成一定数量严重破坏。震区典型房屋灾害如图 4所示。
框架结构多为2010年后新建办公用房,抗震能力好,未产生结构性破坏,但出现大面积填充墙开裂,修复量大。
通过对震区69个调查点进行均匀抽样调查(孙景江等,2011),最后得到本次地震震区房屋破坏比,如表 2所示。
表 2 震区各类结构房屋的破坏情况Table 2. Statistial results of building damages of various structures in the earthquake area行政区 单位 毁坏 严重破坏 中等破坏 轻微破坏 破坏合计 不具备修复价值 县城 m2 26156 54751 74645 341811 497363 99568 间 1308 2738 3732 17091 24869 4979 户 327 685 933 4273 6218 1245 乡镇 m2 80958 151041 194444 260836 687279 280610 间 4048 7552 9722 13042 34364 14031 户 1012 1888 2431 3261 8592 3508 2.2 基础设施与生命线工程的破坏
在市政设施方面,地震造成42km供排水管道、34km供暖管道和4座供热站受损,造成供水厂、污水厂氧化池及构筑物受损;交通系统方面,共86km道路损毁,350m隧道严重损坏,9座桥涵、135处涵洞和20km道路防护损坏。塔什库尔干县村庄道路局部塌陷情况如图 5所示。
在水利系统方面,地震造成114km水渠受损,对震区农作物灌溉造成一定影响。在达布达尔乡,草场水渠破坏造成库什吾尼可尔村、恰特尔塔什村、土拉村及库什吾尼可尔村等30余户、约0.12km2棉作地灌溉受影响,对震区居民的收入造成一定影响,恢复时间需要1个月左右。塔什库尔干县达布达尔乡阿特加依里村草场水渠地基失稳,在地震作用下完全破坏(图 6)。
在电力系统方面,地震造成63座(110kV、220kV)塔基局部受损,1座110kV变电所和11座35kV变电所受损。电力系统破坏造成塔什库尔干乡布依阿勒村、加隆且特村和吐尔得库勒村等近60户居民用电中断,经过5天的抢修,断电区域已经恢复供电。
在通讯系统方面,地震造成移动、联通、电信公司7个核心机房受损(图 7),3座铁塔损坏,5km光缆倒伏。通讯系统破坏造成塔什库尔县城辖区内部分居民通信不稳定、少数居民固定电话不能呼入进户,经过2天的紧急抢修,通讯基本恢复正常。
3. 安居富民工程减灾效益分析
在近年来新疆发生的历次破坏性地震中,震区建设的安居富民工程和抗震安居房(张勇,2005)在减少人员伤亡和经济损失中发挥了显著作用(谭明等,2014)。在此次地震中,塔什库尔干县绝大部分倒塌的房屋为老旧的简易房,造成人员伤亡的房屋均为土石木房屋,宏观震中附近的安居富民房屋均完好。新疆大规模实施安居富民工程后,建造的农居符合设计规范要求,无一受到毁坏或者严重破坏,抗震性能得到检验(唐丽华等,2016)。
震后通过对灾区进行抽样调查,统计了安居房及安居工程改造的土木、砖木及砖混结构房屋所占比例,并根据灾区安居房未改造前的土木、砖木及砖混结构房屋数据,结合新疆地区安居富民房震害矩阵,对塔什库尔干5.5级地震的减灾效益进行了计算和分析。在地震灾害损失评估中,将抗震安居房和安居富民房面积替换为改造前的简易房屋面积,计算抗震房减灾效益(刘军等,2016),具体数据见表 3。
表 3 塔什库尔干县震区安居富民房减灾效益对比Table 3. Statistical results of reducing damage with anti-seismic living room project类别 未进行安居工程改造损失 实际损失 减少损失 受伤人数 68 31 37 死亡人数 34 8 26 受灾人数 53438 26486 26952 房屋直接经济损失/亿元 68.8 20.05 38.75 需紧急安置人数 36783 16194 20589 恢复重建费用/亿元 88.3 29.34 42.96 4. 结语
(1)此次地震属于浅源中强地震,震源深度8km,地面振动强。极震区位于地震断裂上方,灾害破坏较集中,对震中附近的库孜滚村造成了毁灭性破坏,与同级别地震相比较灾情较重。
(2)塔什库尔干盆地是1个冰碛堆积盆地,其地下沉积物质具有强烈的不均一性,这种分选性极差的场地地基条件对地震动有一定放大效应,因此造成县城城区内的砖混结构房屋出现了不同程度的破坏,多数框架结构房屋填充墙大面积开裂。
(3)灾区位于帕米尔高原,自然条件恶劣,资源匮乏,经济落后,建设成本高,自建房屋质量差,抗震能力低,也是本次地震震级不大、震害较重的重要原因。
-
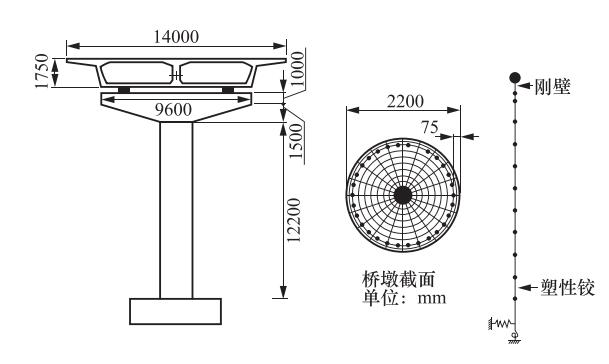
表 1 地震动记录参数
Table 1. Parameters of ground motions
序号 记录名称 断层距/km 震级 场地 PGA/g PGV/m·s-1 PGD/m Ⅱ-1 RSN4458_MONTENE.GRO_ULO090 3.97 7.1 Ⅱ 0.25 0.490 0.196 Ⅱ-2 RSN6886_DARFIELD_CACSN40E 14.48 7.0 Ⅱ 0.19 0.294 0.294 Ⅱ-3 RSN6886_DARFIELD_CACSN50W 7.58 7.0 Ⅱ 0.20 0.490 0.490 Ⅱ-4 RSN6897_DARFIELD_DSLCN27W 7.29 7.0 Ⅱ 0.26 0.392 0.294 Ⅱ-5 RSN6911_DARFIELD_HORCN18E 5.07 7.0 Ⅱ 0.45 1.078 0.490 Ⅱ-6 RSN6960_DARFIELD_RHSCN86W 13.64 7.0 Ⅱ 0.19 0.294 0.196 Ⅱ-7 RSN8124_CCHURCH_RHSCS04W 9.05 6.2 Ⅱ 0.25 0.294 0.098 表 2 各类场地柔性地基模型的土弹簧刚度
Table 2. Stiffness of soil-spring of flexible models at four sites
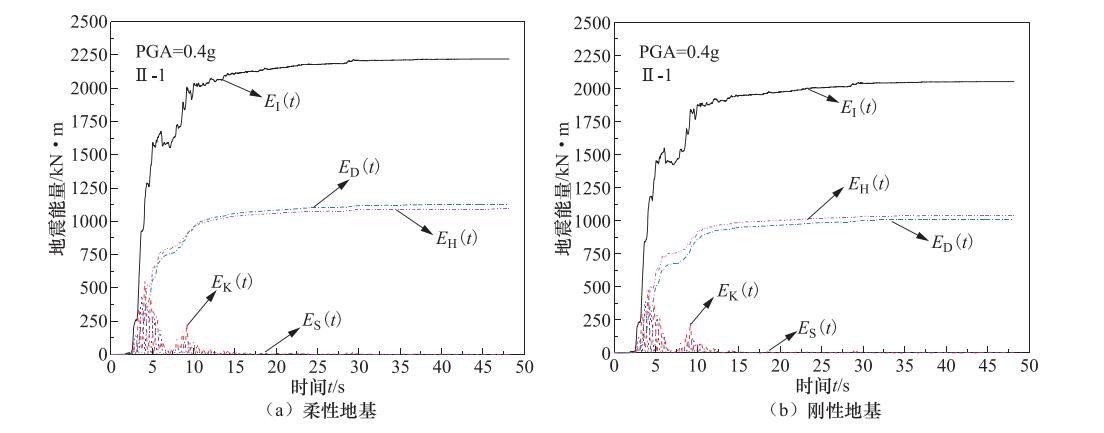
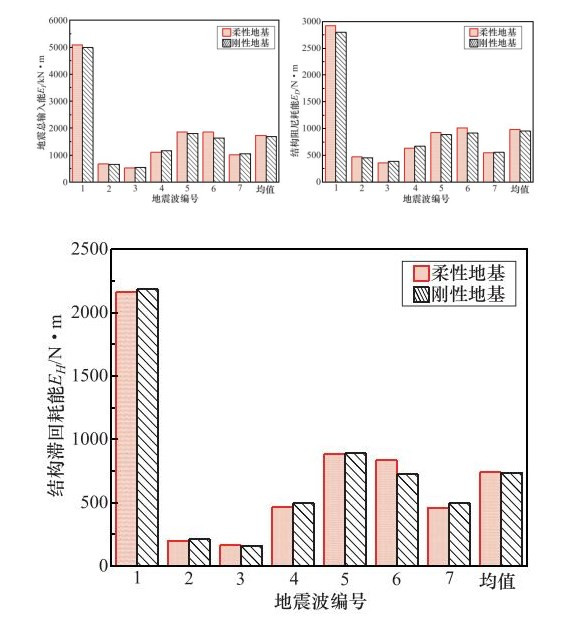
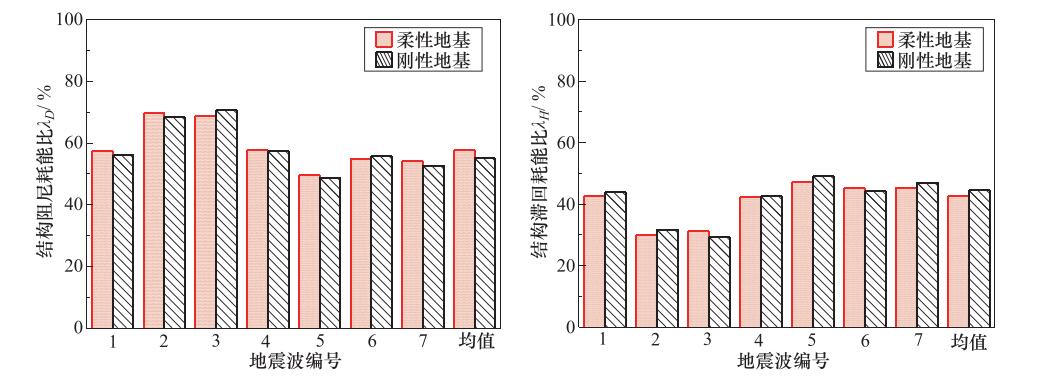
场地 剪切波速/m·s-1 有效剪切模量/MPa 平动刚度/kN·m-1 转动刚度/kN·m·rad-1 Ⅱ 375 212.8 150.83×105 56.33×106 Ⅲ 200 57.9 39.98×105 14.93×106 Ⅳ 115 13.9 8.52×105 3.18×106 表 3 场地类型对简支梁桥的地震能量响应及分配规律的影响
Table 3. Effect of site on seimic energy response and its distribution
场地 剪切波速/m·s-1 基阶周期T/s 地震总输入能EI/kN·m 滞回耗能EH/kN·m 阻尼耗能ED/kN·m 滞回耗能比λH/% 阻尼耗能比λD/% Ⅰ 750 1.02 688.64 270.62 418.02 39.30 60.70 Ⅱ 375 1.08 733.10 282.98 450.12 38.60 61.40 Ⅲ 200 2.36 1100.22 225.52 874.70 20.50 79.50 Ⅳ 115 4.73 1176.15 138.59 1037.56 11.78 88.22 -
卜一, 吕西林, 周颖等, 2009.钢框架-混凝土核心筒混合结构滞回耗能比研究.结构工程师, 25(1):55-61, 74. http://d.old.wanfangdata.com.cn/Periodical/jggcs200901012 经杰, 叶列平, 钱稼茹, 2003.基于能量概念的剪切型多自由度体系弹塑性地震位移反应分析.工程力学, 20(3):31-37. http://d.old.wanfangdata.com.cn/Periodical/gclx200303006 李宇, 2010.考虑残余位移和土-结构相互作用的桥梁结构基于性能的抗震设计及评估.北京: 北京交通大学. 刘哲锋, 沈蒲生, 2009.高层混合结构滞回耗能比的研究.地震工程与工程振动, 29(2):73-78. http://d.old.wanfangdata.com.cn/Periodical/dzgcygczd200902011 王建民, 2006.基于概率的桥梁结构抗震性能研究.北京: 北京交通大学. 肖明葵, 2004.基于性能的抗震结构位移及能量反应分析方法研究.重庆: 重庆大学. 中华人民共和国交通运输部, 2008.公路桥梁抗震设计细则(JTG/T B02-01-2008).北京:人民交通出版社. 中华人民共和国交通运输部, 2007.公路桥涵地基与基础设计规范(JTG D63-2007).北京:人民交通出版社. American Society of Civil Engineers, 2000. Prestandard and commentary for the seismic rehabilitation of buildings. FEMA 356, Washington: Federal Emergency Management Agency. Amiri V. J., Amiri G. G., Ganjavi B., 2008. Seismic vulnerability assessment of multi-degree-of-freedom systems based on total input energy and momentary input energy responses. Canadian Journal of Civil Engineering, 35(1):41-56. doi: 10.1139/L07-085 Arroyo D., Ordaz M., 2007. On the estimation of hysteretic energy demands for SDOF systems. Earthquake Engineering & Structural Dynamics, 36(15):2365-2382. doi: 10.1002/eqe.93/abstract Chou C. C., Uang C. M., 2003. A procedure for evaluating seismic energy demand of framed structures. Earthquake Engineering & Structural Dynamics, 32(2):229-244. http://home.iitk.ac.in/~vinaykg/Iset469.pdf Housner G. W., 1956. Limit design of structures to resist earthquakes. In: Proceedings of the 1st World Conference on Earthquake Engineering. California: Earthquake Engineering Research Institute. Surahman A., 2007. Earthquake-resistant structural design through energy demand and capacity. Earthquake Engineering & Structural Dynamics, 36(14):2099-2117. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ028281987/ 期刊类型引用(1)
1. 彭刚辉,姚国文,贾宏宇,郑史雄,李世亚. 基于能量法的隔震连续梁桥地震响应分析. 铁道标准设计. 2023(09): 89-95 .  百度学术
百度学术其他类型引用(0)
-





 下载:
下载:






 下载:
下载: