Comparison of Four Simplified Analytical Methods for Seismic Design of Underground Structures
-
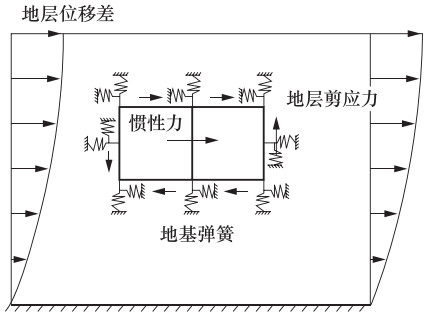
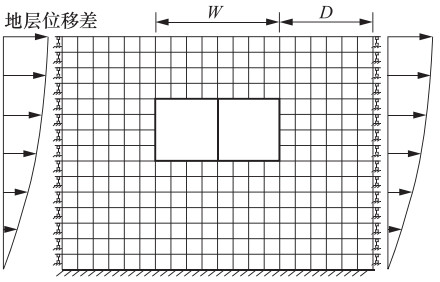
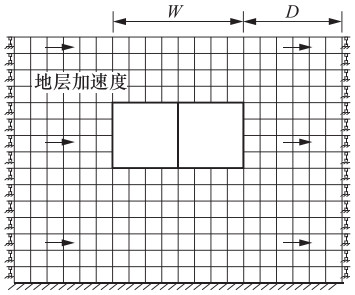
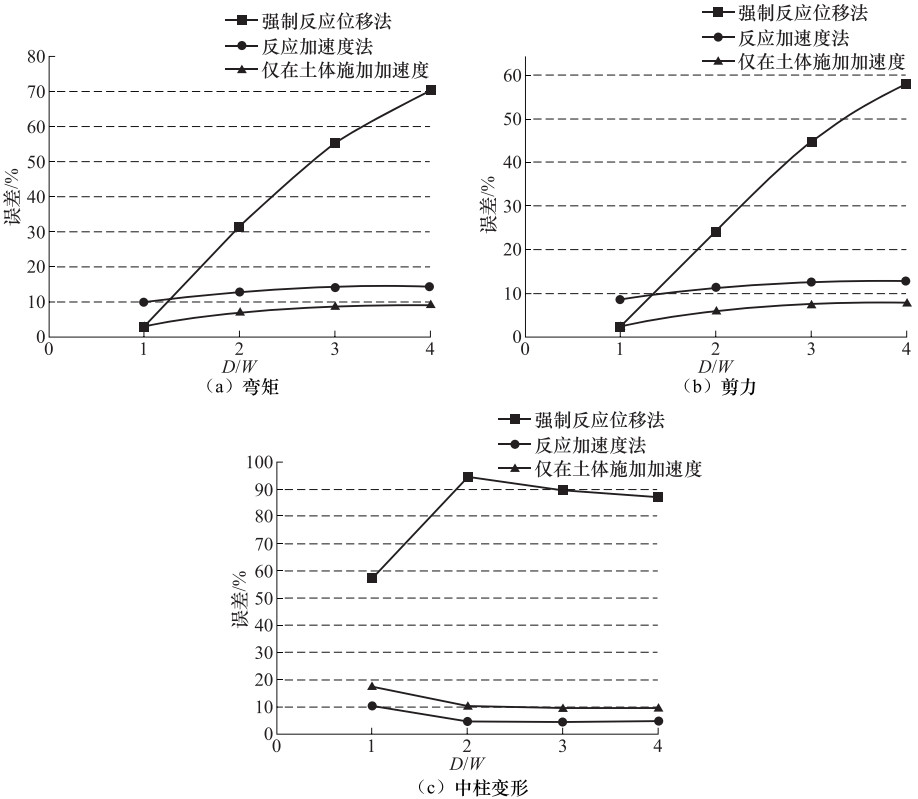
摘要: 在地下结构抗震设计简化分析方法中,强制反应位移法将土层变形施加在有限元模型侧边界模拟地震作用,反应加速度法将土层加速度施加到整个有限元模型上模拟地震作用,此外还有仅将土层加速度施加到土层模型上模拟地震作用的方法。上述方法均规避了反应位移法中关于弹簧刚度的取值问题,提高了计算效率。本文以1个双跨箱形结构为例,用动力时程分析的计算结果作为校核,分析了强制反应位移法、反应加速度法和仅将土层加速度施加到土体中的简化分析方法在不同侧边距条件下的计算精度,再结合常用的反应位移法,对比分析了4种简化分析方法的误差。分析结果表明:使用强制反应位移法时,侧边距取为1倍结构宽度导致的误差最小,反应加速度法和仅在土体施加加速度的简化方法对侧边距取值不敏感,反应位移法在角点造成的误差最大。Abstract: The theoretical basis of the simplified analytical method for seismic design of underground structure is that the deformation of underground structure depends on the deformation of foundation soil during earthquake, which fails to reflect the dynamic characteristics of the structure. Based on the most commonly used seismic displacement method, three kinds of simplified analytical methods are discussed in this paper. The forced displacement method is by applying the ground deformation to the boundary of the soil finite element model to simulate seismic load. The response acceleration method is by applying the ground acceleration to the integral finite element model to simulate seismic load. The third method is only by applying the ground acceleration to the soil finite element model to simulate seismic load. The above three simplified analytical methods can avoid calculating the value of the spring stiffness and improve the computational efficiency. Taking a double-span box structure as example, the calculations by applying three kinds of simplified analytical methods above with different lateral margins are carried out, and the analysis results are verified with the results of the dynamic time history method to calculate precisions. Combined with seismic displacement method, the error of four kinds of the simplified analytical methods is compared. The results show that the lateral margin should be equal to structure width when using the forced displacement method, and the response acceleration method and the method with only soil acceleration are not sensitive to the value of the lateral margin, and the error caused by the seismic displacement method reaches the maximum at the corner.
-
引言
中国大陆东部断裂系统是由一系列北东和北西走向断裂构成的共轭剪切破裂系统,其中北西向断裂处于破裂初期,活动性较强,多断续,集中成带分布并一定程度上控制着中强地震的发生(邵云惠,1980;李祖武,1983;冯希杰,1988;徐杰等,2003)。针对该组断裂的研究成果将对防震减灾产生重要意义。由于平原区NW向断裂都是隐伏断裂,所以对其具体位置尚需更深入的研究。通过测量断层气来探测隐伏断裂带已成为行之有效的方法之一,目前已在多条隐伏断裂探测工作中取得了良好的效果(汪成民等,1991;陈刚等,1995;苏鹤军等,2005;刘菁华等,2006;邵永新等,2007;姚道平等,2008;刘学领等,2011;冯军等,2011;张慧等,2013)。该方法将断裂视为天然地质体中连接深部与浅部的“通道”,大量深部气体如H2、Rn和Hg气等可经该通道向上逸出,导致以上气体浓度在断裂位置附近异常升高,利用该原理则可通过圈定断层气异常点位来探测断裂的空间位置。
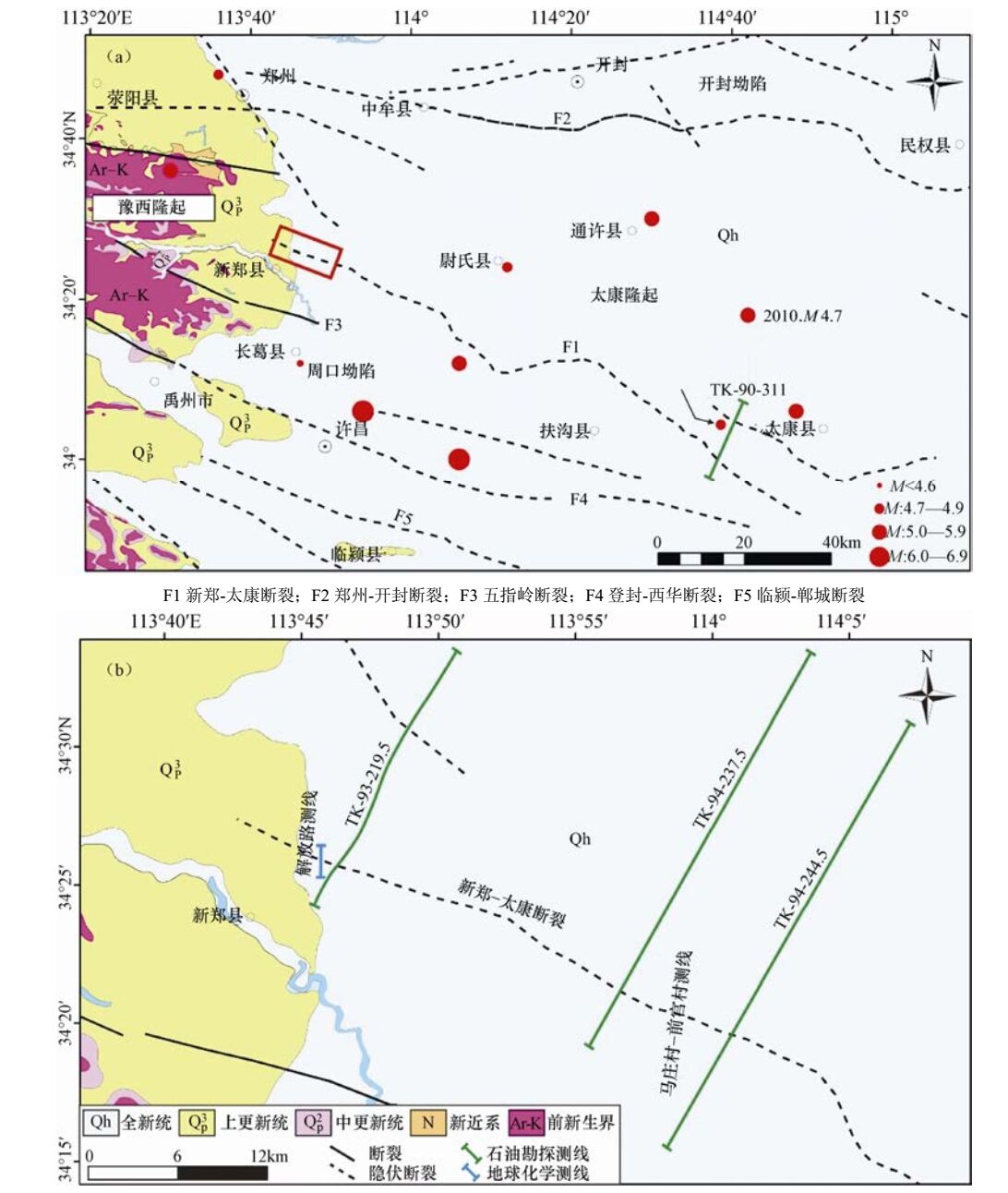
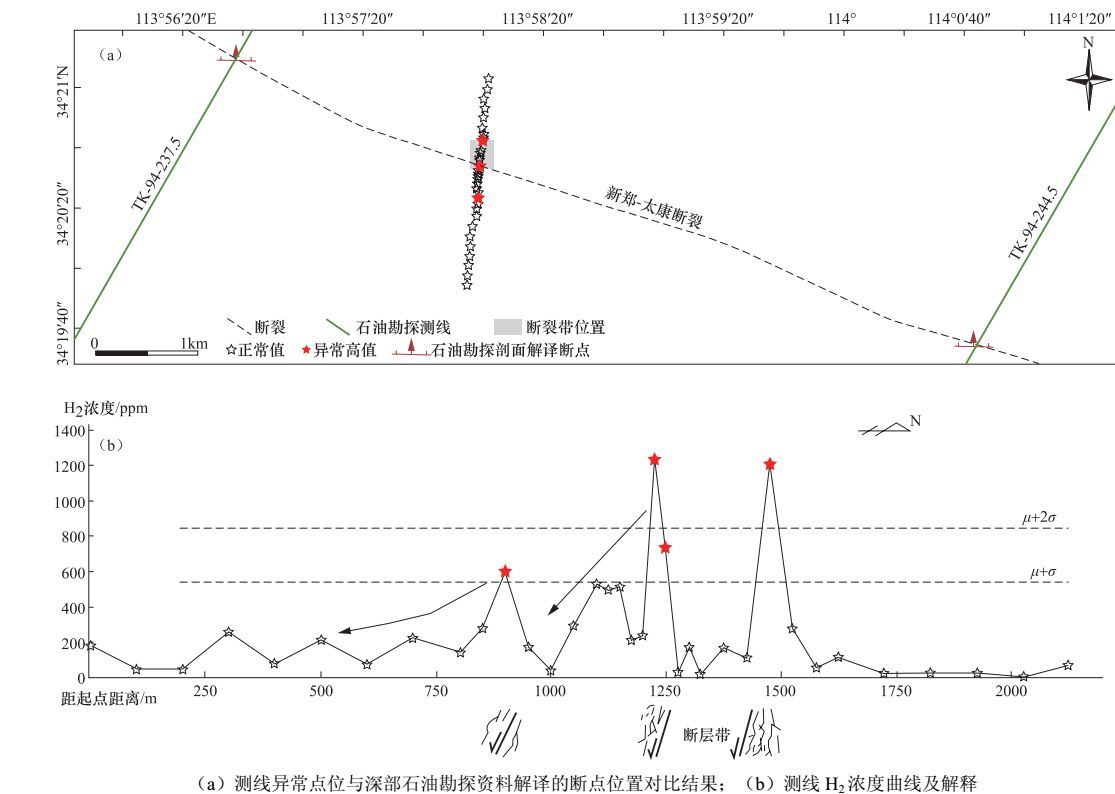
研究区位于华北平原西部,区内第四系厚度达150—300m以上(河南省地质矿产局,1989)。新郑-太康断裂为一条北西向隐伏断裂(王志铄等,2017),由于缺乏系统的浅层地震勘探和钻孔资料,目前对其空间展布仍然存在争议。本次在利用石油勘探资料对新郑-太康断裂进行深部定位的基础上,在新郑地区布置2条与断层走向近垂直的地球化学测线,通过测量土壤中的H2浓度并分析H2分布特征,以确定该断裂的浅层位置。
1. 区域地震地质背景
太康隆起西接豫西隆起,东邻鲁西隆起,南北分别为周口坳陷和开封坳陷。该区是豫西隆起与鲁西隆起延伸、连接的过渡单元,其基底以元古代五指岭组花岗片麻岩、黑云母片麻岩为主,上覆震旦系坚硬的安山玢岩(河南石油勘探指挥部地质大队,1976),进入新生代以来该区整体下沉并伴随强烈的断裂活动(石油化工部物探局,1975)。南部周口坳陷是第三系及上古生界的沉积坳陷,基底埋深约3—6km,岩性以五指岭组片岩、嵩山组石英岩和登封杂岩为主(燃化部六四六厂研究所,1972)。其内部发育多条具有一定规模的北西向隐伏断裂(图 1),如登封-西华断裂(F4)和临颍-郸城断裂(F5),将周口坳陷切割为多个次级凹陷与凸起。
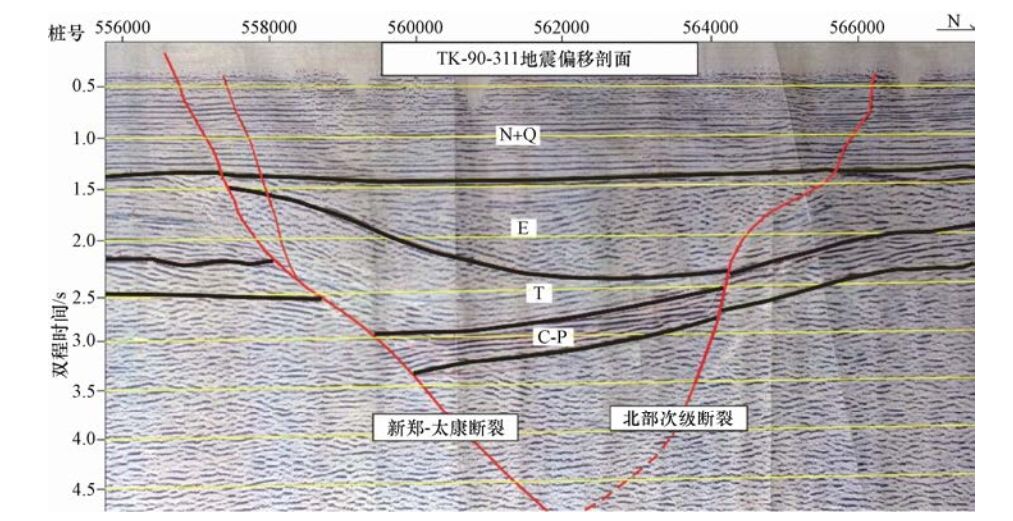
新郑-太康断裂(F1)起于新郑市北,向东经鄢陵,延伸至太康县以南,长147km,是太康隆起和周口坳陷的分界断裂(图 1)。最新的深部石油勘探资料表明该断裂整体倾向北,倾角较陡,在东段显示出正断特征,中段和西段表现为逆断层。新郑-太康断裂在浅部向上分叉,与北部次级断裂组合为负花状构造,具有明显的离散走滑特征,且新近纪以来活动明显,新近系底界断距可达150m(图 2,据河南油田勘探开发研究院内部资料)。
 图 2 新郑-太康断裂东段TK-90-311地震偏移剖面(剖面位置见图 1(a))Figure 2. Seismic profile No.TK-90-311 across the eastern segment of Xinzheng-Taikang fault
图 2 新郑-太康断裂东段TK-90-311地震偏移剖面(剖面位置见图 1(a))Figure 2. Seismic profile No.TK-90-311 across the eastern segment of Xinzheng-Taikang fault此外,周口坳陷内发生过多次5.0—6.0级地震,地震活动水平略高于太康隆起,区内最新的地震为2010年太康4.7级地震,震中位置见图 1(a),发震构造为新郑-太康断裂(孙杰等,2014)。
2. 探测实施与结果
2.1 探测仪器及测试方法
本次H2测试采用的仪器为德国德尔格公司生产的X-am 7000型多气体检测仪,传感器为电化学传感器,当气体扩散进入传感器后,在反应电极表面经过氧化或还原反应产生电流,并通过外电路流经2个电极,可通过外电路的负荷电阻测量气体浓度。该传感器可检测H2浓度上限为2000ppm,灵敏度为5ppm,测量误差±5%。该仪器稳定性和抗干扰能力均较强。
测线位置选择在新郑市解放路路侧和马庄村-前宫村的村间道路旁,测线位置见图 1(b),附近无污染源,地下水位稳定,埋深约10—15m。测量前,先对仪器进行校正,稳定后开始测量。测量时,先用锤将1.2m的钢钎打入土中成孔,拔出后立即将锥形麻花钻收集管拧入土中封孔,土壤气体通过锥形麻花钻收集管、胶皮管后进入测试仪器,由于H2比重小,逸出迅速。测量多次取峰值作为最终结果。
2.2 数据处理及断裂浅部定位
异常值的判定采用数值分析法,考虑到:① 测试区附近无污染源,空气中H2浓度背景值较低(2.113—5.998ppm);② 松散的第四系覆盖较厚,H2逸出条件复杂多变;③ 该断裂东段(太康段)向上延伸并分叉形成花状构造,分支断裂数目多且规模不等(中国地震局地球物理勘探中心,2015),同时断裂两侧裂隙发育,气体的逸出通道彼此相连或将导致产生于次级断裂深部的H2沿逸出条件更好的主断裂逸出,即气体发生偏移,我们认为异常值下限定得过高会导致部分次级断裂信息的丢失,因此将平均值与1倍标准差之和、平均值与2倍标准差之和综合考虑来划分异常。
断层的活动性质、几何特征(Ciotoli等,2007)以及破碎带和断层的发育程度(King等,1996;Annunziatellis等,2008)在一定程度上决定了地下裂隙的产生、分布及闭合,进而通过改变地下通道的连通状况来影响气体由深至浅的迁移过程。断层气浓度曲线蕴含丰富的地质信息,以下将对2条测线分别进行讨论:
(1)解放路测线
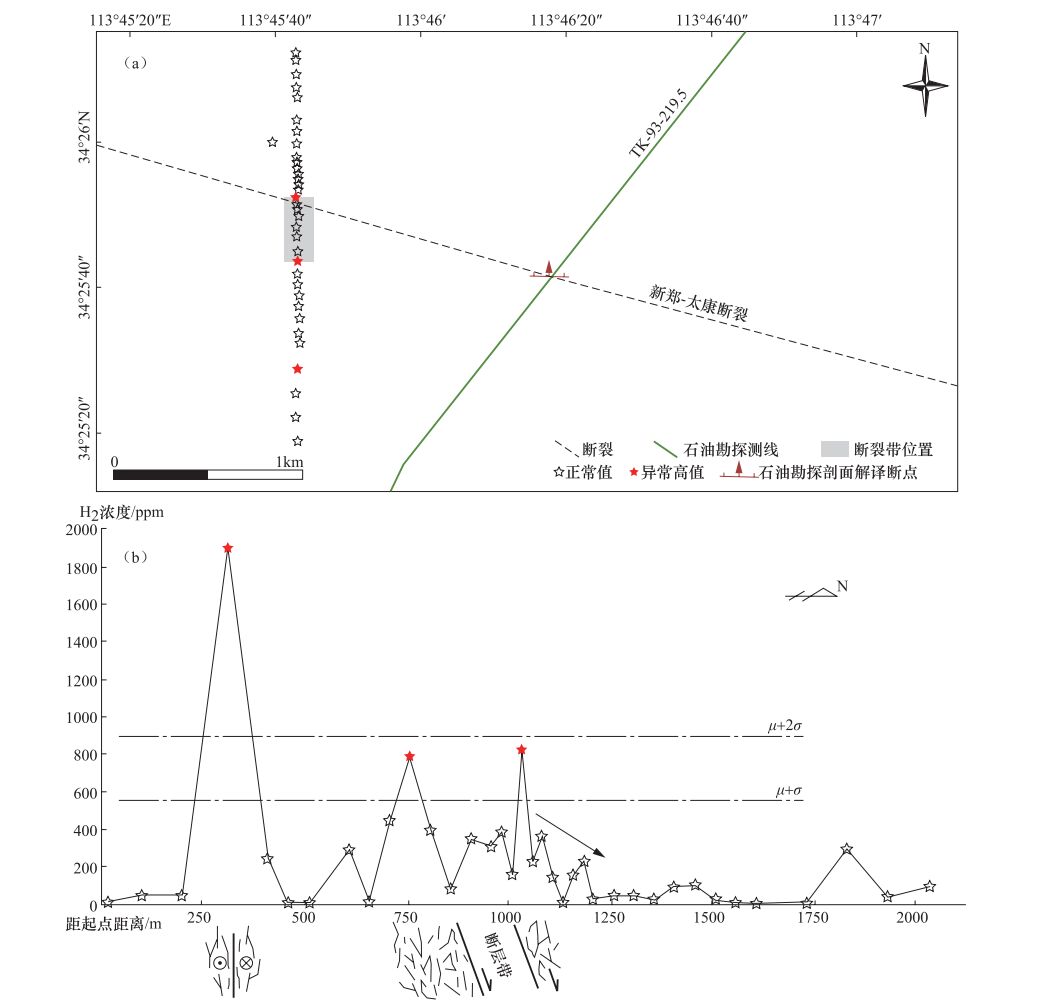
该测线位于新郑市西北解放路路侧(图 1(b)),共39个测点,长2035m,平均测点间距50m,当H2浓度明显高时,加密到每25m一个测点。至测线两端,测点间距扩大至100m。
各测点H2浓度的平均值μ=214.6ppm,标准差σ=341.4ppm,我们将浓度高于μ+2σ的异常点定为A类异常,表示异常幅度大,异常形态明显,对应规模较大的断裂;将高于μ+σ的异常点定为B类异常,该类异常幅度稍小,对应规模稍小的次级断裂。该测线异常点位置及类型见表 1,解释后的断裂见图 3。H2浓度在310m处异常高(1901ppm),为A类异常,且明显高于两侧,反映气体的逸出条件向两侧逐渐变差,破碎带集中在一个较窄的范围,故判断该处发育近直立的走滑断裂(Ciotoli等,2007);在760m与1035m处H2浓度接近(分别为789ppm和814.1ppm),为B类异常,在气体浓度曲线上表现出“双峰”态。由于新郑-太康断裂长期活动,带内充分发育断层泥等细粒物质导致断裂带渗透性降低,气体绕过渗透性低的断层带而从其两侧逸出,造成860—1010m间H2浓度低(340—360ppm)的现象,该处对应断裂带(King等,1996;Annunziatellis等,2008),其宽约150m。H2浓度在1035m处向北迅速下降,判断该断裂带倾向北。根据异常类型判断,该断裂带的规模、活动性不及南部的走滑断裂(张大其,1991;孟广魁等,1997;焦德成等,2012)。
表 1 解放路测线异常点位及类型Table 1. Location and type of anomalies from the Jiefang road survey line距测线起始点位置/m H2浓度/ppm 异常类型 断层倾向 310 1901 A 近直立 760 789 B N 1035 814.1 B N 平面上,将各异常点位置与断裂深部资料相比对可以发现,北部断裂带位置与断裂深部位置吻合较好。该测线H2浓度的高异常准确地反映了断裂的浅层位置(图 3)。
(2)马庄村-前宫村测线
该测线位于解放路测线的东南(图 1(b)),测线长2125m,共35个测点,点间距在测线两端为100m,高值附近加密到每25m一个点。各测点间H2浓度平均值μ为256.8ppm,标准差σ为302.7ppm。测线异常点位及类型见表 2,解释后的断裂见图 4。
表 2 马庄村-前宫村测线异常点位及类型Table 2. Location and types of anomalies from the Mazhuang-qiangong survey line距测线起始点距离/m H2浓度/ppm 异常类型 断层倾向 900 600 B S 1225 1250 A S 1475 1210 A S 根据表 2,该测线共有2处A类异常:H2浓度在测线1225m和1475m处分别为1250ppm和1210ppm,在浓度曲线上表现出与解放路类似的“双峰”形态,1275—1425m之间H2浓度低(25—175ppm),对应断裂带宽约150m。自1175m处向南H2浓度逐渐下降,故判断该断裂带倾向南。900m附近存在一处B类异常,H2浓度为600ppm,对应活动性稍弱的次级断裂(图 4)。
断裂在该段的深部位置由TK-94-237.5测线和TK-94-244.5测线控制,可以看到H2浓度异常点位置与石油勘探资料确定的断裂深部位置吻合较好,前者较准确地反映了断裂的浅层位置。
对比解放路和马庄村-前宫村H2浓度曲线可知,2条H2浓度曲线解释出了相近的断裂组合,即北部存在宽约150m的断裂带,以及向南约325—450m处发育的单支断裂。不同之处在于断裂带的倾向:解放路H2浓度曲线显示断裂带北倾,而马庄村-前宫村H2浓度曲线显示断裂带倾向南,倾向的变化表明新郑-太康断裂具有明显的走滑特征(漆家福等,2003;宋明春等,2015)。
不仅如此,我们还发现如下现象:
就单一测线而言,解放路H2浓度曲线表明该处南部的断裂规模更大、活动性更强,而马庄村-前宫村H2浓度曲线则表明该处北部断裂带的规模更大、活动性更强;就H2浓度沿断裂走向的变化规律而言,由北西向南东,北部断裂带的H2浓度升高,而南部断裂的H2浓度降低。
3. 结论与讨论
通过圈定和分析解放路与马庄村-前宫村测线上H2浓度的异常点,与深部石油勘探资料相对比,获得的主要结论如下:
(1)2条测线出现的高水平H2点位与石油勘探资料确定的断裂深部位置吻合度较高,准确地反映了断裂的浅层位置。
(2)2条测线同步解释出一条走滑断裂,以及其北部发育的一条宽约150m、产状变化的断裂带,产状变化与其走滑性质有关。
值得注意的是,就气体浓度沿断裂走向的变化规律而言,由北西向南东,北部断裂带H2浓度升高,而南部断裂的H2浓度降低。在气体逸出条件相近的前提下,南、北2条断裂的活动性呈“此消彼长”的关系。考虑到2条测线相距约20km,我们认为这2条测线可能位于一组左旋左阶断裂的阶区部位。但由于受到测线密度的限制,该结论有待进一步证实。
此外,关于该区氢气来源的问题亦值得讨论。华北地台具有由结晶基底和沉积盖层组成的“双重结构”(翟明国,2011;朱日祥等,2012;滕吉文等,2014):中条运动形成的结晶基底及上覆未经变质的中元古代以来的沉积盖层,盖层以碳酸盐岩和碎屑岩为主,而这些岩层中产生的H2微不足道(Ware等,1984)。目前已有大量实验表明,H2与CO2和CH4不同,其主要为无机过程的产物(Giardini等,1976;Ruff等,1980;Sugisaki等,1983)。构造应力作用下,破碎的矿物如石英(Hughes-Schrader等,1961;Wakita等,1980)、长石及硅酸盐矿物(Sugisaki,1980)表面的原子团与渗入裂隙中的水反应可以生成H2(Scharder机制,即Si+H2O→Si-OH+H2),含有黑云母的花岗岩可释放更多的H2(Sugisaki等,1983)。前已提及,太康隆起的沉积盖层下为震旦系火成岩、以花岗片麻岩及黑云母片麻岩为主的结晶基底,富含石英、黑云母等矿物,在动力破碎后通过反应释放出的大量H2,可能是本区土壤氢气的主要来源,由此可初步判断新郑-太康断裂至少为发育于上地壳的断裂,但由于缺乏更广泛、更精确的断层气及钻孔资料,该结论有待进一步研究和证实。
-
表 1 土体与结构的物理力学参数
Table 1. Physical and mechanical parameters of soil and structure
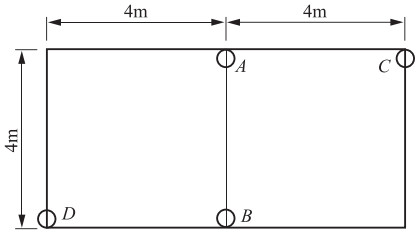
类型 密度/g·cm-3 弹性模量/MPa 泊松比 内摩擦角/° 粘聚力/kPa 土体 1.9 30 0.3 30 30 结构 2.3 30000 0.167 表 2 不同侧边距的弯矩计算结果
Table 2. Bending moment of structure with different lateral margins
D/W 使用方法 弯矩/kN·m 截面A 截面B 截面C 截面D 1倍 强制反应位移法 292.9 -291.3 280.8 353.9 反应加速度法 319.4 -324.5 294.0 367.1 仅在土体施加加速度 296.5 -300.6 278.4 351.8 2倍 强制反应位移法 179.5 -181.3 200.7 282.1 反应加速度法 328.1 -33.3 301.0 372.2 仅在土体施加加速度 309.3 -313.6 287.9 360.0 3倍 强制反应位移法 100.4 -101.3 147.7 224.7 反应加速度法 331.7 -336.9 303.8 374.9 仅在土体施加加速度 314.5 -18.9 291.8 363.9 4倍 强制反应位移法 50.2 -50.4 113.8 187.4 反应加速度法 332.6 -37.8 304.3 375.5 仅在土体施加加速度 316.2 -20.6 292.8 365.0 反应位移法 286.5 -290.1 201.0 199.8 动力分析 282.5 -89.1 272.3 345.2 表 3 不同侧边距的剪力计算结果
Table 3. Shear force of structure with different lateral margins
D/W 使用方法 剪力/kN 截面A 截面B 截面C 截面D 1倍 强制反应位移法 -182.6 -182.6 -213.5 -263.8 反应加速度法 -195.8 -204.0 -205.6 -271.7 仅在土体施加加速度 -186.6 -186.6 -196.8 -266.3 2倍 强制反应位移法 -112.8 -112.8 -169.0 -249.3 反应加速度法 -201.3 -209.4 -210.0 -274.3 仅在土体施加加速度 -194.7 -194.7 -202.0 -270.7 3倍 强制反应位移法 -63.0 -63.0 -144.4 -219.7 反应加速度法 -203.6 -211.7 -211.9 -276.2 仅在土体施加加速度 -198.0 -198.0 -204.2 -273.3 4倍 强制反应位移法 -31.4 -31.4 -128.2 -199.6 反应加速度法 -204.1 -212.3 -212.0 -276.4 仅在土体施加加速度 -199.0 -199.0 -204.5 -273.8 反应位移法 -174.8 -183.0 -106.2 -106.2 动力分析 -175.7 -180.6 -194.0 -266.7 表 4 不同侧边距的结构变形计算结果
Table 4. Deformation of structure with different lateral margins
D/W 使用方法 中柱顶、底相对位移/mm 1倍 强制反应位移法 2.396 反应加速度法 5.011 仅在土体施加加速度 4.636 2倍 强制反应位移法 0.302 反应加速度法 5.340 仅在土体施加加速度 5.026 3倍 强制反应位移法 0.584 反应加速度法 5.354 仅在土体施加加速度 5.071 4倍 强制反应位移法 0.722 反应加速度法 5.346 仅在土体施加加速度 5.077 反应位移法 3.628 动力分析 5.596 表 5 Kobe波下弯矩计算结果
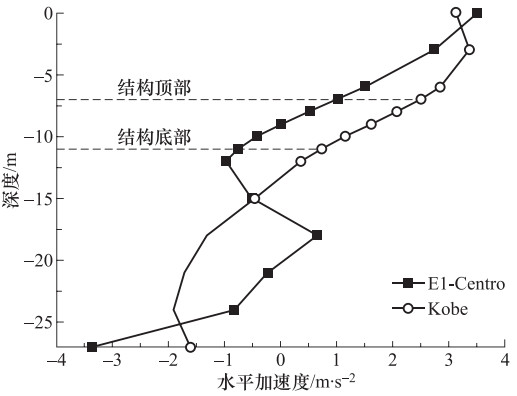
Table 5. Bending moment calculation results of structure under Kobe seismic wave
使用方法 弯矩/kN·m 平均误差/% 截面A 截面B 截面C 截面D 动力分析 375.3 -383.7 332.1 410.6 反应位移法 400.2 -403.7 284.3 276.0 10.5 强制反应位移法 298.8 -310.4 275.9 364.3 18.0 反应加速度法 341.9 -349.2 308.4 384.7 8.0 仅在土体施加加速度 312.4 -317.7 288.0 364.5 15.0 表 6 Kobe波下剪力计算结果
Table 6. Shear force calculation results of structure under Kobe seismic wave
使用方法 剪力/kN 平均误差/% 截面A 截面B 截面C 截面D 动力分析 -230.8 -242.9 -218.3 -299.0 反应位移法 -242.6 -257.5 -159.9 -139.9 13.7 强制反应位移法 -190.4 -190.4 -180.2 -290.7 27.0 反应加速度法 -207.4 -222.2 -209.8 -281.2 4.4 仅在土体施加加速度 -196.9 -196.9 -198.1 -275.4 5.6 表 7 Kobe波下结构变形计算结果
Table 7. Deformation calculation results of structure under Kobe seismic wave
使用方法 中柱顶、底相对位移/mm 平均误差/% 动力分析 5.808 反应位移法 4.768 17.9 强制反应位移法 3.232 44.4 反应加速度法 5.371 7.5 仅在土体施加加速度 4.900 15.6 -
陈国兴, 陈苏, 杜修力等, 2016.城市地下结构抗震研究进展.防灾减灾工程学报, 36(1):1-23. http://d.old.wanfangdata.com.cn/Periodical/jzgcjsysj2018063865 陈晓强, 钱七虎, 2010.我国城市地下空间综合管理的探讨.地下空间与工程学报, 6(4):666-671. http://d.old.wanfangdata.com.cn/Periodical/dxkj201004002 川岛一彦, 1994.地下构筑物の耐震设计.日本:鹿岛出版会. 林皋, 1990a.地下结构抗震分析综述(上).世界地震工程, (2):1-10. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000004586861 林皋, 1990b.地下结构抗震分析综述(下).世界地震工程, (3):1-10, 42. http://d.old.wanfangdata.com.cn/Periodical/dbdzyj201201005 刘晶波, 刘祥庆, 李彬, 2008.地下结构抗震分析与设计的Pushover分析方法.土木工程学报, 41(4):73-80. doi: 10.3321/j.issn:1000-131X.2008.04.011 刘晶波, 王文晖, 赵冬冬等, 2013.地下结构抗震分析的整体式反应位移法.岩石力学与工程学报, 32(8):1618-1624. http://d.old.wanfangdata.com.cn/Periodical/yslxygcxb201308014 刘如山, 胡少卿, 石宏彬, 2007.地下结构抗震计算中拟静力法的地震荷载施加方法研究.岩土工程学报, 29(2):237-242. doi: 10.3321/j.issn:1000-4548.2007.02.014 片山幾夫, 足立正信, 嶋田穰等, 1985.地下埋設構造物の実用的な準動的解析手法「応答震度法」の提案.土木学会年次学術講演会講演概要集, (40):737-738. 钱七虎, 2017.推进城市地下空间规划建设的思考.城乡建设, (18):60-65. doi: 10.3969/j.issn.1002-8455.2017.18.024 权登州, 王毅红, 井彦林等, 2015.黄土地区地铁地下结构抗震研究综述.震灾防御技术, 10(3):565-574. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20150310&journal_id=zzfyjs 同济大学, 2009.DG/TJ 08-2064-2009地下铁道建筑结构抗震设计规范.上海: 上海市建筑建材业市场管理总站. 杨智勇, 黄宏伟, 张冬梅等, 2012.盾构隧道抗震分析的静力推覆方法.岩土力学, 33(5):1381-1388. doi: 10.3969/j.issn.1000-7598.2012.05.016 中华人民共和国建设部, 2006.GB 50111-2006铁路工程抗震设计规范.北京:中国计划出版社. 中华人民共和国住房和城乡建设部, 2014.GB 50909-2014城市轨道交通结构抗震设计规范.北京:中国计划出版社. Hashash Y. M. A., Hook J. J., Schmidt B., et al., 2001. Seismic design and analysis of underground structures. Tunnelling and Underground Space Technology, 16(4):247-293. doi: 10.1016/S0886-7798(01)00051-7 Iida H., Hiroto T., Yoshida N., et al., 1996. Damage to daikai subway station. Soils and Foundations, 36(S):283-300. Penzien J., 2000. Seismically induced racking of tunnel linings. Earthquake Engineering Structural Dynamics, 29(5):683-691. doi: 10.1002/(ISSN)1096-9845 Tateishi A., 2005. A study on seismic analysis methods in the cross section of underground structures using static finite element method. Structural Engineering, 22(1):41-53. doi: 10.2208/jsceseee.22.41s Wang J. N., 1993. Seismic design of tunnels:a simple state-of-the-art design approach. New York:Parsons Brinckerhoff Quade and Douglas Inc. -





 下载:
下载:


 下载:
下载: