Analysis of Influence of Floor Lateral Stiffness Ratio on Seismic Vulnerability of Masonry Structures
-
摘要: 砌体结构的震害现象表明楼层侧向刚度不均匀分布是造成其破坏的重要原因之一。本文开展楼层侧向刚度变化对结构易损性的影响分析。以3层和6层砌体结构为例，采用等效多自由度层间剪切模型，基于非线性动力时程分析，定量研究了竖向刚度不规则性对砌体结构易损性的影响。以结构最大层间位移角为地震反应参数，借助增量动力分析及回归拟合方法，建立了基于峰值加速度的结构易损性曲线。通过改变楼层的侧向刚度值来模拟薄弱层，研究了楼层刚度变化对结构不同破坏状态超越概率的影响。通过改变底层与二层的侧向刚度比，分析了底部刚度突变对结构不同破坏状态超越概率分布的影响。研究表明：与规则结构相比，当刚度突变位于结构底层时，在地震作用下结构易损性相对较高；随着底层与二层的侧向刚度比从0.5增大至1.2，结构易损性逐渐降低。当刚度比为1.5时，结构薄弱层由底层转移至二层，结构整体易损性增加；当底层与二层侧向刚度比小于1时，结构倒塌易损性要显著高于规则结构。Abstract: Earthquake damage investigations have shown that the irregular vertical stiffness is one of the important causes of the serious destruction of masonry structures. In this paper, based on multi-degree-of-freedom shear type models, we take the vertical stiffness irregular masonry structure of three-story and six-story as example to evaluate structural behavior through change the position of irregulars tiffness from story drift ratios obtained through nonlinear time history analysis. Then, the structural response was obtained through incremental dynamic analysis, and the vulnerability curve of masonry structure based on the peak ground acceleration was established. At last, the influence of the stiffness change on the probability of the structure beyond the different damage states is analyzed. Meanwhile, the different lateral stiffness ratio of two adjacent structures is analyzed. The results show that under a given ground motion, in contrast to regular tifness, it could be found that the greater the change of the structure bottom stiffness, the more severe the structural damage in the earthquake. When the lateral stiffness ratio of the bottom two layers increase from 0.5 to 1.2, the earthquake resistance capacity of the structure increase, but when the lateral stiffness ratio increase to 1.5, structural weak-layer has been transferred, and its seismic capacity decreased. This study can be used to estimate the probability of vertical stiffness irregular masonry structures exceeding different damage states in future earthquakes.
-
Key words:
- Masonry structure /
- Incremental dynamic analysis /
- Stiffness ratio /
- Seismic vulnerability /
- Weak-layer
-
引言
控制建筑结构抗侧力系统的刚度变化是结构抗震设计的重要内容。结构的损伤、修复以及结构物用途的改变，都会引起结构楼层刚度的变化。在地震作用下，结构竖向刚度的变化容易导致薄弱层出现，引起结构抗震性能的退化。砌体结构广泛存在于我国城镇、农村地区，且普遍存在竖向刚度不规则现象。近年来，多次工程震害现象表明一些砌体结构在设计上存在缺陷，如结构竖向刚度不均匀，导致薄弱层的出现，造成房屋结构严重破坏甚至倒塌。汶川8.0级和芦山7.2级地震的砌体结构震害特征中，结构平面和立面布置不规则现象较为常见，特别是二层结构外挑的多层砌体结构，竖向刚度存在突变，结构物“头重脚轻”，引起结构底层严重破坏。此外，我国农村地区许多自建的砌体结构，开洞过多且位置布局不合理，造成结构抗侧刚度不足，在地震作用时出现应力局部集中，加重了结构的破坏程度（李小军等，2008；温增平等，2009；徐超等，2013）。尤其对于由砌体材料建造的教学楼，为满足其功能要求，结构开间和进深较大，因其采光和通风的需要，纵墙设置较多门窗，这使得结构抗侧刚度变化，在地震中破坏较为严重（周强等，2010）。
针对上述工程震害调查中竖向刚度不规则砌体结构呈现的破坏现象，学者们展开了一系列对竖向刚度不规则砌体结构抗震性能的研究。孙柏涛等（2012）对竖向变刚度约束砌体结构进行数值模拟，发现刚度由下到上逐渐变小的砌体结构抗震能力有一定程度提高；刘红彪（2012）通过两栋开洞较多砌体结构的振动台倒塌对比实验分析，得出结构层间同方向各墙体抗侧刚度应基本相等，对于开洞较大墙体设置构造柱可以使地震剪力在各墙段分配均匀，结构的抗震能力得到一定提高；刘砚山等（2013）研究了不同侧向刚度比对底框砌体结构抗震性能的影响，发现适当增大底层抗震墙刚度可以提高结构的抗震性能；孙雷等（2013）对单层层高超限、设置大开间的典型村镇自建竖向不规则砌体房屋进行数值模拟，发现抗震构造措施可以显著改变砌体结构动力特性，芯柱和墙体协同工作机制可以向弱框架结构转化，对结构抗震性能产生影响；孙柏涛等（2015）以汶川地震中1栋破坏严重的底框砌体结构为例，通过有限元分析发现，裙房对主体结构刚度影响较大，加剧了结构的地震反应，建议在结构设计时裙房和主体结构采用抗震缝隔开。
国内学者对砌体结构地震易损性做了大量的研究工作。杨玉成等（1982）对多层房屋结构易损性及其震害预测开展过研究；高小旺等（1990）研究了在不同烈度下砌体结构超越不同破坏状态的概率；张令心（2002）采用非线性时程分析研究了多层砌体结构地震易损性，计算了在不同烈度下多层砌体结构的易损性曲线；熊立红等（2003）对2栋新型砌块房屋进行非线性时程分析，研究了砌体房屋在抗震设防“三水准”下的结构出现不同破坏状态的概率。这些研究成果主要是针对规则砌体结构开展的地震易损性分析。
目前，针对竖向刚度不规则性对砌体结构地震易损性影响的研究较少，但在我国城镇及农村，许多自建的砌体结构普遍存在抗侧刚度不均匀的情况，当在这些地区开展震害预测时常常需要分析不规则的砌体结构易损性。为了考虑竖向刚度不规则性对砌体结构地震易损性影响，本文定量研究了楼层侧向刚度变化对结构超越不同破坏状态概率的影响，以3层和6层典型砌体结构为例，采用等效多自由度层间剪切模型，基于增量动力法（Incremental Dynamic Analysis，IDA）及回归拟合分析，建立结构地震易损性曲线。通过改变结构楼层侧向刚度分布形式来模拟薄弱层，分析了楼层刚度突变对结构不同破坏状态超越概率的影响。通过改变底层与二层侧向刚度比，研究了刚度变化对结构不同破坏状态超越概率的影响。
1. 基于IDA方法的砌体结构地震易损性
1.1 结构易损性模型
在易损性分析中，通常采用对数正态分布作为易损性概率模型（Singhal等，1996；Shinozuka等，2000），基于地震动参数的易损性可表示为：
$$ F(IM = x) = P(I{M_c} \le x) = \Phi \left({\frac{{{\rm{ln}}(x) - {\mu _{{\rm{ln}}\left({I{M_c}} \right)}}}}{{{\beta _{I{M_c}}}}}} \right) $$ (1) 由对数正态分布的性质可知，自然对数的均值等于中值的自然对数，则式（1）可表示为：
$$ F(x) = \Phi \left({\frac{{{\rm{ln}}(x/{\eta _{I{M_c}}})}}{{{\beta _{I{M_c}}}}}} \right) $$ (2) 式中，IM为描述地震动强度的随机变量；IMc为达到不同破坏状态时的地震动强度；F（IM）为易损性函数；Φ为标准正态分布累计函数。$ {\mu _{{\rm{ln}}\left({I{M_c}} \right)}}$、${\eta _{I{M_c}}}$、${\beta _{I{M_c}}}$分别为随机变量IMc的对数均值、中值和对数标准差，一般可以根据结构动力反应分析结果及回归拟合方法进行确定。
1.2 基于IDA的砌体结构地震反应分析
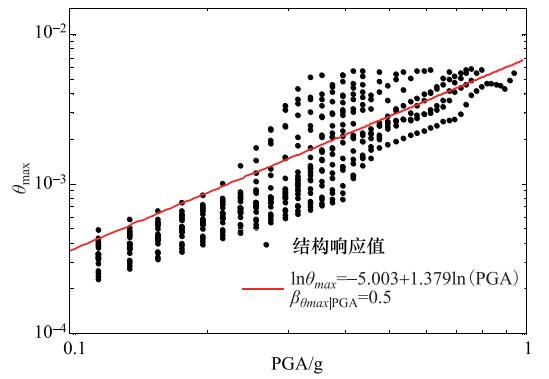
IDA作为1种有效的地震易损性分析方法，已广泛应用于不同结构类型的易损性分析（Vamvatsikos等，2002）。IDA曲线反应了结构响应参数与地震动参数之间的联系。本文选用结构地震响应值（最大层间位移角${\theta _{\max }}$）为曲线纵坐标，选用地震动输入参数（峰值加速度PGA）为横坐标。
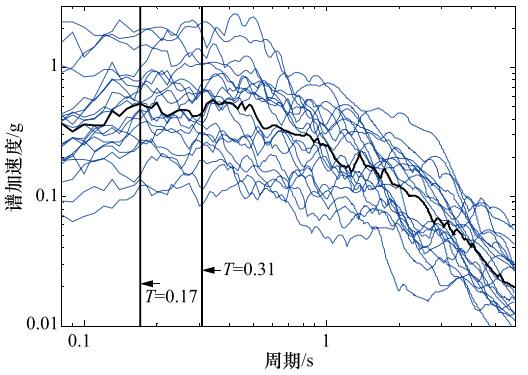
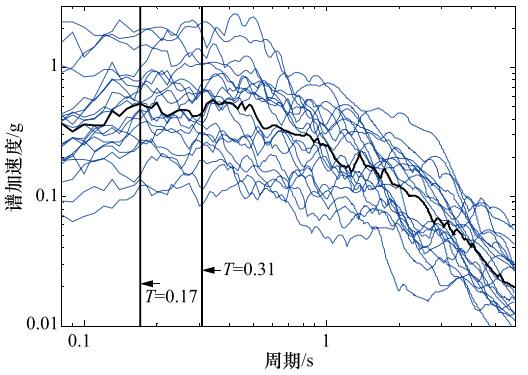
Vamvatsikos等（2002）研究表明，采用20条地震记录作为地震动输入可以很好地反映地震动的不确定性。本文从美国太平洋地震中心选择21条地震记录，震级分布为6—8级，PGA≥0.05g，VS30分布区间为210—510m/s，场地条件大致相当于我国Ⅱ类场地。挑选的地震动记录见表 1，其加速度反应谱见图 1，黑色实线为50%分位线。
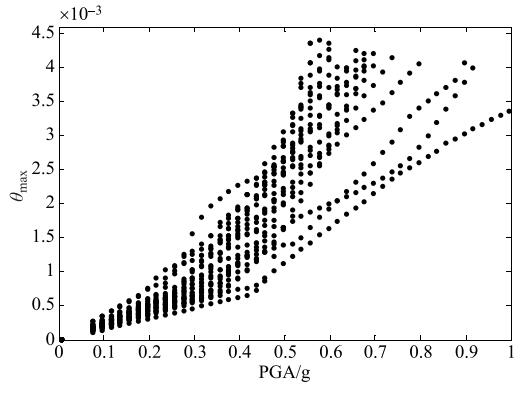
表 1 选择的地震动记录Table 1. Selected records of ground motion地点 台站 年份 Mw 断层距/km VS30/m·s-1 美国，圣费尔南多 Buena Vista–Taft 1971 6.61 111.37 386 伊朗，曼吉尔 Abhar 1990 7.37 75.58 303 美国，旧金山 El Centro Imp. Co. Cent 1987 6.54 18.2 292 美国，旧金山 Poe Road (temp) 1987 6.54 11.16 317 土耳其，迪兹杰 Bolu 1999 7.14 12.02 294 美国，兰德斯 Coolwater 1992 7.28 19.74 353 美国，兰德斯 Desert Hot Springs 1992 7.28 21.78 359 中国台湾，集集 HWA033 1999 7.62 39.55 379 中国台湾，集集 CHY046 1999 7.62 24.1 442 美国，阿拉斯加德纳利 Carlo (temp) 2002 7.9 49.94 399 美国，摩根山丘 Gilroy Array #3 1984 6.19 13.01 350 美国，赫克托矿 Hesperia - 4th & Palm 1999 7.13 89.87 358 意大利，伊尔皮纳 Calitri 1980 6.2 8.81 456 土耳其，科贾埃利 Goynuk 1999 7.51 31.74 348 土耳其，科贾埃利 Iznik 1999 7.51 30.73 477 美国，洛马普雷塔 Anderson Dam (L Abut) 1989 6.93 19.9 489 日本，阪神 Kakogawa 1995 6.9 22.5 312 美国，帝国谷 Cerro Prieto 1979 6.53 15.19 472 美国，帝国谷 Delta 1979 6.53 22.03 242 美国，帝国谷 El Centro Array #9 1940 6.95 6.09 213 日本，阪神 Shin-Osaka 1995 6.9 19.15 256 
图 1 挑选的地震动的加速度反应谱Figure 1. The acceleration response spectrum of selected ground motion以所选的地震记录作为输入对结构进行IDA分析，可得到结构的动力反应值，砌体结构最大层间位移角与峰值加速度对应关系的IDA分析结果，如图 2所示。研究表明，在给定强度地震动作用下，可认为结构的反应服从对数正态分布（Shome，1999），因此可采用对数线性概率地震需求模型建立结构反应与地震动参数之间的统计关系：
$$ \ln \left({{\theta _{\max }}|IM} \right) = A + B\ln \left({IM} \right) + \ln \varepsilon $$ (3) 式中，${\theta _{\max }}|IM$为给定强度IM地震动作用下的结构最大层间位移角。A、B为回归系数，$\varepsilon $为估计误差。
采用${\mu _{\ln ({\theta _{\max }}|IM)}}$表示$ln({\theta _{max}}|IM)$均值、${\eta _{{\theta _{\max }}|IM}}$表示${\theta _{\max }}|IM$中值、${\beta _{{\theta _{\max }}|IM}}$表示$\ln ({\theta _{\max }}|IM)$标准差，则有：
$$ {\mu _{\ln ({\theta _{\max }}|IM)}} = \ln ({\eta _{{\theta _{\max }}|IM}}) = A + B\ln (IM) $$ (4) $$ {\beta _{{\theta _{{\rm{max}}}}{\rm{|}}IM}} = {\sigma _{{\rm{ln}}\varepsilon }} $$ (5) 基于式（3）对图 2中的砌体结构反应与地震动参数进行回归拟合，可确定回归系数A、B及拟合标准差${\sigma _{{\rm{ln}}\varepsilon }}$，示意图如图 3所示。
图 3 砌体结构动力反应值与地震参数对数拟合结果Figure 3. Logarithmic fitting results of dynamic response and seismic parameters of masonry structure1.3 不同破坏状态对应的层间位移角限值
结构物破坏等级通常可分为5类（中华人民共和国国家质量监督检验检疫总局，中国国家标准化管理委员会，2009），分别为“基本完好”、“轻微破坏”、“中等破坏”、“严重破坏”和“倒塌”，用DSk（k=0，1，……，4）对应表示。
采用层间位移角作为变形指标对砌体结构的破坏等级进行划分。夏敬谦等（1988）和熊立红（2004）通过对砌体墙体进行大量试验，给出了不同破坏状态对应的最大层间位移角限值(${\theta _{\max }}|DS$)的参考均值，其变异系数${\delta _{{\theta _{\max }}|DS}}$取0.35（庄一舟等，1999）。
一般可以认为结构不同破坏状态对应的最大层间位移角限值服从对数正态分布，根据对数正态分布的性质，可采取式（6）、（7）计算不同破坏状态对应的层间位移角限值的中值${\eta _{{\theta _{\max }}|DS}}$和对数标准差${\beta _{{\theta _{\max }}|DS}}$，进而得到其概率分布参数（表 2）。
表 2 层间位移角限值Table 2. Allowable value of story drift ratios破坏状态 层间位移角均值（${\mu _{{\theta _{\max }}|DS}}$） 变异系数（${\delta _{{\theta _{\max }}|DS}}$） 层间位移角中值（${\eta _{{\theta _{\max }}|DS}}$） 对数标准差（${\beta _{{\theta _{\max }}|DS}}$） 轻微破坏（LS1） 1/1600（0.000625） 0.35 0.00059 0.34 中等破坏（LS2） 1/700（0.00143） 0.35 0.00135 0.34 严重破坏（LS3） 1/350（0.0028） 0.35 0.00264 0.34 接近倒塌（LS4） 1/200（0.005） 0.35 0.0047 0.34 $${\eta _{{\theta _{\max }}|DS}} = \frac{{{\mu _{{\theta _{\max }}|DS}}}}{{\sqrt {1 + {\delta ^2}_{{\theta _{\max }}|DS}} }}$$ (6) $${\beta _{{\theta _{\max }}|DS}} = \sqrt {\ln \left({1 + {\delta ^2}_{{\theta _{\max }}|DS}} \right)} $$ (7) 1.4 易损性曲线的生成
以结构反应参数为中间变量，易损性也可表示为：
$$ F(x) = P[{\theta _{\max }}|IM > {\theta _{\max }}|DS] = P[\ln {\theta _{\max }}|IM - \ln {\theta _{\max }}|DS > 0] $$ (8) 由前文可知，${\theta _{\max }}|IM$和${\theta _{\max }}|DS$均服从对数正态分布且相互独立，则$ {\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}IM){\rm{ }} - $${\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}DS){\rm{ }}$为正态分布随机变量，其统计参数分别为：
$$ {\eta _{{\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}IM){\rm{ }} - {\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}DS){\rm{ }}}} = {\rm{ln}}{\eta _{{\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}IM){\rm{ }} - {\rm{ }}}}{\rm{ln}}{\eta _{{\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}DS){\rm{ }}}} $$ (9) $$ {\beta _{{\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}IM){\rm{ }} - {\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}DS){\rm{ }}}} = \sqrt {{\beta ^2}_{{\theta _{{\rm{max}}}}{\rm{|}}IM} + {\beta ^2}_{{\theta _{{\rm{max}}}}{\rm{|}}D{\rm{S}}}} $$ (10) 则易损性函数可以表示为：
$$ F{\rm{(}}x{\rm{)}} = P[{\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}IM){\rm{ }} - {\rm{ln}}({\theta _{{\rm{max}}}}{\rm{|}}DS){\rm{ > 0]}} = \Phi \left({\frac{{{\rm{ln(}}x/{{{\rm{(}}{\eta _{{\theta _{{\rm{max}}}}|DS}}/{{\rm{e}}^A}{\rm{)}}}^{1/B}}{\rm{)}}}}{{\sqrt {{\beta ^2}_{{\theta _{{\rm{max}}}}{\rm{|}}IM} + {\beta ^2}_{{\theta _{{\rm{max}}}}{\rm{|}}D{\rm{S}}}} }}} \right) $$ (11) 对比式（2），可以得到易损性曲线的双参数${\eta _{I{M_c}|DS}}$和${\beta _{I{M_c}|DS}}$：
$$ {\eta _{I{M_c}|DS}} = {{\rm{(}}{\eta _{{\theta _{{\rm{max}}}}|DS}}/{{\rm{e}}^A}{\rm{)}}^{1/B}} $$ (12) $$ {\beta _{I{M_c}{\rm{|}}DS}} = \frac{1}{B}\sqrt {\beta _{{\theta _{\max }}{\rm{|}}IM}^2 + \beta _{{\theta _{\max }}{\rm{|}}DS}^2} $$ (13) 2. 结构模型
2.1 建筑概况
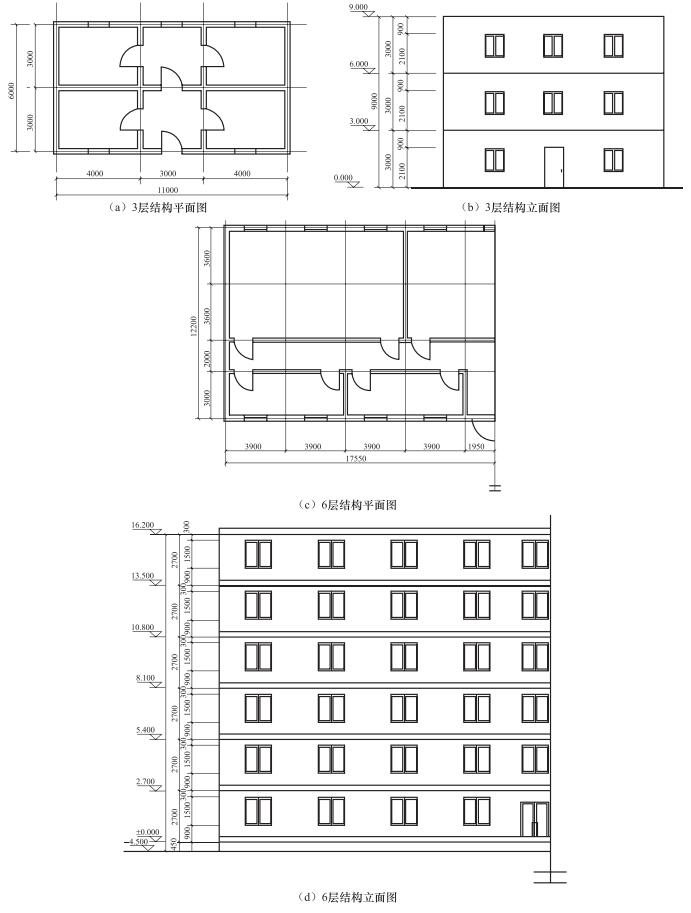
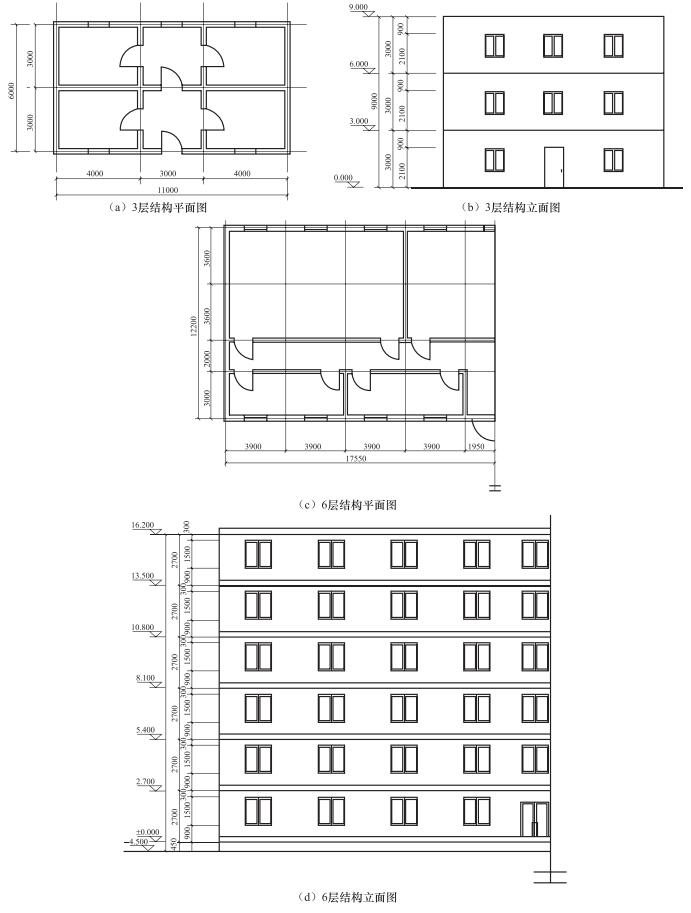
以3层和6层典型砌体结构为例，结构设防烈度Ⅶ度，设计场地为Ⅱ类。采用烧结黏土砖，等级MU15，砂浆强度等级M5。3层砌体层高均为3m，纵横墙承重，墙厚均为240mm。6层砌体层高均为2.7m，外墙厚370mm，内墙厚240mm。屋面均采用120mm厚混凝土楼板，选用C20混凝土。楼面活荷载3.5kN/m2，屋面活荷载4.5kN/m2。构造符合《砌体结构设计规范》（中华人民共和国住房和城乡建设部，2012）要求。在横墙与纵墙交会、外墙转角以及开间大于4.2m的位置设置构造柱，截面尺寸240mm×240mm，纵向配筋4Φ14，箍筋Φ6@ 150mm。结构布置图如图 4所示。
2.2 计算模型
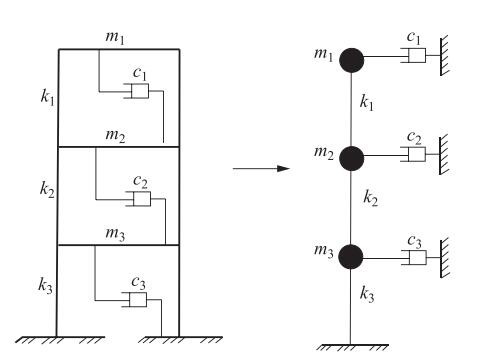
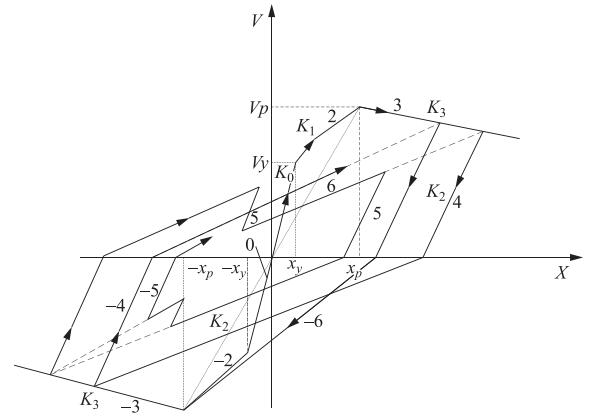
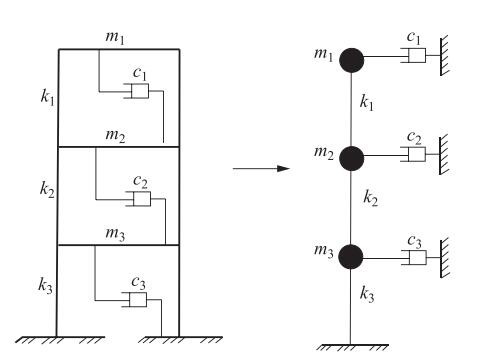
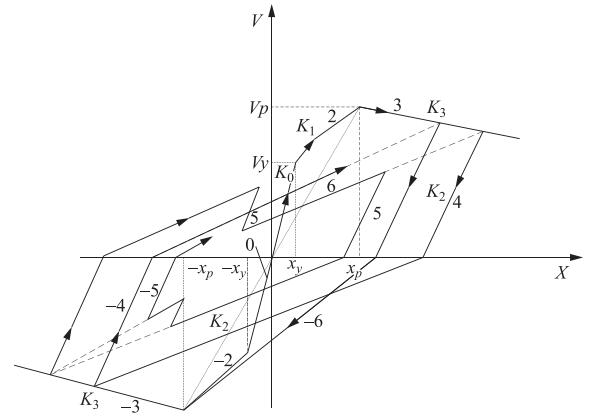
由于砌体结构高宽比较小，在地震作用下易表现出较为明显的剪切破坏。可以采用多自由度层间剪切模型对结构进行非线性时程分析（Xiong等，2016）。通常认为砌体结构楼板刚度无限大且忽略楼板转动位移，该模型将每层简化为1个质点，将每层的质量都集中在楼面上。多自由度层间剪切模型的示意图如图 5所示，其中c1、c2、c3为楼层阻尼，m1、m2、m3为楼层等效质量，k1、k2、k3为示意的楼层等效刚度。动力分析选用考虑刚度退化的三线性恢复力模型（刘锡荟等，1981），该模型有4个独立参数Vy、Vp、K0、K1，见图 6，其中Vy、Vp分别代表屈服荷载、极限荷载，xy、xp分别代表屈服位移、极限位移，K0为初始刚度，K1为硬化刚度，K2为卸载刚度，K3为软化刚度。
对于砌体结构而言，结构的横向和纵向抗震性能有所不同，纵向墙体开洞较多结构抗震性能较差（林旭川等，2008），本文主要对结构纵向进行非线性时程分析。
2.3 恢复力模型参数标定
刘锡荟等（1981）对带构造柱的砖墙足尺墙片进行侧向往复荷载加载实验，建立了砌体结构合理的滞回模型，给出了恢复力模型参数标定的方法；并选择2栋典型砌体结构进行对比验证，计算结果与实验结果拟合良好。《设置钢筋混凝土构造柱多层砖房抗震技术规程》（中华人民共和国建设部，1994）和张令心等（2002）对该恢复力模型进行进一步修正，给出了其恢复力参数的确定方法。
2.3.1 墙体抗侧移刚度
初始刚度的计算公式为：
$$ {K_0} = \frac{{{\lambda _0}G{A_m}}}{{\xi h}} $$ (14) 其中，G为砖砌体剪切模量（MPa）；Am为墙段水平截面总面积（m2）；$\xi $为剪力不均匀影响系数，矩形取1.2；h为墙段计算高度（m）；λ0为墙段考虑开孔和弯曲影响的刚度修正系数，
当墙的高宽比h/b≤1时，
$$ {\lambda _0} = \frac{{{\varphi _0}{A_z}}}{{{A_m}}} $$ (15) 当1≤h/b≤4时，
$$ {\lambda _0} = \frac{{{\varphi _0}}}{{\left({1 + \frac{{G{A_z}}}{\xi } \times \frac{{{h^2}}}{{12EI}}} \right)}} $$ (16) $$ {A_z} = {A_{jz}} + {\eta _0}\frac{{{E_c}}}{E}{A_c} $$ (17) 其中，Az表示墙段内混凝土构造柱的水平截面积之和（m2）；Ajz为墙段扣除混凝土构造柱后的砌体水平截面积（m2）；Ec为混凝土弹性模量（MPa）；E为砌体弹性模量（MPa）；η0为构造柱钢筋参与墙体的工作系数，η0=0.26；I为构造柱折算后与墙净截面一起计算的惯性矩（m4）；Ac为构造柱水平向截面面积（m2）；$ {\varphi _{\rm{0}}}$为开洞影响系数（中华人民共和国住房和城乡建设部，2012）。
硬化刚度K1=αK0，考虑构造柱影响时α取0.075。
2.3.2 墙段截面强度
屈服强度表示为：
$$ {V_y} = \frac{{A{\tau _{k, X}}}}{\xi }\sqrt {1 + {\sigma _0}/{\tau _{k, X}}} $$ (18) 极限强度表示为：
$$ {V_p} = {\alpha _y}{V_y}, \;\;\;{\alpha _y} = 1.11 $$ (19) 其中，${\tau _{k, X}}$为砌体沿灰缝抗剪强度（MPa）；${\sigma _0}$为首层墙段中间高度平均压应力（MPa）；A为墙段净截面面积（m2）。
3. 砌体结构地震易损性分析结果
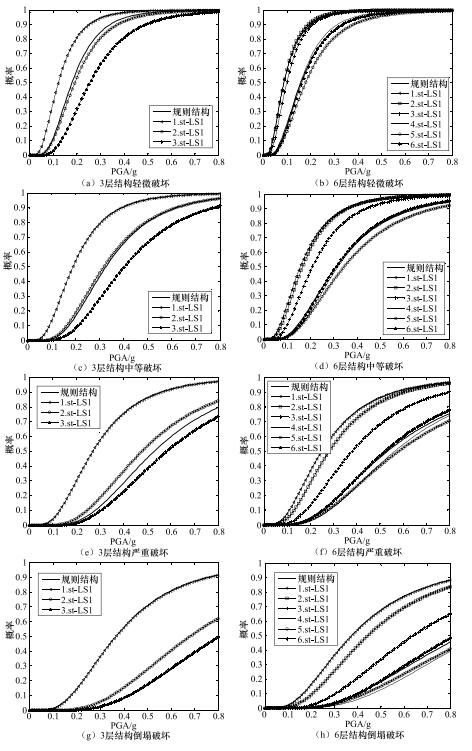
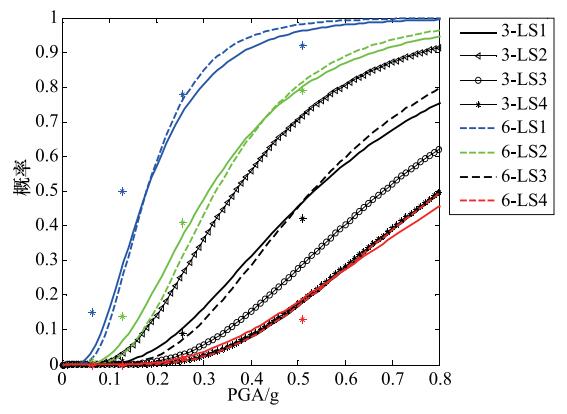
分别对3层和6层规则砌体结构进行增量动力分析，结合公式（3）得到回归系数A、B，见表 3。带入公式（8）、（9）和（10）得到易损性曲线双参数${\eta _{I{M_c}|DS}}$和${\beta _{I{M_c}|DS}}$，拟合结构易损性曲线，如图 7所示。图中LS1、LS2、LS3和LS4分别代表结构处于轻微破坏、中等破坏、严重破坏和接近倒塌4种破坏状态的超越概率曲线，6-LS1表示6层砌体结构达到轻微破坏的超越概率曲线，以此类推。
表 3 规则结构回归系数A、BTable 3. Regression coefficients A, B of regular structures砌体结构 A B $ {\beta _{{\theta _{{\rm{max|PGA}}}}}}$ 3层 -5.003 1.379 0.589 6层 -5.105 1.234 0.569 
图 7 震害资料统计结果与砌体结构基于PGA的易损性曲线对比Figure 7. Comparison of statistical data of earthquake disasters and fragility curves of masonry structure based on PGA为验证本文易损性结构的实用性，选用孙柏涛等（2014）给出的设防砖混结构的震害矩阵（表 4）与得到的规则结构易损性曲线进行对比。根据中国地震烈度（中华人民共和国国家质量监督检验检疫总局，2009）给出的烈度与PGA的对应关系，将震害矩阵与易损性曲线绘制同一图中，见图 7。
表 4 设防砖混结构震害矩阵Table 4. Earthquake damage matrix of fortified masonry structures破坏等级 设防烈度 Ⅵ Ⅶ Ⅶ Ⅸ Ⅹ 基本完好 85 50 22 8 1 轻微破坏 14 36 37 13 3 中等破坏 1 13 30 24 11 严重破坏 0 1 9 42 50 毁坏 0 0 2 13 35 从图 7中可以看出，计算得到的砌体结构易损性曲线与统计得到的震害矩阵结果基本一致，超越概率最大相差10%以内。鉴于地震烈度与PGA对应关系存在较大的不确定性，群体结构震害调查与单体结构地震易损性计算相比也有较大的不确定性，超越概率最大相差10%可以接受，故本文方法可以较为精确地计算结构的地震易损性。
3.1 楼层侧向刚度突变对砌体结构易损性的影响
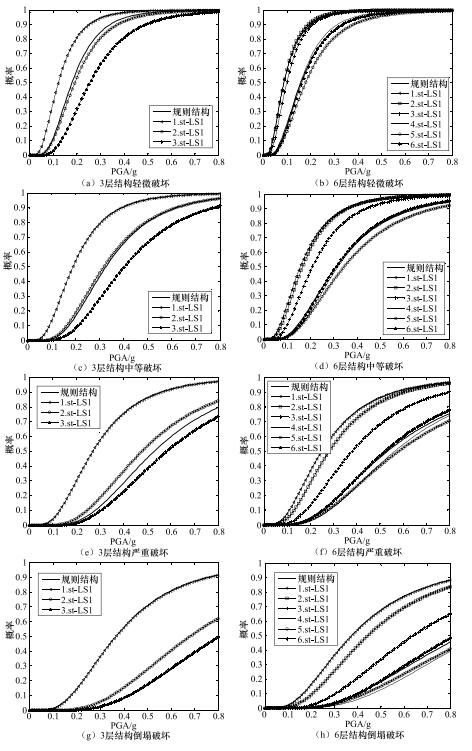
建筑结构抗震规范将结构上下层刚度比为0.5时定义为严重不规则结构。为研究竖向不同位置刚度变化对砌体结构易损性的影响，在其它层刚度保持不变的情况下，逐次将结构每层的刚度折减50%，通过人为控制刚度变化的位置，运用增量动力分析得到不同位置刚度变化时结构的动力反应，通过公式（3）对IDA结果做回归分析得到回归系数A、B，见表 5表。将回归系数代入公式（8）、（9）得到易损性曲线双参数${\eta _{I{M_c}|DS}}$和${\beta _{I{M_c}|DS}}$，拟合结构易损性曲线，如图 8所示。1.st-LS1表示第1层出现刚度突变时结构达到轻微破坏的超越概率曲线，以此类推。
表 5 回归系数A、B的值Table 5. Regression coefficients A, B结构 刚度变化位置 A B $ {\beta _{{\theta _{{\rm{max|PGA}}}}}}$ 3层 第1层 -3.419 1.877 1.011 第2层 -4.67 1.636 0.775 第3层 -4.903 1.804 0.851 6层 第1层 -3.896 1.429 0.845 第2层 -4.264 1.274 0.680 第3层 -4.799 1.116 0.544 第4层 -5.21 1.169 0.525 第5层 -5.19 1.273 0.665 第6层 -5.038 1.288 0.631 由图 8可以看出：①对于3层结构，刚度变化位于第1层时，随着地震动强度的增加结构超越不同破坏状态的概率上升幅度最大。以倒塌破坏状态为例（图 8（g）），首层与规则结构对应的倒塌破坏状态超越概率相比最大增长幅度达到41%。②对于6层结构，底部2层刚度突变对结构易损性的影响规律基本相同，都会增加整体结构的易损性。对于倒塌破坏状态而言，首层与规则结构对应的倒塌破坏状态超越概率相比最大增长幅度也超过40%，与第2层出现刚度变化对应的倒塌破坏状态超越概率相比最大增长幅度不足10%。第3层出现刚度变化与底部2层出现刚度变化相比，结构轻微破坏对应的易损性曲线基本重合；结构严重破坏和倒塌破坏易损性明显低于底部2层出现刚度变化的情况。此外，当刚度变化出现在结构第4层以上时，其对结构的易损性影响较小，结构第4、5层出现刚度突变对应的严重破坏和倒塌破坏状态易损性曲线基本重合。但是结构顶层刚度突变对应的易损性比第4、5层刚度突变时更高，这是因为顶层刚度突变造成了鞭梢效应，加重了结构顶层的破坏程度。③与规则结构相比，6层结构第4、5、6层出现刚度突变时，在给定地震动强度下破坏概率相差较小；3层结构顶层刚度变化和规则结构倒塌破坏状态曲线基本重合。说明结构顶部刚度减小对结构易损性的影响较小。
3.2 不同楼层刚度比对砌体结构易损性影响
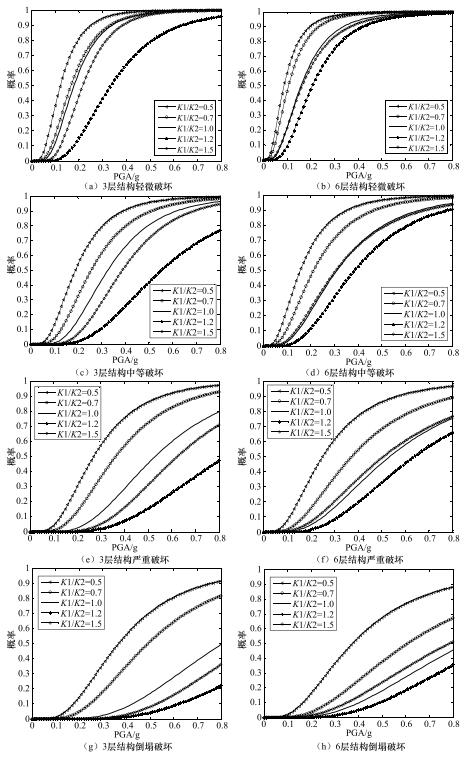
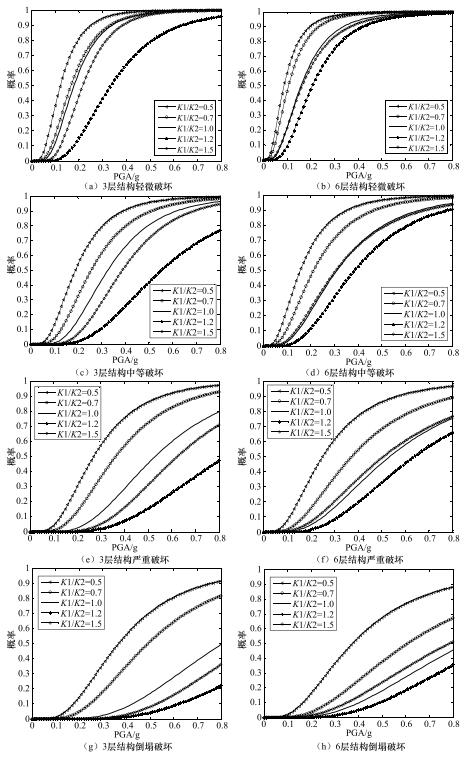
结构底部2层刚度变化对结构易损性影响较大。为了进一步研究结构刚度过渡区，即首层与相邻层（第2层）刚度比（K1/K2）的变化对结构易损性的影响，依次选择刚度比为0.5、0.7、1、1.2、1.5的3层和6层砌体结构，对其进行非线性时程分析，得到对应的易损性曲线双参数${\eta _{{\theta _{\max }}|DS}}$和${\beta _{{\theta _{\max }}|DS}}$，并拟合易损性曲线，如图 9所示。
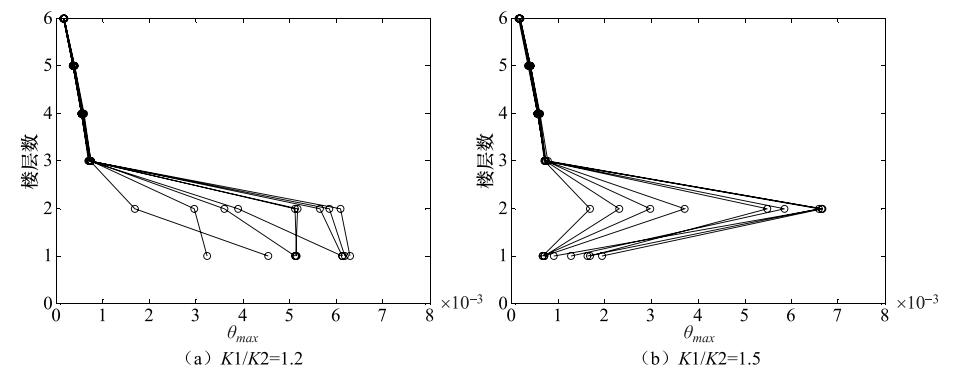
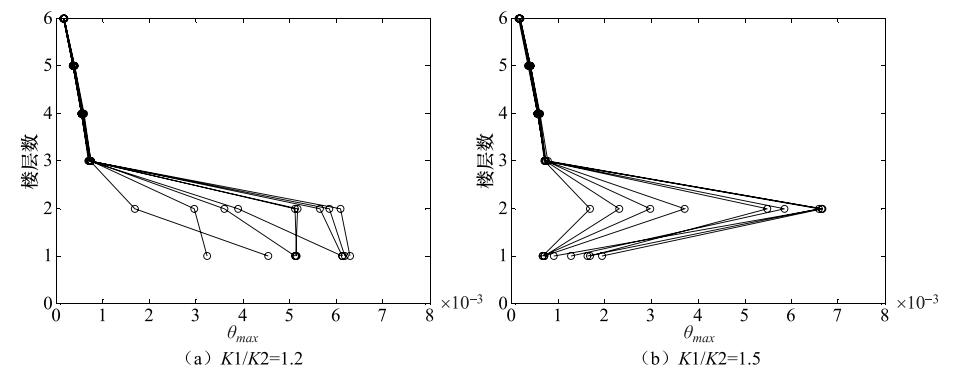
图 9 不同刚度比对应的结构易损性曲线Figure 9. Fragility curves of masonry structure corresponding to different stiffness ratios由图 9可以看出：①对于3层和6层结构，随着刚度比从0.5增加到1.2，在相同地震动强度下结构超越不同破坏状态的概率逐渐减少，结构易损性逐渐降低。②当刚度比增加到1.5时，在强地震动作用下结构出现了薄弱层的转移，结构的易损性较刚度比1.2时明显增加。以6层结构为例，当PGA调幅至0.4g时，选取其中9条地震记录对应的地震反应分析结果，得到结构刚度比为1.2和1.5时不同楼层的最大层间位移角（图 10）。刚度比为1.2时，结构底层较第2层最大层间位移角稍大，结构底层破坏较重，薄弱层位于第1层；刚度比为1.5时，结构第2层变形明显大于其它楼层，薄弱层出现在第2层。③当刚度比小于1时，刚度比增加对结构对应的倒塌破坏易损性曲线影响明显；当刚度比大于1时，刚度比变化对倒塌破坏状态易损性曲线的影响较小。以图 9（h）为例，刚度比从0.5增加到0.7倒塌破坏状态易损性曲线幅度最大下降约20%，从0.7增加到1时，其下降幅度最大超过20%，当刚度比增大至1.2时，其下降幅度只有10%左右。这表明结构底层刚度不宜小于相邻层刚度，结构刚度宜从下到上由大到小均匀变化。
图 10 PGA输入为0.4g时各楼层最大层间位移角Figure 10. Maximum interstory drift with the input of PGA is 0.4g4. 结论
本文以3层和6层典型砌体结构为例，采用层间剪切模型，借助增量动力法及回归拟合开展了基于峰值加速度的易损性分析，建立了结构地震易损性曲线。通过改变楼层的侧向刚度值模拟薄弱层，定量分析了楼层刚度变化对结构不同破坏状态超越概率的影响。通过改变底层与二层的侧向刚度比，定量研究了底部刚度突变对结构不同破坏状态超越概率的影响。研究得到以下几点认识：
（1）结构底层出现刚度突变对结构的易损性影响最为显著。与规则结构相比，首层刚度突变会明显增加结构的易损性。以倒塌破坏状态为例，3层和6层结构底层刚度突变都可导致其超越概率增加40%左右。结构上部出现刚度突变对结构易损性的影响不明显。对于6层结构，顶层出现刚度变化时容易出现鞭梢效应，会一定程度加重结构顶层破坏。
（2）在一定范围内，随着结构底层与二层侧向刚度比的增大（K1/K2从0.5增加至1.2），结构的易损性降低。但是侧向刚度比过大（K1/K2=1.5），会造成薄弱层位置的转移，结构易损性增加。当底层与二层侧向刚度比从0.7增加到1时，结构倒塌易损性的降低幅度最多超过20%。当底层与二层侧向刚度比从1.0增大至1.2时，结构倒塌易损性下降幅度只有10%左右。通过合理控制侧向刚度比值，可以一定程度上降低结构的易损性，结构刚度宜由大到小、从下到上均匀变化。
-
图 1 挑选的地震动的加速度反应谱
Figure 1. The acceleration response spectrum of selected ground motion
图 3 砌体结构动力反应值与地震参数对数拟合结果
Figure 3. Logarithmic fitting results of dynamic response and seismic parameters of masonry structure
图 7 震害资料统计结果与砌体结构基于PGA的易损性曲线对比
Figure 7. Comparison of statistical data of earthquake disasters and fragility curves of masonry structure based on PGA
图 9 不同刚度比对应的结构易损性曲线
Figure 9. Fragility curves of masonry structure corresponding to different stiffness ratios
图 10 PGA输入为0.4g时各楼层最大层间位移角
Figure 10. Maximum interstory drift with the input of PGA is 0.4g
表 1 选择的地震动记录
Table 1. Selected records of ground motion
地点 台站 年份 Mw 断层距/km VS30/m·s-1 美国，圣费尔南多 Buena Vista–Taft 1971 6.61 111.37 386 伊朗，曼吉尔 Abhar 1990 7.37 75.58 303 美国，旧金山 El Centro Imp. Co. Cent 1987 6.54 18.2 292 美国，旧金山 Poe Road (temp) 1987 6.54 11.16 317 土耳其，迪兹杰 Bolu 1999 7.14 12.02 294 美国，兰德斯 Coolwater 1992 7.28 19.74 353 美国，兰德斯 Desert Hot Springs 1992 7.28 21.78 359 中国台湾，集集 HWA033 1999 7.62 39.55 379 中国台湾，集集 CHY046 1999 7.62 24.1 442 美国，阿拉斯加德纳利 Carlo (temp) 2002 7.9 49.94 399 美国，摩根山丘 Gilroy Array #3 1984 6.19 13.01 350 美国，赫克托矿 Hesperia - 4th & Palm 1999 7.13 89.87 358 意大利，伊尔皮纳 Calitri 1980 6.2 8.81 456 土耳其，科贾埃利 Goynuk 1999 7.51 31.74 348 土耳其，科贾埃利 Iznik 1999 7.51 30.73 477 美国，洛马普雷塔 Anderson Dam (L Abut) 1989 6.93 19.9 489 日本，阪神 Kakogawa 1995 6.9 22.5 312 美国，帝国谷 Cerro Prieto 1979 6.53 15.19 472 美国，帝国谷 Delta 1979 6.53 22.03 242 美国，帝国谷 El Centro Array #9 1940 6.95 6.09 213 日本，阪神 Shin-Osaka 1995 6.9 19.15 256 
下载: 导出CSV
表 2 层间位移角限值
Table 2. Allowable value of story drift ratios
破坏状态 层间位移角均值（${\mu _{{\theta _{\max }}|DS}}$） 变异系数（${\delta _{{\theta _{\max }}|DS}}$） 层间位移角中值（${\eta _{{\theta _{\max }}|DS}}$） 对数标准差（${\beta _{{\theta _{\max }}|DS}}$） 轻微破坏（LS1） 1/1600（0.000625） 0.35 0.00059 0.34 中等破坏（LS2） 1/700（0.00143） 0.35 0.00135 0.34 严重破坏（LS3） 1/350（0.0028） 0.35 0.00264 0.34 接近倒塌（LS4） 1/200（0.005） 0.35 0.0047 0.34
下载: 导出CSV
表 3 规则结构回归系数A、B
Table 3. Regression coefficients A, B of regular structures
砌体结构 A B $ {\beta _{{\theta _{{\rm{max|PGA}}}}}}$ 3层 -5.003 1.379 0.589 6层 -5.105 1.234 0.569
下载: 导出CSV
表 4 设防砖混结构震害矩阵
Table 4. Earthquake damage matrix of fortified masonry structures
破坏等级 设防烈度 Ⅵ Ⅶ Ⅶ Ⅸ Ⅹ 基本完好 85 50 22 8 1 轻微破坏 14 36 37 13 3 中等破坏 1 13 30 24 11 严重破坏 0 1 9 42 50 毁坏 0 0 2 13 35
下载: 导出CSV
表 5 回归系数A、B的值
Table 5. Regression coefficients A, B
结构 刚度变化位置 A B $ {\beta _{{\theta _{{\rm{max|PGA}}}}}}$ 3层 第1层 -3.419 1.877 1.011 第2层 -4.67 1.636 0.775 第3层 -4.903 1.804 0.851 6层 第1层 -3.896 1.429 0.845 第2层 -4.264 1.274 0.680 第3层 -4.799 1.116 0.544 第4层 -5.21 1.169 0.525 第5层 -5.19 1.273 0.665 第6层 -5.038 1.288 0.631
下载: 导出CSV
-
高小旺, 钟益村, 1990.底层全框架砖房震害预测方法.建筑科学, (2):47-53. http://d.old.wanfangdata.com.cn/Conference/188058 李小军, 于爱勤, 甘朋霞等, 2008.汶川8.0级地震北川县城区灾害调查与分析.震灾防御技术, 3(4):352-362. doi: 10.3969/j.issn.1673-5722.2008.04.004 林旭川, 陆新征, 叶列平, 2008.砌体结构的地震倒塌模拟与分析.见: 2008年汶川地震建筑震害分析与重建研讨会论文集.北京: 中国土木工程学会, 中国建筑学会, 中国工程院. 刘红彪, 郭迅, 2012.极震区典型砌体结构的振动台倒塌对比试验分析.土木工程学报, 45(12):18-28. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QKV20122013041200077994 刘锡荟, 张鸿熙, 刘经伟等, 1981.用钢筋混凝土构造柱加强砖房抗震性能的研究.建筑结构学报, 2(6):47-55. doi: 10.1016-j.hrthm.2009.06.009/ 刘砚山, 童丽萍, 2013.抗侧刚度比对底框砌体房屋抗震性能的影响.工程抗震与加固改造, 35(4):15-21, 31. doi: 10.3969/j.issn.1002-8412.2013.04.003 孙柏涛, 陈洪富, 闫培雷等, 2014.南北地震带房屋建筑抗震能力分区特征研究——以四川省为例.土木工程学报, 47(S1):6-10. http://d.old.wanfangdata.com.cn/Conference/8608721 孙柏涛, 邓宏宇, 2015.底框结构房屋抗震性能研究.建筑结构学报, 36(S2):131-137. http://d.old.wanfangdata.com.cn/Periodical/jzjgxb2015z2020 孙柏涛, 梅云弟, 2012.竖向变刚度约束砌体结构抗震能力研究.地震工程与工程振动, 32(2):1-13. http://d.old.wanfangdata.com.cn/Periodical/dzgcygczd201202001 孙雷, 刘福胜, 王少杰等, 2013.短周期村镇砌体建筑振动特性实测与抗震性能分析.土木工程学报, 46(S2):57-62. http://d.old.wanfangdata.com.cn/Conference/7986632 温增平, 徐超, 陆鸣等, 2009.汶川地震重灾区典型钢筋混凝土框架结构震害现象.北京工业大学学报, 35(6):753-760. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=SciencePaper201303040000222922 夏敬谦, 丁世文, 周四骏等, 1988.水平荷载下砖墙恢复力特性及能量耗损特性的研究.世界地震工程, (2):5-14. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000002726787 熊立红, 夏锦江, 2003.新型混凝土空心砌块房屋抗震性能研究.地震工程与工程振动, 23(3):132-137. doi: 10.3969/j.issn.1000-1301.2003.03.021 熊立红, 2004.多层混凝土砌块结构性态抗震研究.北京: 中国地震局工程力学研究所. http://cdmd.cnki.com.cn/Article/CDMD-85406-2005127949.htm 徐超, 陈波, 李小军等, 2013.芦山MS7.0地震建筑结构震害特征.地震学报, 35(5):749-758. doi: 10.3969/j.issn.0253-3782.2013.05.013 杨玉成, 杨柳, 高云学等, 1982.现有多层砖房震害预测的方法及其可靠度.地震工程与工程振动, 2(3):75-86. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000000199313 张令心, 江近仁, 刘洁平, 2002.多层住宅砖房的地震易损性分析.地震工程与工程振动, 22(1):49-55. doi: 10.3969/j.issn.1000-1301.2002.01.009 中华人民共和国国家质量监督检验检疫总局, 中国国家标准化管理委员会, 2009.中国地震烈度表(GB/T 17742-2008).北京: 中国标准出版社. 中华人民共和国国家质量监督检验检疫总局, 中国国家标准化管理委员会, 2009.建(构)筑物地震破坏等级划分(GB/T 24335-2009).北京: 中国标准出版社. 中华人民共和国建设部, 1994.设置钢筋混凝土构造柱多层砖房抗震技术规程(JGJT13-1994).北京: 中国建筑工业出版社. 中华人民共和国住房和城乡建设部, 2012.砌体结构设计规范(GB 50003-2011).北京: 中国计划出版社. 周强, 孙柏涛, 2010.汶川地震中极震区砌体结构教学楼典型震害分析.震灾防御技术, 5(4):467-476. doi: 10.3969/j.issn.1673-5722.2010.04.009 庄一舟, 谢狄敏, 1999.砌体结构抗震可靠度分析方法.工程力学, (S1):920-925. http://d.old.wanfangdata.com.cn/Conference/75229 Shinozuka M., Feng M. Q., Lee J., et al., 2000. Statistical analysis of fragility curves. Journal of Engineering Mechanics, 126 (12):1224-1231. doi: 10.1061/(ASCE)0733-9399(2000)126:12(1224) Shome N., 1999. Probabilistic seismic demand analysis of nonlinear structures. California: Stanford University. Singhal A., Kiremidjian A. S., 1996. Method for probabilistic evaluation of seismic structural damage. Journal of Structural Engineering, 122 (12):1459-1467. doi: 10.1061/(ASCE)0733-9445(1996)122:12(1459) Vamvatsikos D., Cornell C. A., 2002. Incremental dynamic analysis. Earthquake Engineering & Structural Dynamics, 31 (3):491-514. http://d.old.wanfangdata.com.cn/Periodical/tjdxxb201002006 Xiong C., Lu X. Z., Lin X. C., et al., 2016. Parameter determination and damage assessment for THA-based regional seismic damage prediction of multi-story buildings. Journal of Earthquake Engineering, 21(3):461-485. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=10.1080/13632469.2016.1160009 期刊类型引用(3)
1. 闫凯，魏瑞祥，任鹏飞，张倩，刘政，赵考重. 钢筋混凝土加强墙的底层大开洞砌体结构抗震性能试验研究. 防灾减灾工程学报. 2024(06): 1336-1347+1367 . 
百度学术2. 郭猛，李超，徐福泉. 多层砌体结构底部加强层概念与抗震设计理论研究. 自然灾害学报. 2022(06): 27-41 . 百度学术3. 梁志强，徐超，温增平. 考虑填充墙影响的钢筋混凝土厂房结构地震易损性分析. 震灾防御技术. 2019(04): 707-718 . 本站查看其他类型引用(7)
-
下载:
点击查看大图
计量
- 文章访问数: 115
- HTML全文浏览量: 28
- PDF下载量: 2
- 被引次数: 10
