Stochastic Finite Fault Method and Its Engineering Applications
-
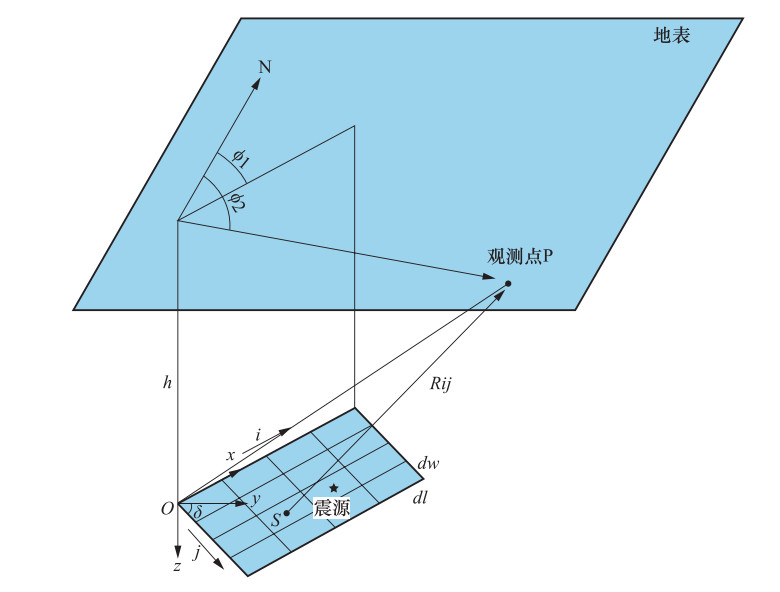
摘要: 随机有限断层法作为半经验半理论的地震动合成方法,综合考虑了震源、传播路径以及场地条件对地震动的影响,可在工程关注的频率范围内模拟地震动时程,为实现较大区域地震动模拟提供了一种有效的方法。本文系统阐述了随机有限断层法的基本原理、静力学拐角频率模型及动拐角频率模型的发展;分析了主要模型参数(断层尺度、破裂速度、剪切波速及应力降)、Kappa因子和介质品质因子的取值原则,并结合中国大陆的实际情况给出了相应的取值范围;最后讨论了现有模拟方法在考虑参数取值、子断层划分和子断层之间相互作用等方面的不足以及能量处理方式上的缺陷,明确了随机有限断层法发展的方向。Abstract: As a semi-empirical and semi-theoretical synthetic method of ground motion, stochastic finite fault method can comprehensively consider the impact of source, propagation path and site conditions, and can effectively simulate the seismic time history within the frequency range of engineering interest. It provides an effective means for the ground motion simulation in a large region. In this paper, the basic principle of stochastic finite fault method and the development of static corner frequency model and dynamic corner frequency model are firstly systematically expounded. Principles of the values of the major source parameters (fault size, rupture velocity, shear wave velocity, stress drop), kappa factor and Q value are analyzed. According to the actual situation in China, the corresponding range of values are provied. Finally, the shortcomings of present existing simulation methods in considering the parameter value, the interaction among sub-faults, energy treatment methods and fault division have been discussed. The development direction of stochastic finite fault method are somehow clarified.
-
Key words:
- Ground motion simulation /
- Stochastic finite fault method /
- Stress drop /
- Kappa factor /
- Quality factor
-
引言
地震小区划是对特定区域范围内可能遭遇到的地震影响进行划分,包括设计地震动参数小区划和地震地质灾害小区划。相比地震区划,地震小区划工作更重视局部场地条件的影响,更为详细地考虑周围的地震地质环境,其成果可为抗震设计、土地利用规划、震害防御等提供更精确的资料(胡聿贤,1999)。
地震小区划工作内容涉及地震活动性评价、地震构造评价、场地地震动分析等,工作量大,获取的数据也很丰富。传统地震小区划成果通常以文字报告、专题图件的形式提交,类型较单一,展示度较差,难以满足目前公共服务的需要。管理现有数据并充分利用数据推出更多服务型产品是目前包括地震小区划工作在内的地震工作信息化和现代化迫切需要。
随着GIS技术的发展,国内很多研究者逐步将GIS技术引入地震小区划研究工作中(张苏平等,2003;王庆满等,2011;李程程等,2014),建立有关数据库和数据管理系统(田勤虎等,2011;龚磊等,2015)。但已建系统多以数据查询、展示为主,缺少分析功能和实际产出服务。本文以嘉兴科技城地震小区划工作为基础,将基础数据与地理信息系统(GIS)相结合,形成集查询、分析于一体的地震小区划成果数字化系统,可为不同用户提供不同数据服务。
1. 研究区概况
研究区位于嘉兴科技城,行政区划属于嘉兴市南湖区,面积约30km2,是浙江省四大科技平台之一。现聚集了包括浙江清华长三角研究院、浙江中科院应用技术研究院在内的一批高端院所,还建有多个国际技术合作平台、工程中心和产学研基地,是嘉兴市乃至浙江省重要的创业创新平台。
查询《中国地震动参数区划图》(GB 18306—2015)(中华人民共和国国家质量监督检验检疫总局等,2015)可知,嘉兴市大部分地区由原来的Ⅵ度设防提升为Ⅶ度设防,设防水准的提高将对区内建设工程抗震设防、国土利用规划、社会经济发展等提出更高的要求。嘉兴科技城现处于Ⅶ度设防区内,加之人才产业聚集、产值高,地震灾害风险大。在该区进行地震小区划工作,可为该区提供更精确的抗震设防和土地规划资料,有效降低地震灾害风险。
2. 基础数据
根据相关规范要求,地震小区划工作内容包括地震地质及地震活动性资料的收集、场地钻探、场地土层反应分析等,涉及地质、地震、地球物理、工程勘察等多方面的数据,根据系统建设的需要归纳如下:
(1) 地震地质及地震活动性数据
研究区内地震地质及地震活动性专题图包括区域地震分布图、场地地震分布图、历史地震等震线分布图、区域构造图、区域断层分布图、近场构造图等,均为Mapinfo格式的矢量文件。
(2) 场地地震工程地质条件勘察数据
区划范围内布设60个钻孔,其中标准钻孔1个(孔深232.8m),地震钻孔29个(深度不小于105m),勘察孔30个(深度小于30m),钻探总进尺4204m,浅层人工地震探测测线共21534m。系统建设收集的数据包括60幅钻孔柱状图、18条工程地质剖面、5幅浅层人工地震探测解译图,其中钻孔资料均以柱状图的形式提供,格式为AUTO CAD矢量文件,钻孔的其他信息以Excel表格的形式提供;工程地质剖面图和地震探测测线数据为PDF文档和栅格图片;勘察报告以PDF文档的形式提供。
场地内60个钻孔均进行了波速测试,钻孔代表性土层动三轴土样80件,收集的波速数据和动三轴数据以Excel表格的形式提供。
(3) 地震动分析与参数区划数据
根据土层地震动分析的需要,建立29个场地钻孔模型,数据格式为TXT文本。收集全球范围内天然地震记录1692条,人工合成地震波522条(每个钻孔3个超越概率各6条),以TXT文本的形式提供。
地震小区划成果图件主要为地震动参数区化图,以Mapinfo矢量文件的形式提供。另外,还包括小区划报告的文本,以PDF文档的形式提供。
3. 系统设计
3.1 面向对象实现
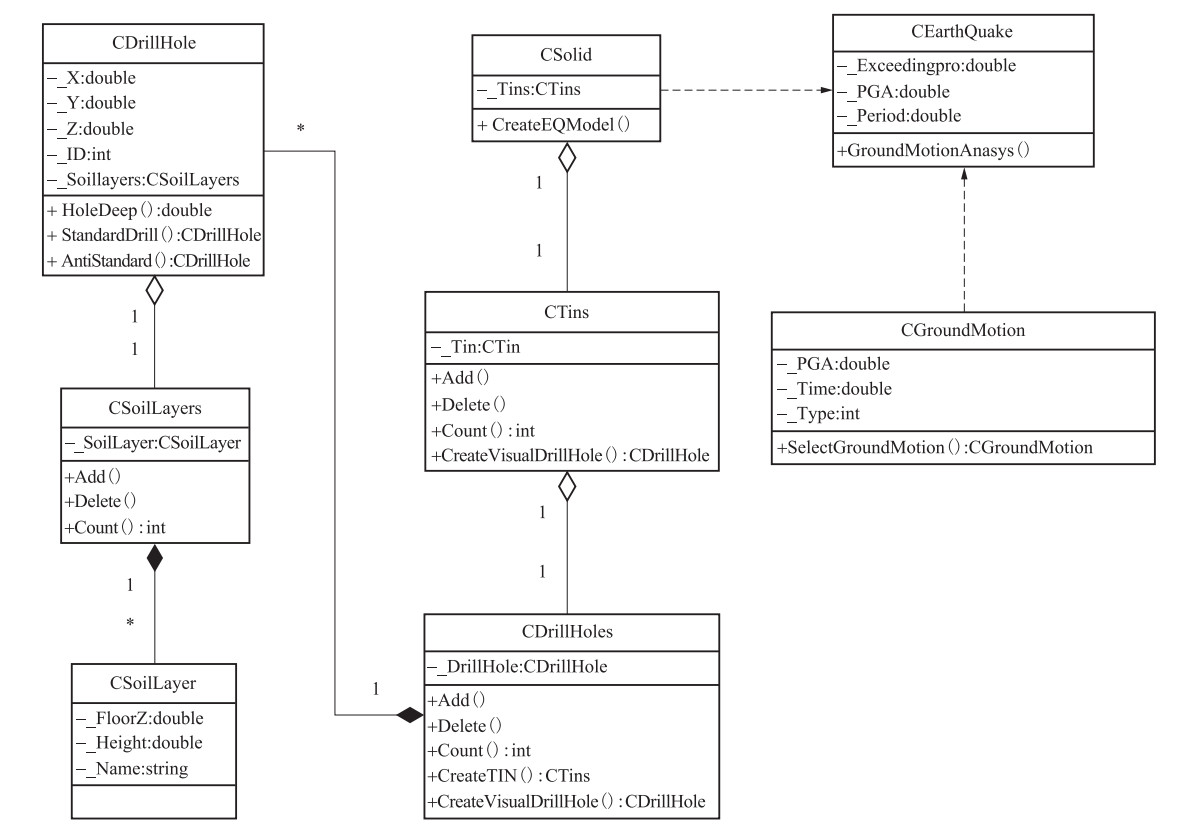
地震小区划涉及钻孔、场地、强震记录、地震灾害等概念,其对象层次清晰,易于以面向对象的方式实现,形成地震小区划数字化系统。根据需求分析,首先将地震小区划数字化涉及的问题抽象成类,并建立类之间的消息机制及类之间的关系,即进行地震小区划数字化概念设计。
根据概念模型抽象出类,相应的类关系如图 1所示,地震小区划数字化主要类有CSolid(工程地质三维实体类)、CDrillHoles(钻孔集合类)、CSoilLayers(土层集合类)、CGroundMotion(强震动观测记录类)、CEarthQuake(场地地震动类)等。CDrillHoles类由CDrillHole类组合形成,并通过CreateTIN方法实现CTins类,由CTins类实现CSolid类,利用CGroundMotion类中SelectEarthQuake方法筛选出符合地质条件的历史强震记录,导入CSolid类,进行地震动分析,计算场地地震动参数峰值加速度、特征周期,并绘制峰值加速度等值线图和特征周期等值线图,由此生成场地地震小区划成果。
3.2 数据库设计
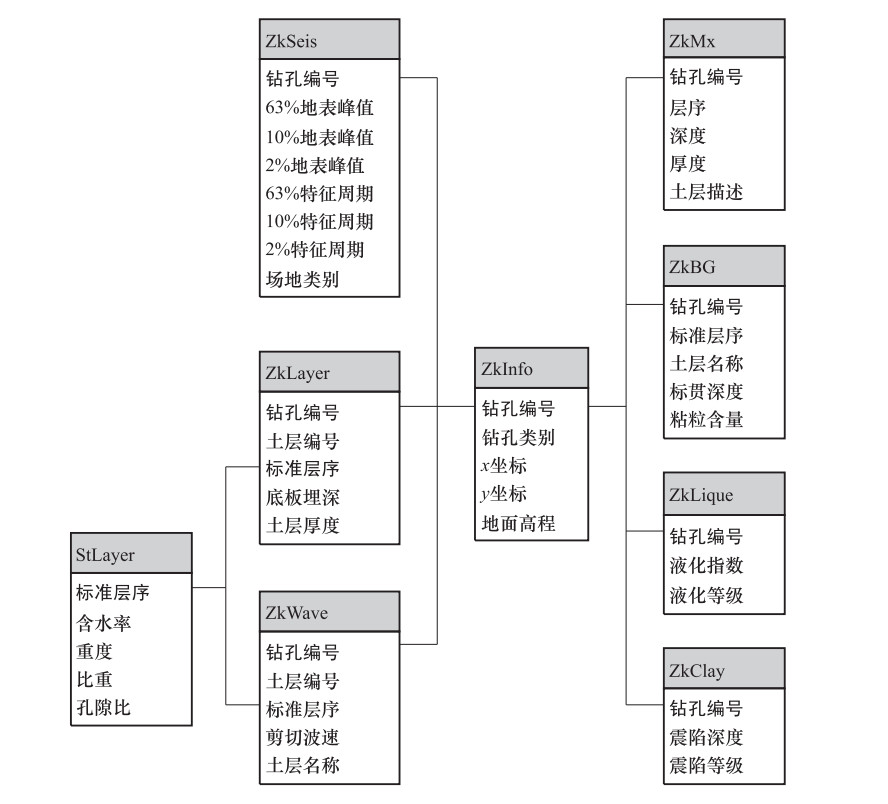
系统采用Access小型数据库实现场地基础地理信息数据、地震动数据、地震震陷数据、地震液化数据等的建库,结合GIS技术实现空间数据与属性数据的整合、矢量数据与栅格数据的整合、信息数据与分析数据的整合。数据库共包含9张数据表,分类如表 1所示。
表 1 数据库汇总Table 1. Database summary序号 表名 功能说明 1 ZkInfo 钻孔基本信息 2 ZkLayer 钻孔土层信息 3 StLayer 标准层序土层信息 4 ZkLique 钻孔砂土液化信息 5 ZkClay 钻孔软土震陷信息 6 ZkWave 钻孔土层剪切波速信息 7 ZkBG 钻孔土层标贯信息 8 ZkMx 钻孔动力分析模型信息 9 ZkSeis 钻孔动参数区划信息表 数据库逻辑设计如图 2所示。
3.3 系统物理设计
系统采用MapX作为GIS开发组件,数据库采用Access,在VB环境下完成开发,可运行于多个版本的windows操作系统中。
4. 系统功能实现
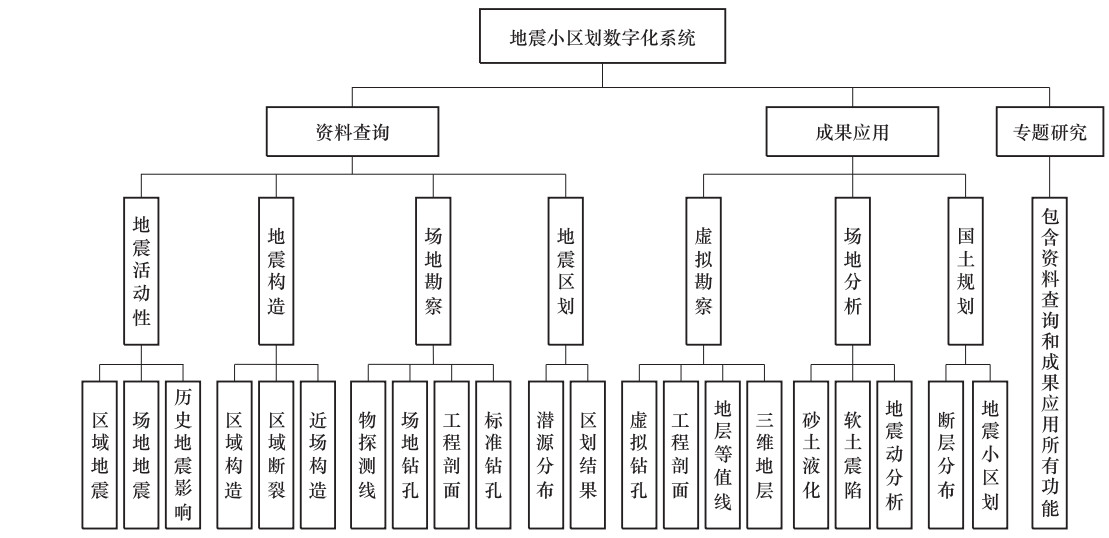
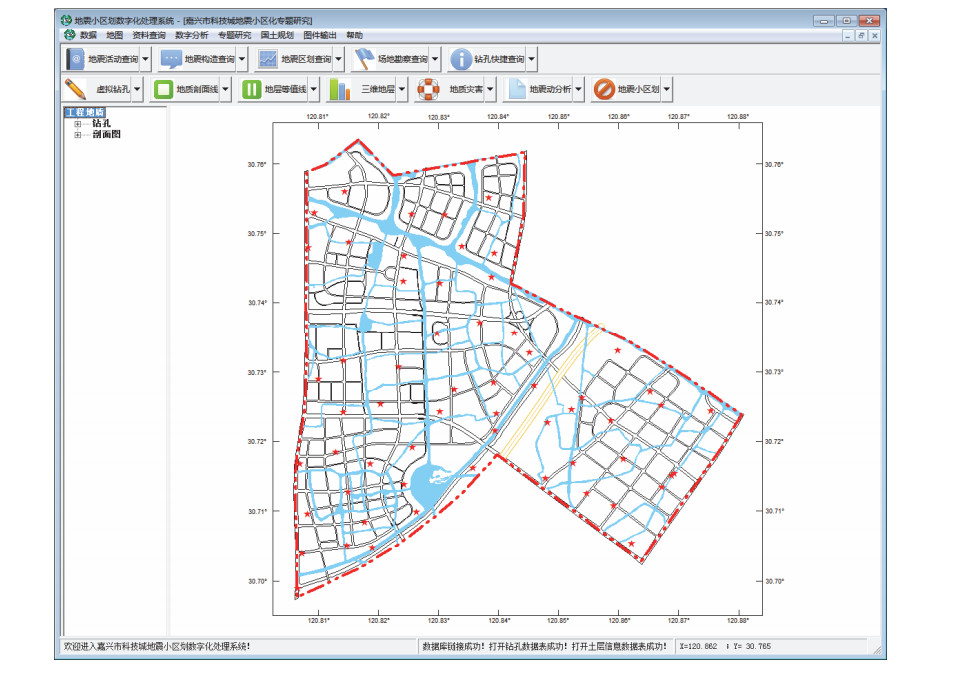
系统功能分为资料查询、成果应用、专题研究三大模块,其中专题研究整合了资料查询、成果应用模块的所有功能,详细的功能设计如图 3所示,系统主界面(专题研究模块)如图 4所示,主要对资料查询、成果应用模块进行介绍。
4.1 资料查询模块
该模块主要对地震小区划工作的基础资料及成果数据进行可视化展示,内容包括地震活动性、地震构造、场地勘察、地震区划等。由于部分功能展示的形式类似,对其中的部分功能进行介绍。
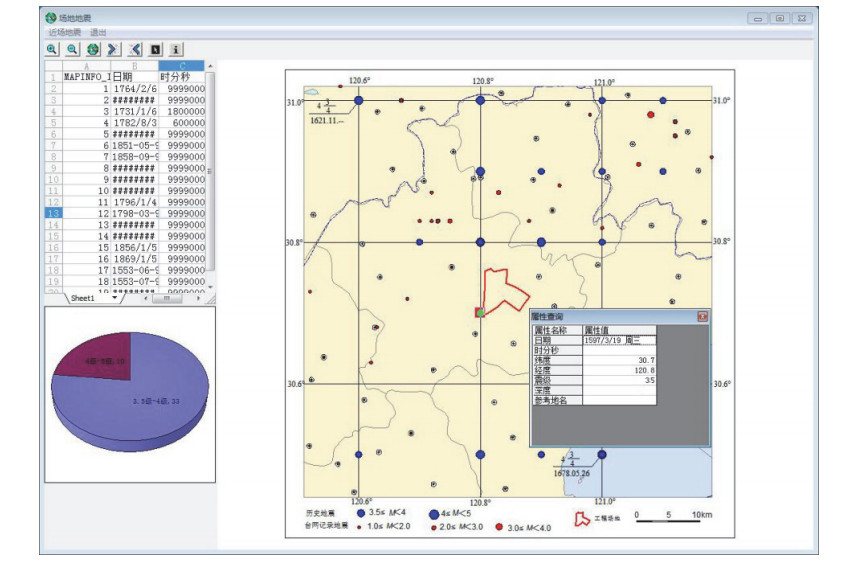
(1) 地震活动性查询:可查询区域地震、场地地震、历史地震影响烈度及场地地震综合评价。按地震震级自动统计地震活动性情况,通过地点地图及数据表的方式查询当前地震发生日期、经度、纬度、震级、震源深度等相关信息,成果展示如图 5所示。
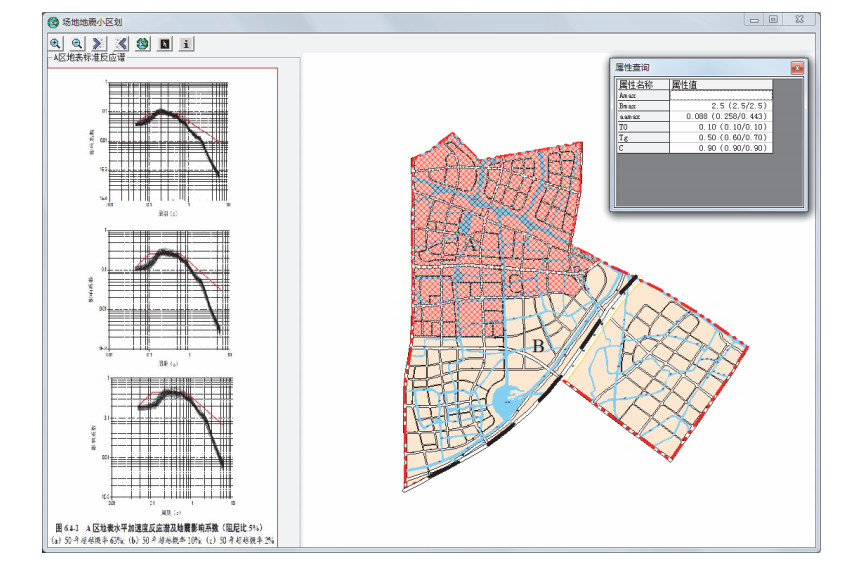
(2) 地震区划:对区内潜在震源区的划分情况及地震小区划成果进行查询,成果以可视化的形式展示,包括区划的范围、相关的地震动参数及标定的反应谱,成果展示如图 6所示。
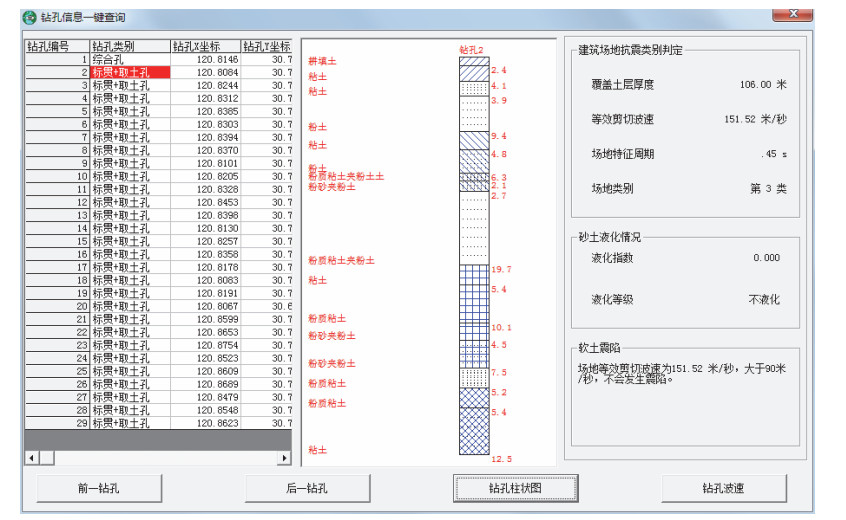
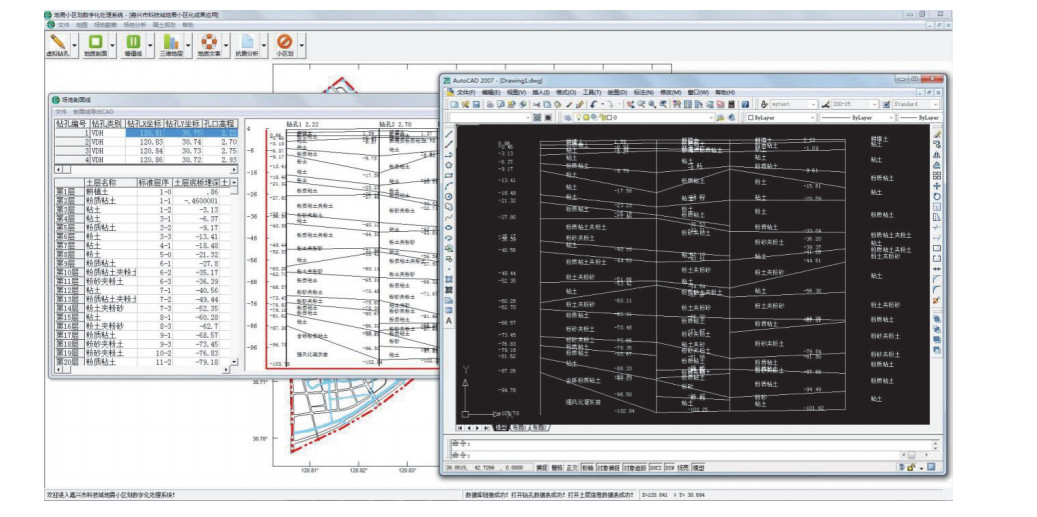
(3) 钻孔快捷查询:可对区内所有勘察钻孔进行集中展示,包括钻孔柱状图、钻孔土层剪切波速、抗震类别判定、砂土液化和软土震陷情况判断等所有信息,成果展示如图 7所示。
4.2 成果应用
该模块基于现有基础资料,通过建立分析模型对数据进行数字化分析,生成相应的成果数据。该模块主要包括虚拟勘察、场地分析及国土规划3个子模块,其中虚拟勘察模块包括虚拟钻孔、工程地质剖面、地层等值线等,场地分析模块包括砂土液化、软土震陷分析及场地抗震类别判定,国土规划模块包括断层分布及地震小区划。
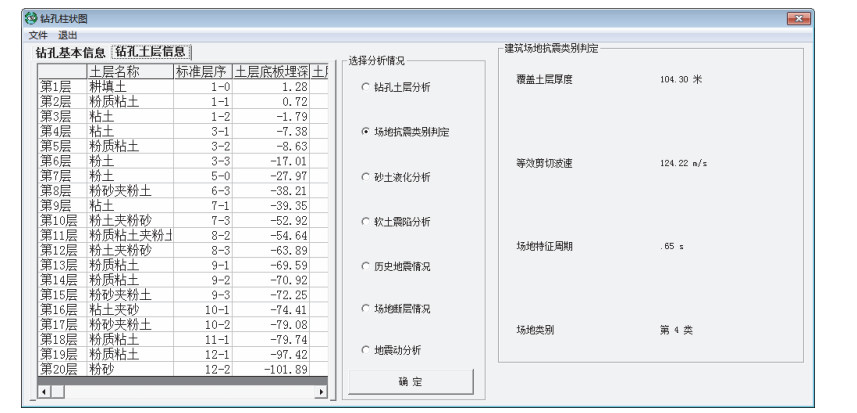
(1) 虚拟钻孔:采用delaunay三角网上的线性内插算法,根据坐标输入或地图点选获得示范区内任意位置钻孔(虚拟)的相关资料,包括钻孔柱状图、场地抗震类别判定、砂土液化和软土震陷分析、历史地震、地震动参数等。虚拟钻孔的柱状图以栅格图片或CAD矢量文件的形式导出,所有虚拟钻孔信息还可以Word文档的形式导出,内容包括场地土层信息、场地地震动参数、场地类别等,涵盖了地震工程大部分基础资料,可供相关单位参考使用,如图 8所示。
(2) 工程地质剖面:根据连续的坐标输入或在底图上绘制剖面线生成研究区内相应的工程地质剖面图(见图 9),可将剖面图以栅格图片或CAD矢量文件的形式导出。
(3) 地震地质灾害:包括场地内的砂土液化和软土震陷。根据部分钻孔试验参数,采用建规中的判别方法对场地内钻孔进行判定,给出其液化程度和震陷情况。
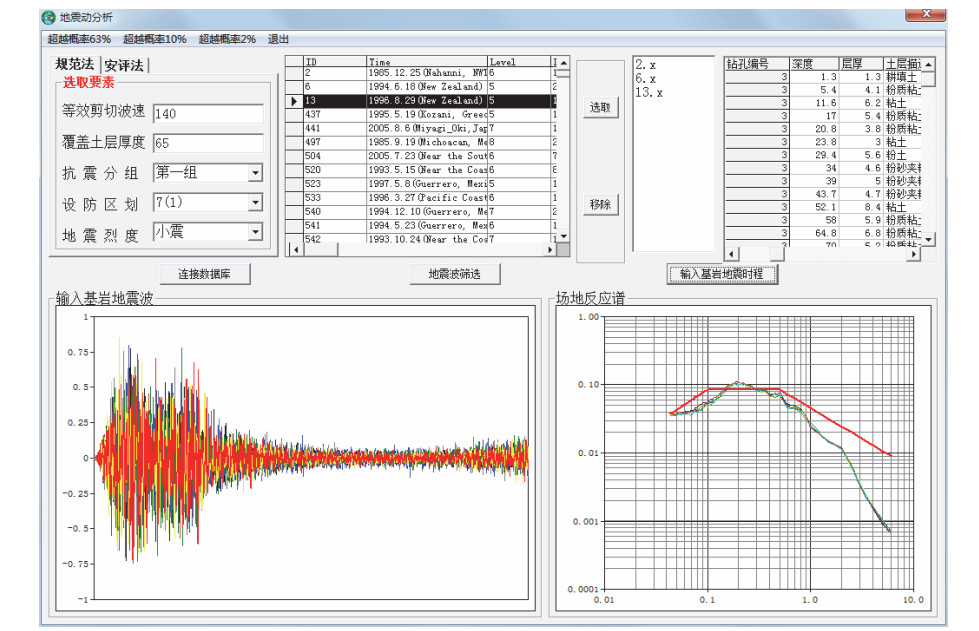
(4) 地震动分析:根据点选的坐标位置,选择距离最近的地震钻孔数据,输入筛选要素,从天然地震时程库中选取符合要求的天然地震时程;根据人工合成的基岩地震动计算得到各超越概率下的场地地表地震动参数,成果展示如图 10所示。
5. 结论与建议
通过对地震小区划数据的整理,基于GIS软件开发地震小区划成果数字化系统。在实现项目成果数据查询与可视化展示的同时,还可导出数字化分析成果。相比传统的地震小区划工作,该系统的成果更丰富,形式更多样,为地震工作服务能力的提升进行了尝试。同时,需指出的是,此次建立的系统仅为单机版,与实现公共服务仍存在一定差距,后续还需进行更多的研究,补充和完善相关功能,以期建立高效便捷的在线服务系统。
-
表 1 断层尺度与矩震级的统计关系
Table 1. Statistical relationship between the fault scale and moment magnitude
断层类型 断层长度/km 断层面积/km2 走滑断层 $ \lg L=-2.57+0.62{{M}_\text{W}} $ $ \lg S\text{=}-3.42+0.90{{M}_\text{W}} $ 正断层 $ \lg L\text{=}-1.88+0.50{{M}_\text{W}} $ $ \lg S\text{=}-2.87+0.82{{M}_\text{W}} $ 逆断层 $ \lg L\text{=}-2.42+0.58{{M}_\text{W}} $ $ \lg S\text{=}-3.99+0.98{{M}_\text{W}} $ 所有断层类型 $\lg L\text{=}-2.44+0.59{{M}_\text{W}} $ $ \lg S\text{=}-3.49+0.91{{M}_\text{W}} $ 表 2 中国大陆Q0值范围
Table 2. The range of Q0 value in Chinese mainland
区域 Q0 n 青藏高原褶皱区 200—250 0.5—0.7 松潘甘孜褶皱区 250—300 滨太平洋褶皱区 275—300 0.5—0.7 昆仑-秦岭褶皱区 275—325 塔里木地台 350—425 0.3—0.5 扬子块体 325—400 0.45—0.55 中朝块体 325—400 0.3—0.5 华南褶皱系(西高东低) 275—400 0.45—0.55 天山-兴安褶皱区 300—425 0.3—0.5 西伯利亚地台南端 450—500 0.45—0.7 注:青藏高原褶皱区包括冈底斯念-青唐古拉褶皱系、喀拉昆仑-唐古拉褶皱系和三江褶皱系;滨太平洋褶皱区包括东南沿海褶皱区、延边褶皱区和那丹哈达优地槽褶皱带;昆仑-秦岭褶皱区包括东昆仑褶皱系、秦岭褶皱系以及祁连褶皱系。 表 3 云南地区Q值公式
Table 3. The formula of calculating Q value in Yunnan Province
区域 Q 云南地区 $ Q\text{=}238{{f}^{0.338}} $ 云南东部地区 $Q\text{=}199.6{{f}^{0.434}} $ 云南西部地区 $ Q\text{=}281{{f}^{0.349}} $ 滇西地区 $ Q\text{=}102.6{{f}^{0.687}} $ 滇中地区 $ Q\text{=}92.7{{f}^{0.553}} $ -
常莹, 2011.基于断层破裂过程分解法模拟近断层宽频地震动.北京:中国地震局地球物理研究所. 陈晓非, 2009.浅源大地震超剪切破裂机理研究.见: 中国地球物理·2009.合肥: 中国地球物理学会. 丛连理, 胡家富, 傅竹武等, 2002.中国大陆及邻近地区Lg尾波的Q值分布.中国科学(D辑), 32(8):617-624. http://d.old.wanfangdata.com.cn/Periodical/zgkx-cd200208001 高阳, 潘华, 汪素云, 2014.中强地震随机有限断层模型应力降参数的确定方法.震灾防御技术, 9(4):733-747. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20140401&journal_id=zzfyjs 高阳, 2016.随机有限断层法模拟中强地震近场地震动的研究及应用.国际地震动态, (1):43-44. http://www.cnki.com.cn/Article/CJFDTOTAL-GJZT201601012.htm 郭明珠, 贾连军, 铁瑞等, 2011.剪切波速测试方法的现状分析.西北地震学报, 33(S1):21-23. http://d.old.wanfangdata.com.cn/Periodical/xbdzxb2011z1005 胡家富, 丛连理, 苏有锦等, 2003.云南及周边地区Lg尾波Q值的分布特征.地球物理学报, 46(6):809-813. doi: 10.3321/j.issn:0001-5733.2003.06.013 李英民, 赖明, 白绍良, 2002.从结构抗震设计理论看地震动输入.工程力学, (S1):549-554. http://d.old.wanfangdata.com.cn/Conference/4110927 笠原太一, 1984.地震力学.赵仲和, 译.北京: 地震出版社. 梁俊伟, 2015.基于能量的随机有限断层法研究.南昌: 南昌航空大学. http://www.cnki.com.cn/Article/CJFDTotal-NCHK201502002.htm 刘建华, 刘福田, 阎晓蔚等, 2004.华北地区Lg尾波衰减研究——Lg尾波Q0地震成像.地球物理学报, 47(6):1044-1052. doi: 10.3321/j.issn:0001-5733.2004.06.017 刘丽芳, 苏有锦, 刘杰等, 2010.云南和四川中小地震应力降时空特征研究.地震研究, 33(3):314-319. doi: 10.3969/j.issn.1000-0666.2010.03.012 卢育霞, 石玉成, 2003.1988年肃南地震近场地面运动的随机模拟.岩石力学与工程学报, 22(S2):2794-2799. http://d.old.wanfangdata.com.cn/Periodical/yslxygcxb2003z2054 马宏生, 刘杰, 张国民等, 2006.云南地区尾波Qc值的分布特征及其初步解释.地震, 26(3):37-43. http://d.old.wanfangdata.com.cn/Periodical/diz200603005 毛燕, 徐彦, 王彬等, 2005.四川及邻区Lg尾波的Q值分布特征.地震研究, 28(1):38-42. doi: 10.3969/j.issn.1000-0666.2005.01.008 秦嘉政, 阚荣举, 1986.用近震尾波估算昆明及其周围地区的Q值和地震矩.地球物理学报, 29(2):145-156. doi: 10.3321/j.issn:0001-5733.1986.02.005 秦建增, 刘昌铨, 嘉世旭等, 1997.唐山地震区地壳Q值结构的研究.见: 1997年中国地球物理学会第十三届学术年会论文集.上海: 中国地球物理学会. 师海阔, 朱新运, 贺永忠等, 2011.利用Sato模型对宁夏及邻区尾波Q值的研究.地震, 31(1):118-126. http://d.old.wanfangdata.com.cn/Periodical/diz201101013 石玉成, 陈厚群, 李敏等, 2005.随机有限断层法合成地震动的研究与应用.地震工程与工程振动, 25(4):18-23. doi: 10.3969/j.issn.1000-1301.2005.04.003 苏伟, 吴建平, 明跃红等, 2006.Lg尾波Q0值与中国大陆及邻区的地质构造关系.地震学报, 28(2):132-140. doi: 10.3321/j.issn:0253-3782.2006.02.003 苏有锦, 2009.云南地区地震波衰减(Q值)结构反演成像研究.合肥: 中国科学技术大学. http://cdmd.cnki.com.cn/article/cdmd-10358-2010042981.htm 孙吉泽, 俞言祥, 何金刚等, 2013.2013年乌鲁木齐MS5.6和MS5.1地震强地震动模拟研究.地震学报, 39(5):751-763. http://d.old.wanfangdata.com.cn/Periodical/dizhen201705010 孙晓丹, 陶夏新, 王国新等, 2009.地震动随机合成中与震源谱相关的动力学拐角频率.地震学报, 31(5):537-543. doi: 10.3321/j.issn:0253-3782.2009.05.006 孙晓丹, 2010.强地震动场估计中若干问题的研究.哈尔滨: 哈尔滨工业大学. http://cdmd.cnki.com.cn/article/cdmd-10213-2011012837.htm 陶夏新, 陈富, 孙晓丹, 2012.强地震动随机合成中震源谱模型的改进.岩土工程学报, 34(3):504-507. http://d.old.wanfangdata.com.cn/Thesis/D259854 王国新, 2001.强地震动衰减研究.哈尔滨:中国地震局工程力学研究所. 王国新, 史家平, 2008.近场强地震动合成方法研究及地震动模拟.东北地震研究, 24(2):4-10. doi: 10.3969/j.issn.1674-8565.2008.02.002 王海云, 2004.近场强地震动预测的有限断层震源模型.哈尔滨:中国地震局工程力学研究所. 王振宇, 2017.高频地震动随机模拟方法研究.哈尔滨:中国地震局工程力学研究所. 姚虹, 冯锐, 1988.中国东西剖面的地壳Q结构研究.地震研究, 11(6):539-550. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000000384145 袁一凡, 田启文, 2012.工程地震学.北京:地震出版社. 臧绍先, 1984.地震应力降与岩石破裂应力降.地震学报, 6(2):182-194. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000000375738 张翠然, 陈厚群, 李敏, 2011.采用随机有限断层法生成最大可信地震.水利学报, 42(6):721-728. http://www.cnki.com.cn/Article/CJFDTOTAL-SLXB201106017.htm 张龙文, 2013.地震加速度时程合成方法研究.南昌: 南昌航空大学. http://cdmd.cnki.com.cn/Article/CDMD-10406-1014006798.htm 赵翠萍, 陈章立, 华卫等, 2011.中国大陆主要地震活动区中小地震震源参数研究.地球物理学报, 54(6):1478-1489. doi: 10.3969/j.issn.0001-5733.2011.06.007 郑天愉, 姚振兴, 1993.用近场记录研究唐山地震的震源过程.地球物理学报, 36(2):174-184. doi: 10.3321/j.issn:0001-5733.1993.02.006 钟菊芳, 2014.近场强地震动模拟现状分析.南昌航空大学学报:自然科学版, 28(2):1-8. http://d.old.wanfangdata.com.cn/Periodical/nchkgyxyxb201402001 朱百慧, 2016.强震数据Kappa值影响因素的讨论.哈尔滨:中国地震局工程力学研究所. Abe K., 1975. Reliable estimation of the seismic moment of large earthquakes. Journal of Physics of the Earth, 23(4):381-390. doi: 10.4294/jpe1952.23.381 Abercrombie R. E., 1995. Earthquake source scaling relationships from −1 to 5 ML using seismograms recorded at 2.5km depth. Journal of Geophysical Research, 100 (B12):24015-24036. doi: 10.1029/95JB02397 Aki K., Lee W. H. K., 1976. Determination of three-dimensional velocity anomalies under a seismic array using first P arrival times from local earthquakes:1. A homogeneous initial model. Journal of Geophysical Research, 81 (23):4381-4399. doi: 10.1029/JB081i023p04381 Aki K., Richards P. G., 1980. Quantitative seismology theory and methods. San Francisco:W.H. Freeman and Company. Allmann B. P., Shearer P. M., 2007. Spatial and temporal stress drop variations in small earthquakes near Parkfield, California. Journal of Geophysical Research, 112 (B4):B04305. doi: 10.1029-2006JB004395/ Anderson J. G., Hough S. E., 1984. A model for the shape of the Fourier amplitude spectrum of acceleration at high frequencies. Bulletin of the Seismological Society of America, 74 (5):1969-1993. http://www.researchgate.net/publication/215754549_A_model_for_the_shape_of_the_Fourier_amplitude_spectrum_at_high_frequencies Anderson J. G., 1991. A preliminary descriptive model for the distance dependence of the spectral decay parameter in southern California. Bulletin of the Seismological Society of America, 81 (6):2186-2193. Andrews D. J., 1976. Rupture propagation with finite stress in Antiplane Strain. Journal of Geophysical Research, 81 (20):3575-3582. doi: 10.1029/JB081i020p03575 Atkinson G. M., 1996. The high-frequency shape of the source spectrum for earthquakes in eastern and western Canada. Bulletin of the Seismological Society of America, 86 (1A):106-112. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=d6b37d6f73367ec43ed271cbb0813fa6 Atkinson G. M., 2004. Empirical attenuation of ground-motion spectral amplitudes in southeastern Canada and the northeastern United States. Bulletin of the Seismological Society of America, 94 (6):2419-2423. doi: 10.1785/0120040161 Atkinson G. M., Assatourians K., Boore D. M., et al., 2009. A guide to differences between stochastic point-source and stochastic finite-fault simulations. Bulletin of the Seismological Society of America, 99 (6):3192-3201. doi: 10.1785/0120090058 Atkinson G. M., Goda K., Assatourians K., 2011. Comparison of nonlinear structural responses for accelerograms simulated from the stochastic finite-fault approach versus the hybrid broadband approach. Bulletin of the Seismological Society of America, 101(6):2967-2980. doi: 10.1785/0120100308 Beresnev I. A., Atkinson G. M., 1997. Modeling finite-fault radiation from the ωn spectrum. Bulletin of the Seismological Society of America, 87 (1):67-84. Beresnev I. A., Atkinson G. M., 1998. FINSIM-a FORTRAN program for simulating stochastic acceleration time histories from finite faults. Seismological Research Letters, 69 (1):27-32. doi: 10.1785/gssrl.69.1.27 Beresnev I. A., Atkinson G. M., 1998. Stochastic finite-fault modeling of ground motions from the 1994 Northridge, California, earthquake. Ⅰ. Validation on rock sites. Bulletin of the Seismological Society of America, 88 (6):1392-1401. Beresnev I. A., Atkinson G. M., 1999. Generic finite-fault model for ground-motion prediction in eastern North America. Bulletin of the Seismological Society of America, 89 (3):608-625. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=455821ae43b43aa59ca91d713fc0ae43 Boore D. M., ,Joyner WB, 1997. Site amplifications for generic rock sites. Bulletin of the Seismological Society of America. 87 (2):327-341. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0210394413/ Boore D. M., 2009. Comparing stochastic point-source and finite-source ground-motion simulations:SMSIM and EXSIM. Bulletin of the Seismological Society of America, 99 (6):3202-3216. doi: 10.1785/0120090056 Bouchon M., Sekiguchi H., Irikura K., et al., 1998. Some characteristics of the stress field of the 1995 Hyogo-ken Nanbu (Kobe) earthquake. Journal of Geophysical Research, 103 (B10):24271-24282. doi: 10.1029/98JB02136 Brune. J.N., 1970. Tectonic stress and the spectra of seismic shear waves from earthquakes. Journal of Geophysical Research. 75 (26):4997-5009. doi: 10.1029/JB075i026p04997 Chandler A. M., Lam N. T. K., Tsang H. H., 2006. Near-surface attenuation modelling based on rock shear-wave velocity profile. Soil Dynamics and Earthquake Engineering, 26 (11):1004-1014. doi: 10.1016/j.soildyn.2006.02.010 Chen S. Z., Atkinson G. M., 2002. Global comparisons of earthquake source spectra. Bulletin of the Seismological Society of America, 92 (92):885-895. doi: 10.1785-0120010152/ Chopra S., Kumar D., Choudhury P., et al., 2012. Stochastic finite fault modelling of Mw4.8 earthquake in Kachchh, Gujarat, India. Journal of Seismology, 16 (3):435-449. doi: 10.1007/s10950-012-9280-0 Cotton F., Scherbaum F., Bommer J. J., et al., 2006. Criteria for selecting and adjusting ground-motion models for specific target regions:application to central Europe and rock sites. Journal of Seismology, 10 (2):137-156. doi: 10.1007/s10950-005-9006-7 Edwards B., Fäh D., Giardini D., 2011. Attenuation of seismic shear wave energy in Switzerland. Geophysical Journal International, 185 (2):967-984. doi: 10.1111/gji.2011.185.issue-2 Fukuyama E., Olsen K. B., 2002. A condition for super-shear rupture propagation in a heterogeneous stress field. Pure and Applied Geophysics, 159 (9):2047-2056. doi: 10.1007/s00024-002-8722-y Guatteri M., Mai P. M., Beroza G. C., et al., 2003. Strong ground-motion prediction from stochastic-dynamic source models. Bulletin of the Seismological Society of America, 93 (1):301-313. doi: 10.1785/0120020006 Hanks T. C., McGuire R. K., 1981. The character of high-frequency strong ground motion. Bulletin of the Seismological Society of America, 71 (6):2071-2095. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=df58c2f1273e0436e69f9d0b2bab856c Hardebeck J. L., Hauksson E., 1997. Static stress drop in the 1994 Northridge, California, aftershock sequence. Bulletin of the Seismological Society of America, 87 (6):1495-1501. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=8e5d0c04733ff45ba677296a892a1f08 Hardebeck J. L., Aron A., 2009. Earthquake stress drops and inferred fault strength on the Hayward fault, East San Francisco Bay, California. Bulletin of the Seismological Society of America, 99 (3):1801-1814. doi: 10.1785/0120080242 Harris R. A., Day S. M., 1999. Dynamic 3D simulations of earthquakes on En Echelon Faults. Geophysical Research Letters, 26 (14):2089-2092. doi: 10.1029/1999GL900377 Hartzell S. H., Heaton T. H., 1983. Inversion of strong ground motion and teleseismic waveform data for the fault rupture history of the 1979 Imperial Valley, California, earthquake. Bulletin of the Seismological Society of America, 73 (6A):1553-1583. https://www.researchgate.net/publication/251592818_Inversion_of_strong_ground_motion_and_teleseismic_waveform_data_for_the_fault_rupture_history_of_the_1979_Imperial_Valley_California_earthquake Heaton T. H., 1990. Evidence for and implications of self-healing pulses of slip in earthquake rupture. Physics of the Earth and Planetary Interiors, 64 (1):1-20. doi: 10.1016/0031-9201(90)90002-F Housner G. W., 1947. Characteristics of strong-motion earthquakes. Bulletin of the Seismological Society of America, 37 (l):19-31. http://d.old.wanfangdata.com.cn/OAPaper/oai_doaj-articles_1418725f443cdbac5adcbbbb6187f02a Jin A. S., Aki K., 1988. Spatial and temporal correlation between coda Q and seismicity in China. Bulletin of the Seismological Society of America, 78 (2):741-769. https://www.researchgate.net/publication/290791515_Spatial_and_temporal_correlation_between_coda_Q_and_seismicity_in_China Jin A. S., Moya C. A., Ando M., 2000. Simultaneous determination of site responses and source parameters of small earthquakes along the atotsugawa fault zone, Central Japan. Bulletin of the Seismological Society of America, 90 (6):1430-1445. doi: 10.1785/0119990140 Kamae K, Irikura K, Pikarta A, 1998. A Technique for Simulating Strong Ground Motion Using Hybrid Green's Function. Bulletin of the Seismological Society of America, 88 (2):357-367. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=eaad532fbb0c1cf44b3111ddae5dc308 Kanamori H., Anderson D. L., 1975. Theoretical basis of some empirical relations in seismology. Bulletin of the Seismological Society of America, 65 (5):1073-1095. http://www.researchgate.net/publication/228077798_Theoretical_basis_of_some_empirical_relations_in_seismology Kanamori H., Rivera L., 2004. Static and dynamic scaling relations for earthquakes and their implications for rupture speed and stress drop. Bulletin of the Seismological Society of America, 94 (1):314-319. doi: 10.1785/0120030159 Kilb D., Biasi G., Anderson J., et al., 2012. A Comparison of Spectral Parameter Kappa from Small and Moderate Earthquakes Using Southern California ANZA Seismic Network Data. Bulletin of the Seismological Society of America, 102 (1):284-300. doi: 10.1785/0120100309 Mayeda K., Walter W. R., 1996. Moment, energy, stress drop, and source spectra of western United States earthquakes from regional coda envelopes. Journal of Geophysical Research, 101 (B5):11195-11208. doi: 10.1029/96JB00112 McGuire, R.K., Hanks, T.C., 1980. RMS accelerations and spectral amplitudes of strong gound motion during the San Femando, Califomia earthquake. Bull. Seism Soc. Amer, 70:1907-1919. Miyake H., Iwata T., Irikura K., 2003. Source characterization for broadband ground-motion simulation:kinematic heterogeneous source model and strong motion generation area. Bulletin of the Seismological Society of America, 93 (6):2531-2545. doi: 10.1785/0120020183 Motazedian D., Atkinson G. M., 2005. Stochastic finite-fault modeling based on a dynamic corner frequency. Bulletin of the Seismological Society of America, 95 (3):995-1010. doi: 10.1785/0120030207 Nuttli, O. W., 1988. Lg magnitudes and yield estimates for underground Novaya Zemlya nuclear explosions. Bulletin of the Seismological Society of America, 78 (2): 873-884. Papageorgiou A. S., Aki K., 1983. A specific barrier model for the quantitative description of inhomogeneous faulting and the prediction of strong ground motion. Part Ⅱ. Applications of the model. Bulletin of the Seismological Society of America, 73 (4):953-978. https://www.sciencedirect.com/science/article/pii/0148906284904984 Sato R., 1999. Theoretical basis on relationships between focal parameters and earthquake magnitude. Journal of Physics of the Earth, 27 (5):353-372. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=J-STAGE_3391661 Scholz C. H., 2002. The mechanics of earthquakes and faulting. 2nd ed. Cambridge:Cambridge University Press. Shi J. H., Kim W. Y., Richards P. G., 1998. The corner frequencies and stress drops of intraplate earthquakes in the northeastern United States. Bulletin of the Seismological Society of America, 88 (2):531-542. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=7dde414834ae2d5316d89f6d2346edaa Shih X. R., Chun K. Y., Zhu T., 1994. Attenuation of 1-6s Lg waves in Eurasia. Journal of Geophysical Research, 99 (B12):23859-23874. doi: 10.1029/94JB02163 Somerville P., Irikura K., Graves R., et al., 1999. Characterizing crustal earthquake slip models for the prediction of strong ground motion. Seismological Research Letters, 70 (1):59-80. doi: 10.1785/gssrl.70.1.59 Sun X. D., Tao X. X., Chen F., 2010. Improvements of corner frequency and scaling factor for stochastic finite-fault modeling. Earthquake Engineering and Engineering Vibration, 9 (4):503-511. doi: 10.1007/s11803-010-0032-2 Sun X. D., Tao X. X., Duan S. S., et al., 2013. Kappa (k) derived from accelerograms recorded in the 2008 Wenchuan mainshock, Sichuan, China. Journal of Asian Earth Sciences, 73:306-316. doi: 10.1016/j.jseaes.2013.05.008 Sun Xiaodan, Tao Xiaxin, Wang Guoxin, Liu Taojun, 2009. Dynamic corner frequency in source spectral model for stochastic synthesis of ground motion. Earthquake Science, 22 (3):271-276. doi: 10.1007/s11589-009-0271-3 Tao X.X., Sun X.D., Wang G.X, 2008. An dynamic corner frequency based source spectral model. Proc. of the 14 World Conference on Earthquake Engineering. Tsai C. C. P., Chen K. C., 2000. A model for the high-cut process of strong-motion accelerations in terms of distance, magnitude, and site condition:an example from the SMART 1 Array, Lotung, Taiwan. Bulletin of the Seismological Society of America, 90 (6):1535-1542. doi: 10.1785/0120000010 Tusa G., Gresta S., 2008. Frequency-dependent attenuation of P waves and estimation of earthquake source parameters in southeastern Sicily, Italy. Bulletin of the Seismological Society of America, 98 (6):2772-2794. doi: 10.1785/0120080105 Van Houtte C., Drouet S., Cotton F., 2011. Analysis of the origins of k (Kappa) to compute hard rock to rock adjustment factors for GMPEs. Bulletin of the Seismological Society of America, 101 (6):2926-2941. doi: 10.1785/0120100345 Wang G. Q., 2001. Comparisons of ground motions from five aftershocks of the 1999 Chi-Chi, Taiwan, earthquake with empirical predictions largely based on data from California. Bulletin of the Seismological Society of America, 91 (5):1212-1217. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=5d7512a3024e09f35edfb0fa0a109ceb Wells D. L., Coppersmith K. J., 1994. New empirical relationships among magnitude, rupture length, rupture width, rupture area, and surface displacement. Bulletin of the Seismological Society of America, 84 (4):974-1002. http://www.researchgate.net/publication/215755871_New_empirical_relationships_among_magnitude_rupture_length_rupture_width_rupture_area_and_surface_displacement Zhao D. P., Hasegawa A., Horiuchi S., 1992. Tomographic imaging of P and S wave velocity structure beneath northeastern Japan. Journal of Geophysical Research, 97 (B13):19909-19928. doi: 10.1029/92JB00603 期刊类型引用(1)
1. 赵密,宋军,缪惠全,钟紫蓝,杜修力. 考虑地震强度空间变异性的地铁系统连通可靠性分析. 交通运输系统工程与信息. 2023(04): 203-210 .  百度学术
百度学术其他类型引用(2)
-





 下载:
下载:









 下载:
下载: