The Study of Sample Size on b-value Statistics in the Gutenberg-Richter's Law
-
摘要: b值是研究地震活动的重要指标,其广泛应用于地震危险性分析和地震预测研究之中,与实际资料的完整性、样本量的大小、计算方法等因素有着重要的关系。常见的b值计算方法有最小二乘法和最大似然法,样本量的大小对这2种方法影响很大。本文利用蒙特卡罗模拟地震目录和汾渭地震带实际目录作为样本,从中抽取不同大小的样本量进行计算,研究不同样本量下这2种方法计算得到的b值与设定值或真实值之间的差别。结果表明,最小二乘法需要的最低样本量为1000,最大似然法为200;当样本量达不到要求时,计算出的b值是不可靠的;由于对样本量的要求不同,前者适用于计算区域的整体b值,而后者在研究某区域b值在时间轴上的变化方面更有优势。本研究为确定2种b值计算方法对样本量的最低要求提供了参考依据。Abstract: The b-value is an important indicator for evaluating the level of seismicity, and is widely used in seismic hazard analysis and earthquake prediction research. The b-value is basically effected by some factors, such as the actual data integrity, the quantity of earthquake samples, calculation methods and so on. The most widely used two methods for calculating b values include the least square method (LSM) and the maximum likelihood method (MLM), and the results from both methods can remarkably vary with different the quantity of earthquake samples. In this paper, based on the real catalog of the Fen-Wei seismic zone and the Monte-Carlo simulated earthquake catalog, we use different number of samples to calculate the b values and try to find the differences between the calculated results and the set values/true values. It turns out that the threshold value of samples is 1000 and 200 for LSM and MLM, respectively. When the sample size does not meet the requirements, the calculated b-value is not reliable. It suggets that LSM is suitable for calculating the b-value of the whole region, while MLM is more advantageous in studying the b-value of a region on the time axis. This study provides a reference for the threshold values of the samples for two methods, which is of great significance to the seismic hazard analysis and earthquake prediction.
-
Key words:
- b-value /
- The least square method /
- The maximum likelihood method /
- Sample size
-
引言
众所周知,我国是地震频发的国家,地震可能造成高速铁路列车脱轨事故,为此,我国建立了相应的地震报警及紧急处置系统,力争在潜在破坏性地震到达之前发出报警,降低列车发生脱轨或倾覆的概率。目前广泛采用的地震报警参数主要为峰值加速度PGA,然而PGA主要体现地震波幅值,未考虑频谱及持时影响,对于无破坏性的高频小震可能产生误报现象。为避免这种干扰,郭恩栋等(2015)引入谱强度SI作为高速铁路地震报警参数,并给出相应的计算方法,SI从能量方面出发,考虑了幅值及频谱影响,忽略持时的影响,但相比PGA更合理。美国电力研究所EPRI(O'Hara 等,1991)在核电厂地震监测报警中引入累积绝对速度CAV作为报警参数,研究发现低频震动对CAV的贡献明显高于高频震动。我国三代核电站AP1000参考国外标准也引入了CAV作为地震报警参数(胡进军等,2013)。林宜娴(2002)提出CAV、PGA、PGV等参数可作为地震报警参数,其稳定性好,且确定性较高。Kostov(2005)根据欧洲几次强地震动数据,分析得出标准化累积绝对速度CAVSTD在预测地震动破坏能力方面明显优于PGA。伊斯坦布尔在地震预报警系统中利用累积绝对速度快速判别强地震动(Erdik等,2003)。Fahjan等(2011)在地震报警的触警算法中引入CAV,并提出新的用于报警系统的参数。黄俊等(2014)引入CAV作为报警参数,分析其在高速铁路报警中的适用性,研究发现CAV可有效排除破坏性小的高频小震对高速铁路列车地震报警的干扰。目前虽对CAV在地震报警中的应用有了深入研究,但在高速铁路列车地震报警中的研究较少,仅通过相关的地震波数据处理得出,未考虑车轨动力响应关系,且给出的计算参数为CAVSTD,为此,需探讨考虑车轨关系的基于相关起算阈值的CAV计算方法。
1. CAV相关参数
目前,地震工程界主要研究CAV、CAVSTD、CAV5。其中CAV于1988年由美国电力研究所(O'Hara等,1991)在核电厂报警中第2级判别标准中提出的,其概念为记录地震加速度数据的绝对值对记录时间进行积分,记为累积绝对速度CAV:
$${\rm{ CAV}} = \mathop \int \nolimits_0^{{{{t}}_{{\rm{max}}}}} \left| {{{a}}\left( {{t}} \right)} \right|{\rm{d}}t$$ (1) 为提高CAV在核电厂报警中的准确度,EPRI在报告中引入标准化累积绝对速度CAVSTD,其可有效避免长持时记录的地震波尾部对应的幅值小且破坏性小的地震动对其数值的影响,其定义如下:将记录的加速度时程分成以1 s为时间间隔的N个小段,当1 s时间段内存在加速度绝对值≥0.025 g时,对该秒内加速度数值的绝对值进行积分,否则该秒内的积分值记为零,然后将整个记录时程进行相加求和,表达式为:
$$ {\rm{CA{V_{STD}}}} = \mathop \sum \limits_{{i=1}}^n \mathop \int \nolimits_{{i}}^{{{i}} + 1} {W_i}\left| {{{a}}\left( {{t}} \right)} \right|{\rm{d}}t,\;\;\;\;\;\;\;\;{W_i} = \left\{ {\begin{array}{*{20}{c}} {0,\left| {{{a}}\left( {{t}} \right)} \right| < 0.025\;g}\\ {1,\left| {{{a}}\left( {{t}} \right)} \right| \geqslant 0.025\;{{g}}} \end{array}} \right.$$ (2) Kramer等(2002)于2002年提出CAV5,用于场地液化判别,其概念为:将记录的加速度时程中对加速度数值的绝对值≥0.005 g进行积分求和,表达式为:
$${{\rm{ CAV}}_5} = \displaystyle\int \nolimits_0^\infty \left\langle {\rm{\chi }} \right\rangle \left| {{{a}}\left( {{t}} \right)} \right|{\rm{d}}t,\;\;\;\left\langle {\rm{\chi }} \right\rangle = \left\{ {\begin{array}{*{20}{c}} {0\;\left| {{{a}}\left( {{t}} \right)} \right| < 0.005\;g}\\ {1\;\left| {{{a}}\left( {{t}} \right)} \right| \geqslant 0.005\;{{g}}} \end{array}} \right. $$ (3) 式中,a(t)为加速度时程,Wi为权重。
2. 改进的标准化累积绝对速度应用于高速铁路地震报警阈值中的计算方法
2.1 改进的标准化累积绝对速度起算阈值确定
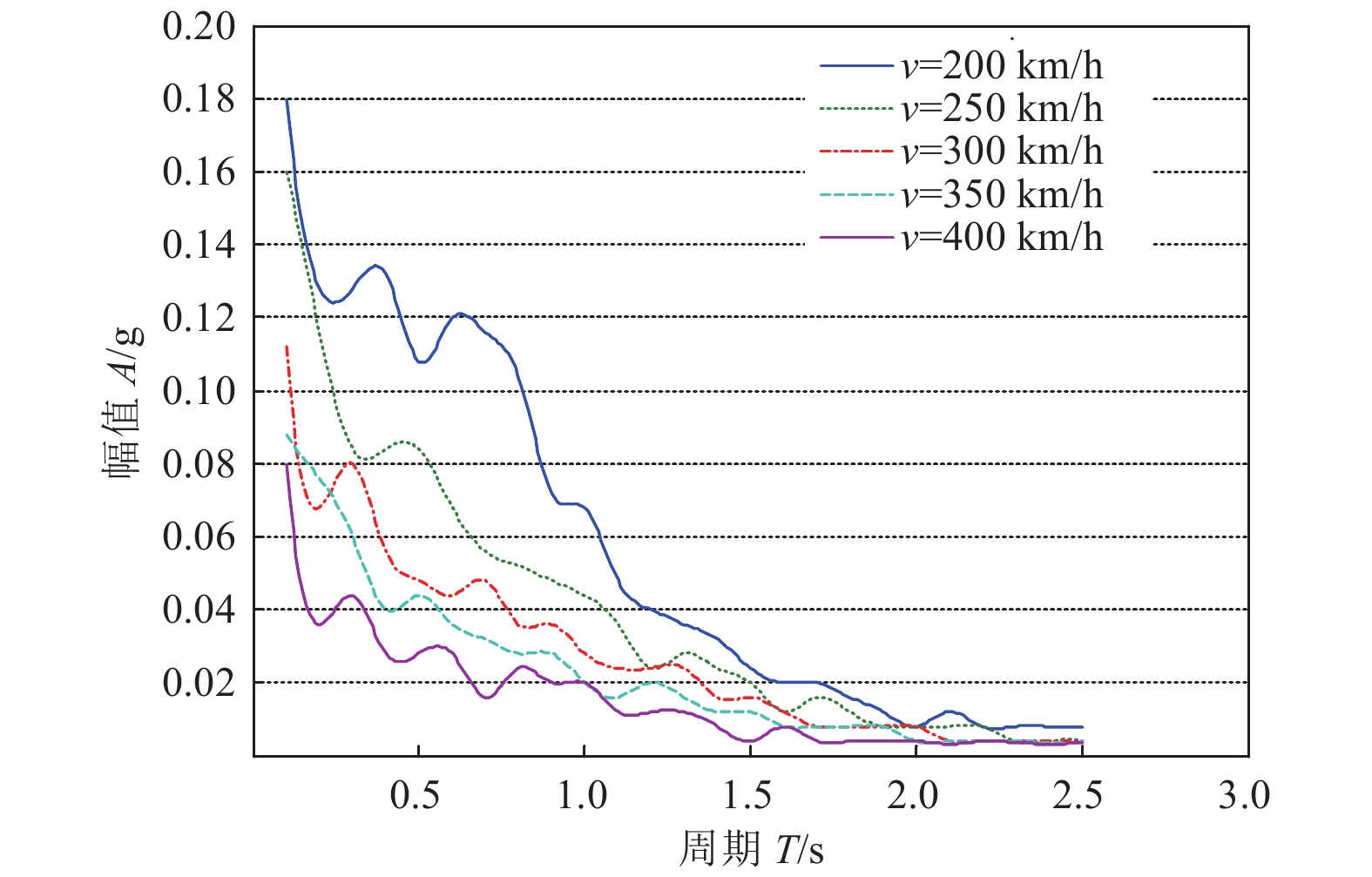
本文引入31自由度的SY97477车辆模型(崔恩文,2014),结合车轨典型非线性接触关系,依据列车安全行驶的3项评判指标(脱轨系数、轮重减载率及横向力),分析车体在轨道不平顺(我国高速铁路无砟轨道标准谱)与简谐波(仅考虑横向输入,与列车行驶方向垂直)共同激励下的动态响应,考虑95%的安全保证,引入2.55倍的动力放大系数(孙汉武等,2007),计算安全极限状态(超出3项指标任意1个限值)下不同车速对应的简谐波幅值随周期的变化关系,如图1所示。具体模型参数、车轨接触关系及评判标准可参考崔恩文(2014)的研究。
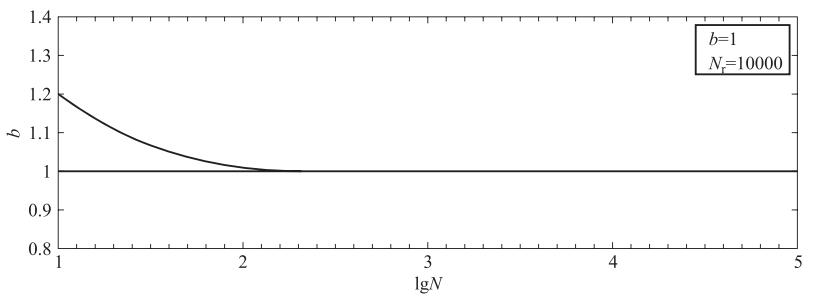
由图1可知,车速一定时,即使幅值很小(<0.025 g)的低频简谐波也可能使列车脱轨,因此不能简单地套用标准化累积绝对速度CAVSTD计算公式,有必要研究改进的标准化累积绝对速度应用在高速铁路地震报警阈值中的计算方法。车速一定时,简谐波幅值随周期增加逐渐递减,最终趋于常数,且周期越大,不同车速对应的简谐波幅值之间的差值越小。车速为200 km/h时,当简谐波周期达2.2 s时,其幅值开始趋于常数,该常数约为0.008 g,本文规定此时的周期为简谐波幅值趋于常数对应的起始周期Tst,该常数记为Amin。不同车速下简谐波幅值趋于常数对应的起始周期及该常数的数值如表1所示。
表 1 不同车速下简谐波幅值趋于常数对应的起始周期及该常数的数值Table 1. The initial period of the simple harmonic wave amplitude tending to be constant at different speeds and the value of the constant项目 车速/(km·h−1) 200 250 300 350 400 起始周期Tst/s 2.2 2.3 2.1 2.0 1.7 Amin/g 0.008 0.004 0.004 0.004 0.004 由表1可知,车速为200 km/h与车速≥250 km/h时对应的Amin不同,车速≥250 km/h时Amin相同,但起始周期Tst随车速的增加呈递减趋势,说明车速越大,简谐波趋于常数所需的周期越小。
车速为200 km/h时,幅值低于0.008 g的简谐波对列车运行安全的影响可忽略不计。车速≥250 km/h时,幅值低于0.004 g的简谐波对列车运行安全的影响可忽略不计。由此可推算出改进的标准化累积绝对速度起算阈值,如表2所示。
表 2 不同车速下改进的标准化累积绝对速度起算阈值Table 2. Starting threshold of improved calculation of standardized cumulative absolute velocity at different speeds项目 车速/(km·h−1) 200 ≥250 改进的标准化累积绝对速度起算阈值/g 0.008 0.004 2.2 改进的标准化累积绝对速度应用在地震报警中的可行性分析
考虑改进的标准化累积绝对速度为累积过程的算法,其值对于加速度超过0.004 g或0.008 g的有限个数的高频小震会很小,因此,可较好地排除高速铁路沿线的高频小震,是较好的地震监测报警参数。
魏智祥(2015)分析了京武高速铁路线布置的强震仪监测到的列车运行时震动记录数据,发现列车运行产生的震动记录明显高于背景噪声,且发现东西、南北、垂直方向列车振动噪声幅值均≤0.002 g,由此可知本文设定的不同车速下改进的标准化累积绝对速度可有效排除列车振动的影响。
综上所述,本文给出改进的标准化累积绝对速度用于高速铁路地震报警是可行的。
2.3 改进的标准化累积绝对速度计算公式的确定
参照CAVSTD计算方法,本文给出车速为200 km/h时改进的标准化累积绝对速度CAV8计算公式如下:
$${{\rm{CAV}}_8} = \mathop \sum \limits_{i=1}^n \mathop \int \nolimits_{{i}}^{{{i}} + 1} {W_i}\left| {{{a}}\left( {{t}} \right)} \right|{\rm{d}}t,\;\;\;\;{W_i} = \left\{ {\begin{array}{*{20}{c}} {0,\;\left| {{{a}}\left( {{t}} \right)} \right| < 0.008\;g}\\ {1,\;\left| {{{a}}\left( {{t}} \right)} \right| \geqslant 0.008\;{{g}}} \end{array}} \right.$$ (4) 当
$ a\left(t\right) $ $< 0.008\;g$ 时,${{W}}_{{i}}$ =0;当$\left|{a}\left({t}\right)\right|\geqslant 0.008\;g$ 时,${{W}}_{{i}}$ =1。本文给出的CAV8定义为:将加速度时程按1 s为时间间隔进行划分,如果在这1 s内加速度绝对值≥0.008 g,则对该秒内的加速度绝对值进行积分,否则忽略该秒内的积分值,然后将整个时程中每秒积分得到的数值进行累积求和。
车速为250 km/h时改进的标准化累积绝对速度CAV4计算公式如下:
$${ {\rm{CAV}}_4} = \mathop \sum \limits_{i=1}^{{n}} \mathop \int \nolimits_{{i}}^{{{i}} + 1} {W_i}\left| {{{a}}\left( {{t}} \right)} \right|{\rm{d}}t,\;\;\;\;\;{W_i} = \left\{ {\begin{array}{*{20}{c}} {0,\;\left| {{{a}}\left( {{t}} \right)} \right| < 0.004\;g}\\ {1,\;\left| {{{a}}\left( {{t}} \right)} \right| \geqslant 0.004\;{{g}}} \end{array}} \right.$$ (5) 当
${a}\left({t}\right)$ $< 0.004\;g$ 时,${{W}}_{{i}}=0$ ;当$\left|{a}\left({t}\right)\right|\geqslant 0.004\;{g}$ 时,${{W}}_{{i}}$ =1。本文给出的CAV4定义为:将加速度时程按1 s为时间间隔进行划分,如果在这1 s内加速度绝对值≥0.004 g,则对该秒内的加速度绝对值进行积分,否则忽略该秒内的积分值,然后将整个时程中每秒积分得到的数值进行累积求和。
3. 高速铁路地震报警阈值确定
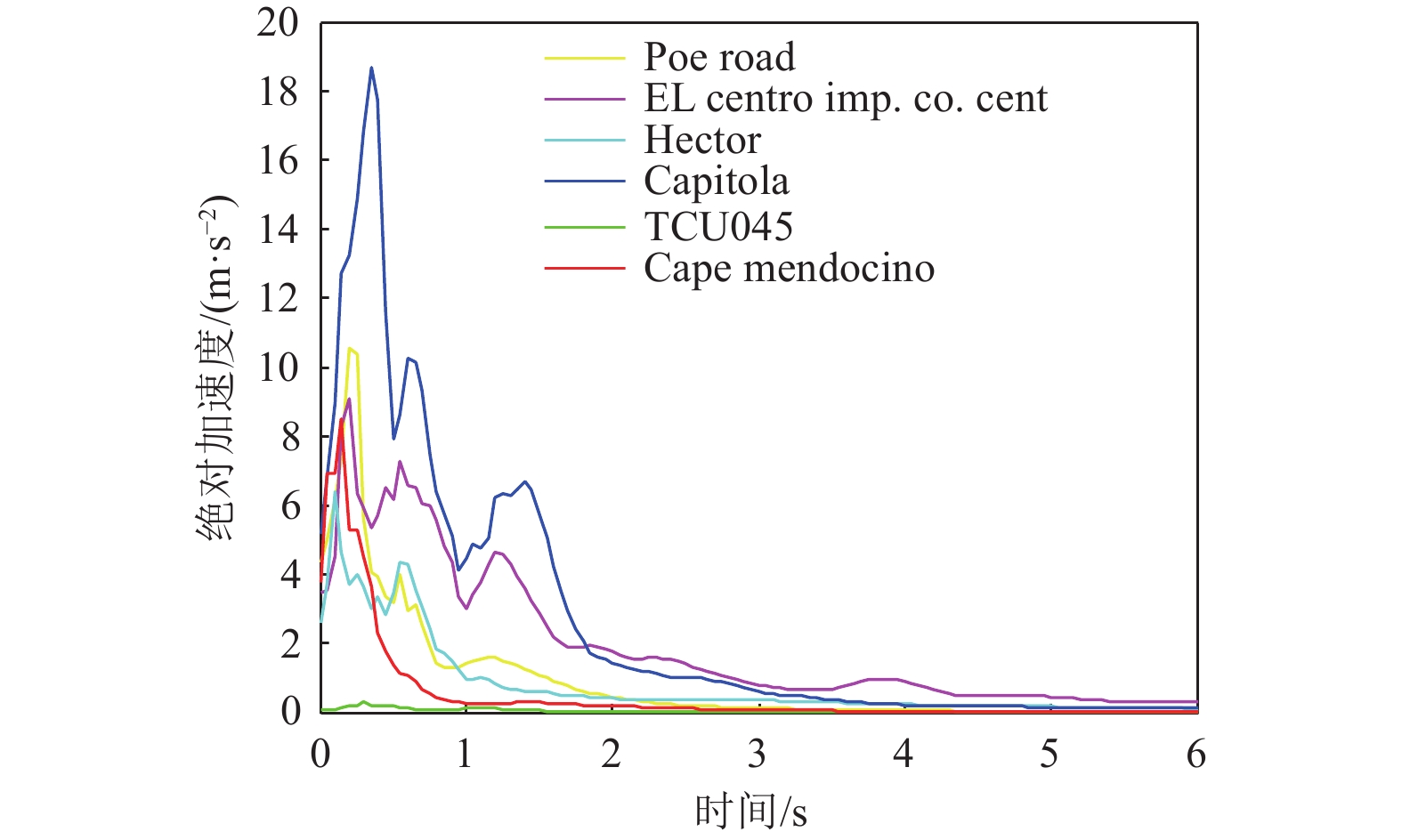
为确定基于改进的标准化累积绝对速度高速铁路地震报警阈值,本文从Peer数据库选取6条不同场地地震波(表3),对应的加速度反应谱如图2所示,对其进行归一化处理,分析加速度幅值与评判列车脱轨3项指标之间的关系,确定不同车速下影响行车安全的6条地震波加速度上限值,按照本文给出不同车速下改进的标准化累积绝对速度计算公式,给出6条地震波对应的改进的标准化累积绝对速度上限值,如表4所示。
表 3 地震波相关信息Table 3. Seismic wave information项目 地震波台站名称 Cape Mendocino TCU045 Capitola Hector EL Centro Imp. Co. Cent Poe Road 震级 7.01 7.62 6.93 7.13 6.54 6.54 峰值加速度/g 0.376 5 0.008 1 0.528 5 0.265 6 0.357 9 0.446 3 v30/(m·s−1) 567.78 704.64 288.62 726.00 192.05 316.64 加速度记录持时/s 36.0 45.0 39.9 45.3 40.0 22.3 表 4 不同车速下6条地震波加速度上限值与改进的标准化累积绝对速度上限值Table 4. The upper limit of acceleration amplitudes and upper limit improved calculation of standardized cumulative absolute velocity CAV of six seismic waves at different speeds地震波台站名称 车速200 km/h 车速250 km/h 车速300 km/h 车速350 km/h 车速400 km/h 加速度
上限值/
g改进的标准化
累积绝对速度
上限值/g-sec加速度
上限值/
g改进的标准化
累积绝对速度
上限值/g-sec加速度
上限值/
g改进的标准化
累积绝对速度
上限值/g-sec加速度
上限值/
g改进的标准化
累积绝对速度
上限值/g-sec加速度
上限值/
g改进的标准化
累积绝对速度
上限值/g-secCape Mendocino 0.074 0.17 0.070 0.17 0.055 0.13 0.040 0.09 0.039 0.09 TCU045 0.101 0.55 0.090 0.49 0.078 0.43 0.062 0.34 0.039 0.21 Capitola 0.109 0.34 0.078 0.25 0.062 0.20 0.054 0.17 0.039 0.11 Hector 0.101 0.27 0.070 0.19 0.062 0.17 0.054 0.14 0.047 0.12 EL Centro Imp. Co. Cent 0.078 0.16 0.070 0.18 0.054 0.13 0.039 0.08 0.027 0.05 Poe Road 0.074 0.19 0.054 0.14 0.043 0.11 0.039 0.10 0.035 0.08 由表4可知,车速相同时,即使地震波加速度相同,其对应的改进的标准化累积绝对速度不一定相同,如车速为200 km/h时,台站名称为Cape Mendocino与Poe Road的地震波加速度相同,但对应的改进的标准化累积绝对速度不同,且Cape Mendocino台站加速度记录持时较大,说明改进的标准化累积绝对速度与频谱紧密相关。车速≥250 km/h时,对于同一台站的地震波,峰值加速度越大其对应的改进的标准化累积绝对速度越大,如台站名称为Cape Mendocino的地震波,说明对于同一地震波而言,加速度影响累积绝对速度。根据改进的标准化累积绝对速度定义,加速度记录持时影响其数值。综上所述,改进的标准化累积绝对速度数值不仅与加速度幅值有关,还与频谱及加速度记录持时密切相关,可较好地反映地震动三要素。
选取6条地震波改进的标准化累积绝对速度最小值作为报警阈值,给出不同车速下基于改进的标准化累积绝对速度高速铁路地震报警阈值,如表5所示。由表5可知,改进的标准化累积绝对速度报警阈值与车速整体呈线性递减关系,中间车速对应的改进的标准化累积绝对速度可按线性插入计算。
表 5 不同车速下改进的标准化累积绝对速度高速铁路地震报警阈值Table 5. High-speed earthquake alarm threshold based on improved calculation of standardized cumulative absolute velocity thresholds at different vehicle speeds项目 车速/(km·h−1) 200 250 300 350 400 报警阈值/g-sec 0.16 0.14 0.11 0.08 0.05 4. 结论
(1)通过建立车轨模型,分析列车安全运行极限状态下简谐波幅值与周期的关系,得出不同车速下改进的标准化累积绝对速度起算阈值,并论证改进的标准化累积绝对速度在高速铁路地震报警中的可行性。
(2)参照标准化累积绝对速度CAVSTD计算公式,本文给出不同车速下改进的标准化累积绝对速度计算公式。
(3)根据本文给出的改进的标准化累积绝对速度计算公式,选取6条不同场地的地震波,分析列车在轨道不平顺及地震波共同激励下的动力响应,计算不同车速下改进的标准化累积绝对速度报警阈值,以供参考。
-
表 1 最大似然法模拟结果
Table 1. Simulation results by the maximum likelihood method
N b1 Pr- Pr Pr+ σ 10 1.221 0.46 0.14 0.40 0.42 20 1.211 0.46 0.25 0.29 0.38 50 1.145 0.44 0.30 0.16 0.16 100 1.061 0.53 0.37 0 0.13 200 1.039 0.52 0.48 0 0.11 400 1.036 0.47 0.53 0 0.09 500 1.025 0.45 0.55 0 0.08 1000 1.003 0.30 0.70 0 0.08 表 2 最小二乘法模拟结果
Table 2. Simulation results by the least squares method
N b1 Pr- Pr Pr+ σ 10 0.870 0.79 0.08 0.13 0.52 20 0.895 0.76 0.07 0.17 0.48 50 0.918 0.68 0.27 0.05 0.42 100 0.941 0.64 0.28 0.08 0.37 200 0.942 0.46 0.48 0.06 0.32 400 0.955 0.48 0.52 0 0.28 500 0.982 0.45 0.50 0.05 0.16 1000 0.996 0.20 0.80 0 0.12 表 3 汾渭地震带地震分档统计和年平均发生率
Table 3. The annual average incidence of different magnitude-class in Fen-Wei seismic zone
震级档M 地震个数 年平均发生率 2.0—2.4 5038 119.95 2.5—2.9 2006 47.76 3.0—3.4 734 17.48 3.5—3.9 230 5.61 4.0—4.4 110 2.62 4.5—4.9 91 2.17 5.0—5.4 15 0.36 5.5—5.9 10 0.23 6.0—8.5 3 0.07 注:年平均发生率指震级≥M的年均地震数,代表地震活动水平。 表 4 汾渭地震带b值拟合情况(1500—2010年)
Table 4. The b value fitting of earthquakes from 1500—2010 in the Fen-Wei seismic zone
样本量 均匀抽样计算b值 最小二乘法 最大似然法 10 0.60 0.897 50 0.62 0.923 100 0.63 0.816 200 0.63 0.728 300 0.65 0.767 500 0.69 0.752 700 0.70 0.756 1000 0.72 0.746 2000 0.74 0.751 5000 0.75 0.753 -
韩渭宾, 2003.b值在地震预测中的三类应用及其物理基础与须注意的问题.四川地震, (1):1-5. doi: 10.3969/j.issn.1001-8115.2003.01.001 韩晓明, 张文韬, 王树波等, 2016.河套地震带的b值时空变化特征分析.中国地震, 32(3):522-532. doi: 10.3969/j.issn.1001-4683.2016.03.009 胡聿贤, 1999.地震安全性评价技术教程.北京:地震出版社. 黄玮琼, 时振梁, 曹学锋, 1989.b值统计中的影响因素及危险性分析中b值的选取.地震学报, 11(4):351-361. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000005188048 黄玮琼, 李文香, 曹学锋, 1994a.中国大陆地震资料完整性研究之一——以华北地区为例.地震学报, 16(3):273-280. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199400057115 黄玮琼, 李文香, 曹学锋, 1994b.中国大陆地震资料完整性研究之二——分区地震资料基本完整的起始年分布图象.地震学报, 16(4):423-432. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199400057138 黄玮琼, 李文香, 1998.地震区划中b值统计时空范围的确定.地震学报, 20(5):2-6. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199800071609 焦远碧, 吴开统, 杨满栋, 1990.我国地震台网监测能力及台网观测条件质量评定.中国地震, 6(4):3-9. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000003894076 刘方斌, 曲均浩, 田兆阳等, 2017.鲁西南聊考断裂带地震危险性评价与活动性分布.大地测量与地球动力学, 37(8):802-807. http://d.old.wanfangdata.com.cn/Periodical/dkxbydz201708007 刘静伟, 吕悦军, 2016.川滇地区b值空间分布特征及其与震源类型关系的初步探讨.震灾防御技术, 11(3):561-572. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20160312&journal_id=zzfyjs 潘华, 李金臣, 2006.地震统计区地震活动性参数b值及ν4不确定性研究.震灾防御技术, 1(3):218-224. doi: 10.3969/j.issn.1673-5722.2006.03.006 任雪梅, 2011.地震区划中b值统计的若干问题研究.北京: 中国地震局地球物理研究所. 任雪梅, 高孟潭, 冯静, 2011.地震目录的完整性对b值计算的影响.震灾防御技术, 6(3):257-268. doi: 10.3969/j.issn.1673-5722.2011.03.005 沈建文, 余湛, 邱瑛, 2007.地震安评中地震活动性的统计区域与b值.国际地震动态, (3):1-6. doi: 10.3969/j.issn.0253-4975.2007.03.001 王熠熙, 张辉, 刘双庆等, 2015.河北平原地震带b值时空变化特征.地震工程学报, 37(01):188-195. doi: 10.3969/j.issn.1000-0844.2015.01.0188 吴兆营, 薄景山, 刘志平等, 2005.东北地震区b值和地震年平均发生率的统计分析.东北地震研究, 21(3):27-32, 72. doi: 10.3969/j.issn.1674-8565.2005.03.002 谢卓娟, 吕悦军, 张力方, 等, 2012a.基于现代地震资料确定汾渭地震带分区及其地震活动性参数.地球物理学进展, 27(3):894-902. http://d.old.wanfangdata.com.cn/Periodical/dqwlxjz201203009 谢卓娟, 吕悦军, 彭艳菊等, 2012b.东北地震区小震资料完整性分析及其对地震活动性参数的影响研究.中国地震, 28(3):256-265. http://d.old.wanfangdata.com.cn/Periodical/zgdz201203003 谢卓娟, 吕悦军, 兰景岩等, 2013.b值和V4的统计分析及其不确定性对地震危险性分析结果的影响研究.地震研究, 36(1):86-92. doi: 10.3969/j.issn.1000-0666.2013.01.013 谢卓娟, 李山有, 吕悦军, 2015.滇西南地区主要活动断裂的b值空间分布特征.地球科学(中国地质大学学报), 40(10):1755-1766. http://d.old.wanfangdata.com.cn/Periodical/dqkx201510016 徐伟进, 高孟潭, 2014.中国大陆及周缘地震目录完整性统计分析.地球物理学报, 57(9):2802-2812. http://qikan.cqvip.com/article/detail.aspx?id=662579475 鄢家全, 韩炜, 高孟潭, 1996.地震活动性参数的不确定性及其对区划结果的影响.中国地震, 12(S1):71-77. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199600869176 张广伟, 2016.云南地区地震的重新定位及b值研究.中国地震, 32(1):54-62. doi: 10.3969/j.issn.1001-4683.2016.01.005 张建中, 1974a.蒙特卡洛方法(Ⅰ).数学的实践与认识, (01):28-40. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000002863962 张建中, 1974b.蒙特卡洛方法(Ⅱ).数学的实践与认识, (02):43-56. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK000002863976 周公威, 张伯明, 吴忠良等, 2007.中国数字地震台网(CDSN)的近期发展.地球物理学进展, 22(4):1130-1134. doi: 10.3969/j.issn.1004-2903.2007.04.018 Maximum likelihood estimate of b in the formula logN=a-bM and its confidence limits. Bulletin of the Earthquake Research Institute, 43(2): 237-239. Gulia L., Wiemer S., 2010. The influence of tectonic regimes on the earthquake size distribution:A case study for Italy. Geophysical Research Letters, 37(10):L10305. http://adsabs.harvard.edu/abs/2010GeoRL..3710305G Nava F. A., Márquez-Ramírez V. H., Zúñiga F. R., et al., 2017. Gutenberg-Richter b-value maximum likelihood estimation and sample size. Journal of Seismology, 21(1):127-135. doi: 10.1007/s10950-016-9589-1 Rundle J. B., 1989. Derivation of the complete Gutenberg-Richter magnitude-frequency relation using the principle of scale invariance. Journal of Geophysical Research:Solid Earth, 94(B9):12337-12342. doi: 10.1029/JB094iB09p12337 Rotondi R., Garavaglia E., 2002. Statistical Analysis of the Completeness of a Seismic Catalogue. Natural Hazards, 25(3):245-258. doi: 10.1023/A:1014855822358 Schorlemmer D., Wiemer S., Wyss M., 2005. Variations in earthquake-size distribution across different stress regimes. Nature, 437(7058):538-542. http://cn.bing.com/academic/profile?id=ecb926e431866325a8ddc74b59472232&encoded=0&v=paper_preview&mkt=zh-cn Utsu T., 1965. A method for determining the value of b in a formula logN=a-bM showing the magnitude-frequency relation for earthquakes. Geophysical Bulletin of Hokkaido University, (13):99-103. Wiemer S., Wyss M., 2000. Minimum magnitude of completeness in earthquake catalogs:examples from Alaska, the Western United States, and Japan. Bulletin of the Seismological Society of America, 90(4):859-869. doi: 10.1785/0119990114 -





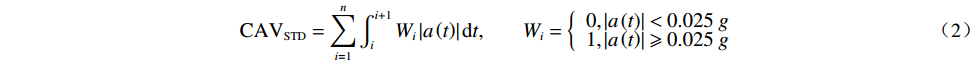
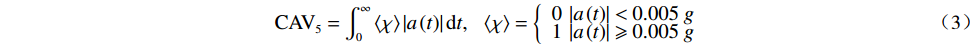

 下载:
下载:
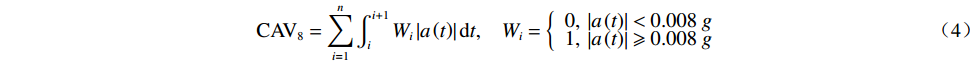
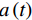
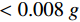
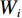
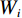
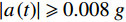
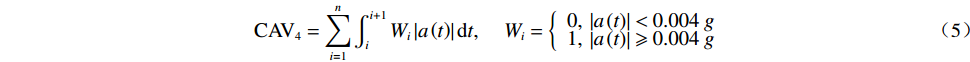
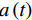
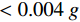
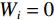


 下载:
下载: