Comparative Study of Base Shear Force Method in the Seismic Design Codes of China, the U.S.A. and Europe
-
摘要: 目前，底部剪力法是各国计算水平地震作用的基本方法，应用该方法时需要使用各自国家的抗震设计反应谱。本文汇总了中、美、欧抗震设计规范的反应谱和底部剪力法，在相同重现期和场地条件的基础上，对比了不同烈度下3本规范反应谱的异同，并通过算例对比了分别采用3本规范的底部剪力法算出的不同设防烈度下同一结构的底部地震剪力和层间地震剪力。对比结果表明，3本规范的反应谱和底部剪力法在本质上是相同的，只在表达形式和参数设置上存在差异。Abstract: Base shear force method is the basic for calculating the horizontal earthquake action in different countries in the world, and the seismic design response spectrum of each country is needed when using this method. This paper summarizes base shear force method in Chinese, the U.S.A. and European seismic codes. On the basis of the same recurrence period and the same site conditions, the differences and similarities of the three standard response spectra were compared under different intensities. Taking a case study as an example, the earthquake shear forces at the bottom and between the layers are contrasted by using base shear force method in different seismic codes. Our results show that the essence of base shear force method of the three seismic codes from three different countries is similar, while the expression form and parameter settings are different.
-
Key words:
- Seismic design code /
- Response spectrum /
- Base shear force method /
- Seismic action
-
引言
建筑结构震害预测是地震工程学科的重要内容之一（于天洋等，2018；贾晗曦等，2019），其研究方法主要分为专家经验法、模糊类比法和基于机器学习相关算法组成的方法。其中，多层砖房作为国内量大面广的结构形式之一，许多学者对其进行震害预测研究，如尹之潜等（1991）基于易损性概率分析方法得到震害等级与平均折算抗剪强度的关系；欧盛（2011）基于最小二乘法对尹之潜方法中的修正系数进行修正；刘章军等（2007）使用模糊数学理论对多层砖房进行震害预测；部分学者基于反向传播神经网络（刘本玉等，2002；姜伟等，2011）和蚁群聚类径向基网络模型（杨秀萍等，2013）对多层砖房进行震害评估。
通过不同方法均可进行震害预测，但机器学习算法模型无需进行任何假设，适合内部因素错综复杂的问题，因此机器学习算法更适用于震害预测。而机器学习模型中，最关键的问题是选择合适的输入参数，即多层砖房震害影响因素。汤皓等（2006）使用灰色关联分析法对不同因素进行分析，但仅对内部关系进行分析，并未得到因素的重要性排序。集成学习算法被证明在因素重要度评估中具有良好表现（Jia等，2019）。因此，本文使用集成学习中的AdaBoost算法（Asim等，2018）对多层砖房震害影响因素进行重要度分析，以期弥补灰色关联分析的不足。
1. 影响因素与数据选取
影响多层砖房地震破坏的因素较多，主要源于以下3个方面：地震、场地和结构本身。本文选取汤皓等（2006）与姜伟等（2011）研究中的影响因素作为模型输入参数，包括房屋高度、施工质量、砂浆等级、结构合理性、砖墙面积率、房屋整体性、场地条件和地震峰值加速度（PGA）。在地震方面未选择震级和烈度是因为其与PGA具有一定关系，三者仅选择其一避免重复，且震级和烈度变化小、分级少，1次地震只出现1个震级（余震不计），不同地区烈度基本从Ⅵ度到Ⅹ度变化，仅有5个分级。
震害对应的破坏状态分为以下5个等级：基本完好、轻微破坏、中等破坏、严重破坏和倒塌，各影响因素具体分类及特征和取值见表 1，多层砖房影响因素部分数据集见表 2。
表 1 多层砖房震害影响因素特征及取值Table 1. Characteristics and influencing factors of seismic damage of multi-storey brick buildings影响因素 特征 取值 房屋高度 — — 施工质量 其他条件相同时，施工质量越好，破坏程度越低 优 10 中 8 差 6 砂浆等级 — 按实际等级取值 结构合理性 结构合理度越大，破坏程度越低 取结构抗震性能良好隶属度的值（谭克艰等，1997） 砖墙面积率 $砖墙面积率= \frac{砖墙净面积}{建筑面积}$ 按实际计算取值 房屋整体性 整体性越好，破坏程度越低 楼盖 现浇 5 预制 4 木制 3 屋盖 现浇 5 预制 4 木制 3 圈梁 有 1 构造柱 有 1 是否有地下室和筏板基础 是 1 否 0 房屋是否开裂 是 -1 否 0 场地条件 场地条件越好，破坏程度越低 Ⅰ类 10 Ⅱ类 8 Ⅲ类 6 场地条件 场地条件越好，破坏程度越低 Ⅳ类 4 地形地貌是否不利 是 -1 否 0 地下水位是否较高 是 -1 否 0 PGA PGA越大，破坏程度越高 按实际值取值 表 2 数据集Table 2. Data set序号 房屋层数 施工质量 砂浆等级/MPa 结构合理性 砖墙面积率/% 房屋整体性 场地条件 PGA/g 破坏程度 1 2 10 25 0.50 8.20 8.00 6 0.20 中等破坏 2 2 10 10 0.40 10.00 8.00 7 0.25 严重破坏 3 3 10 10 0.30 11.50 9.00 8 0.25 中等破坏 4 2 10 35 0.45 9.70 9.50 7 0.15 轻微破坏 5 4 10 25 0.36 7.60 7.50 10 0.20 中等破坏 6 2 10 25 0.55 4.30 10.00 9 0.10 轻微破坏 7 3 10 25 0.43 6.87 8.00 3 0.25 严重破坏 8 3 10 10 0.40 7.52 9.50 7 0.30 中等破坏 9 2 10 35 0.38 8.32 8.50 6 0.20 轻微破坏 10 5 10 10 0.46 11.50 8.00 8 0.25 基本完好 11 2 10 25 0.41 4.65 7.50 9 0.05 轻微破坏 12 3 10 10 0.50 9.42 12.00 8 0.20 基本完好 13 3 10 10 0.47 13.60 9.00 5 0.25 轻微破坏 14 2 10 25 0.52 10.70 8.50 7 0.20 轻微破坏 15 4 10 25 0.48 3.60 7.50 4 0.15 倒塌 16 3 10 25 0.54 6.69 8.00 8 0.25 轻微破坏 17 3 10 10 0.40 8.40 9.50 8 0.20 轻微破坏 18 4 10 25 0.45 8.50 9.46 8 0.30 严重破坏 19 2 10 25 0.47 10.30 7.00 7 0.25 中等破坏 20 4 10 25 0.47 3.23 8.00 8 0.15 轻微破坏 21 2 10 10 0.48 3.50 9.00 8 0.10 轻微破坏 22 3 10 5 0.35 8.64 8.00 7 0.20 严重破坏 23 3 10 10 0.37 6.00 8.00 7 0.20 严重破坏 24 3 10 10 0.34 9.00 10.00 7 0.25 严重破坏 25 3 10 10 0.40 9.00 7.00 7 0.30 倒塌 26 3 10 10 0.44 6.00 9.00 7 0.15 中等破坏 27 3 10 25 0.40 10.00 10.00 8 0.30 倒塌 28 3 10 25 0.40 9.00 10.00 7 0.25 倒塌 29 2 10 10 0.50 9.00 12.00 10 0.20 基本完好 30 4 10 10 0.50 9.00 9.00 9 0.15 轻微破坏 2. AdaBoost算法
AdaBoost算法是集成学习中具有代表性的一种算法，其原理是将若干个弱分类器训练集合成一个强分类器（Shin等，2009）。该算法具有强自适应性，排在前位的弱分类器算错的数据被重新计算，用于训练下个弱分类器，在这个过程中加入新的弱分类器。整个AdaBoost算法结束的标志为达到期望的错误率或达到自设的最大迭代次数，达到二者其一，迭代终止。AdaBoost算法具体迭代步骤如下：
（1）对数据集中的每个数据权值进行初始化处理。样本总数为N，将1/N的权重赋予每个数据，这些权重组成向量S。
（2）训练基本分类器。在每轮训练中，数据分为以下2种情况：当被正确分类时，加入下个训练集中时权重被自动减小；未被正确分类时，权重增加。被更新过的训练集用于训练新增的基本分类器，不断重复这个过程。AdaBoost算法为每个分类器都分配1个权重$\partial $。
（3）把每次训练后得到的基本分类器集成为1个强分类器。每轮迭代结束后，误差率$\varepsilon $低的基本分类器权重被增加，在集成的强分类器中占比更大，重要度也更高；误差率$\varepsilon $高的基本分类器权重被减小，在集成的强分类器中占比更小，重要度也更低。
具体计算公式如下：
$$ \varepsilon \rm{=}\frac{未正确分类的样本数目}{N}$$ (1) $$\partial = \frac{1}{2}\left({\frac{{1 - \varepsilon }}{\varepsilon }} \right)$$ (2) 数据被正确分类后的权重向量值为：
$${\boldsymbol{S}}_i^{(t + 1)} = \frac{{{\boldsymbol{S}}_i^{(t)}{e^\partial }}}{{sum({\boldsymbol{S}})}}$$ (3) 数据未被正确分类后的权重向量值为：
$${\boldsymbol{S}}_i^{(t + 1)} = \frac{{{\boldsymbol{S}}_i^{(t)}{e^{(- \partial)}}}}{{sum({\boldsymbol{S}})}}$$ (4) 式中，i是弱分类器，t是迭代次数。
3. 评估模型训练及结果
3.1 AdaBoost算法训练过程
本模型直接调用sklearn库中的AdaBoostClassifier分类器，默认使用CART决策树。分类算法选择基于类别概率、效果更好的SAMME.R算法，具体参数选择为：max_depth=2，min_samples_split=20，min_samples_leaf=5，n_estimators=300，learning_rate=0.5。
n_estimators表示采用的最大弱分类器个数，分类器过多模型易出现过拟合情况，但太少会出现欠拟合情况。learning_rate表示学习率，即整个模型迭代速度，学习率越小，迭代次数越多，该值下降会影响模型精度。n_estimators和learning_rate对整个模型的影响最大，因此进行重点调整，其取值不同时的系统分类精度如图 1。由图 1可知，当n_estimators和learning_rate分别为300和0.5时，模型精度最高。同时也可看出AdaBoost算法精度较高。选用的模型算法除须保证精度外，还须确保模型的稳定性，每次计算结果不能相差太大。模型3次计算结果如图 2，由图 2可知，每次计算不同参数的重要度虽不相同，但排序基本一致。这证明AdaBoost算法精度和稳定性均较好，适用于多层砖房震害影响因素评估。
图 1 不同参数下的模型精度Figure 1. Model accuracy under different parameters importance of influencing factors of seismic fatality3.2 AdaBoost算法训练结果
模型计算结果即为每个因素的重要度，AdaBoost算法训练结果见表 3，并列出灰色关联分析法中的排序。由表 3可知，多层砖房结构合理性的重要度最高，约占1/4；PGA的重要度仅次于结构合理性，约占1/5；砖墙面积率与场地条件的重要度相近；结构合理性、PGA、砖墙面积率与场地条件的重要度占比超过80%，远超其他因素，可见房屋整体性、砂浆等级、房屋高度、施工质量的重要性较低。以上分析仅针对AdaBoost算法训练结果，并未分析数据本身。
表 3 影响因素重要度排序及与灰色关联分析法结果的对比Table 3. Ranking of factors of influence factors and comparison with results of grey correlation analysis method影响因素 重要度（AdaBoost结果） 灰色关联分析法中的排名 结构合理性 0.266878512 4 PGA 0.196731755 1 砖墙面积率 0.170314083 2 场地条件 0.168439716 5 房屋整体性 0.089834515 8 砂浆等级 0.067375887 6 房屋高度 0.040425532 7 施工质量 0.000000000 3 从算法原理和数据本身来看，分级越多的影响因素重要度越高。在本模型中，施工质量全部为优，因此默认分级只有一级，数据无变化，算法默认重要度为0。但在实际工程中，施工质量对震害程度的影响很大，因此灰色关联分析法中其排名为第3。随着数据量的增加，此类问题逐渐减少。
AdaBoost算法训练结果与灰色关联分析法的结果相差较小，只有结构合理性和房屋整体性的排名出入较大。结构合理性在本模型中至关重要，但在灰色关联分析法结果中仅排在中等位置。灰色关联分析法中，如果某影响因素和破坏程度呈正相关性或负相关性，则模型自动认为此因素较重要，模型结果反映各影响因素对震害相关性强弱的顺序。2种算法内部机理不同，灰色关联分析法更注重影响因素与破坏程度的趋势关系，而AdaBoost算法更注重影响因素本身对破坏程度的贡献度及整个模型的自适应性，通过不断迭代筛选最优解。因此，对于重要度排序而言，AdaBoost算法更有优势。综上所述，多层砖房结构合理性更应被重视，而房屋整体性可排在次要位置。
通过以上对比分析可知，AdaBoost算法在处理高度非线性问题上更有优势，训练结果更接近震害经验，调节合适的参数后，其精度高达96%，且稳定性较高。因此，AdaBoost算法在多层砖房震害评估中具有适用性。灰色关联分析法作为特征重要度评估的经典方法，有其可取之处，在特征之间的关系较明晰、数据量较少的情况下同样适用。
4. 结论
本文基于AdaBoost算法对多层砖房震害影响因素重要度进行评估，得到不同影响因素的重要度排序，并将结果与灰色关联分析法进行比较。其中，结构合理性、PGA、砖墙面积率和场地条件最重要，在震害预测影响因素选择中应着重考虑。具体结论如下：
（1）AdaBoost算法具有良好的稳定性和精度，适用于多层砖房震害影响因素重要度评估。
（2）房屋结构合理性在诸多影响因素中排名第1，重要度约占1/4，可见其重要性，设计建造时应着重考虑；地震峰值加速度排名第2，重要度约占1/5，设计阶段应予以高度重视。
（3）砖墙面积率与场地条件的重要度相对较高，而房屋整体性、砂浆等级、房屋高度与施工质量的重要度较低，其中施工质量重要度排序与灰色关联分析法差异最大，这是数据有限的原因。
此外，多层砖房震害影响因素远不止文中所列，由于时间和数据限制，本文仅提出探索性模型，仍需进一步拓展影响因素、补充数据，将数据质量较差的例子删除，以期提升模型准确度和适用性，以便推广使用。
-
图 1 中国规范地震影响系数曲线
α：地震影响系数；αmax：地震影响系数最大值；η1：直线下降段的下降斜率调整系数；γ：衰减指数；Tg：特征周期（s）；η2：阻尼调整系数；T：结构自振周期（s）
Figure 1. Seismic effect coefficient curve in the Chinese code
图 4 不同烈度下中、美、欧抗震规范反应谱对比
Figure 4. Comparison of seismic response spectra among China, the U.S.A. and Europe under different intensities
图 5 中、美、欧基底剪力系数Cs对比
Figure 5. Comparison of base shear coefficient among China, America and Europe
图 6 中、美、欧竖向分配系数Cvi对比
Figure 6. Comparison of vertical distribution coefficient Cvi among China, the U.S.A. and Europe
图 7 中、美、欧层间地震剪力对比
Figure 7. Comparison of seismic shear between layers among China, the U.S.A. and Europe
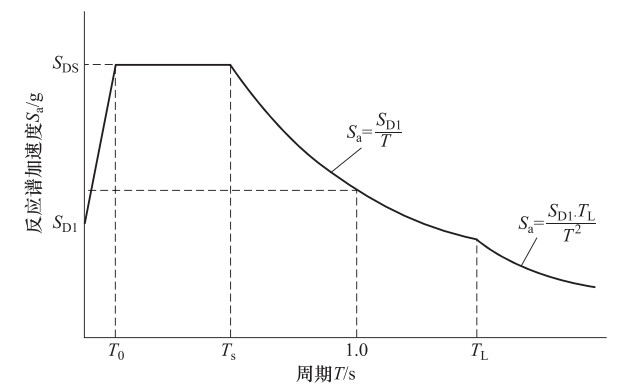
表 1 水平地震影响系数最大值αmax
Table 1. The coefficients of the maximum horizontal seismic influence αmax
地震影响 Ⅵ Ⅶ Ⅷ Ⅸ 多遇地震 0.04 0.08（0.12） 0.16（0.24） 0.32 罕遇地震 0.28 0.50（0.72） 0.90（1.20） 1.40 注：括号中的数值分别用于设计基本地震加速度为0.15g和0.30g的地区。 
下载: 导出CSV
表 2 特征周期值Tg(s)
Table 2. The value of characteristic period Tg(s)
设计地震分组 场地类别 Ⅰ0 Ⅰ1 Ⅱ Ⅲ Ⅳ 第一组 0.20 0.25 0.35 0.45 0.65 第二组 0.25 0.30 0.40 0.55 0.75 第三组 0.30 0.35 0.45 0.65 0.90
下载: 导出CSV
表 3 场地系数Fa
Table 3. Site Coefficient Fa
场地类别 短周期（0.2s）最大考虑地震反应谱加速度参数 Ss≤0.25 Ss=0.5 Ss=0.75 Ss=1.0 Ss≥1.25 A 0.8 0.8 0.8 0.8 0.8 B 1.0 1.0 1.0 1.0 1.0 C 1.2 1.2 1.1 1.0 1.0 D 1.6 1.4 1.2 1.1 1.0 E 2.5 1.7 1.2 0.9 0.9 F 场地反应分析按特殊场地的地震动确定方法执行 注：对处于中间的Ss，场地系数Fa可按线性插入法取值。
下载: 导出CSV
表 4 场地系数Fv
Table 4. Site Coefficient Fv
场地类别 周期1s最大考虑地震反应谱加速度参数 S1≤0.1 S1=0.2 S1=0.3 S1=0.4 S1≥0.5 A 0.8 0.8 0.8 0.8 0.8 B 1.0 1.0 1.0 1.0 1.0 C 1.7 1.6 1.5 1.4 1.3 D 2.4 2.0 1.8 1.6 1.5 E 3.5 3.2 2.8 2.4 2.4 F 场地反应分析按特殊场地的地震动确定方法执行 注：对处于中间的S1，场地系数Fv可按线性插入法取值。
下载: 导出CSV
表 5 Ⅰ类弹性反应谱的各参数值
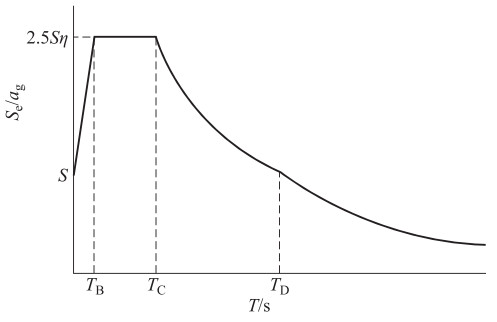
Table 5. Values of the parameters of the recommended TypeⅠelastic response spectra
场地类别 S TB/s TC/s TD/s A 1.0 0.15 0.4 2.0 B 1.2 0.15 0.5 2.0 C 1.15 0.20 0.6 2.0 D 1.35 0.20 0.8 2.0 E 1.4 0.15 0.5 2.0
下载: 导出CSV
表 6 Ⅱ类弹性反应谱的各参数值
Table 6. Values of the parameters of the recommended TypeⅡelastic response spectra
场地类别 S TB/s TC/s TD/s A 1.0 0.05 0.25 1.2 B 1.35 0.05 0.25 1.2 C 1.5 0.10 0.25 1.2 D 1.8 0.10 0.30 1.2 E 1.6 0.05 0.25 1.2
下载: 导出CSV
表 7 中、美、欧抗震设防目标
Table 7. Comparison of seismic fortification targets from China, the U.S.A. and Europe
国家或地区 设防目标 50年内超越概率 重现期 中国 小震不坏 63.2% 50年 中震可修 10% 475年 大震不倒 2%—3% 约2000年 美国 倒塌概率极小 2% 2500年 欧洲 破坏极限要求 40% 50年 不倒塌要求 10% 475年
下载: 导出CSV
表 8 中国规范中震对应的水平地震影响系数最大值
Table 8. The maximum horizontal seismic influence coefficient under medium earthquake in the Chinese code
烈度 Ⅵ（0.05g） Ⅶ（0.10g） Ⅶ（0.15g） Ⅷ（0.20g） Ⅷ（0.30g） Ⅸ（0.40g） αmax 0.11 0.22 0.33 0.44 0.67 0.89
下载: 导出CSV
表 9 美国规范的谱加速度参数（C类场地）
Table 9. Spectral acceleration parameters in the U.S.A. standard(Site class C)
参数名称 Ⅵ（0.05g） Ⅶ（0.10g） Ⅶ（0.15g） Ⅷ（0.20g） Ⅷ（0.30g） Ⅸ（0.40g） SS 0.38 0.65 1.01 1.13 1.38 1.58 S1（第一组） 0.09 0.16 0.22 0.25 0.33 0.39 S1（第二组） 0.11 0.18 0.26 0.30 0.39 0.48 S1（第三组） 0.12 0.21 0.30 0.35 0.46 0.55
下载: 导出CSV
表 10 欧洲规范的设计地面加速度ag值（B类场地）（g）
Table 10. The design ground acceleration ag in the European standard(Site class B)(g)
反应谱类别 Ⅵ（0.05g） Ⅶ（0.10g） Ⅶ（0.15g） Ⅷ（0.20g） Ⅷ（0.30g） Ⅸ（0.40g） Ⅰ类 0.042 0.083 0.125 0.167 0.250 0.333 Ⅱ类 0.037 0.074 0.111 0.148 0.222 0.296
下载: 导出CSV
表 11 顶部附加地震作用系数
Table 11. Additional seismic action coefficient at the top
Tg/s T1＞1.4Tg T1≤1.4Tg Tg≤0.35 0.08T1+0.07 0 0.35＜Tg≤0.55 0.08T1+0.01 Tg＞0.55 $ 0.08{\tilde T_1}0.02$
下载: 导出CSV
表 12 中、美、欧规范基底剪力系数
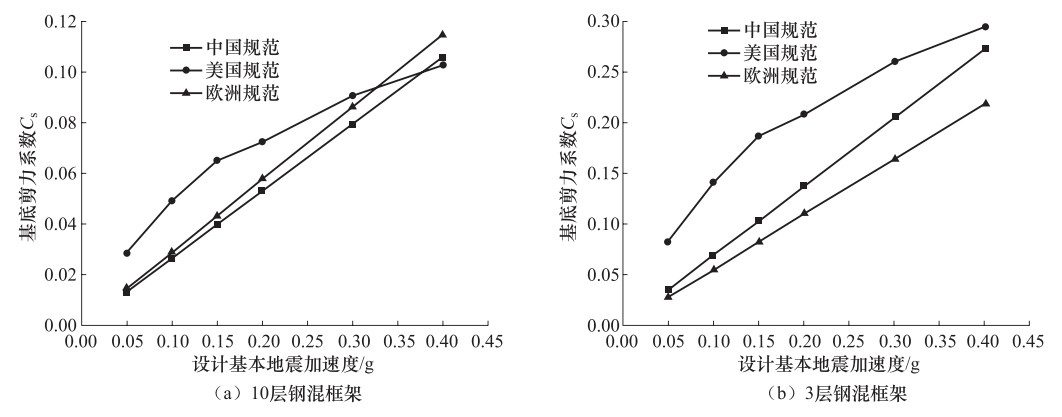
Table 12. Base shear coefficient in the standard of China, the U.S.A. and Europe
比较项 中国 美国 欧洲 底部地震剪力（kN） ${F_{{\rm{Ek}}}} = {\alpha _1}{G_{{\rm{eq}}}} = 0.85{\alpha _1}W$ $V = {C_{\rm{s}}}W$ ${F_{\rm{b}}} = {S_{\rm{d}}}({T_1})\; \cdot \; m\lambda = \lambda {S_{\rm{d}}}({T_1}){\rm{ }}W/{\rm{g}}$ 基底剪力系数Cs ${C_{\rm{s}}} = 0.85{\alpha _1}$ ${C_{\rm{s}}} = \frac{{{S_{{\rm{DS}}}}}}{{(R/{I_{\rm{e}}})}}$ ${C_{\rm{s}}} = \lambda {S_{\rm{d}}}({T_1})/{\rm{g}}$ 注：g为重力加速度。
下载: 导出CSV
表 13 中、美、欧规范基本自振周期T1（10层钢混框架）
Table 13. Basic natural vibration period T1 in the standard of China, the U.S.A. and Europe (A 10-storey reinforced concrete frame)
比较项 中国 美国 欧洲 基本自振周期T估算公式 $T = 0.1n$ $T = {C_t}h_n^x$ $T = {C_t}{H^{3/4}}$ 相关参数 $n = 10$ ${C_t} = 0.0466$
$x = 0.9$
${h_{10}} = 4.2 + 3.6 \times 9 = 36.6\left({\rm{m}} \right)$${C_t} = 0.075$
$H = 4.2 + 3.6 \times 9 = 36.6\left({\rm{m}} \right)$10层钢混框架的基本自振周期T1（s） ${T_1} = 0.1 \times 10 = 1$ ${T_1} = 0.0466 \times {36.6^{0.9}} = 1.190$ ${T_1} = 0.075 \times {36.6^{3/4}} = 1.116$
下载: 导出CSV
表 14 中国规范的基底剪力系数Cs（10层钢混框架）
Table 14. Base shear coefficient Cs in the Chinese standard(A 10-storey reinforced concrete frame)
参数 Ⅵ度（0.05g） Ⅶ度（0.10g） Ⅶ度（0.15g） Ⅷ度（0.20g） Ⅷ度（0.30g） Ⅸ度（0.40g） αmax 0.04 0.08 0.12 0.16 0.24 0.32 ${\alpha _1} = {\left({\frac{{{T_g}}}{{{T_1}}}} \right)^{0.9}}{\alpha _{\max }}$ 0.016 0.031 0.047 0.062 0.093 0.124 ${C_{\rm{s}}} = 0.85{\alpha _1}$ 0.013 0.026 0.040 0.053 0.079 0.106 注：根据设计地震分组为第1组，场地类别为Ⅱ类，查得特征周期值Tg=0.35s。
下载: 导出CSV
表 15 美国规范的基底剪力系数Cs（10层钢混框架）
Table 15. Base shear coefficient Cs in the U.S.A. standard(A 10-storey reinforced concrete frame)
参数 Ⅵ度（0.05g） Ⅶ度（0.10g） Ⅶ度（0.15g） Ⅷ度（0.20g） Ⅷ度（0.30g） Ⅸ度（0.40g） Ss 0.38 0.65 1.01 1.13 1.38 1.58 Fa 1.2 1.14 1 1 1 1 S1 0.09 0.16 0.22 0.25 0.33 0.39 Fv 1.7 1.64 1.58 1.55 1.47 1.41 ${S_{{\rm{DS}}}} = 2/3{F_a}{S_{\rm{s}}}$ 0.304 0.494 0.673 0.753 0.920 1.053 ${S_{{\rm{D}}1}} = 2/3{F_v}{S_1}$ 0.102 0.175 0.232 0.258 0.323 0.367 ${T_0} = 0.2{S_{{\rm{D}}1}}/{S_{{\rm{DS}}}}$（s） 0.067 0.071 0.069 0.069 0.070 0.070 ${T_{\rm{s}}} = {S_{{\rm{D}}1}}/{S_{{\rm{DS}}}}$（s） 0.336 0.354 0.344 0.343 0.352 0.348 TL（s） 4 4 4 4 4 4 ${C_{\rm{s}}} = \frac{{{S_{{\rm{DS}}}}}}{{(R/{I_{\rm{e}}})}}$ 0.101 0.165 0.224 0.251 0.307 0.351 $\frac{{{S_{{\rm{D}}1}}}}{{T(R/{I_{\rm{e}}})}}$ 0.029 0.049 0.065 0.072 0.091 0.103 $0.044{S_{{\rm{DS}}}}{I_{\rm{e}}}$ 0.013 0.022 0.030 0.033 0.040 0.046 Cs最终值 0.029 0.049 0.065 0.072 0.091 0.103 注：规范中查得反应谱修正系数R=3，重要性系数Ie=1.0。
下载: 导出CSV
表 16 欧洲规范的基底剪力系数Cs（10层钢混框架）
Table 16. Base shear coefficient Cs in the European standard(A 10-storey reinforced concrete frame)
参数 Ⅵ度（0.05g） Ⅶ度（0.10g） Ⅶ度（0.15g） Ⅷ度（0.20g） Ⅷ度（0.30g） Ⅸ度（0.40g） ag/g 0.042 0.083 0.125 0.167 0.250 0.333 ${S_{\rm{d}}}({T_1})/{\rm{g}} = {a_{\rm{g}}}S\frac{{2.5}}{q}\left({\frac{{{T_{\rm{C}}}}}{{{T_1}}}} \right)/{\rm{g}}$ 0.014 0.029 0.043 0.058 0.086 0.115 βag/g 0.008 0.017 0.025 0.033 0.050 0.067 ${C_{\rm{s}}} = \lambda {S_{\rm{d}}}({T_1})/{\rm{g}}$ 0.014 0.029 0.043 0.058 0.086 0.115 注：规范中查得场地土系数S=1.2，TB=0.15s，TC=0.5s，TD=2.0s，β=0.2；性能系数q=3.9；因T1＞2TC，故λ=1.0。
下载: 导出CSV
表 17 中、美、欧规范基本自振周期T1（3层钢混框架）
Table 17. Basic natural vibration period T1 in the standard of China, the U.S.A. and Europe (A 3-storey reinforced concrete frame)
比较项 中国 美国 欧洲 基本自振周期T估算公式 $T = 0.1n$ $T = {C_t}h_n^x$ $T = {C_t}{H^{3/4}}$ 相关参数 $n = 3$ ${C_t} = 0.0466$
$x = 0.9$
${h_3} = 4.2 + 3.6 \times 2 = 11.4\left({\rm{m}} \right)$${C_t} = 0.075$
$H = 4.2 + 3.6 \times 2 = 11.4\left({\rm{m}} \right)$3层钢混框架的基本自振周期T1（s） ${T_1} = 0.1 \times 3 = 0.3$ ${T_1} = 0.0466 \times {11.4^{0.9}} = 0.416$ ${T_1} = 0.075 \times {11.4^{3/4}} = 0.465$
下载: 导出CSV
表 18 中国规范的基底剪力系数Cs（3层钢混框架）
Table 18. Base shear coefficient Cs in the Chinese standard(A 3-storey reinforced concrete frame)
参数 Ⅵ度（0.05g） Ⅶ度（0.10g） Ⅶ度（0.15g） Ⅷ度（0.20g） Ⅷ度（0.30g） Ⅸ度（0.40g） αmax 0.04 0.08 0.12 0.16 0.24 0.32 ${\alpha _1} = {\alpha _{\max }}$ 0.04 0.08 0.12 0.16 0.24 0.32 ${C_{\rm{s}}} = 0.85{\alpha _1}$ 0.034 0.068 0.102 0.136 0.204 0.272
下载: 导出CSV
表 19 美国规范的基底剪力系数Cs（3层钢混框架）
Table 19. Base shear coefficient Cs in the U.S.A. standard(A 3-storey reinforced concrete frame)
参数 Ⅵ度（0.05g） Ⅶ度（0.10g） Ⅶ度（0.15g） Ⅷ度（0.20g） Ⅷ度（0.30g） Ⅸ度（0.40g） ${C_{\rm{s}}} = \frac{{{S_{{\rm{DS}}}}}}{{(R/{I_{\rm{e}}})}}$ 0.101 0.165 0.224 0.251 0.307 0.351 $\frac{{{S_{{\rm{D}}1}}}}{{T(R/{I_{\rm{e}}})}}$ 0.082 0.140 0.186 0.207 0.259 0.294 $0.044{S_{{\rm{DS}}}}{I_{\rm{e}}}$ 0.013 0.022 0.030 0.033 0.040 0.046 Cs最终值 0.082 0.140 0.186 0.207 0.259 0.294 注：其它相关参数与楼层或房屋高度无关，均与10层钢混框架的参数相同，故该表未列出。
下载: 导出CSV
表 20 欧洲规范的基底剪力系数Cs（3层钢混框架）
Table 20. Base shear coefficient Cs in the European standard(A 3-storey reinforced concrete frame)
参数 Ⅵ度（0.05g） Ⅶ度（0.10g） Ⅶ度（0.15g） Ⅷ度（0.20g） Ⅷ度（0.30g） Ⅸ度（0.40g） ag/g 0.042 0.083 0.125 0.167 0.250 0.333 ${S_{\rm{d}}}\left({{T_1}} \right)/{\rm{g}} = {a_{\rm{g}}}S\frac{{2.5}}{q}/{\rm{g}}$ 0.032 0.064 0.096 0.128 0.192 0.256 ${C_{\rm{s}}} = \lambda {S_{\rm{d}}}\left({{T_1}} \right)/{\rm{g}}$ 0.027 0.054 0.082 0.109 0.163 0.218 注：因T1＜2TC，且层数超过2层，故λ=0.85；其它相关参数均与10层钢混框架的参数相同。
下载: 导出CSV
表 21 中、美、欧规范竖向分配系数Cvi
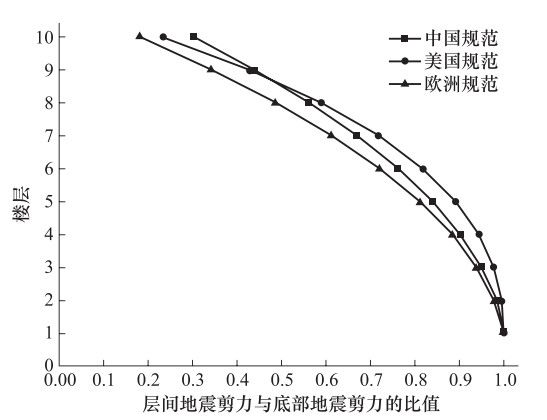
Table 21. The vertical distribution coefficient Cvi in the standard of China, the U.S.A. and Europe
比较项 中国 美国 欧洲 楼层水平地震作用 ${F_i} = \frac{{{G_i}{H_i}}}{{\sum\limits_{j = 1}^n {{G_j}{H_j}} }}{F_{{\rm{Ek}}}}(1 - {\delta _n})$ ${F_x} = {C_{vx}}V = \frac{{{w_x}h_x^k}}{{\sum\limits_{i = 1}^n {{w_i}h_i^k} }}V$ 动力分析方法：${F_i} = {F_{\rm{b}}}\frac{{{s_i}{m_i}}}{{\sum {s_j}{m_j}}}$ 近似计算方法：${F_i} = {F_{\rm{b}}}\frac{{{z_i}{m_i}}}{{\sum {z_j}{m_j}}}$ 竖向分配系数Cvi ${C_{vi}} = \frac{{{G_i}{H_i}}}{{\sum\limits_{j = 1}^n {{G_j}{H_j}} }}(1 - {\delta _n})$ ${C_{vi}} = \frac{{{w_i}h_i^k}}{{\sum\limits_{j = 1}^n {{w_j}h_j^k} }}$ 动力分析方法：${C_{vi}} = \frac{{{s_i}{m_i}}}{{\sum {s_j}{m_j}}}$ 近似计算方法：${C_{vi}} = \frac{{{z_i}{m_i}}}{{\sum {z_j}{m_j}}}$ 相关参数 ${\delta _n} = 0.08{T_1} + 0.07 = 0.15$ $k = 1.69$
下载: 导出CSV
-
曹继涛, 2013.中美欧规范钢筋混凝土框架结构抗震设计对比研究.西安: 西安建筑科技大学. 胡聿贤, 2006.地震工程学.北京:地震出版社. 蒋志楠, 2010.我国建筑抗震规范中部分条款的演变及与欧美规范的对比探讨.哈尔滨: 中国地震局工程力学研究所. 李国强, 李杰, 陈素文等, 2014.建筑结构抗震设计.4版.北京:中国建筑工业出版社. 李慧, 2011.中、美、欧、日建筑抗震规范地震作用对比研究.哈尔滨: 哈尔滨工业大学. 罗开海, 王亚勇, 2006.中美欧抗震设计规范地震动参数换算关系的研究.建筑结构, 36(8):103-107. http://www.cnki.com.cn/Article/CJFDTOTAL-JCJG200608027.htm 孙景江, 2006.建筑结构抗震研究若干基本问题概述及讨论.震灾防御技术, 1(2):87-96. doi: 10.3969/j.issn.1673-5722.2006.02.001 王亚勇, 郭子雄, 吕西林, 1999.建筑抗震设计中地震作用取值——主要国家抗震规范比较.建筑科学, 15(5):36-39, 55. http://www.cnki.com.cn/Article/CJFDTOTAL-JZKX199905016.htm 叶列平, 方鄂华, 2009.关于建筑结构地震作用计算方法的讨论.建筑结构, 39(2):1-7. doi: 10.3321/j.issn:1000-6869.2009.02.001 中华人民共和国住房和城乡建设部, 2012.建筑结构荷载规范(GB 50009-012).北京:中国建筑工业出版社. 中华人民共和国住房和城乡建设部, 中华人民共和国国家质量监督检验检疫总局, 2010.建筑抗震设计规范(GB 50011-2010).北京:中国建筑工业出版社. American Society of Civil Engineers, 2010. ASCE/SEI7-10 Minimum design loads for buildings and other structures. Reston, VA: American Society of Civil Engineers. European committee for Standardization, 2004. Eurocode 8: Design of structures for earthquake resistance-Part 1: General rules, seismic actions and rules for buildings. Brussels: European Committee for Standardization. -
下载:
点击查看大图
计量
- 文章访问数: 128
- HTML全文浏览量: 252
- PDF下载量: 37
- 被引次数: 0
