TMD Control and Analysis of Cranes under Earthquake
-
摘要: 虽然建筑施工周期内地震致险概率并不高,但是由于塔吊使用极其广泛,塔吊致险概率并不低于建筑结构,故而针对塔吊的减震措施亟需研究。本文以某实际超高层建筑施工使用的动臂式塔吊为研究对象,根据塔吊自身和附着于超高层建筑后的动力特性,研究了TMD装置的不同方案对塔吊地震响应的控制效果。结果表明:① TMD装置对于属于高耸结构的塔吊减震控制非常有效;② 设置在塔身顶部的双向TMD不仅可以有效减小塔身偏摆,也可以间接有效地控制起重臂的竖向振动;③ 由于超高层建筑-塔吊结构高阶振型影响明显,此TMD装置对塔吊塔身控制效果不稳定,但对于起重臂仍能起到良好的控制作用,故而此TMD装置可以使用在附着在超高层建筑上的施工塔吊,对于塔身的振动控制仍需进一步研究。Abstract: Due to the universality and the characteristics of cranes, cranes risk probability is not lower than building structure, so It's nessesary to research the crane shock absorption measures although during the construction period, the earthquake risk probability is not high. Based on its dynamic characteristics of cranes and attached to the high-rise building, the dynamic characteristics of the TMD of different schemes on the earthquake response of the tower crane control effect. The results show that:① TMD for tall structures of cranes seismic vibration control is effective. ② In order to control cranes tower deflection of lateral and vertical vibration boom, set up in the tower at the top of the two-way TMD can't only effectively reduce the deflection of the tower, but also can indirectly effectively control the crane boom vertical vibration. ③ The TMD device has a good control effect on the tower crane body, but it also has a good control effect for the boom, so the TMD device can be used in the super high-rise building on the construction tower crane, but for the tower body's vibration control still need further study.
-
Key words:
- Cranes /
- TMD /
- Super high-rise building /
- Analysis on seismic response
-
引言
随着我国经济的快速发展,高层或超高层建筑得以大量兴建。虽然建筑结构的抗震安全成为大家普遍关心的问题,但在施工过程中大型起重设备的抗震问题却尚未引起足够重视。在我国,通常认为施工过程属于“短暂设计状况”,而塔式起重机(塔吊)是建筑施工的重要设备,地震本属于小概率事件,其作用在起重设备上的可能性更是微乎其微,不必进行抗震设计。然而,相关的灾害事故并不鲜见。例如,在日本神户地震中,大量港口起重机和塔吊遭受不同程度的损坏(章崇任,2007)。在汶川地震中,许多建筑工地的塔吊受损甚至倒塌(李云岭等,2011)。虽然在施工周期内地震致险概率不高,但是塔吊使用极其广泛,是每个建筑工程不可或缺的起重设备,而且塔吊是周转使用的,周转期限一般为20年甚至更长,因此致险概率也相应增加。因此,对塔吊在运营状态下的抗震性能和安全性进行研究具有重要的工程意义。
从国外的研究现状来看,新西兰、日本等国家已有塔式起重机的抗震设计守则(Otani等,2002;吉見雅行等,2004),日本研发了安装在塔吊上的抵御地震和强风灾害的减震装置。而我国在这方面还尚无规范,制约了施工设备抗震安全性的发展。
从国内研究现状来看,已有部分学者就塔机的地震响应进行了研究探索。针对地震荷载造成塔式起重机受损的问题,采用时程分析法和有限元法对塔式起重机进行三向地震作用下的动态响应研究,结果表明:塔臂受地震波作用影响最为显著,竖向地震波是造成塔臂断裂的主要原因(吉军等,2009)。王武奇(2009)利用ANSYS软件的瞬态分析模块对25m和36m这两种高度的QTL630型塔式起重机进行地震作用下的时程分析,得出塔式起重机顶点的最大位移随高度增加而增大的结论。谢瑞等(2009)针对汶川地震西安市塔吊受损情况进行了分析,得到其主要破坏形式及特点,并分析不同部位出现损坏的原因。从上述研究中发现,在建筑施工过程中,塔吊抗震安全性不足,一旦发生地震,将导致严重的后果。另外,虽然部分学者研究发现塔吊抗震性能低,地震作用会造成塔吊受损及破坏(何银晖等,2012),然而,关于起重设备减震措施的研究目前比较薄弱,需要进行深入探索。
为研究塔吊地震响应的减震措施,本文以某动臂式塔吊为研究对象,建立有限元模型,通过Sadek法设计调谐质量阻尼器(Tuned Mass Damper,简称TMD)最优参数,通过TMD控制塔身顶部位移,达到间接控制起重臂位移响应的目的。选用多条地震加速度记录研究不同TMD方案对塔吊的地震响应控制结果,同时将它应用于超高层建筑中观察其地震响应控制效果。
1. 施工塔吊简介与动力特性分析
为了研究地震作用下塔吊的动力性能,本文以某型号的动臂式塔吊为研究对象,如图 1所示。此类塔吊特点是依靠起重臂升降实现变幅,能充分利用起重臂的有效高度(卿龙邦等,2013)。另外,此类塔吊适用于高层或是超高层建筑施工中,当建筑施工至低层时,动臂式塔吊附着于地面进行施工,随着建筑施工层数增加,动臂式塔吊附着在核心筒内部进行爬升,自身重量全部由建筑承担(阎红霞等,2010;姚佳琳等,2014),且在施工过程中,超高层建筑主体结构的层数不断增加,其自身的动力特性也不断变化。
动臂式塔吊主要由起重臂、机室、A形架、平衡臂、塔身、支撑结构和回转机构等组成(卿龙邦等,2013)。基于SAP2000建立了该塔吊的三维模型,根据该塔吊的实际杆件构造进行建模分析,以塔身高54m,起重臂长60m,起重臂角度为30°为例建模,探究此塔吊固着于地面和附着于建筑后整体结构的动力特性。
采用SAP2000有限元软件进行建模分析,在建模时结合动臂式塔吊的结构特点,对模型进行简化,使模型更能贴近实际的塔吊,并且能够准确地反映结构的受力特点和变形。通过调整材料的密度和弹性模量调节模型的质量,使其最终质量贴近实际塔吊。塔吊模型的边界条件反映其在施工中的实际安装情况,标准节连接之间构造复杂,所以起连接作用的耳板、销轴和螺栓等在建模时不予考虑,视为刚接;塔身通过支撑框和牛腿支撑梁等与建筑物筒体结构进行高强螺栓锚固连接,视为刚接;塔身固定于地面时,塔身与地面通过高强螺栓相连,视为固接。塔吊模型所受荷载的类型、大小、方向和位置与动臂式塔吊在实际工作中承受的荷载一致。
通过比较周期和振型可以看出,动臂式塔吊有限元模型可以较好地反映原结构的动力特性,塔吊结构周期如表 1所示。
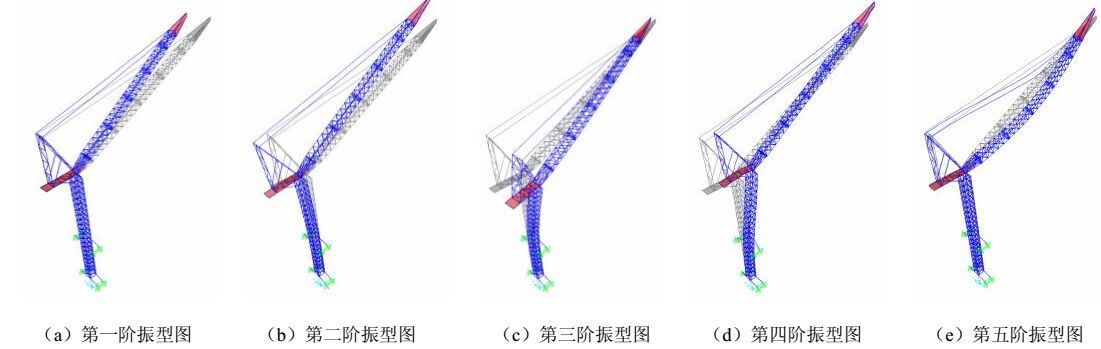
表 1 结构周期和振型质量参与系数Table 1. Natural period and participation mass ratio of structures模态/阶 周期/s UX UY SX SY 1 6.24 5.40E-07 0.3829 5.4E-07 0.3829 2 4.72 0.3353 8.31E-05 0.3353 0.3830 3 2.61 0.0003 0.3923 0.3356 0.7753 4 2.14 0.5070 4.32E-05 0.8426 0.7753 5 0.92 7.57E-05 4.46E-05 0.8427 0.7754 注:UX、UY分别为某一振型在X、Y方向的质量参与比;SX、SY为X、Y方向的质量参与比振型顺序累积值。 动臂式塔吊有限元模型前5阶振型如图 2所示,分别是:① 第一阶:起重臂在回转平面内绕回转中心扭转;② 第二阶:塔机上部整体后倾,上段塔身向后弯曲,起重臂随塔身变形而向上偏摆;③ 第三阶:塔机上部整体侧向偏摆,上段塔身侧向弯曲;④ 第四阶:塔机上部整体向前偏摆,上段塔身向前弯曲;⑤ 第五阶:塔机塔身略微向前倾斜,起重臂中部弯曲变形。总体而言,振动过程中塔身和起重臂刚度较小,变形较大,相对而言,平衡臂刚度比较大,变形较小。
塔吊结构以前4阶振型为主,其中与起重臂夹角为30°的水平方向为X向,垂直于X的水平方向为Y向,垂直于地面的方向为Z向。第一阶振型Y向质量参与系数为38.29%,绕Z轴质量参与系数为98.38%,结构以绕Z轴转动为主。第二阶振型X向质量参与系数为33.53%,结构以X向平动为主。第三阶振型Y向质量参与系数为39.23%,结构以Y向平动为主。第四阶振型X向质量参与系数为50.70%,结构以X向平动为主。
2. TMD参数的选取
土木工程领域,结构振动控制可以分为被动控制、主动控制和半主动控制3种。高层建筑或是高耸结构本身体型巨大,主动控制所需的外加能源比较大,控制机构设计复杂难以实施(Mijailović等,2009;Wang等,2014;Longarini等,2014;刘小萌等,2015)。而与主动控制相比,被动控制造价低廉、构造简单而且维修方便(Kim等,2013)。
对于属于高耸结构的塔吊来说,在不影响塔吊正常施工且方便安装的前提下,为了使其顶端具有更好的减震效果,选取被动控制调谐质量阻尼器(TMD)作为控制塔吊地震响应的方法。
根据塔吊的破坏形式和结构特点,其起重臂较为薄弱,且在施工过程中仰角与吊重不断改变。本文采用控制塔身顶部位移的方法间接控制塔吊起重臂端部的位移。
2.1 TMD参数设计方法
TMD系统是由弹簧、阻尼器以及质量块组成的振动系统。系统中的质量块用来提供惯性力,以此减少被控结构的振动(闫维明等,2010;张俊卫,2014)。
Sadek等(1997)设计并发明了一种调谐质量阻尼器(TMD)最优参数的选择方法,它可以有效减少结构的地震响应。此种方法基于给定的质量比、频率比和阻尼比等参数计算TMD最优参数,使TMD系统能吸收更多的能量,进而减轻结构的振动响应。此种计算方法不仅适用于单自由度结构,还适用于多自由度结构,其最大的优势在于可用在高层建筑减震上,能够显著减少高层建筑的地震响应。因此,对于塔式起重机这种高耸结构来说,采用此种方法减震较为适宜。
采用Sadek等(1997)TMD最优参数设计步骤如下:
(1)根据经验,设定TMD的质量为塔式起重机结构的5%—10%,使其在合理的范围内:
$$\mu =\frac{{{m}_{\text{TMD}}}}{m_{1}^{*}}=0.05$$ (1) 其中, $m_{1}^{*}$ 为塔式起重机一阶振型质量; ${{m}_{\text{TMD}}}$ 为TMD的质量; $\mu $ 为TMD质量比。
(2)计算TMD对应结构第一振型的最佳频率比:
$$\delta =\frac{1}{1+\mu }(1-\varepsilon _{1}^{\text{*}}\sqrt{\frac{\mu }{1+\mu }})$$ (2) 其中, $\varepsilon _{1}^{\text{*}}$ 为塔式起重机一阶振型阻尼比;δ为TMD与振型频率最佳频比。
(3)计算TMD最佳阻尼比 ${{\varepsilon }_{\text{TMD}}}$ :
$${{\varepsilon }_{\text{TMD}}}=\frac{\varepsilon _{1}^{\text{*}}}{1+\mu }+\sqrt{\frac{\mu }{1+\mu }}$$ (3) (4)计算TMD最佳频率 ${{\omega }_{\text{TMD}}}$ :
$${{\omega }_{\text{TMD}}}=\delta \times \omega _{1}^{\text{*}}$$ (4) 其中, $\omega _{1}^{\text{*}}$ 为塔式起重机一阶频率。
(5)计算TMD的刚度 ${{K}_{\text{TMD}}}$ 和阻尼 ${{C}_{\text{TMD}}}$ :
$${{K}_{\text{TMD}}}={{m}_{\text{TMD}}}\times \omega _{\text{TMD}}^{2}$$ (5) $${{C}_{\text{TMD}}}=2{{m}_{\text{TMD}}}\times {{\omega }_{\text{TMD}}}\times {{\varepsilon }_{\text{TMD}}}$$ (6) 本章主要提供思路分析和计算方法,实际应用时因施工状态或塔式起重机参数不同,需再根据具体情况重新计算TMD参数进行设计。
2.2 TMD参数计算
此型号塔吊结构总质量为198.83t,其中总质量包括了自重和配重等参数。根据上述方法,以1#塔吊起重臂仰角为30°、空载状态下为例进行计算分析。
根据Sadek等(1997)的研究,可依据结构第一振型或是相应控制振型设计TMD最优参数。
根据经验,TMD质量比取值为5%—10%,本章选定质量比5%进行设计。具体计算TMD参数如表 2所示。
表 2 Sadek法TMD参数表Table 2. TMD parameters of the table by Sadek参数 数值 单位 结构总质量 198.83 t 一阶振型质量参与系数 0.383 m1* 76.15 t μ 0.05 mTMD 3.8 t TMD个数 1 单个TMD质量 3.8 t ε1* 0.03 δ 0.9461 εTMD 0.2468 结构振型频率f* 0.1656 Hz 结构振型圆频率ω* 1.0397 rad/s ωTMD 0.9837 rad/s KTMD 3677.1296 N/m CTMD 1845.106 N·s/m 3. TMD控制效果的比较
由于塔机工作地点的不确定性,为了更为全面地研究TMD装置对塔吊地震控制的效果,本文选取典型的Taft波、RSN164-147波和El-Centro波为例作为输入,并假设抗震设防烈度为8度。
根据塔吊破坏的原因和1#塔吊的特点,选取3个控制目标。控制目标一为塔身顶部节点水平X方向的位移;控制目标二为塔身顶部节点水平Y方向的位移;控制目标三为起重臂端部节点竖直Z方向的位移。
三向地震作用输入时,其加速度峰值按水平向X:水平向Y:竖向Z=1.00:0.85:0.65取值(殷伟希等,2010;何志军等,2014)。
本文采用SAP2000有限元软件对结构进行分析。TMD减震装置主要由质量块和弹簧装置组成,故而用Linear模拟弹簧,具有实际质量的实体单元模拟质量块,用Damper模拟支撑体系,主要提供竖向刚度。其中,线性特性Linear Properties中阻尼设置不是等效阻尼比εTMD,而是等效阻尼CTMD。本文采用振型方法进行时程分析,在定义减震单元的等效阻尼时,可直接采用计算所得的等效阻尼;若采用直接积分法进行时程分析,则在定义减震单元的等效阻尼时,应将等效阻尼比减去结构本身的阻尼比后得到的阻尼值作为输入。
以地震振动控制效果最佳为目标,TMD安装方案为6种:TMD安装在塔身顶部、TMD安装在塔身中部、单向TMD、双向TMD、TMD安装在超高层建筑施工塔吊顶部并控制2种不同模态。将减震效果与无TMD时对比,观察减震效果。
3.1 不同安放位置对减震效果的影响
为了研究在塔身安装TMD装置的位置对减震效果的影响,采用上述方法计算TMD参数,观察控制效果,得到TMD装置的最优控制位置。
TMD装置一般放在结构响应最大处效果最好,由于塔吊一阶扭转效应明显,塔身顶部和中部变形都较大,根据前面分析的结果,将TMD装置放在塔吊中部和塔身顶部进行对比,分析2种方案减震效果的优劣。安装TMD装置前后塔吊周期对比如表 3所示。
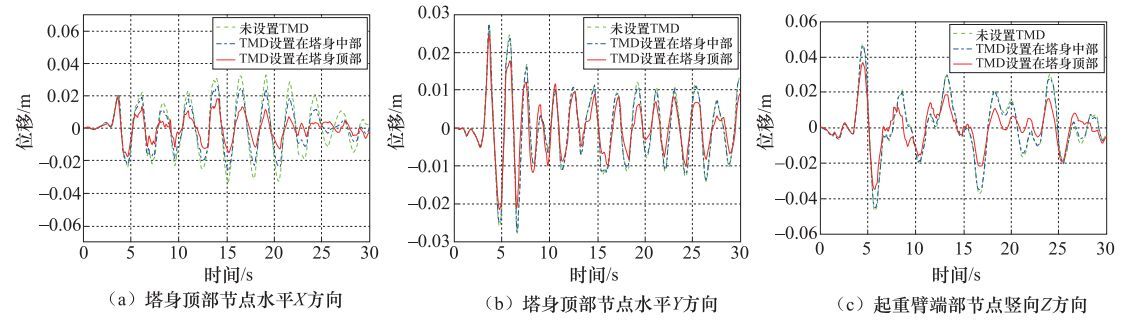
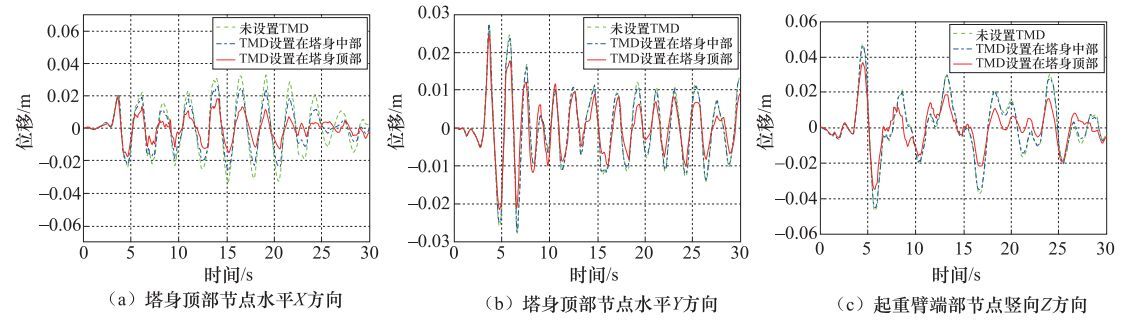
表 3 结构周期对比(单位:s)Table 3. Periods of model方案 第一阶 第二阶 第三阶 未设置TMD 6.24391 4.72713 2.61045 TMD设置在塔身中部 6.24397 4.72714 2.60781 TMD设置在塔身顶部 6.24402 4.73268 2.59619 为了比较安装TMD装置前后塔吊的减震效果,对比塔身顶部节点X向、Y向和起重臂端部Z向的位移响应。以Taft波为例,位移时程曲线如图 3所示,控制节点最大位移及减震率汇总如表 4所示。
表 4 控制位移最大值及减震率汇总Table 4. Maximum displacement and shock absorption rate输入地震波 方向 减震前最大位移/m 方案1 方案2 最大位移/m 减震率/% 最大位移/m 减震率/% Taft波 X 0.031 0.024 22.58 0.020 35.48 Y 0.029 0.028 3.45 0.026 10.34 Z 0.048 0.047 2.08 0.036 25.00 RSN164-147波 X 0.027 0.024 11.11 0.011 59.26 Y 0.023 0.022 4.34 0.015 34.78 Z 0.060 0.052 13.33 0.041 31.67 El-Centro波 X 0.052 0.046 11.54 0.013 75.00 Y 0.077 0.069 10.39 0.048 3.66 Z 0.153 0.150 1.96 0.092 39.87 注:表中X、Y、Z分别对应与塔身顶部X方向、塔身顶部Y方向和起重臂端部Z方向位移。方案1指塔身中部安装TMD装置;方案2指塔身顶部安装TMD装置。 由图 3和表 4可知,在3条地震波作用下,TMD装置安装在塔身顶部比安装在塔身中部可以更有效地减少塔吊3个方向的位移响应。其中塔身X方向平均减震率可达56.58%,虽然对于塔身Y方向的减震效果只有16.26%左右,但间接减少的起重臂端部位移可达32.18%。
综合塔吊3个方向的减震效果可以看出,将TMD安装在塔身顶部减震效果较好。
3.2 单向TMD与双向TMD对减震效果的影响
为了研究单向TMD装置和双向TMD装置对塔吊响应减震效果的影响,采用上述方法计算TMD参数,观察其控制效果,得到TMD装置最优方案。
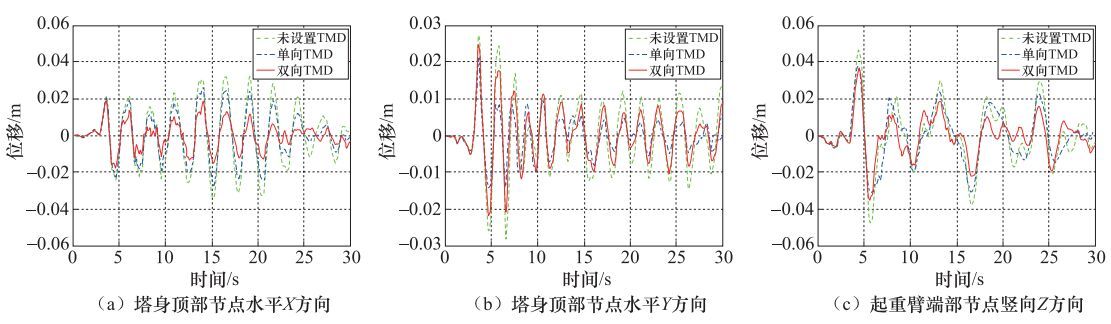
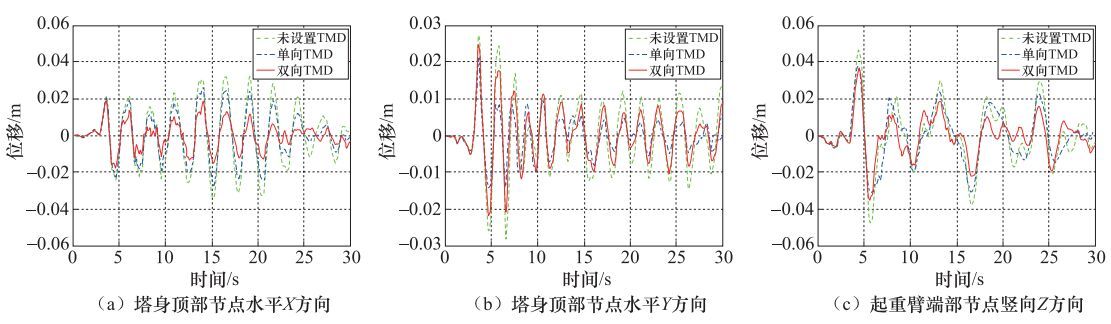
塔吊属于一种高耸结构,且结构X向整体刚度较小。塔吊一阶振型为起重臂绕回转平面内扭转为主要振型。塔吊二阶振型为塔身整体Y向后偏摆引起起重臂竖向振动。由于塔吊起重臂水平振动和侧弯振动的耦合较弱,双向TMD分别控制塔吊X和Y向的侧弯振动,单向TMD主要控制塔吊Y向振动,间接控制起重臂竖向振动。根据上文提到的方法设计TMD基本参数,观察2个方案的减震效果,如图 4和表 5所示。
表 5 控制位移最大值及减震率汇总Table 5. Maximum displacement and shock absorption rate输入地震波 方向 减震前最大位移/m 方案1 方案2 最大位移/m 减震率/% 最大位移/m 减震率/% Taft波 X 0.031 0.020 35.48 0.027 12.90 Y 0.029 0.026 10.34 0.021 27.59 Z 0.048 0.036 25.00 0.039 18.75 RSN164-147波 X 0.027 0.011 59.26 0.024 11.11 Y 0.023 0.015 34.78 0.012 47.83 Z 0.060 0.041 31.67 0.058 3.33 El-Centro波 X 0.052 0.013 75.00 0.043 17.31 Y 0.077 0.048 37.66 0.026 66.23 Z 0.153 0.092 39.87 0.133 13.07 注:表中X、Y、Z分别对应与塔身顶部X方向、塔身顶部Y方向和起重臂端部Z方向位移。方案1指塔身顶部安装双向TMD装置;方案2指塔身顶部安装单向TMD装置。 由图 4和表 5可知,2种方案对于塔吊都有明显的减震效果。双向TMD装置X、Y和Z方向平均减震率约为56.58%、27.59%和32.18%。单向TMD装置X、Y和Z方向平均减震率约为13.77%、47.22%和11.72%。
由于单向TMD主要起控制塔吊Y方向的响应,所以X方向减震效果不佳。而双向TMD虽然控制塔吊Y方向的响应较弱,但是控制起重臂竖向振动的效果要比单向TMD多达20.46%。
综合来看,由于塔吊主要破坏形式为起重臂弯折破坏,为了控制起重臂竖向位移,故而选择双向TMD更具优越性。
3.3 不同控制周期对减震效果的影响
为了研究超高层建筑施工中动臂式塔吊的减震效果,设计了2种方案,分别控制建筑-塔吊结构的不同振型,采用上述方法计算TMD参数,观察控制效果。
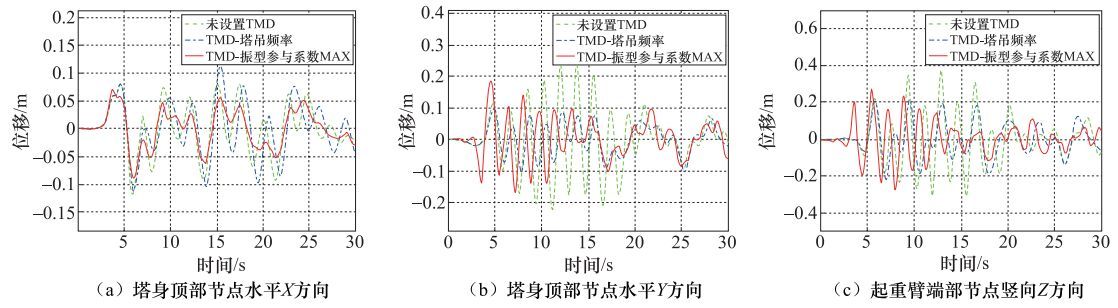
一般高层建筑地震反应以第一阶振型为主,由于塔吊与建筑结构的耦合作用改变了整体的振动特性,造成建筑-塔吊模型振型有所改变。建筑-塔吊模型第一阶振型为建筑Y方向平动引起塔吊结构的整体平动,如图 5(a)所示;第二阶振型为建筑X方向的平动引起塔吊结构的整体平动,如图 5(b)所示;第三阶振型为塔吊起重臂在回转平面内绕回转中心扭转,如图 5(c)所示。2种方案分别控制建筑-塔吊模型所对应的第一阶和第三阶频率。
因此,在设计TMD时,其自振频率ωd应根据塔吊结构的频率ω1及建筑-塔吊振型质量参与系数最大时的频率ω2分别进行计算,并对比分析其减震效果。以施工至70层时为例,塔式起重机安置TMD装置前后结构周期对比如表 6。
表 6 70层建筑-塔吊模型周期对比(单位:s)Table 6. Periods of model by 70 layer building-crane方案 第一阶 第二阶 第三阶 未设置TMD 6.1721 6.0437 5.3924 设置TMD 6.1743 6.0451 5.3931 根据Sadek法计算TMD最优参数,如表 7所示。
表 7 TMD参数表Table 7. TMD parameters of the table by Sadek参数 方案1 方案2 单个TMD质量/t 3.8 3.8 结构振型阻尼比取值ε1* 0.05 0.05 TMD与振型频率最佳频比δ 0.9420 0.9420 TMD阻尼比εTMD 0.2658 0.2658 结构振型频率f*/Hz 0.1633 0.1854 结构振型圆频率ω*/rad·s-1 1.0226 1.1643 TMD最佳圆频率ωTMD/rad·s-1 0.9633 1.0965 TMD刚度KTMD/N·m-1 3526.20 4568.79 TMD阻尼系数CTMD/N·s·m-1 1945.94 2215.02 根据上述2种方案设计TMD安装于超高层建筑施工塔吊塔身顶部,观察2个方案的减震效果,如图 6和表 8所示。
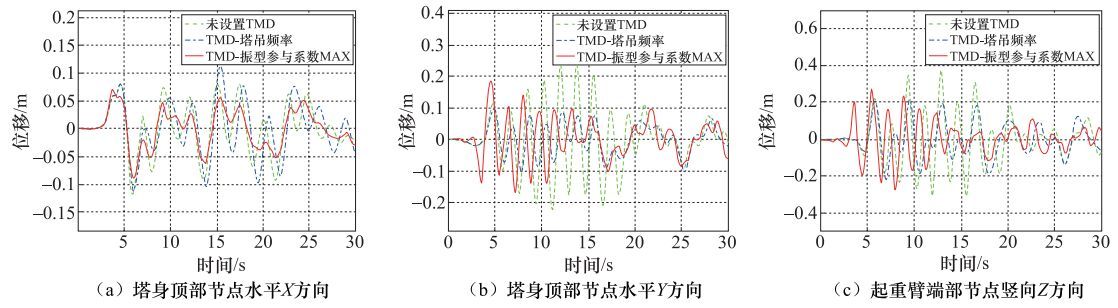
表 8 控制位移最大值及减震率汇总Table 8. Maximum displacement and shock absorption rate输入地震波 方向 减震前最大位移/m 方案1 方案2 最大位移/m 减震率/% 最大位移/m 减震率/% Taft波 X 0.075 0.119 -58.67 0.069 8.00 Y 0.237 0.097 59.07 0.192 18.99 Z 0.389 0.205 47.31 0.278 28.53 RSN164-147波 X 0.153 0.153 0 0.149 2.61 Y 0.178 0.165 7.30 0.164 7.87 Z 0.227 0.227 0 0.163 28.19 El-Centro波 X 0.151 0.158 -4.64 0.103 31.79 Y 0.513 0.382 25.54 0.523 -1.95 Z 0.579 0.579 0 0.491 15.20 注:表中X、Y、Z分别对应与塔身顶部X方向、塔身顶部Y方向和起重臂端部Z方向位移。方案1指计算时对应超高层建筑-塔吊模型第一阶自振周期;方案2指计算时对应超高层建筑-塔吊模型第三阶自振周期。 从表 8可以看出,在不同地震波作用下,TMD减震装置对于塔身顶部虽然控制效果不稳定,但是对于起重臂端部的竖向位移均起到不同程度的控制作用。
分析原因,可归为以下几点:① 地震波不同,其主频不同,所含有超高层建筑结构第一阶和第三阶模态频率的分量不大,致使塔吊地震控制效果具有不稳定性;② 对于控制低阶模态较好的TMD装置不能很好地降低塔吊高阶振型的影响;③ 塔吊是一种刚度质量不对称结构,扭转振型明显,故当触发扭转振型时,TMD装置不可能恰好并一直在其平衡位置保持静止,其带来的质量偏心和惯性力可能会加剧塔吊结构扭转作用。
由于以上几个原因,该TMD装置对塔吊塔身控制效果不稳定,但对于起重臂仍能起到良好的控制作用,可以在附着于超高层建筑上的施工塔吊上使用。
4. 结论
通过对不同方案的施工塔吊TMD地震减震响应控制与分析,可以得出以下结论:
(1)对于塔吊这种质量刚度不对称的高耸结构,利用TMD减震系统对动臂式塔吊进行地震控制是一种非常有效的方法。
(2)为了控制塔吊塔身侧向偏摆和起重臂竖向振动,设置在塔身顶部的双向TMD不仅可以有效减小塔身偏摆,也可以间接有效地控制起重臂竖向振动。
(3)以超高层建筑-塔吊结构为研究对象进行有限元分析发现,由于超高层建筑-塔吊结构高阶振型影响明显,此TMD装置对塔吊塔身控制效果不稳定,但对于起重臂仍能起到良好的控制作用,故而可以在附着于超高层建筑上的施工塔吊上使用,但如何对塔身的振动进行控制仍需进一步研究。
综上所述,虽然建筑施工周期内发生地震的可能性不高,但由于塔吊使用的广泛性和周期性,研究塔吊的地震响应规律和相应的减震措施很有必要。本文提出一种塔吊地震振动控制方法,通过运用Sadek法计算TMD最优参数,分析对比不同方案的减震效果,发现安装在塔身顶部的双向TMD可以有效控制动臂式塔吊的地震响应。
-
表 1 结构周期和振型质量参与系数
Table 1. Natural period and participation mass ratio of structures
模态/阶 周期/s UX UY SX SY 1 6.24 5.40E-07 0.3829 5.4E-07 0.3829 2 4.72 0.3353 8.31E-05 0.3353 0.3830 3 2.61 0.0003 0.3923 0.3356 0.7753 4 2.14 0.5070 4.32E-05 0.8426 0.7753 5 0.92 7.57E-05 4.46E-05 0.8427 0.7754 注:UX、UY分别为某一振型在X、Y方向的质量参与比;SX、SY为X、Y方向的质量参与比振型顺序累积值。 表 2 Sadek法TMD参数表
Table 2. TMD parameters of the table by Sadek
参数 数值 单位 结构总质量 198.83 t 一阶振型质量参与系数 0.383 m1* 76.15 t μ 0.05 mTMD 3.8 t TMD个数 1 单个TMD质量 3.8 t ε1* 0.03 δ 0.9461 εTMD 0.2468 结构振型频率f* 0.1656 Hz 结构振型圆频率ω* 1.0397 rad/s ωTMD 0.9837 rad/s KTMD 3677.1296 N/m CTMD 1845.106 N·s/m 表 3 结构周期对比(单位:s)
Table 3. Periods of model
方案 第一阶 第二阶 第三阶 未设置TMD 6.24391 4.72713 2.61045 TMD设置在塔身中部 6.24397 4.72714 2.60781 TMD设置在塔身顶部 6.24402 4.73268 2.59619 表 4 控制位移最大值及减震率汇总
Table 4. Maximum displacement and shock absorption rate
输入地震波 方向 减震前最大位移/m 方案1 方案2 最大位移/m 减震率/% 最大位移/m 减震率/% Taft波 X 0.031 0.024 22.58 0.020 35.48 Y 0.029 0.028 3.45 0.026 10.34 Z 0.048 0.047 2.08 0.036 25.00 RSN164-147波 X 0.027 0.024 11.11 0.011 59.26 Y 0.023 0.022 4.34 0.015 34.78 Z 0.060 0.052 13.33 0.041 31.67 El-Centro波 X 0.052 0.046 11.54 0.013 75.00 Y 0.077 0.069 10.39 0.048 3.66 Z 0.153 0.150 1.96 0.092 39.87 注:表中X、Y、Z分别对应与塔身顶部X方向、塔身顶部Y方向和起重臂端部Z方向位移。方案1指塔身中部安装TMD装置;方案2指塔身顶部安装TMD装置。 表 5 控制位移最大值及减震率汇总
Table 5. Maximum displacement and shock absorption rate
输入地震波 方向 减震前最大位移/m 方案1 方案2 最大位移/m 减震率/% 最大位移/m 减震率/% Taft波 X 0.031 0.020 35.48 0.027 12.90 Y 0.029 0.026 10.34 0.021 27.59 Z 0.048 0.036 25.00 0.039 18.75 RSN164-147波 X 0.027 0.011 59.26 0.024 11.11 Y 0.023 0.015 34.78 0.012 47.83 Z 0.060 0.041 31.67 0.058 3.33 El-Centro波 X 0.052 0.013 75.00 0.043 17.31 Y 0.077 0.048 37.66 0.026 66.23 Z 0.153 0.092 39.87 0.133 13.07 注:表中X、Y、Z分别对应与塔身顶部X方向、塔身顶部Y方向和起重臂端部Z方向位移。方案1指塔身顶部安装双向TMD装置;方案2指塔身顶部安装单向TMD装置。 表 6 70层建筑-塔吊模型周期对比(单位:s)
Table 6. Periods of model by 70 layer building-crane
方案 第一阶 第二阶 第三阶 未设置TMD 6.1721 6.0437 5.3924 设置TMD 6.1743 6.0451 5.3931 表 7 TMD参数表
Table 7. TMD parameters of the table by Sadek
参数 方案1 方案2 单个TMD质量/t 3.8 3.8 结构振型阻尼比取值ε1* 0.05 0.05 TMD与振型频率最佳频比δ 0.9420 0.9420 TMD阻尼比εTMD 0.2658 0.2658 结构振型频率f*/Hz 0.1633 0.1854 结构振型圆频率ω*/rad·s-1 1.0226 1.1643 TMD最佳圆频率ωTMD/rad·s-1 0.9633 1.0965 TMD刚度KTMD/N·m-1 3526.20 4568.79 TMD阻尼系数CTMD/N·s·m-1 1945.94 2215.02 表 8 控制位移最大值及减震率汇总
Table 8. Maximum displacement and shock absorption rate
输入地震波 方向 减震前最大位移/m 方案1 方案2 最大位移/m 减震率/% 最大位移/m 减震率/% Taft波 X 0.075 0.119 -58.67 0.069 8.00 Y 0.237 0.097 59.07 0.192 18.99 Z 0.389 0.205 47.31 0.278 28.53 RSN164-147波 X 0.153 0.153 0 0.149 2.61 Y 0.178 0.165 7.30 0.164 7.87 Z 0.227 0.227 0 0.163 28.19 El-Centro波 X 0.151 0.158 -4.64 0.103 31.79 Y 0.513 0.382 25.54 0.523 -1.95 Z 0.579 0.579 0 0.491 15.20 注:表中X、Y、Z分别对应与塔身顶部X方向、塔身顶部Y方向和起重臂端部Z方向位移。方案1指计算时对应超高层建筑-塔吊模型第一阶自振周期;方案2指计算时对应超高层建筑-塔吊模型第三阶自振周期。 -
何银晖, 谷立臣, 姬鹏斌, 2012.基于ANSYS的塔式起重机抗震分析.机械设计与制造, (7):188-190. http://www.cnki.com.cn/Article/CJFDTOTAL-JSYZ201207069.htm 何志军, 丁洁民, 陆天天, 2014.上海中心大厦巨型框架-核心筒结构竖向地震作用反应分析.建筑结构学报, 35(1):27-33. http://www.cnki.com.cn/Article/CJFDTOTAL-JZJB201401004.htm 吉军, 张辉, 张顺利等, 2009.塔式起重机地震响应研究.西北师范大学学报(自然科学版), 45 (4):43-47. http://cdmd.cnki.com.cn/Article/CDMD-10141-1012395206.htm 李云岭, 艾兵, 李博, 2011.塔式起重机在地震作用下的受损原因及动态分析现状.特种结构, 28 (2):66-68. http://www.cnki.com.cn/Article/CJFDTOTAL-TZJG201102031.htm 刘小萌, 张长会, 卢志强等, 2015.黏滞阻尼器在煤矿井塔结构抗震加固中的应用.震灾防御技术, 10(1):126-134. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20150113&journal_id=zzfyjs 卿龙邦, 姚雄, 肖成志等, 2013.外爬式塔吊对高层钢板剪力墙结构影响的分析.华北地震科学, 31(S):24-27. http://www.cnki.com.cn/Article/CJFDTOTAL-HDKD2013S1007.htm 王武奇, 2009. QTZ630型塔式起重机结构有限元分析及地震影响研究. 西安: 长安大学. 谢瑞, 田太明, 田奇, 2009.汶川地震中西安市塔式起重机的损毁分析.起重运输机械, (4):1-3. http://www.cnki.com.cn/Article/CJFDTOTAL-QZJJ200904001.htm 阎红霞, 杨庆山, 张丽英, 2010. ABAQUS在超高层结构动力弹塑性分析中的应用.震灾防御技术, 5(1):108-115. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20100113&journal_id=zzfyjs 闫维明, 纪金豹, 蒋华戈等, 2010.新型悬吊式TMD及其在某标志塔风振控制中的应用.建筑结构学报, 31(2):55-60. http://www.cnki.com.cn/Article/CJFDTOTAL-JZJB201002007.htm 姚佳琳, 张家伟, 王泽云, 2014.超高层"框-筒"结构中内爬式塔吊附着节点力学性能分析.四川建筑科学研究, 40(2):345-349, 360. http://www.cnki.com.cn/Article/CJFDTOTAL-ACZJ201402086.htm 殷伟希, 谭平, 周福霖等, 2010.近场地震动下偏心结构的减震控制研究.震灾防御技术, 5(2):199-207. http://zzfy.eq-j.cn/zzfyjs/ch/reader/view_abstract.aspx?flag=1&file_no=20100206&journal_id=zzfyjs 章崇任, 2007.日本内爬式塔式起重机的发展趋势.工程机械, 38(4):73-75. http://www.cnki.com.cn/Article/CJFDTOTAL-GCJA200704022.htm 张俊卫, 2014. 超高层建筑的风致横风向响应分析和TMD控制. 大连: 大连理工大学. http://cdmd.cnki.com.cn/Article/CDMD-10141-1015572495.htm Kim J., Lee S., Choi H., 2013. Progressive collapse resisting capacity of moment frames with viscous dampers. The Structural Design of Tall and Special Buildings, 22(5):399-414. doi: 10.1002/tal.v22.5 Longarini N., Zucca M., 2014. A chimney's seismic assessment by a tuned mass damper. Engineering Structures, 79:290-296. doi: 10.1016/j.engstruct.2014.05.020 Mijailović R., Kastratović G., 2009. Cross-section optimization of tower crane lattice boom. Meccanica, 44(5):599-611. doi: 10.1007/s11012-009-9204-4 Otani A., Nagashima K., Suzuki J., 2002. Vertical seismic response of overhead crane. Nuclear Engineering and Design, 212(1-3):211-220. doi: 10.1016/S0029-5493(01)00483-6 Sadek F., Mohraz B., Taylor A. W., et al., 1997. A method of estimating the parameters of tuned mass dampers for seismic applications. Earthquake Engineering & Structural Dynamics, 26(6):617-635. doi: 10.1002/%28SICI%291096-9845%28199706%2926%3A6%3C617%3A%3AAID-EQE664%3E3.0.CO%3B2-Z Wang P., Scherler D., Liu-Zeng J., et al., 2014. Tectonic control of Yarlung Tsangpo Gorge revealed by a buried canyon in Southern Tibet. Science, 346(6212):978-981. doi: 10.1126/science.1259041 -





 下载:
下载:






 下载:
下载: