The Seismic Response Effect Analysis of Scaled Models of CAP1400 Nuclear Island Structure
-
摘要: 针对核电厂CAP1400核岛结构地震反应问题，构建了核岛屏蔽厂房和辅助厂房整体结构的3个分析模型：原型和1/16、1/40缩尺模型，并在AP000谱和RG1.60谱地震动输入下进行了有限元模拟对比分析，探讨了振动台试验模型缩尺处理的合理性和精确性。研究表明，基于缩尺模型得到的结构自振频率相对于原型结构模型有所降低，降低幅度在8.5%以内；结构模型的缩尺对结构反应峰值加速度和高频（大于3Hz）加速度反应谱的影响较为显著，但对较低频（小于3Hz）的加速度反应谱影响较小；模型缩尺对结构不同方向反应的影响中，刚度越大的方向其影响越大。进一步将结构模型数值模拟结果与1/16缩尺模型的振动台试验结果进行了比较分析，试验给出的结构自振频率远低于模型数值模拟结果，但原型和1/16缩尺模型数值模拟得到的结构反应均与试验结果较为接近。基于模型数值模拟和振动台试验研究，认为对于缩尺比1/16或更大的模型可以忽略模型的缩尺效应。Abstract: Aiming at the seismic response problem of CAP1400 nuclear island structure of the nuclear power plant 3 analytical models, prototype and 1/16, 1/40 scale models, were constructed for the whole structure of the shielding and auxiliary factory, and comparative analysis of the seismic responses of the 3 models was done by finite element method under the ground motions matching AP000 spectrum and the RG1.60 spectrum, the rationality and accuracy were discussed on the scaled model for the shaking table testing. The research shown that natural frequencies of structure response from scaled models are smaller than that produced in the prototype structure model, the reduction was within 8.5%; the effect of scaled models is obviously on the peak acceleration and high frequency (more than 3Hz) spectral accelerations of structure response, but less on the low frequency (less than 3Hz) spectral accelerations; the effect of scaled model on the structure response is larger in direction of larger stiffness. In addition, the contrastive analysis between numerical simulation of different scale models and a shaking table testing of 1/16 scale model was carried out, which indicates that the natural frequencies from the shaking table testing is far lower than those from numerical simulation, but the simulated structure responses of the prototype and 1/16 scale models and the test results of 1/16 scale model were relatively close. The study reveals that scale effect of analysis and testing models can be ignored for scaled models of 1/16 or greater.
-
Key words:
- Nuclear power plant /
- Scaled model /
- Numerical analysis /
- Shaking table test /
- Spectral acceleration
-
引言
CAP1400核电技术是我国第三代核电技术自主创新的标志，也是我国目前大力推广使用的核电技术。核电工程由于涉及高放射性物项，核岛结构在地震作用下一旦出现问题发生核泄露，其后果将难以承受。因此，对核岛结构的地震安全要求极高，特别是对于新型核岛结构，必须对其进行严格的抗震分析和设计（林皋，2011）。
复杂结构抗震分析的方法有数值模拟和模型试验两大类。随着计算机性能大幅提升和计算方法的飞速发展，采用有限元数值模拟很容易进行复杂结构的地震反应分析，但由于数值模拟分析中采用了大量的简化处理，其分析结果的真实性和可靠性需要给予论证。利用模型试验可以展现和获取复杂结构的动力反应特征，以分析和了解结构的抗震性能，也可以基于模型试验结果验证数值模拟计算模型的合理性和模拟结果的可靠性。对于重要性极高的核岛结构，往往需要综合利用数值模拟和模型试验来完成抗震分析。
利用振动台进行模型试验是研究工程结构抗震能力和破坏机理的重要手段，随着振动台试验技术的发展（Severn，2011），大量针对不同形式工程结构采用不同试验方式的振动台试验得以开展（Paolucci等，2008；李振宝等，2010；蔡新江等，2011；李勇等，2013）。但受振动台台面尺寸和承载能力的限制，一般只能采用缩尺模型进行试验。缩尺模型试验首先采用相似原理进行缩尺模型设计，而后进行模型试验，并将得到的试验结果通过相似关系反推到足尺结构。设计结构试验的缩尺模型时，模型比尺、模型材料、荷载的大小，以及模型与原型间的应力、变形、承载力等的换算，都必须依据相似理论，遵循相似准则。针对试验模型设计和地震动输入处理的相似性问题，国内外学者开展了一系列的研究，在试验的理论、方法和技术方面均取得了较丰富的成果（张敏政，1997；Žarnić等，2001；周颖等，2006；赵作周等，2010；Shi等，2013；黄思凝等，2013；权登州等，2015；张佳等，2016；刘红彪等，2016）。随着模型试验的发展，基于缩尺模型试验获取的实际结构抗震性能结果的合理性和精确性则成为人们关注和研究的问题，特别是对于结构尺寸和质量较大——如核岛结构——而试验模型缩尺比较小的情况。不同学者分别通过缩尺模型的数值模拟分析（杨树标等, 2007, 2008；柳春光等，2012；杜修力等，2013）、试验分析（孟庆利等，2008；宋二祥等，2008；吕西林等，2008；姜忻良等，2010）以及数值模拟与试验结果的对比分析（Pitilakisa等，2008；郑升宝等，2010；钱德玲等，2013）等手段，研究了振动台试验缩尺结构模型的合理性问题。研究中具体对比分析了缩尺模型结构与原型结构的自振频率及加速度、位移、应力和应变等反应值，也包括一些破坏现象和破坏部位等，给出了缩尺模型结构与原型结构反应一致性或差异方面的一些研究结果，例如吕西林等（2008）的研究表明，针对钢-混凝土混合结构房屋采用1/30的小缩尺比模型进行振动台试验仍然是可行的；杜修力等（2013）研究认为由于土-结构相似比不完全一致，与原型数值试验结构应变比较时，模型数值试验的应变不能直接依据相似比采用。但至目前，针对尺寸和质量较大的核岛结构的较小缩尺比试验模型，振动台试验结果的合理性和精确性问题还缺乏研究。
本文基于相似原理，对采用1/1（原型）、1/16、1/40这3种缩尺比的CAP1400核岛结构模型进行地震反应数值模拟分析，并与CAP1400核岛结构缩尺比1/16模型的振动台试验结果进行对比，从结构自振频率和节点反应加速度特性等方面进行评价，分析不同缩尺比模型的适用性。
1. 核岛结构缩尺模型及输入地震动
1.1 缩尺模型和设计参数
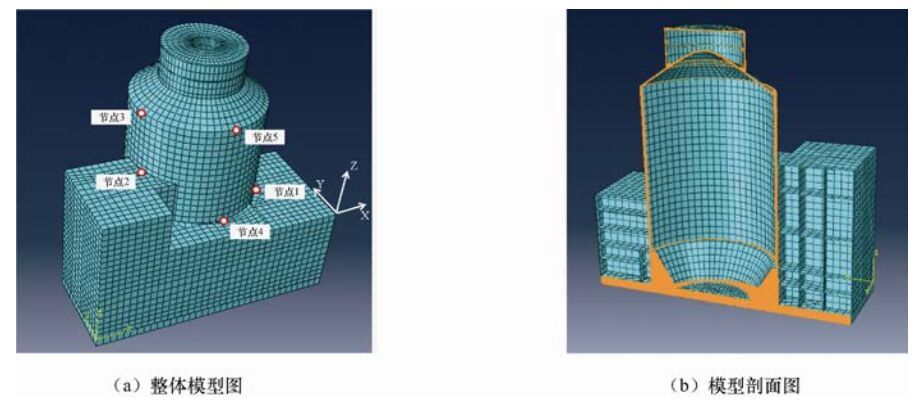
CAP1400核电厂核岛结构分为屏蔽厂房、安全壳结构、辅助厂房和钢筋混凝土基础等结构。屏蔽厂房为圆形筒状斜屋顶结构，总高约87.75m（含地下室），底部外径为49.97m，壁厚1.10m，地面高75.55m，地下深12.00m。辅助厂房为钢筋混凝土剪力墙结构，3面围绕屏蔽厂房布置，地上3部分的屋顶标高呈阶梯状，分别为18.05m、20.35m和35.30m，2层地下室深12.20m。屏蔽厂房与辅助厂房相交的部分为钢筋混凝土剪力墙结构，不相交的部分为钢板混凝土剪力墙结构，屋顶采用型钢混凝土组合结构，屋顶上部设有钢筋混凝土结构环形冷却系统储水箱。图 1为CAP1400核岛结构示意图。
图 1 CAP1400核岛结构屏蔽厂房与辅助厂房示意图Figure 1. A diagram of the shielding and auxiliary factory structure of nuclear power plant CAP1400根据本文分析的核岛结构的尺寸和质量以及开展模型试验的振动台承载力，分别设计了几何比尺为1/16、1/40的CAP1400核岛屏蔽厂房和辅助厂房整体结构缩尺模型，缩尺模型的相似系数列于表 1。
表 1 核岛结构缩尺模型设计相似关系Table 1. Similitude relation of scaled models of nuclear power plant structure物理量 量纲 相似系数（1/16模型） 相似系数（1/40模型） 尺寸L [L] 1/16 1/40 线位移δ [L] 1/16 1/40 角位移β 1 1 弹性模量E [FL-2] 0.340 0.233 密度ρ [FL-4T2] 1 1 泊松比μ 1 1 应变ε 1 1 应力σ [FL-2] 0.340 0.233 等效质量密度ρε [FL-4T2] 1.74 1.74 集中荷载F [F] 1.328×10-3 1.458×10-4 弯矩M [FL] 8.300×10-5 3.645×10-6 时间t [T] 1.414×10-1 6.827×10-2 自振频率ω [T-1] 7.072 14.647 阻尼比ξ 1 1 加速度幅值ɑ [LT-2] 3.126 5.363 加速度频率υ [T-1] 7.072 14.647 结构刚度k [FL-1] 2.125×10-2 5.832×10-3 结构自重m [FL-1T2] 4.248×10-4 2.719×10-5 1.2 有限元计算模型
运用abaqus软件建立了CAP1400核岛结构原型（1/1缩尺模型）和1/16、1/40缩尺模型的三维有限元计算模型。考虑到钢制安全壳虽然与屏蔽厂房共用同一基础，但其位于屏蔽厂房内，结构部分与屏蔽厂房结构相互独立，在本文分析中忽略钢制安全壳对屏蔽厂房的影响，在有限元建模中，不考虑钢制安全壳。有限元计算模型中，屏蔽厂房采用solid建模（并忽略墙体钢板混凝土中钢板的作用），辅助厂房采用shell建模，solid和shell采用abaqus中自带的壳与实体耦合连接，可以消除两者因为自由度不相同带来的误差。本文建立的有限元分析模型如图 2。
图 2 CAP1400核岛结构有限元模型Figure 2. The finite element model of nuclear power plant CAP1400 structure1.3 计算地震动的选择和输入
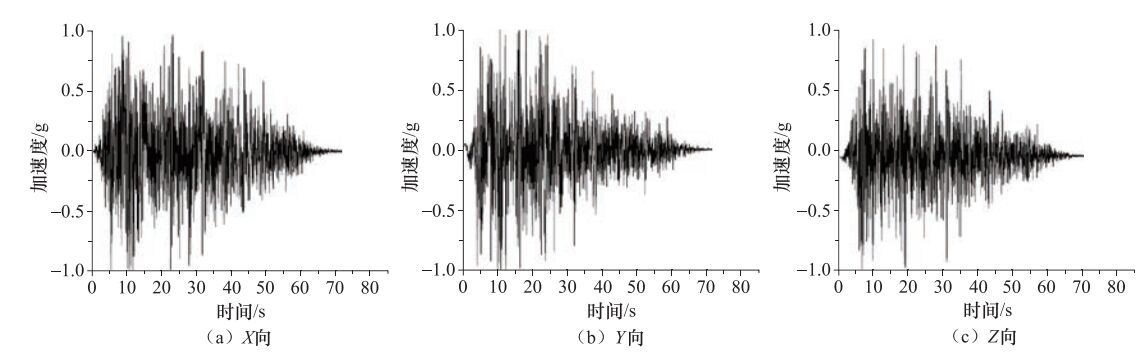
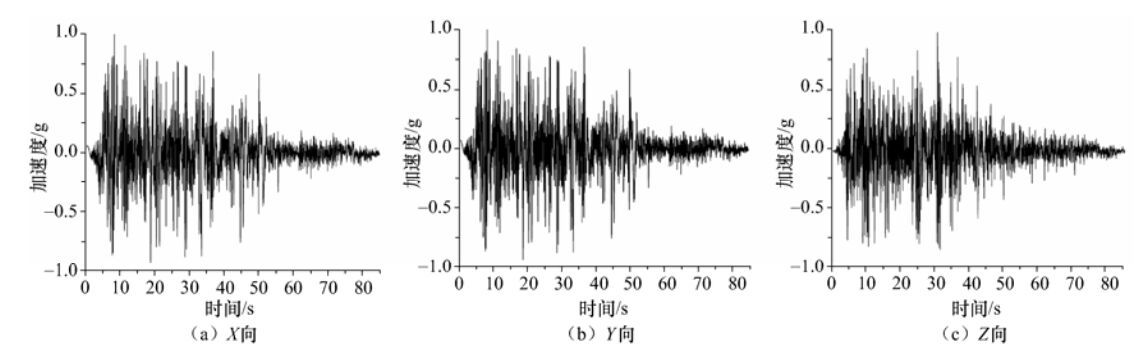
有限元计算分析中，核岛结构基础处的输入地震动分别采用核电厂设计标准加速度反应谱AP1000谱、RG1.60谱相应的地震动时程。RG1.60谱、AP1000谱的区别为频率大于10Hz的AP1000谱值更大（图 3），即相应的地震动具有更丰富的高频成分。实际计算中，根据表 1给定的相似关系对输入地震动进行压缩处理，并且根据振动台地震动输入有效控制范围（设为0.1－50Hz）进行滤波，以便与振动台试验结果进行比较分析。
本文采用拟合多阻尼反应谱的人工地震动合成技术（Dai等，2014），合成核电厂设计标准加速度反应谱相应的地震动时程（侯春林等，2016），其中AP1000谱相应的三向（2个水平向和1个竖向）地震动时程（峰值加速度标定为1.0g）如图 4，RG1.60谱相应的三向地震动时程（峰值加速度标定为1.0g）如图 5。考虑到我国CAP1400核电厂建设选址中确定的核电厂安全停堆地震动（SSE）峰值加速度一般不超过0.30g的实际情况，原型模型分析中结构基础处的输入地震动峰值加速度取为核电厂安全停堆地震动（SSE）值，即X向、Y向和Z向加速度幅值分别为0.30g、0.30g和0.20g（这里X、Y和Z方向参看图 2），而根据相似理论，1/16缩尺模型分析的模型基底输入三向地震动峰值加速度分别取为0.938g、0.938g和0.625g，1/40缩尺模型分析的模型基底输入三向地震动峰值加速度分别取为1.624g、1.624g和1.083g。计算过程中，视结构处于可实现安全停堆要求状态，即考虑结构处于弹性反应阶段。
图 4 AP1000谱人工地震动加速度时程Figure 4. Artificial acceleration time-history corresponding to AP1000 spectra
图 5 RG1.60谱人工地震动加速度时程Figure 5. Artificial acceleration time-history corresponding to RG1.60 spectra2. 模型计算结果及对比分析
2.1 结构自振频率
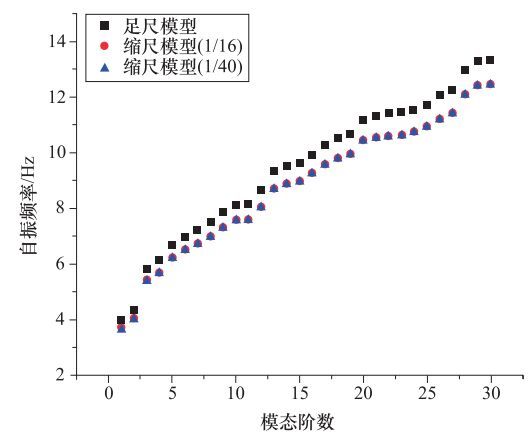
自振频率是建筑结构的重要动力特性参数，反映结构的振动特征。采用abaqus中线性摄动中的频率分析模块，选择Lanczos特征值求解器对结构进行模态分析。将计算值反推到原型结构并提取前30阶自振频率，结果如图 6所示，其中前4阶自振频率值列于表 2。
图 6 不同缩尺比模型计算得到的结构反应前30阶自振频率Figure 6. The first 30 natural frequencies of structure response from different scale structure models表 2 不同缩尺比模型计算得到的结构反应前4阶自振频率Table 2. The first 4 natural frequencies of structure response from different scaled structure models振型序号 1 2 3 4 1/1模型自振频率/Hz 3.99 4.35 5.82 6.14 1/16模型自振频率 计算值/Hz 26.34 28.70 38.38 40.60 反推值/Hz 3.72 4.05 5.42 5.74 与1/1模型的相对误差/% -6.77 -6.90 -6.70 -6.51 1/40模型自振频率 计算值/Hz 53.49 58.88 79.18 83.41 反推值/Hz 3.65 4.02 5.41 5.69 与1/1模型的相对误差/% -8.52 -7.59 -7.04 -7.33 振型分析表明，结构反应的前2阶振型分别为沿着Y方向的一阶振型和X方向的一阶振型；从第三阶开始，出现了结构壳体的扭转振型。从图 6和表 2给出的计算结果可以看出，随着结构模型缩尺比的减小，基于模型计算得到的结构自振频率值减小，但相对于原结构模型的自振频率值减小幅度均在8.5%以内，且1/16和1/40缩尺模型计算结果之间的差别很小。也就是说，相对于原结构模型，缩尺模型所模拟的结构自振频率有所降低，缩尺比越小的模型所模拟的结构自振频率降低越多。
2.2 结构反应峰值加速度
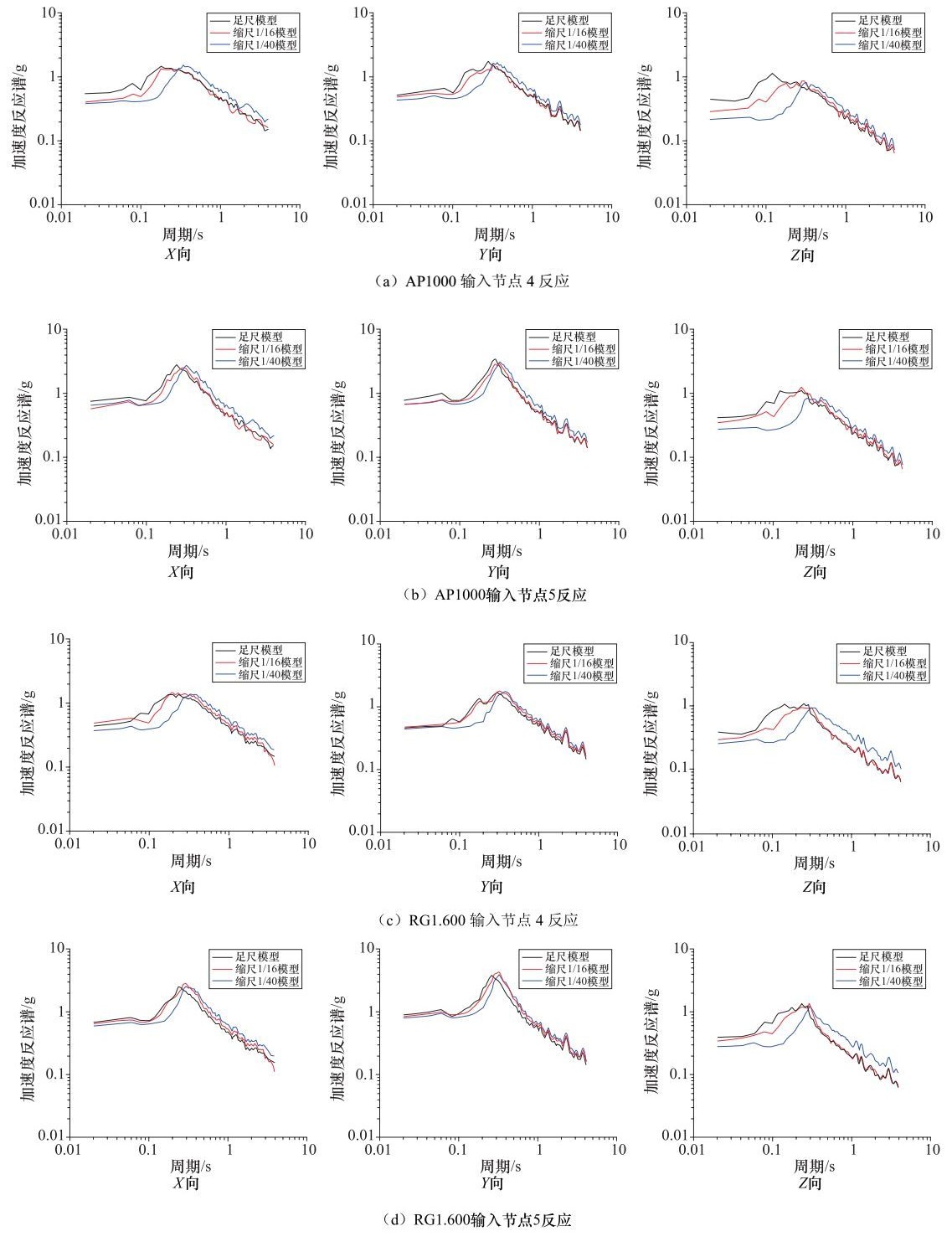
分析结构地震动反应时选用了5个控制性节点（图 2），节点1和4位于辅助厂房较低部分的屋顶面与屏蔽厂房交界处，标高分别为+33.95m和+36.25m；节点2位于辅助厂房较高部分与屏蔽厂房交界处，标高为+51.20m；节点3和5位于屏蔽厂房墙体与屋顶交界处，标高均为+72.05m。根据模型结构相似关系，以缩尺模型的计算加速度反应反推出原型结构对应的加速度反应。表 3给出了5个节点处基于不同模型的计算结构反应峰值加速度，表中括号内的值为相对于足尺模型的误差百分数。图 7给出了5个节点处基于不同模型的计算结构反应峰值加速度相对于基底输入的放大系数变化情况。
表 3 控制性节点处结构反应的峰值加速度（单位：g）Table 3. Peak accelerations of structural response at the control points节点 输入地震动：AP1000时程 输入地震动：RG1.60时程 缩尺比 1/1 1/16 1/40 缩尺比 1/1 1/16 1/40 1 X向 0.489 0.474（-3.1） 0.351（-28.2） X向 0.539 0.526（-2.4） 0.359（-33.3） Y向 0.482 0.481（-2.1） 0.408（-15.3） Y向 0.500 0.470（-6.0） 0.388（-22.4） Z向 0.386 0.292（-24.4） 0.220（-43.0） Z向 0.376 0.306（-18.6） 0.258（-31.4） 2 X向 0.546 0.54（-1.1） 0.478（-12.5） X向 0.574 0.652（13.6） 0.449（-21.8） Y向 0.561 0.596（6.2） 0.554（-1.2） Y向 0.522 0.562（7.7） 0.540（34.5） Z向 0.378 0.298（-21.2） 0.248（-34.4） Z向 0.348 0.301（-13.5） 0.258（-25.8） 3 X向 0.756 0.569（-24.7） 0.652（-13.8） X向 0.770 0.817（6.1） 0.704（-8.6） Y向 0.817 0.708（-13.3） 0.717（-12.2） Y向 0.789 0.704（-10.8） 0.737（-6.6） Z向 0.396 0.328（-17.2） 0.267（-32.6） Z向 0.410 0.354（-13.7） 0.264（-35.6） 4 X向 0.511 0.380（-25.6） 0.378（-26.0） X向 0.420 0.504（20.0） 0.386（-8.1） Y向 0.520 0.486（-6.5） 0.444（-14.6） Y向 0.448 0.464（35.7） 0.435（-2.9） Z向 0.414 0.292（-29.5） 0.223（-46.1） Z向 0.348 0.283（-18.7） 0.257（-26.1） 5 X向 0.554 0.520（-6.1） 0.565（19.9） X向 0.659 0.642（-2.6） 0.595（-9.7） Y向 0.920 0.830（-9.8） 0.789（-14.2） Y向 0.897 0.850（-5.2） 0.811（-9.6） Z向 0.381 0.328（-13.9） 0.242（-36.5） Z向 0.402 0.346（-13.9） 0.289（-28.1） 
图 7 制控节点处结构反应峰值加速度放大系数Figure 7. Peak acceleration amplification factor of structural response at control points从计算结果可以看出结构模型的缩尺对结构反应峰值加速度的影响：① 总体影响较为显著，反应值以减小为主，但也有增加的情况出现，并且缩尺比越小总体影响越显著；② 对结构3个方向反应的影响中，竖向（Z向）的最大、水平Y向的最小，也就是对结构刚度越大的方向影响也越大；③ 对结构不同高度处反应的影响程度基本一致；④ 2个不同的地震动基底输入下，结构模型缩尺的影响趋势和程度基本一致。
2.3 结构反应加速度反应谱
图 8给出了节点4和5处基于不同模型的计算结构反应加速度反应谱变化情况（受篇幅的限制，这里没有给出节点1、2和3处的图形）。从计算结果可以得到结构模型的缩尺对结构地震反应的影响：① 总体上，不同的结构缩尺模型和不同的地震动基底输入情况下，结构反应的加速度反应谱计算结果的变化特征和趋势与结构反应的峰值加速度基本一致；② 相对而言，结构模型的缩尺对结构反应的高频（ > 3Hz）加速度反应谱影响显著，但对较低频（≤3Hz）加速度反应谱影响较小；③ 1/16缩尺模型加速度反应谱的计算结果与原型结构模型很接近，认为对于缩尺比1/16或更大的模型可以忽略模型的缩尺效应。另外，对节点1、2和3处结构反应的计算结果进行分析，发现其特征与节点4和5处的基本一致。
图 8 控制性节点处结构反应加速度反应谱（一）Figure 8. Spectral accelerations of structural response at control points
图 8 控制性节点处结构反应加速度反应谱（二）Figure 8. Spectral accelerations of structural response at control points3. 模型数值计算与振动台试验的对比
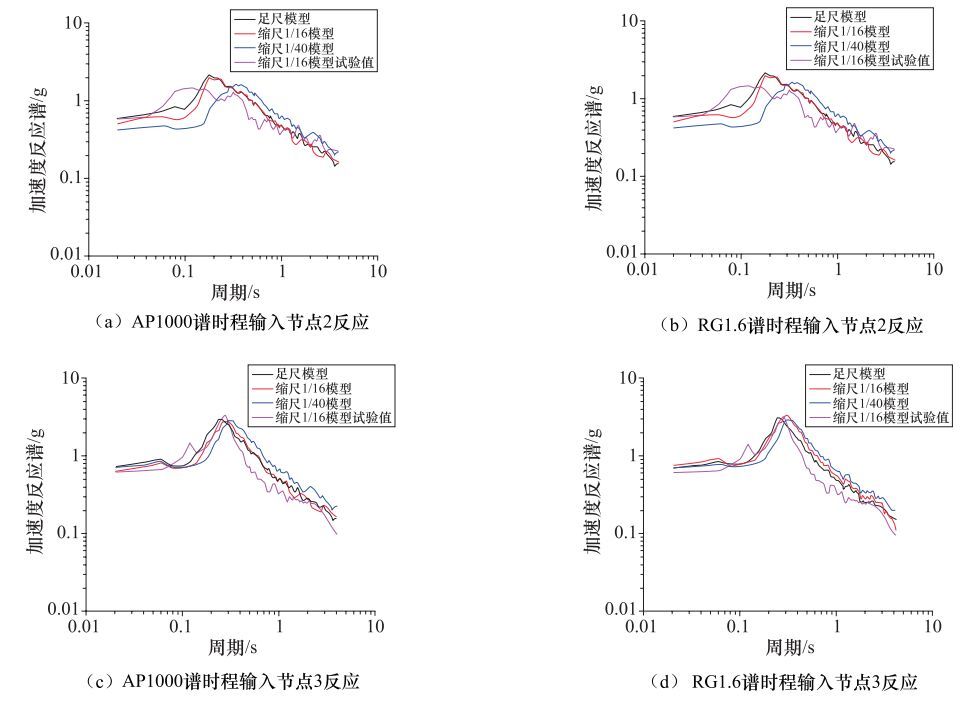
课题组制作了缩尺比为1/16的CAP1400核岛屏蔽厂房和辅助厂房整体结构的试验模型，并进行了振动台试验。本节将本文上述结构模型的数值模拟结果与该缩尺模型试验结果进行对比分析，具体分析了结构模型基底X向地震动输入情况下结构的自振频率及节点2和3处结构反应的数值模拟与模型试验结果。
3.1 结构自振频率对比
1/16缩尺模型试验测得的模型X向（结构长轴方向）一阶自振频率为2.31Hz，这一值远小于前文基于1/1、1/16和1/40的缩尺结构模型数值计算给出的结构自振频率值4.35Hz、4.05Hz和4.02Hz。产生这一差距的原因可能主要归于试验模型制作中缩尺相似条件与理想模型之间存在的差距。另一方面，构建有限元计算时对结构进行了很大程度的简化处理，如钢板混凝土墙体的简化处理等也会带来计算结果的差异。对于这里展现出的自振频率结果的差异原因分析还有待于进一步的研究工作。
3.2 结构反应加速度反应谱的对比
图 9为结构模型节点2和3处反应的加速度反应谱的数值计算结果与振动台试验结果。通过1/16缩尺模型试验结果和模型数值计算结果的对比分析，可以看到：① 原型和较大缩尺比（1/16）模型的数值计算结果与模型试验结果具有较好的一致性，但较小缩尺比（1/40）模型的计算结果与试验结果之间有显著的差异；② 不同高度位置（节点）的结构反应的数值计算结果与试验结果之间的差异程度有所不同，其差异程度在不同频段范围也有所不同，高度较低处结构反应的高频（ > 3Hz）加速度反应谱的差异显著，而高度较高处结构反应的低频（≤3Hz）加速度反应谱的差异显著（这可能与高度较低的辅助厂房结构影响有关）；③ 2个不同的地震动基底输入下，数值计算结果与试验结果的差异特征和程度基本一致。
图 9 结构反应加速度反应谱的数值计算结果与试验结果的比较Figure 9. Comparison of spectral accelerations between the numerical analysis and model testing4. 结语
本文通过核电厂CAP1400核岛屏蔽厂房和辅助厂房整体结构的原型和1/16、1/40缩尺模型等3个有限元模型的数值模拟对比分析，结合1/16缩尺模型的振动台试验结果，探讨了尺寸和质量较大的核岛结构的振动台试验缩尺模型相似性处理的合理性和精确性问题，获得了以下认识：
（1）对于结构自振频率，缩尺模型的有限元模拟结果相对于原结构模型有所降低，但减小幅度均在8.5%以内，且1/16和1/40的缩尺模型结果之间的差异很小。而1/16缩尺模型振动台试验得到的结构自振频率却远低于模型的数值分析结果。
（2）结构模型的缩尺对结构反应峰值加速度和高频（ > 3Hz）加速度反应谱的影响较为显著，而对较低频（≤3Hz）加速度反应谱的影响较小，且结构反应值以减小为主，缩尺比越小反应值减小越多。但1/16缩尺模型的加速度反应谱计算结果与原型结构模型接近，认为对于缩尺比1/16或更大的模型可以忽略模型的缩尺效应。
（3）在结构刚度较大的方向，缩尺对结构反应的影响越大，具体表现为对竖向（Z向）的影响最大、水平向Y向的最小。
（4）原型和较大缩尺比（1/16）模型的数值模拟结果与模型振动台试验结果具有较好的一致性，但较小缩尺比（1/40）模型带来了显著的差异；不同高度位置（如控制性节点）结构反应的数值计算结果与试验结果之间的差异程度有所不同，其差异程度在不同频段范围也有所不同，但在2个不同的基础处输入地震动下的数值计算结果与试验结果的差异特征和程度基本一致。
-
图 1 CAP1400核岛结构屏蔽厂房与辅助厂房示意图
Figure 1. A diagram of the shielding and auxiliary factory structure of nuclear power plant CAP1400
图 2 CAP1400核岛结构有限元模型
Figure 2. The finite element model of nuclear power plant CAP1400 structure
图 4 AP1000谱人工地震动加速度时程
Figure 4. Artificial acceleration time-history corresponding to AP1000 spectra
图 5 RG1.60谱人工地震动加速度时程
Figure 5. Artificial acceleration time-history corresponding to RG1.60 spectra
图 6 不同缩尺比模型计算得到的结构反应前30阶自振频率
Figure 6. The first 30 natural frequencies of structure response from different scale structure models
图 7 制控节点处结构反应峰值加速度放大系数
Figure 7. Peak acceleration amplification factor of structural response at control points
图 8 控制性节点处结构反应加速度反应谱（一）
Figure 8. Spectral accelerations of structural response at control points
图 8 控制性节点处结构反应加速度反应谱（二）
Figure 8. Spectral accelerations of structural response at control points
图 9 结构反应加速度反应谱的数值计算结果与试验结果的比较
Figure 9. Comparison of spectral accelerations between the numerical analysis and model testing
表 1 核岛结构缩尺模型设计相似关系
Table 1. Similitude relation of scaled models of nuclear power plant structure
物理量 量纲 相似系数（1/16模型） 相似系数（1/40模型） 尺寸L [L] 1/16 1/40 线位移δ [L] 1/16 1/40 角位移β 1 1 弹性模量E [FL-2] 0.340 0.233 密度ρ [FL-4T2] 1 1 泊松比μ 1 1 应变ε 1 1 应力σ [FL-2] 0.340 0.233 等效质量密度ρε [FL-4T2] 1.74 1.74 集中荷载F [F] 1.328×10-3 1.458×10-4 弯矩M [FL] 8.300×10-5 3.645×10-6 时间t [T] 1.414×10-1 6.827×10-2 自振频率ω [T-1] 7.072 14.647 阻尼比ξ 1 1 加速度幅值ɑ [LT-2] 3.126 5.363 加速度频率υ [T-1] 7.072 14.647 结构刚度k [FL-1] 2.125×10-2 5.832×10-3 结构自重m [FL-1T2] 4.248×10-4 2.719×10-5 
下载: 导出CSV
表 2 不同缩尺比模型计算得到的结构反应前4阶自振频率
Table 2. The first 4 natural frequencies of structure response from different scaled structure models
振型序号 1 2 3 4 1/1模型自振频率/Hz 3.99 4.35 5.82 6.14 1/16模型自振频率 计算值/Hz 26.34 28.70 38.38 40.60 反推值/Hz 3.72 4.05 5.42 5.74 与1/1模型的相对误差/% -6.77 -6.90 -6.70 -6.51 1/40模型自振频率 计算值/Hz 53.49 58.88 79.18 83.41 反推值/Hz 3.65 4.02 5.41 5.69 与1/1模型的相对误差/% -8.52 -7.59 -7.04 -7.33
下载: 导出CSV
表 3 控制性节点处结构反应的峰值加速度（单位：g）
Table 3. Peak accelerations of structural response at the control points
节点 输入地震动：AP1000时程 输入地震动：RG1.60时程 缩尺比 1/1 1/16 1/40 缩尺比 1/1 1/16 1/40 1 X向 0.489 0.474（-3.1） 0.351（-28.2） X向 0.539 0.526（-2.4） 0.359（-33.3） Y向 0.482 0.481（-2.1） 0.408（-15.3） Y向 0.500 0.470（-6.0） 0.388（-22.4） Z向 0.386 0.292（-24.4） 0.220（-43.0） Z向 0.376 0.306（-18.6） 0.258（-31.4） 2 X向 0.546 0.54（-1.1） 0.478（-12.5） X向 0.574 0.652（13.6） 0.449（-21.8） Y向 0.561 0.596（6.2） 0.554（-1.2） Y向 0.522 0.562（7.7） 0.540（34.5） Z向 0.378 0.298（-21.2） 0.248（-34.4） Z向 0.348 0.301（-13.5） 0.258（-25.8） 3 X向 0.756 0.569（-24.7） 0.652（-13.8） X向 0.770 0.817（6.1） 0.704（-8.6） Y向 0.817 0.708（-13.3） 0.717（-12.2） Y向 0.789 0.704（-10.8） 0.737（-6.6） Z向 0.396 0.328（-17.2） 0.267（-32.6） Z向 0.410 0.354（-13.7） 0.264（-35.6） 4 X向 0.511 0.380（-25.6） 0.378（-26.0） X向 0.420 0.504（20.0） 0.386（-8.1） Y向 0.520 0.486（-6.5） 0.444（-14.6） Y向 0.448 0.464（35.7） 0.435（-2.9） Z向 0.414 0.292（-29.5） 0.223（-46.1） Z向 0.348 0.283（-18.7） 0.257（-26.1） 5 X向 0.554 0.520（-6.1） 0.565（19.9） X向 0.659 0.642（-2.6） 0.595（-9.7） Y向 0.920 0.830（-9.8） 0.789（-14.2） Y向 0.897 0.850（-5.2） 0.811（-9.6） Z向 0.381 0.328（-13.9） 0.242（-36.5） Z向 0.402 0.346（-13.9） 0.289（-28.1）
下载: 导出CSV
-
蔡新江, 田石柱, 2011.振动台试验方法的研究进展.结构工程师, 27(S):42-46. http://www.cnki.com.cn/Article/CJFDTOTAL-JGGC2011S1010.htm 杜修力, 韩俊艳, 李立云, 2013.长输埋地管道振动台试验设计中相似关系的选取.防灾减灾工程学报, 33(3):246-252. http://www.cnki.com.cn/Article/CJFDTOTAL-DZXK201303003.htm 侯春林, 李小军, 潘蓉等, 2016.地震动持时对核岛结构设计地基场地非线性地震响应影响分析.工业建筑, 46(10):9-12, 21. http://www.cnki.com.cn/Article/CJFDTOTAL-GYJZ201610003.htm 黄思凝, 郭迅, 孟庆利等, 2013.地震模拟试验中单质点体系小比例尺模型的分级相似关系.应用基础与工程科学学报, 21(1):71-77. http://www.cnki.com.cn/Article/CJFDTOTAL-YJGX201301009.htm 姜忻良, 徐炳伟, 李竹, 2010.土-桩-结构振动台模型试验相似理论及其实施.振动工程学报, 23(2):225-229. http://www.cnki.com.cn/Article/CJFDTOTAL-ZDGC201002016.htm 李勇, 闫维明, 刘晶波等, 2013.桥梁结构缩尺模型模拟地震振动台试验研究进展.工程抗震与加固改造, 35(5):1-10. http://www.cnki.com.cn/Article/CJFDTOTAL-GCKZ201305001.htm 李振宝, 李晓亮, 唐贞云等, 2010.土-结构动力相互作用的振动台试验研究综述.震灾防御技术, 5(4):439-450. doi: 10.11899/zzfy20100406 林皋, 2011.核电工程结构抗震设计研究综述(Ⅰ).人民长江, 42(19):1-6. doi: 10.3969/j.issn.1001-4179.2011.19.001 柳春光, 孙国帅, 韩亮等, 2012.桥梁水下桩墩结构振动台模型试验相似律验证.地震工程与工程振动, 32(4):13-18. http://www.cnki.com.cn/Article/CJFDTOTAL-DGGC201204003.htm 刘红彪, 李宏男, 2016.地震模拟振动台试验模型相似设计中的材料弹性模量取值问题研究.振动与冲击, 35(3):114-120. http://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201603019.htm 吕西林, 沈德建, 2008.不同比例钢-混凝土混合结构高层建筑动力相似关系试验研究.地震工程与工程振动, 28(4):50-57. http://www.cnki.com.cn/Article/CJFDTOTAL-DGGC200804008.htm 孟庆利, 黄思凝, 郭迅, 2008.钢筋混凝土结构小比例尺模型的相似性研究.世界地震工程, 24(4):1-6. http://cdmd.cnki.com.cn/Article/CDMD-85406-2009057338.htm 钱德玲, 李元鹏, 刘杰, 2013.高层建筑结构振动台模型试验与原型对比的研究.振动工程学报, 26(3):436-442. http://www.cnki.com.cn/Article/CJFDTOTAL-ZDGC201303019.htm 权登州, 王毅红, 井彦林等, 2015.黄土地区地铁车站数值模型及测试位置研究.震灾防御技术, 10(1):108-115. doi: 10.11899/zzfy20150111 宋二祥, 武思宇, 王宗纲, 2008.地基-结构系统振动台模型试验中相似比的实现问题探讨.土木工程学报, 41(10):87-92. doi: 10.3321/j.issn:1000-131X.2008.10.013 杨树标, 李荣华, 刘建平等, 2007.振动台试验模型和原型相似关系的理论研究.河北工程大学学报(自然科学板), 24(1):8-11. http://www.cnki.com.cn/Article/CJFDTOTAL-HJXU200701002.htm 杨树标, 杜广辉, 李荣华等, 2008.结构动力模型相似关系研究及验证.河北工程大学学报(自然科学板), 25(3):4-7. http://www.cnki.com.cn/Article/CJFDTOTAL-HJXU200803001.htm 张佳, 王毅红, 权登州等, 2016.黄土地区地铁地下车站地震变形特性研究.震灾防御技术, 11 (2):272-282. doi: 10.11899/zzfy20160210 张敏政, 1997.地震模拟实验中相似律应用的若干问题.地震工程与工程振动, 17(2):52-58. http://www.cnki.com.cn/Article/CJFDTOTAL-DGGC702.006.htm 赵作周, 管桦, 钱稼茹, 2010.欠人工质量缩尺振动台试验结构模型设计方法.建筑结构学报, 31 (7):78-85. http://www.cnki.com.cn/Article/CJFDTOTAL-JZJB201007013.htm 郑升宝, 蒋树屏, 王晓雯等, 2010.隧道振动台模型试验的相似关系.重庆交通大学学报(自然科学板), 29(6):875-879. http://www.cnki.com.cn/Article/CJFDTOTAL-CQJT201006011.htm 周颖, 吕西林, 卢文胜, 2006.不同结构的振动台试验模型等效设计方法.结构工程师, 22(4):37-40. http://www.cnki.com.cn/Article/CJFDTOTAL-JGGC200604008.htm Dai Z. J., Li X. J., Hou C. L., 2014. An optimization method for the generation of ground motions compatible with multi-damping design spectra. Soil Dynamics and Earthquake Engineering, 66:199-205. doi: 10.1016/j.soildyn.2014.06.032 Paolucci R., Shirato M., Yilmaz M. T., 2008. Seismic behaviour of shallow foundations:shaking table experiments vs numerical modelling. Earthquake Engineering & Structural Dynamics, 37(4):577-595. doi: 10.1002/eqe.773 Pitilakis D., Dietz M., Wood D. M., et al., 2008. Numerical simulation of dynamic soil-structure interaction in shaking table testing. Soil Dynamics and Earthquake Engineering, 28(6):453-467. doi: 10.1016/j.soildyn.2007.07.011 Severn R. T., 2011. The development of shaking tables-A historical note. Earthquake Engineering & Structural Dynamics, 40(2):195-213. doi: 10.1002/eqe.1015/epdf Shi T. H., Cheng S. G., 2013. Dynamic similitude law design of shaking table model test for high-rise steel structures. In:5th International Conference on Advances in Experimental Structural Engineering. Taipei, Taiwan:NCREE. Žarnić R., Gostič S., Crewe A. J., et al., 2001. Shaking table tests of 1:4 reduced-scale models of masonry infilled reinforced concrete frame buildings. Earthquake Engineering & Structural Dynamics, 30(6):819-834. -
下载:
点击查看大图
计量
- 文章访问数: 140
- HTML全文浏览量: 25
- PDF下载量: 29
- 被引次数: 0
